Next Article in Journal
Computational Fluid Dynamics Investigation of Flow and Flame Behavior in Natural Gas Burners for Borax Pentahydrate Furnaces
Previous Article in Journal
Scheduling Strategy of Virtual Power Plant Alliance Based on Dynamic Electricity and Carbon Pricing Using Master–Slave Game
Previous Article in Special Issue
Design and Adaptability Analysis of Integrated Pressurization–Gas Lifting Multifunctional Compressor for Enhanced Shale Gas Production Flexibility
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Processes
Volume 13
Issue 6
10.3390/pr13061659
processes-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

Impact of Gravity Segregation on Gas Injection Development in Condensate Gas Reservoirs: A Numerical Simulation Study

by
Fangfang Chen
1,2,3,
Mengqin Li
1,2,3,*,
Yang Yang
1,2,3,
Qizhu Zhang
4,
Ning Lin
1,2,3 and
Keliu Wu
4
1
Tarim Oilfield Company, PetroChina, Korla 841000, China
2
R&D Center for Ultra-Deep Complex Reservoir Exploration and Development, CNPC—China National Petroleum Corporation, Korla 841000, China
3
Engineering Research Center for Ultra-Deep Complex Reservoir Exploration and Development, CNPC—China National Petroleum Corporation, Korla 841000, China
4
State Key Laboratory of Petroleum Resources and Prospecting, China University of Petroleum (Beijing), Beijing 102249, China
*
Author to whom correspondence should be addressed.
Processes 2025, 13(6), 1659; https://doi.org/10.3390/pr13061659
Submission received: 18 March 2025 / Revised: 8 May 2025 / Accepted: 19 May 2025 / Published: 26 May 2025
(This article belongs to the Special Issue 2nd Edition of Artificial Intelligent Techniques in the Optimal Operation of Oil and Gas Production Systems)
Browse Figures
Versions Notes

Abstract

:
Gravity segregation is a critical phenomenon in thick condensate gas reservoirs, significantly influencing fluid composition and phase behavior. Reservoir-scale numerical simulation, serving as an indispensable technical approach in modern petroleum engineering, provides both quantitative data support and theoretical frameworks for development strategy optimization. However, the impact of gravity segregation on the distribution of initial fluid compositions is often overlooked in conventional numerical simulations due to data limitations or underestimated importance. This oversight leads to systematic deviations between simulated reservoir performance and actual field observations, ultimately compromising the efficient development of reservoirs. This study analyzed PVT data from reservoir fluid samples at different depths to determine the initial fluid composition distribution. Two models were developed: one incorporating gravity segregation and another neglecting it, to evaluate their performance during gas injection. Key findings include: (i) Gravity segregation alters the initial fluid composition, creating lighter components near the reservoir top and heavier ones at the bottom, resulting in distinct phase behaviors and production dynamics. (ii) The model accounting for gravity segregation aligns better with historical production data, while the model neglecting it underestimates oil production rates by about 9% and overestimates oil recovery by 2–5% during gas injection, due to inaccurate fluid composition assumptions. (iii) The model without gravity segregation also underestimates differences in oil recovery between injection–production strategies, such as top versus bottom injection. This study highlights the critical role of gravity segregation in reservoir simulation and provides valuable insights for optimizing the development of condensate gas reservoirs with complex fluid distributions. The findings reveal that accounting for gravity segregation in reservoir simulation models through proper initialization of fluid distribution leads to improved simulation accuracy, thereby enabling more precise development strategy design.

1. Introduction

At present, China’s natural gas consumption has exceeded 3.917 × 1011 m3, of which 1.656 × 1011 m3 were imported [1]. As a result, there is a strong demand for China to improve domestic natural gas production. Condensate gas, as a unique reservoir type that exists between conventional oil and gas reservoirs, has exceptionally high economic value and is relatively abundant in China. Therefore, condensate gas reservoirs are an indispensable part of China’s natural gas resources. These resources in China are primarily distributed in the Tarim Basin and Sichuan Basin, with the Tarim Basin accounting for approximately 80% of the total condensate gas reserves in the country, making it a key area for development [2,3]. However, the burial depth of most condensate gas reservoirs in the Tarim Basin exceeds 4000 m, resulting in complex phase behavior during reservoir development [4,5,6,7,8]. For some condensate gas reservoirs with relatively large reservoir thickness, the effect of gravity segregation becomes more pronounced, further complicating the fluid phase behavior. Therefore, to develop the condensate gas reservoir effectively, it is of great significance to research the phase behavior of deep condensate gas reservoirs and the influence of gravity segregation on phase behavior.
Once two immiscible fluids are simultaneously injected into a porous medium, three distinct regions of constant saturation initially form. Subsequently, under the influence of gravity, the three regions will evolve into two horizontal layers, each fully saturated with a single phase [9,10,11]. This process is referred to as gravity segregation. To optimize the utilization of this phenomenon, strategies such as top gas injection and bottom water injection are commonly applied during reservoir development, utilizing gravity as the driving force to displace hydrocarbons and stabilize the displacement front.
However, gravity segregation is not only evident in different fluids but also impacts compositional gradients within the same fluid. In many reservoirs, a consistent variation in hydrocarbon composition with depth occurs due to the influence of gravity [12,13,14]. To illuminate the relationship between consistent variation and depth, Liu et al. (2001) [15] analyzed four condensate gas samples from wells at varying depths, and the results showed that both the condensate content and saturation (dew point) pressure increased with depth, which was attributed to the increase in restructured hydrocarbons. In conventional oil reservoirs, a similar trend of consistent variation is observed, typically manifesting as an increase in density and a decrease in solution gas–oil ratio and saturation pressure of oil with increasing depth [16,17]. However, the influence of gravity segregation on the development of condensate gas reservoirs is more significant, primarily due to the complex phase behavior involved. Therefore, numerous researchers have attempted to apply various equations of state to quantitatively characterize the distribution of initial fluid composition in gas condensate reservoirs [18,19,20,21]. Other researchers have focused on the influence of gravity segregation in reservoir scale and have proposed some methods to mitigate the adverse effects of gravity segregation during the development of condensate gas reservoirs [22,23]. Therefore, the effect of gravity segregation significantly influences the distribution of initial fluids and the development process.
In recent years, significant progress has been made in simulating fluid flow in fractured and heterogeneous porous media, enhancing the understanding of subsurface multiphase flow processes. Traditional multi-continuum models [24,25] are effective when there is clear scale separation between the matrix and fractures, but they become inadequate in complex geological settings with overlapping fracture scales [26]. To improve accuracy, explicit fracture modeling approaches have been proposed, such as the Discrete Fracture Model (DFM) [27,28] and the Embedded Discrete Fracture Model (EDFM) [29,30], which couple matrix and fracture flow through conformal grids or transmissibility functions, respectively. Meanwhile, numerical methods like the Extended Finite Element Method (XFEM) and Embedded Finite Element Method (E-FEM) introduce enriched degrees of freedom to capture discontinuities caused by fractures [31,32] and have been extended to handle single-phase and multiphase flow [33,34]. These developments have expanded the robustness and applicability of simulation tools, providing strong theoretical and computational support for investigating flow in complex fractured and heterogeneous reservoirs.
Commercial simulators such as tNavigator have integrated many of these concepts, allowing for fully implicit compositional modeling with robust nonlinear and linear solvers (e.g., MILU(0) preconditioning) and adaptive time-stepping strategies. These capabilities make it feasible to assess gravitational effects, phase redistribution, and compositional gradient development in thick, stratified reservoirs.
Many studies have researched the vertical consistent variation in condensate gas reservoirs and tried to quantitatively characterize the distribution of initial fluid composition. Few studies have systematically investigated the impacts of compositional variations induced by gravity segregation on production performance in gas condensate reservoirs, particularly concerning gas injection development. Particularly in the condensate gas reservoir, the distribution of initial fluid composition has a significant impact on the efficiency of gas injection development. Therefore, it is essential to study the consistent variation and quantitatively characterize its influence on the efficiency of gas injection in condensate gas reservoirs.
In this work, we first analyzed PVT data of reservoir fluid samples from different depths within a condensate gas reservoir in the Tarim Basin. Based on this analysis, the distribution of the initial fluid composition influenced by gravity segregation was preliminarily established. Subsequently, the geologic model of this reservoir was applied to model the development process of gas injection, both with and without the initial fluid composition affected by gravity segregation. Finally, a comparative analysis of the simulation results was conducted to evaluate the impact of gravity segregation on the effectiveness of gas injection in condensate gas reservoirs.

2. Distribution of Initial Fluid Composition Influenced by Gravity Segregation

2.1. Phase Behavior Characteristics in Different Depths

In this paper, a fault-controlled fracture–vug condensate gas reservoir situated in the central part of the Aman transition zone within the northern depression of the Tarim Basin is selected for research. The effective reservoir space types are primarily composed of caves, vugs, and fractures. Furthermore, the reservoir exhibits a remarkable thickness exceeding 700 m and a burial depth greater than 7000 m. Due to the combination of high vertical permeability, considerable thickness, and significant depth, the reservoir demonstrates complex phase behavior and pronounced gravity segregation. To investigate the phase characteristics of this reservoir, two fluid samples from different depths were collected, and comprehensive laboratory experiments were conducted on these samples.
To obtain fluid samples from different reservoir depths, gas and oil samples were collected at the separators from two production wells located at depths of 7508 m and 7990 m. Recombination was performed based on the corresponding production gas–oil ratio (GOR) data to reconstruct representative reservoir fluids. Subsequent PVT experiments were conducted to determine the compositional characteristics and other relevant fluid properties. Significant differences in fluid components and properties were observed between the two fluid samples. Table 1 presents the components of Sample 1 (7508 m) and Sample 2 (7990 m). It is observed that the wellstream component of Sample 1 is lighter, containing 86.736% C1 (methane) and 4.369% C7+ (heptane and all heavier hydrocarbon components). In contrast, Sample 2 has a C1 content of 75.656% and a C7+ content of 8.342%. Additionally, the single-stage de-oiling of Sample 1 is also lighter. These findings indicate that heavier hydrocarbons accumulate with increasing depth, further confirming the presence of the gravity segregation effect. In this study, C1, C2, and C3, etc., represent hydrocarbon components with 1, 2, 3, and more carbon atoms, corresponding to methane, ethane, propane, and so on, respectively.
Among all the experiments, the constant volume depletion (CVD) experiment is particularly important, as it can accurately represent the actual depletion process of a gas condensate reservoir in some cases [15]. Consequently, analyzing the results of the CVD experiment is of critical importance. Figure 1 illustrates the correlation between the liquid volume percentage and pressure for two fluid samples. The results reveal that, due to the lighter composition, the condensate oil of Sample 1 gradually separates as pressure decreases. In contrast, the liquid volume of Sample 2 increases sharply as pressure drops below the dew point. Based on the previously observed compositional data, it is assumed that a significant amount of condensate oil containing heavy components separates upon reaching the dew point pressure, significantly altering the properties of the condensate gas. Subsequently, as pressure continues to decrease, the condensate oil separates progressively. This initially leads to a pronounced variation in liquid volume, which diminishes at lower pressures.
Based on the analysis above, it is evident that the components and phase behavior of the two samples differ significantly, confirming the pronounced gravity segregation present in this reservoir. Therefore, incorporating gravity segregation into the simulation of reservoir development is essential, as neglecting it may lead to results that deviate substantially from actual reservoir behavior.

2.2. Confirmation of Initial Fluid Composition Distribution

To analyze the distribution of the initial fluid composition, two simulation components were created in the numerical simulation software tNavigator 22.1, based on the aforementioned compositional information (Table 1). The compositional information was first imported into the numerical simulation software and then combined into seven pseudo-components to enhance computational efficiency. Based on the original eight-component splitting scheme for similar condensate gas reservoirs [35], this study proposes an optimized seven-component scheme (CO2, N2, CH4, C2–C4, C5–C6, C7–C16, C17+) through a comprehensive analysis of fluid compositional characteristics and development requirements. Notably, CO2 was separated from the originally lumped C₁₊ group despite its low concentration, considering its critical role as an injection medium for subsequent gas flooding simulations. Furthermore, the merging of C2–C4 and C5–C6 fractions was implemented based on both the continuity of their physical properties and development sensitivities, achieving an optimal balance between computational accuracy and efficiency. This refined scheme demonstrates particular advantages in gas injection development optimization and heavy component precipitation prediction.
The EOS parameters, particularly the critical properties of heavier components, were subsequently modified to align with the experimental results from Constant Composition Expansion (CCE) and Constant Volume Depletion (CVD) tests. Upon completion of the regression process, compositional models were successfully established for both Sample 1 and Sample 2.
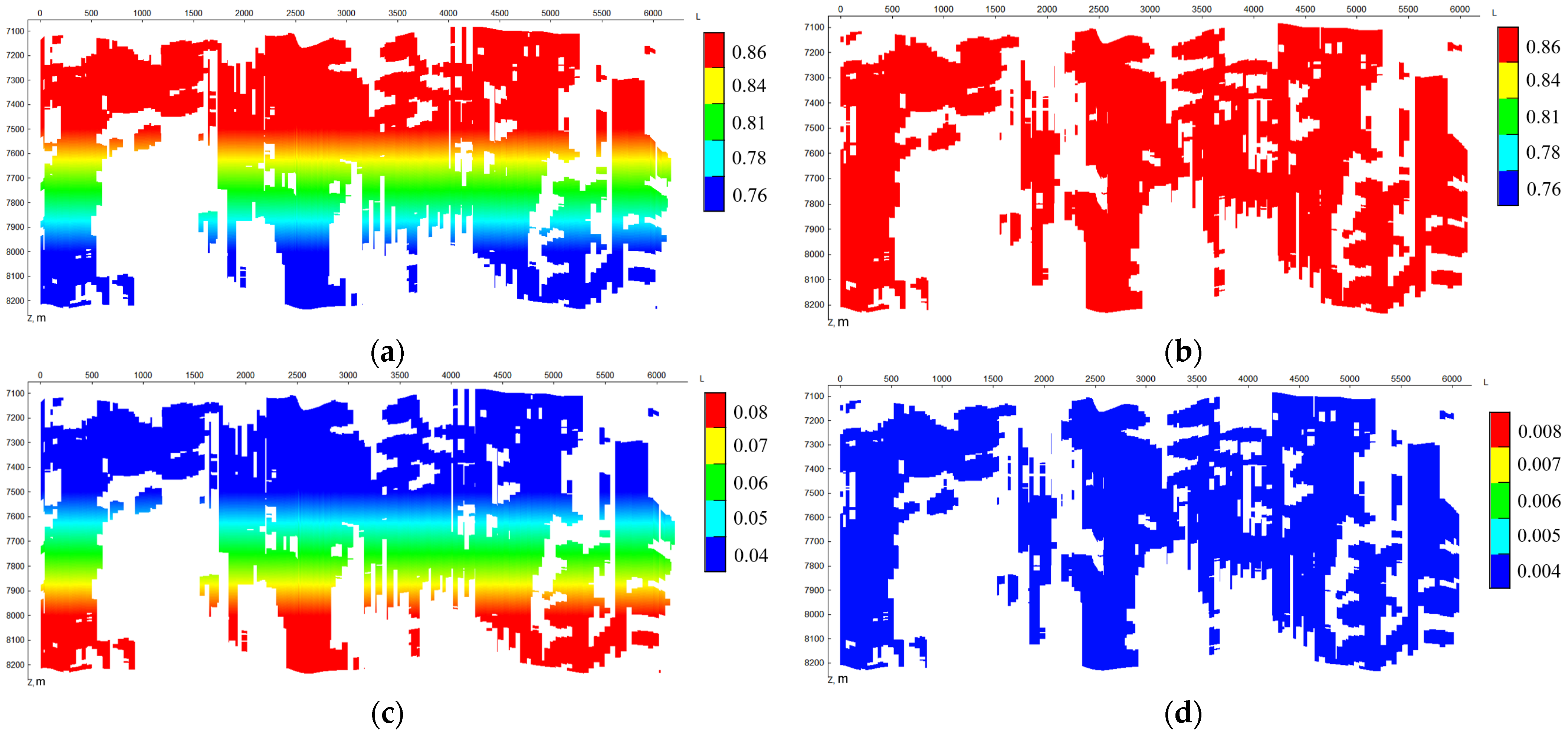
A compositional model was then established by integrating the created components with the geological model through equilibrium initialization. To account for the influence of gravity segregation on the initial fluid composition distribution, a function called ZMFVD was applied. This function employs the constant extrapolation method to calculate fluid composition within the reservoir based on compositional data from different depths (Sample 1 at 7508 m and Sample 2 at 7990 m). For comparison, another compositional model without this function was constructed, utilizing data from Sample 1, which is located near the middle depth of the reservoir. The latter approach is more commonly used in conventional reservoir numerical simulations. The distribution of the initial fluid composition in the two models is demonstrated in Figure 2.
It is observed that the proportion of light components gradually decreases with increasing depth, while the proportion of heavy components increases correspondingly in the model accounting for gravity segregation (Figure 2a,c). In summary, the initial fluid composition exhibits the characteristic distribution of “Light at the top, heavy at the bottom”, consistent with the distribution influenced by gravity segregation in theory. In contrast, the component distribution in the alternative model is uniform (Figure 2b,d), deviating from actual conditions. This comparison underscores the importance of incorporating gravity segregation into numerical simulations. Nevertheless, qualitative analysis alone is insufficient, quantitative comparisons are equally essential and will be conducted in subsequent sections.

2.3. History Matching of Numerical Model

In the previous section, we compared the distribution of initial fluid composition in two models (one accounting for gravity segregation and the other not) and confirmed that the model incorporating gravity segregation better aligns with actual reservoir conditions. However, to further analyze the difference between the two models during production, a real well group consisting of five wells was incorporated into the previously established models for subsequent simulations, and the well locations are illustrated in Figure 3.
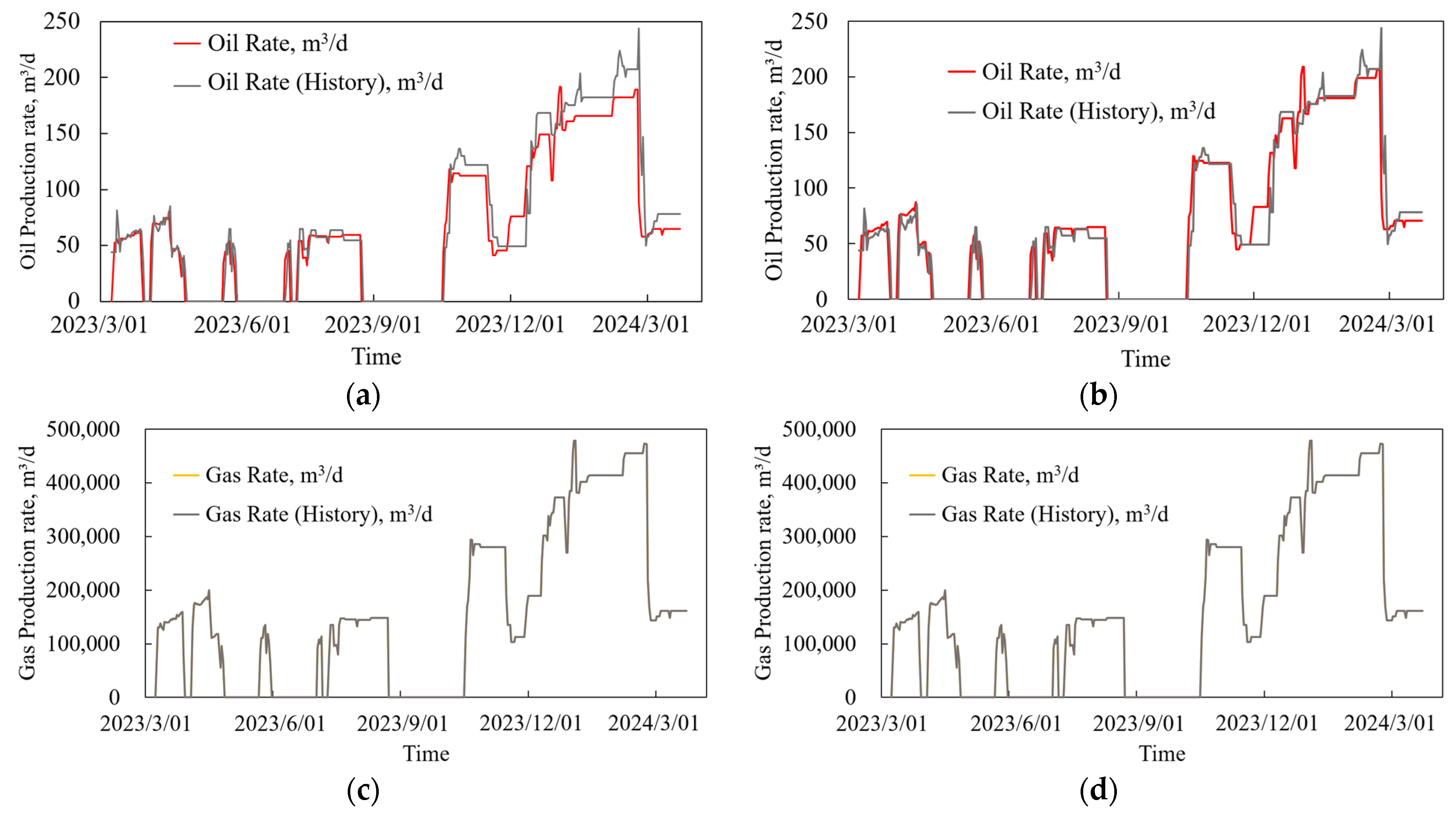
Well C and Well D commenced production in 2023, with production allocation optimized following a short-term production testing period from March to September 2023 due to the reservoir’s excellent petrophysical properties and sufficient formation energy. To evaluate the impact of initial fluid composition distribution on production performance and improve the reliability of models, history matching was carried out for both models, and the results are demonstrated in Figure 4.
The simulated gas production rates of the two models closely align with the historical data, as shown in Figure 4c,d. That is because the gas production rate was defined as a limiting parameter in numerical simulation software, and the excellent petrophysical properties of the reservoir allowed the wells to meet their production allocations.
In Figure 4a,b, the simulated oil production rates of two models during the short-term production testing phase also match the historical data. However, in the subsequent production period, the oil production rate of the model without accounting for gravity segregation is obviously lower than the historical data, whereas the model that includes gravity segregation maintains a strong alignment with the historical data.
During the historical production period, the formation pressure remained above the dew point pressure, preventing the separation of condensate oil. Under this condition, if the gas production rate is fixed, the oil production rate depends entirely on fluid properties. In the short-term production testing stage, production primarily mobilized mid-shallow reservoir fluids, whose properties are similar to those of Sample 1. Therefore, both models’ simulated oil production rates match the historical data during this phase. As production continued, the high production allocation gradually mobilized deeper reservoir fluids, causing the oil production rate to increase. In this stage, the model without gravity segregation failed to capture the complex production characteristics of the reservoir, resulting in a significant underestimation of oil production rates. In contrast, the model that accounted for gravity segregation closely aligns with the historical data, accurately reflecting the impact of deeper fluid mobilization.
To quantitatively evaluate the historical matching performance of oil rates between the two models, the root mean square error (RMSE) was calculated for 158 data points at different time steps. The results demonstrate that the model incorporating gravity segregation achieves a superior RMSE of 6.82, compared to 9.15 for the model neglecting gravity segregation effects, confirming better historical matching accuracy when accounting for gravitational compositional gradients.
In conclusion, qualitative and quantitative analyses demonstrate that the model incorporating gravity segregation is more consistent with the actual reservoir conditions.

3. Gravity Segregation on the Effectiveness of Gas Injection

Although we have concluded that the model incorporating gravity segregation aligns more closely with the actual reservoir conditions, most existing simulations failed to account for gravity segregation due to insufficient data or a lack of emphasis on its significance. This limitation becomes even more critical when considering gas injection, a typical and effective strategy for condensate gas reservoir production. Gas injection alters the phase behavior of reservoir fluids and amplifies the effects of gravity segregation. Therefore, investigating the impact of gravity segregation on gas injection recovery in numerical simulations of condensate gas reservoirs is of great importance.
Based on the compositional model built before, the tNavigator software is employed to simulate and compare the impact of gravity segregation on gas injection recovery. In the compositional model of tNavigator, the finite volume method (FVM) is employed for spatial discretization to ensure local mass conservation at the control volume level. Time discretization is conducted using a fully implicit method (FIM), where all primary unknowns, including pressure and component molar densities, are solved simultaneously at each time step. The resulting nonlinear system is addressed through the Newton–Raphson method, with convergence criteria based on both the norm of the nonlinear residual and the changes in the primary variables. During each Newton iteration, the corresponding linearized system is solved using an iterative solver with preconditioning based on modified incomplete LU factorization (MILU(0)). The convergence of the linear solver is monitored through the normalized residual of the linear system. Moreover, an adaptive implicit method (AIM) is adopted to dynamically adjust the time step size based on changes in key variables such as pressure and saturation, thereby enhancing both simulation stability and computational efficiency. The numerical simulation results for both models during gas injection production are demonstrated as follows.

3.1. Model Without Accounting for Gravity Segregation

To optimize resource utilization in the reservoir, the model incorporates wells at varying depths, with three classified as shallow wells and two as deep wells. These depth variations allow for the application of different injection–production strategies, such as top injection and bottom production (well B, C, and D as injection wells and well A and E as production wells) or bottom injection and top production (well A, E as injection wells and well B, C, D as production wells). The choice of injection medium—commonly natural gas or nitrogen—also plays a critical role in influencing reservoir development, as it affects phase behavior and displacement efficiency. To evaluate the impact of these strategies and identify the optimal development plan, the production dynamics over a 20-year period were simulated using numerical software. The results are presented as follows.
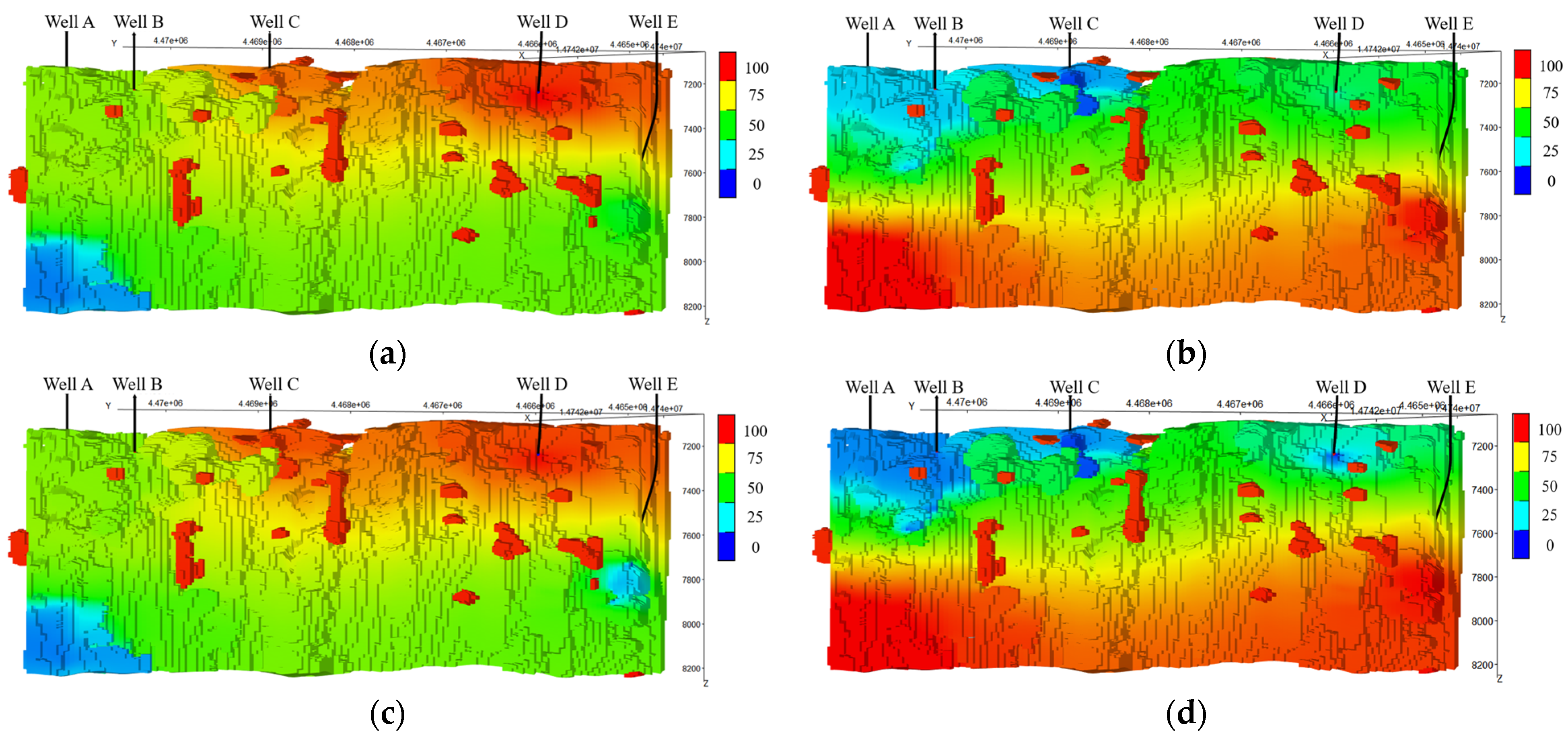
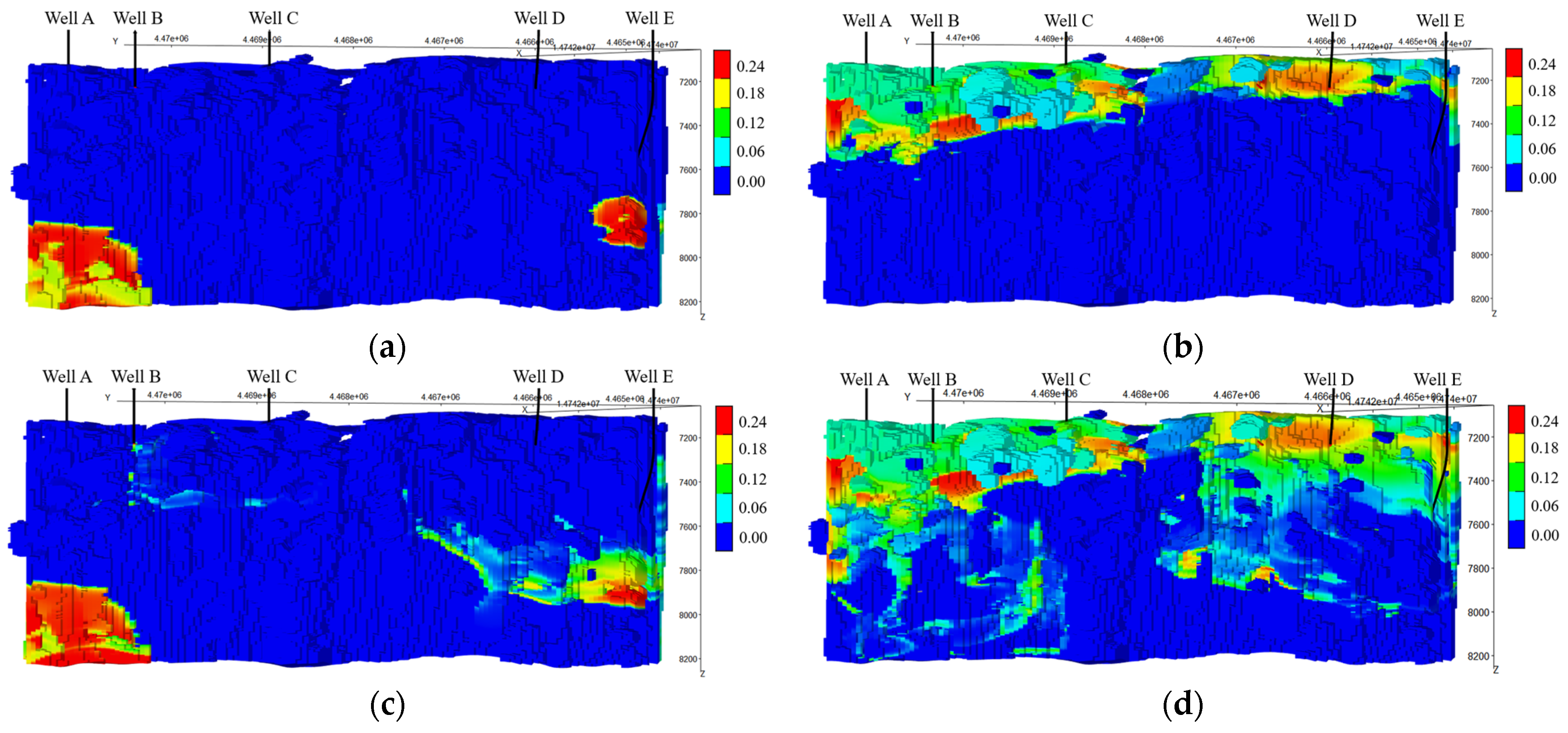
Figure 5a,b show the pressure distribution at the end of the simulation for natural gas injection from the top and bottom, respectively, while Figure 6a,b illustrate the condensate oil distribution under the same scenarios. It is evident that the top injection provides better pressure maintenance, with only a small low-pressure zone present at the bottom of the reservoir. In contrast, bottom injection results in a significant low-pressure area at the top of the reservoir, leading to increased condensate oil separation and retention. Consequently, this results in lower oil recovery, as shown in Figure 9. The poorer performance of bottom injection can primarily be attributed to two factors. First, the inferior petrophysical properties of the lower reservoir layers, where fracture–cavity development is less pronounced (Figure 3), limit the injection capacity of wells A and E. Second, the higher initial reservoir pressure in the lower layers further constrains the injection efficiency. These combined factors reduce gas injection volumes (Figure 10) and lead to lower oil recovery rates than top injection.
When nitrogen is applied as an injection medium, the pressure near the injection point is noticeably higher than with the natural gas injection. However, the pressure in the area farthest from the injection point is significantly lower, resulting in greater condensate oil separation and reduced oil recovery (Figure 5c,d and Figure 6c,d). This phenomenon can be attributed to nitrogen’s lower compressibility and diffusivity, which arise from its larger molecular weight. These properties make nitrogen effectively supplement formation energy near the injection point; its ability to propagate pressure to distant areas is significantly limited. Additionally, the elevated pressure near the injection point further constrains the injection capacity of the well, leading to reduced gas injection volumes (Figure 10). As a non-hydrocarbon gas, nitrogen does not improve fluid phase behavior like natural gas, failing to prevent condensate oil separation. As a result, significant amounts of condensate oil remain in the reservoir, ultimately lowering oil recovery.

3.2. Model Accounting for Gravity Segregation

In this model, the development strategies and production parameters are identical to the model without accounting for gravity segregation. However, the simulation results differ significantly due to the varying distribution of initial fluid components. The pressure distribution for top injection is consistent with the previous model, whereas in the bottom injection scenario, the pressure in regions farthest from the injection point is notably lower (Figure 7). This disparity can be attributed to the accumulation of heavy components in the lower part of the reservoir, which limits gas injection volumes and reduces the diffusivity of the injected gas. Consequently, the amount of separated condensate oil is greater in this model than in the previous one (Figure 8), as heavier fluid components exacerbate condensate oil trapping in the reservoir. These effects collectively lead to a lower overall oil recovery than the model without accounting for gravity segregation. Moreover, the differences in oil recovery between injection–production strategies (e.g., top vs. bottom injection) are more pronounced in this model, reflecting the substantial variations in fluid composition between the upper and lower reservoir regions.
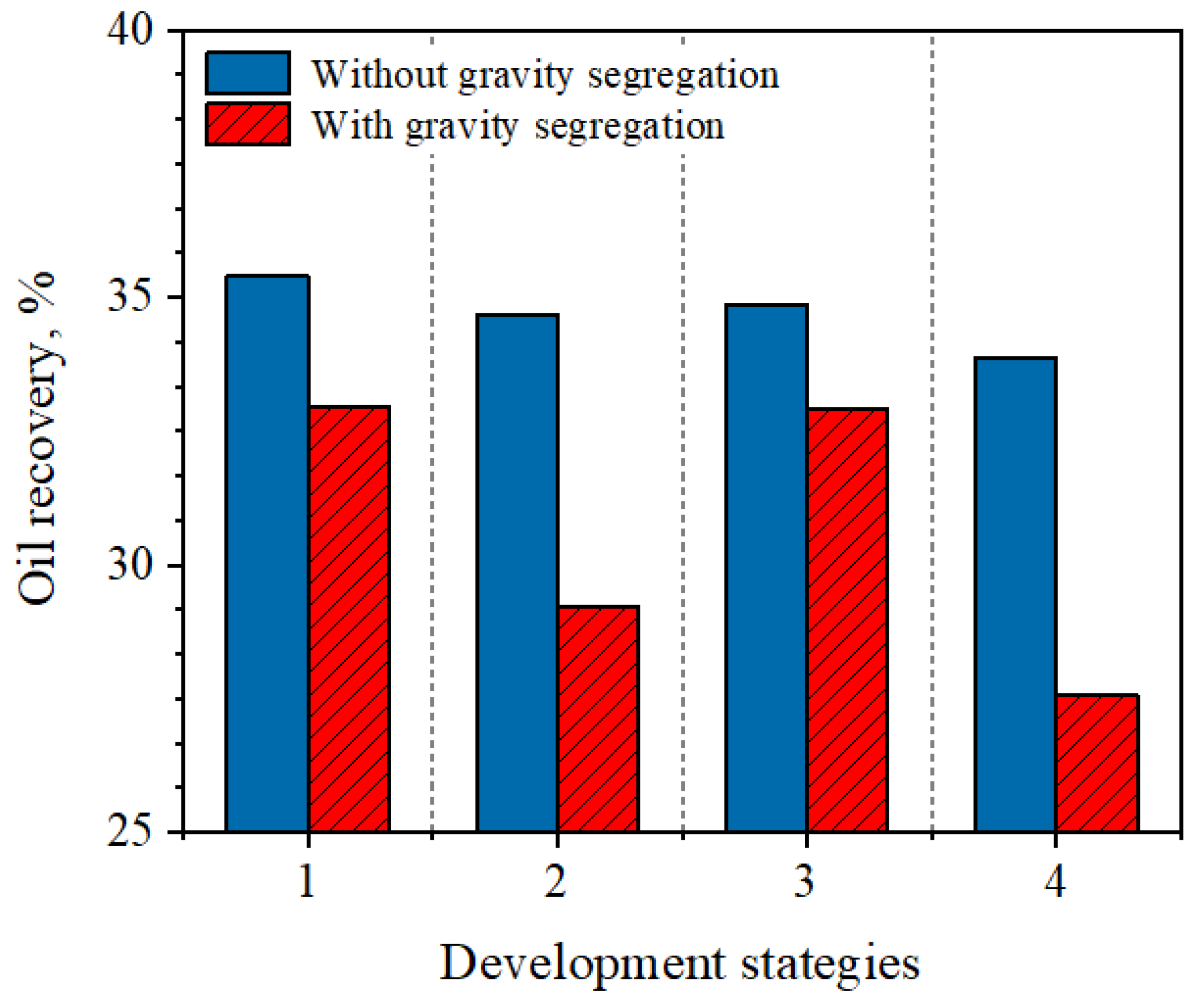
Comparative analysis of ultimate oil recovery under different injection–production scenarios (Figure 9) reveals that: 1. the top gas injection strategy yields higher recovery than bottom injection; 2. models neglecting gravity segregation overestimate ultimate recovery while underestimating inter-strategy differentials and; 2. although top natural gas injection achieves peak recovery in both models, its marginal advantage over top nitrogen injection is merely 0.56% (without gravity segregation model) and 0.06% (with gravity segregation-aware model). Furthermore, Figure 10 also illustrates the differences in gas injection volumes under various injection–production scenarios. The results indicate that in the model accounting for gravity segregation, the altered fluid distribution leads to increased difficulty in gas injection, resulting in lower gas injection volumes compared to the model neglecting gravity segregation.
Given the negligible performance difference (<0.6%) contrasted with significant economic benefits, top nitrogen injection is recommended as the optimal development approach.

4. Conclusions and Discussion

This study systematically investigates the impact of gravity segregation on fluid distribution and production performance in thick condensate gas reservoirs. By integrating depth-dependent compositional analysis with numerical simulations, the research demonstrates that gravity segregation significantly influences phase behavior and production forecasts. The results clearly show that accounting for gravity segregation leads to more accurate modeling outcomes and provides valuable guidance for optimizing field development.
  • Gravity segregation significantly influences fluid distribution in thick reservoirs, with lighter components accumulating near the top and heavier ones at the bottom, leading to varying phase behavior with depth.
  • Neglecting gravity segregation in simulations leads to underestimation of oil production due to inaccurate fluid composition assumptions, while models incorporating it better match historical data.
  • Models without gravity segregation overestimate recovery during gas injection and fail to capture performance differences across injection–production strategies caused by fluid composition variation.
  • Accounting for gravity segregation enhances the accuracy of reservoir simulations and supports better optimization of development strategies in condensate gas reservoirs.
This study highlights the critical influence of gravity segregation on reservoir fluid distribution and development strategies. The validated model demonstrates that accounting for gravity segregation significantly improves simulation accuracy, enabling optimized gas injection placement (e.g., top injection) for enhanced recovery. These findings provide practical guidance for field operations while underscoring the need for further research into dynamic compositional changes and advanced simulation methods to better capture the gravity segregation effect under various reservoir conditions. The improved predictive capability of such models can directly support more economical and sustainable field development decisions.
The gravity segregation effects discussed in this study, and their impact on gas injection development simulation, are primarily applicable to ultra-thick fractured-vuggy condensate gas reservoirs characterized by strong gravitational forces and complex fluid phase behavior. While the findings also offer some guidance for gas injection development in condensate gas reservoirs with moderate reservoir thickness, their applicability is limited in cases where the reservoir is relatively thin (resulting in weak gravity segregation) or when the reservoir fluid is not of the condensate gas type (exhibiting minimal phase behavior complexity).
Although this study emphasizes the critical role of gravity segregation in gas injection development, the initial fluid distribution in the gravity segregation model is constrained by limited data availability, primarily due to the challenges associated with reservoir fluid sampling. Moreover, the applicability of this study to other types of reservoirs remains to be further investigated. Further research is necessary to refine and extend the current findings. Future work should focus on incorporating more comprehensive fluid sampling at multiple depths to better characterize compositional variations induced by gravity segregation, as well as on evaluating the applicability of the current findings to other types of reservoirs. Additionally, laboratory-scale experiments, such as high-pressure core flooding tests under controlled gravity conditions, are also recommended to validate and calibrate simulation results. These improvements would contribute to a more robust understanding of gravity segregation mechanisms and their implications for optimizing condensate gas reservoir development.

Author Contributions

Conceptualization, F.C. and M.L.; methodology, Y.Y.; software, Q.Z.; validation, N.L. and Q.Z.; formal analysis, F.C. and K.W.; investigation, M.L.; resources, K.W.; writing—original draft preparation, Q.Z.; writing—review and editing, K.W. All authors have read and agreed to the published version of the manuscript.

Funding

This research was funded by Research on Efficiency Enhancement and Enhanced Recovery Technology for Complex Carbonate Gas Reservoirs and Research on the detailed description and profit-stable production technology of carbonate rock reservoir in Fuman Oilfield, grant number 2023ZZ16YJ03 and T202408. The APC was funded by Research on Efficiency Enhancement and Enhanced Recovery Technology for Complex Carbonate Gas Reservoirs.

Data Availability Statement

The original data used in this study are included in this article. Further inquiries can be directed to the corresponding author.

Conflicts of Interest

Authors Fangfang Chen, Mengqin Li, Yang Yang and Ning Lin were employed by the Tarim Oilfield Company, PetroChina and China National Petroleum Corporation. The remaining authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

References

  1. Duan, Z.; Zhang, X.; Wu, M.; Cui, Z.; Duan, T. China’s natural gas markets review in 2023 and outlook for 2024. Int. Pet. Econ. 2024, 32, 19–28. (In Chinese) [Google Scholar]
  2. Haotian, S. Research on Rational Development Mode of Abnormally High Pressure Fractured Condensate Gas Reservoir. Master’s Thesis, China University of Petroleum (Beijing), Beijing, China, 2022. (In Chinese). [Google Scholar]
  3. Xu, Z.; Yue, D.; Wu, S.; Zhang, X.; Chen, C.; Ni, Y. An analysis of the types and distribution characteristics of natural gas reservoirs in China. Pet. Sci. 2009, 6, 38–42. [Google Scholar] [CrossRef]
  4. Kun, H. Study on Multiphase Flow Mechanism of Deep Condensate Gas Reservoirs. Doctoral Dissertation, University of Science and Technology Beijing, Beijing, China, 2021. (In Chinese). [Google Scholar]
  5. Zhang, S.; Huang, H.; Su, J.; Liu, M. Ultra-deep liquid hydrocarbon exploration potential in the cratonic region of the Tarim Basin inferred from gas condensate genesis. Fuel 2015, 160, 583–595. [Google Scholar] [CrossRef]
  6. Wang, Q. Origin of gas condensate reservoir in Fuman Oilfield, Tarim Basin, NW China. Pet. Explor. Dev. 2023, 50, 1295–1307. [Google Scholar] [CrossRef]
  7. Zhang, S.; Su, J.; Huang, H.; He, K.; Wang, Y.; Wang, H.; Zhang, B.; Wang, X.; Hu, J. Genetic origin of sour gas condensates in the Paleozoic dolomite reservoirs of the Tazhong Uplift, Tarim Basin. Mar. Pet. Geol. 2015, 68, 107–119. [Google Scholar] [CrossRef]
  8. Zhang, G.; Chen, L.; Zhang, Z.; Liu, T.; Yang, H.; Chen, W.; Zhao, K.; Yan, H. Composition and origin of molecular compounds in the condensate oils of the Dabei gas field, Tarim Basin, NW China. Pet. Explor. Dev. 2019, 46, 504–517. [Google Scholar]
  9. Rabinovich, A.; Bedrikovetsky, P.; Tartakovsky, D.M. Analytical model for gravity segregation of horizontal multiphase flow in porous media. Phys. Fluids 2020, 32, 106722. [Google Scholar] [CrossRef]
  10. Ribe, N.M. Theory of melt segregation—A review. J. Volcanol. Geotherm. Res. 1987, 33, 241–253. [Google Scholar] [CrossRef]
  11. Rossen, W.; Van Duijn, C. Gravity segregation in steady-state horizontal flow in homogeneous reservoirs. J. Pet. Sci. Eng. 2004, 43, 99–111. [Google Scholar] [CrossRef]
  12. Kusochkova, E.V.; Indrupskii, I.M.; Surnachev, D.V.; Alekseeva, Y.V.; Drozdov, A.N. Modelling of compositional gradient for reservoir fluid in a gas condensate deposit with account for scattered liquid hydrocarbons. J. Min. Inst. 2024, 270, 904–918. [Google Scholar]
  13. Chen, Q.; Kristensen, M.; Johansen, Y.B.; Achourov, V.; Betancourt, S.S.; Mullins, O.C. (Eds.) Analysis of Lateral Fluid Gradients from DFA Measurements and Simulation of Reservoir Fluid Mixing Processes over Geologic Time. In Proceedings of the SPWLA Annual Logging Symposium, Banff, AB, Canada, 20–24 June 2020. [Google Scholar]
  14. Goncharov, I.V.; Veklich, M.A.; Oblasov, N.V.; Samoilenko, V.V.; Fadeeva, S.V.; Kashapov, R.S.; Trushkov, P.V.; Zherdeva, A.V. Nature of hydrocarbon fluids at the fields in the north of Western Siberia: The geochemical aspect. Geochem. Int. 2023, 61, 103–126. [Google Scholar] [CrossRef]
  15. Liu, J.; Wilkins, J.; Al-Qahtani, M.; Al-Awami, A. (Eds.) Modeling a rich gas condensate reservoir with composition grading and faults. In Proceedings of the SPE Middle East Oil and Gas Show and Conference, Manama, Bahrain, 17–20 March 2001; SPE: Richardson, TX, USA, 2001. [Google Scholar]
  16. Whitson, C.H.; Brule, M.R. Phase Behavior; Society of Petroleum Engineers: Richardson, TX, USA, 2000. [Google Scholar]
  17. Pedersen, K.S.; Christensen, P.L.; Shaikh, J.A.; Christensen, P.L. Phase Behavior of Petroleum Reservoir Fluids; CRC Press: Boca Raton, FL, USA, 2006. [Google Scholar]
  18. Schulte, A. (Ed.) Compositional variations within a hydrocarbon column due to gravity. In Proceedings of the SPE Annual Technical Conference and Exhibition, Dallas, TX, USA, 21–24 September 1980; SPE: Richardson, TX, USA, 1980. [Google Scholar]
  19. Whitson, C.H.; Belery, P. (Eds.) Compositional gradients in petroleum reservoirs. In Proceedings of the SPE University of Tulsa Centennial Petroleum Engineering Symposium, Tulsa, OK, USA, 29–31 August 1994; SPE: Richardson, TX, USA, 1994. [Google Scholar]
  20. Kusochkova, E.; Indrupskiy, I.; Kuryakov, V. (Eds.) Distribution of the initial fluid composition in an oil-gas-condensate reservoir with incomplete gravity segregation. In Proceedings of the IOP Conference Series: Earth and Environmental Science, Banda Aceh, Indonesia, 21 September 2021; IOP Publishing: Bristol, UK, 2021. [Google Scholar]
  21. Baghooee, H.; Montel, F.; Galliero, G.; Yan, W.; Shapiro, A. A new approach to thermal segregation in petroleum reservoirs: Algorithm and case studies. J. Pet. Sci. Eng. 2021, 201, 108367. [Google Scholar] [CrossRef]
  22. Poordad, S.; Forutan, M.K. A review of the potential for CO2 sequestration and enhanced gas recovery in an Iranian gas condensate reservoir from a fluid properties point of view. Pet. Sci. Technol. 2013, 31, 2157–2165. [Google Scholar] [CrossRef]
  23. Rossen, W.R.; Van Duijn, C.; Nguyen, Q.P.; Shen, C.; Vikingstad, A.K. Injection strategies to overcome gravity segregation in simultaneous gas and water injection into homogeneous reservoirs. SPE J. 2010, 15, 76–90. [Google Scholar] [CrossRef]
  24. Warren, J.; Root, P.J. The behavior of naturally fractured reservoirs. SPE J. 1963, 3, 245–255. [Google Scholar] [CrossRef]
  25. Berryman, J.G.; Wang, H.F. The elastic coefficients of double-porosity models for fluid transport in jointed rock. J. Geophys. Res. Solid Earth 1995, 100, 24611–24627. [Google Scholar] [CrossRef]
  26. Moinfar, A.; Narr, W.; Hui, M.H.; Mallison, B.T.; Lee, S.H. (Eds.) Comparison of discrete-fracture and dual-permeability models for multiphase flow in naturally fractured reservoirs. In Proceedings of the SPE Reservoir Simulation Symposium, The Woodlands, TX, USA, 21–23 February 2011; SPE: Richardson, TX, USA, 2011. [Google Scholar]
  27. Karimi-Fard, M.; Durlofsky, L.; Aziz, K. An efficient discrete-fracture model applicable for general-purpose reservoir simulators. SPE J. 2004, 9, 227–236. [Google Scholar] [CrossRef]
  28. Wang, L.; Chen, W.; Vuik, C. Hybrid-dimensional modeling for fluid flow in heterogeneous porous media using dual fracture-pore model with flux interaction of fracture-cavity network. J. Nat. Gas Sci. Eng. 2022, 100, 104450. [Google Scholar] [CrossRef]
  29. Hajibeygi, H.; Karvounis, D.; Jenny, P. A hierarchical fracture model for the iterative multiscale finite volume method. J. Comput. Phys. 2011, 230, 8729–8743. [Google Scholar] [CrossRef]
  30. Ţene, M.; Bosma, S.B.; Al Kobaisi, M.S.; Hajibeygi, H. Projection-based embedded discrete fracture model (pEDFM). Adv. Water Resour. 2017, 105, 205–216. [Google Scholar] [CrossRef]
  31. Moës, N.; Dolbow, J.; Belytschko, T. A finite element method for crack growth without remeshing. Int. J. Numer. Meth. Eng. 1999, 46, 131–150. [Google Scholar] [CrossRef]
  32. Linder, C.; Armero, F. Finite elements with embedded strong discontinuities for the modeling of failure in solids. Int. J. Numer. Meth. Eng. 2007, 72, 1391–1433. [Google Scholar] [CrossRef]
  33. Flemisch, B.; Berre, I.; Boon, W.; Fumagalli, A.; Schwenck, N.; Scotti, A.; Stefansson, I.; Tatomir, A. Benchmarks for single-phase flow in fractured porous media. Adv. Water Resour. 2018, 111, 239–258. [Google Scholar] [CrossRef]
  34. Fumagalli, A.; Scotti, A. A numerical method for two-phase flow in fractured porous media with non-matching grids. Adv. Water Resour. 2013, 62, 454–464. [Google Scholar] [CrossRef]
  35. Huang, Z. Study on Operation Law and Scheme Design of Gas Storage for Edge and Bottom Water Condensate Gas Reservoir. Master’s Thesis, China University of Petroleum (Beijing), Beijing, China, 2022. (In Chinese). [Google Scholar]
Processes 13 01659 g001
Figure 1. Liquid Volumes measured in CVD Tests. Sample 1 exhibits a higher content of heavy components and consequently demonstrates greater liquid volume.
Figure 1. Liquid Volumes measured in CVD Tests. Sample 1 exhibits a higher content of heavy components and consequently demonstrates greater liquid volume.
Processes 13 01659 g001
Processes 13 01659 g002
Figure 2. Vertical distribution of initial fluid composition. (a) Distribution of C1 in the model accounting for gravity segregation, (unit: %). (b) Distribution of C1 in the model without accounting for gravity segregation, (unit: %). (c) Distribution of C17+ in the model accounting for gravity segregation, (unit: %). (d) Distribution of C17+ in the model without accounting for gravity segregation, (unit: %). The model accounting for gravity segregation exhibits an initial fluid composition distribution characterized by heavier components in the upper reservoir and lighter components in the lower zones, whereas the model without accounting for gravity segregation demonstrates a more homogeneous initial composition profile throughout the reservoir.
Figure 2. Vertical distribution of initial fluid composition. (a) Distribution of C1 in the model accounting for gravity segregation, (unit: %). (b) Distribution of C1 in the model without accounting for gravity segregation, (unit: %). (c) Distribution of C17+ in the model accounting for gravity segregation, (unit: %). (d) Distribution of C17+ in the model without accounting for gravity segregation, (unit: %). The model accounting for gravity segregation exhibits an initial fluid composition distribution characterized by heavier components in the upper reservoir and lighter components in the lower zones, whereas the model without accounting for gravity segregation demonstrates a more homogeneous initial composition profile throughout the reservoir.
Processes 13 01659 g002
Processes 13 01659 g003
Figure 3. Distribution of permeability (mD) in the target reservoir. The target reservoir exhibits well-developed fracture–cavity systems with strong heterogeneity.
Figure 3. Distribution of permeability (mD) in the target reservoir. The target reservoir exhibits well-developed fracture–cavity systems with strong heterogeneity.
Processes 13 01659 g003
Processes 13 01659 g004
Figure 4. Historically matching curves of production indicators: (a) daily oil production in the model accounting for gravity segregation; (b) daily oil production in the model without accounting for gravity segregation; (c) daily gas production in the model accounting for gravity segregation; (d) daily gas production in the model without accounting for gravity segregation. The model incorporating gravity segregation demonstrates superior history-matching accuracy in oil production rates, indicating closer alignment with actual reservoir behavior.
Figure 4. Historically matching curves of production indicators: (a) daily oil production in the model accounting for gravity segregation; (b) daily oil production in the model without accounting for gravity segregation; (c) daily gas production in the model accounting for gravity segregation; (d) daily gas production in the model without accounting for gravity segregation. The model incorporating gravity segregation demonstrates superior history-matching accuracy in oil production rates, indicating closer alignment with actual reservoir behavior.
Processes 13 01659 g004
Processes 13 01659 g005
Figure 5. Pressure distribution at the end of the simulation under different injection strategies (MPa): (a) natural gas, top injection; (b) natural gas, bottom injection; (c) nitrogen, top injection; (d) nitrogen, bottom injection. Nitrogen injection demonstrates superior energy supplementation efficiency for reservoir pressure maintenance.
Figure 5. Pressure distribution at the end of the simulation under different injection strategies (MPa): (a) natural gas, top injection; (b) natural gas, bottom injection; (c) nitrogen, top injection; (d) nitrogen, bottom injection. Nitrogen injection demonstrates superior energy supplementation efficiency for reservoir pressure maintenance.
Processes 13 01659 g005
Processes 13 01659 g006
Figure 6. Condensate oil distribution at the end of the simulation under different injection strategies (%): (a) natural gas, top injection; (b) natural gas, bottom injection; (c) nitrogen, top injection; (d) nitrogen, bottom injection. Nitrogen injection induces enhanced condensate dropout within the reservoir formation.
Figure 6. Condensate oil distribution at the end of the simulation under different injection strategies (%): (a) natural gas, top injection; (b) natural gas, bottom injection; (c) nitrogen, top injection; (d) nitrogen, bottom injection. Nitrogen injection induces enhanced condensate dropout within the reservoir formation.
Processes 13 01659 g006
Processes 13 01659 g007
Figure 7. Pressure distribution at the end of the simulation under different injection strategies (MPa): (a) natural gas, top injection; (b) natural gas, bottom injection; (c) nitrogen, top injection; (d) nitrogen, bottom injection. The model accounting for gravity segregation demonstrates greater gas injectivity challenges, resulting in comparatively weaker pressure maintenance performance.
Figure 7. Pressure distribution at the end of the simulation under different injection strategies (MPa): (a) natural gas, top injection; (b) natural gas, bottom injection; (c) nitrogen, top injection; (d) nitrogen, bottom injection. The model accounting for gravity segregation demonstrates greater gas injectivity challenges, resulting in comparatively weaker pressure maintenance performance.
Processes 13 01659 g007
Processes 13 01659 g008
Figure 8. Condensate oil distribution at the end of the simulation under different injection strategies (%): (a) natural gas, top injection; (b) natural gas, bottom injection; (c) nitrogen, top injection; (d) nitrogen, bottom injection. The model accounting for gravity segregation exhibits two characteristic behaviors: (1) suboptimal pressure maintenance due to gas injectivity constraints (18–22% lower pressure buildup compared to conventional models), and (2) accumulation of heavy components (C7+) in lower zones, promoting 25–30% higher condensate dropout volumes.
Figure 8. Condensate oil distribution at the end of the simulation under different injection strategies (%): (a) natural gas, top injection; (b) natural gas, bottom injection; (c) nitrogen, top injection; (d) nitrogen, bottom injection. The model accounting for gravity segregation exhibits two characteristic behaviors: (1) suboptimal pressure maintenance due to gas injectivity constraints (18–22% lower pressure buildup compared to conventional models), and (2) accumulation of heavy components (C7+) in lower zones, promoting 25–30% higher condensate dropout volumes.
Processes 13 01659 g008
Processes 13 01659 g009
Figure 9. Final oil recovery of different strategies: 1. Natural gas, top injection; 2. Natural gas, bottom injection; 3. Nitrogen, top injection; 4. Nitrogen, bottom injection. The model without accounting for gravity segregation systematically overestimates oil recovery and underestimates the production variation between different injection–production strategies due to unaccounted compositional stratification effects.
Figure 9. Final oil recovery of different strategies: 1. Natural gas, top injection; 2. Natural gas, bottom injection; 3. Nitrogen, top injection; 4. Nitrogen, bottom injection. The model without accounting for gravity segregation systematically overestimates oil recovery and underestimates the production variation between different injection–production strategies due to unaccounted compositional stratification effects.
Processes 13 01659 g009
Processes 13 01659 g010
Figure 10. Gas injection volume: 1. Natural gas, top injection; 2. Natural gas, bottom injection; 3. Nitrogen, top injection; 4. Nitrogen, bottom injection. The gas injection volumes of gravity segregation-free model is higher.
Figure 10. Gas injection volume: 1. Natural gas, top injection; 2. Natural gas, bottom injection; 3. Nitrogen, top injection; 4. Nitrogen, bottom injection. The gas injection volumes of gravity segregation-free model is higher.
Processes 13 01659 g010
Table 1. Wellstream composition of Sample 1 (7508 m) and Sample 2 (7990 m).
Table 1. Wellstream composition of Sample 1 (7508 m) and Sample 2 (7990 m).
ComponentSingle Stage De-Oiling mol%Single Stage Degassing mol%Wellstream mol%
Sample 1Sample 2Sample 1Sample 2Sample 1Sample2
CO2//2.3780.3782.2640.343
N2//1.1963.6541.1383.319
C1//91.11983.29286.73675.656
C20.280.213.1478.6913.0097.913
C30.480.511.1252.5531.0942.366
C41.381.190.7151.1310.7471.137
C52.852.250.2660.2580.390.441
C64.444.850.041/0.2530.484
C710.7216.08//0.5261.474
C815.7414.91//0.7591.367
C913.4713.94//0.6481.278
C1012.269.93//0.5900.910
C118.887.01//0.4270.643
C126.585.39//0.3170.494
C135.085.32//0.2440.488
C143.913.75//0.1880.344
C152.873.61/ 0.1380.331
C162.532.40/ 0.1220.220
C171.742.35//0.0840.215
C181.341.33//0.0640.122
C191.210.98//0.0580.090
C200.910.76//0.0440.070
C210.670.61//0.0320.056
C220.520.55//0.0250.050
C230.440.45//0.0210.041
C240.370.36//0.0180.033
C250.330.29//0.0160.027
C260.250.25//0.0120.023
C270.200.19//0.0100.017
C280.150.16//0.0070.015
C290.120.12//0.0060.011
C30+0.280.25//0.0130.023
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

Share and Cite

MDPI and ACS Style

Chen, F.; Li, M.; Yang, Y.; Zhang, Q.; Lin, N.; Wu, K. Impact of Gravity Segregation on Gas Injection Development in Condensate Gas Reservoirs: A Numerical Simulation Study. Processes 2025, 13, 1659. https://doi.org/10.3390/pr13061659

AMA Style

Chen F, Li M, Yang Y, Zhang Q, Lin N, Wu K. Impact of Gravity Segregation on Gas Injection Development in Condensate Gas Reservoirs: A Numerical Simulation Study. Processes. 2025; 13(6):1659. https://doi.org/10.3390/pr13061659

Chicago/Turabian Style

Chen, Fangfang, Mengqin Li, Yang Yang, Qizhu Zhang, Ning Lin, and Keliu Wu. 2025. "Impact of Gravity Segregation on Gas Injection Development in Condensate Gas Reservoirs: A Numerical Simulation Study" Processes 13, no. 6: 1659. https://doi.org/10.3390/pr13061659

APA Style

Chen, F., Li, M., Yang, Y., Zhang, Q., Lin, N., & Wu, K. (2025). Impact of Gravity Segregation on Gas Injection Development in Condensate Gas Reservoirs: A Numerical Simulation Study. Processes, 13(6), 1659. https://doi.org/10.3390/pr13061659

Note that from the first issue of 2016, this journal uses article numbers instead of page numbers. See further details here.

Article Metrics

Back to TopTop