A Numerical Study of Aerodynamic Drag Reduction and Heat Transfer Enhancement Using an Inclined Partition for Electronic Component Cooling
Abstract
1. Introduction
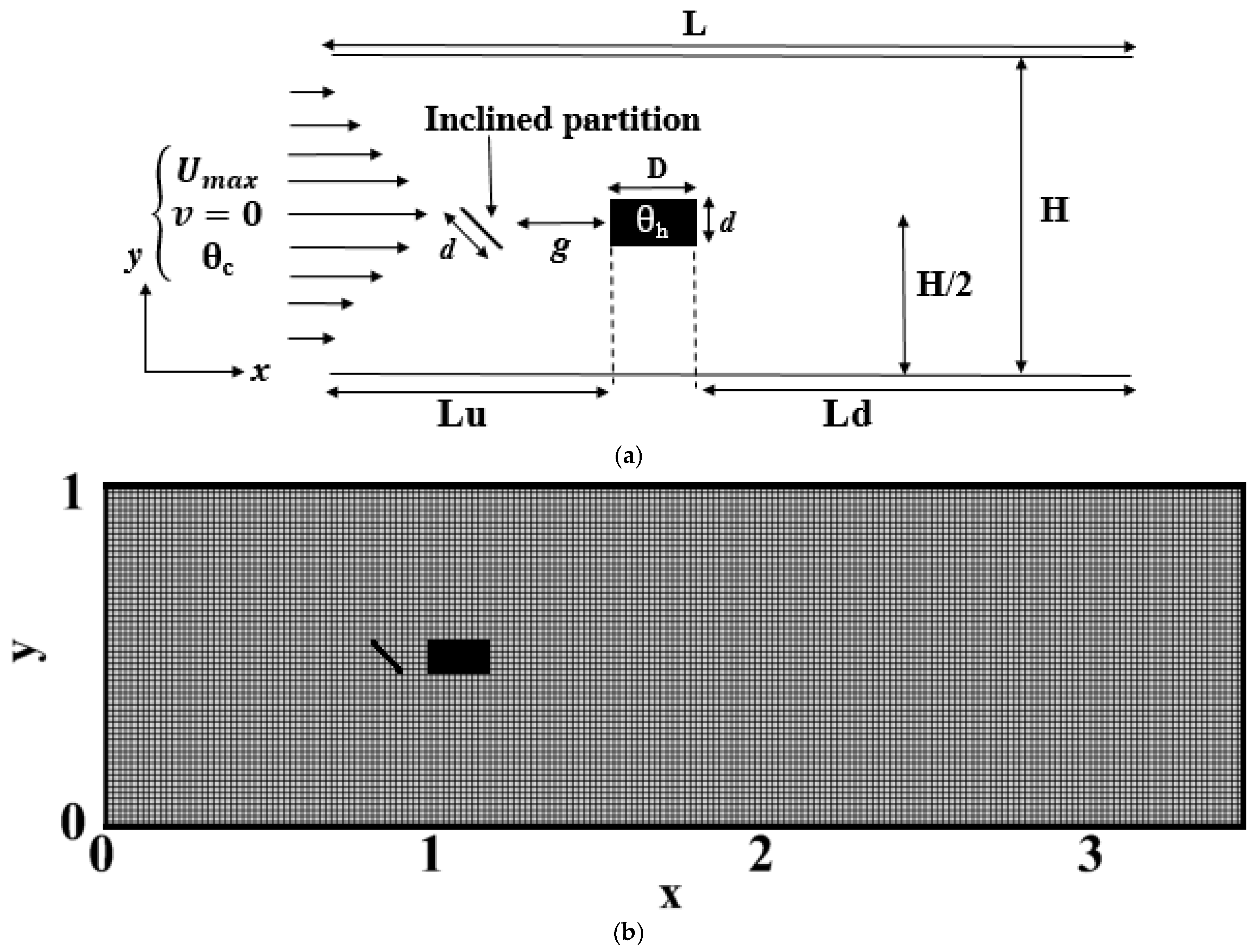
2. Physical Problem
2.1. Geometry Studied
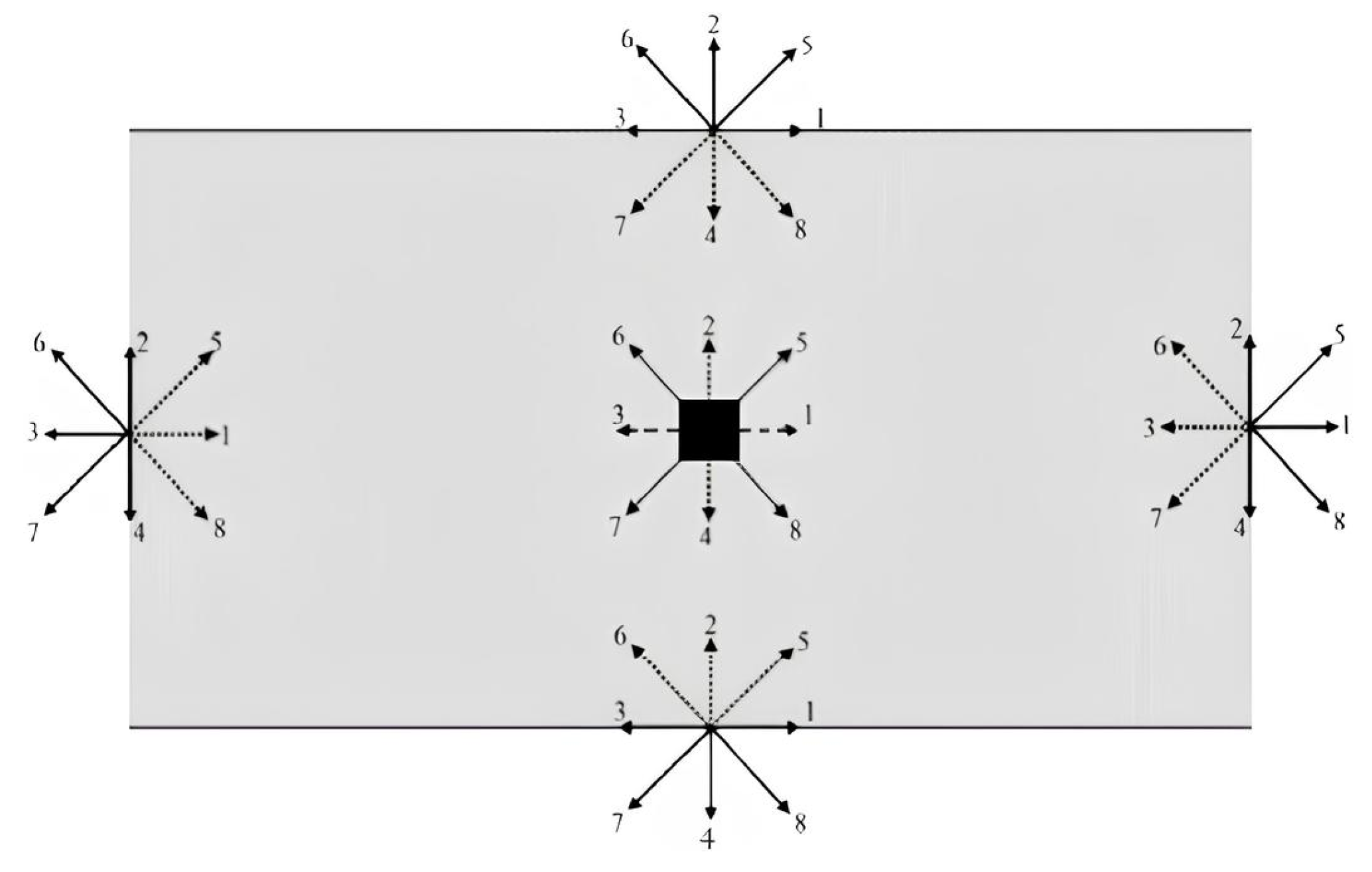
2.2. Boundary Conditions
3. Methodology
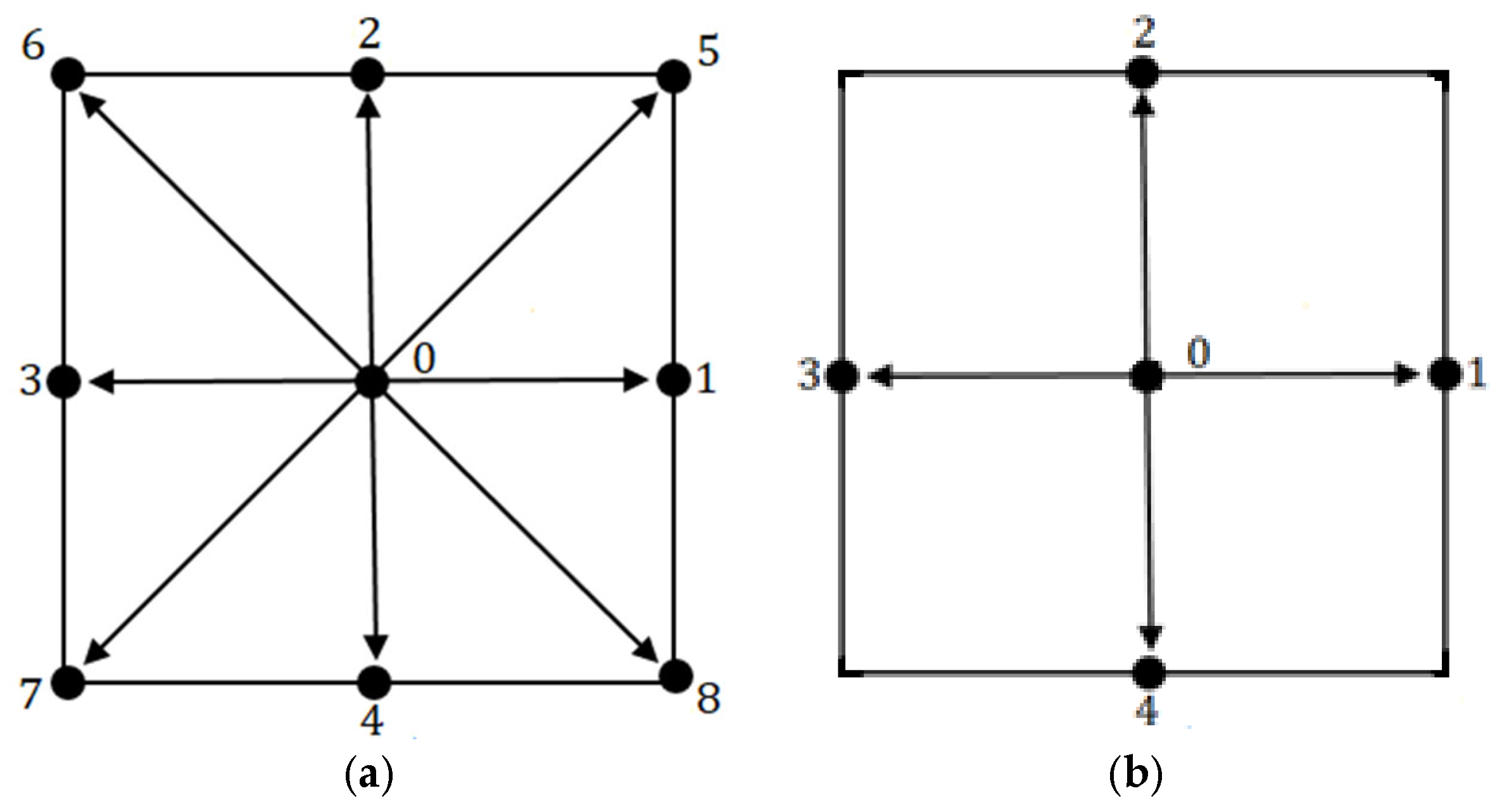
3.1. Lattice Boltzmann Method for Fluid Flow
3.2. Thermal Lattice Boltzmann Method
- ❖
- Collisions,
- ❖
- Propagation (streaming),
- ❖
- Boundary conditions,
- ❖
- Macroscopic quantities.
3.3. Physical Parameters
- Nusselt number
- Drag coefficient
- Reynolds number
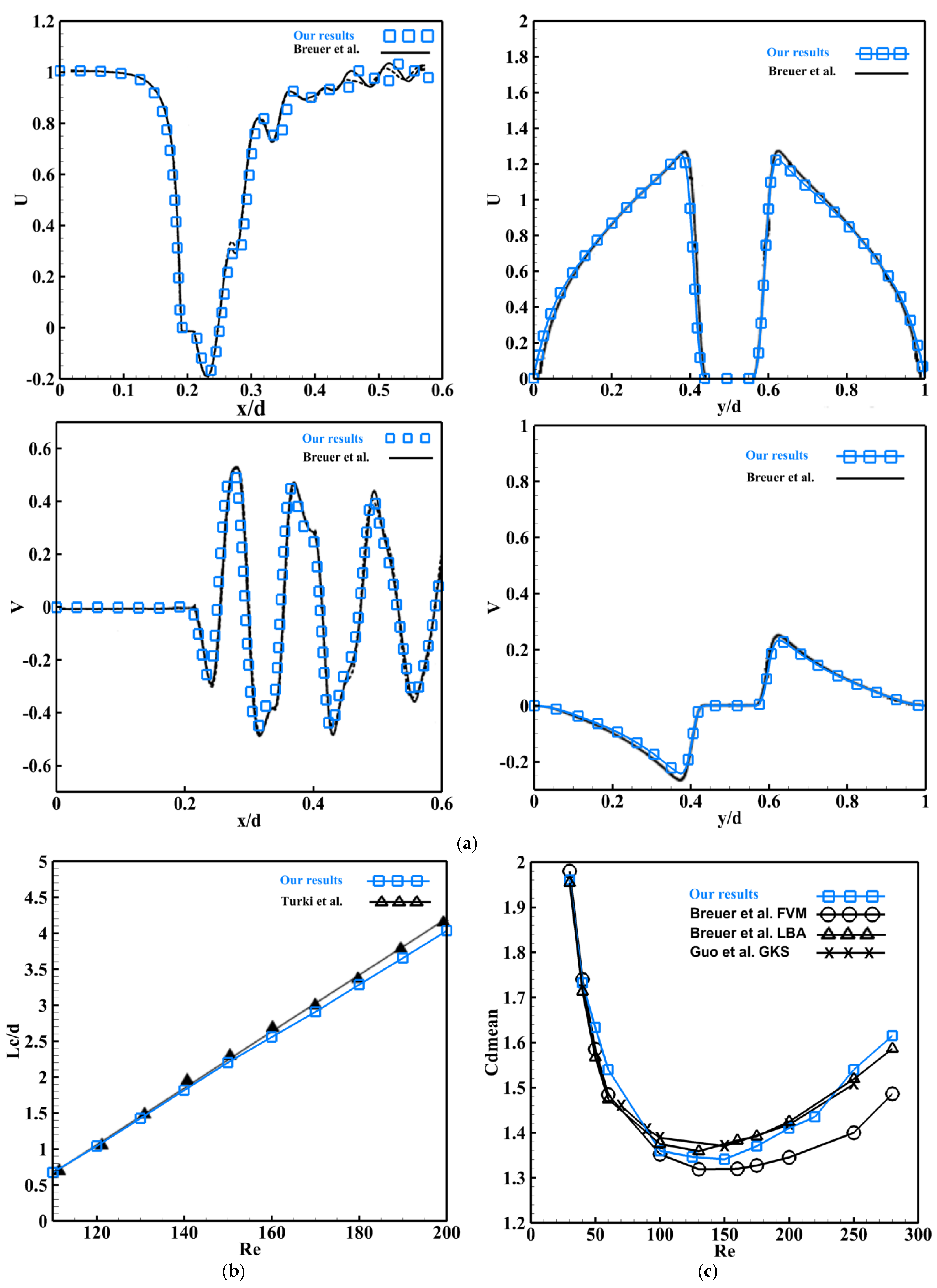
4. Validation of Numerical Code
5. Results and Discussion
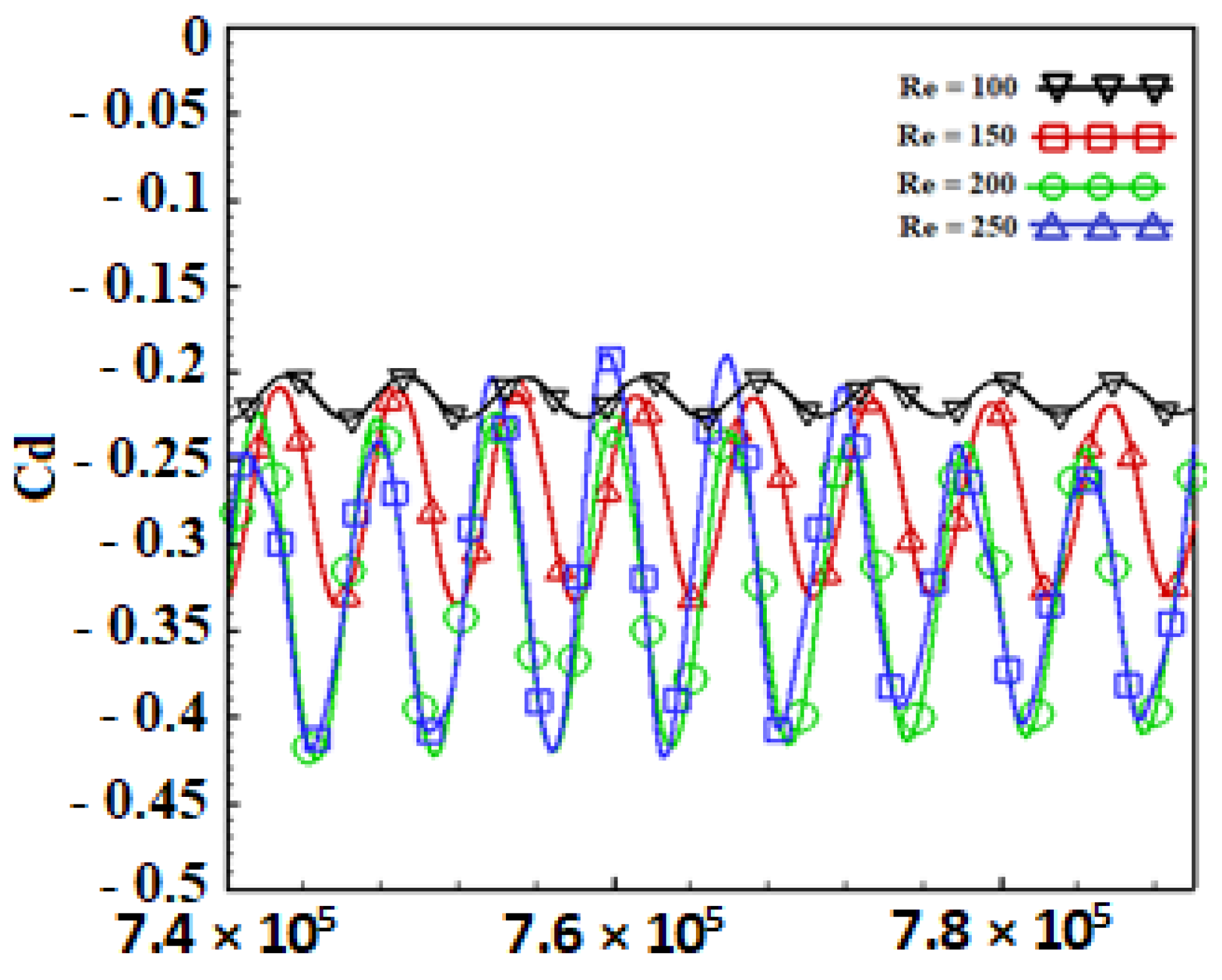
5.1. Reynolds Number Effect
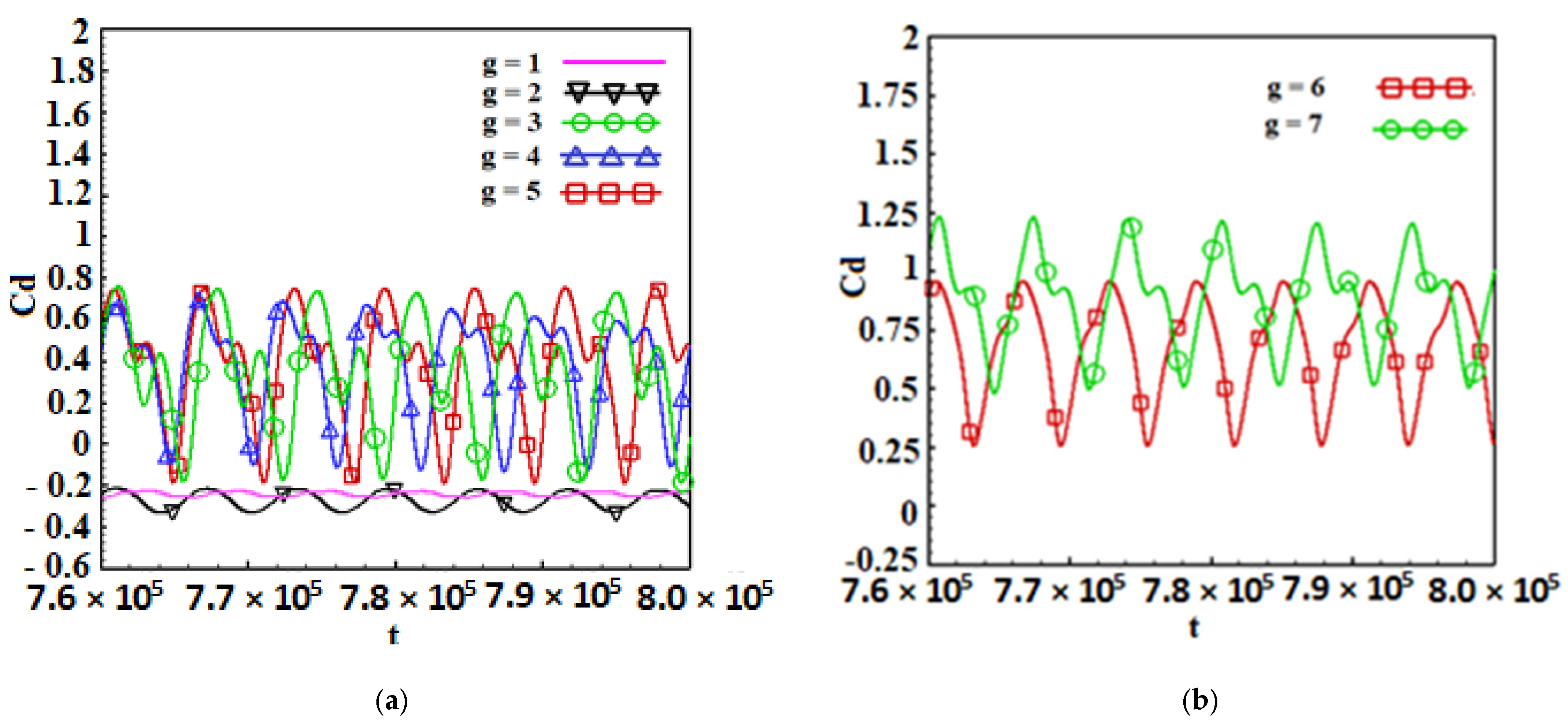
5.1.1. Variation in the Drag Coefficient for
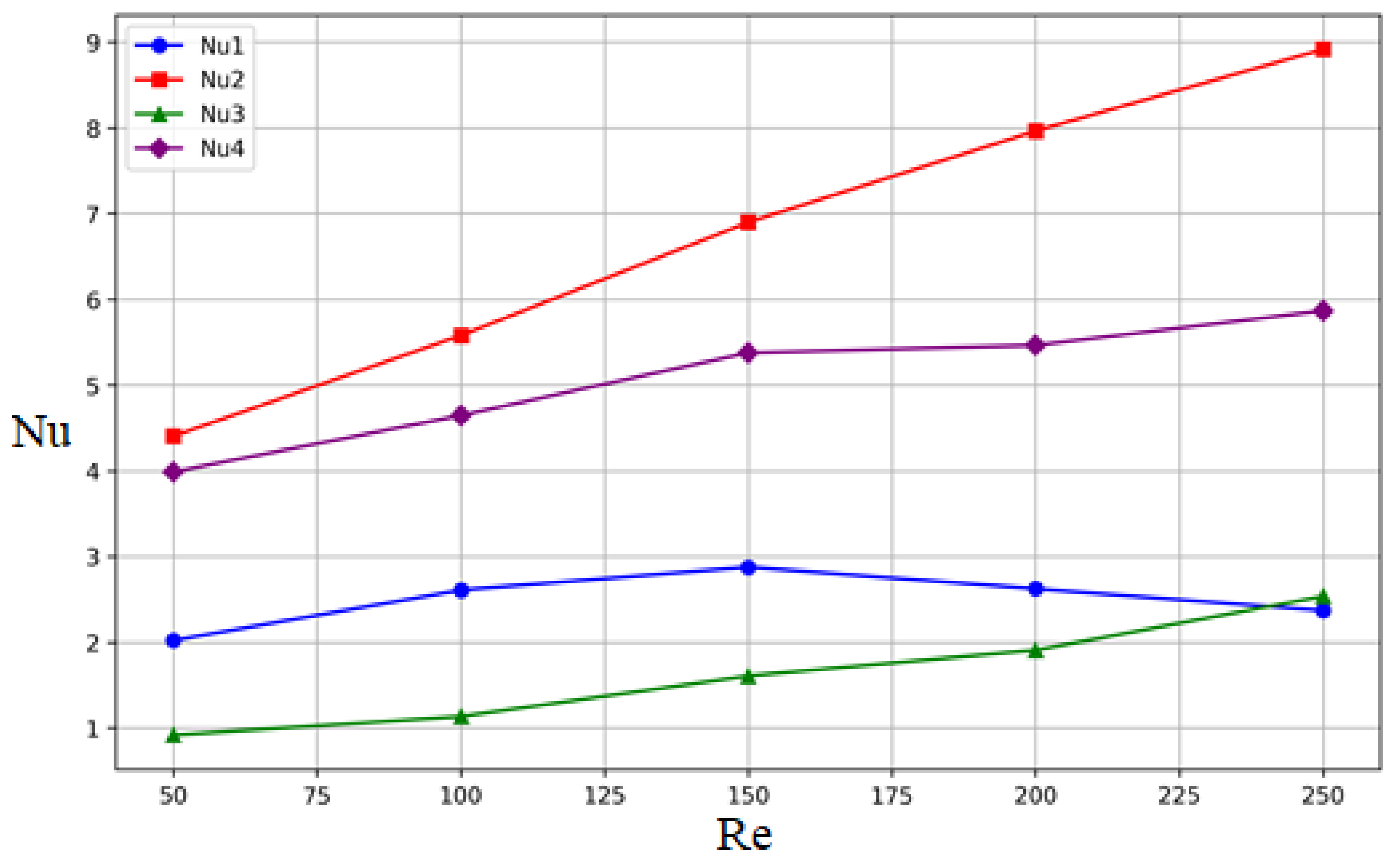
5.1.2. Nusselt Number Variation for
5.2. Gap Spacing Effect
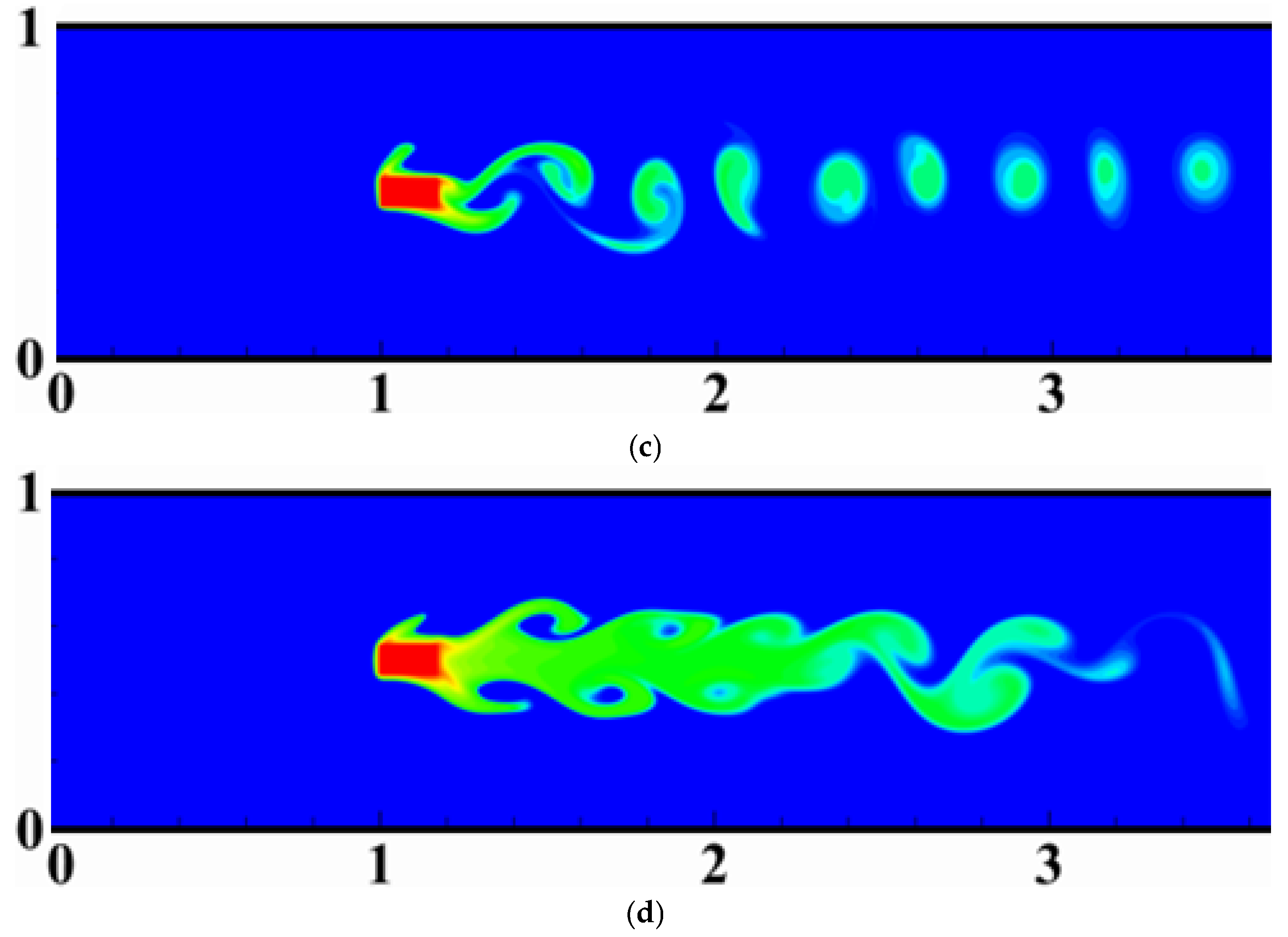
5.2.1. Influence of a Spacing Gap on Thermal Vortex Shedding
5.2.2. Drag Coefficient Variation for at
- ❖
- For
- -
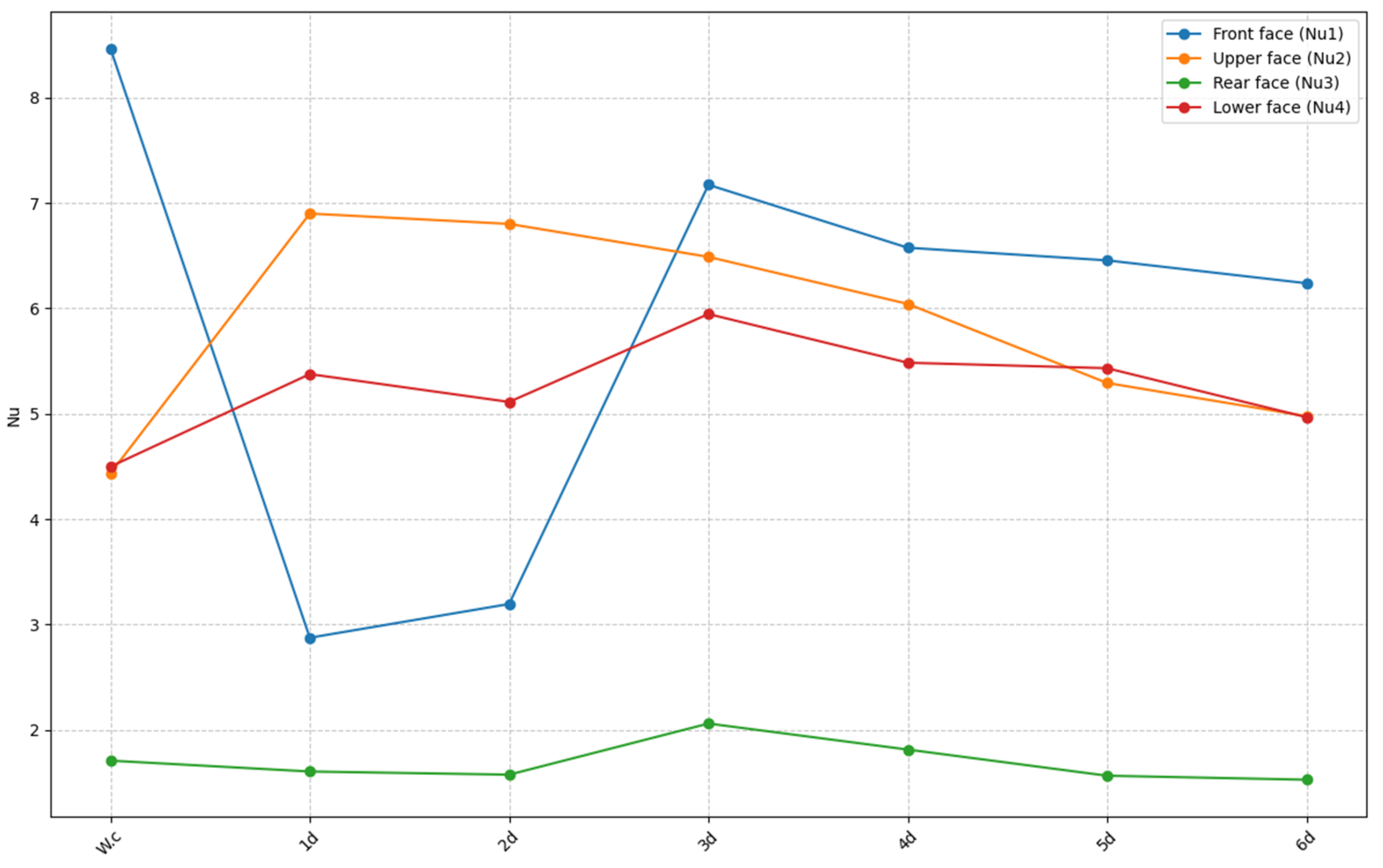
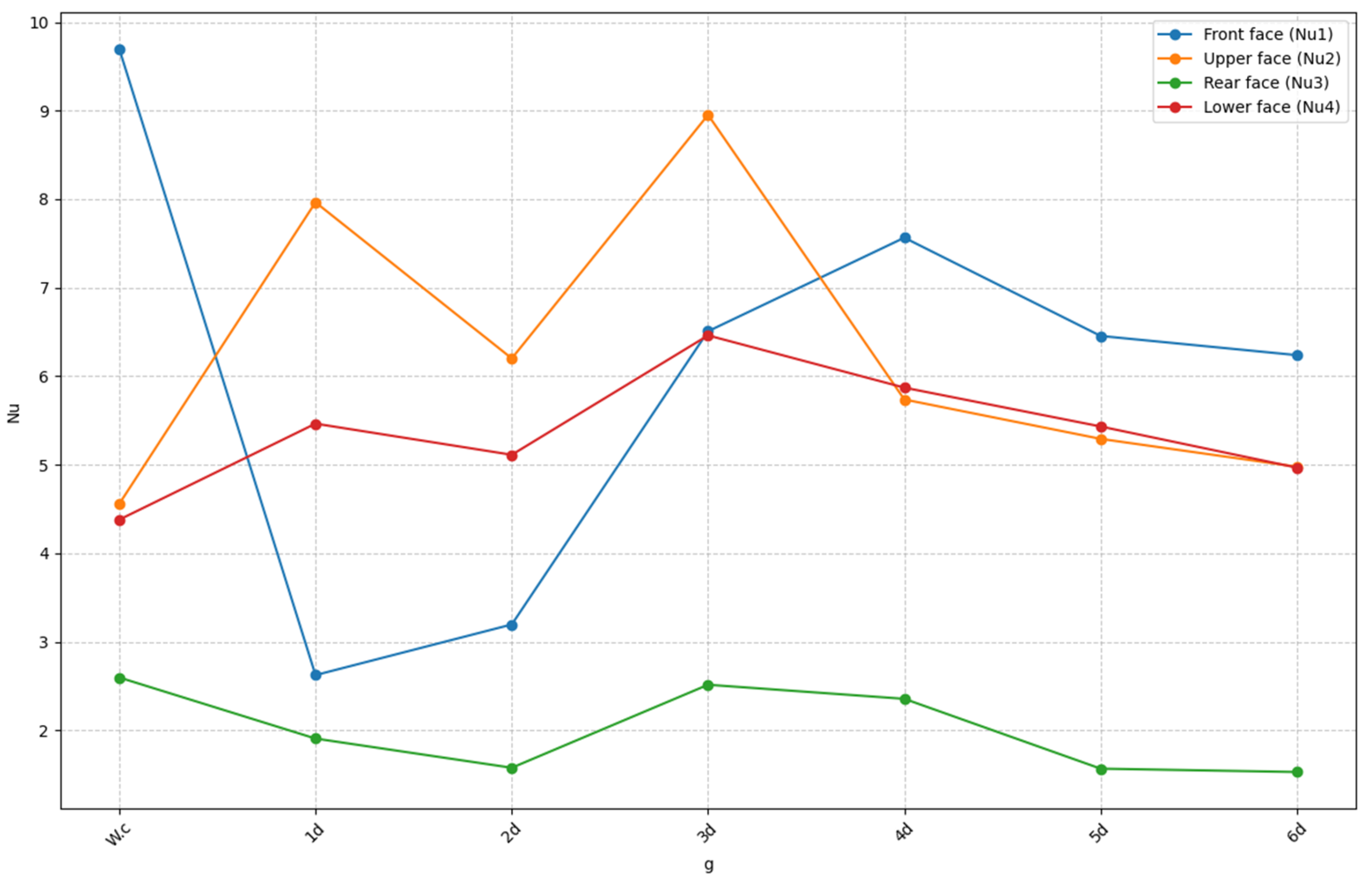
- For the front face: The graph representing shows a maximum value of for the Wc case. In the presence of the partition, drops drastically for , remains low for and 3d, before increasing slightly for .
- -
- For the upper face: The graph representing shows a clear improvement compared to the Wc case. increases progressively with g, reaching a maximum at , then decreases slightly for .
- -
- For the rear face: The graph representing shows low and stable values, with a slight increase in as g increases.
- -
- For the bottom face: The graph representing shows a significant increase compared to the Wc case. progresses with g, reaching a maximum at before stabilizing for higher spacings.
- ❖
- For
- ❖
- For
6. Conclusions
- ❖
- Three distinct thermal regimes emerge with increasing Reynolds numbers, intensifying thermal vortex shedding and improving heat transfer efficiency.
- ❖
- Drag reduction reaches a maximum of at , while heat transfer enhancement, quantified by the Nusselt number, increases with the Reynolds number, peaking at for .
- ❖
- Increasing the gap spacing () between the partition and the cylinder raises the drag coefficient, reducing drag reduction efficiency. Optimal drag reduction () occurs at , while optimal heat transfer is observed at , particularly on the top and bottom faces.
- ❖
- The top face achieves a maximum improvement of at , while the front face consistently experiences reduced heat transfer due to partition shielding.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Cd | Drag coefficient, | Greek symbols | |
| Cdmean | Average drag coefficient, | Β | Blocking ratio, D/H |
| Ci | Discrete velocities (m/s) | θh | Dimensionless hot temperature (°C) |
| d | Width of the rectangular cylinder. | θc | Dimensionless cold temperature (°C) |
| D | Length of the rectangular cylinder. | Density | |
| FD | Drag force (N) | Thermal diffusivity (m2/s) | |
| fi | Flow distribution function | Kinematic viscosity (m2/s) | |
| g | Gap spacing (m) | Ω | Collision operator |
| gi | Temperature distribution function | Subscripts | |
| H | Height of channel (m) | CFDs | Computational fluid dynamics |
| L | Length of channel (m) | LBM | Lattice Boltzmann method |
| Lu | Upstream position (m) | ||
| Ld | Downstream position (m) | MRT | Multi-Relaxation-time |
| Lp | Length of partition (m) | w | Wall |
| M | 9 × 9 transformation matrix for flow field | D2Q5 | Two dimensions and five directions |
| Inverse matrix of the transformation matrix | D2Q9 | Two dimensions and nine directions | |
| mi | Momentum vectors of velocity field | SRT | Single relaxation time |
| Equilibrium momentum vectors of velocity field | TLBE | Thermal lattice Boltzmann equation | |
| N | Thermal field 5 × 5 transformation matrix | ||
| ni | Thermal field momentum vector | ||
| Equilibrium momentum vectors of thermal field | |||
| Average Nusselt number for each face | |||
| Pr | Prandtl number, | ||
| Relaxation time matrix for the thermal problem | |||
| : Aspect ratio | |||
| Re | Reynolds number, | ||
| Si | Diagonal relaxation time matrix for flow field | ||
| t | Time (s) | ||
| T | Temperature (°C) | ||
| u | Component of the velocity in x-axis (m/s) | ||
| Umax | Maximum velocity (m/s) | ||
| v | Component of the velocity in y-axis (m/s) |
Appendix A. Unit Conversion
References
- Gonzalez-Zamudio, E.A.; Olivares-Robles, M.A.; Andrade-Vallejo, A.A. A Novel Case of Cooling and Heating in Rectangular Lid-Driven Cavities: Interplay of Richardson Numbers in Streamlines and Isotherms. Processes 2025, 13, 432. [Google Scholar] [CrossRef]
- Elshaer, A.; Bitsuamlak, G.; El Damatty, A. Enhancing wind performance of tall buildings using corner aerodynamic optimization. Eng. Struct. 2017, 136, 133–148. [Google Scholar] [CrossRef]
- Mena-Pacheco, S.; Tuza, P.V. Synthesis of Combined Heat-and Mass-Exchanger Networks with Multiple Utilities Using the Pinch Technology and Microsoft Excel and GAMS Programs for Comparing Process Flowsheets. Processes 2025, 13, 142. [Google Scholar] [CrossRef]
- Maghrabie, H.M.; Olabi, A.G.; Sayed, E.T.; Wilberforce, T.; Elsaid, K.; Doranehgard, M.H.; Abdelkareem, M.A. Microchannel heat sinks with nanofluids for cooling electronic components: Performance enhancement, challenges, and limitations. Therm. Sci. Eng. Prog. 2023, 37, 101608. [Google Scholar] [CrossRef]
- Lv, Y.G.; Zhang, G.P.; Wang, Q.W.; Chu, W.X. Thermal management technologies used for high heat flux automobiles and aircraft: A review. Energies 2022, 15, 8316. [Google Scholar] [CrossRef]
- Xue, Z.; Wang, P.; Yue, Z.; Lian, C.; Zhang, T.; Gao, M. Advanced cooling channel structures for enhanced heat dissipation in aerospace. Appl. Therm. Eng. 2024, 248, 123346. [Google Scholar] [CrossRef]
- Zhou, L.; Cheng, M.; Hung, K.C. Suppression of fluid force on a square cylinder by flow control. J. Fluids Struct. 2005, 21, 151–167. [Google Scholar] [CrossRef]
- Islam, S.U.; Rahman, H.; Abbasi, W.S.; Noreen, U.; Khan, A. Suppression of fluid force on flow past a square cylinder with a detached flat plate at low Reynolds number for various spacing ratios. J. Mech. Sci. Technol. 2014, 28, 4969–4978. [Google Scholar] [CrossRef]
- Turki, S. Numerical Simulation of Passive Control on Vortex Shedding Behind Square Cylinder Using Splitter Plate. Eng. Appl. Comput. Fluid Mech. 2008, 2, 514–524. [Google Scholar] [CrossRef]
- Dash, S.M.; Triantafyllou, M.S.; Alvarado, P.V.Y. A numerical study on the enhanced drag reduction and wake regime control of a square cylinder using dual splitter plates. Comput. Fluids 2020, 199, 104421. [Google Scholar] [CrossRef]
- Admi, Y.; Moussaoui, M.A.; Mezrhab, A. Effect of Control Partitions on Drag Reduction and Suppression of Vortex Shedding Around a Bluff Body Cylinder. In International Conference on Advanced Technologies for Humanity; Springer: Cham, Switzerland, 2021; pp. 453–463. [Google Scholar] [CrossRef]
- Rashidi, S.; Hayatdavoodi, M.; Esfahani, J.A. Vortex shedding suppression and wake control: A review. Ocean Eng. 2016, 126, 57–80. [Google Scholar] [CrossRef]
- Zou, Q.; He, X. On pressure and velocity boundary conditions for the lattice Boltzmann BGK model. Phys. Fluids. 1997, 9, 1591–1598. [Google Scholar] [CrossRef]
- Bouzidi, M.; Firdaouss, M.; Lallemand, P. Momentum transfer of a Boltzmann lattice fluid with boundaries. Phys. Fluids 2001, 13, 3452–3459. [Google Scholar] [CrossRef]
- Mezrhab, A.; Moussaoui, M.A.; Jami, M.; Naji, H.; Bouzidi, M. Double MRT thermal lattice Boltzmann method for simulating convective flows. Phys. Lett. A 2010, 374, 3499–3507. [Google Scholar] [CrossRef]
- Trouette, B. Lattice Boltzmann simulations of a time-dependent natural convection problem. Comput. Math. Appl. 2013, 66, 1360–1371. [Google Scholar] [CrossRef]
- Li, Z.; Yang, M.; Zhang, Y. Double MRT thermal lattice Boltzmann method for simulating natural convection of low Prandtl number fluids. Int. J. Numer. Methods Heat Fluid Flow. 2016, 26, 1889–1909. [Google Scholar] [CrossRef]
- Mohamad, A.A. Lattice Boltzmann Method: Fundamentals and Engineering Applications with Computer Codes; Springer: Berlin/Heidelberg, Germany, 2011. [Google Scholar] [CrossRef]
- Timm, K.; Kusumaatmaja, H.; Kuzmin, A.; Shardt, O.; Silva, G.; Viggen, E. The Lattice Boltzmann Method: Principles and Practice; Springer International Publishing: Cham, Switzerland, 2017. [Google Scholar] [CrossRef]
- Admi, Y.; El Hassani, S.; Benhamou, J.; Moussaoui, M.A.; Mezrhab, A. Numerical research on the wake characteristics and drag reduction of a square cylinder in the presence of control partitions. Ocean Eng. 2024, 301, 117341. [Google Scholar] [CrossRef]
- D’Humières, D.; Lallemand, P.; Frisch, U. Lattice gas models for 3d hydrodynamics. EPL 1986, 2, 291–297. [Google Scholar] [CrossRef]
- Admi, Y.; Lahmer, E.B.; Benhamou, J.; Moussaoui, M.A.; Mezrhab, A. Heat transfer improvement and drag force reduction around three heated square cylinders controlled by partitions. Phys. Fluids 2024, 36. [Google Scholar] [CrossRef]
- Guo, Z.; Shu, C. Lattice Boltzmann Method and Its Applications in Engineering; World Scientific: Singapore, 2013. [Google Scholar]
- Janjua, M.M.; Khan, N.U.; Khan, W.A.; Khan, W.S.; Ali, H.M. Numerical study of forced convection heat transfer across a cylinder with various cross sections. J. Therm. Anal. Calorim. 2021, 143, 2039–2052. [Google Scholar] [CrossRef]
- Admi, Y.; Channouf, S.; Lahmer, E.B.; Moussaoui, M.A.; Jami, M.; Mezrhab, A. Effect of a Detached Bi-Partition on the Drag Reduction for Flow Past a Square Cylinder. Int. J. Renew. Energy Dev. 2022, 11, 902–915. [Google Scholar] [CrossRef]
- Gao, Y.; Yu, Y.; Yang, L.; Qin, S.; Hou, G. Development of a coupled simplified lattice Boltzmann method for thermal flows. Comput. Fluids 2021, 229, 105042. [Google Scholar] [CrossRef]
- Huang, H.; Sukop, M.; Lu, X. Multiphase Lattice Boltzmann Methods Theory and Application; John Wiley & Sons: Hoboken, NJ, USA, 2015. [Google Scholar] [CrossRef]
- Breuer, M.; Bernsdorf, J.; Zeiser, T.; Durst, F. Durst, Accurate computations of the laminar flow past a square cylinder based on two different methods: Lattice-Boltzmann and finite-volume. Int. J. Heat Fluid Flow 2000, 21, 186–196. [Google Scholar] [CrossRef]
- Guo, Z.; Liu, H.; Luo, L.-S.; Xu, K. A comparative study of the LBE and GKS methods for 2D near incompressible laminar flows. J. Comput. Phys. 2008, 227, 4955–4976. [Google Scholar] [CrossRef]
- Islam, S.U.; Zhou, C.Y.; Shah, A.; Xie, P. Numerical simulation of flow past rectangular cylinders with different aspect ratios using the incompressible lattice Boltzmann method. J. Mech. Sci. Technol. 2012, 26, 1027–1041. [Google Scholar] [CrossRef]
- Dhiman, A.; Chhabra, R.; Eswaran, V. Flow and heat transfer across a confined square cylinder in the steady flow regime: Effect of Peclet number. Int. J. Heat Mass Transf. 2005, 48, 4598–4614. [Google Scholar] [CrossRef]
- Buckingham, E. On physically similar systems; illustrations of the use of dimensional equations. Phys. Rev. 1914, 4, 345. [Google Scholar] [CrossRef]
- Bao, C.; Jiang, Z.; Zhang, X. A general approach of unit conversion system in lattice Boltzmann method and applications for convective heat transfer in tube banks. Int. J. Heat Mass Transf. 2019, 135, 873–884. [Google Scholar] [CrossRef]
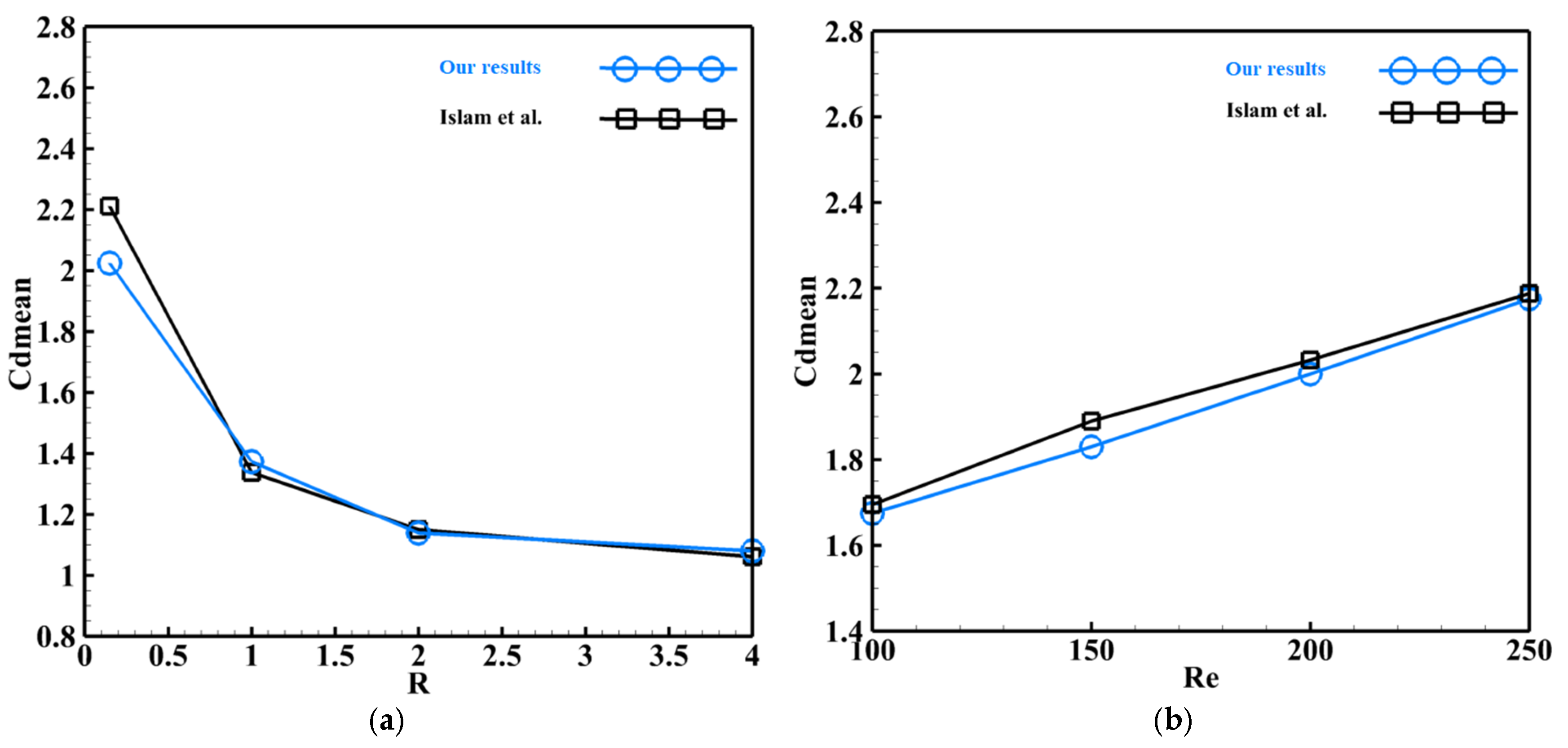
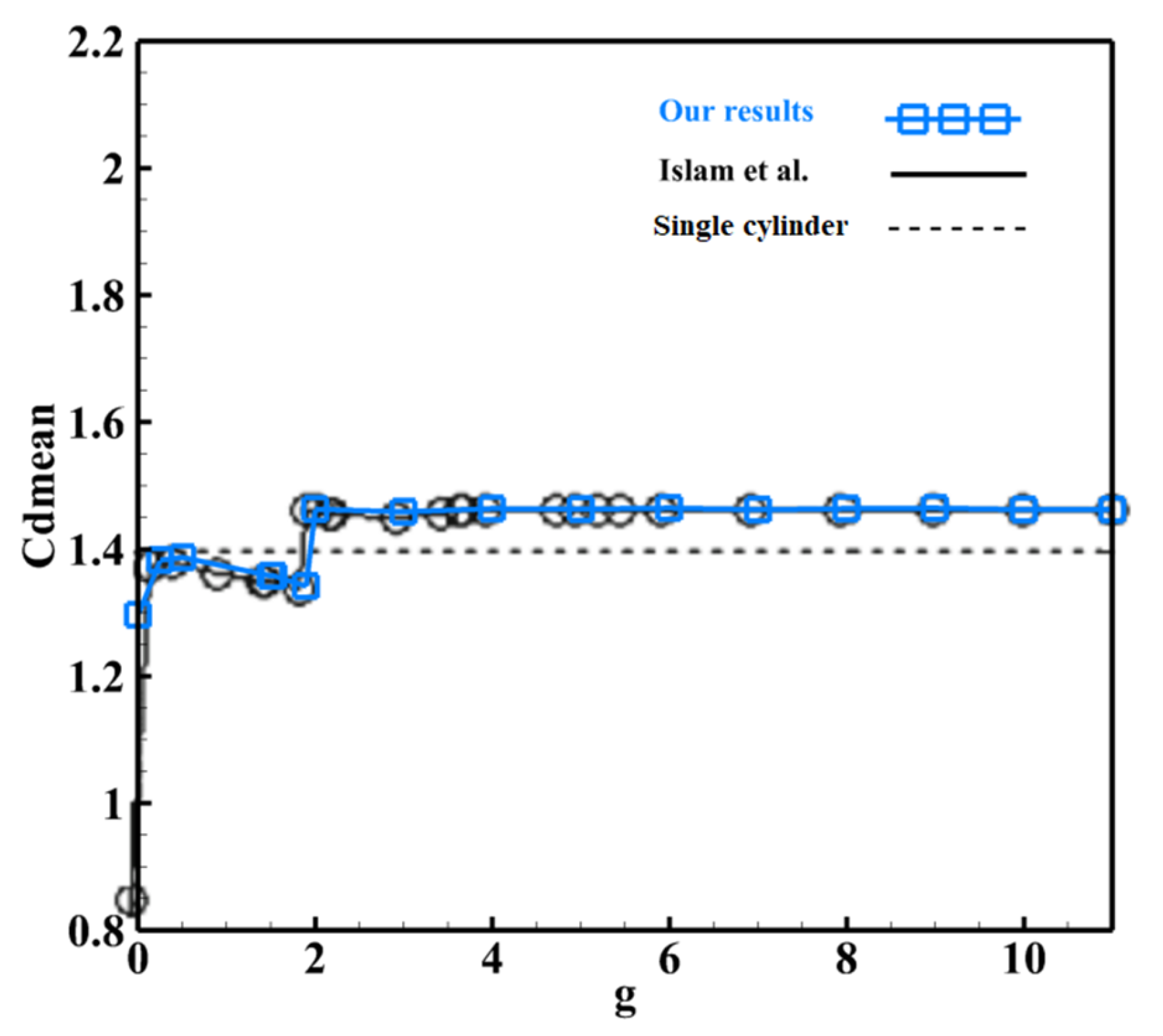
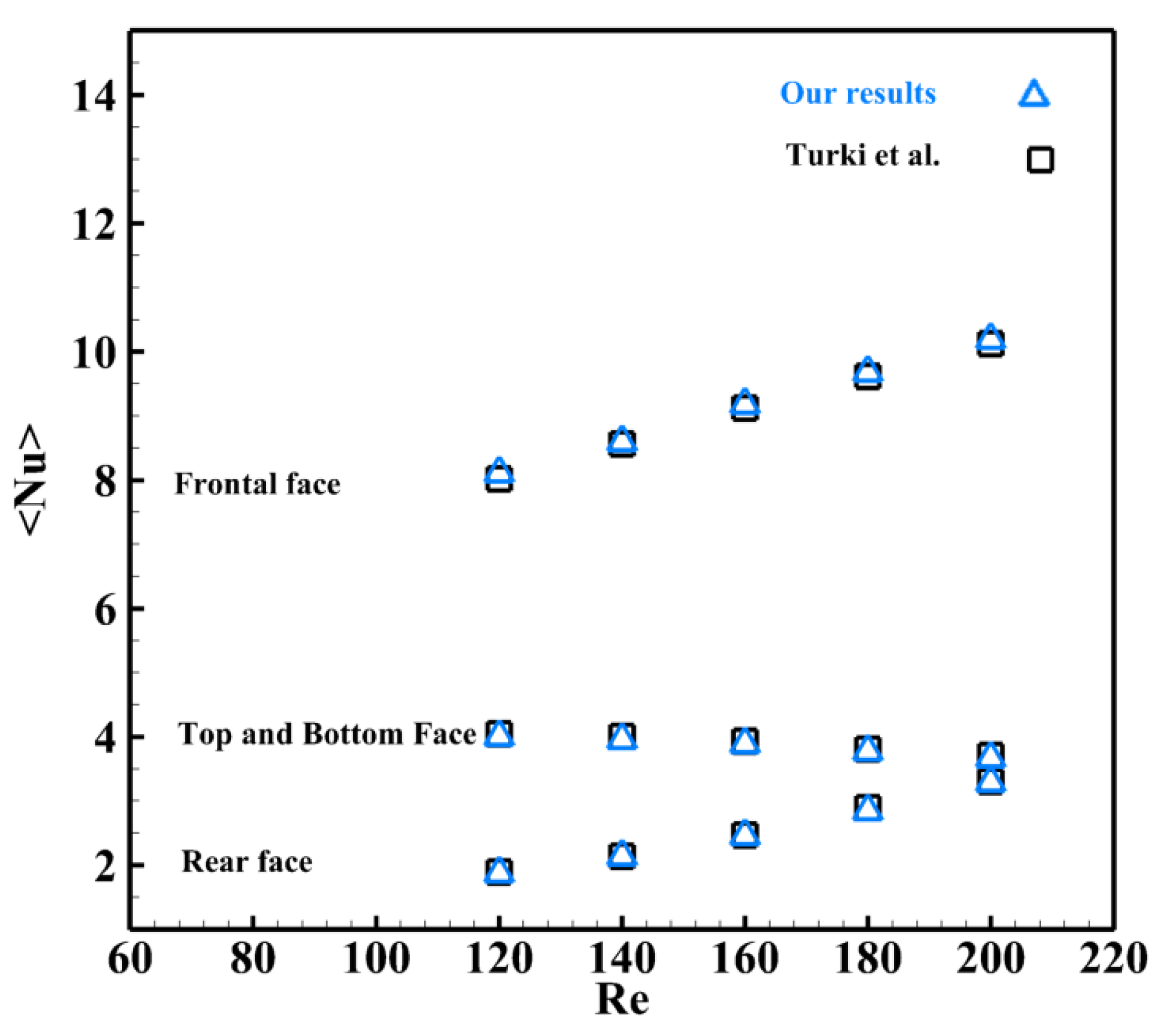





| Source (Frontal Face) | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| % | % | % | % | Nu | % | |||||
| Our results | 3.55 0.84% 3.58 | 3.88 0.78% 3.85 | 4.19 0.47% 4.21 | 4.48 1.10% 4.53 | 4.76 0.21% 4.75 | |||||
| Dhiman et al. [31] | ||||||||||
| Source (Top or Bottom face) | ||||||||||
| % | % | % | % | % | ||||||
| Our results | 0.91 0.011% 0.90 | 0.93 2.197% 0.91 | 0.96 1.05% 0.95 | 0.986 0.61% 0.98 | 1.019 0.098% 1.02 | |||||
| Dhiman et al. [31] | ||||||||||
| Source (Rear face) | ||||||||||
| % | % | % | % | % | ||||||
| Our results | 2.27 1.33% 2.24 | 2.37 0.85% 2.35 | 2.45 0.4% 2.46 | 2.52 1.94% 2.57 | 2.58 1.9% 2.63 | |||||
| Dhiman et al. [31] | ||||||||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Admi, Y.; Makaoui, A.; Moussaoui, M.A.; Mezrhab, A. A Numerical Study of Aerodynamic Drag Reduction and Heat Transfer Enhancement Using an Inclined Partition for Electronic Component Cooling. Processes 2025, 13, 1137. https://doi.org/10.3390/pr13041137
Admi Y, Makaoui A, Moussaoui MA, Mezrhab A. A Numerical Study of Aerodynamic Drag Reduction and Heat Transfer Enhancement Using an Inclined Partition for Electronic Component Cooling. Processes. 2025; 13(4):1137. https://doi.org/10.3390/pr13041137
Chicago/Turabian StyleAdmi, Youssef, Abdelilah Makaoui, Mohammed Amine Moussaoui, and Ahmed Mezrhab. 2025. "A Numerical Study of Aerodynamic Drag Reduction and Heat Transfer Enhancement Using an Inclined Partition for Electronic Component Cooling" Processes 13, no. 4: 1137. https://doi.org/10.3390/pr13041137
APA StyleAdmi, Y., Makaoui, A., Moussaoui, M. A., & Mezrhab, A. (2025). A Numerical Study of Aerodynamic Drag Reduction and Heat Transfer Enhancement Using an Inclined Partition for Electronic Component Cooling. Processes, 13(4), 1137. https://doi.org/10.3390/pr13041137






