Numerical Study of Influence of Nanofluids on the Optimization of Heat Transfer in Immersion Cooling Systems
Abstract
1. Introduction
2. Materials and Methods
2.1. Physical Model
2.2. The Numerical Simulation
2.2.1. Reviewing the LBM
2.2.2. Boundary Conditions
2.3. Nanofluid Properties
3. Results and Discussion
3.1. Mesh Study
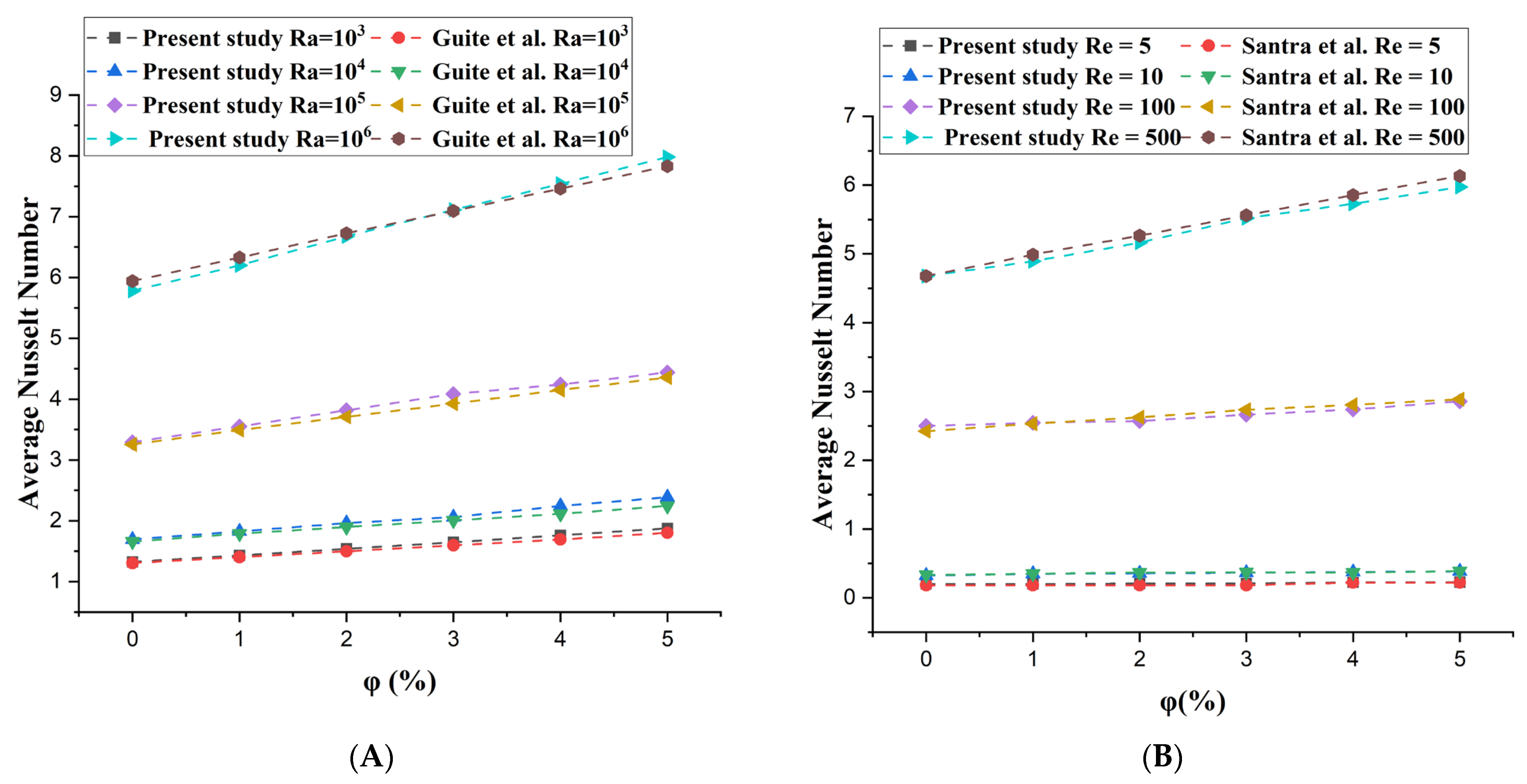
3.2. Validation Model
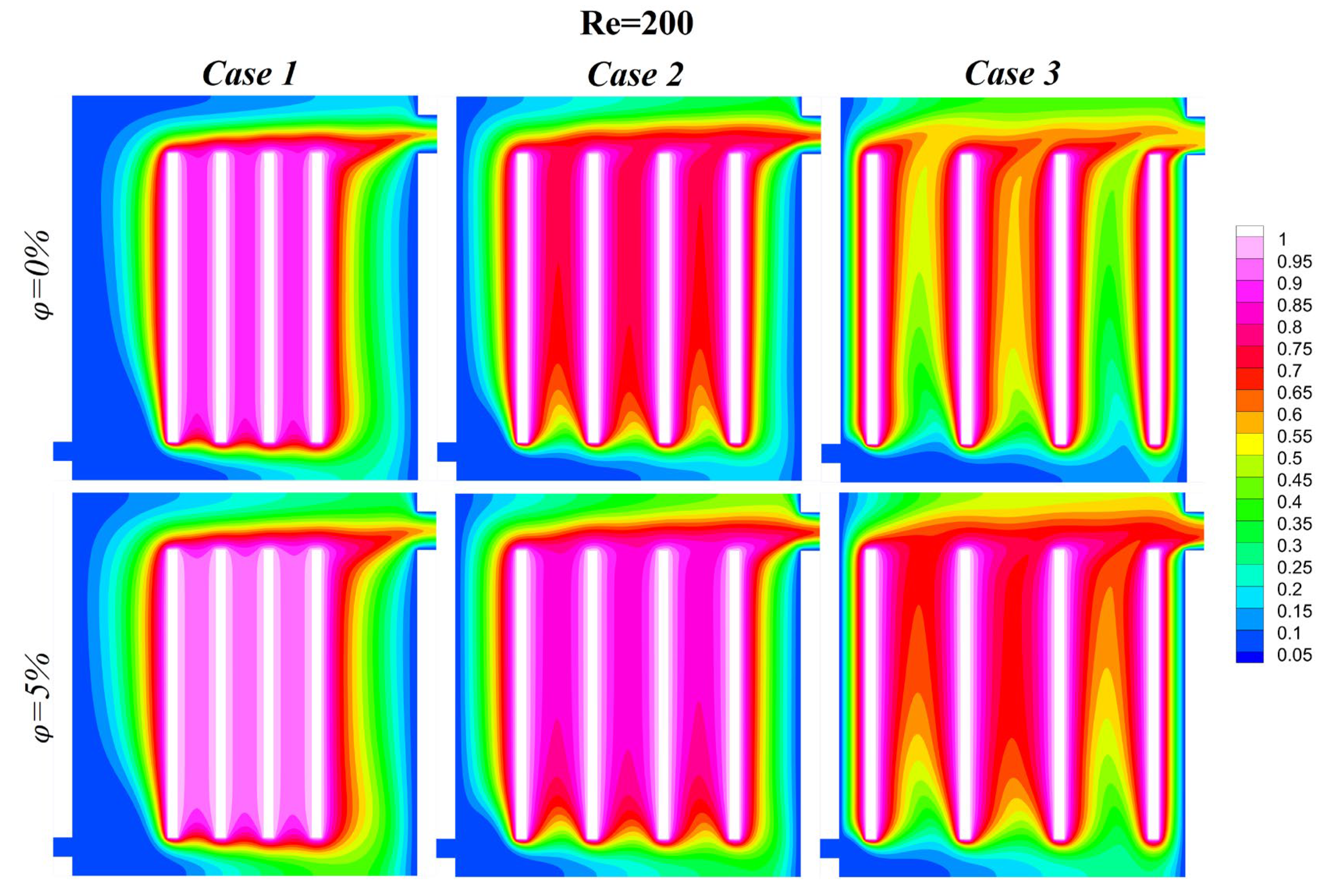
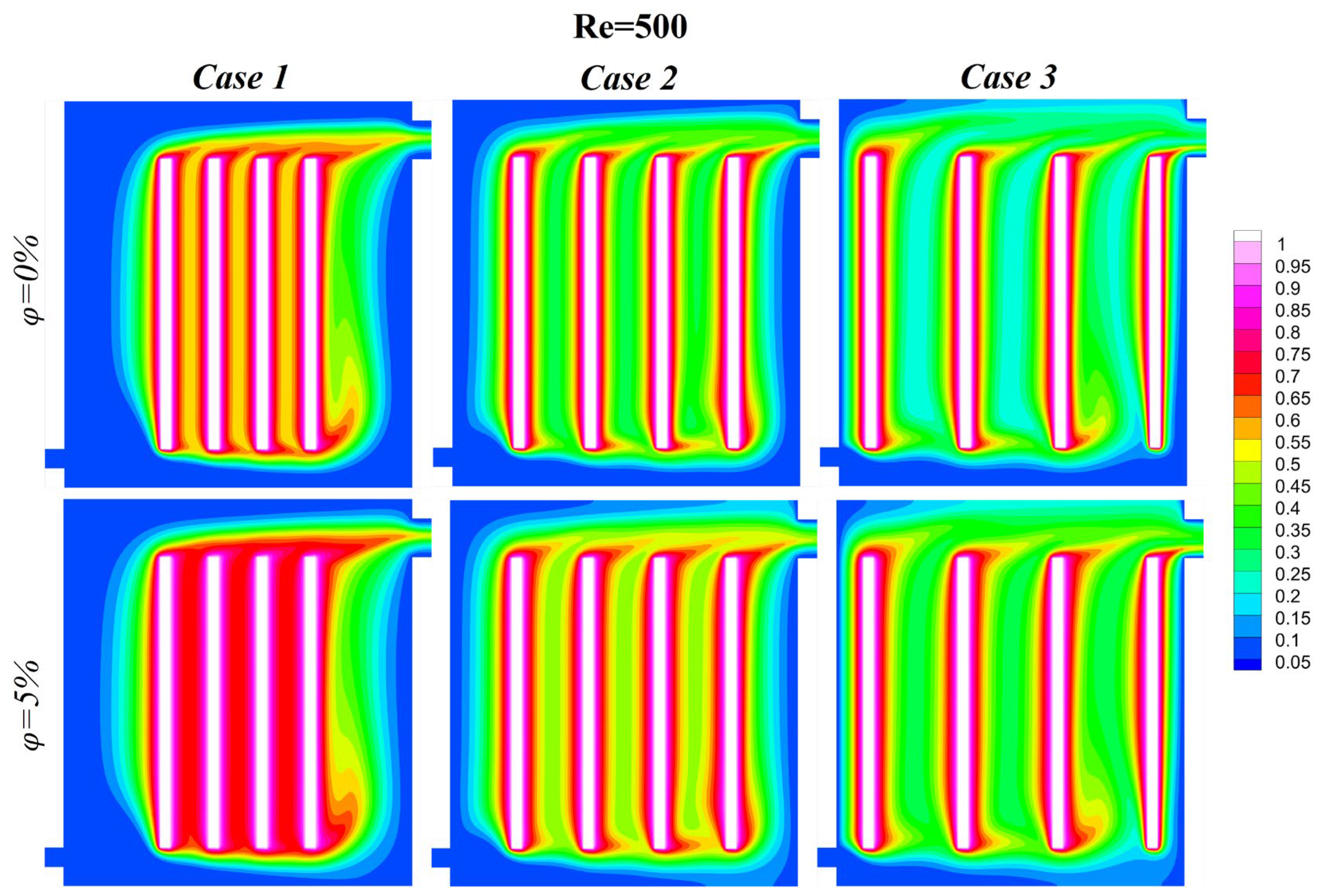
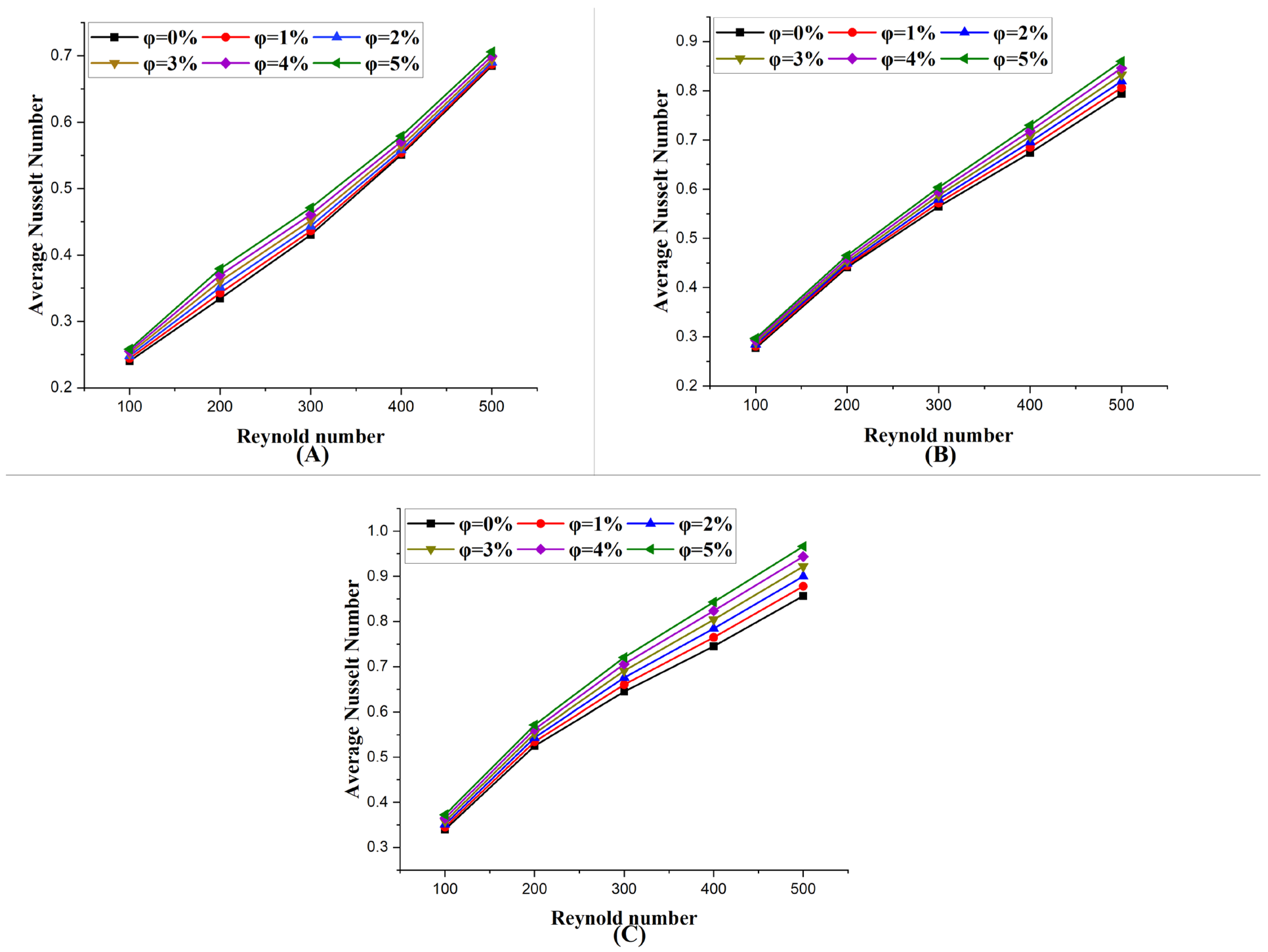
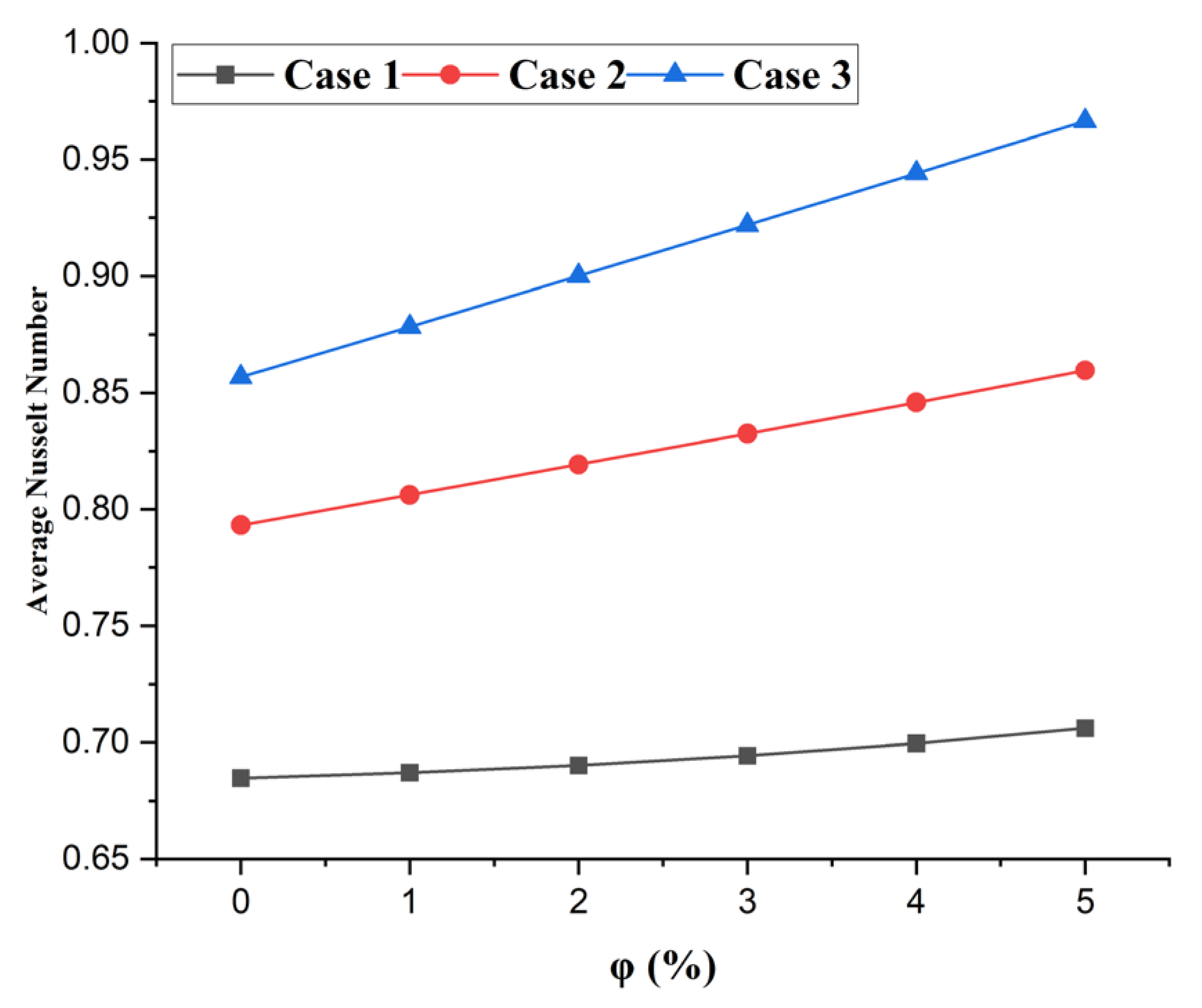
3.3. Impact of Reynolds Numbers and Nanoparticle Volume Fractions
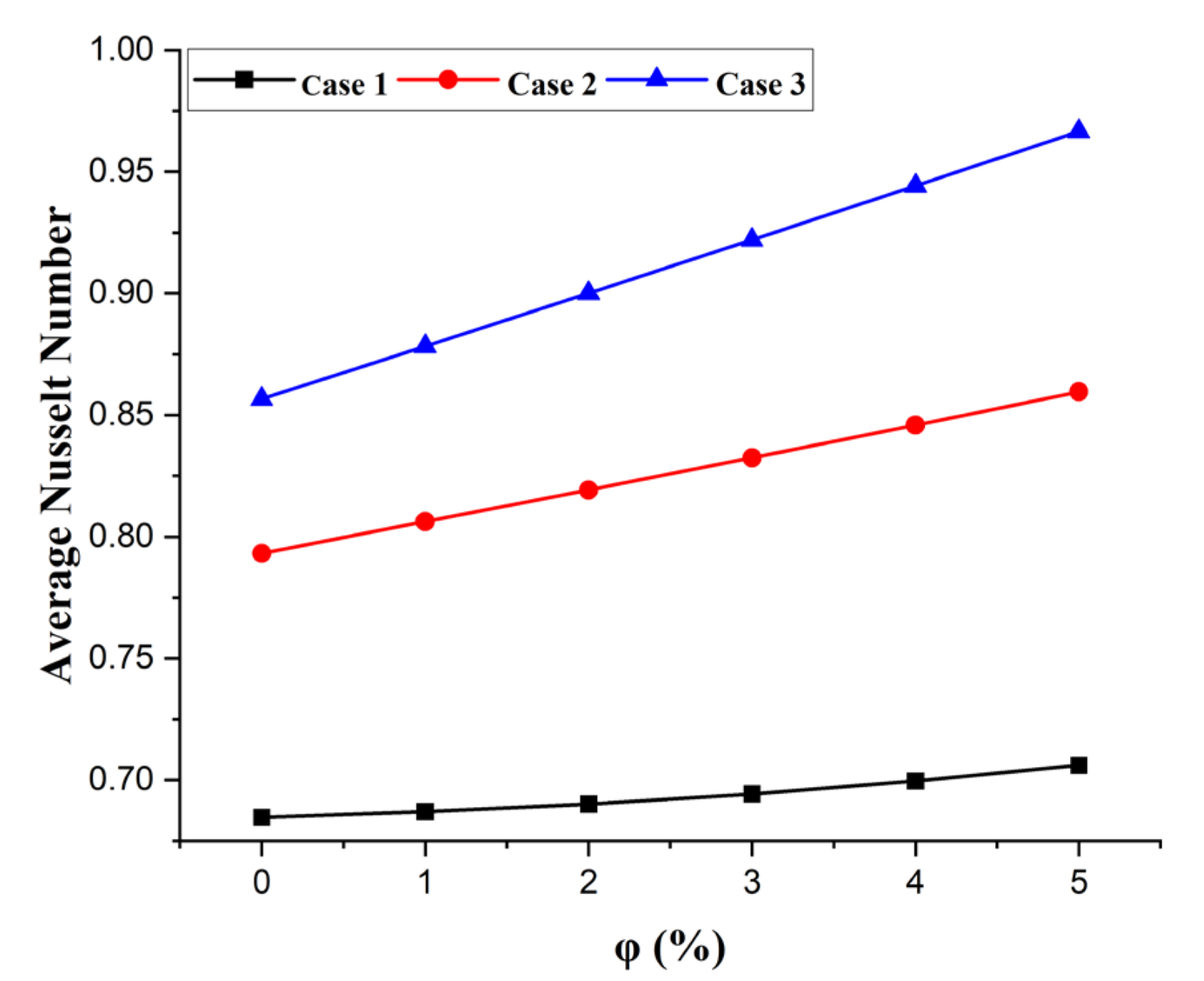
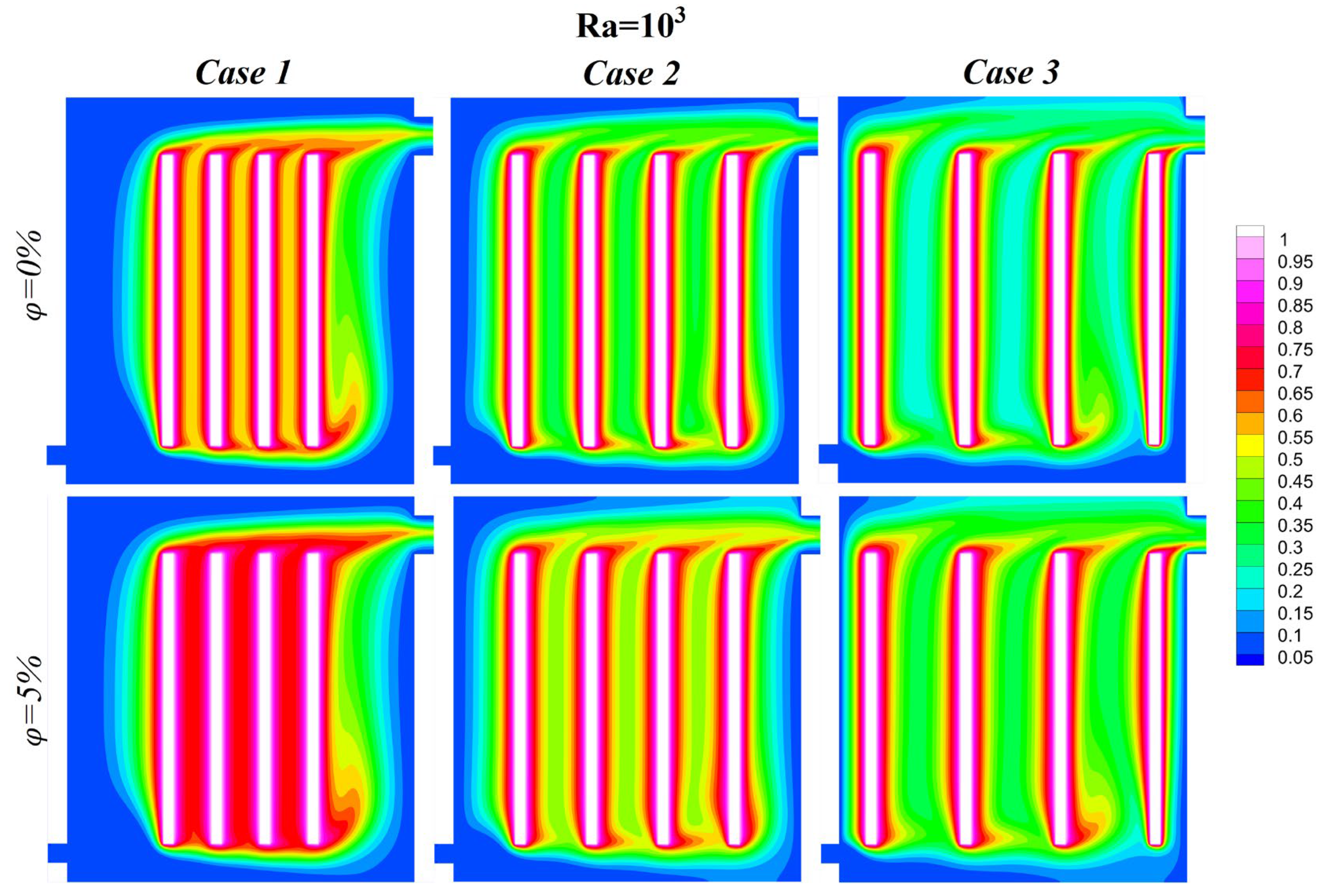
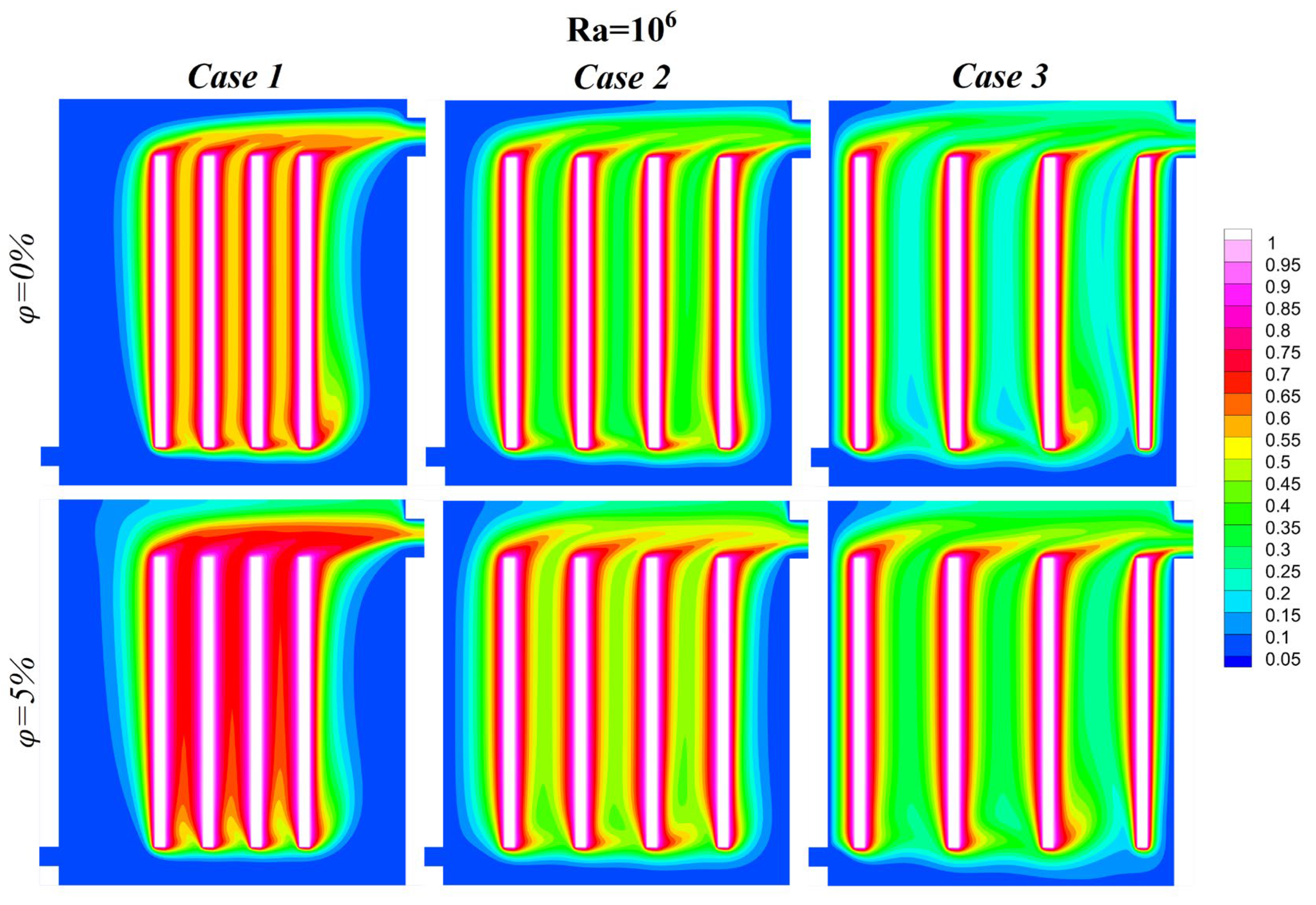
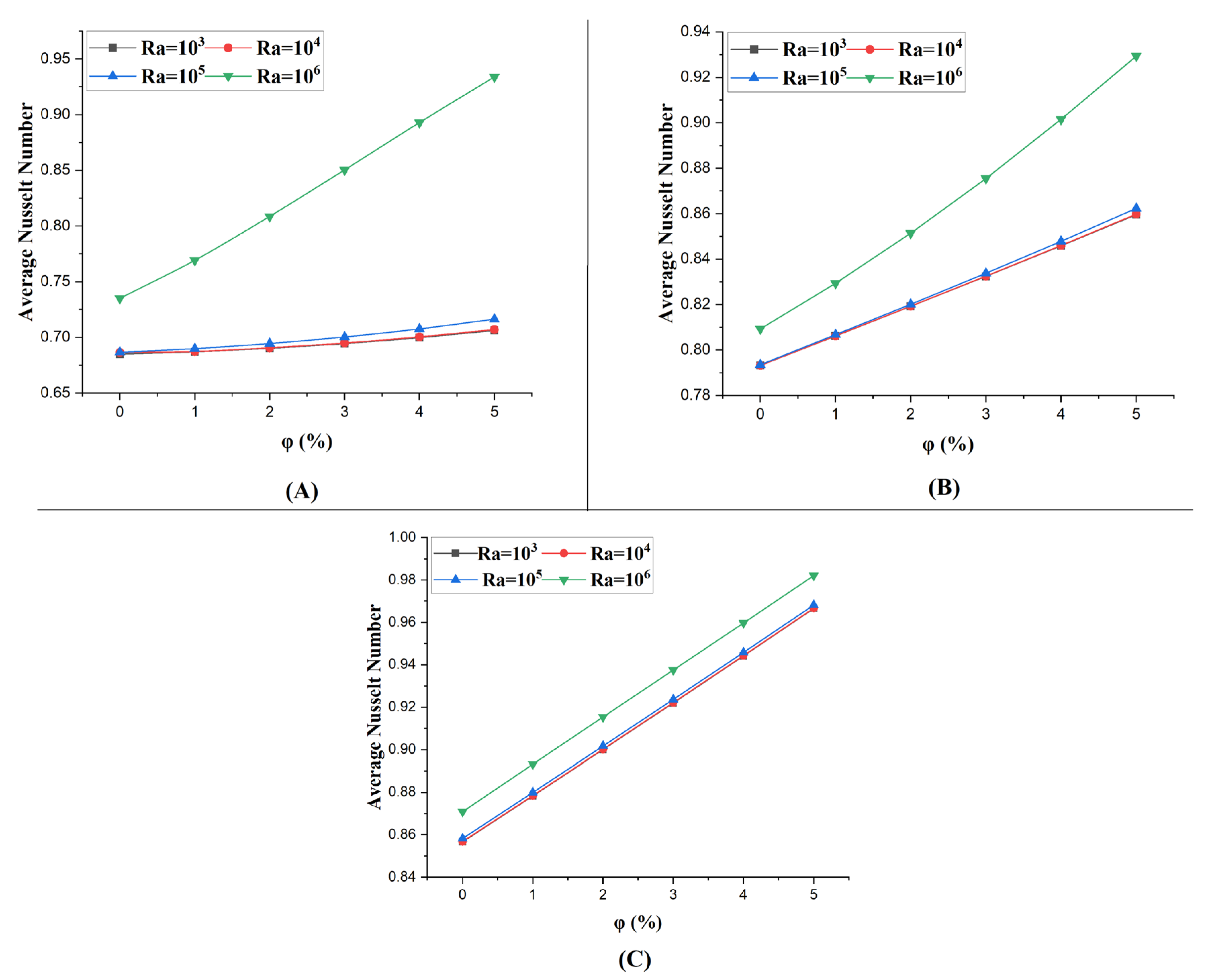
3.4. Impact of Rayleigh Numbers and Nanoparticle Volume Fractions
3.5. Derivation of the Nusselt Number Correlation
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| Distance between blocks, | |
| y-component of gravitational acceleration, | |
| Cavity height, | |
| Height of the blocks, | |
| Cavity length, | |
| Blocks length, | |
| Average Nusselt number | |
| Rayleigh number, | |
| Reynolds number, | |
| Time, | |
| Temperature of fluid, K | |
| x-component of velocity, | |
| y-component of velocity | |
| Cartesian coordinates | |
| Greek symbols | |
| Specific Heat, | |
| Thermal conductivity, | |
| Density, | |
| Diffusivity, | |
| Dynamic viscosity, | |
| Kinematic viscosity, | |
| Volume fraction, % | |
| Subscripts | |
| Cold | |
| Fluid | |
| Hot | |
| Solid | |
| Nanofluid | |
| Abbreviations | |
| Computational Fluid Dynamics | |
| Fluorocarbon liquid | |
| Lattice Boltzmann Method | |
| Single Relaxation Time |
References
- Luo, M.; Cao, J.; Liu, N.; Zhang, Z.; Fang, X. Experimental and Simulative Investigations on a Water Immersion Cooling System for Cylindrical Battery Cells. Front. Energy Res. 2022, 10, 803882. [Google Scholar] [CrossRef]
- Wakabayashi, D.; Yu, Q.; Nakamura, Y. Development of the high efficiency cooling structure of the liquid immersion cooling SR motor. In Proceedings of the 2017 IEEE 19th Electronics Packaging Technology Conference (EPTC), Singapore, 6–9 December 2017; IEEE: Piscataway, NJ, USA, 2017; pp. 1–4. [Google Scholar]
- Xiang, H.; Wang, Y.; Zhu, L.; Han, X.; Sun, Y.; Zhao, Z. 3D numerical simulation on heat transfer performance of a cylindrical liquid immersion solar receiver. Energy Convers. Manag. 2012, 64, 97–105. [Google Scholar] [CrossRef]
- Lionello, M.; Rampazzo, M.; Beghi, A.; Varagnolo, D.; Vesterlund, M. Graph-based modelling and simulation of liquid immersion cooling systems. Energy 2020, 207, 118238. [Google Scholar] [CrossRef]
- Sarangi, S.; McAfee, E.; Damm, D.; Gullbrand, J. Thermal Characterization of Single-Phase Immersion Cooling Capability. In Proceedings of the 2023 22nd IEEE Intersociety Conference on Thermal and Thermomechanical Phenomena in Electronic Systems (ITherm), Orlando, FL, USA, 30 May–2 June 2023; pp. 1–6. [Google Scholar]
- Gao, T.; Tang, H.; Cui, Y.; Luo, Z. A Test Study of Technology Cooling Loop in a Liquid Cooling System. In Proceedings of the 2018 17th IEEE Intersociety Conference on Thermal and Thermomechanical Phenomena in Electronic Systems (ITherm), San Diego, CA, USA, 29 May–1 June 2018; IEEE: Piscataway, NJ, USA, 2018; pp. 740–747. [Google Scholar]
- Joe, E.S.; Arumuga Perumal, D. Computational analysis of fluid immersed active cooling for battery thermal management using thermal lattice Boltzmann method. Eur. Phys. J. Spec. Top. 2022, 231, 2865–2877. [Google Scholar] [CrossRef]
- Saidina, D.S.; Abdullah, M.Z.; Hussin, M. Metal oxide nanofluids in electronic cooling: A review. J. Mater. Sci. Mater. Electron. 2020, 31, 4381–4398. [Google Scholar] [CrossRef]
- Ruan, H.; Xie, H.; Wang, J.; Liao, J.; Sun, L.; Gao, M.; Li, C. Numerical investigation and comparative analysis of nanofluid cooling enhancement for TEG and TEC systems. Case Stud. Therm. Eng. 2021, 27, 101331. [Google Scholar] [CrossRef]
- Niazmand, A.; Murthy, P.; Saini, S.; Shahi, P.; Bansode, P.; Agonafer, D. Numerical Analysis of Oil Immersion Cooling of a Server Using Mineral Oil and Al2O3 Nanofluid. In Proceedings of the International Electronic Packaging Technical Conference and Exhibition, Virtual, 27–29 October 2020. [Google Scholar] [CrossRef]
- Jilte, R.D.; Kumar, R.; Ahmadi, M.H. Cooling performance of nanofluid submerged vs. nanofluid circulated battery thermal management systems. J. Clean. Prod. 2019, 240, 118131. [Google Scholar] [CrossRef]
- Moghaieb, H.S.; Abdel-Hamid, H.M.; Shedid, M.H.; Helali, A.B. Engine cooling using Al2O3/water nanofluids. Appl. Therm. Eng. 2017, 115, 152–159. [Google Scholar] [CrossRef]
- Catherine Joy, R.; Albert Rajan, A.; Brusly Solomon, A.; Ramachandran, K.; Pillai, B.C. Experimental investigation on the critical heat flux of Cu-water, Al-water nanofluids for precise cooling of electronic systems. IOP Conf. Ser.Mater. Sci. Eng. 2019, 561, 012036. [Google Scholar] [CrossRef]
- Cheng, C.-C.; Chang, P.-C.; Li, H.-C.; Hsu, F.-I. Design of a single-phase immersion cooling system through experimental and numerical analysis. Int. J. Heat Mass Transf. 2020, 160, 120203. [Google Scholar] [CrossRef]
- Mehrizi, A.A.; Besharati, F.; Jahanian, O.; Hassanzadeh Afrouzi, H. Numerical investigation of conjugate heat transfer in a microchannel with a hydrophobic surface utilizing nanofluids under a magnetic field. Phys. Fluids 2021, 33, 052002. [Google Scholar] [CrossRef]
- Yousefzadeh, S.; Rajabi, H.; Ghajari, N.; Sarafraz, M.M.; Akbari, O.A.; Goodarzi, M. Numerical investigation of mixed convection heat transfer behavior of nanofluid in a cavity with different heat transfer areas. J. Therm. Anal. Calorim. 2020, 140, 2779–2803. [Google Scholar] [CrossRef]
- Al-Rashed, A.A.A.A.; Kalidasan, K.; Kolsi, L.; Velkennedy, R.; Aydi, A.; Hussein, A.K.; Malekshah, E.H. Mixed convection and entropy generation in a nanofluid filled cubical open cavity with a central isothermal block. Int. J. Mech. Sci. 2018, 135, 362–375. [Google Scholar] [CrossRef]
- Zhang, Y.; Xie, G.; Sundén, B.; Li, Y. An LBM-based investigation of thermal buoyancy and arrangement angle on flow characteristics and heat transfer over four heated square cylinders. Numer. Heat Transf. Part B Fundam. 2021, 79, 278–301. [Google Scholar] [CrossRef]
- Rajpoot, R.S.; Dhinakaran, S.; Alam, M.M. Numerical Analysis of Mixed Convective Heat Transfer from a Square Cylinder Utilizing Nanofluids with Multi-Phase Modelling Approach. Energies 2021, 14, 5485. [Google Scholar] [CrossRef]
- Mahmoodi, M.; Sohankar, A.; Joulaei, A. Investigations of nanofluid flow and heat transfer in a rotating microchannel using single- and two-phase approaches. Numer. Heat Transf. Part A Appl. 2023, 83, 80–115. [Google Scholar] [CrossRef]
- Barnoon, P.; Toghraie, D.; Eslami, F.; Mehmandoust, B. Entropy generation analysis of different nanofluid flows in the space between two concentric horizontal pipes in the presence of magnetic field: Single-phase and two-phase approaches. Comput. Math. Appl. 2019, 77, 662–692. [Google Scholar] [CrossRef]
- Abdelrehim, O.; Khater, A.; Mohamad, A.A.; Radwan, A. Two-phase simulation of nanofluid in a confined single impinging jet. Case Stud. Therm. Eng. 2019, 14, 100423. [Google Scholar] [CrossRef]
- Karpenko, M.; Stosiak, M.; Šukevičius, Š.; Skačkauskas, P.; Urbanowicz, K.; Deptuła, A. Hydrodynamic Processes in Angular Fitting Connections of a Transport Machine’s Hydraulic Drive. Machines 2023, 11, 355. [Google Scholar] [CrossRef]
- Tan, Y.; Ni, Y.; Wu, J.; Li, L.; Tan, D. Machinability evolution of gas–liquid-solid three-phase rotary abrasive flow finishing. Int. J. Adv. Manuf. Technol. 2024, 131, 2145–2164. [Google Scholar] [CrossRef]
- Guo, X.; Yang, M.; Li, F.; Zhu, Z.; Cui, B. Investigation on Cryogenic Cavitation Characteristics of an Inducer Considering Thermodynamic Effects. Energies 2024, 17, 3627. [Google Scholar] [CrossRef]
- Garoosi, F. Presenting two new empirical models for calculating the effective dynamic viscosity and thermal conductivity of nanofluids. Powder Technol. 2020, 366, 788–820. [Google Scholar] [CrossRef]
- Lahmer, E.B.; Benhamou, J.; Admi, Y.; Moussaoui, M.; Phanden, R. Evaluation of Heat Transfer Rate of Double-Layered Heat Sink Cooling System with High Energy Dissipation. J. Enhanc. Heat Transf. 2024, 31, 19–37. [Google Scholar] [CrossRef]
- Moussaoui, M.A.; Admi, Y.; Lahmer, E.B.; Mezrhab, A. Numerical investigation of convective heat transfer in fluid flow past a tandem of triangular and square cylinders in channel. IOP Conf. Ser. Mater. Sci. Eng. 2021, 1091, 012058. [Google Scholar] [CrossRef]
- Admi, Y.; el Hassani, S.; Benhamou, J.; Moussaoui, M. Numerical research on the wake characteristics and drag reduction of a square cylinder in the presence of control partitions. Ocean Eng. 2024, 301, 117341. [Google Scholar] [CrossRef]
- Lahmer, E.B.; Moussaoui, M.A.; Bode, F.; Mezrhab, A. Quality of heat transfer assessment of two microprocessors by double-layered mini channel heat sink cooling system for moderate Reynolds number. Therm. Sci. Eng. Prog. 2023, 41, 101804. [Google Scholar] [CrossRef]
- Moussaoui, M.A.; Derfoufi, S.; Mezrhab, A. Radiation Effect in a Square Cavity Containing a Circular Cylinder with Discrete Bottom Heating. In Advanced Intelligent Systems for Sustainable Development (AI2SD’2020); Advances in Intelligent Systems and Computing Series; Kacprzyk, J., Balas, V.E., Ezziyyani, M., Eds.; Springer International Publishing: Cham, Switzerland, 2022; Volume 1418, pp. 615–625. ISBN 978-3-030-90638-2. [Google Scholar]
- Admi, Y.; Channouf, S.; Lahmer, E.B.; Moussaoui, M.A.; Jami, M.; Mezrhab, A. Effect of a Detached Bi-Partition on the Drag Reduction for Flow Past a Square Cylinder. Int. J. Renew. Energy Dev. 2022, 11, 902–915. [Google Scholar] [CrossRef]
- Zou, Q.; He, X. On pressure and velocity boundary conditions for the lattice Boltzmann BGK model. Phys. Fluids 1997, 9, 1591–1598. [Google Scholar] [CrossRef]
- Patel, H.E.; Anoop, K.B.; Sundararajan, T.; Das, S.K. A micro-convection model for thermal conductivity of nanofluids. In Proceedings of the International Heat Transfer Conference 13, Sydney, Australia, 13–18 August 2006; Begel House Inc.: Danbury, CT, USA, 2006. [Google Scholar]
- Namburu, P.K.; Kulkarni, D.P.; Misra, D.; Das, D.K. Viscosity of copper oxide nanoparticles dispersed in ethylene glycol and water mixture. Exp. Therm. Fluid Sci. 2007, 32, 397–402. [Google Scholar] [CrossRef]
- Mehta, S.; Chauhan, K.P.; Kanagaraj, S. Modeling of thermal conductivity of nanofluids by modifying Maxwell’s equation using cell model approach. J. Nanoparticle Res. 2011, 13, 2791–2798. [Google Scholar] [CrossRef]
- Chon, C.H.; Kihm, K.D. Thermal Conductivity Enhancement of Nanofluids by Brownian Motion. J. Heat Transf. 2005, 127, 810. [Google Scholar] [CrossRef]
- Brinkman, H.C. The Viscosity of Concentrated Suspensions and Solutions. J. Chem. Phys. 2004, 20, 571. [Google Scholar] [CrossRef]
- Guiet, J.; Reggio, M.; Vasseur, P. Natural Convection of Nanofluids in a Square Enclosure with a Protruding Heater. Adv. Mech. Eng. 2012, 4, 167296. [Google Scholar] [CrossRef]
- Santra, A.K.; Sen, S.; Chakraborty, N. Study of heat transfer due to laminar flow of copper–water nanofluid through two isothermally heated parallel plates. Int. J. Therm. Sci. 2009, 48, 391–400. [Google Scholar] [CrossRef]
- Mansour, R.B.; Galanis, N.; Nguyen, C.T. Experimental study of mixed convection with water–Al2O3 nanofluid in inclined tube with uniform wall heat flux. Int. J. Therm. Sci. 2011, 50, 403–410. [Google Scholar] [CrossRef]
- Albojamal, A.; Vafai, K. Analysis of single phase, discrete and mixture models, in predicting nanofluid transport. Int. J. Heat Mass Transf. 2017, 114, 225–237. [Google Scholar] [CrossRef]
- Kimouche, A.; Mataoui, A.; Oztop, H.F.; Abu-Hamdeh, N. Analysis of heat transfer of different nanofluids flow through an abrupt expansion pipe. Appl. Therm. Eng. 2017, 112, 965–974. [Google Scholar] [CrossRef]






| Cases | Distance Ratio | Reynolds Number | Rayleigh Number | Volume Fraction of Nanoparticles |
|---|---|---|---|---|
| Case 1 | 100–500 | 0–5% | ||
| Case 2 | 100–500 | 0–5% | ||
| Case 3 | 100–500 | 0–5% |
| Properties | Water | Copper (Cu) |
|---|---|---|
| Density | ||
| Dynamic viscosity | --- | |
| Specific heat | ||
| Thermal conductivity |
| Grids | |
|---|---|
| 50 × 50 | 0.18033 |
| 100 × 100 | 0.19867 |
| 125 × 125 | 0.21604 |
| 150 × 150 | 0.24094 |
| 200 × 200 | 0.24188 |
| 250 × 250 | 0.24193 |
| 300 × 300 | 0.24202 |
| 350 × 350 | 0.24202 |
| 400 × 400 | 0.24206 |
| Case 1 | Case 2 | Case 3 |
|---|---|---|
| 193.8% | 210.1% | 184.4% |
| Case 1 | Case 2 | Case 3 |
|---|---|---|
| 36.3% | 17.1% | 14.6% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Makaoui, A.; Admi, Y.; Moussaoui, M.A.; Mezrhab, A. Numerical Study of Influence of Nanofluids on the Optimization of Heat Transfer in Immersion Cooling Systems. Processes 2025, 13, 620. https://doi.org/10.3390/pr13030620
Makaoui A, Admi Y, Moussaoui MA, Mezrhab A. Numerical Study of Influence of Nanofluids on the Optimization of Heat Transfer in Immersion Cooling Systems. Processes. 2025; 13(3):620. https://doi.org/10.3390/pr13030620
Chicago/Turabian StyleMakaoui, Abdelilah, Youssef Admi, Mohammed Amine Moussaoui, and Ahmed Mezrhab. 2025. "Numerical Study of Influence of Nanofluids on the Optimization of Heat Transfer in Immersion Cooling Systems" Processes 13, no. 3: 620. https://doi.org/10.3390/pr13030620
APA StyleMakaoui, A., Admi, Y., Moussaoui, M. A., & Mezrhab, A. (2025). Numerical Study of Influence of Nanofluids on the Optimization of Heat Transfer in Immersion Cooling Systems. Processes, 13(3), 620. https://doi.org/10.3390/pr13030620






