Effects of Fuel Input on Pulsation Reactor Behavior—An Experimental Study
Abstract
:1. Introduction
1.1. Pulsation Reactors for Material Treatment
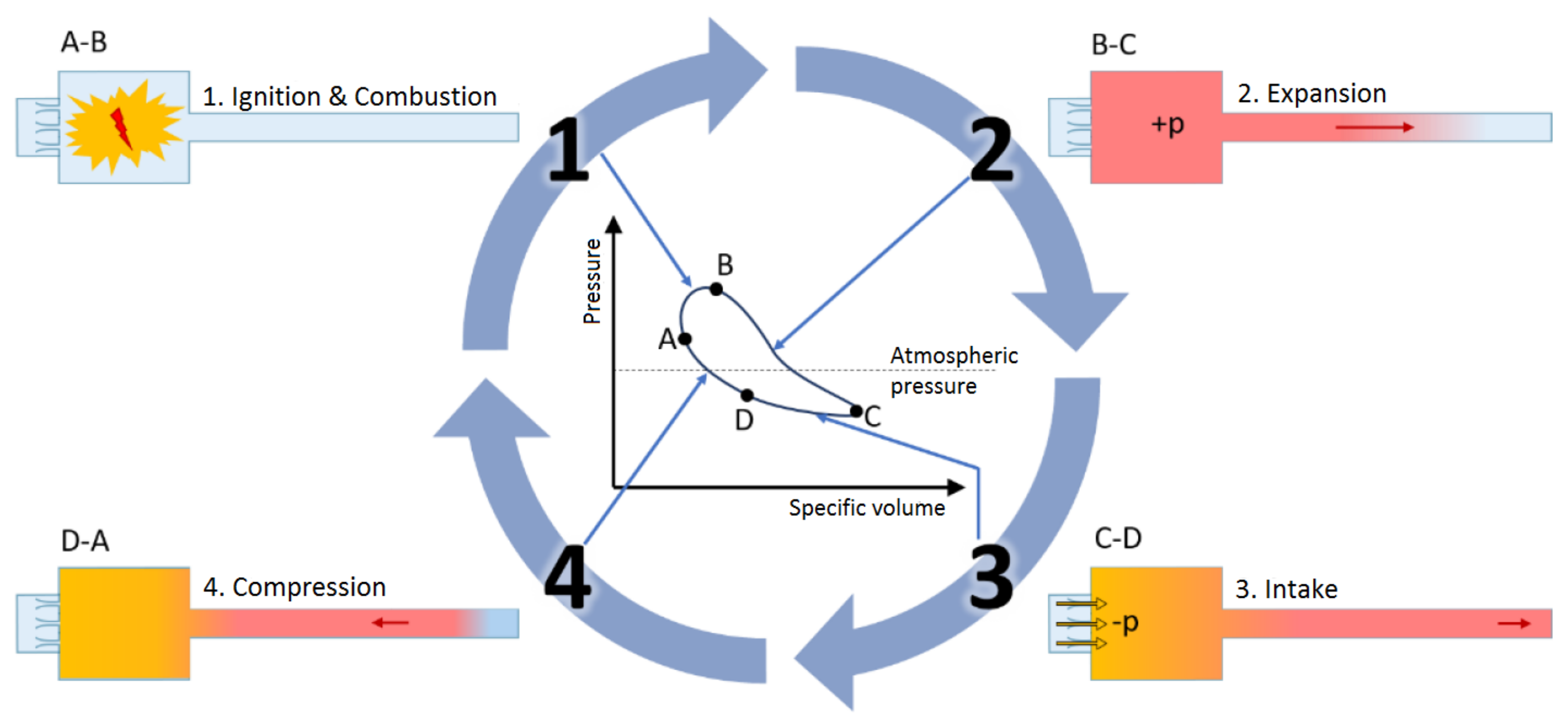
1.2. Pulsating Combustion & the Theory of Pulsation Reactors
- 1.
- Ignition & combustion (A→B): The combustible mixture (air and gaseous fuel) is driven into the combustion chamber where it is ignited. To initialize the operation, a spark plug is typically used. Once self-excited periodic combustion is present, no additional energy source is needed and the spark plug might be switched off.
- 2.
- Expansion (B→C): The combustion is accompanied by a rapid increase of temperature and pressure, pushing the combustion products further towards the end of the exhaust pipe. The excess pressure causes mechanical valves to close completely, preventing both a flow into the combustion chamber and a potential backflow. May aerodynamic valves are used, a fraction of the flue gas might also travel back through the inlet valves.
- 3.
- Intake (C→D): Inertia of the outward traveling gases eventually causes the pressure in the combustion chamber to fall below the ambient pressure, resulting in a fresh mixture being sucked into the combustion chamber.
- 4.
- Compression (D→A): As a consequence of the negative gauge pressure in the combustion chamber, a portion of the flue gas originally moving downstream through the tailpipe reverses its direction and is pulled back into the combustion chamber. The inertia of the fluid entering from both sides of the combustion chamber results in the pressure rising above the ambient level. As the fresh mixture gets in contact with the hot combustion products, it is re-ignited and the cycle repeats.
2. Method
2.1. Investigated PR Characteristics
- (a)
- Operation frequency:
- (b)
- Amplitude of pressure oscillations:
- (c)
- Amplitude of velocity oscillations:
- (d)
- Temperature in the tailpipe: T
2.2. Experimental Setup
2.3. Operation Points
2.4. Data Processing & Related Calculations
3. Results & Discussion
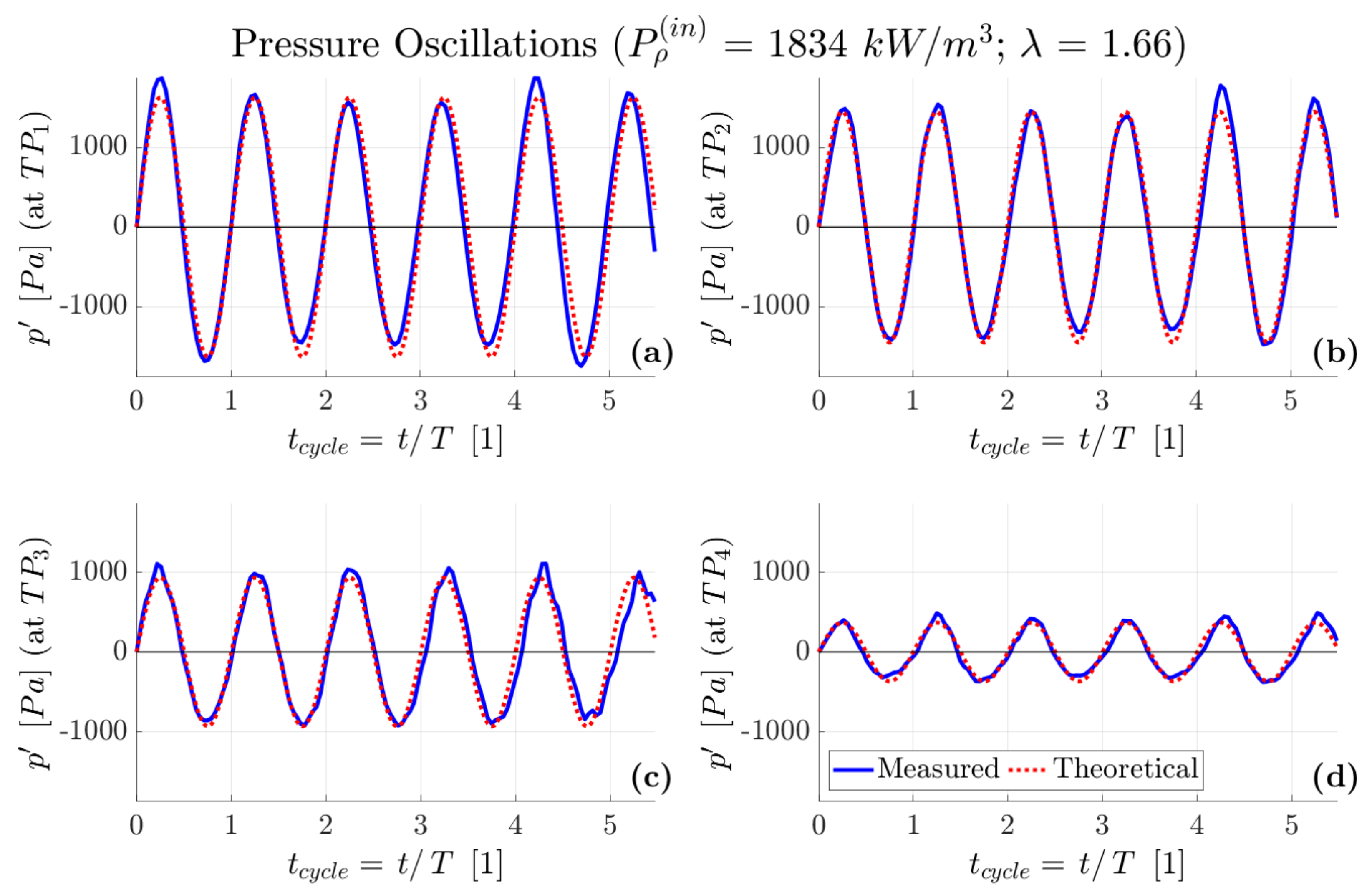
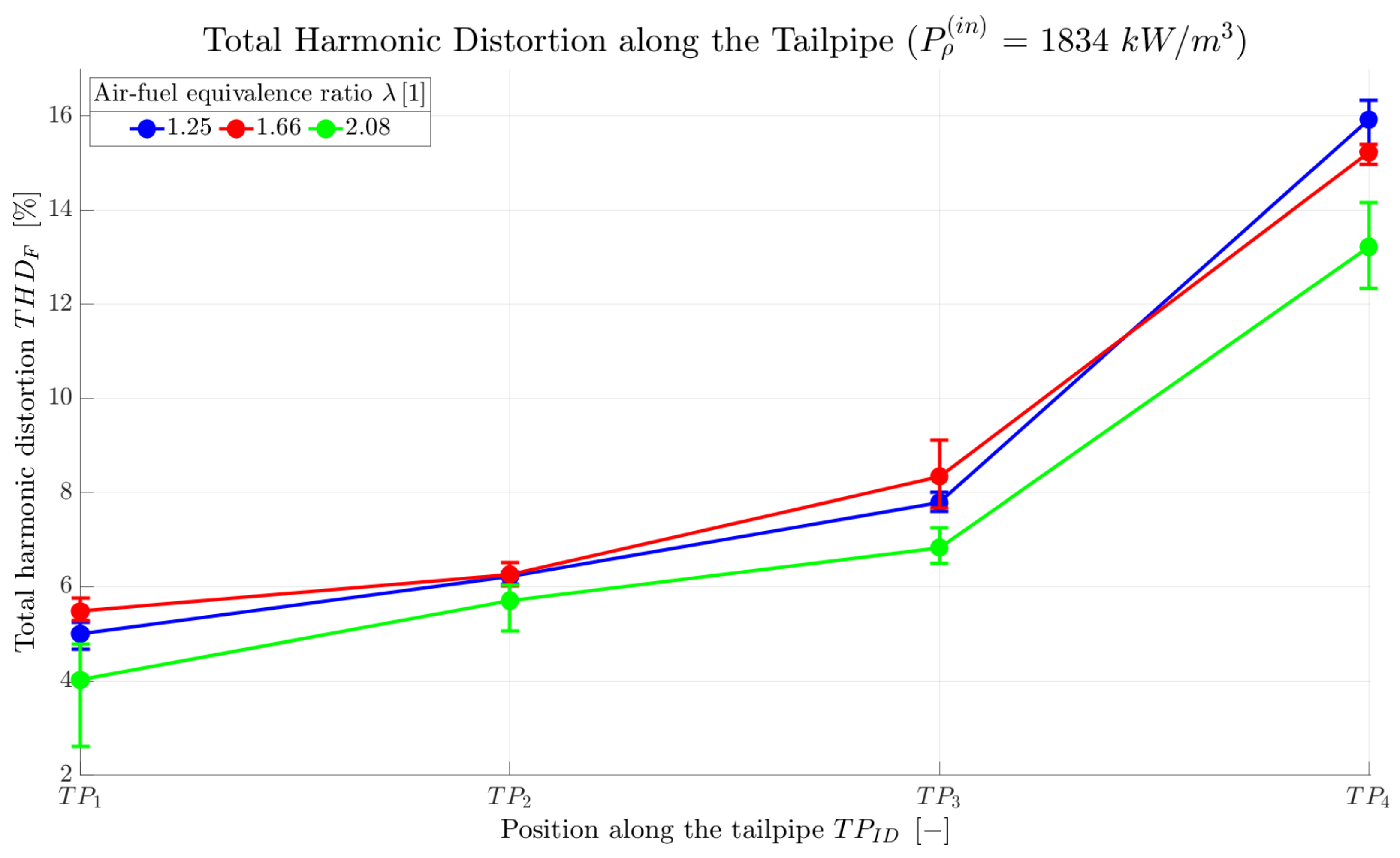
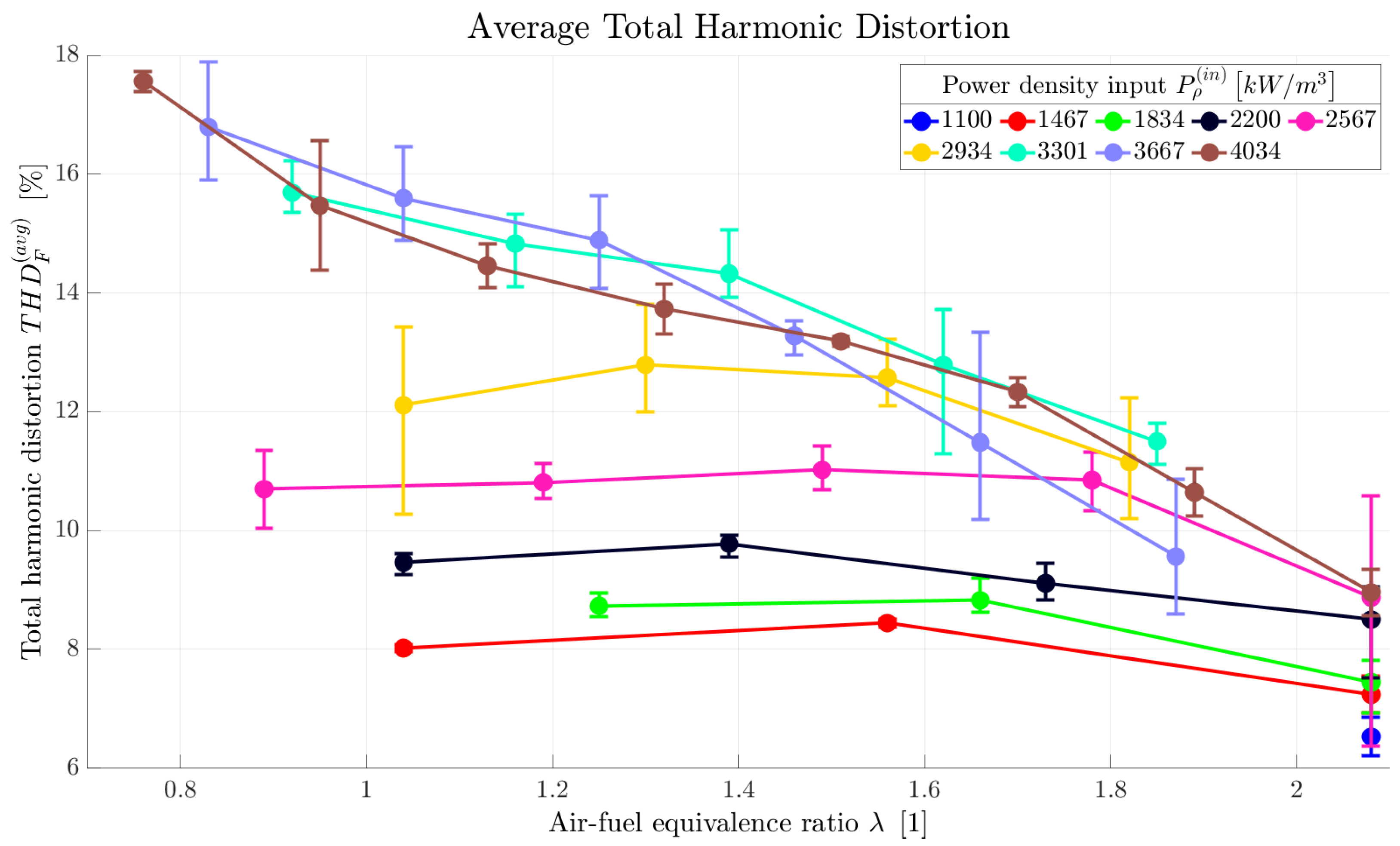
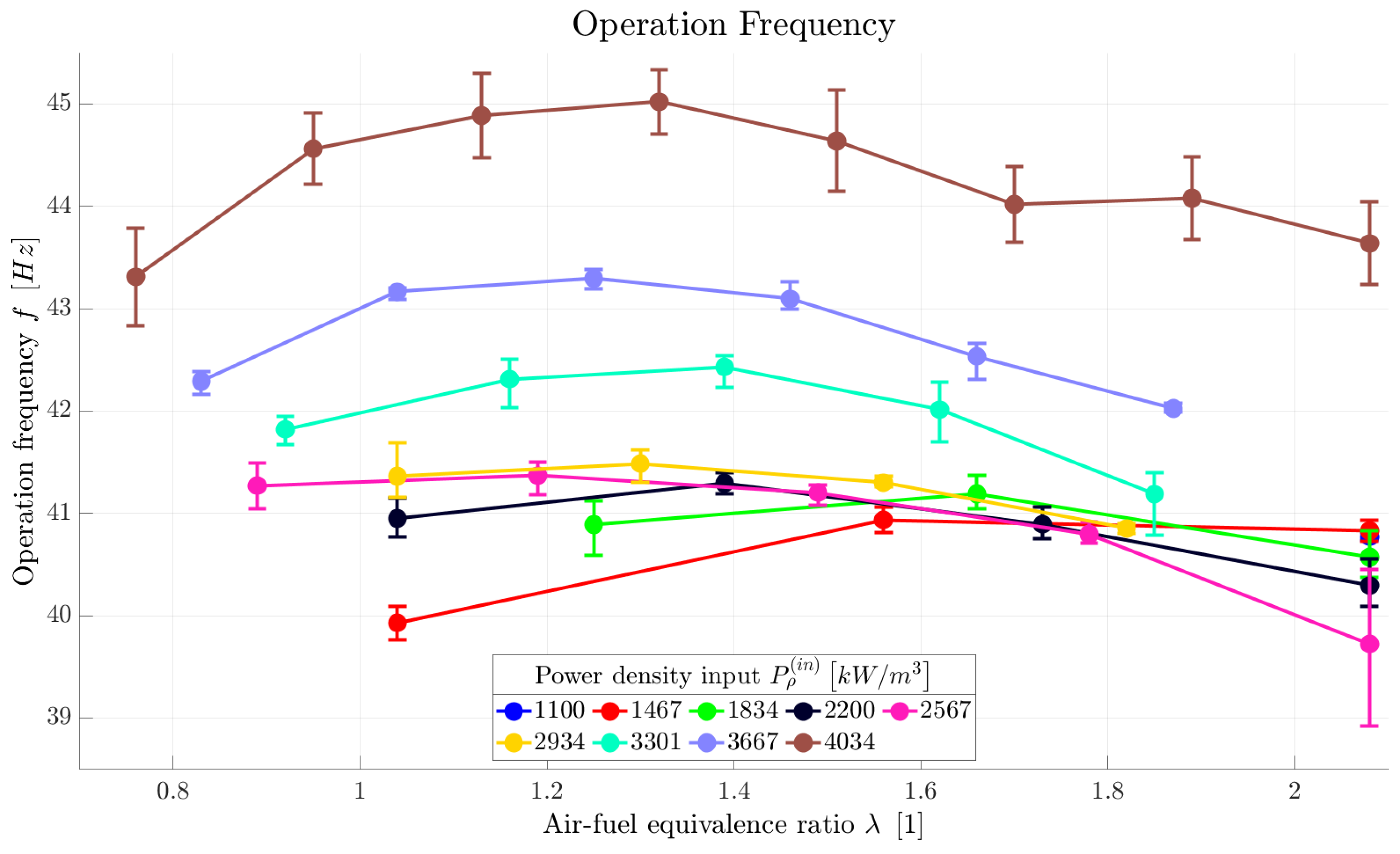
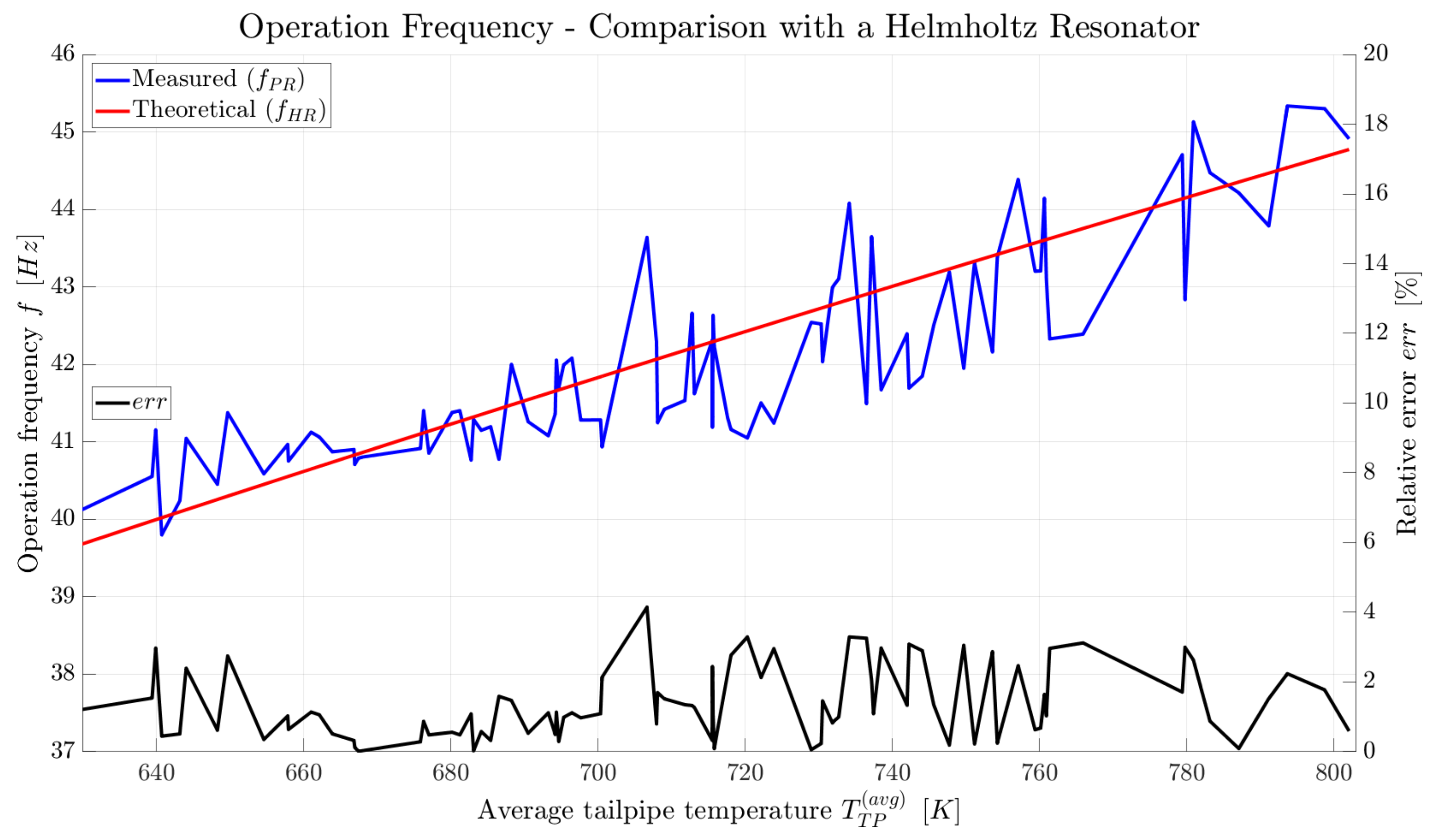
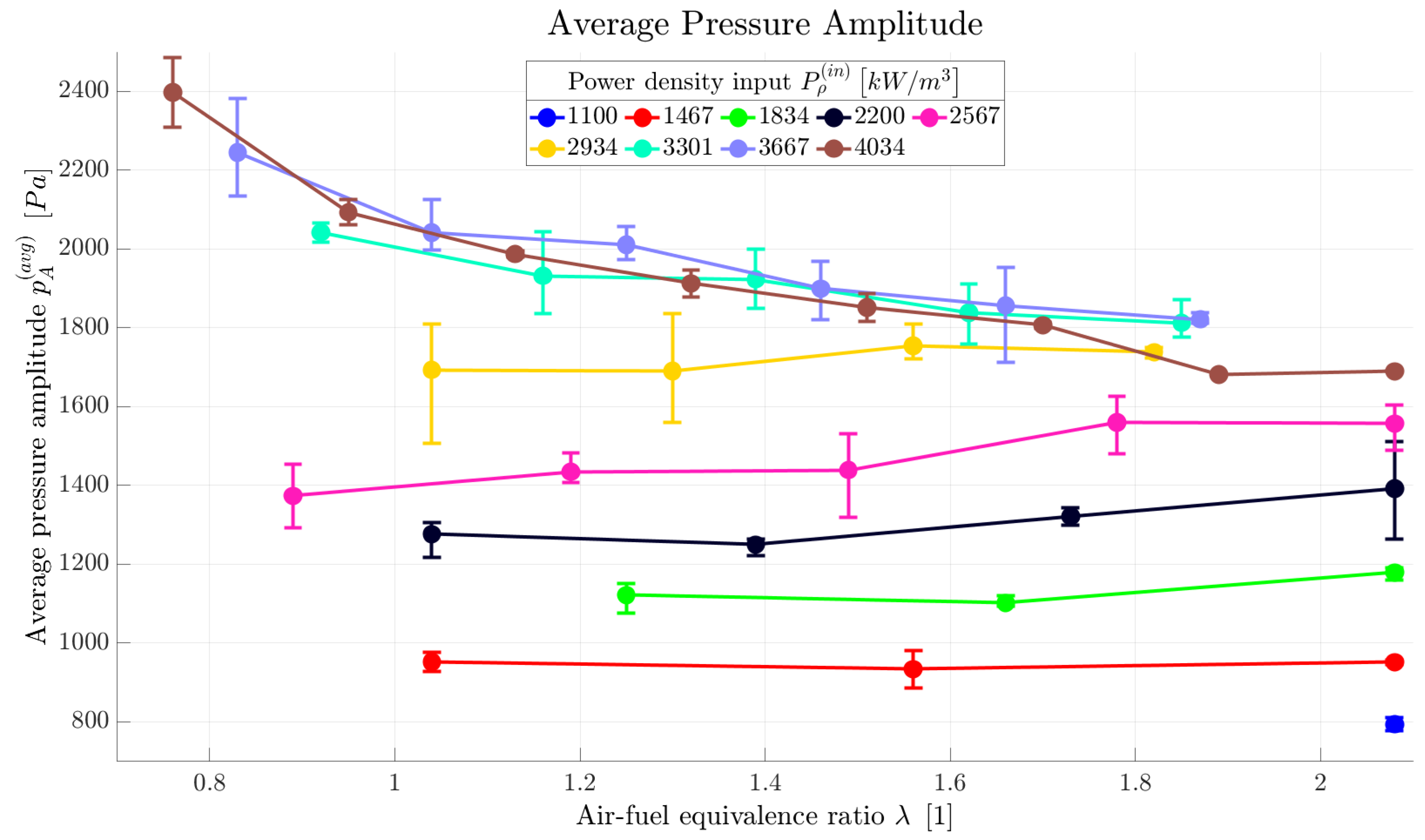
3.1. Pressure Data Results
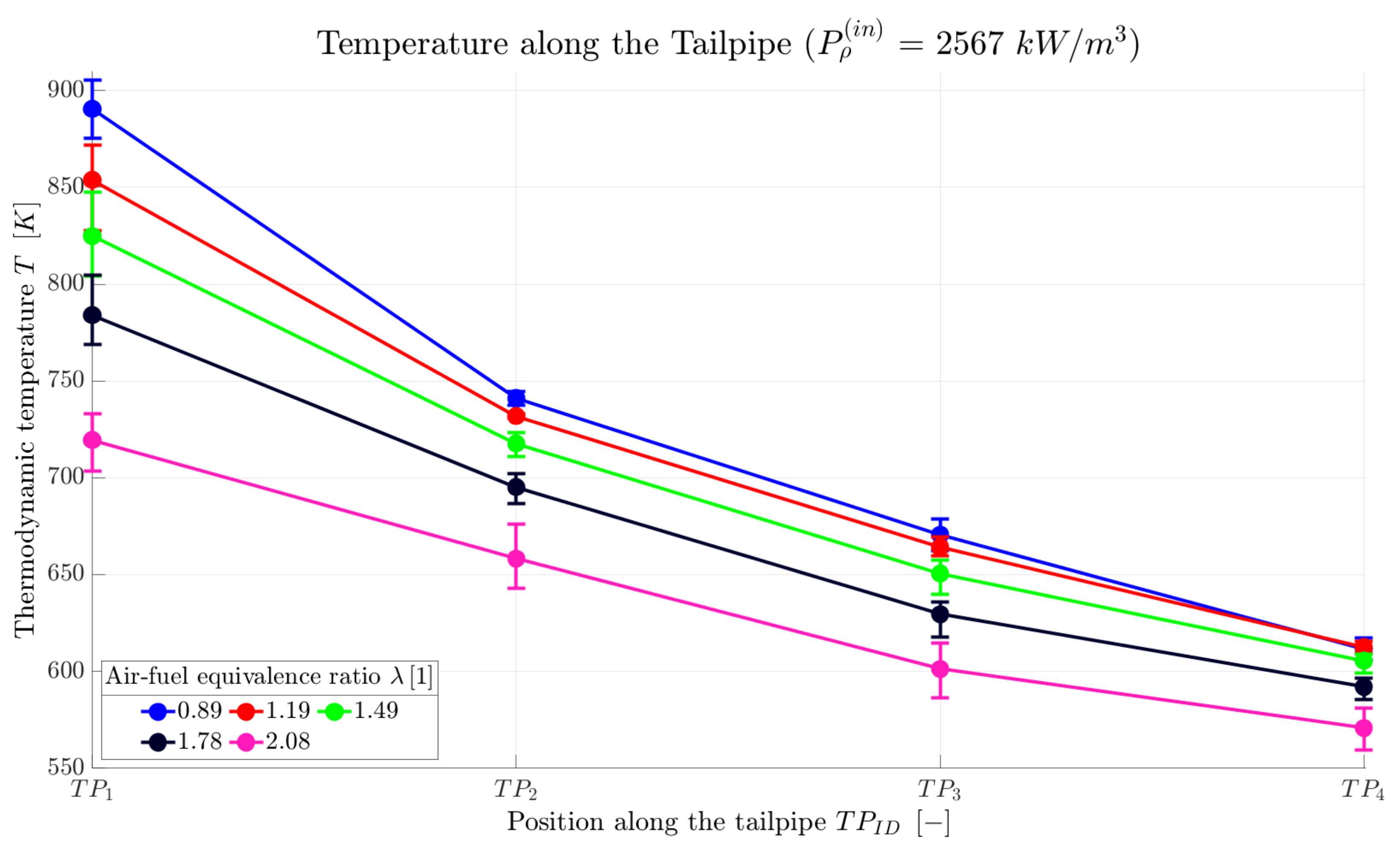
3.2. Temperature Data Results & the Energy Balance
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Arabic | |
| A | Area |
| c | Speed of sound |
| Heat capacity at constant pressure | |
| f | Frequency |
| Operation frequency of the pulsation reactor | |
| Sampling frequency of the pressure sensor | |
| Enthalpy of formation (mass-specific) | |
| Enthalpy flow change | |
| Chemical energy flow change | |
| L | Length |
| Mass flow rate | |
| M | Molar mass |
| p | Pressure |
| Power input | |
| Effective power input | |
| Power density input | |
| Heat loss | |
| r | Specific gas constant |
| t | Time |
| T | Period/thermodynamic temperature |
| Total harmonic distortion | |
| v | Velocity |
| V | Volume |
| Combustion chamber to the tailpipe volume ratio | |
| Normal flow rate | |
| Y | Mass fraction |
| Greek | |
| Heat capacity ratio | |
| Air-fuel equivalence ratio | |
| Density | |
| Mole fraction | |
| Indices | |
| Average | |
| A | Air/amplitude |
| Amplitude-to-mean value ratio | |
| G | Gas |
| Input | |
| M | Mean value |
Abbreviations
| Combustion chamber | |
| Combustion-driven oscillations | |
| Discrete Fourier transform | |
| Helmholtz resonator | |
| Operation point | |
| Particle Image Velocimetry | |
| Pulsation reactor | |
| Root mean square | |
| Tailpipe |
References
- Putnam, A.A.; Belles, F.E.; Kentfield, J.A.C. Pulse Combustion. Prog. Energy Combust. Sci. 1986, 12, 43–79. [Google Scholar] [CrossRef]
- Zinn, B.T. Pulse combustion: Recent applications and research issues. Symp. (Int.) Combust. 1992, 24, 1297–1305. [Google Scholar] [CrossRef]
- Plavnik, G. (Ed.) Pulse Combustion Technology. In Proceedings of the 14th Annual North American Waste-to-Energy Conference, North American Waste-to-Energy Conference, Tampa, FL, USA, 1–3 May 2006. [Google Scholar] [CrossRef]
- Klaus, C.; Wegner, K.; Ommer, M. (Eds.) Partikelsynthese im Pulsationsreaktor: Von der Idee zur Produktion. In Proceedings of the 30. Deutscher Flammentag, Hannover-Garbsen, Germany, 28–29 September 2021; pp. 28–29. [Google Scholar] [CrossRef]
- Hoffmann, C.; Ommer, M. Reaktoren für Fluid-Feststoff-Reaktionen: Pulsationsreaktoren. In Handbuch Chemische Reaktoren; Reschetilowski, W., Ed.; Springer Reference Naturwissenschaften; Springer: Berlin/Heidelberg, Germany, 2019; pp. 1–19. [Google Scholar] [CrossRef]
- Klaus, C.; Wegner, K.; Rammelt, T.; Ommer, M. New Challenges in Thermal Processing. Interceram-Int. Ceram. Rev. 2021, 70, 22–25. [Google Scholar] [CrossRef]
- Begand, S. Herstellung nanoskaliger Oxidpulver durch thermisch gesteuerte Synthese in einem Pulsationsreaktor. Ph.D. Thesis, Universität des Saarlandes, Saarbrücken, Germany, 2001. [Google Scholar]
- Heidinger, S.; Spranger, F.; Dostál, J.; Zhang, C.; Klaus, C. Material Treatment in the Pulsation Reactor—From Flame Spray Pyrolysis to Industrial Scale. Sustainability 2022, 14, 3232. [Google Scholar] [CrossRef]
- Mädler, L.; Kammler, H.K.; Mueller, R.; Pratsinis, S.E. Controlled synthesis of nanostructured particles by flame spray pyrolysis. J. Aerosol Sci. 2002, 33, 369–389. [Google Scholar] [CrossRef]
- Meierhofer, F.; Mädler, L.; Fritsching, U. Nanoparticle evolution in flame spray pyrolysis—Process design via experimental and computational analysis. AIChE J. 2020, 66, e16885. [Google Scholar] [CrossRef]
- Heidinger, S.; Unz, S.; Beckmann, M. Simple Particle Relaxation Modeling in One-Dimensional Oscillating Flows. Processes 2022, 10, 1322. [Google Scholar] [CrossRef]
- Heidinger, S.; Unz, S.; Beckmann, M. Heat and Mass Transfer to Particles in One-Dimensional Oscillating Flows. Processes 2023, 11, 173. [Google Scholar] [CrossRef]
- Dec, J.E.; Keller, J.O. Pulse Combustor Tail-Pipe Heat-Transfer Dependence on Frequency, Amplitude, and Mean Flow Rate. Combust. Flame 1989, 77, 359–374. [Google Scholar] [CrossRef]
- Xu, Y.; Dong, P.; Zhai, M.; Zhu, Q. (Eds.) Heat Transfer in Helmholtz-type Valveless Self-excited Pulse Combustor Tailpipe. In Proceedings of the 2012 Asia-Pacific Power and Energy Engineering Conference, Shanghai, China, 26–28 March 2012. [Google Scholar] [CrossRef]
- Dahm, B. A Contribution to Non-Steady Heat Transfer at Particles for Process Optimization of the Schmidt Tube. Ph.D. Thesis, Hochschule für Architektur und Bauwesen Weimar, Weimar, Germany, 1975. [Google Scholar]
- Rayleigh, L.; Strutt, J.W. The Explanation of Certain Acoustical Phenomena. Nature 1878, 18, 319–321. [Google Scholar] [CrossRef]
- Rayleigh, L.; Strutt, J.W. The Theory of Sound; Cambridge University Press: Cambridge, MA, USA, 2011. [Google Scholar] [CrossRef]
- Lieuwen, T.C.; Yang, V. Combustion Instabilities in Gas Turbine Engines: Operational Experience, Fundamental Mechanisms, and Modeling; American Institute of Aeronautics and Astronautics, Inc.: Reston, VA, USA, 2005. [Google Scholar] [CrossRef]
- Uemichi, A.; Kanetsuki, I.; Kaneko, S. Combustion Oscillation in Gas Turbine Combustor for Fuel Mixture of Hydrogen and Natural Gas. In Proceedings of the ASME 2017 Pressure Vessels and Piping Conference, Waikoloa, HI, USA, 16–20 July 2017. [Google Scholar] [CrossRef]
- Meng, X.; de Jong, W.; Kudra, T. A State-of-the-Art Review of Pulse Combustion: Principles, Modeling, Applications and R&D Issues. Renew. Sustain. Energy Rev. 2016, 55, 73–114. [Google Scholar]
- Zinn, B.T. A Theoretical Study of Non-Linear damping by helmholtz resonators. J. Sound Vib. 1970, 13, 347–356. [Google Scholar] [CrossRef]
- Tang, P.K.; Sirignano, W.A. Theory of a Generalized Helmholtz Resonator. J. Sound Vib. 1973, 26, 247–262. [Google Scholar] [CrossRef]
- Ahrens, F.W.; Kartsounes, G.T. Pulse Combustion Technology for Heating Applications: Quarterly Progress Report April-June 1978; Argonne National Lab.: Lemont, IL, USA, 1978. [Google Scholar]
- Panton, R.L.; Miller, J.M. Resonant Frequencies of Cylindrical Helmholtz Resonators. J. Acoust. Soc. Am. 1975, 57, 1533–1535. [Google Scholar] [CrossRef]
- Howe, M.S. On the Helmholtz Resonator. J. Sound Vib. 1976, 45, 427–440. [Google Scholar] [CrossRef]
- Großgebauer, S. Mathematische Modellierung und experimentelle Untersuchung von selbstständig pulsierenden Brennern zur Stoffbehandlung. Ph.D. Thesis, Technische Universität Dresden, Dresden, Germany, 2008. [Google Scholar]
- Zhonghua, W. Mathematical Modeling of Pulse Combustion and its Applications to Innovative Thermal Drying Techniques. Ph.D. Thesis, National University of Singapore, Singapore, 2007. [Google Scholar]
- Ha, M.Y.; Yavuzkurt, S. A Theoretical Investigation of Acoustic Enhancement of Heat and Mass Transfer-I. Pure Oscillating Flow. Int. J. Heat Mass Transf. 1993, 36, 2183–2192. [Google Scholar] [CrossRef]
- Ha, M.Y.; Yavuzkurt, S.; Kim, K.C. Heat Transfer Past Particles Entrained in an Oscillating Flow with and without a Steady Velocity. Int. J. Heat Mass Transf. 1993, 36, 949–959. [Google Scholar] [CrossRef]
- Alassar, R.S.; Badr, H.M.; Mavromatis, H.A. Heat Convection from a Sphere Placed in an Oscillating Free Stream. Int. J. Heat Mass Transf. 1999, 42, 1289–1304. [Google Scholar] [CrossRef]
- Xu, W.; Jiang, G.; An, L.; Liu, Y. Numerical and Experimental Study of Acoustically Enhanced Heat Transfer from a Single Particle in Flue Gas. Combust. Sci. Technol. 2018, 190, 1158–1177. [Google Scholar] [CrossRef]
- PCB Piezotronics. Probe Microphone ICP Model 377B26: Installation and Operating Manual. 2013. Available online: https://www.pcb.com/products?m=377b26 (accessed on 7 September 2022).
- Mondal, S.; Mukhopadhyay, A.; Sen, S. Dynamic Characterization of a Laboratory-Scale Pulse Combustor. Combust. Sci. Technol. 2014, 186, 139–152. [Google Scholar] [CrossRef]
- Faramawy, S.; Zaki, T.; Sakr, A.E. Natural Gas Origin, Composition, and Processing: A review. J. Nat. Gas Sci. Eng. 2016, 34, 34–54. [Google Scholar] [CrossRef]
- Engineering ToolBox. Air-Composition and Molecular Weight. 2003. Available online: https://www.engineeringtoolbox.com/air-composition-d_212.html (accessed on 19 September 2022).
- Kinsler, L.E.; Frey, A.R.; Coppens, A.B.; Sanders, J.V. Fundamentals of Acoustics, 4th ed.; John Wiley & Sons, Inc.: New York, NY, USA, 2000. [Google Scholar]
- McBride, B.J.; Heimel, S.; Ehlers, J.G.; Gordon, S. Thermodynamic Properties to 6000 K for 210 Substances Involving The First 18 Elements; National Aeronautics and Space Administration: Washington, DC, USA, 1963.
- Keller, J.O.; Bramlette, T.T.; Dec, J.E.; Westbrook, C.K. Pulse Combustion: The Importance of Characteristic Times. Combust. Flame 1989, 75, 33–44. [Google Scholar] [CrossRef]
- Keller, J.O.; Bramlette, T.T.; Westbrook, C.K.; Dec, J.E. Pulse Combustion: The Quantification of Characteristic Times. Combust. Flame 1990, 79, 151–161. [Google Scholar] [CrossRef]
- Hongo, I.; Saito, K. Development of Small Twin-Valveless Pulse Combustors: Effect of Injection System. Combust. Sci. Technol. 1993, 94, 43–55. [Google Scholar] [CrossRef]
- Offord, T.; Miller, R.; Dawson, J.; Heffer, J.; Mason, S.; Taylor, M. (Eds.) Improving the Performance of a Valveless Pulse Combustor Using Unsteady Fuel Injection; American Institute of Aeronautics and Astronautics, Inc.: Reston, VA, USA, 2008. [Google Scholar] [CrossRef]
- Xu, Y.; Zhai, M.; Dong, P.; Wang, F. (Eds.) Experimental Research on Operational Characteristics of a Helmholtz-Type ValvelessSelf-Excited Pulse Combustor Self-excited Pulse Combustor. In Proceedings of the 2011 Asia-Pacific Power and Energy Engineering Conference, Wuhan, China, 28–28 March 2011; IEEE: Piscataway, NJ, USA, 2011. [Google Scholar] [CrossRef]








| [Nm3/h] | 0.75 | 1.00 | 1.25 | 1.50 | 1.75 | 2.00 | 2.25 | 2.50 | 2.75 | |
|---|---|---|---|---|---|---|---|---|---|---|
| [Nm3/h] | ||||||||||
| 10 | - | 1.04 | - | - | - | - | - | - | - | |
| 15 | 2.08 | 1.56 | 1.25 | 1.04 | 0.89 | - | - | - | - | |
| 20 | - | 2.08 | 1.66 | 1.39 | 1.19 | 1.04 | 0.92 | 0.83 | 0.76 | |
| 25 | - | - | 2.08 | 1.73 | 1.49 | 1.30 | 1.16 | 1.04 | 0.95 | |
| 30 | - | - | - | 2.08 | 1.78 | 1.56 | 1.39 | 1.25 | 1.13 | |
| 35 | - | - | - | - | 2.08 | 1.82 | 1.62 | 1.46 | 1.32 | |
| 40 | - | - | - | - | - | - | 1.85 | 1.66 | 1.51 | |
| 45 | - | - | - | - | - | - | - | 1.87 | 1.70 | |
| 50 | - | - | - | - | - | - | - | - | 1.89 | |
| 55 | - | - | - | - | - | - | - | - | 2.08 | |
| [kW/m3] | 1100 | 1467 | 1834 | 2200 | 2567 | 2934 | 3301 | 3667 | 4034 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dostál, J.; Heidinger, S.; Klaus, C.; Unz, S.; Beckmann, M. Effects of Fuel Input on Pulsation Reactor Behavior—An Experimental Study. Processes 2023, 11, 444. https://doi.org/10.3390/pr11020444
Dostál J, Heidinger S, Klaus C, Unz S, Beckmann M. Effects of Fuel Input on Pulsation Reactor Behavior—An Experimental Study. Processes. 2023; 11(2):444. https://doi.org/10.3390/pr11020444
Chicago/Turabian StyleDostál, Jakub, Stefan Heidinger, Christian Klaus, Simon Unz, and Michael Beckmann. 2023. "Effects of Fuel Input on Pulsation Reactor Behavior—An Experimental Study" Processes 11, no. 2: 444. https://doi.org/10.3390/pr11020444
APA StyleDostál, J., Heidinger, S., Klaus, C., Unz, S., & Beckmann, M. (2023). Effects of Fuel Input on Pulsation Reactor Behavior—An Experimental Study. Processes, 11(2), 444. https://doi.org/10.3390/pr11020444






