Statistical Inference for the Beta Coefficient
Abstract
1. Introduction
2. Estimated Beta Coefficient and Its Distributional Properties
2.1. Sample Distribution of the Estimated Beta Coefficient
2.2. Interval Estimation and Test Theory
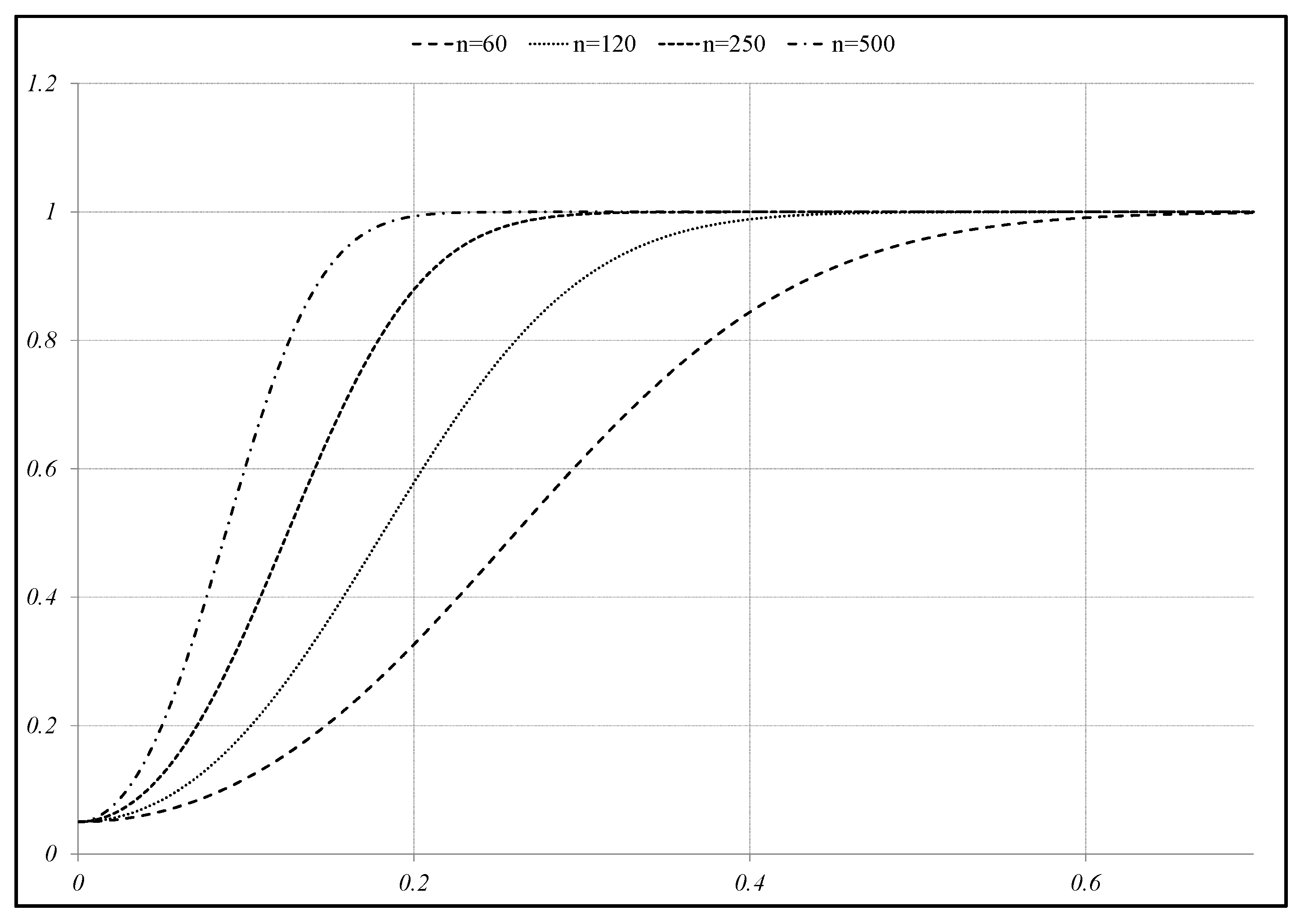
3. Empirical Illustration
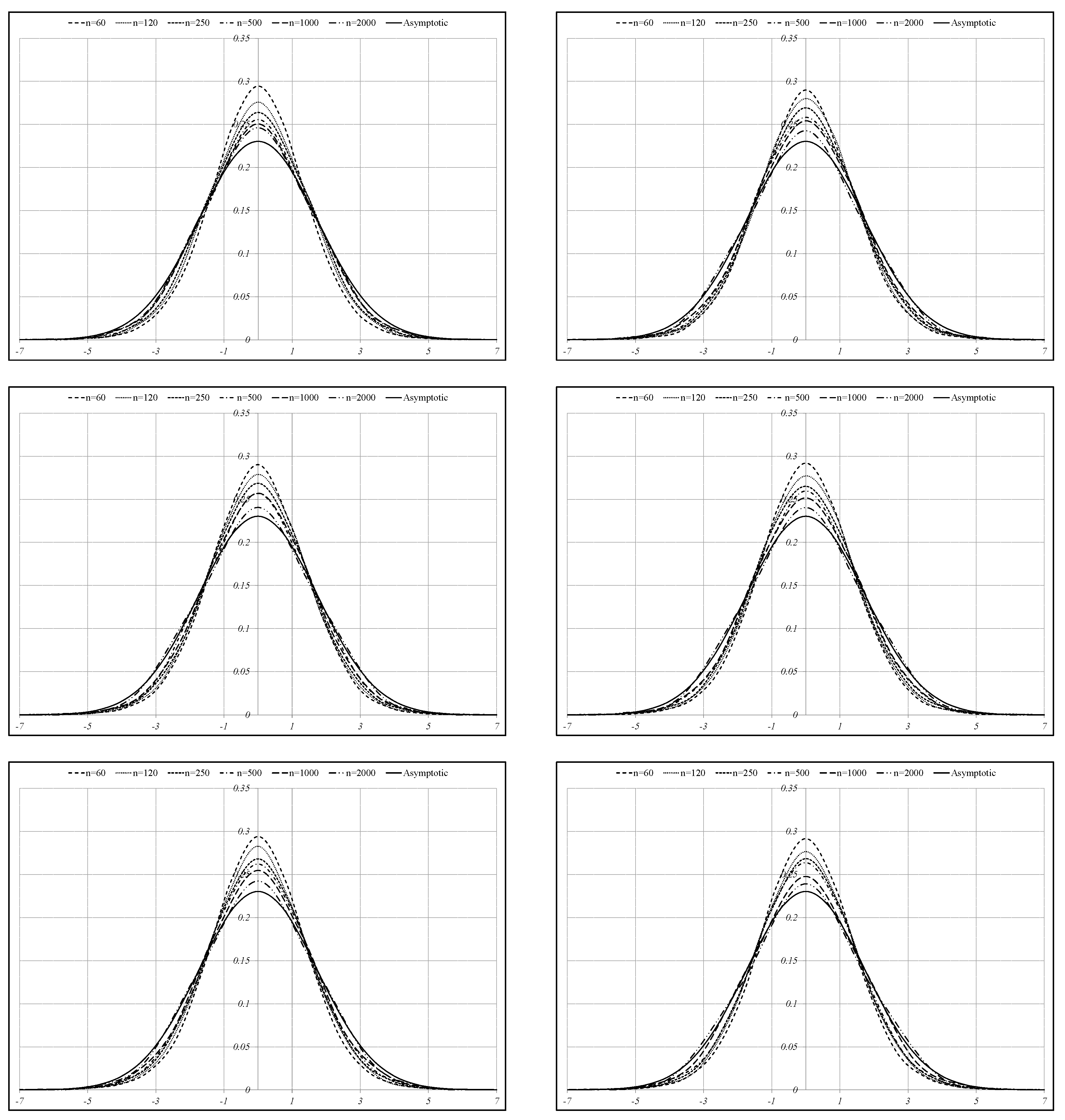
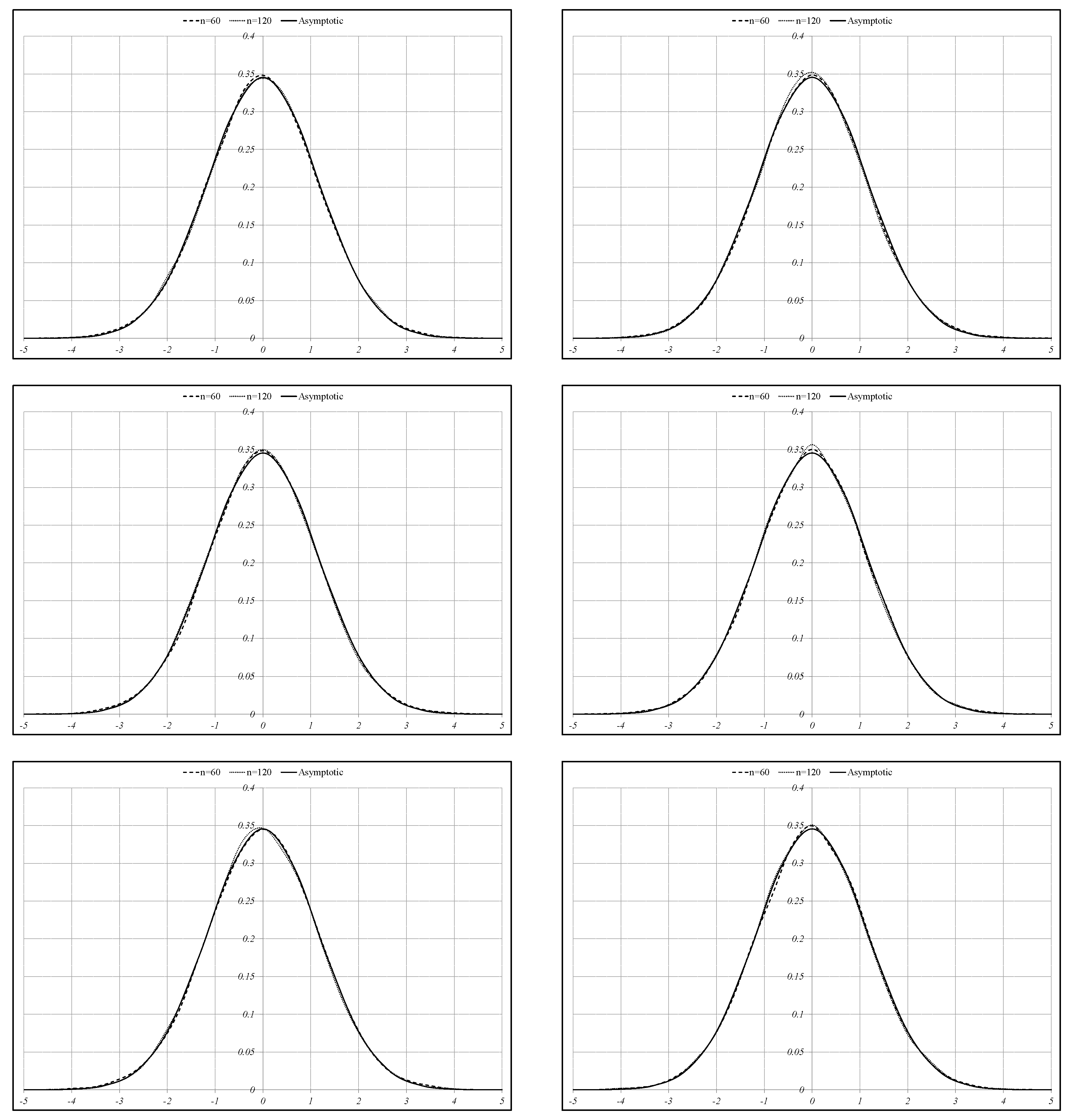
4. Robustness to the Violation of the Normality Assumption
5. Summary
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Aitchison, John. 1964. Confidence-region tests. Journal of the Royal Statistical Society: Series B (Methodological) 26: 462–76. [Google Scholar] [CrossRef]
- Alexander, Carol. 2001. Market Models: A Guide to Financial Data Analysis. Hoboken: John Wiley & Sons. [Google Scholar]
- Alexander, Gordon J., and Alexandre M. Baptista. 2002. Economic implications of using a mean-var model for portfolio selection: A comparison with mean-variance analysis. Journal of Economic Dynamics and Control 26: 1159–93. [Google Scholar] [CrossRef]
- Alexander, Gordon J., and Alexandre M. Baptista. 2004. A comparison of var and cvar constraints on portfolio selection with the mean-variance model. Management Science 50: 1261–73. [Google Scholar] [CrossRef]
- Bauder, David, Rostyslav Bodnar, Taras Bodnar, and Wolfgang Schmid. 2019. Bayesian estimation of the efficient frontier. Scandinavian Journal of Statistics. forthcoming. [Google Scholar] [CrossRef]
- Berk, Jonathan B. 1997. Necessary conditions for the capm. Journal of Economic Theory 73: 245–57. [Google Scholar] [CrossRef][Green Version]
- Bodnar, Taras, Wolfgang Schmid, and Tara Zabolotskyy. 2012. Minimum var and minimum cvar optimal portfolios: Estimators, confidence regions, and tests. Statistics & Risk Modeling with Applications in Finance and Insurance 29: 281–314. [Google Scholar]
- Bodnar, Taras, and Wolfgang Schmid. 2009. Econometrical analysis of the sample efficient frontier. The European Journal of Finance 15: 317–35. [Google Scholar] [CrossRef]
- Bodnar, Taras, Nestor Parolya, and Wolfgang Schmid. 2018. Estimation of the global minimum variance portfolio in high dimensions. European Journal of Operational Research 266: 371–90. [Google Scholar] [CrossRef]
- Damodaran, Aswath. 2012. Investment Valuation: Tools and Techniques for Determining the Value of any Asset. Hoboken: John Wiley & Sons. [Google Scholar]
- Dhrymes, Phoebus. 2017. Introductory Economerics. Berlin: Springer. [Google Scholar]
- Frahm, Gabriel, and Christoph Memmel. 2010. Dominating estimators for minimum-variance portfolios. Journal of Econometrics 159: 289–302. [Google Scholar] [CrossRef]
- Gibbons, Michael R., Stephen A. Ross, and Jay Shanken. 1989. A test of the efficiency of a given portfolio. Econometrica 57: 1121–52. [Google Scholar] [CrossRef]
- Greene, William H. 2003. Econometric Analysis. Chennai: Pearson Education India. [Google Scholar]
- Gupta, Arjun K., Tamas Varga, and Taras Bodnar. 2013. Elliptically Contoured Models in Statistics and Portfolio Theory. New York: Springer. [Google Scholar]
- Hodgson, Douglas J., Oliver Linton, and Keith Vorkink. 2002. Testing the capital asset pricing model efficiently under elliptical symmetry: A semiparametric approach. Journal of Applied Econometrics 17: 617–39. [Google Scholar] [CrossRef]
- Lintner, John. 1965. The valuation of risk assets and the selection of risky investments in stock portfolios and capital budgets. The Review of Economics and Statistics 47: 13–37. [Google Scholar] [CrossRef]
- Markowitz, Harry. 1952. Portfolio selection. The Journal of Finance 7: 77–91. [Google Scholar]
- Merton, Robert C. 1972. An analytic derivation of the efficient portfolio frontier. Journal of Financial and Quantitative Analysis 7: 1851–72. [Google Scholar] [CrossRef]
- Mossin, Jan. 1966. Equilibrium in a capital asset market. Econometrica: Journal of the Econometric Society 34: 768–83. [Google Scholar] [CrossRef]
- Muirhead, Robb J. 1982. Aspects of Multivariate Statistical Theory. New York: Wiley. [Google Scholar]
- Ross, Stephen A. 1976. The arbitrage theory of capital asset pricing. Journal of Economic Theory 13: 341–60. [Google Scholar] [CrossRef]
- Schmid, Wolfgang, and Taras Zabolotskyy. 2008. On the existence of unbiased estimators for the portfolio weights obtained by maximizing the sharpe ratio. AStA Advances in Statistical Analysis 92: 29–34. [Google Scholar] [CrossRef]
- Sharpe, William F. 1964. Capital asset prices: A theory of market equilibrium under conditions of risk. The Journal of Finance 19: 425–42. [Google Scholar]
- Sharpe, William F. 1994. The sharpe ratio. Journal of Portfolio Management 21: 49–58. [Google Scholar] [CrossRef]
- Zhou, Guofu. 1993. Asset-pricing tests under alternative distributions. The Journal of Finance 48: 1927–42. [Google Scholar] [CrossRef]

© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bodnar, T.; Gupta, A.K.; Vitlinskyi, V.; Zabolotskyy, T. Statistical Inference for the Beta Coefficient. Risks 2019, 7, 56. https://doi.org/10.3390/risks7020056
Bodnar T, Gupta AK, Vitlinskyi V, Zabolotskyy T. Statistical Inference for the Beta Coefficient. Risks. 2019; 7(2):56. https://doi.org/10.3390/risks7020056
Chicago/Turabian StyleBodnar, Taras, Arjun K. Gupta, Valdemar Vitlinskyi, and Taras Zabolotskyy. 2019. "Statistical Inference for the Beta Coefficient" Risks 7, no. 2: 56. https://doi.org/10.3390/risks7020056
APA StyleBodnar, T., Gupta, A. K., Vitlinskyi, V., & Zabolotskyy, T. (2019). Statistical Inference for the Beta Coefficient. Risks, 7(2), 56. https://doi.org/10.3390/risks7020056




