On the Optimal Risk Sharing in Reinsurance with Random Recovery Rate

Abstract

This paper studies a Pareto-optimal reinsurance contract in the presence of negative statistical dependence between the insurance claim and the random recovery rate. In the context of symmetric information model and asymmetric information model, we investigate properties of the Pareto-optimal indemnity schedules. For risk neutral reinsurer with proportional cost and associated expense, we present possible forms of the Pareto-optimal indemnity schedule as well.

1. Introduction

In reinsurance market, due to the conflict between the interest of the insurer and that of the reinsurer, it is impossible to build an optimal reinsurance contract simultaneously maximizing the interest of both parties. However, one can resort to a Pareto-optimal reinsurance contract, under which there is no other contract making one party better off without worsening the other. Among the first Borch (1960) studied the Pareto-optimal contracts in insurance market and derived optimal retentions of the quota-share and stop-loss reinsurance by maximizing the product of the expected utility of the insurer’s and reinsurer’s terminal wealth. For the insurer with cost consisting with the additive fixed component and the variable one, a necessary and sufficient condition for the Pareto-optimal deductible to be zero in complete insurance market was presented in Raviv (1979) for risk averse insurer. Afterward, Aase (2002) investigated a competitive equilibrium, Pareto optimality, and representative agent pricing. At the meantime, Golubin (2006) studied the problem of designing Pareto-optimal insurance indemnity functions for risk averse insurer and insured when the premium is based on the actuarial value of the insurer’s risk. Recently, Jiang et al. (2017) and Cai et al. (2016) solved the Pareto-optimal reinsurance when the insurer and reinsurer both measure the risk by using Value-at-Risk. Along this line, Lo and Tang (2018) investigated the problem by using the Neyman–Pearson approach and Cai et al. (2017) solved the problem under the frame of Tail-Value-at-Risk. Lately, Jiang et al. (2018) studied the Pareto-optimal reinsurance with constraints under distortion risk measures.

It was pointed out in Doherty and Schlesinger (1983) that typically some risky assets of the individual wealth are often insurable while the others (i.e., market valuation of stocks, inflation, and general economic conditions etc.) are usually not. In particular, the association between insurable risks and uninsurable ones may play a part in the optimal level of insurance coverage. As was remarked also in Gollier (1996), insurers prefer to covering risks of different sources by different contracts, and some risks impacting the final wealth of the agent may not be insured, and the uninsurable risk is actually a kind of background risk. As a consequence, it is of interest to revisit the insurance problems in the context of the background risk. In the context of some dependence structures for the insurable and uninsurable risks, Dana and Scarsini (2007) investigated qualitative properties of Pareto-optimal insurance contracts.

Besides the additive one, the multiplicative background risk is quite common in the insurance practice. The counter-party risk in a reinsurance contract, which means the little chance that the reinsurer fails to pay the entire promised benefit to the insurer, is the default risk of the reinsurer. Readers may refer to Franke et al. (2006) for a comprehensive study on the multiplicative background risk. In the existing literature, researchers studied various characterizations of the counter-party risk together with its impact on the design of optimal reinsurance contract. For example, Tapiero et al. (1986) employed the perceived probability of ruin to characterize the probability of the insurer being affected by the reinsurer’s default risk, and Schlesinger and Schulenburg (1987) considered a three-state model in which a loss that can not be indemnified occurs with a nonnegative probability, and discussed how insurance purchases are affected by the insurer’s level of risk aversion. In the four-state model of Doherty and Schlesinger (1990), both the reinsurer and the insurer know the default probability, and the partial insurance is proved to be optimal when the reinsurer has a positive probability of total default. Later, such a result was extended to partial default by Mahul and Wright (2007). On the other hand, Cummins and Mahul (2003) derived the optimal insurance in the context that the reinsurer has a positive probability of total default and the reinsurer and insurer have divergent beliefs about this probability. Recently, Bernard and Ludkovski (2012) considered loss-dependent probability of default and partial recovery in the event of contract non-performance, and studied the Pareto-optimal reinsurance contracts with counter-party risk for risk neutral reinsurer and risk averse insurer. Thus, Bernard and Ludkovski (2012) extended the model of Dana and Scarsini (2007) to the case of multiplicative but not additive background risk.

Usually, the background risk has significant impact on the Pareto-optimal reinsurance contract, and as is pointed out in Franke et al. (2006) and Bernard and Ludkovski (2012), the presence of multiplicative background risk can be more complex than the additive risk. On the other hand, in the presence of multiplicative background risk, Bernard and Ludkovski (2012) only dealt with binary recovery rate and risk neutral insurer with linear cost. In this study, we consider the reinsurance with counter-party risk under the following framework.

(i)

For the random insurance claim $X_1$ with support $[0,\hslash ]$, by signing a reinsurance contract the insurer pays the reinsurance premium p to the reinsurer and thus transfers a portion $r(X_1)$ to the reinsurer. Intuitively, the ceded loss should be nonnegative and never exceed the initial risk, i.e., the indemnity schedule $r(x)\in [0,x]$ for all $x\in [0,\hslash ]$. Correspondingly, the insurer gets the retained loss function $\overline{r}(x)=x-r(x)$ for all $x\in [0,\hslash ]$. Owing to the no rip-off principle of premium, we assume that $p\le \hslash$.

(ii)

For the random recovery rate $X_2\in [0,1]$, the reinsurer undertakes the loss $X_{2}r(X_1)$. Specifically, there is no counter-party risk when $X_2\equiv 1$.

(iii)

Denote $u(x)$ and $v(x)$, both increasing and continuously differentiable, utilities of the insurer and reinsurer, respectively. Assume that $u(x)$ is strictly concave and $v(x)$ is concave. Let $u(-\infty )=-\infty$ and $v(0)=0$, i.e., the reinsurer gets 0 utility whenever the profit is 0.

(iv)

The reinsurer has the cost function $c(x):[0,\hslash ]\mapsto [0,\infty )$, which is increasing, convex and continuously differentiable, and the corresponding associated expense $c(x)-x\ge 0$ for all $x\in [0,\hslash ]$. Since nonreinsurance payment incurs no associated expense we assume $c(0)=0$ and $c^{\prime }(0)\ge 1$.

(v)

With the initial wealth $\omega \ge 0$ the insurer attains the final wealth $\omega -X_1+X_{2}r(X_1)-p$, and the reinsurer gets the profit $p-c(X_{2}r(X_1))$.

When the reinsurer and insurer share a common view about the default risk, one has the so-called symmetric information model, under which we consider the Pareto-optimal problem:

$$\left\{\begin{array}{c}\underset{(p,r)}{max}\mathsf{E}[u(\omega -X_1+X_{2}r(X_1)-p)]\hfill \\ \mathrm{s}.\mathrm{t}.0\le r(x)\le x\mathrm{and}\mathsf{E}[v(p-c(X_{2}r(X_1)))]\ge 0.\hfill \end{array}\right.$$

(1)

Without loss of generality, one may always assume1 that the reinsurer’s initial wealth 0 and the utility function v such that $v(0)=0$. As per Bernard and Ludkovski (2012), although the insurer and reinsurer have the common belief on the default risk of reinsurer, it is commonly accepted that the reinsurer is more optimistic than the insurer about his or her own default risk. In actual, the insurer often believes that the reinsurer underestimates the likelihood of nonperformance. In the extreme case, the reinsurer ignores the default (background) risk in calculating his or her expected return, and this gives rise to the asymmetric information model, under which we consider the Pareto-optimal problem:

$$\left\{\begin{array}{c}\underset{(p,r)}{max}\mathsf{E}[u(\omega -X_1+X_{2}r(X_1)-p)]\hfill \\ \mathrm{s}.\mathrm{t}.0\le r(x)\le x\mathrm{and}\mathsf{E}[v(p-c(r(X_1)))]\ge 0.\hfill \end{array}\right.$$

(2)

In this paper, we will discuss the existence and uniqueness of the Pareto-optimal reinsurance contracts for Problems (1) and (2). Note that the shape of the optimal reinsurance contract may crucially depends on the statistical dependence between the random insurance claim and recovery rate, which can be either independent or negatively dependent because a larger insurance claim usually leads to higher default risk. Due to the mathematical tractability and practical interest, we will present possible structures of the optimal reinsurance contracts for Problems (1) and (2) in the presence of statistical independence or stochastic monotonicity between insurance claim and recovery rate, and this will provide theoretical support for the insurer to come up with the Pareto-optimal reinsurance contracts in practice.

The remaining part of this manuscript is rolled out as follows: In Section 2, we recall some concerned notions, including definitions of rearrangement and supermodularity etc., and introduce several technical lemmas. Section 3 presents qualitative properties of the Pareto-optimal indemnity schedule for symmetric information model in the context of independence between the insurance claim and the recovery rate. For the recovery rate independent of and stochastically decreasing in the insurance claim, we investigate properties of the Pareto-optimal indemnity schedules for asymmetric information model in Section 4 and Section 5, respectively. Section 6 concludes this study by making some remarks. To be coherent, all proofs of main results are deferred to the Appendix A, Appendix B, Appendix C, Appendix D, Appendix E, Appendix F, Appendix G, Appendix H, Appendix I, Appendix J, Appendix K and Appendix L.

2. Some Preliminaries

Before proceeding to the main sections, let us recall one important technical lemma on supermodularity and two useful theoretical results on the conditional expectation concerning the utility of the insurer.

A function $\tilde{r}(x)$ is a rearrangement of $r(x)$ with respect to a random variable X if $\tilde{r}(X)$ and $r(X)$ have the same distribution. For more please refer to Hardy et al. (1988). Also, a function $\varphi$ defined on a lattice2 $\mathcal{L}$ is said to be supermodular if $\varphi (x_1,y_1)+\varphi (x_2,y_2)\ge \varphi (x_1,y_2)+\varphi (x_2,y_1)$ for all $(x_1,y_2),(x_2,y_1)\in \mathcal{L}$ such that $x_1\le x_2$ and $y_1\le y_2$. For more on supermodularity one may refer to Marshall et al. (2011). The following lemma is useful in deriving our main results in the sequel.

Lemma 1

(Dana and Scarsini 2007, Lemma 3.4). If $\tilde{r}(x)$ is a nondecreasing rearrangement of $r(x)$ with respect to X, a bounded random variable with continuous distribution, then,

$$\mathsf{E}[\varphi (\tilde{r}(X),X)]\ge \mathsf{E}[\varphi (r(X),X)],foranysupermodularfunction\varphi .$$

For an utility function u, the risk aversion coefficient ${\ell }_u(x)=-\frac{u^{″}(x)}{u^{\prime }(x)}$ measures the degree of risk aversion: the larger the coefficient the more risk averse the utility is, and thus the more premium will the investor be willing to pay for the same risk. For more details, please refer to Kaas et al. (2008). Also recall that a random variable $X_2$ is said to be stochastically decreasing in $X_1$, denoted by $X_2{\downarrow }_{\mathrm{st}}{X}_1$, if $\mathsf{E}[f(X_2)\mid X_1=x]$ is nonincreasing in x for every nondecreasing function f, for which expectations exist. Such a stochastic monotonicity is suitable for modeling the statistical dependence between the insured risk and the recovery rate.

Next, we present two technical lemmas on monotonicity and supermodularity concerned with the recovery rate stochastically decreasing in the insured risk, which will be employed to build the important results in the sequel.

Lemma 2.

If $X_2{\downarrow }_{\mathrm{st}}{X}_1$ and

$$ty{\ell }_u(\omega -x+ty-p)\le 1,foranyt\in [0,1]and0\le y\le x\le \hslash ,$$

(3)

then both $\mathsf{E}[X_2{u}^{\prime }(\omega -(1-X_2)x-X_{2}y-p)\mid X_1=x]$ and $\mathsf{E}[X_2{u}^{\prime }(\omega -x+X_{2}y-p)\mid X_1=x]$ are non-increasing in $x\in [y,+\infty )$ for any $0\le y\le x$.

One can easily verified that $u_1(x)={(x+2\hslash )}^c$ for $0<c<1$, $u_2(x)=log(x+2\hslash )$ and exponential utility function $u_3(x)=-e^{-\beta x}$ for $\beta \le {\hslash }^{-1}$ all fulfill (3) in Lemma 2.

Lemma 3.

If $X_2{\downarrow }_{\mathrm{st}}{X}_1$ and (3) holds, then,

$$\psi (x,y)=\mathsf{E}[u(\omega -(1-X_2)x-X_{2}y-p)\mid X_1=x]$$

is supermodular in $\{(x,y):0\le y\le x\le \hslash \}$.

As per Dana and Scarsini (2007), a reinsurance contract is said to have disappearing deductible if the indemnity function $r(x)$ is nondecreasing, $r(x)=0$ for $x\in [0,a]$ with some $a\in [0,\hslash ]$ and $\overline{r}(x)$ is nonincreasing on $[a,\hslash ]$. Moreover, it is called a full reinsurance contract if $r(x)=x$ for all $x\in [0,\hslash ]$, and a nonreinsurance contract if $r(x)=0$ for all $x\in [0,\hslash ]$.

3. Symmetric Information Model

Let $(p^{*},r^{*})$ be a Pareto-optimal contract of Problem (1). Assume $\mathsf{E}[v(p^{*}-c(X_2{r}^{*}(X_1)))]>0$. Due to increasing $v(x)$ with $v(0)=0$, it holds that

$$\mathsf{E}[v(-c(X_2{r}^{*}(X_1)))]\le 0<\mathsf{E}[v(p^{*}-c(X_2{r}^{*}(X_1)))].$$

Since v is increasing and concave, v is continuous. Thus, there exists $p_1^{*}\in [0,p^{*})$ such that $\mathsf{E}[v(p_1^{*}-c(X_2{r}^{*}(X_1)))]=0$. Also, since u is increasing, we have

$$\mathsf{E}[u(\omega -X_1+X_2{r}^{*}(X_1)-p^{*})]<\mathsf{E}[u(\omega -X_1+X_2{r}^{*}(X_1)-p_1^{*})],$$

and this contradicts the Pareto-optimality of $(p^{*},r^{*})$. Thus, it holds that $\mathsf{E}[v(p^{*}-c(X_2{r}^{*}(X_1)))]=0$.

For any other contract $(p,r)$, we have (i) $\mathsf{E}[v(p-c(X_{2}r(X_1)))]>\mathsf{E}[v(p^{*}-c(X_2{r}^{*}(X_1)))]=0$ and $\mathsf{E}[u(\omega -X_1+X_2{r}^{*}(X_1)-p^{*})]>\mathsf{E}[u(\omega -X_1+X_{2}r(X_1)-p)]$, or (ii) $\mathsf{E}[v(p-c(X_{2}r(X_1)))]=\mathsf{E}[v(p^{*}-c(X_2{r}^{*}(X_1)))]=0$ and $\mathsf{E}[u(\omega -X_1+X_2{r}^{*}(X_1)-p^{*})]\ge \mathsf{E}[u(\omega -X_1+X_{2}r(X_1)-p)]$. By (i), for $0\le \lambda \le \frac{\mathsf{E}[u(\omega -X_1+X_2{r}^{*}(X_1)-p^{*})]-\mathsf{E}[u(\omega -X_1+X_{2}r(X_1)-p)]}{\mathsf{E}[v(p-c(X_{2}r(X_1)))]-\mathsf{E}[v(p^{*}-c(X_2{r}^{*}(X_1)))]}$, we have

$$\begin{array}{cc}& \mathsf{E}[u(\omega -X_1+X_{2}r(X_1)-p)]+\lambda \mathsf{E}[v(p-c(X_{2}r(X_1)))]\hfill \\ \le & \mathsf{E}[u(\omega -X_1+X_2{r}^{*}(X_1)-p^{*})]+\lambda \mathsf{E}[v(p^{*}-c(X_2{r}^{*}(X_1)))].\hfill \end{array}$$

(4)

By (ii), we have (4) also for any $\lambda \ge 0$.

Now, we conclude that for a Pareto-optimal contract $(p^{*},r^{*})$ of (1), there exists a multiplier $\lambda >0$ such that $(p^{*},r^{*})$ is the solution of

$$\underset{p\ge 0,0\le r(x)\le x}{max}\mathsf{E}[u(\omega -X_1+X_{2}r(X_1)-p)]+\lambda \mathsf{E}[v(p-c(X_{2}r(X_1)))].$$

(5)

By the duplicate expectation, at the optimal reinsurance premium $p^{*}$, for every $x\in [0,\hslash ]$, $r^{*}(x)$ is the solution of the state by state maximization problem

$$\underset{0\le r(x)\le x}{max}\mathsf{E}[u(\omega -x+X_{2}r(x)-p^{*})\mid X_1=x]+\lambda \mathsf{E}[v(p^{*}-c(X_{2}r(x)))\mid X_1=x].$$

(6)

We first present the existence and uniqueness of the Pareto-optimal contract of (1).

Proposition 1.

The optimization problem (1) has an unique Pareto-optimal contract.

As for the Pareto-optimal indemnity $r^{*}$, all zero points are at the forepart of $r^{*}$ if they do occur.

Proposition 2.

For the Pareto-optimal contract $(p^{*},r^{*})$ of (1), $r^{*}(x)=0$ for all $x\in [0,x_1]$ whenever $r^{*}(x_1)=0$.

Note that Propositions 1 and 2 actually hold irrespective of the dependence between the insured risk and the recovery rate. In casualty, if $X_1$ denotes the loss due to the injury and death of the insured, then in contrast to the financial catastrophic events (bond market downturn and stock market crash), the recovery rate $X_2$ usually gets a relatively smaller impact from the insured risk $X_1$, and it is reasonable to assume the independence between them. In other occasions, for example, the huge loss $X_1$ due to hurricanes, tornados and earthquake usually has a significant impact on the recovery rate $X_2$, and then the statistical dependence between them should not be ignored. In what follows, we study the structure of the indemnity schedule $r^{*}$ in the context of independence between the insured risk and the recovery rate. Let us start with the global property of $r^{*}$.

Proposition 3.

If $X_1$ and $X_2$ are independent, then unique Pareto-optimal contract $r^{*}(x)$ of Problem (1) is nondecreasing.

As a consequence, to have a further look into the Pareto-optimal contract of (1) we only need to focus on the nondecreasing indemnity schedule, which means the more claim the more transferred to the reinsurer. Here, we take the view that the risk neutral reinsurer is of proportional cost and hence associated expense.

Proposition 4.

For $v(x)=ax$ and $c(x)=(1+m)x$ with some $a>0$ and $m\ge 0$, if $X_1$ and $X_2$ are independent, then,

(i) 

$r^{*}(x)=x$ for all $x\ge x_1$ whenever $r^{*}(x_1)=x_1$,

(ii) 

$r^{*}(x_2)-r^{*}(x_1)\ge x_2-x_1$ for $x_2>x_1\ge 0$ whenever $x_i>r^{*}(x_i)>0$, $i=1,2$, and

(iii) 

$r^{*}(x)$ has a disappearing deductible.

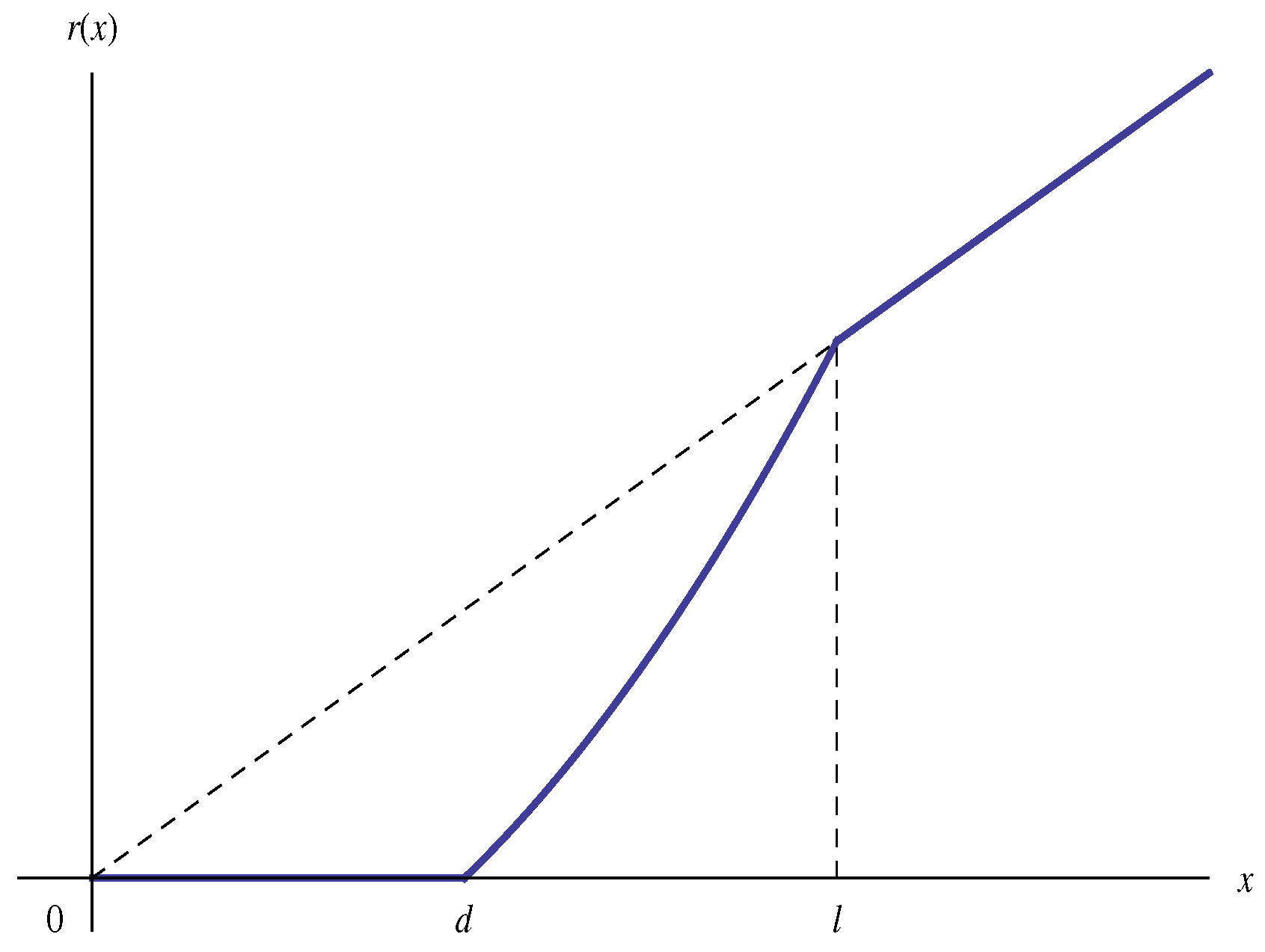
As per Proposition 4(i), for the risk neutral reinsurer with proportional associated expense, $r^{*}(x)=x$ occurs and only occurs at the rear part of $r^{*}$ if $r^{*}(x)=x$ for some $x\in (0,\hslash ]$. Also Proposition 4(ii) reiterates that ${\overline{r}}^{*}$ is nonincreasing when the Pareto-optimal contract is interior. Further, as depicted in Figure 1, in the context of Proposition 4, the $(p^{*},r^{*})$ is a disappearing deductible. That is, the optimal reinsurance contract takes the form of deductible followed by coinsurance and then full insurance. Also, for the coinsurance part the indemnity function is nondecreasing and ${\overline{r}}^{*}$ is nonincreasing.

4. Asymmetric Information Model—Scenario of Independence

For a Pareto-optimal contract $(p^{*},r^{*})$ of (2), there exists a multiplier $\lambda >0$ such that $(p^{*},r^{*})$ is the solution of

$$\underset{p\ge 0,0\le r(x)\le x}{max}\mathsf{E}[u(\omega -X_1+X_{2}r(X_1)-p)]+\lambda \mathsf{E}[v(p-c(r(X_1)))].$$

(7)

Again due to the duplicate expectation, at the optimal reinsurance premium $p^{*}$, for every $x\in [0,\hslash ]$, $r^{*}(x)$ is the solution of a state by state maximization problem

$$\underset{0\le r(x)\le x}{max}\mathsf{E}[u(\omega -x+X_{2}r(x)-p^{*})\mid X_1=x]+\lambda v(p^{*}-c(r(x))),\mathrm{for}\mathrm{all}x\in [0,\hslash ].$$

(8)

The existence and uniqueness of the Pareto-optimal contracts of (2) can be built in a completely similar manner to that of (1) and hence we present them with the proof omitted.

Proposition 5.

The optimization problem (2) has an unique Pareto-optimal contract.

As for the structure of the indemnity schedule $r^{*}(x)$, we consider that the recovery rate and the insured risk with the absence of dependence. The global property of $r^{*}(x)$ in Proposition 6 can be accomplished in a similar manner to Proposition 3, and thus we omit the proof for briefness.

Proposition 6.

If $X_1$ and $X_2$ are independent, then the unique Pareto-optimal contract $r^{*}$ of Problem (2) is nondecreasing.

As a result of Proposition 6, to study the Pareto-optimal contract of (2) we only pay attention to the nondecreasing indemnity schedule, having all zero points at the forepart if it does have some zero points. That is, the reinsurer will undertake more loss when the claim is larger, and $r^{*}(x)=0$ for all $x\in [0,x_1]$ whenever $r^{*}(x_1)=0$. In practice, the increase of the reinsurance payment usually results in an increase of the associated expense. That is, $c(x)-x$ is increasing in $x\in [0,\hslash ]$, or equivalently, $c^{\prime }(x)>1$ for $x\in [0,\hslash ]$. In the next proposition, we present a sufficient condition for the existence of zero point of $r^{*}(x)$.

Proposition 7.

For $c(x)$ with $c^{\prime }(x)>1$ on $x\in [0,\hslash ]$, if $X_1$ and $X_2$ are independent, then (i) the full reinsurance is not Pareto-optimal, (ii) $r(x)\in (0,x)$ for all $x\in (0,\hslash ]$ is not Pareto-optimal, and (iii) $r^{*}(x)$ must have zero points on $x\in (0,\hslash ]$.

According to Proposition 7, neither full insurance nor coinsurance are optimal, and the optimal reinsurance contract must have noninsurance part. For the risk neutral reinsurer with proportional associated expense, we further have the following.

Proposition 8.

For $v(x)=ax$ and $c(x)=(1+m)x$ with $a,m>0$, if $X_1$ and $X_2$ are independent, then,

(i) 

$r^{*}(x)=x$ for all $x\ge x_1$ whenever $r^{*}(x_1)=x_1$,

(ii) 

$r^{*}(x_2)-r^{*}(x_1)\ge x_2-x_1$ whenever $x_2>x_1\ge 0$ and $x_i>r^{*}(x_i)>0$, for $i=1,2$, and

(iii) 

$r^{*}$ takes one structure of (a) – (d):

(a) 

$r^{*}(x)=0$ for all $x\ge 0$ (nonreinsurance),

(b) 

$r^{*}(x)=0$ followed by $r^{*}(x)=x$,

(c) 

$r^{*}(x)=0$ followed by $r^{*}$ with $0<r^{*}(x)<x$, $r^{*}$ is nondecreasing, and ${\overline{r}}^{*}$ is nonincreasing,

(d) 

$r^{*}(x)=0$ followed by $r^{*}$ with $0<r^{*}(x)<x$, $r^{*}$ is nondecreasing, and ${\overline{r}}^{*}$ is nonincreasing, followed by $r^{*}(x)=x$.

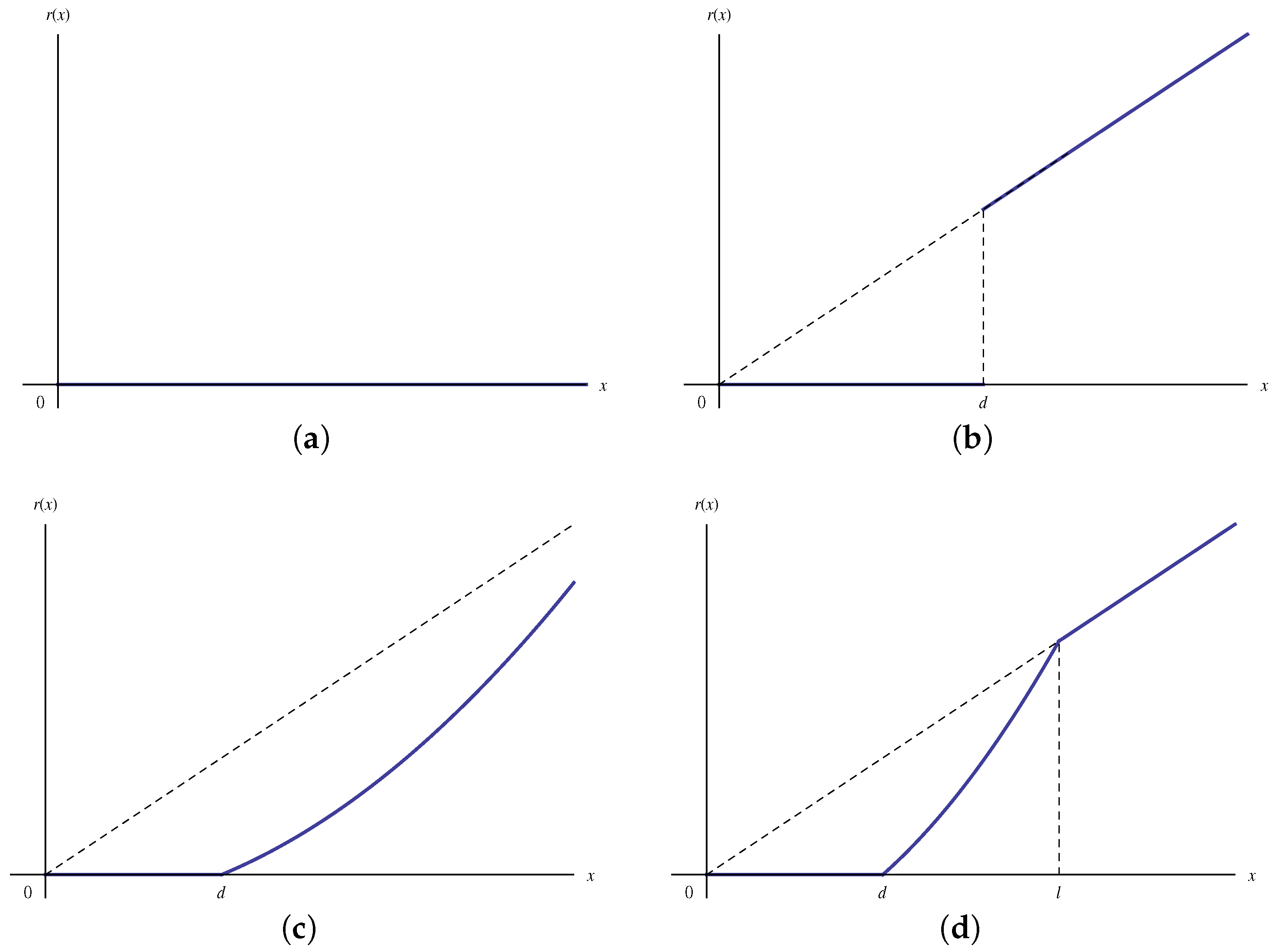
For the risk neutral reinsurer with proportional associated expense, Proposition 8(i) guarantees that $r^{*}(x)=x$ occurs and only occurs at the rear part of $r^{*}$ if there exists $x\in (0,\hslash ]$ such that $r^{*}(x)=x$. By Proposition 8(ii), the indemnity function ${\overline{r}}^{*}$ is nonincreasing when Pareto-optimal contract is interior. Also Proposition 8(iii) asserts that $(p^{*},r^{*})$ only has one of the four possible structures depicted in Figure 2. Also, for the coinsurance part the indemnity function is nondecreasing with nonincreasing ${\overline{r}}^{*}$. For example, the stop-loss contract $r(x)={(x-d)}_{+}$ with some $d>0$ may be Pareto-optimal while the change-loss contract $r(x)=a{(x-d)}_{+}$ with $a\in (0,1)$ and $d>0$ is not.

5. Asymmetric Information Model—Scenario of Dependence

Since a larger loss is more likely to make the reinsurer at default risk, it is desired that the recovery rate and the insured risk are negatively dependent. In this section we model such a negative dependence by using the stochastic decreasing property.

For the reinsurers with upper bounded risk aversion coefficient, Proposition 9 builds the global property of the Pareto-optimal indemnity. According to Lemma 3, $\mathsf{E}[u(w-(1-X_2)x-X_{2}y-p)\mid X_1=x]$ is supermodular. The proof is similar to that of Proposition 3 and thus omitted.

Proposition 9.

If $X_2{\downarrow }_{\mathrm{st}}{X}_1$ and (3) holds, then the unique Pareto-optimal contract ${\overline{r}}^{*}(x)$ of Problem (2) is nondecreasing.

This proposition suggests us to only focus on nondecreasing $\overline{r}$ if the reinsurer has an upper-bounded risk aversion coefficient. That is, the more claim the more will be retained by the insurer. According to the next proposition, $r^{*}(x)=x$ occurs and only occurs at the forepart of $r^{*}$ if $r^{*}(x)=x$ for some $x\in (0,\hslash ]$.

Proposition 10.

If $X_2{\downarrow }_{\mathrm{st}}{X}_1$ and (3) holds, then $r^{*}(x)=x$ for all $x\in [0,x_1]$ whenever $r^{*}(x_1)=x_1$.

In the remaining of this section, we address the sufficient condition for the existence a zero point.

Proposition 11.

If $X_2{\downarrow }_{\mathrm{st}}{X}_1$ and $c^{\prime }(x)>1$ for all $x\in [0,\hslash ]$, then, (i) the full reinsurance is not Pareto-optimal, (ii) $r(x)\in (0,x)$ for all $x\in (0,\hslash ]$ is not Pareto-optimal, and (iii) $r^{*}(x)$, for $x\in (0,\hslash ]$ must contain a zero point.

As per Proposition 11, neither full insurance nor coinsurance are optimal, and the optimal reinsurance contract must have noninsurance part. Also, we pay particular attention to the risk neutral reinsurer with proportional associated expense and study the possible structure of the Pareto-optimal indemnity schedule.

Proposition 12.

If $X_2{\downarrow }_{\mathrm{st}}{X}_1$, $v(x)=ax$ and $c(x)=(1+m)x$ for some $a,m>0$, then,

(i) 

$r^{*}(x)=0$ for all $x\ge x_1$ whenever $r^{*}(x_1)=0$.

If further (3) holds, then,

(ii) 

$r^{*}(x_1)\ge r^{*}(x_2)$ whenever $x_2>x_1\ge 0$, $x_i>r^{*}(x_i)>0$ for $i=1,2$, and

(iii) 

$r^{*}$ takes one of the following structures:

(a) 

$r^{*}(x)=0$ for all $x\ge 0$ (nonreinsurance),

(b) 

$r^{*}(x)=x$ followed by $r^{*}(x)=0$,

(c) 

$r^{*}(x)=x$ followed by $r^{*}$ such that $r^{*}(x)\in (0,x)$, $r^{*}$ is non-increasing, and ${\overline{r}}^{*}(x)$ is nondecreasing, followed by $r^{*}(x)=0$.

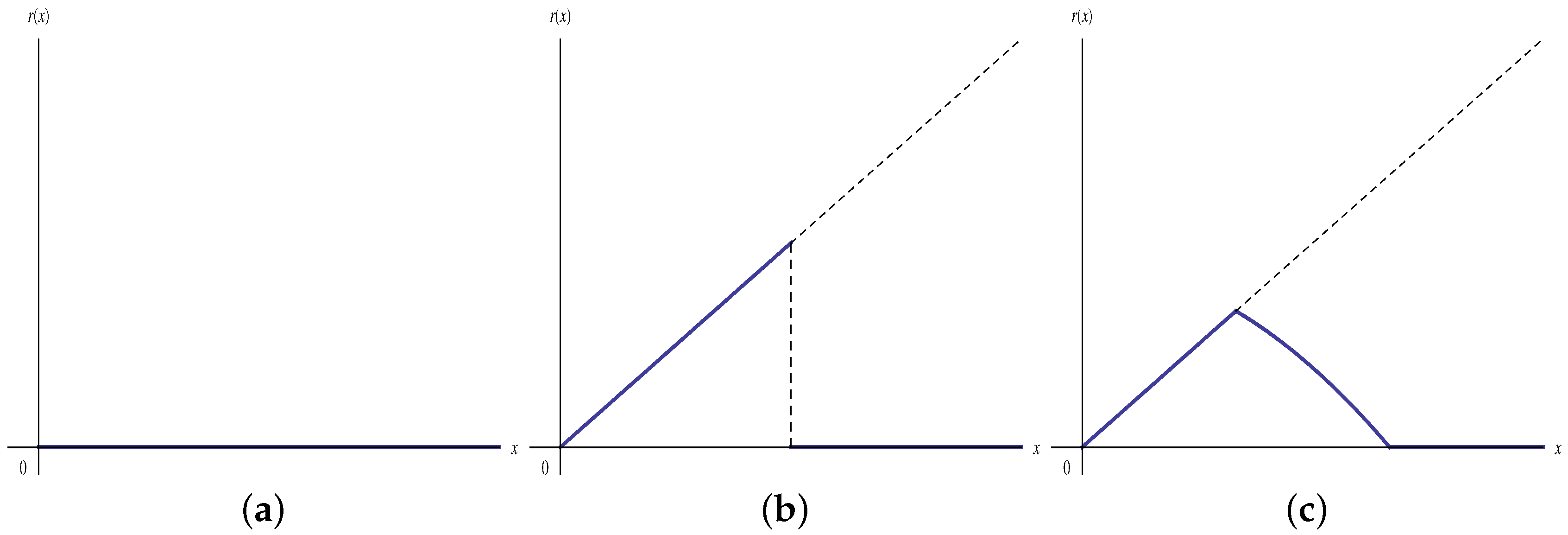
According to Proposition 12(i), for the risk neutral reinsurer with proportional associated expense, the zero points occurs and only occurs at the rear part of $r^{*}$ if $r^{*}$ does have some zero points. Proposition 12(ii) pronounces that $r^{*}$ is nonincreasing when Pareto-optimal contract is interior. Furthermore, Proposition 12(iii) asserts that $r^{*}$ can take only three possible structures depicted in Figure 3. Also, for the coinsurance part the indemnity function is nonincreasing with nondecreasing ${\overline{r}}^{*}$. It should be noted that a truncated contract $r^{*}(x)=x$ for $x\in [0,l]$, $r^{*}(x)=l$ for $x\in [l,d)$, and $r^{*}(x)=0$ for $x\in [d,\hslash ]$ belongs to structure (c), for some $0\le l<d<\hslash$.

6. Concluding Remarks

Dana and Scarsini (2007) and Bernard and Ludkovski (2012) dealt with Pareto-optimal reinsurance contracts in the presence of additive background risk and multiplicative one, respectively. When $v(x)=ax$ and $c(x)=x$, the optimization problems (1) and (2) reduce to the models due to Bernard and Ludkovski (2012). Our models differ from theirs in the following two aspects.

(i)

Bernard and Ludkovski (2012) specified $v(x)=ax$ and $c(x)=x$, meaning that the seller is risk neutral and there is no extra cost when dealing with the ceded loss. However, the seller sometimes are risk averse and there does exist extra cost besides the ceded loss itself.

(ii)

In the case of dependence, Bernard and Ludkovski (2012) dealt with the case $X_2$ takeing value only on $\{x_0,1\}$, with $0\le x_0<1$, whereas, we only assume $0\le X_2\le 1$ and $X_2\neg \equiv 1$, a more general situation. Rather than assuming a conditional Bernoulli distribution for the recovery rate $X_2$ in Bernard and Ludkovski (2012), we deal with $X_2{\downarrow }_{\mathrm{st}}{X}_1$, which is of more practical interest.

As a result, our research complement those in Dana and Scarsini (2007) by incorporating the multiplicative background risk, and generalize the model in Bernard and Ludkovski (2012) through considering risk averse reinsurer with extra cost and recovery rate within $[0,1]$.

According to Propositions 1 and 6, Problems (1) and (2) both have an unique Pareto-optimal contract. Furthermore, if $X_1$ and $X_2$ are independent, we only need to pay attention to nondecreasing indemnity functions (Propositions 3 and 6), and if $X_2$ is stochastically decreasing in $X_1$, we only need to focus on the indemnity function r with nondecreasing $\overline{r}$ (Proposition 9). Specifically, for risk neutral reinsurer with proportional cost Propositions 4, 8 and 12 provide possible structures for the optimal indemnity functions, which helps the insurer to further investigate the closed form of the optimal reinsurance contract.

Since there probably exists discontinuity and drop-down in the optimal reinsurance contract in Problems (1) and (2), the insurers may have the incentive to underreport or overreport the loss. To avoid the moral hazard, one may consider the following feasible set of the indemnity functions

$$\mathcal{I}=\left\{r:0\le r(x)\le x,\mathrm{and}r(x)\mathrm{and}x-r(x)\mathrm{are}\mathrm{both}\mathrm{increasing}\mathrm{for}x\ge 0\right\},$$

and deal with the corresponding Pareto-optimal problems

$$\left\{\begin{array}{c}\underset{(p,r)}{max}\mathsf{E}[u(\omega -X_1+X_{2}r(X_1)-p)]\hfill \\ \mathrm{s}.\mathrm{t}.r\in \mathcal{I}\mathrm{and}\mathsf{E}[v(p-c(X_{2}r(X_1)))]\ge 0\hfill \end{array}\right.$$

(9)

and

$$\left\{\begin{array}{c}\underset{(p,r)}{max}\mathsf{E}[u(\omega -X_1+X_{2}r(X_1)-p)]\hfill \\ \mathrm{s}.\mathrm{t}.r\in \mathcal{I}\mathrm{and}\mathsf{E}[v(p-c(r(X_1)))]\ge 0.\hfill \end{array}\right.$$

(10)

Similar to Propositions 1 and 9, we have the following proposition.

Proposition 13.

Both the optimization Problems (9) and (10) have unique Pareto-optimal contract.