A Threshold Type Policy for Trading a Mean-Reverting Asset with Fixed Transaction Costs
Abstract
1. Introduction
2. Problem Description and Method
2.1. Formulation of the Optimal Trading Problem
2.2. Bounds of the Value Functions
2.3. The HJB Equations
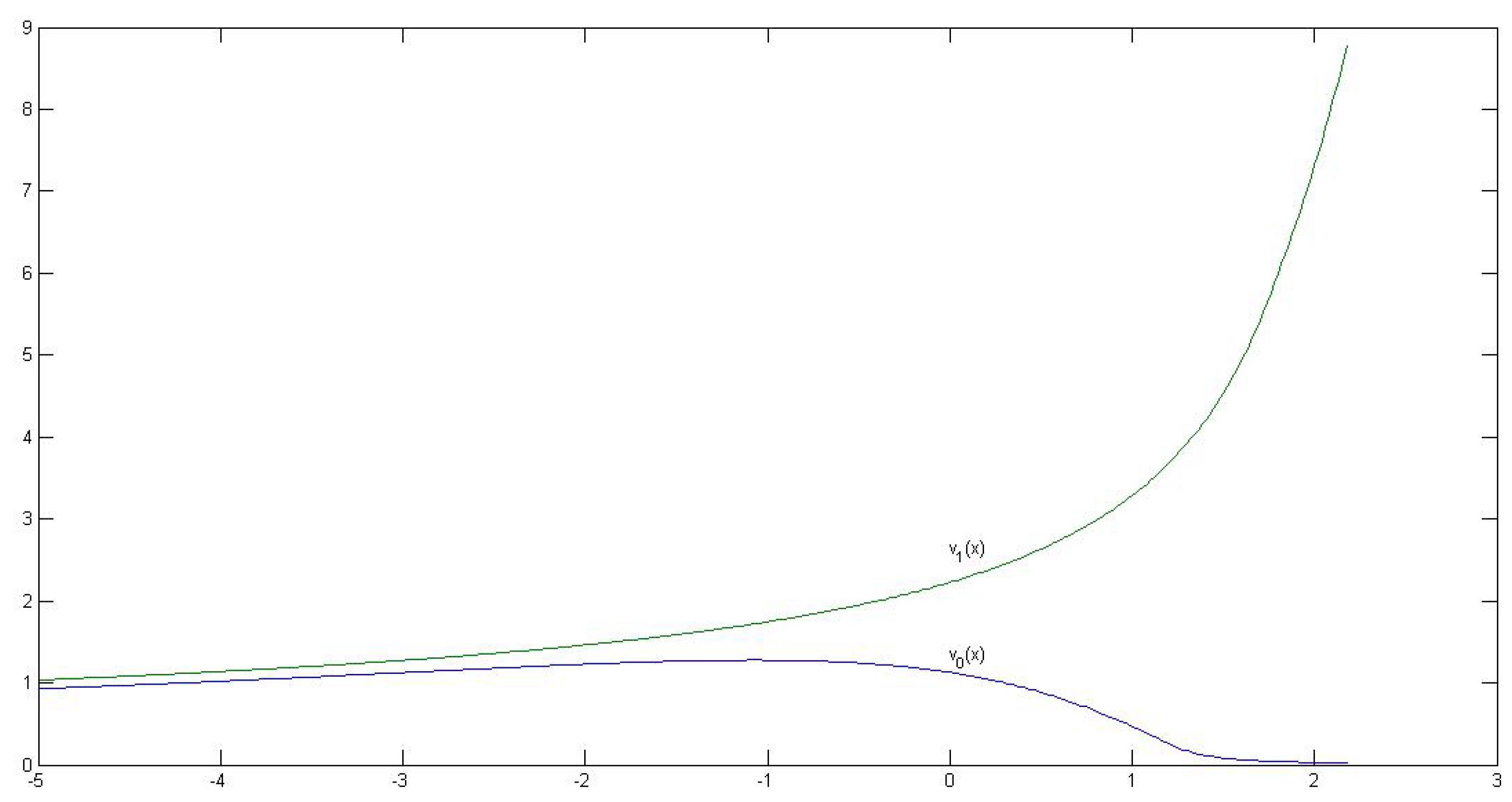
2.4. Solutions of the HJB Equations
2.5. A Verification Theorem
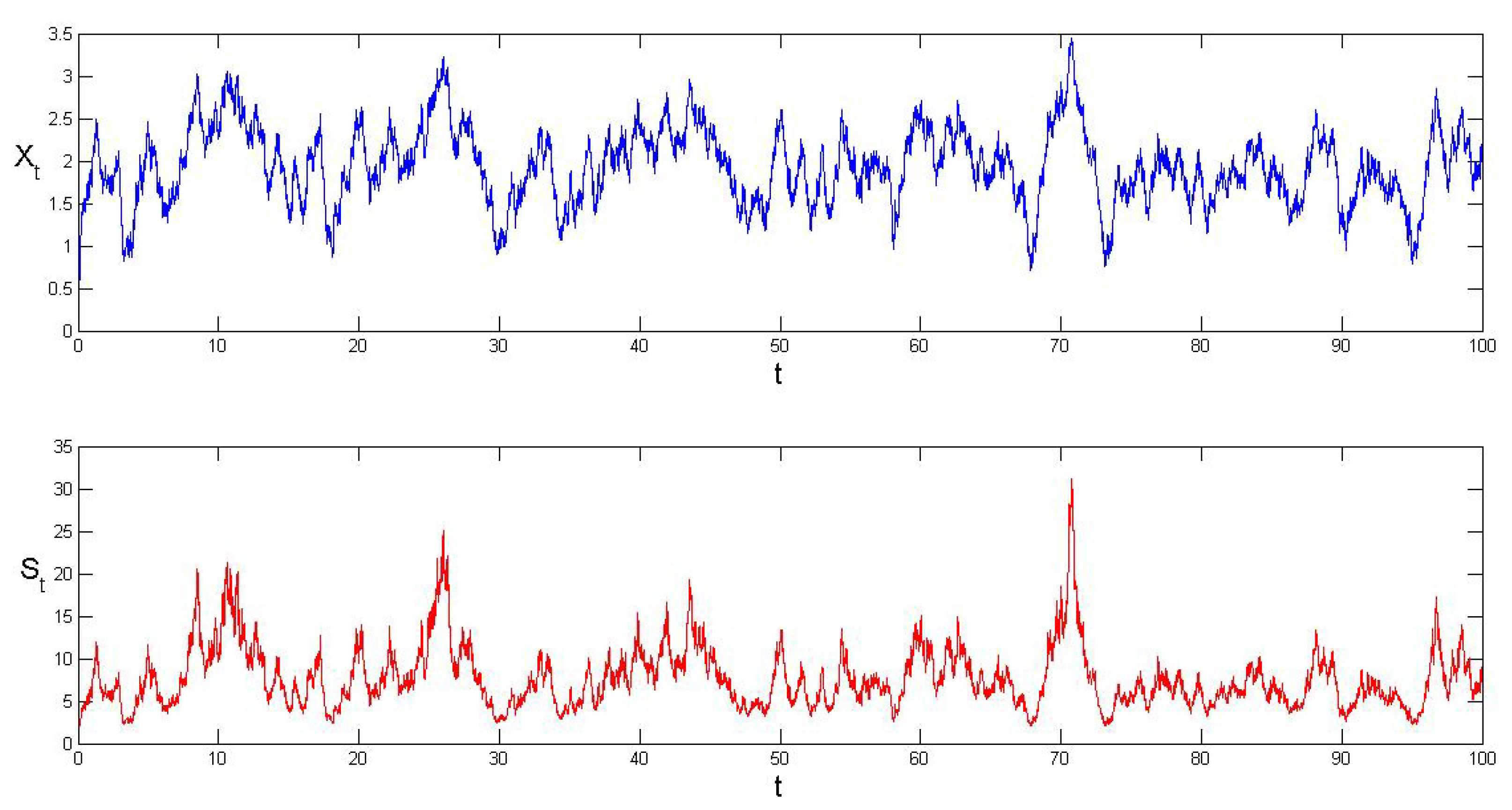
3. Numerical Results and Discussion
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Baccarin, Stefano, and Daniele Marazzina. 2014. Optimal impulse control of a portfolio with a fixed transaction cost. Central European Journal of Operations Research 22: 355–72. [Google Scholar] [CrossRef]
- Baccarin, Stefano, and Daniele Marazzina. 2016. Passive portfolio management over a finite horizon with a target liquidation value under transaction costs and solvency constraints. IMA Journal of Management Mathematics 27: 471–504. [Google Scholar] [CrossRef]
- Blanco, Carlos, and David Soronow. 2001. Mean reverting process—Energy price processes used for derivatives pricing and risk management. Commodities Now, 68–72. [Google Scholar]
- Cowles, Alfred, and Herbert E. Jones. 1937. Some a posteriori probabilities in stock market action. Econometrica 5: 280–94. [Google Scholar] [CrossRef]
- Dai, Min, Qing Zhang, and Qiji Zhu. 2010. Trend following trading under a regime switching model. SIAM Journal on Financial Mathematics 1: 780–810. [Google Scholar] [CrossRef]
- Eloe, Paul, Ruihua Liu, M. Yatsuki, George Yin, and Qing Zhang. 2008. Optimal selling rules in a regime-switching exponential gaussian diffusion model. SIAM Journal on Applied Mathematics 69: 810–29. [Google Scholar] [CrossRef]
- Fama, Eugene F., and Kenneth R. French. 1988. Permanent and temporary components of stock prices. Journal of Political Economy 96: 246–73. [Google Scholar] [CrossRef]
- Gallagher, Liam A., and Mark P. Taylor. 2002. Permanent and temporary components of stock prices: Evidence from assessing macroeconomic shocks. Southern Economic Journal 69: 345–62. [Google Scholar] [CrossRef]
- Guo, Xin, and Qing Zhang. 2005. Optimal selling rules in a regime switching model. IEEE Transactions on Automatic Control 50: 1450–55. [Google Scholar] [CrossRef]
- Hafner, Christian M., and Helmut Herwartz. 2001. Option pricing under linear autoregressive dynamics, heteroskedasticity, and conditional leptokurtosis. Journal of Empirical Finance 8: 1–34. [Google Scholar] [CrossRef]
- Hull, John. 2003. Options, Futures & Other Derivatives. Prentice Hall Finance Series: Options, Futures. Derivatives; Upper Saddle River: Prentice Hall. [Google Scholar]
- Iwarere, Sesan, and B. Ross Barmish. 2010. A confidence interval triggering method for stock trading via feedback control. Paper presented at 2010 American Control Conference, Baltimore, MD, USA, June 30–July 2; pp. 6910–16. [Google Scholar] [CrossRef]
- Leung, Tim, Xin Li, and Zheng Wang. 2015. Optimal multiple trading times under the exponential ou model with transaction costs. Stochastic Models 31: 554–87. [Google Scholar] [CrossRef]
- Løkka, Arne, and Mihail Zervos. 2013. Long-term optimal real investment strategies in the presence of adjustment costs. SIAM Journal Control and Optimization 51: 996–1034. [Google Scholar] [CrossRef]
- Luu, Phong. 2016. Optimal Pairs Trading Rules and Numerical Methods. Ph.D. dissertation, University of Georgia, Athens, GA, USA. [Google Scholar]
- Merhi, Amal, and Zervos Zervos. 2007. A model for reversible investment capacity expansion. SIAM Journal on Control and Optimization 46: 839–76. [Google Scholar] [CrossRef]
- Øksendal, Bernt. 2003. Stochastic Differential Equations: An Introduction with Applications. New York: Springer. [Google Scholar]
- Øksendal, Bernt, and Agnès Sulem. 2002. Optimal consumption and portfolio with both fixed and proportional transaction costs. SIAM Journal on Control and Optimization 40: 1765–90. [Google Scholar] [CrossRef]
- Song, Qingshuo, George Yin, and Qing Zhang. 2009. Stochastic optimization methods for buying-low-and-selling-high strategies. Stochastic Analysis and Applications 27: 523–42. [Google Scholar] [CrossRef]
- Vasicek, Oldrich. 1977. An equilibrium characterization of the term structure. Journal of Financial Economics 5: 177–88. [Google Scholar] [CrossRef]
- Xia, Jianmin, and Xunyu Zhou. 2007. Stock loans. Mathematical Finance 17: 307–17. [Google Scholar] [CrossRef]
- Zhang, Hanqin, and Qing Zhang. 2008. Trading a mean-reverting asset: Buy low and sell high. Automatica 44: 1511–18. [Google Scholar] [CrossRef]
- Zhang, Qing. 2001. Stock trading: An optimal selling rule. SIAM Journal on Control and Optimization 40: 64–87. [Google Scholar] [CrossRef]
- Zhang, Qing, and Xunyu Zhou. 2009. Valuation of stock loans with regime switching. SIAM Journal on Control and Optimization 48: 1229–50. [Google Scholar] [CrossRef]
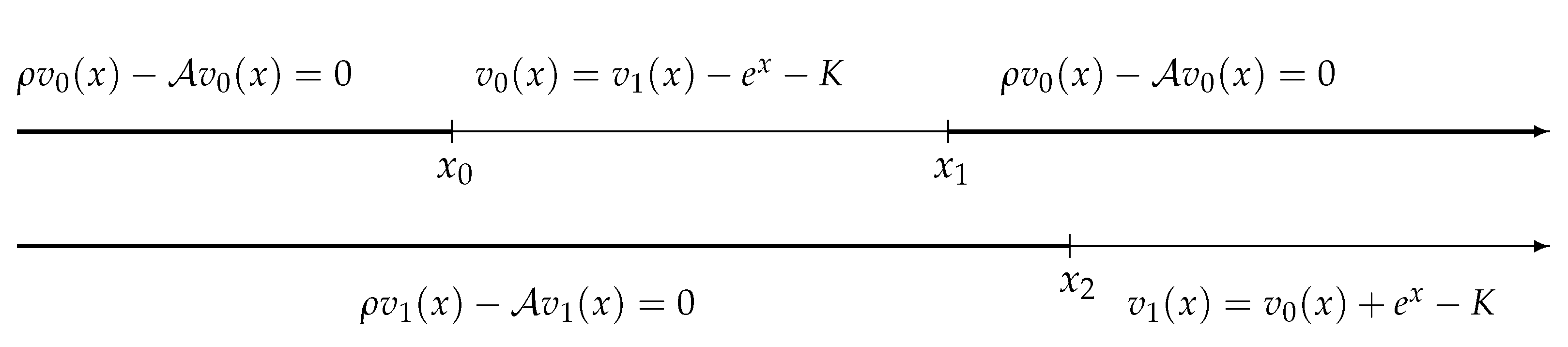
| b | 1 | 1.5 | 2 | 2.5 | 3 |
|---|---|---|---|---|---|
| −4.36 | −4.46 | −4.58 | −4.66 | −4.74 | |
| 0 | 0.64 | 1.22 | 1.78 | 2.34 | |
| 0.74 | 1.22 | 1.7 | 2.18 | 2.66 |
| a | 0.6 | 0.7 | 0.8 | 0.9 | 1 |
|---|---|---|---|---|---|
| −4.2 | −4.4 | −4.58 | −4.72 | −4.86 | |
| 0.98 | 1.14 | 1.22 | 1.3 | 1.36 | |
| 1.58 | 1.62 | 1.7 | 1.74 | 1.78 |
| 0.3 | 0.4 | 0.5 | 0.6 | 0.7 | |
|---|---|---|---|---|---|
| −4.56 | −4.56 | −4.58 | −4.58 | −4.6 | |
| 1.12 | 1.16 | 1.22 | 1.28 | 1.36 | |
| 1.54 | 1.62 | 1.7 | 1.8 | 1.9 |
| 0.3 | 0.4 | 0.5 | 0.6 | 0.7 | |
|---|---|---|---|---|---|
| −5 | −4.86 | −4.58 | −4.32 | −4.12 | |
| 1.56 | 1.36 | 1.22 | 1.06 | 0.9 | |
| 1.94 | 1.84 | 1.7 | 1.56 | 1.42 |
| K | 0.01 | 0.05 | 0.1 | 0.5 | 1 |
|---|---|---|---|---|---|
| −5 | −5 | −4.58 | −2.56 | −1.6 | |
| 1.46 | 1.3 | 1.22 | 0.7 | −0.46 | |
| 1.56 | 1.68 | 1.7 | 1.78 | 1.86 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Luu, P.; Tie, J.; Zhang, Q. A Threshold Type Policy for Trading a Mean-Reverting Asset with Fixed Transaction Costs. Risks 2018, 6, 107. https://doi.org/10.3390/risks6040107
Luu P, Tie J, Zhang Q. A Threshold Type Policy for Trading a Mean-Reverting Asset with Fixed Transaction Costs. Risks. 2018; 6(4):107. https://doi.org/10.3390/risks6040107
Chicago/Turabian StyleLuu, Phong, Jingzhi Tie, and Qing Zhang. 2018. "A Threshold Type Policy for Trading a Mean-Reverting Asset with Fixed Transaction Costs" Risks 6, no. 4: 107. https://doi.org/10.3390/risks6040107
APA StyleLuu, P., Tie, J., & Zhang, Q. (2018). A Threshold Type Policy for Trading a Mean-Reverting Asset with Fixed Transaction Costs. Risks, 6(4), 107. https://doi.org/10.3390/risks6040107






