An Optimal Investment Strategy for Insurers in Incomplete Markets
Abstract
:1. Introduction
2. The Stochastic Volatility Model
- A Poisson process with intensity and jump times ;
- A sequence of i.i.d. positive random variables with common distribution G;
- The Cramér-Lundberg process , where is the initial reserve of the insurance company and c is the constant premium rate;
- and are independent standard Brownian motions;
3. Existence of a Solution for the Exponential Utility Function
Bounds for and
4. Numerical Methods
4.1. The Monte Carlo Method
4.2. The Mixed Finite Difference-Monte Carlo Method
4.3. Stability of the Explicit Numerical Scheme
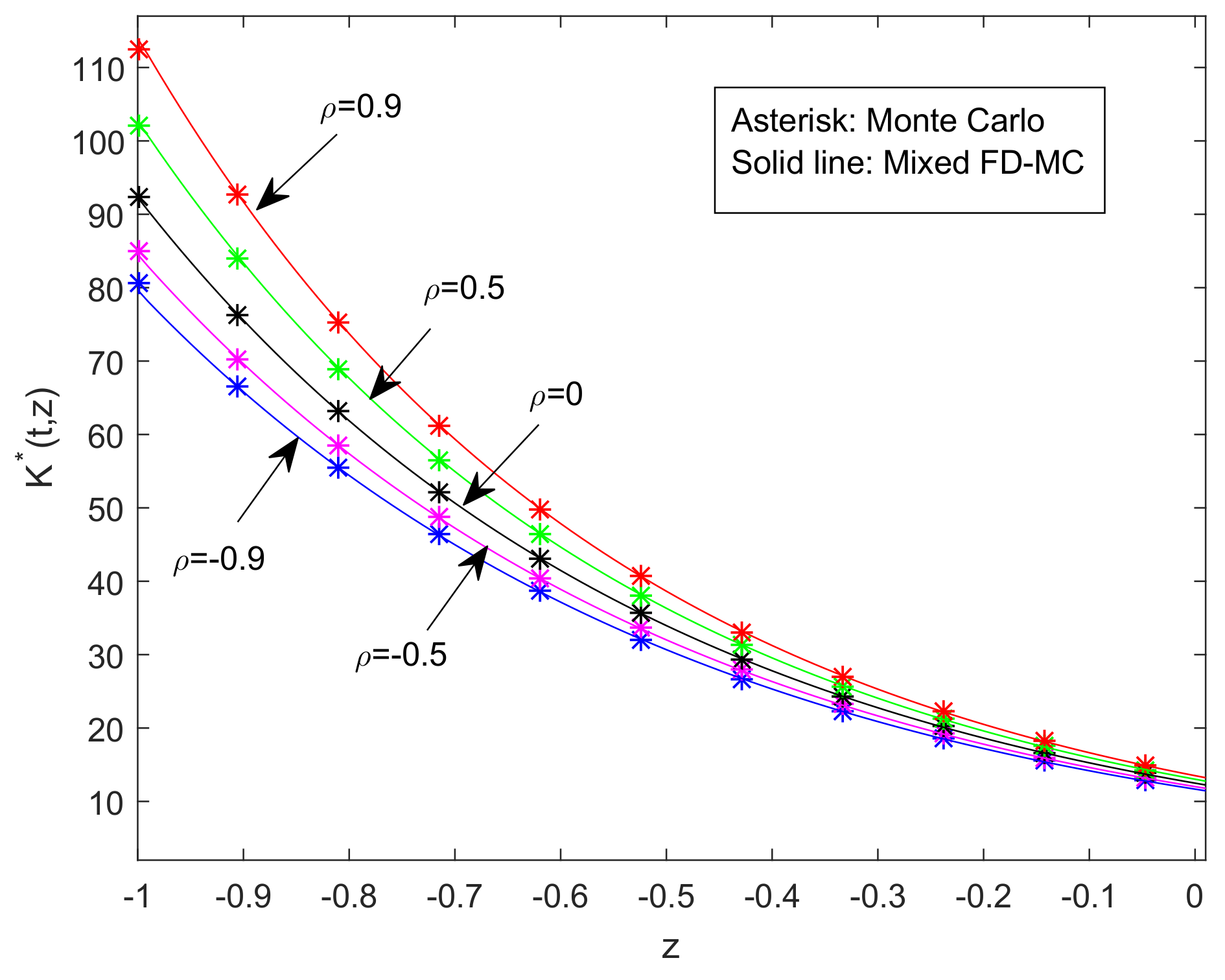
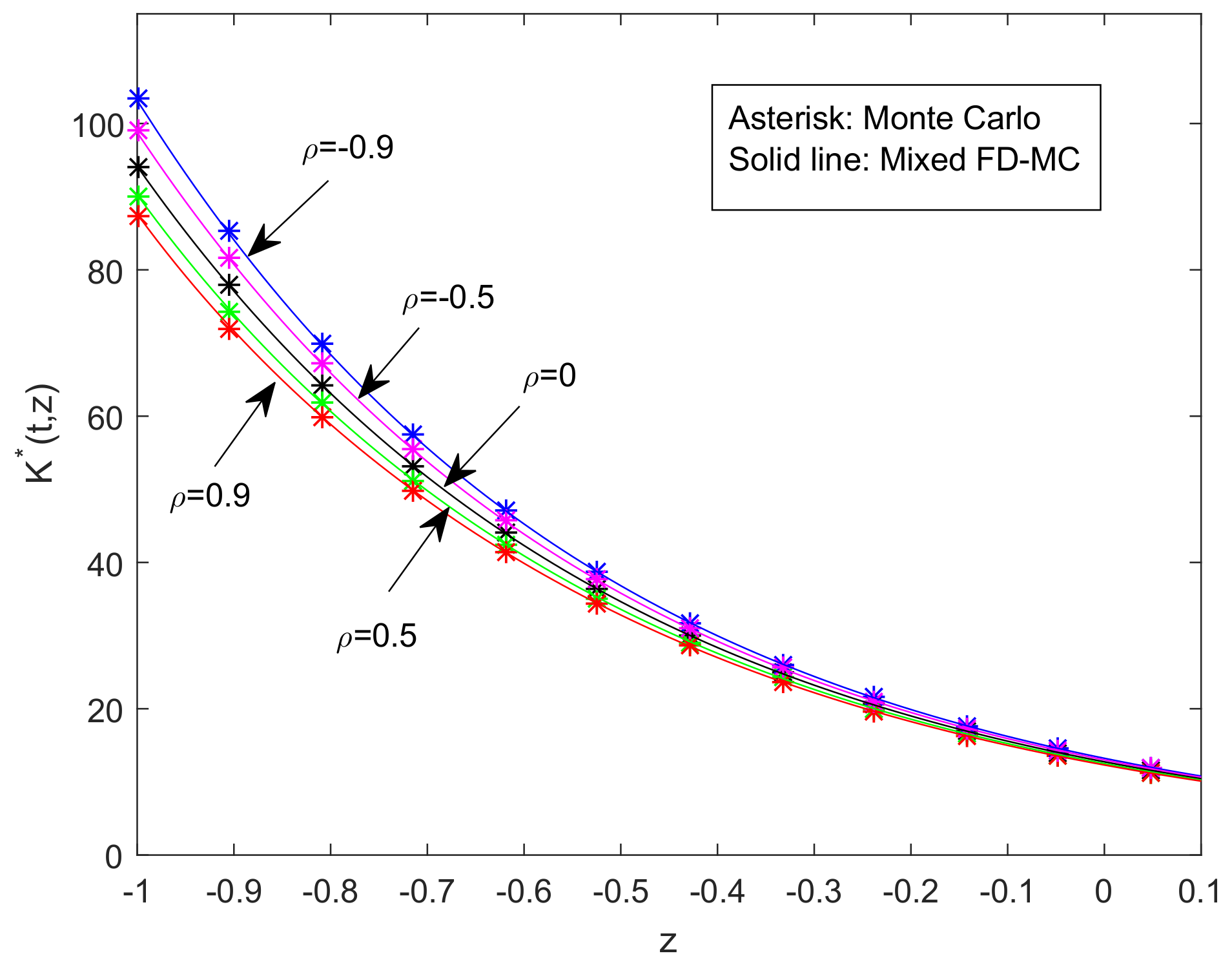
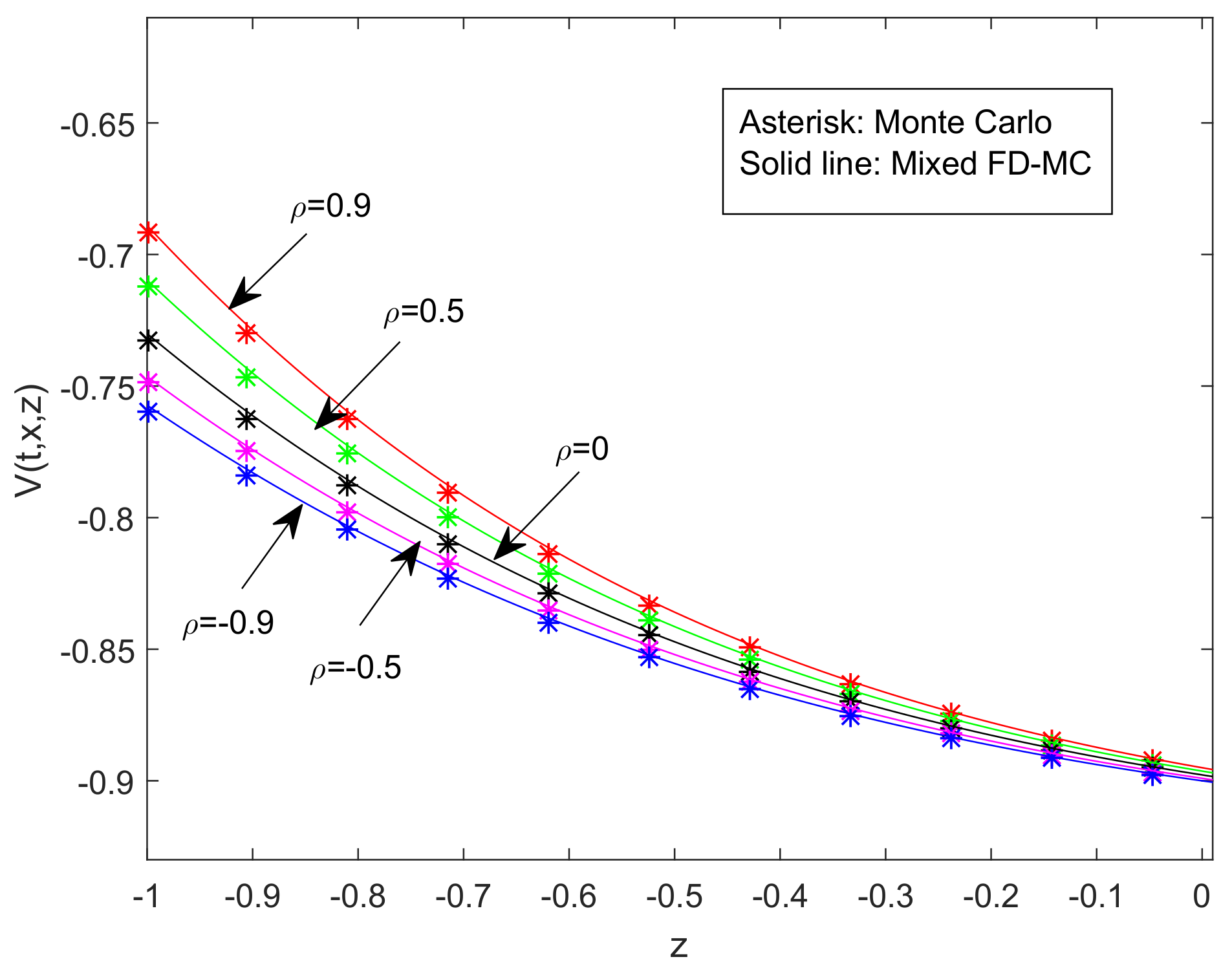
5. Numerical Experiments
5.1. Optimal Investment Strategy and Utility Function
- The optimal strategy as a function of the correlation coefficient ρ increases for and decreases for . When the correlation factor is nonnegative, then the external factor and the risky asset are positively correlated i.e, both are moving in the same direction, which is favorable in a financial environment Schlesinger and Doherty (1985), because the insurer is facing only one source of risk (an increase in the external factor implies an increase in the price of the risky asset) and insurers increase their investment to get more profit. When the correlation coefficient is negative the two risks faced by the insurers are evolving in opposite directions Schlesinger and Doherty (1985), which leads to high risk (an increase in the external factor implies losses in the risky asset), then insurers have to invest less as ρ becomes negatively large, this fact was observed in Zou and Cadenillas (2014) under a deterministic volatility model, when the preferences of the investor are Logarithmic or Exponential.
- Initial Surplus
- does not depend on x.
- , then is increasing as a function of x.
- Premium Rate
- does not depend on c.
- , then increases with the premium rate.
- Risk Aversion
- may be negative or positive depending on the parameters of the model.
- Then the behavior of V (t,x,z) as a function of α depends on the sign of:
- Average Claim size
- If is the average claim size in the Cramér-Lundberg model, then does not depend on .
- which depends on the probability distribution of Y. For instance, if the claims are exponentially distributed with parameter we know from (55) that:andthen decreases as a function of .
- Jump Intensity
- does not depend on .
- then is deceasing as a function of .
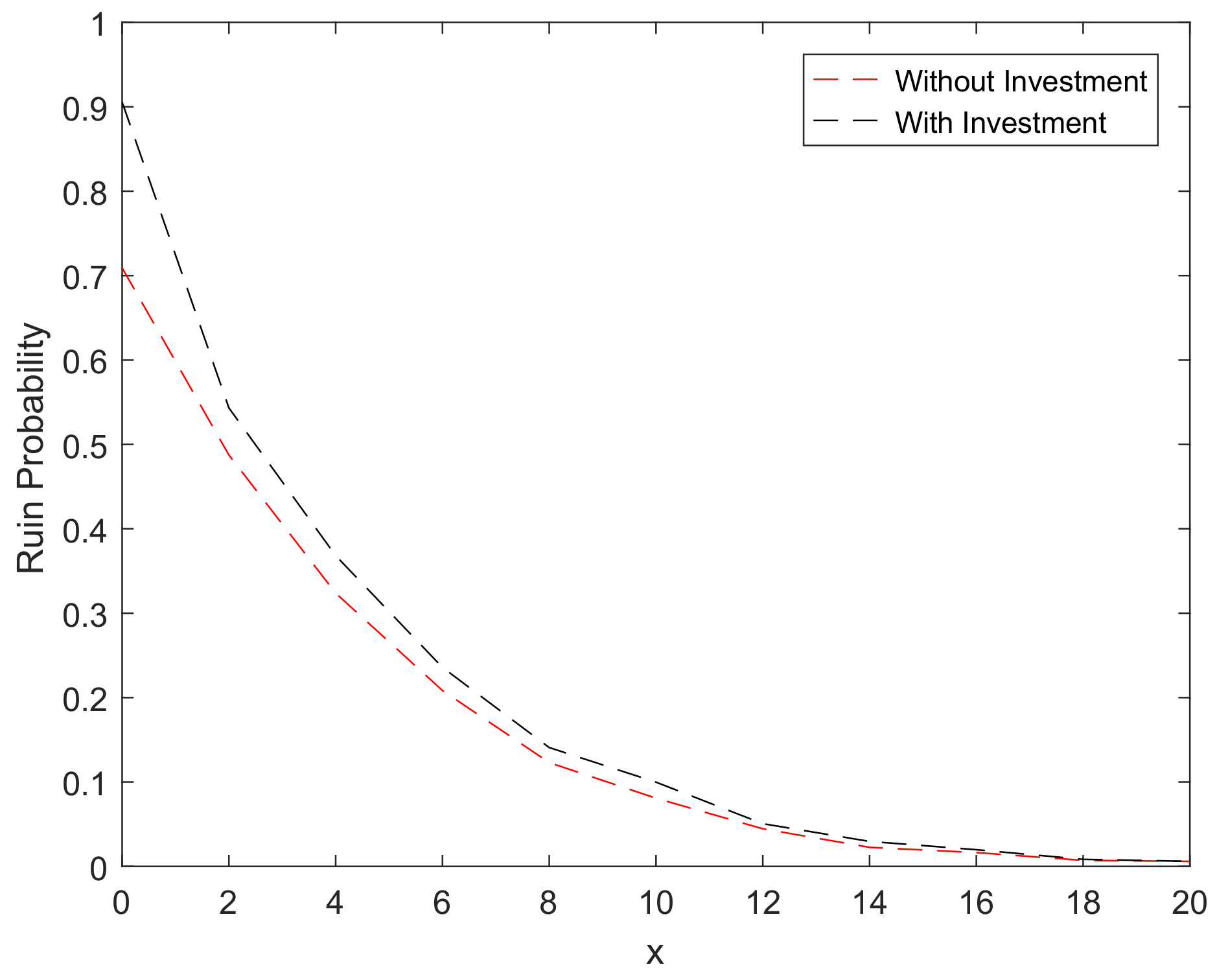
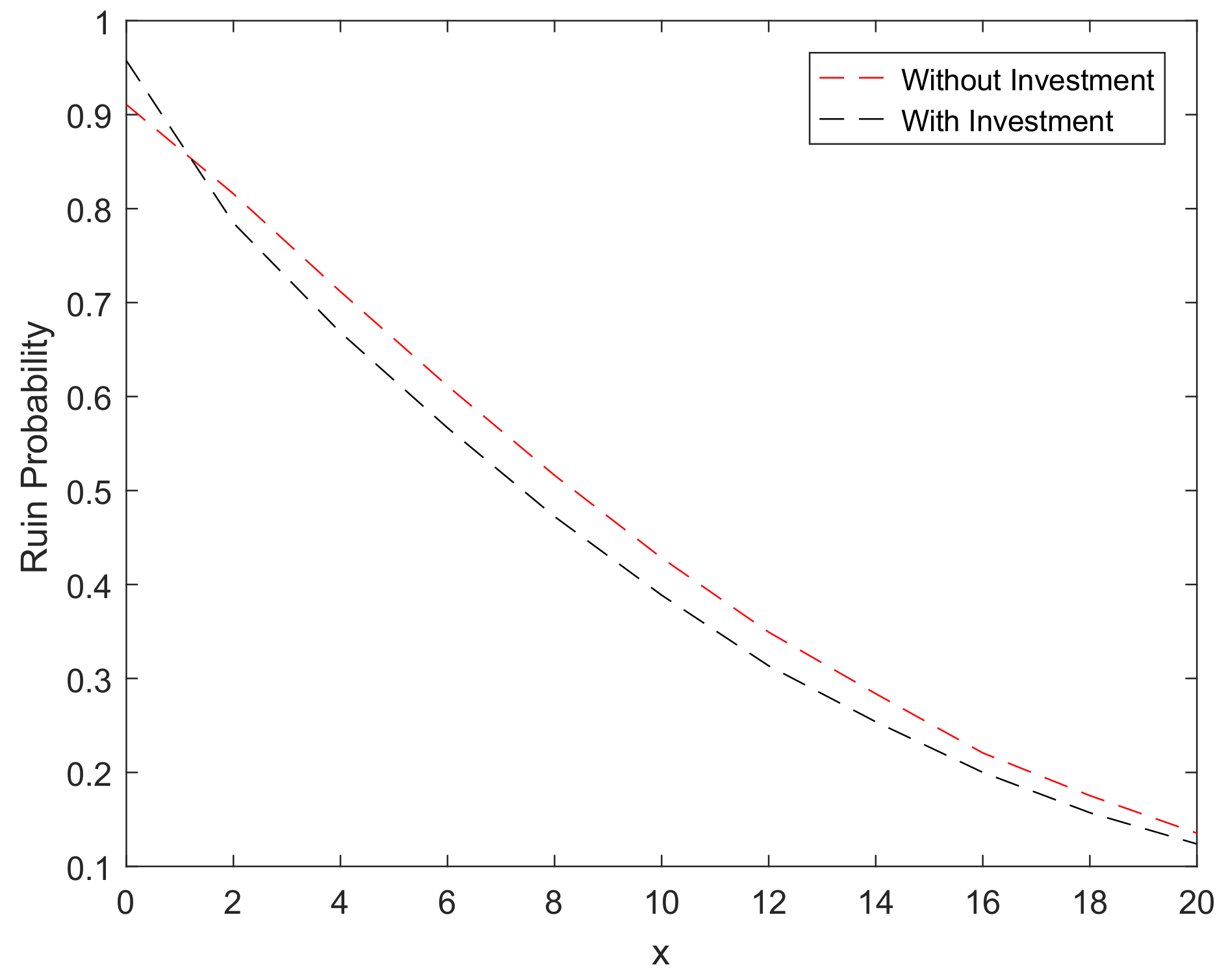
5.2. Ruin Probability
6. Discussion
- The approach used in this work is more general because it includes both the study of Complete and Incomplete markets. In the case of , the presence of the second Brownian motion cannot be eliminated, which means that the external factor cannot be traded through the risky asset.
- One consequence of our approach is to recover and improve the behavior of both expected utility and optimal strategy obtained in Badaoui and Fernández (2013), since there is no need to impose artificial boundary conditions, which in this work are approximated via the Monte Carlo method.
- When the insurer’s preferences are of exponential type, the optimal strategy depends only on time and the external factor regardless of the level of wealth.
- The Monte Carlo method is a good alternative for the finite difference method, when stochastic representation of the solution is available.
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Asmussen, Søren, and Peter W. Glynn. 2007. Stochastic Simulation, Algorithms and Analysis. New York: Springer. [Google Scholar]
- Badaoui, Mohamed, and Begoña Fernández. 2013. An optimal investment strategy with maximal risk aversion and its ruin probability in the presence of stochastic volatility on investments. Insurance: Mathematics and Economics 53: 1–13. [Google Scholar] [CrossRef]
- Borch, Karl. 1962. Equilibrium in a reinsuarnce market. Econometrica 30: 424–44. [Google Scholar] [CrossRef]
- Browne, Sid. 1995. Optimal investment policies for a firm with a random risk process: Exponential utility and minimizing the ruin probability of ruin. Mathematics of Operations research 20: 937–58. [Google Scholar] [CrossRef]
- Calfisch, Russel E., and Bradley Moskowitz. 1995. Modified Monte Carlo methods using quasi-random sequences. In Lecture Notes in Statistics. New York: Springer, vol. 106, pp. 1–16. [Google Scholar]
- Castañeda, Netzahualcóyotl, and Daniel Hernández-Hernández. 2005. Optimal consumption-investment problems in incomplete markets with stochastic coefficients. SIAM Journal on Control and Optimization 44: 1322–44. [Google Scholar] [CrossRef]
- Cont, Rama, and Peter Tankov. 2003. Financial Modelling with Jump Processes. Orange: Chapman & Hall. [Google Scholar]
- Ferguson, Thomas S. 1965. Betting systems which minimize the probability of ruin. Journal of the Society for Industrial and Applied Mathematics 13: 795–818. [Google Scholar] [CrossRef]
- Fernández, Begoña, Daniel Hernández-Hernández, Ana Meda, and Patricia Saavedra. 2008. An optimal investment strategy with maximal risk aversion and its ruin probability. Mathematical Methods of Operations Research 68: 159–79. [Google Scholar]
- Fleming, Wendell H., and Daniel Hernández-Hernández. 2005. The tradeoff between consumption and investment in incomplete financial markets. Applied Mathematics and Optimization 52: 219–35. [Google Scholar] [CrossRef]
- Fouque, Jean-Pierre, George Papanicolaou, and K. Ronnie Sircar. 2000. Derivatives in Financial Markets with Stochastic Volatility. Cambridge: Cambridge Univerrsity Press. [Google Scholar]
- Friedman, Avner. 1975. Stochastic Differential Equations and Applications. New York: Academic Press. [Google Scholar]
- Guerra, Manuel, and Maria de Lourdes Centeno. 2008. Optimal reinsurance policy: The adjustment coefficient and the expected utility criteria. Insurance: Mathematics and Economics 42: 529–39. [Google Scholar] [CrossRef]
- Hata, Hiroaki, and Kazuhiro Yasuda. 2017. Expected exponential utility maximization of insurers with a linear Gaussian stochastic factor model. Scandinavian Actuarial Journal, 1–22. [Google Scholar] [CrossRef]
- Henderson, Vicky. 2005. Explicit solutions to an optimal portfolio choice problem with stochastic income. Journal of Economic Dynamics & Control 29: 1237–66. [Google Scholar]
- Karatzas, Ioannis, and Steven Shreve. 1988. Brownian Motion and Stochastic Calculus. New York: Springer. [Google Scholar]
- Kloeden, Peter E., and Eckhard Platen. 1992. Numerical Solution of Stochastic Differential Equations. Berlin: Springer. [Google Scholar]
- Liang, Zhibin, Kam Chuen Yuen, and Junyi Guo. 2011. Optimal proportional reinsurance and investment in a stock market with Ornstein-Uhlenbeck process. Insurance: Mathematics and Economics 49: 207–15. [Google Scholar] [CrossRef]
- Merton, Robert C. 1969. Lifetime portfolio selection under uncertainty: The continuous time case. The Review of Economics and Statistics 51: 247–57. [Google Scholar] [CrossRef]
- Merton, Robert C. 1971. Optimum consumption and portfolio rules in a continuous time model. Journal of Economic Theory 3: 373–413. [Google Scholar] [CrossRef]
- Øksendal, Bernt. 2003. Stochastic Differential Equations: An Introduction with Application. Berlin: Springer. [Google Scholar]
- Schlesinger, Harris, and Neil A. Doherty. 1985. Incomplete markets for insurance: An overview. The Journal of Risk and Insurance 52: 402–23. [Google Scholar] [CrossRef]
- Scott, Louis O. 1997. Pricing stock options in a jump-diffusion model with stochastic volatility and interest rates: Applications of Fourier inversion methods. Mathematical Finance 7: 413–26. [Google Scholar] [CrossRef]
- Talay, Denis. 1996. Probabilistic numerical methods for partial differential equations. Elements of Analysis. In Lecture Notes in Mathematics. Berlin: Springer, pp. 148–196. [Google Scholar]
- Wang, Nan. 2007. Optimal investment for an insurer with exponential utility preference. Insurance: Mathematics and Economics 40: 77–84. [Google Scholar] [CrossRef]
- Yang, Hailiang, and Lihong Zhang. 2005. Optimal investment for insurer with jump-diffusion risk process. Insurance: Mathematics and Economics 37: 615–34. [Google Scholar] [CrossRef]
- Zariphopoulou, Thaleia. 2001. A solution approach to valuation with unhedgeable risks. Finance Stochast 5: 61–82. [Google Scholar] [CrossRef]
- Zou, Bin, and Abel Cadenillas. 2014. Optimal investment and risk control policies for an insurer: Expected utility maximization. Insurance: Mathematics and Economics 58: 57–67. [Google Scholar] [CrossRef]



| c | T | r | a | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| 5 | 3 | 1 | 0.3 | 0.04 | 0.02 | 0.3 | 0.1 | 0 | 2 | 1 |
| c | r | z | |||||||
|---|---|---|---|---|---|---|---|---|---|
| 5 | 3 | 0.3 | 0 | 0.3 | 0.1 | 0 | 2 | 0 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Badaoui, M.; Fernández, B.; Swishchuk, A. An Optimal Investment Strategy for Insurers in Incomplete Markets. Risks 2018, 6, 31. https://doi.org/10.3390/risks6020031
Badaoui M, Fernández B, Swishchuk A. An Optimal Investment Strategy for Insurers in Incomplete Markets. Risks. 2018; 6(2):31. https://doi.org/10.3390/risks6020031
Chicago/Turabian StyleBadaoui, Mohamed, Begoña Fernández, and Anatoliy Swishchuk. 2018. "An Optimal Investment Strategy for Insurers in Incomplete Markets" Risks 6, no. 2: 31. https://doi.org/10.3390/risks6020031
APA StyleBadaoui, M., Fernández, B., & Swishchuk, A. (2018). An Optimal Investment Strategy for Insurers in Incomplete Markets. Risks, 6(2), 31. https://doi.org/10.3390/risks6020031






