The Convergence Rate of Option Prices in Trinomial Trees
Abstract
1. Introduction
2. -Nomial Models
3. The Main Theorem
4. Verification of the Result
4.1. Comparison with Chang and Palmer’s Binomial Model
4.2. Application of Theorem 1 to Trinomial Models
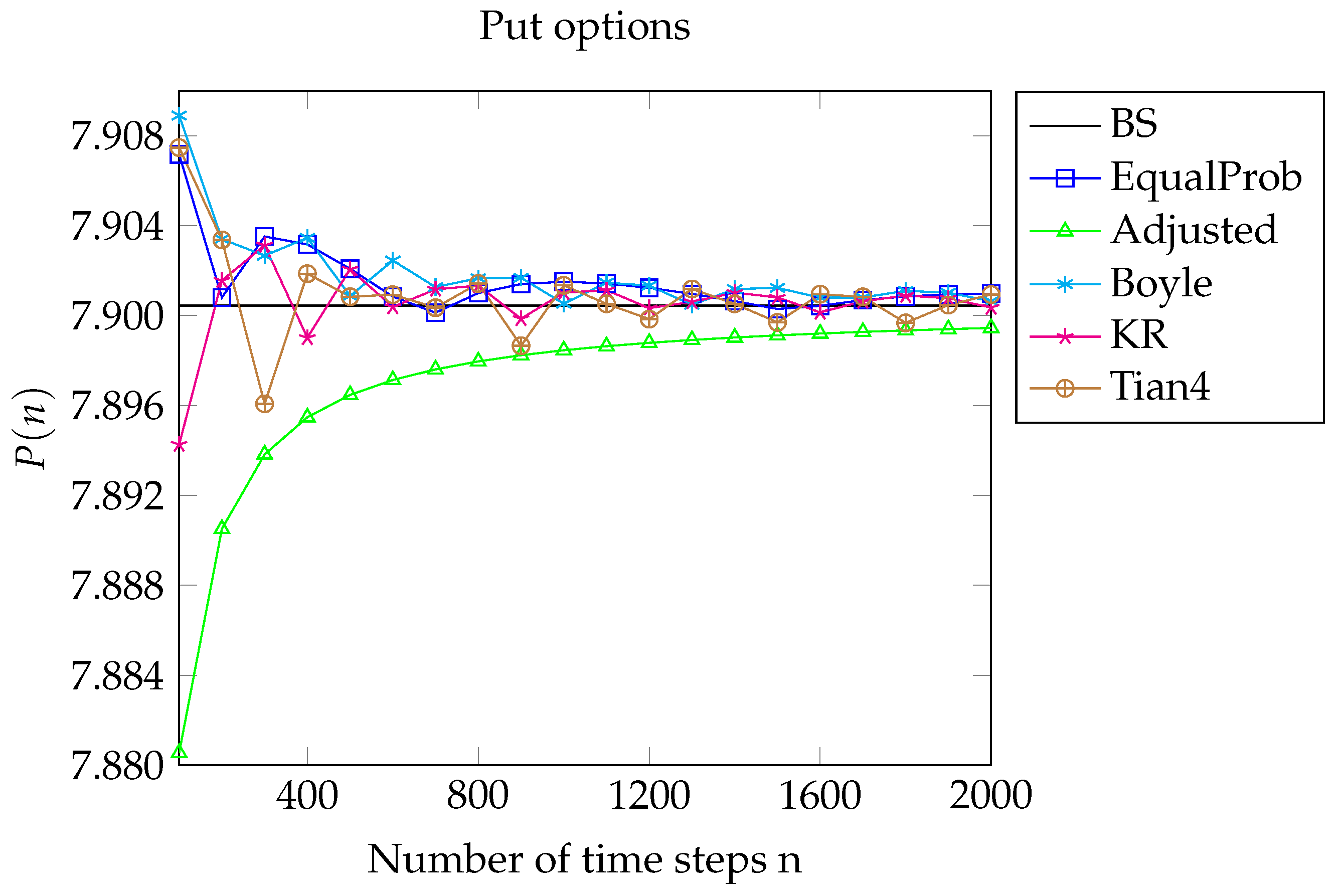
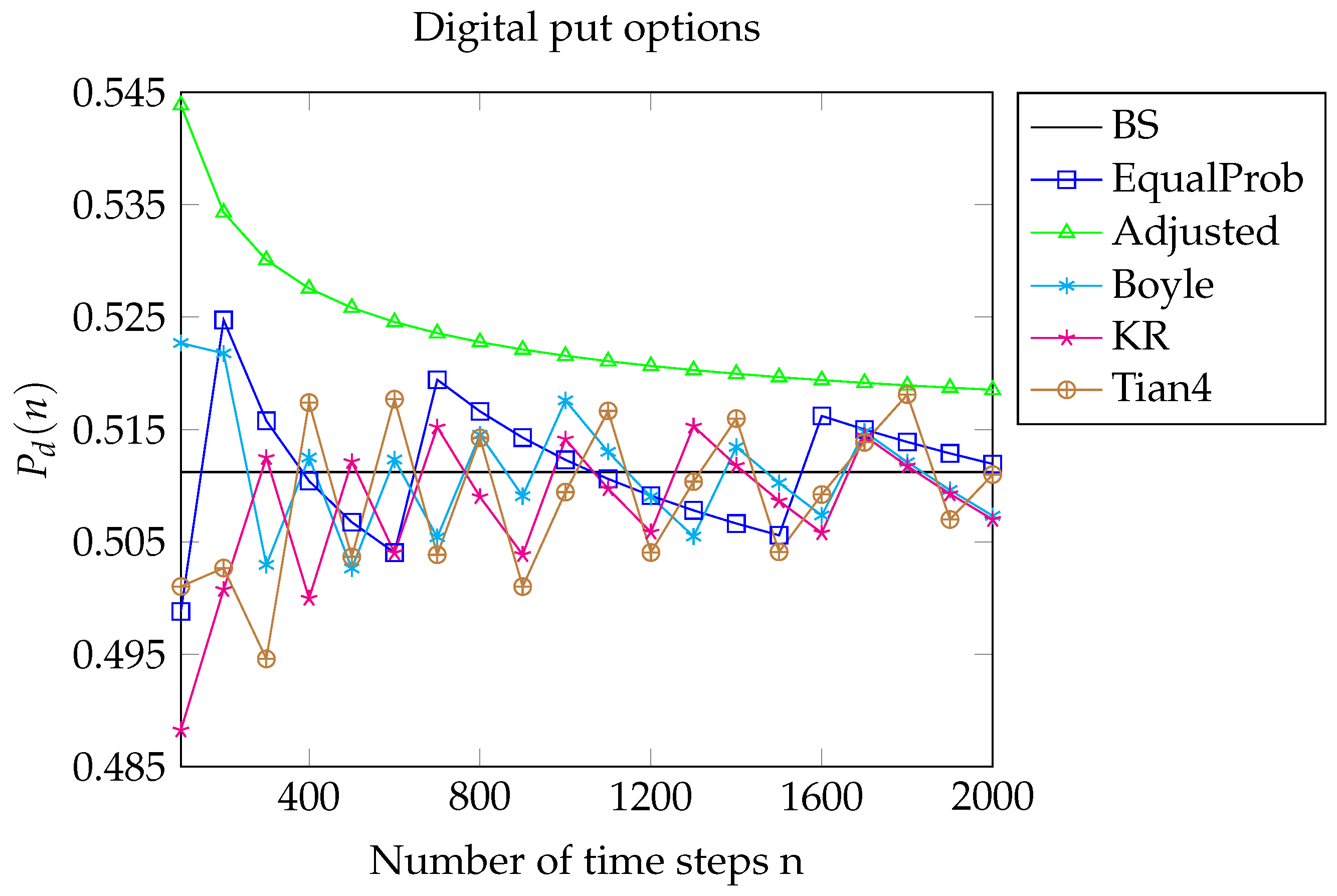
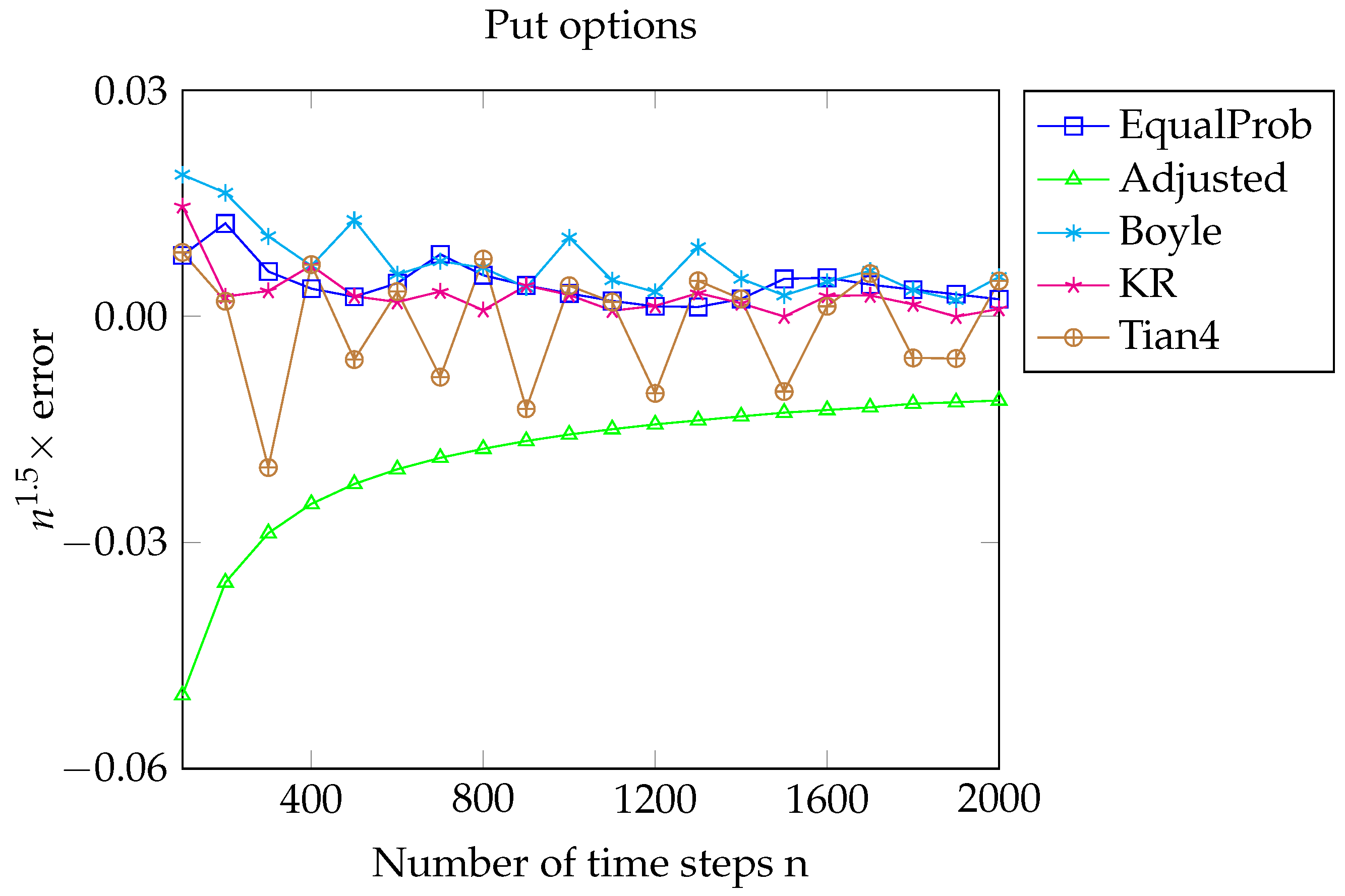
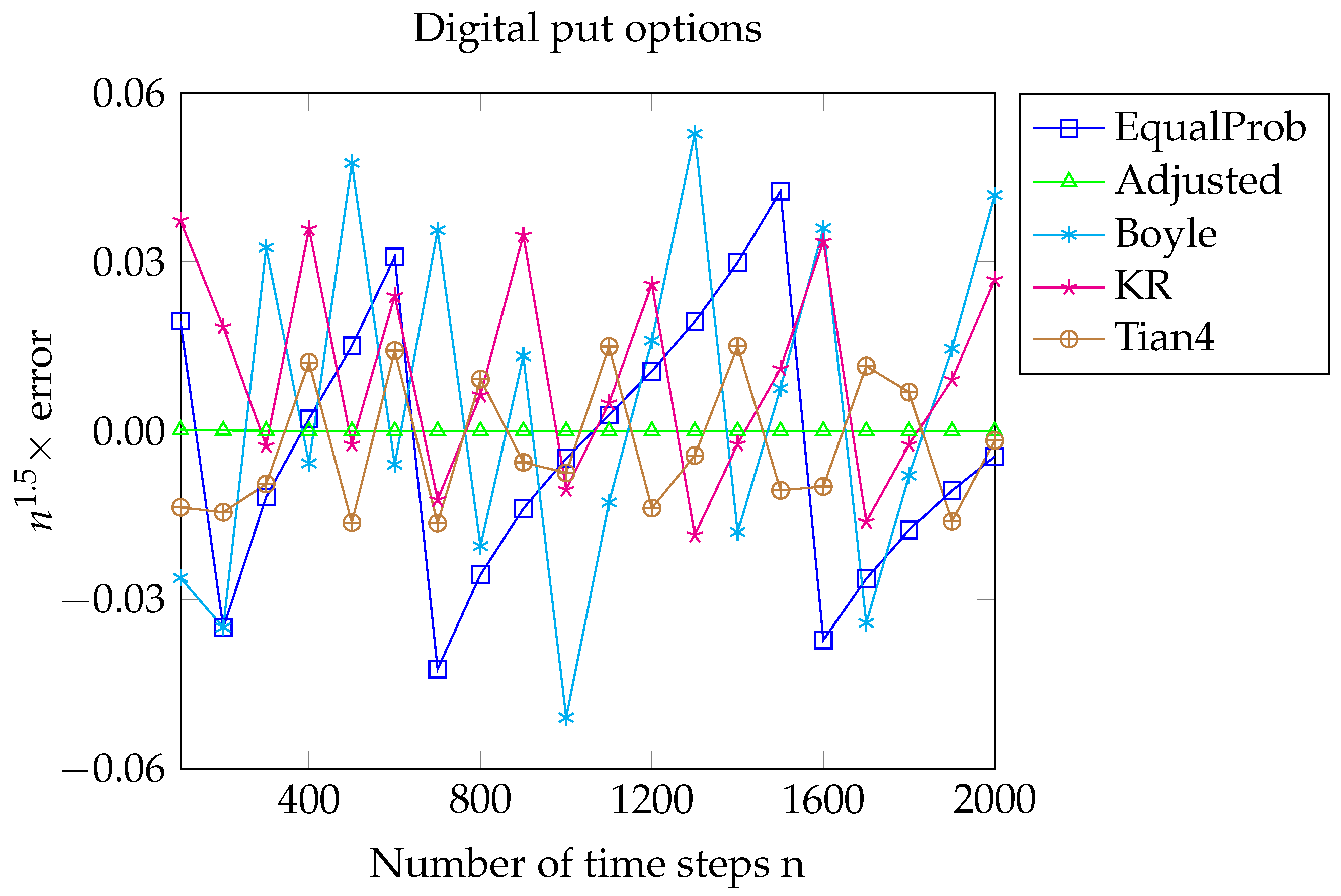
4.3. Numerical Results for the Trinomial Models
5. Proof of Theorem 1
6. Proof of Theorem 2
7. Proof of Theorem 3
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
Appendix A.1. Properties of
- (i)
- , , and for , is a polynomial of degree at most ; in fact, for and ;
- (ii)
- the are bounded as functions of n.
Appendix A.2. On the Power Series of the Exponential of a Power Series
Appendix A.3. Properties of ψ
- (i)
- If for ,
- (ii)
- If is bounded and for , then for ,
- (iii)
- If is bounded and for , where α is real, then
- (iv)
- Suppose that
Appendix A.4. Replacing the Variance by the Sheppard-Corrected Variance in ψ
References
- Akyıldırım, Erdinç, Yan Dolinsky, and H. Mete Soner. 2014. Approximating stochastic volatility by recombinant trees. The Annals of Applied Probability 24: 2176–205. [Google Scholar] [CrossRef]
- Appolloni, Elisa, Marcellino Gaudenzi, and Antonino Zanette. 2014. The binomial interpolated lattice method for step double barrier options. International Journal of Theoretical and Applied Finance 17: 1450035. [Google Scholar] [CrossRef]
- Bhattacharya, Rabi N., and R. Ranga Rao. 2010. Normal Approximation and Asymptotic Expansions. Philadelphia: SIAM. [Google Scholar]
- Bock, Alona. 2014. Edgeworth Expansions for Lattice Triangular Arrays. Report in Wirtschaftsmathematik (WIMA Report). Kaiserslautern: University of Kaiserslautern, vol. 149. [Google Scholar]
- Bock, Alona, and Ralf Korn. 2016. Improving convergence of binomial schemes and the Edgeworth expansion. Risks 4: 15. [Google Scholar] [CrossRef]
- Boyle, Phelim P. 1986. Option valuation using a three-jump process. International Options Journal 3: 7–12. [Google Scholar]
- Chan, Jiun Hong, Mark Joshi, Robert Tang, and Chao Yang. 2009. Trinomial or binomial: Accelerating American put option price on trees. Journal of Futures Markets 29: 826–39. [Google Scholar] [CrossRef]
- Chang, Lo-Bin, and Ken Palmer. 2007. Smooth convergence in the binomial model. Finance and Stochastics 11: 91–105. [Google Scholar] [CrossRef]
- Cox, John C., and Mark Rubinstein. 1985. Options Markets. Englewood Cliffs: Prentice-Hall. [Google Scholar]
- Diener, Francine, and Marc Diener. 2004. Asymptotics of the price oscillations of a European call option in a tree model. Mathematical Finance 14: 271–93. [Google Scholar] [CrossRef]
- Esseen, Carl-Gustav. 1945. Fourier analysis of distribution functions. A mathematical study of the Laplace-Gaussian law. Acta Mathematica 77: 1–125. [Google Scholar] [CrossRef]
- Gambaro, Anna Maria, Ioannis Kyriakou, and Gianluca Fusai. 2020. General lattice methods for arithmetic Asian options. European Journal of Operational Research 282: 1185–99. [Google Scholar] [CrossRef]
- Gaudenzi, Marcellino, and Antonino Zanette. 2017. Fast binomial procedures for pricing Parisian/ParAsian options. Computational Management Science 14: 313–31. [Google Scholar] [CrossRef]
- Grosse-Erdmann, Karl, and Fabien Heuwelyckx. 2016. The pricing of lookback options and binomial approximation. Decisions in Economics and Finance 39: 33–67. [Google Scholar] [CrossRef]
- Heuwelyckx, Fabien. 2014. Convergence of european lookback options with floating strike in the binomial model. International Journal of Theoretical and Applied Finance 17: 1450025. [Google Scholar] [CrossRef]
- Hsu, William W. Y., and Yuh-Dauh Lyuu. 2011. Efficient pricing of discrete Asian options. Applied Mathematics and Computation 217: 9875–94. [Google Scholar] [CrossRef]
- Joshi, Mark S. 2009a. Achieving smooth asymptotics for the prices of European options in binomial trees. Quantitative Finance 9: 171–76. [Google Scholar] [CrossRef]
- Joshi, Mark S. 2009b. The convergence of binomial trees for pricing the American put. The Journal of Risk 11: 87–108. [Google Scholar] [CrossRef]
- Joshi, Mark S. 2010. Achieving higher order convergence for the prices of European options in binomial trees. Mathematical Finance 20: 89–103. [Google Scholar] [CrossRef]
- Kamrad, Bardia, and Peter Ritchken. 1991. Multinomial approximating models for options with k state variables. Management Science 37: 1640–52. [Google Scholar] [CrossRef]
- Klassen, Timothy. 2001. Simple, fast and flexible pricing of Asian options. Journal of Computational Finance 4: 89–124. [Google Scholar] [CrossRef]
- Kolassa, John E., and Peter McCullagh. 1990. Edgeworth series for lattice distributions. The Annals of Statistics 18: 981–85. [Google Scholar] [CrossRef]
- Korn, Ralf, and Stefanie Müller. 2013. The optimal-drift model: An accelerated binomial scheme. Finance and Stochastics 17: 135–60. [Google Scholar] [CrossRef]
- Lamberton, Damien. 1998. Error estimates for the binomial approximation of American put options. Annals of Applied Probability 8: 206–33. [Google Scholar] [CrossRef]
- Lamberton, Damien. 2002. Brownian optimal stopping and random walks. Applied Mathematics and Optimization 45: 283–324. [Google Scholar] [CrossRef]
- Lamberton, Damien. 2020. On the binomial approximation of the American put. Applied Mathematics & Optimization 82: 687–720. [Google Scholar]
- Leduc, Guillaume. 2013. A European option general first-order error formula. The ANZIAM Journal 54: 248–72. [Google Scholar] [CrossRef]
- Leduc, Guillaume. 2016. Can high-order convergence of European option prices be achieved with common CRR-type binomial trees? Bulletin of the Malaysian Mathematical Sciences Society 39: 1329–42. [Google Scholar] [CrossRef]
- Leduc, Guillaume, and Ken Palmer. 2019. Path independence of exotic options and convergence of binomial approximations. Journal of Computational Finance 23: 73–102. [Google Scholar] [CrossRef]
- Leduc, Guillaume, and Kenneth Palmer. 2020. What a difference one probability makes in the convergence of binomial trees. International Journal of Theoretical and Applied Finance 23: 1–26. [Google Scholar] [CrossRef]
- Leduc, Guillaume, and Merima Nurkanovic Hot. 2020. Joshi’s split tree for option pricing. Risks 8: 81. [Google Scholar] [CrossRef]
- Leduc, Guillaume, and Xiangchen Zeng. 2017. Convergence rate of regime-switching trees. Journal of Computational and Applied Mathematics 319: 56–76. [Google Scholar] [CrossRef]
- Leisen, Dietmar P. J. 1998. Pricing the American put option: A detailed convergence analysis for binomial models. Journal of Economic Dynamics and Control 22: 1419–44. [Google Scholar] [CrossRef]
- Li, Lingfei, and Gongqiu Zhang. 2018. Error analysis of finite difference and Markov chain approximations for option pricing. Mathematical Finance 28: 877–919. [Google Scholar] [CrossRef]
- Liang, Jin, Bei Hu, Lishang Jiang, and Baojun Bian. 2007. On the rate of convergence of the binomial tree scheme for American options. Numerische Mathematik 107: 333–52. [Google Scholar] [CrossRef]
- Lin, Jhihrong, and Ken Palmer. 2013. Convergence of barrier option prices in the binomial model. Mathematical Finance 23: 318–38. [Google Scholar] [CrossRef]
- Liu, Ruihua. 2010. Regime-switching recombining tree for option pricing. International Journal of Theoretical and Applied Finance 13: 479–99. [Google Scholar] [CrossRef]
- Maller, Ross A., David H. Solomon, and Alex Szimayer. 2006. A multinomial approximation for American option prices in Lévy process models. Mathematical Finance 16: 613–33. [Google Scholar] [CrossRef]
- Muroi, Yoshifumi. 2020. Computation of Greeks Using the Discrete Malliavin Calculus and Binomial Tree. Singapore: Springer. [Google Scholar]
- Muroi, Yoshifumi, and Shintaro Suda. 2022. Binomial tree method for option pricing: Discrete cosine transform approach. Mathematics and Computers in Simulation 198: 312–31. [Google Scholar] [CrossRef]
- Smith, Peter J. 1995. A recursive formulation of the old problem of obtaining moments from cumulants and vice versa. The American Statistician 49: 217–18. [Google Scholar]
- Tian, Yisong. 1993. A modified lattice approach to option pricing. Journal of Futures Markets 13: 563–77. [Google Scholar] [CrossRef]
- Uspensky, James Victor. 1937. Introduction to Mathematical Probability. New York: McGraw-Hill. [Google Scholar]
- Walsh, John B. 2003. The rate of convergence of the binomial tree scheme. Finance and Stochastics 7: 337–61. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Leduc, G.; Palmer, K. The Convergence Rate of Option Prices in Trinomial Trees. Risks 2023, 11, 52. https://doi.org/10.3390/risks11030052
Leduc G, Palmer K. The Convergence Rate of Option Prices in Trinomial Trees. Risks. 2023; 11(3):52. https://doi.org/10.3390/risks11030052
Chicago/Turabian StyleLeduc, Guillaume, and Kenneth Palmer. 2023. "The Convergence Rate of Option Prices in Trinomial Trees" Risks 11, no. 3: 52. https://doi.org/10.3390/risks11030052
APA StyleLeduc, G., & Palmer, K. (2023). The Convergence Rate of Option Prices in Trinomial Trees. Risks, 11(3), 52. https://doi.org/10.3390/risks11030052





