Experimental Study and Mathematical Modeling of a Glyphosate Impedimetric Microsensor Based on Molecularly Imprinted Chitosan Film
Abstract
1. Introduction
2. Materials and Methods
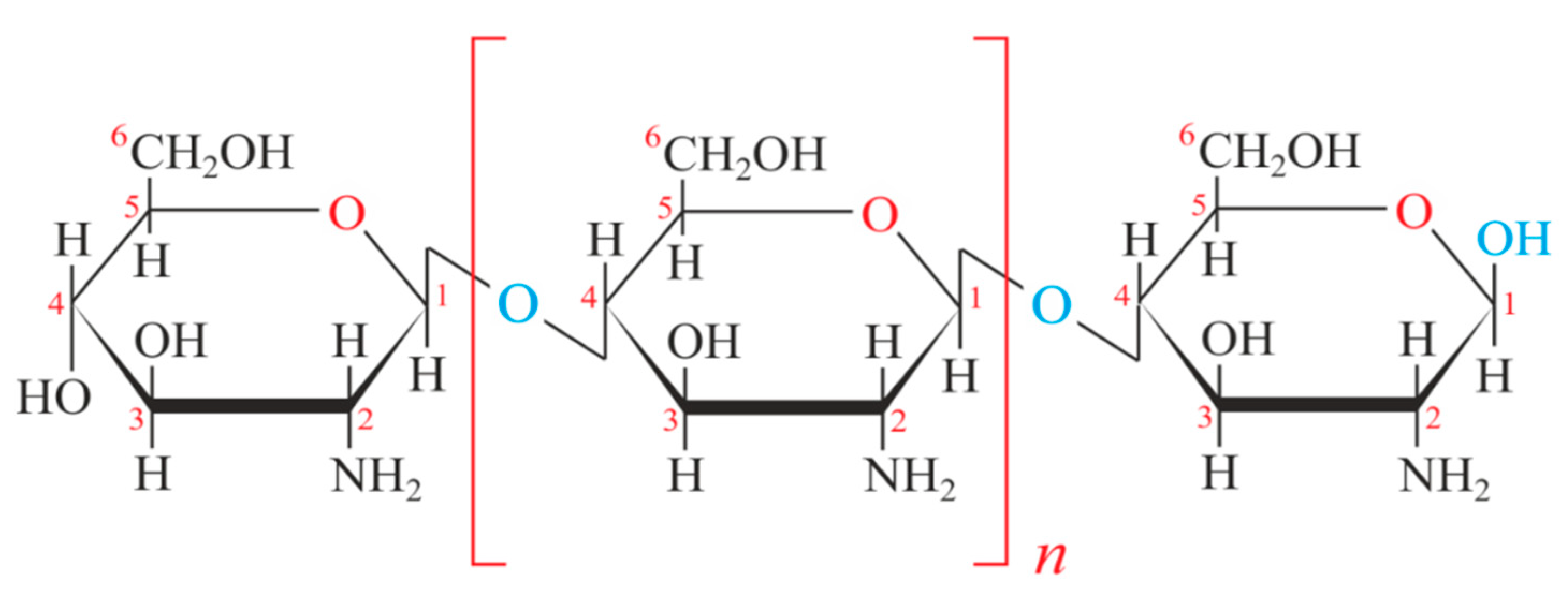
2.1. Reagents
2.2. Apparatus
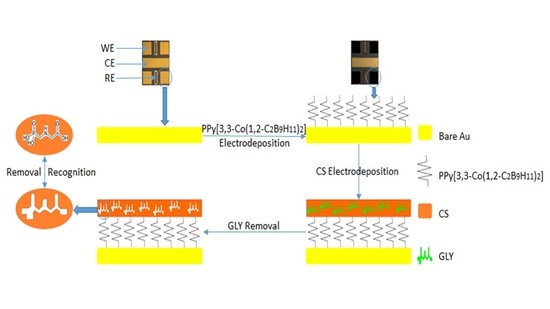
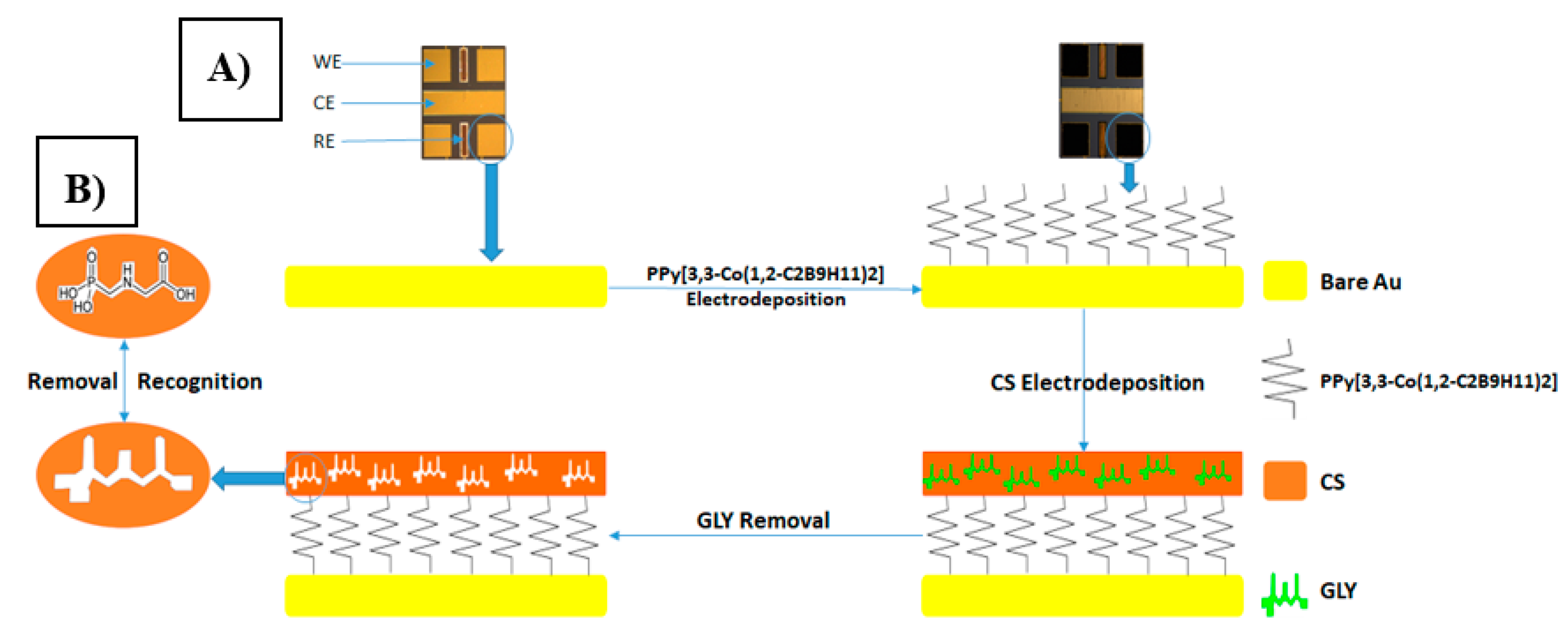
2.3. Preparation CS-MIPs/PPy/Au Sensor
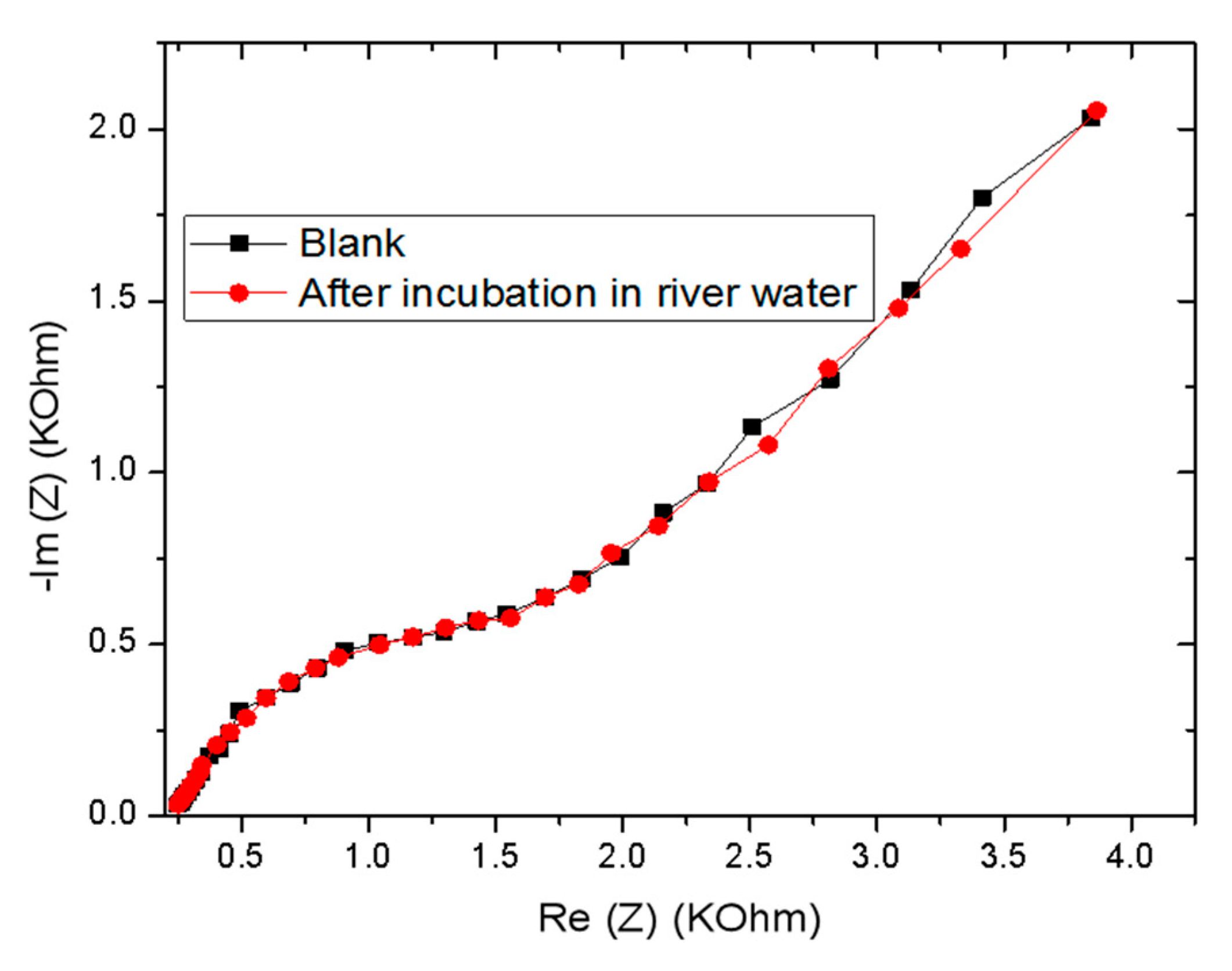
2.4. Electrochemical Measurements
3. Results and Discussion
3.1. Microsensors Characterization
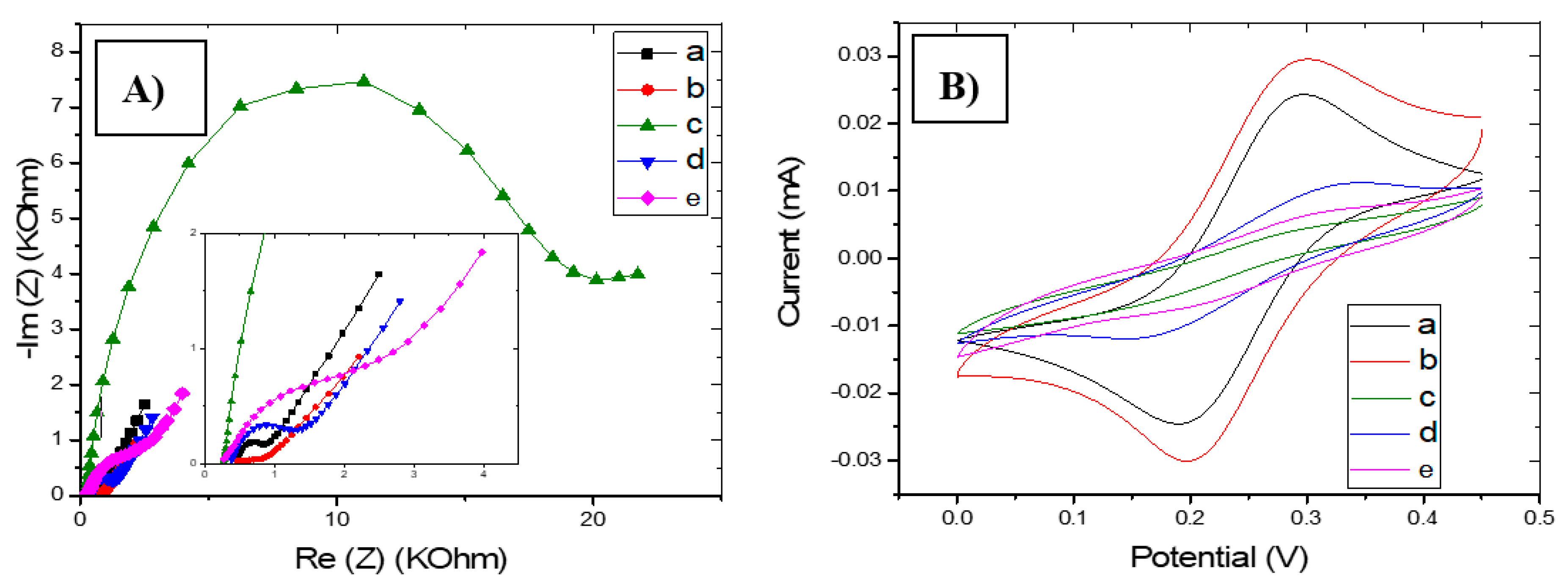
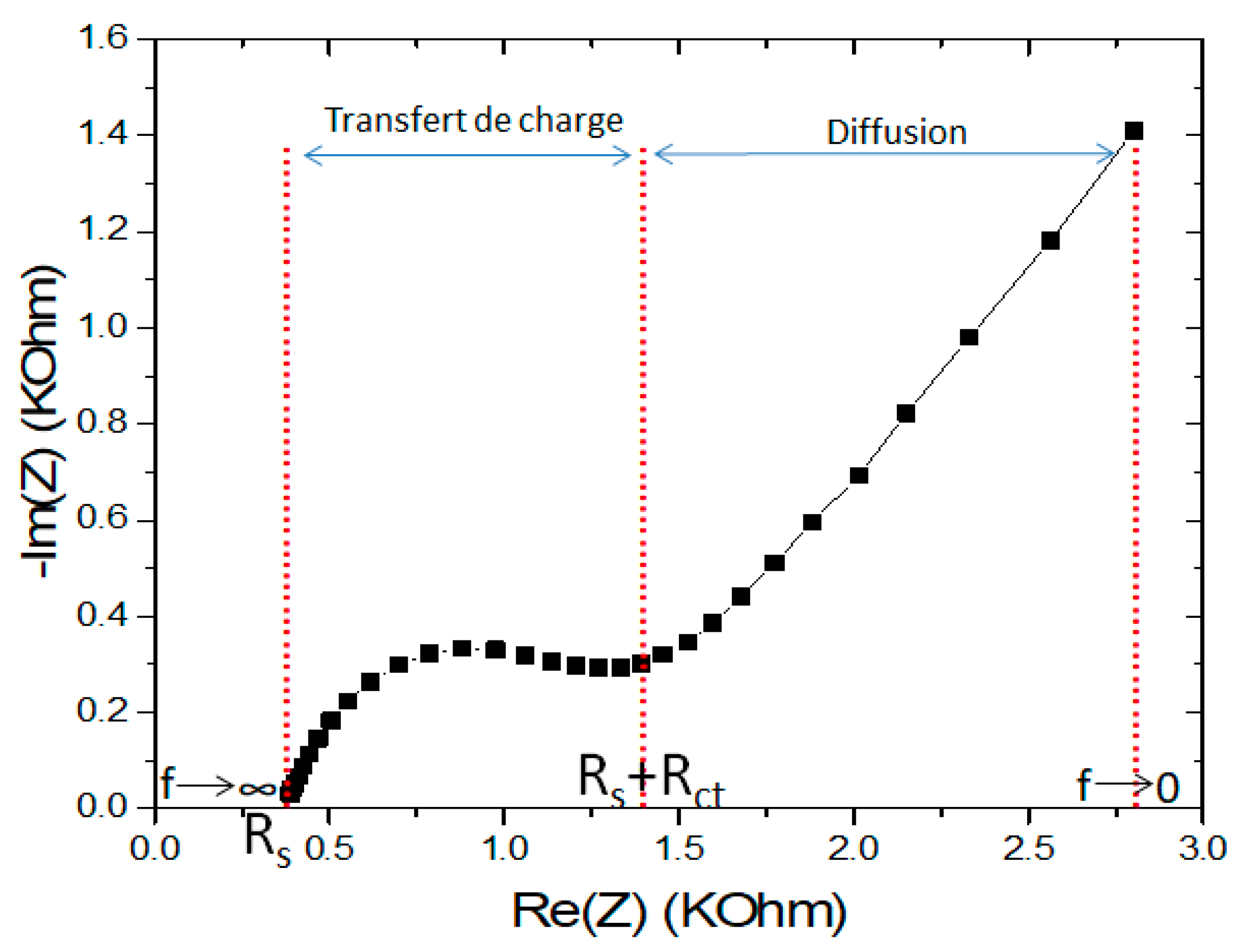
3.1.1. Electrochemical Characterization of CS-MIPs/PPy/Au
3.1.2. Surface Morphology
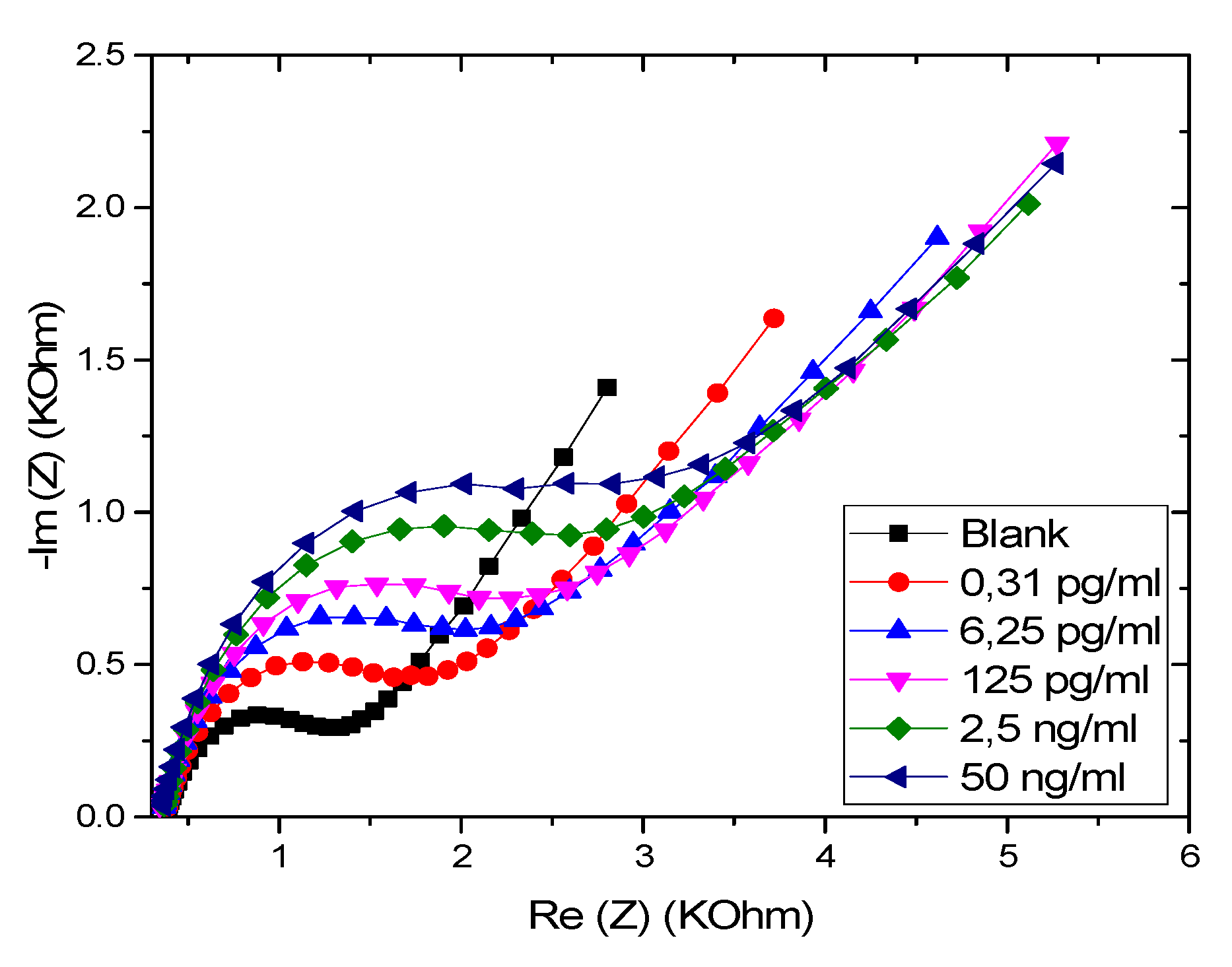
3.2. Electrochemical Responses of the CS-MIPs/PPy/Au
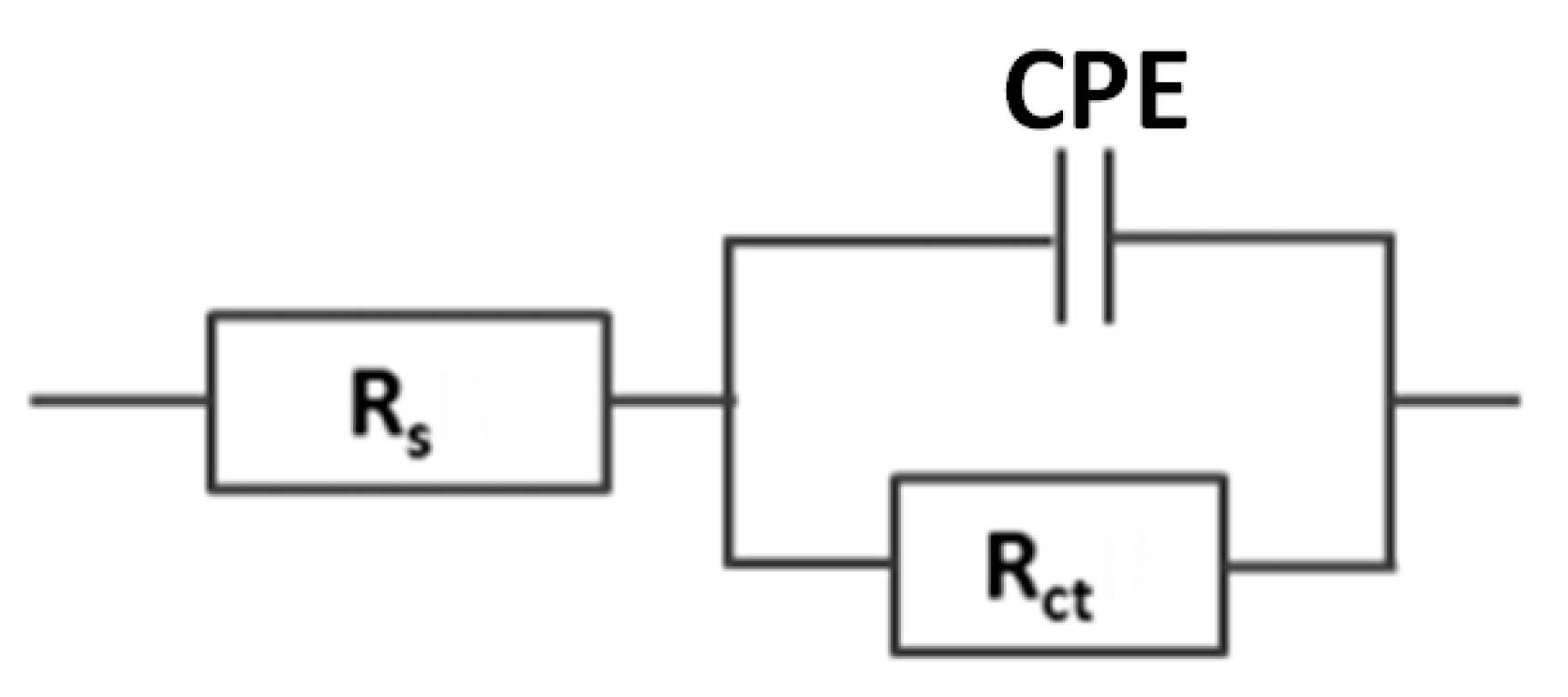
3.3. Modeling of the CS-MIPs/PPy/Au Microsensor
3.3.1. Mathematical Model
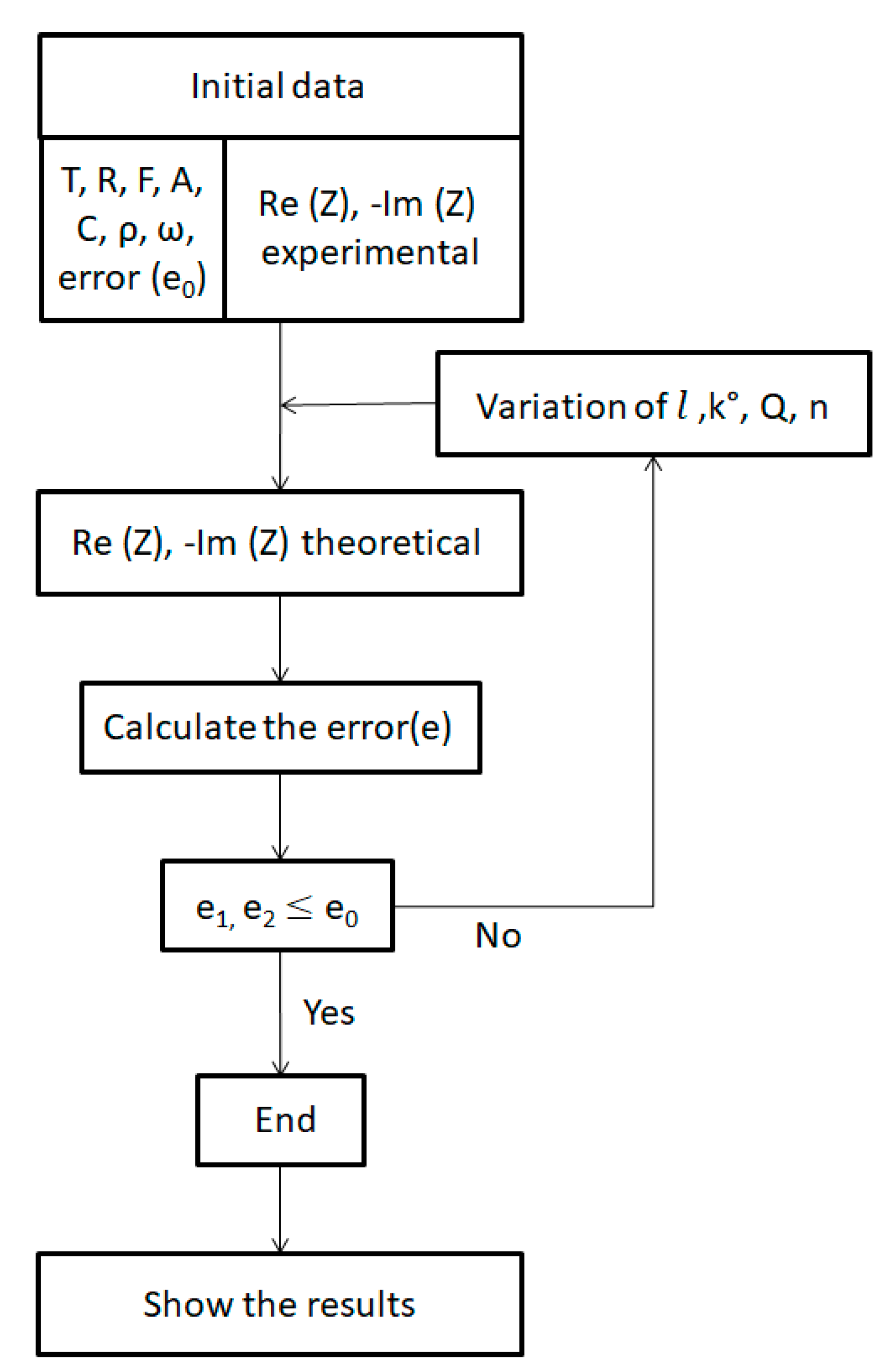
3.3.2. Numerical Simulation
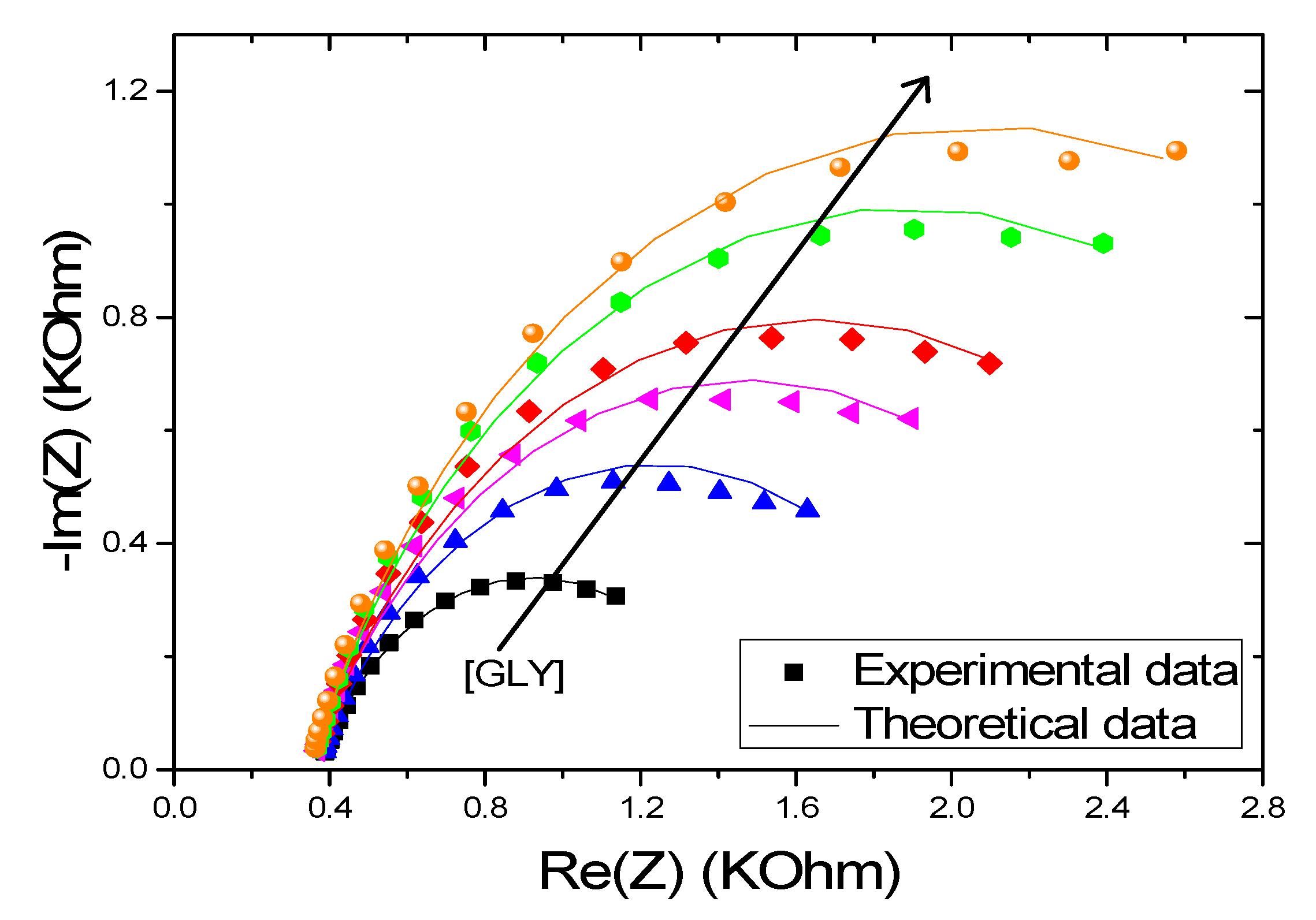
3.3.3. Model Validation
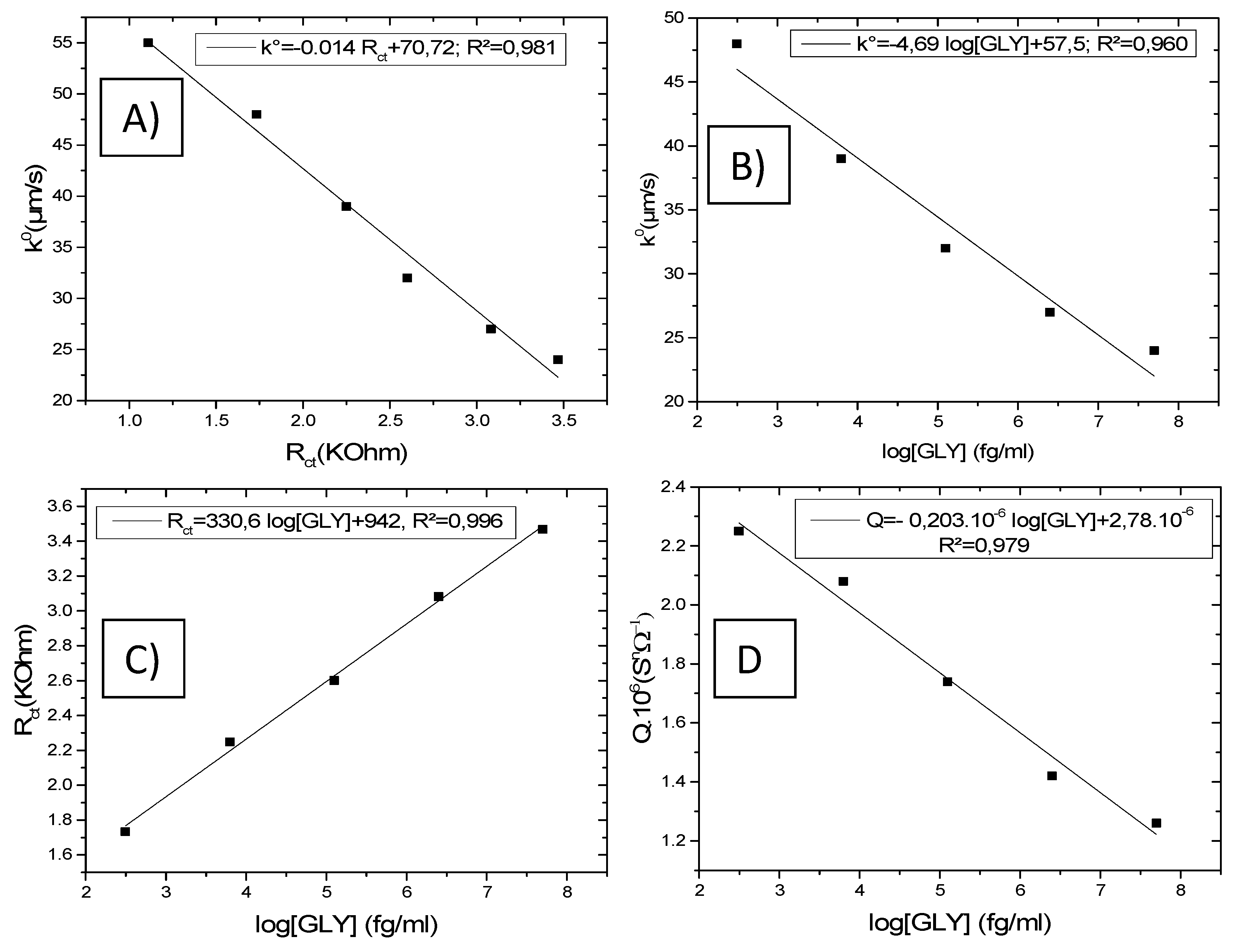
3.3.4. Analysis of Theoretical Results
3.4. Model Exploitation for Optimization of the GLY Microsensor
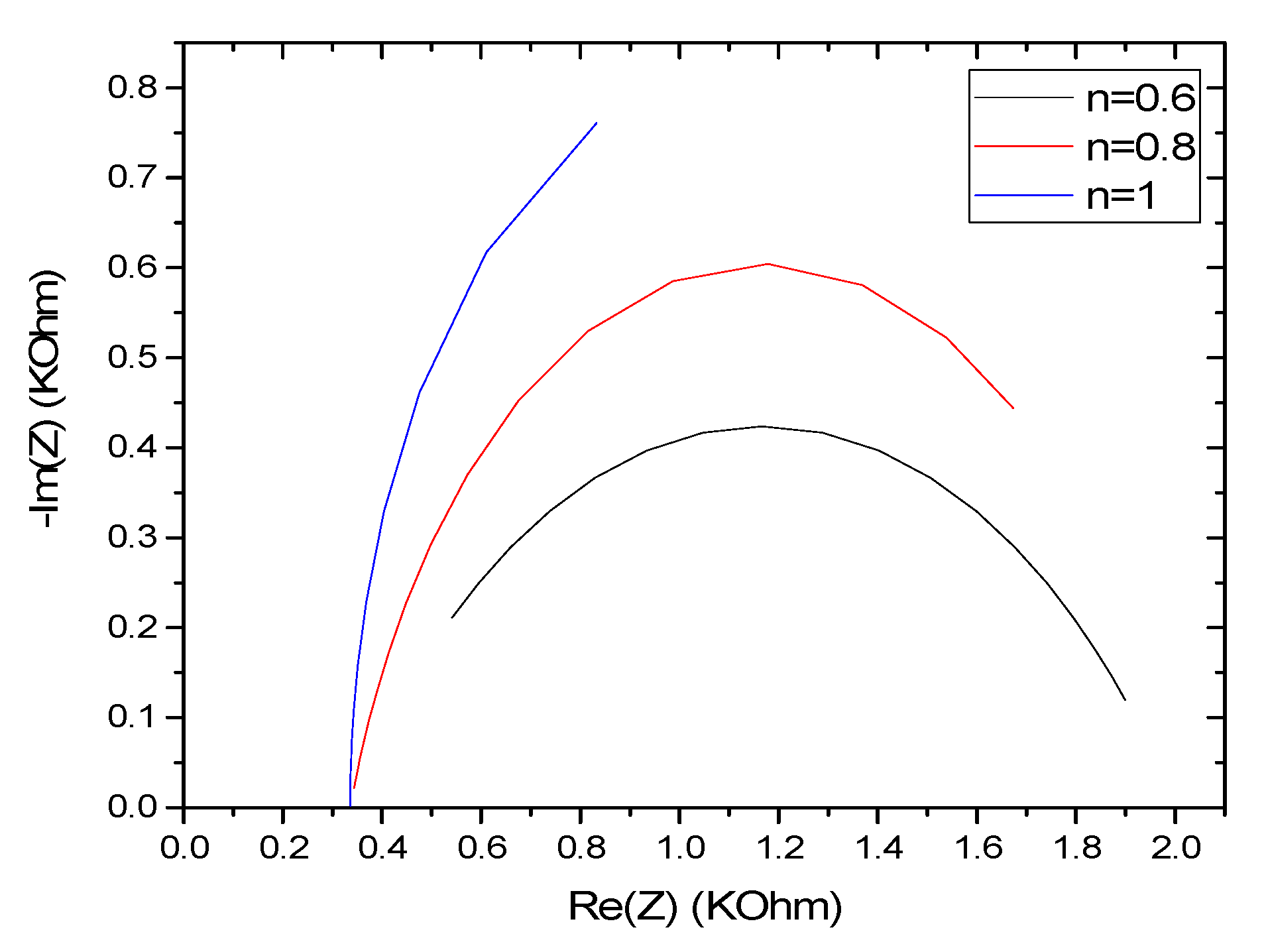
3.4.1. Effect of Coefficient n
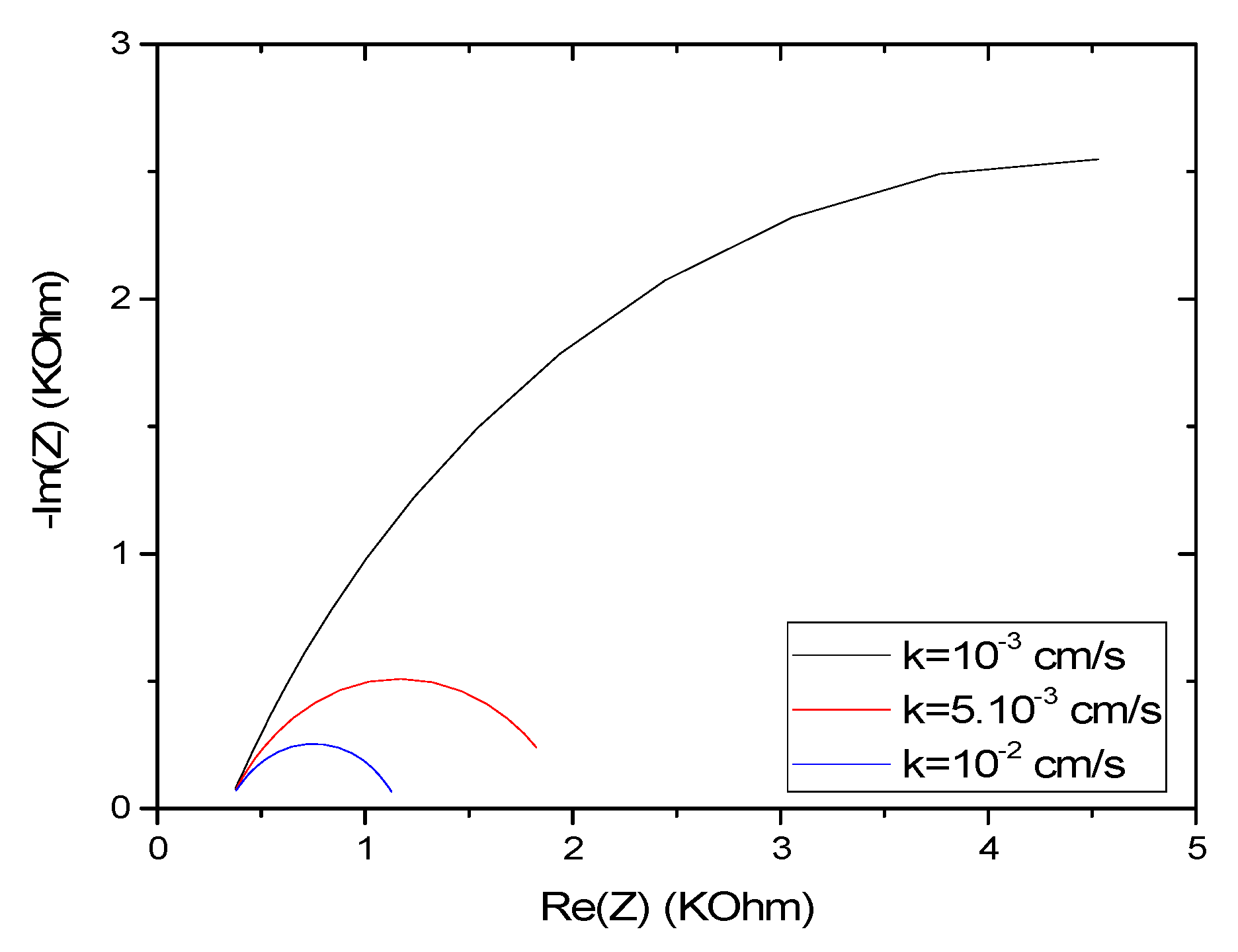
3.4.2. Effect of Electron Transfer Rate Constant k°
3.4.3. Effect of the CPE Coefficient (Q)
3.4.4. Effects of Membrane Thickness and Surface of CS-MIPs
3.4.5. Effects of Temperature and Concentration of the Ferri/Ferrocyanide Solution
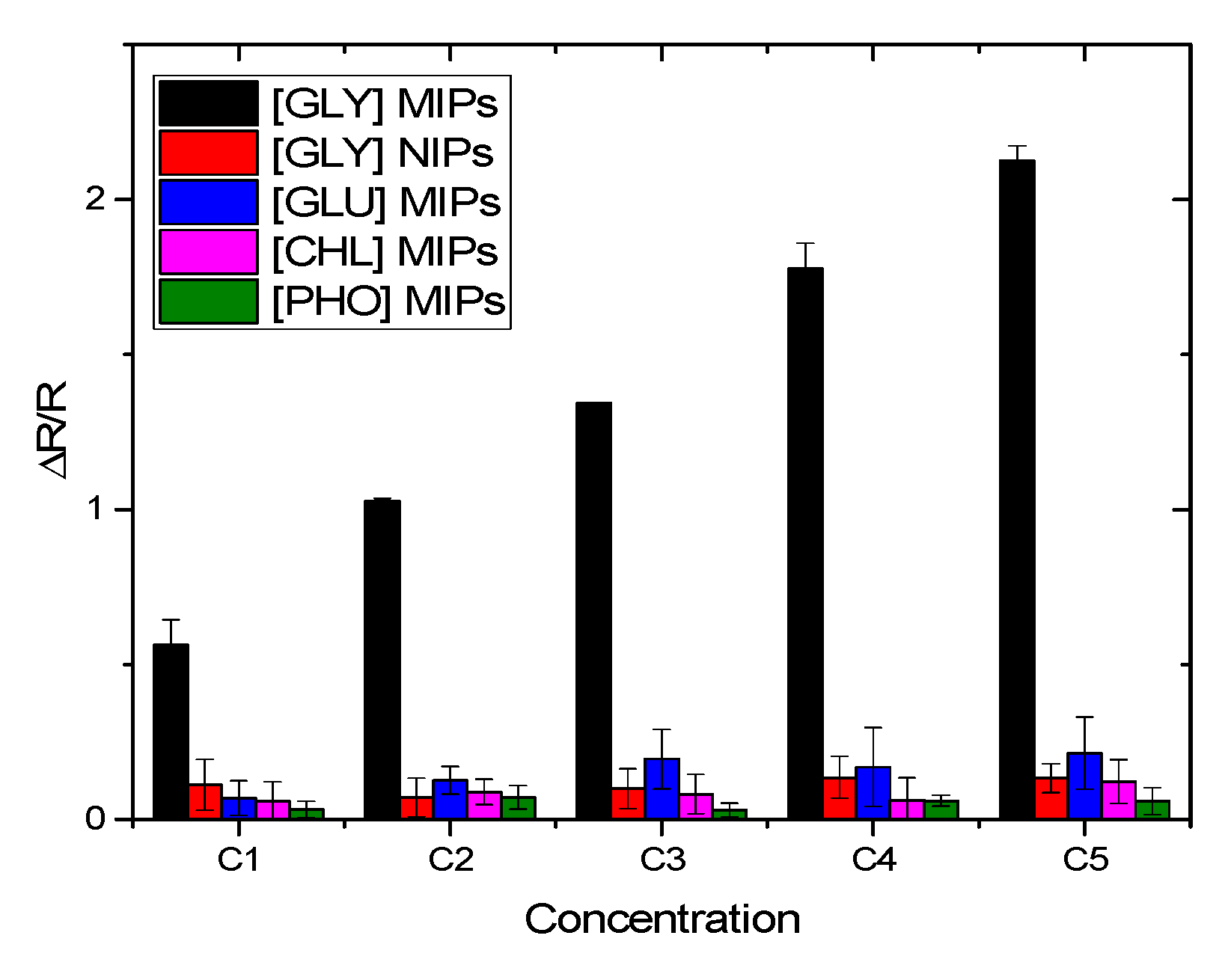
3.5. Analytical Performances of the CS-MIPs/PPy Functionalized Gold Electrode
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Conflicts of Interest
References
- Van Bruggen, A.H.C.; He, M.M.; Shin, K.; Mai, V.; Jeong, K.C.; Finckh, M.R.; Morris, J.G. Environmental and health effects of the herbicide glyphosate. Sci. Total Environ. 2018, 616–617, 255–268. [Google Scholar] [CrossRef] [PubMed]
- Krüger, M.; Schledorn, P.; Schrödl, W.; Hoppe, H.-W.; Lutz, W.; Shehata, A.A. Detection of glyphosate residues in animals and humans. J. Environ. Anal. Toxicol. 2014, 4, 1–5. [Google Scholar]
- Gasnier, C.; Dumont, C.; Benachour, N.; Clair, E.; Chagnon, M.-C.; Séralini, G.-E. Glyphosate-based herbicides are toxic and endocrine disruptors in human cell lines. Toxicology 2009, 262, 184–191. [Google Scholar] [CrossRef] [PubMed]
- Ren, X.; Li, R.; Liu, J.; Huang, K.; Wu, S.; Li, Y.; Li, C. Effects of glyphosate on the ovarian function of pregnant mice, the secretion of hormones and the sex ratio of their fetuses. Environ. Pollut. 2018, 243, 833–841. [Google Scholar] [CrossRef] [PubMed]
- Wang, L.; Bi, Y.; Hou, J.; Li, H.; Xu, Y.; Wang, B.; Ding, H.; Ding, L. Facile, green and clean one-step synthesis of carbon dots from wool: Application as a sensor for glyphosate detection based on the inner filter effect. Talanta 2016, 160, 268–275. [Google Scholar] [CrossRef] [PubMed]
- Ciasca, B.; Pecorelli, I.; Lepore, L.; Paoloni, A.; Catucci, L.; Pascale, M.; Lattanzio, V.M.T. Rapid and reliable detection of glyphosate in pome fruits, berries, pulses and cereals by flow injection—Mass spectrometry. Food Chem. 2020, 310, 125813. [Google Scholar] [CrossRef]
- Wang, D.; Lin, B.; Cao, Y.; Guo, M.; Yu, Y. A Highly Selective and Sensitive Fluorescence Detection Method of Glyphosate Based on an Immune Reaction Strategy of Carbon Dot Labeled Antibody and Antigen Magnetic Beads. J. Agric. Food Chem. 2016, 64, 6042–6050. [Google Scholar] [CrossRef]
- Pichon, V.; Chapuis-Hugon, F. Role of molecularly imprinted polymers for selective determination of environmental pollutants—A review. Anal. Chim. Acta 2008, 622, 48–61. [Google Scholar] [CrossRef]
- Qiao, F.; Sun, H.; Yan, H.; Row, K.H. Molecularly Imprinted Polymers for Solid Phase Extraction. Chromatographia 2006, 64, 625–634. [Google Scholar] [CrossRef]
- Haupt, K.; Mosbach, K. Molecularly Imprinted Polymers and Their Use in Biomimetic Sensors. Chem. Rev. 2000, 100, 2495–2504. [Google Scholar] [CrossRef]
- Cheong, W.J.; Yang, S.H.; Ali, F. Molecular imprinted polymers for separation science: A review of reviews: Other Techniques. J. Sep. Sci. 2013, 36, 609–628. [Google Scholar] [CrossRef] [PubMed]
- El Knidri, H.; Belaabed, R.; Addaou, A.; Laajeb, A.; Lahsini, A. Extraction, chemical modification and characterization of chitin and chitosan. Int. J. Biol. Macromol. 2018, 120, 1181–1189. [Google Scholar] [CrossRef]
- Zouaoui, F.; Bourouina-Bacha, S.; Bourouina, M.; Jaffrezic-Renault, N.; Zine, N.; Errachid, A. Electrochemical sensors based on molecularly imprinted chitosan: A review. TrAC Trends Anal. Chem. 2020, 115982. [Google Scholar] [CrossRef]
- Wang, J.; Xu, Y.; Chen, X.; Sun, X. Capacitance properties of single wall carbon nanotube/polypyrrole composite films. Compos. Sci. Technol. 2007, 67, 2981–2985. [Google Scholar] [CrossRef]
- Wang, L.-X.; Li, X.-G.; Yang, Y.-L. Preparation, properties and applications of polypyrroles. React. Funct. Polym. 2001, 47, 125–139. [Google Scholar] [CrossRef]
- Zine, N.; Bausells, J.; Vocanson, F.; Lamartine, R.; Asfari, Z.; Teixidor, F.; Crespo, E.; de Oliveira, I.A.M.; Samitier, J.; Errachid, A. Potassium-ion selective solid contact microelectrode based on a novel 1,3-(di-4-oxabutanol)-calix[4]arene-crown-5 neutral carrier. Electrochim. Acta 2006, 51, 5075–5079. [Google Scholar] [CrossRef]
- Chen, J.H.; Huang, Z.P.; Wang, D.Z.; Yang, S.X.; Li, W.Z.; Wen, J.G.; Ren, Z.F. Electrochemical synthesis of polypyrrole films over each of well-aligned carbon nanotubes. Synth. Met. 2001, 125, 289–294. [Google Scholar] [CrossRef]
- Rais, J.; Selucký, P.; Kyrš, M. Extraction of alkali metals into nitrobenzene in the presence of univalent polyhedral borate anions. J. Inorg. Nucl. Chem. 1976, 38, 1376–1378. [Google Scholar] [CrossRef]
- Biloivan, O.A.; Dzyadevych, S.V.; El’skaya, A.V.; Jaffrezic-Renault, N.; Zine, N.; Bausells, J.; Samitier, J.; Errachid, A. Development of bi-enzyme microbiosensor based on solid-contact ion-selective microelectrodes for protein detection. Sens. Actuators B Chem. 2007, 123, 1096–1100. [Google Scholar] [CrossRef]
- Gallardo-Gonzalez, J.; Saini, A.; Baraket, A.; Boudjaoui, S.; Alcácer, A.; Streklas, A.; Teixidor, F.; Zine, N.; Bausells, J.; Errachid, A. A highly selective potentiometric amphetamine microsensor based on all-solid-state membrane using a new ion-pair complex, [3,3′-Co(1,2-closo-C2B9H11)2]− [C9H13NH]+. Sens. Actuators B Chem. 2018, 266, 823–829. [Google Scholar] [CrossRef]
- Zouaoui, F.; Bourouina-Bacha, S.; Bourouina, M.; Abroa-Nemeir, I.; Ben Halima, H.; Gallardo-Gonzalez, J.; El Alami El Hassani, N.; Alcacer, A.; Bausells, J.; Jaffrezic-Renault, N.; et al. Electrochemical impedance spectroscopy determination of glyphosate using a molecularly imprinted chitosan. Sens. Actuators B Chem. 2020, 309, 127753. [Google Scholar] [CrossRef]
- Pajkossy, T.; Jurczakowski, R. Electrochemical impedance spectroscopy in interfacial studies. Curr. Opin. Electrochem. 2017, 1, 53–58. [Google Scholar] [CrossRef]
- Randviir, E.P.; Banks, C.E. Electrochemical impedance spectroscopy: An overview of bioanalytical applications. Anal. Methods 2013, 5, 1098. [Google Scholar] [CrossRef]
- Brug, G.; Van Den Eeden, A.; Sluyters-Rehbach, M.; Sluyters, J. The analysis of electrode impedances complicated by the presence of a constant phase element. J. Electroanal. Chem. 1984, 176, 275–295. [Google Scholar] [CrossRef]
- Huang, J.; Li, Z.; Liaw, B.Y.; Zhang, J. Graphical analysis of electrochemical impedance spectroscopy data in Bode and Nyquist representations. J. Power Sources 2016, 309, 82–98. [Google Scholar] [CrossRef]
- Ramaraja P Ramasamy, N.S. Electrochemical Impedance Spectroscopy for Microbial Fuel Cell Characterization. J. Microb. Biochem. Technol. 2013. [Google Scholar] [CrossRef]
- Hinderliter, B.R.; Croll, S.G.; Tallman, D.E.; Su, Q.; Bierwagen, G.P. Interpretation of EIS data from accelerated exposure of coated metals based on modeling of coating physical properties. Electrochim. Acta 2006, 51, 4505–4515. [Google Scholar] [CrossRef]
- GAMRY Instruments. Basics of Electrochemical Impedance Spectroscopy. Available online: https://www.c3-analysentechnik.de/downloads/applikationsberichte/gamry/5657-Application-Note-EIS.pdf (accessed on 20 October 2020).
- Lasia, A. Electrochemical impedance spectroscopy and its applications. In Modern Aspects of Electrochemistry; Springer: Berlin, Germany, 2002; pp. 143–248. [Google Scholar]
- Sellergren, B.; Shea, K.J. Influence of polymer morphology on the ability of imprinted network polymers to resolve enantiomers. J. Chromatogr. A 1993, 635, 31–49. [Google Scholar] [CrossRef]
- Fellner, P.; Matiašovský, K. On the temperature dependence of the double-layer capacitance. Electrochim. Acta 1972, 17, 233–236. [Google Scholar] [CrossRef]
- Pangarov, N.; Christova, I.; Atanasov, M.; Kertov, V. Double-layer capacitance measurements by a pulse potentiostatic method. Electrochim. Acta 1967, 12, 717–721. [Google Scholar] [CrossRef]
- De Levie, R. On porous electrodes in electrolyte solutions: I. Capacitance effects. Electrochim. Acta 1963, 8, 751–780. [Google Scholar] [CrossRef]
- Prasad, B.B.; Jauhari, D.; Tiwari, M.P. Doubly imprinted polymer nanofilm-modified electrochemical sensor for ultra-trace simultaneous analysis of glyphosate and glufosinate. Biosens. Bioelectron. 2014, 59, 81–88. [Google Scholar] [CrossRef] [PubMed]
- Mazouz, Z.; Rahali, S.; Fourati, N.; Zerrouki, C.; Aloui, N.; Seydou, M.; Yaakoubi, N.; Chehimi, M.; Othmane, A.; Kalfat, R. Highly Selective Polypyrrole MIP-Based Gravimetric and Electrochemical Sensors for Picomolar Detection of Glyphosate. Sensors 2017, 17, 2586. [Google Scholar] [CrossRef] [PubMed]
- Xu, J.; Zhang, Y.; Wu, K.; Zhang, L.; Ge, S.; Yu, J. A molecularly imprinted polypyrrole for ultrasensitive voltammetric determination of glyphosate. Microchim. Acta 2017, 184, 1959–1967. [Google Scholar] [CrossRef]

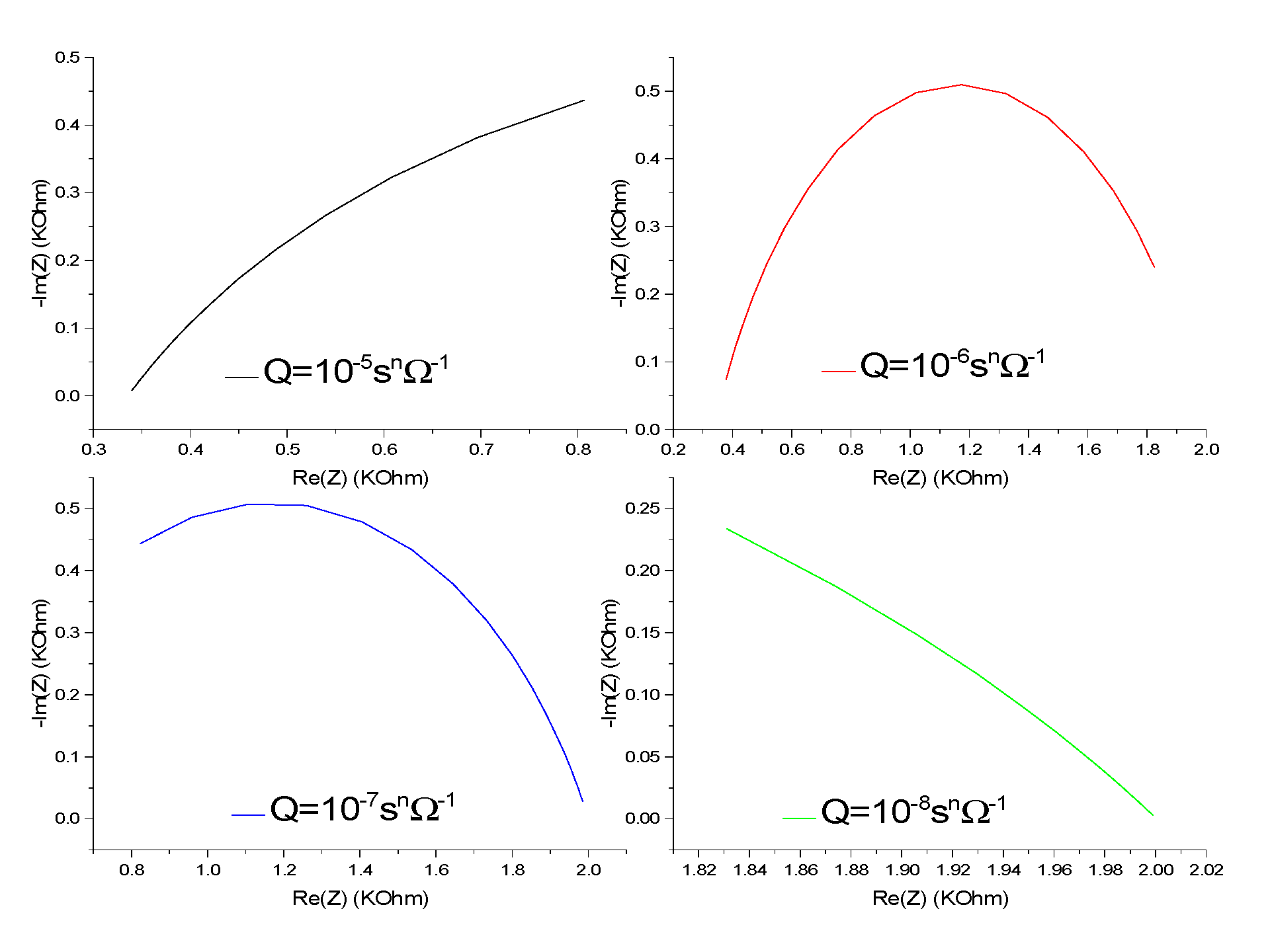
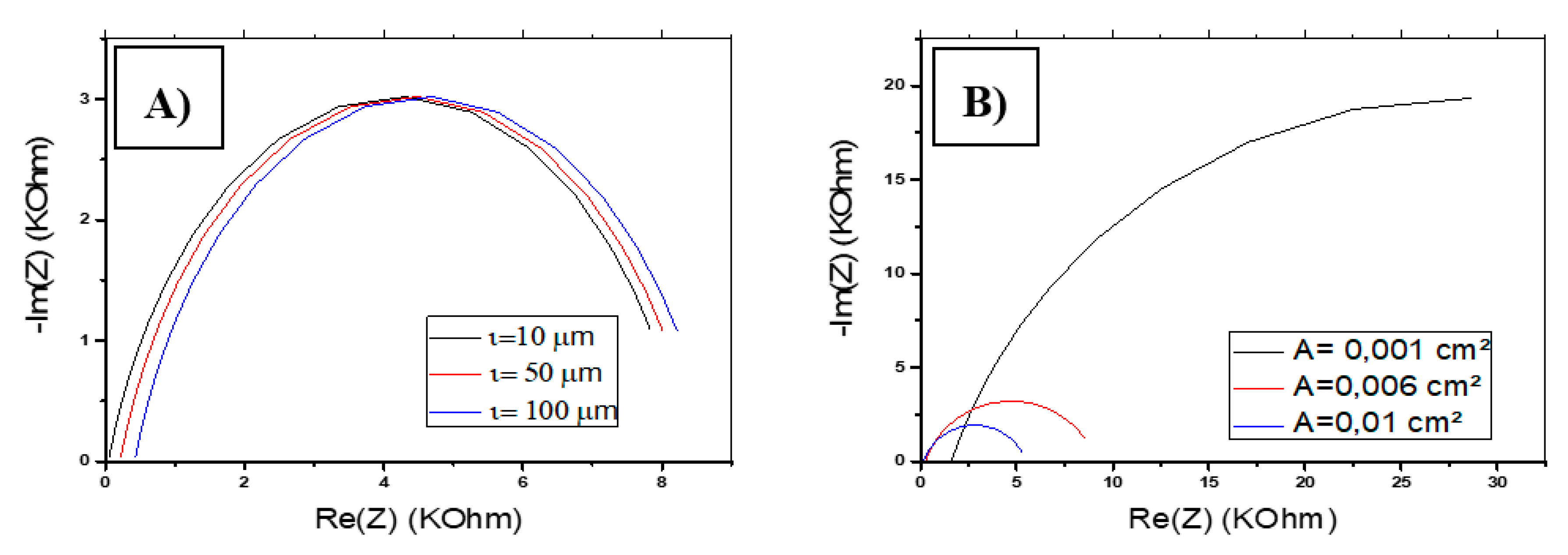
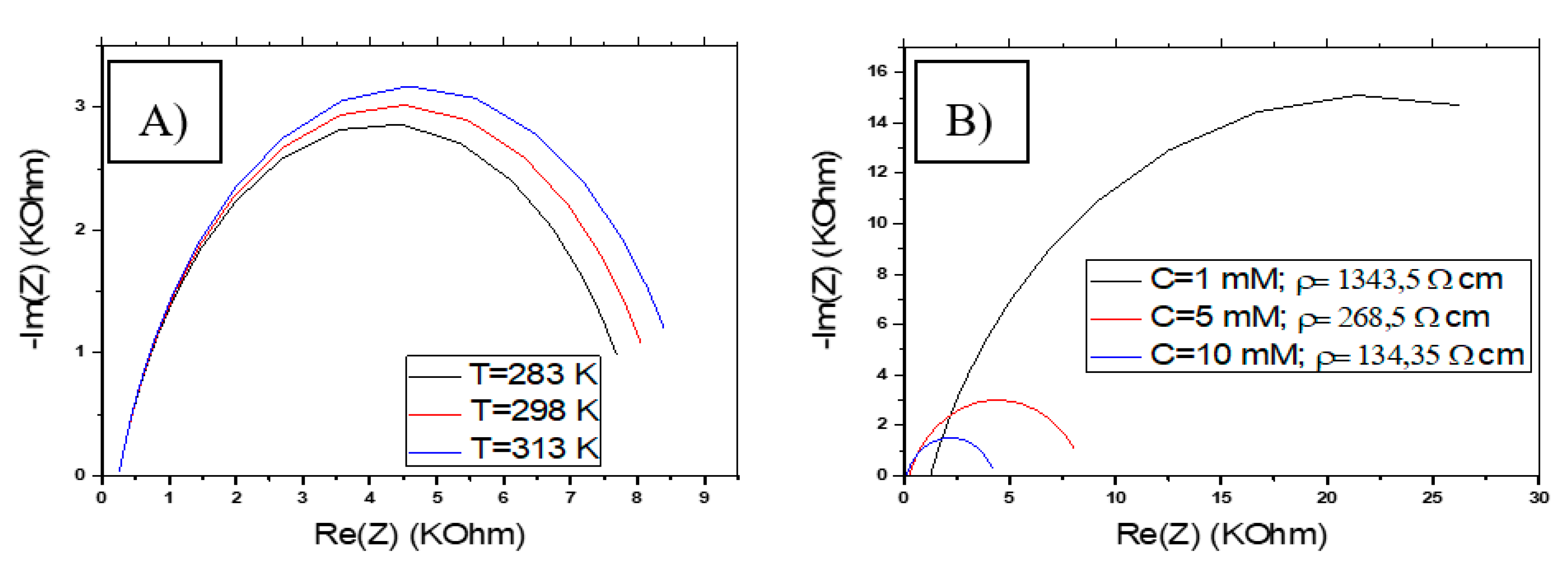

| Input Parameters | Value | Unit | Variables | Variation Range | Unit |
|---|---|---|---|---|---|
| T | 298 | K | [10−3,10−5] | cm | |
| F | 96485 | c·mol−1 | k° | [10−3,10−5] | cm·s−1 |
| R | 8.3145 | J·mol−1·K−1 | Q | [10−5,10−8] | sn·Ω−1 |
| A | 0.0064 | cm2 | n | [−1,1] | / |
| ρ | 268.7 | Ω·cm | |||
| C | 5 × 10−6 | mol·cm−3 | |||
| Ω = 2πf | f = 100 KHz→150 Hz | rad·s−1 | |||
| Re(Z) | / | Ω | |||
| −Im(Z) | / | Ω | |||
| [GLY] | (μm) | Rs (Ω) | k° (μm/s) | Rct (Ω) | Q.106 (Sn Ω−1) | n | e1.104 | e2.104 |
|---|---|---|---|---|---|---|---|---|
| 0 | 88 | 369.5 | 55 | 1109.7 | 2.5 | 0.71 | 3.46 | 4.58 |
| 0.31 pg/mL | 88 | 369.5 | 48 | 1733.9 | 2.25 | 0.71 | 1.81 | 6.34 |
| 6.25 pg/mL | 83 | 348.5 | 39 | 2249.4 | 2.08 | 0.73 | 3.99 | 3.83 |
| 125 pg/mL | 83 | 348.5 | 32 | 2600.9 | 1.74 | 0.73 | 2.97 | 2.75 |
| 2.5 ng/mL | 83 | 348.5 | 27 | 3082.5 | 1.42 | 0.73 | 6.84 | 1.90 |
| 50 ng/mL | 83 | 348.5 | 24 | 3467.8 | 1.26 | 0.73 | 7.81 | 6.93 |
| Electrochemical Technique | Electrode | Linear Range | Limit of Detection | Reference |
|---|---|---|---|---|
| DPASV | MCA-MIPs-GNPs/PGE | 3.98–0.54 ng/mL | 0.35 ng/mL | [34] |
| SWV | PPy-MIPs/Au | 0.017 pg/mL–1.69 ng/mL | 0.17 pg/mL | [35] |
| DPV | PPy-MIPs-PB-HAuCl4/IOT | 400−1200 ng/mL | 92 ng/mL | [36] |
| EIS | CS-MIPs-PPy/Au | 0.31 pg/mL–50 ng/mL | 0.005 pg/mL | this work |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zouaoui, F.; Bourouina-Bacha, S.; Bourouina, M.; Alcacer, A.; Bausells, J.; Jaffrezic-Renault, N.; Zine, N.; Errachid, A. Experimental Study and Mathematical Modeling of a Glyphosate Impedimetric Microsensor Based on Molecularly Imprinted Chitosan Film. Chemosensors 2020, 8, 104. https://doi.org/10.3390/chemosensors8040104
Zouaoui F, Bourouina-Bacha S, Bourouina M, Alcacer A, Bausells J, Jaffrezic-Renault N, Zine N, Errachid A. Experimental Study and Mathematical Modeling of a Glyphosate Impedimetric Microsensor Based on Molecularly Imprinted Chitosan Film. Chemosensors. 2020; 8(4):104. https://doi.org/10.3390/chemosensors8040104
Chicago/Turabian StyleZouaoui, Fares, Saliha Bourouina-Bacha, Mustapha Bourouina, Albert Alcacer, Joan Bausells, Nicole Jaffrezic-Renault, Nadia Zine, and Abdelhamid Errachid. 2020. "Experimental Study and Mathematical Modeling of a Glyphosate Impedimetric Microsensor Based on Molecularly Imprinted Chitosan Film" Chemosensors 8, no. 4: 104. https://doi.org/10.3390/chemosensors8040104
APA StyleZouaoui, F., Bourouina-Bacha, S., Bourouina, M., Alcacer, A., Bausells, J., Jaffrezic-Renault, N., Zine, N., & Errachid, A. (2020). Experimental Study and Mathematical Modeling of a Glyphosate Impedimetric Microsensor Based on Molecularly Imprinted Chitosan Film. Chemosensors, 8(4), 104. https://doi.org/10.3390/chemosensors8040104