Design and Evaluation of Micromixers Fabricated with Alternative Technologies and Materials for Microanalytical Applications In Situ
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials and Equipment
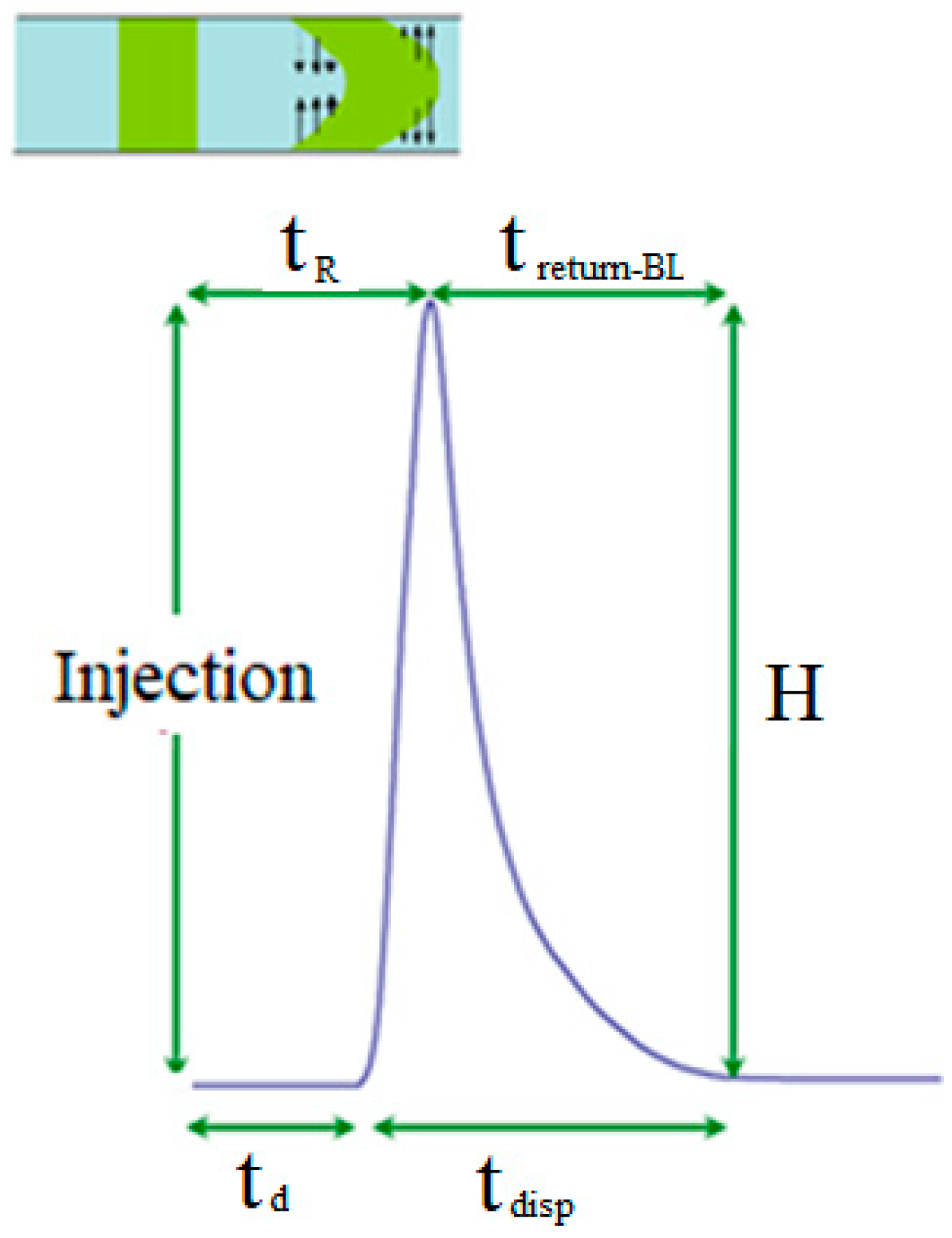
2.2. Analytical Methods
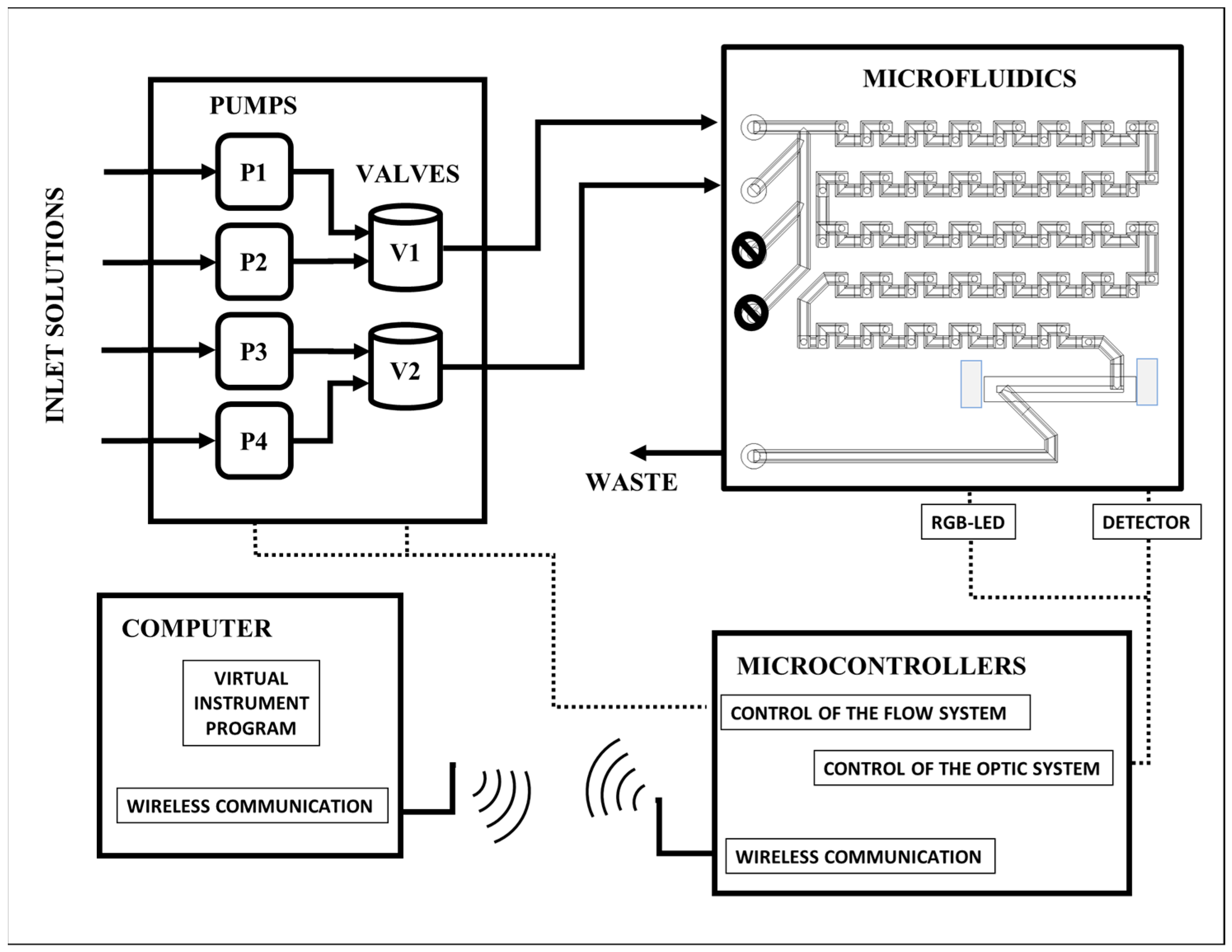
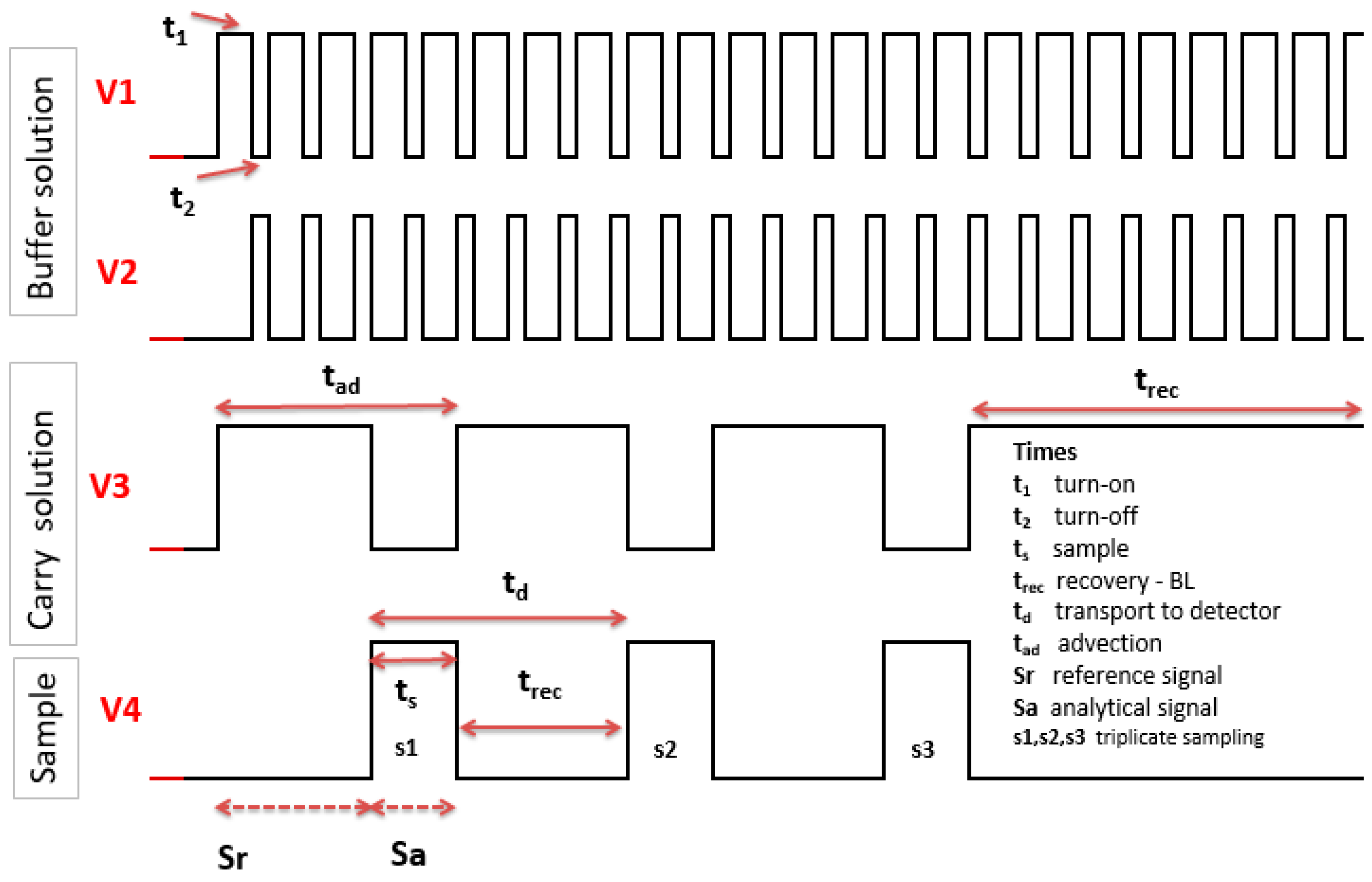
2.3. Automated Microanalytical Process
2.4. Statistical Method
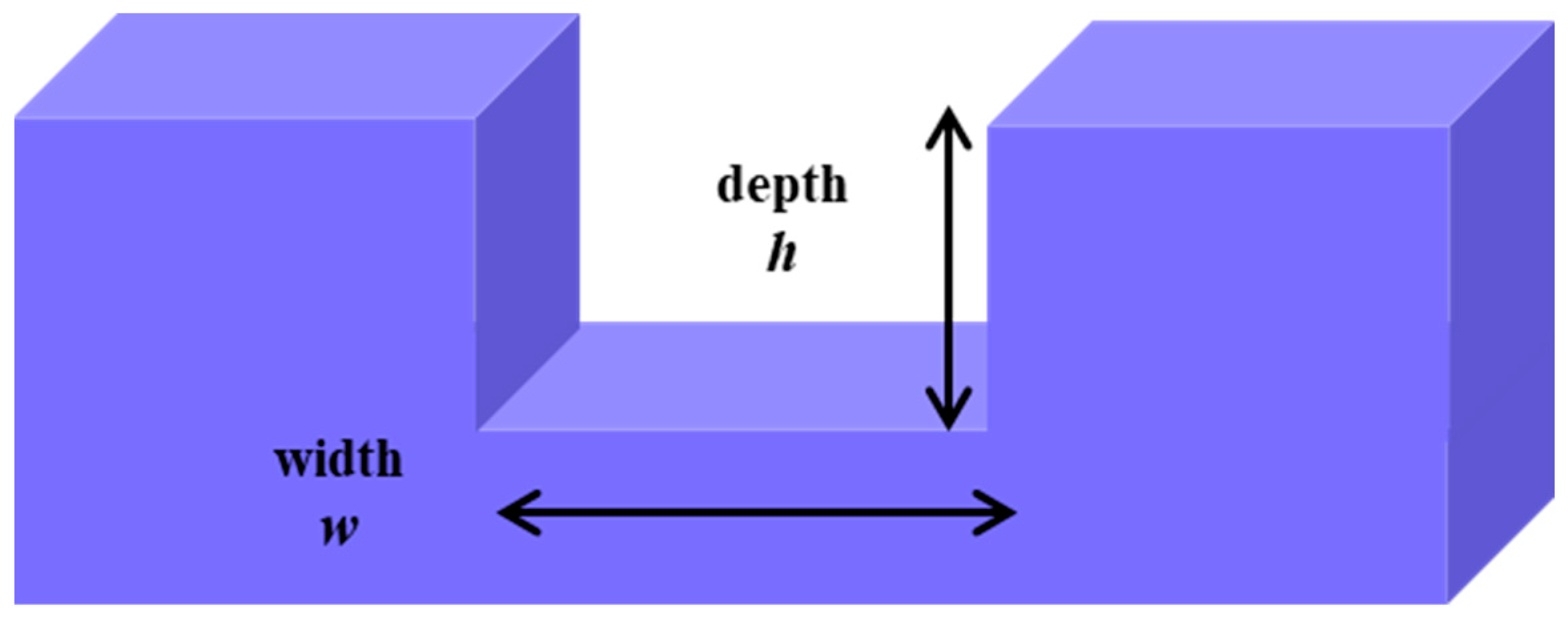
3. Micromixer Design
4. Results
4.1. LTCC Micromixers
4.1.1. 2D LTCC Planar Serpentine Micromixer
4.1.2. 3D LTCC L-Shaped Serpentine Micromixer
4.2. FDM Micromixers
4.2.1. 3D Circular Serpentine Micromixer—PLA
4.2.2. 3D Circular Serpentine Micromixer—PP
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| A | Absorbance | - |
| w | Channel width | m |
| Q | Flow rate | m3/s |
| DM | Molecular diffusion | m2/s |
| D | Dispersion | cm/s |
| C | Concentration | M/L |
| ρ | Density | Kg/m3 |
| d | Diameter | m |
| R2 | Correlation coefficient | - |
| L | Channel length | m |
| BL | Base line | M/L |
| LD | Diffusion length | m |
| h | High (depth) channel | m |
| Pe | Peclet number | - |
| Re | Reynold number | - |
| Rfluid | Fluid resistance (geometry/length channel) | mm |
| Sa | Analytical signal | V |
| Sd | Dark signal | V |
| Sr | Reference signal | V |
| t | Time | s |
| tad | Advection time | s |
| tdif | Diffusion time | s |
| vs | Average velocity | m/s |
| µ | Dynamic viscosity | Kg/m-s |
| v′ | Kinematic viscosity | m2/s |
| V | Volume | m3 |
References
- Ottino, J.M.; Wiggins, S. Introduction: Mixing in Microfluidics. Philos. Trans. Math. Phys. Eng. Sci. 2004, 362, 923–935. [Google Scholar] [CrossRef] [PubMed]
- Manz, A.; Graber, N.; Widmer, H.M. Miniaturized Total Chemical Analysis Systems: A Novel Concept for Chemical Sensing. Sens. Actuators B Chem. 1990, 1, 244–248. [Google Scholar] [CrossRef]
- Capretto, L.; Cheng, W.; Hill, M.; Zhang, X. Micromixing Within Microfluidic Devices. In Topics in Current Chemistry; Lin, B., Ed.; Springer: Berlin/Heidelberg, Germany, 2011; Volume 304, pp. 27–68. ISBN 978-3-642-23049-3. [Google Scholar]
- Shestopalov, I.; Tice, J.D.; Rustem, I.F. Multi-Step Synthesis of Nanoparticles Performed on Millisecond Time Scale in a Microfluidic Droplet-Based System. Lab Chip 2004, 4, 316–321. [Google Scholar] [CrossRef]
- Mason, B.P.; Price, K.E.; Steinbacher, J.L.; Bogdan, A.R.; McQuade, D.T. Greener Approaches to Organic Synthesis Using Microreactor Technology. Chem. Rev. 2007, 107, 2300–2318. [Google Scholar] [CrossRef]
- Aoki, N.; Hasebe, S.; Mae, K. Mixing in Microreactors: Effectiveness of Lamination Segments as a Form of Feed on Product Distribution for Multiple Reactions. Chem. Eng. J. 2004, 101, 323–331. [Google Scholar] [CrossRef]
- Surangalikar, H.; Ouyang, X.; Besser, R. Experimental Study of Hydrocarbon Hydrogenation and Dehydrogenation Reactions in Silicon Microfabricated Reactors of Two Different Geometries. Chem. Eng. J. 2003, 93, 217–224. [Google Scholar] [CrossRef]
- Wilms, D.; Klos, J.; Frey, H. Microstructured Reactors for Polymer Synthesis: A Renaissance of Continuous Flow Processes for Tailor-Made Macromolecules? Macromol. Chem. Phys. 2008, 209, 343–356. [Google Scholar] [CrossRef]
- Nguyen, N.T.; Wu, Z. Micromixers—A Review. J. Micromech. Microeng. 2005, 15, R1–R16. [Google Scholar] [CrossRef]
- Barbosa-Saldaña, J.G.; Jiménez-Bernal, J.A.; Gutiérrez-Torres, C.d.C.; Quinto-Diez, P. Tri-Dimensional Numerical Analysis for Forced Convection over a Forward Facing Step. Inv. Ing. Tecnol. RIIT 2010, XI, 45–55. [Google Scholar]
- Hossain, S.; Ansari, M.A.; Kim, K.Y. Evaluation of the Mixing Performance of Three Passive Micromixers. Chem. Eng. J. 2009, 150, 492–501. [Google Scholar] [CrossRef]
- Ganguli, A.; Bhatt, V.; Yagodnitsyna, A.; Pinjari, D.; Pandit, A. A Review of Pressure Drop and Mixing Characteristics in Passive Mixers Involving Miscible Liquids. Micromachines 2024, 15, 691. [Google Scholar] [CrossRef]
- Wang, X.; Liu, Z.; Cai, Y.; Wang, B.; Luo, X. A Cost-Effective Serpentine Micromixer Utilizing Ellipse Curve. Anal. Chim. Acta 2021, 1155, 338–355. [Google Scholar] [CrossRef]
- Ran, B.; Liu, B.; Chen, C.; Tong, W.; Shi, J.; Du, J.; Yu, Z.; Bai, S.; Chen, H.; Zhu, Y. Acoustic Micromixing in a Serpentine Channel with Sharp Teeth for Controllable Nanomaterial Synthesis. Chem. Eng. J. 2025, 504, 159094. [Google Scholar] [CrossRef]
- Yin, B.; Zhu, H.; Zeng, S.; Sohan, A.M.; Wan, X.; Liu, J.; Zhang, P.; Lin, X. Chip-Based Automated Equipment for Dual-Mode Point-of-Care Testing Foodborne Pathogens. Biosens. Bioelectron. 2024, 257, 116338. [Google Scholar] [CrossRef]
- Gongora-Rubio, M.R.; Espinoza-Vallejos, P.; Sola-Laguna, L.; Santiago-Avilés, J.J. Overview of Low Temperature Co-Fired Ceramics Tape Technology for Meso-System Technology (MsST). Sens. Actuators A Phys. 2001, 89, 222–241. [Google Scholar] [CrossRef]
- Peterson, K.A.; Patel, K.D.; Ho, C.K.; Rohde, S.B.; Nordquist, C.D.; Walker, C.A.; Wroblewski, B.D.; Okandan, M. Novel Microsystem Applications with New Techniques in Low-Temperature Co-Fired Ceramics. Int. J. Appl. Ceram. Technol. 2005, 2, 345–363. [Google Scholar] [CrossRef]
- Golonka, L.J. Technology and Applications of Low Temperature Cofired Ceramic (LTCC) Based Sensors and Microsystems. Bull. Pol. Acad. Sci. Tech. Sci. 2006, 54, 221–231. [Google Scholar]
- Belavic, D.; Hrovat, M.; Dolanc, G.; Santo Zarnik, M.; Holc, J.; Makarovic, K. Design of LTCC-Based Ceramic Structure for Chemical Microreactor. Radioengineering 2012, 21, 195–200. [Google Scholar]
- Baeza, M. Ceramic Microsystem Incorporating a Microreactor with Immobilized Biocatalyst for Enzymatic Spectrophotometric Assays. Anal. Chem. 2010, 82, 1006–1011. [Google Scholar] [CrossRef]
- Malecha, K.; Pijanowska, D.; Golonka, L.; Torbicz, W. LTCC Enzymatic of Microreactors. J. Microelectron. Electron. Packag. 2007, 4, 51–56. [Google Scholar] [CrossRef]
- Malecha, K.; Pijanowska, D.G.; Golonka, L.J.; Piotr, K. Low Temperature Co-Fired Ceramic (LTCC)-Based Biosensor for Continuous Glucose Monitoring. Sens. Actuators B Chem. 2011, 155, 923–929. [Google Scholar] [CrossRef]
- Bhattacharjee, N.; Urrios, A.; Kang, S.; Folch, A. The Upcoming 3D-Printing Revolution in Microfluidics. Lab Chip 2016, 16, 1720–1742. [Google Scholar] [CrossRef]
- Nielsen, A.V.; Beauchamp, M.J.; Nordin, G.P.; Woolley, A.T. 3D Printed Microfluidics. Annu. Rev. Anal. Chem. 2020, 13, 45–65. [Google Scholar] [CrossRef] [PubMed]
- Andrew, J.; Capel, A.; Edmondson, S.; Christie, S.D.R.; Goodridge, R.D.; Bibbd, R.J.; Thurstans, M. Design and Additive Manufacture for Flow Chemistry. Lab Chip 2013, 13, 4583–4590. [Google Scholar] [CrossRef]
- Kitson, P.J.; Rosnes, M.H.; Sans, V.; Dragonea, V.; Cronin, L. Configurable 3D-Printed Millifluidic and Microfluidic ‘Lab on a Chip’ Reactionware Devices. Lab Chip 2012, 12, 3267–3271. [Google Scholar] [CrossRef] [PubMed]
- Symes, M.D.; Kitson, P.J.; Yan, J.; Richmond, C.J.; Cooper, G.J.T.; Bowman, R.W.; Vilbrandt, T.; Cronin, L. Integrated 3D-Printed Reactionware for Chemical Synthesis and Analysis. Lab Chip 2012, 4, 349–354. [Google Scholar] [CrossRef]
- Bishop, G.W.; Satterwhite, J.E.; Bhakta, S.; Kadimisetty, K.; Gillette, K.M.; Chen, E.; Rusling, J.F. 3D-Printed Fluidic Devices for Nanoparticle Preparation and Flow-Injection Amperometry Using Integrated Prussian Blue Nanoparticle-Modified Electrodes. Anal. Chem. 2015, 87, 5437–5443. [Google Scholar] [CrossRef]
- Kadimisetty, K.; Mosa, I.M.; Malla, S.; Satterwhite-Warden, J.E.; Kuhns, T.; Faria, R.C.; Leed, N.H.; Rusling, F.J. 3D-Printed Supercapacitor-Powered Electrochemiluminescent Protein Immunoarray. Biosens. Bioelectron. 2016, 15, 188–193. [Google Scholar] [CrossRef]
- Imanaka, Y. Multilayered Low Temperature Cofired Ceramics (LTCC) Technology, 1st ed.; Springer: New York, NY, USA, 2005; ISBN 978-0-387-23130-3. [Google Scholar]
- Camarillo, R. Sistema Automatizado de Análisis con Comunicación Inalámbrica Utilizando Tecnologia de Ceramicas Verdes de Cocimiento Conjunto a Bajas Temperaturas para Mediciones Espectrofotometricas In-Situ y on-Line de Variables Ambientales e Industriales, Gaceta de la Propiedad Industrial (Mexico) October 2018, 97–98. Mexican Patent No. MX 359936 B. Available online: https://siga.impi.gob.mx/ (accessed on 24 April 2025).
- NMX-AA-077-SCFI-2001; Waters Analysis, Determination of Fluoride in Natural, Wastewaters and Wastewaters Treated, Test Method. Secretaría de Economía: Mexico City, Mexico, 2001.
- Fonseca, A.; Raimundo, I.M. A Multichannel Photometer Based on an Array of Light Emitting Diodes for Use in Multivariate Calibration. Anal. Chim. Acta 2004, 522, 223–229. [Google Scholar] [CrossRef]
- Camarillo Escobedo, R.M.; Flores Nuñez, J.L.; Camarillo Escobedo, J.; García Muñoz, L.; Peña Dominguez, E. 3D Printed Opto-Microfluidic Autonomous Analyzer for Photometric Applications. HardwareX 2023, 14, e00406. [Google Scholar] [CrossRef]
- Standford University Stanford Microfluidics Foundry. Available online: https://www.stanfordmicrofluidics.com/ (accessed on 24 April 2025).
- Camarillo-Escobedo, R.M.; Camarillo-Escobedo, J.M. LTCC Technology Applied to the Development of Microfluidics Systems. In Proceedings of the MEMORIAS DEL XVI CONGRESO INTERNACIONAL ANUAL DE LA SOMIM, Monterrey, Mexico, 22–24 September 2010; Mexican Society of Mechanical Engineering SOMIM: Monterrey, Mexico, 2010; pp. 1–10. [Google Scholar]
- Squires, T.M.; Quake, S.R. Microfluidics: Fluid Physics at the Nanoliter Scale. Rev. Mod. Phys. 2005, 77, 977–1026. [Google Scholar] [CrossRef]
- Ruzicka, J.; Stewart, J. Flow Injection Analysis. II. Ultrafast Determination of Phosphorus in Plant Material by Continuous Flow Spectrophotometry. Anal. Chim. Acta 1975, 79, 79–91. [Google Scholar] [CrossRef]
- Ottino, J.M. Mixing, Chaotic Advection, and Turbulence. Annu. Rev. Fluid. Mech. 1990, 22, 207–254. [Google Scholar] [CrossRef]
- Stroock, A.D.; Dertinger, S.K.W.; Ajdari, A.; Mezić, I.; Stone, H.A.; Whitesides, G.M. Chaotic Mixer for Microchannels. Science 2002, 295, 647–651. [Google Scholar] [CrossRef]
- Lee, C.Y.; Chang, C.L.; Wang, Y.N.; Fu, L.M. Microfluidic Mixing: A Review. Int. J. Mol. Sci. 2011, 12, 3263–3287. [Google Scholar] [CrossRef]
- Nguyen, N.-T.; Wereley, S.; Mousavi, S.A. Fundamentals and Applications of Microfluidics, 3rd ed.; Artech House Publishers: Norwood, MA, USA, 2019; ISBN 978-1-63081-364-2. [Google Scholar]
- Wu, Z.; Nguyen, N.-T.; Huang, X. Nonlinear Diffusive Mixing in Microchannels: Theory and Experiments. J. Micromech. Microeng. 2004, 14, 604–611. [Google Scholar] [CrossRef]
- Ross, C.; Murdoch, L.C.; Freedman, D.L.; Siegrist, R.L. Characteristics of Potassium Permanganate Encapsulated in Polymer. J. Environ. Eng. 2005, 131, 1203–1211. [Google Scholar] [CrossRef]
- ERCROS Acido Sulfúrico-Ficha de Datos; Barcelona: Glen Ave, PA, USA, 2006; Available online: https://www.carlroth.com/medias/SDB-X946-ES-ES.pdf?context=bWFzdGVyfHNlY3VyaXR5RGF0YXNoZWV0c3wzMzg2OTh8YXBwbGljYXRpb24vcGRmfGFHSXdMMmhoTnk4NU1UYzFOelU1T1RFeU9Ua3dMMU5FUWw5WU9UUTJYMFZUWDBWVExuQmtaZ3w2MDQyYTYwZjY1ZDZhMTU3NThhOTUwYWY2ODczNDViNWQ0MDM0NTQxNjU0N2VkZWYzZTMyZDNiNGZkMTNhYzVi (accessed on 24 April 2025).
- Camarillo-Escobedo, R.M.; Flores-Nuñez, J.L.; Garcia-Torales, G.; Hernandez-Campos, E.; Camarillo-Escobedo, J.M. 3D Printed Opto-Microfluidic Autonomous Analyzer for Photometric Applications. Sens. Actuators A Phys. 2022, 337, 113425. [Google Scholar] [CrossRef]
- Zagatto, E.A.G.; Arruda, M.A.Z.; Jacintho, A.O.; Mattos, I.L. Compensation of the Schlieren Effect in Flow-Injection Analysis by Using Dual-Wavelength Spectrophotometry. Anal. Chim. Acta 1990, 234, 153–160. [Google Scholar] [CrossRef]
- Rocha, F.R.P.; Zagatto, E.A.G. Chemical Derivatization in Flow Analysis. Molecules 2022, 27, 1563. [Google Scholar] [CrossRef]
- Zeng, M.; Wang, C.; Li, L. Designed Oscillations of the Fe/H2SO4 System with the Flow Injection in a Partially-Closed Environment. Electrochem. Commun. 2009, 11, 1888–1891. [Google Scholar] [CrossRef]
- Rocha, F.R.P.; Nóbrega, J.A. Overcoming the Schlieren Effect in Flow Injection Spectrophotometry by Introduction of Large Sample Volumes. Determination of Chloride in the Electrolyte of Lead-Acid Batteries. J. Braz. Chem. Soc. 1997, 8, 625–629. [Google Scholar] [CrossRef]
- Dias, A.C.B.; Borges, E.P.; Zagatto, E.A.G.; Worsfold, P.J. A Critical Examination of the Components of the Schlieren Effect in Flow Analysis. Talanta 2006, 68, 1076–1082. [Google Scholar] [CrossRef] [PubMed]

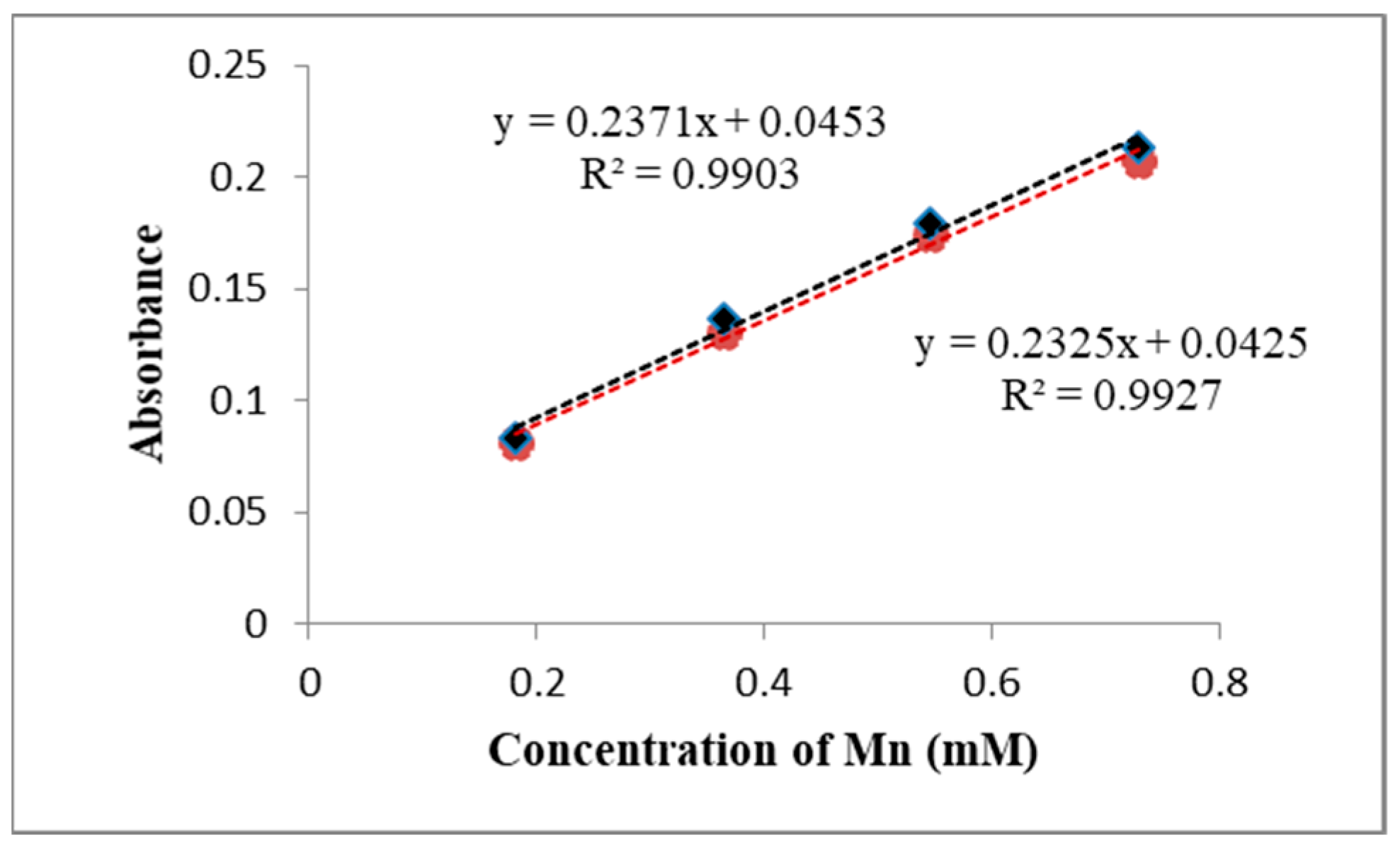

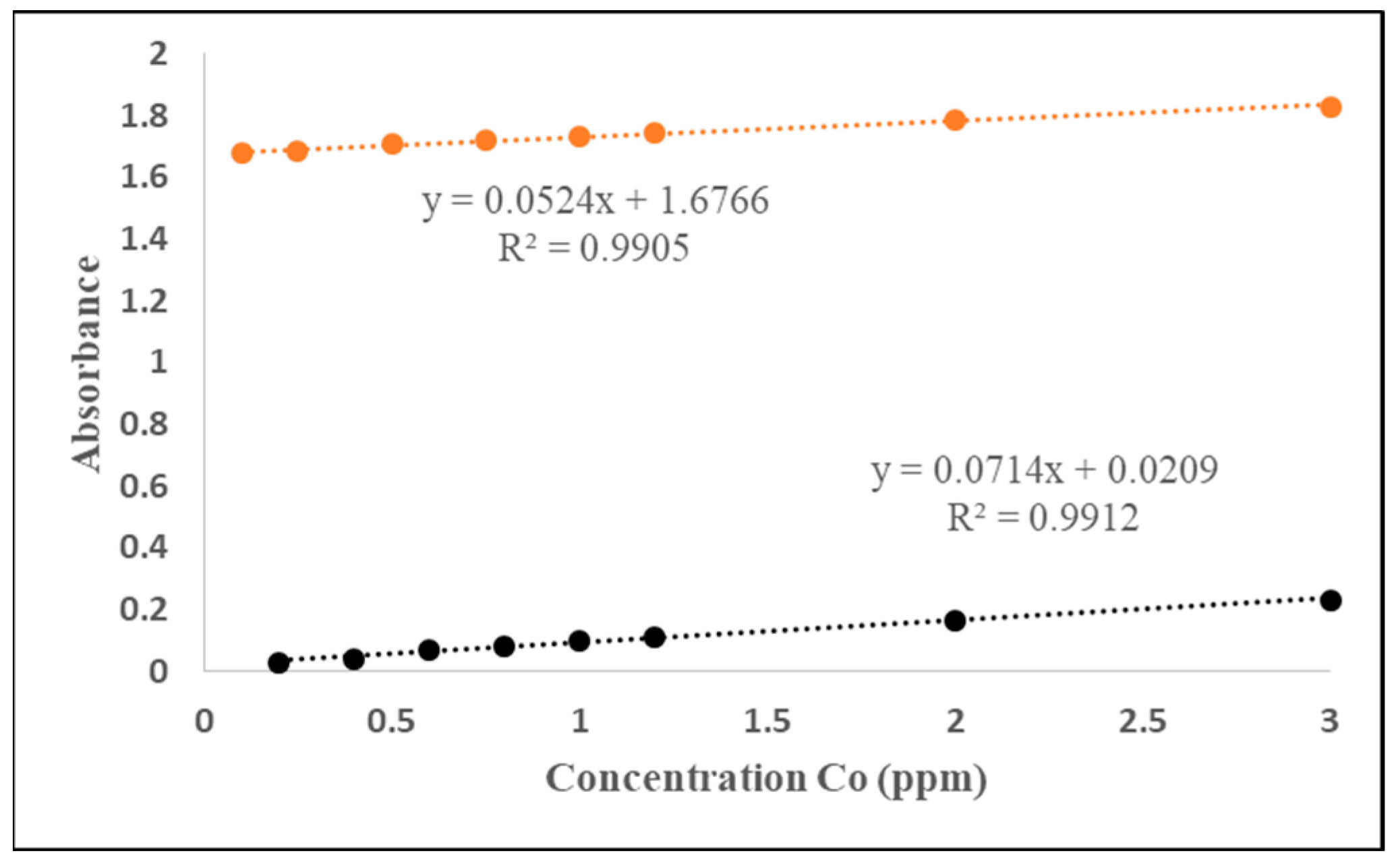
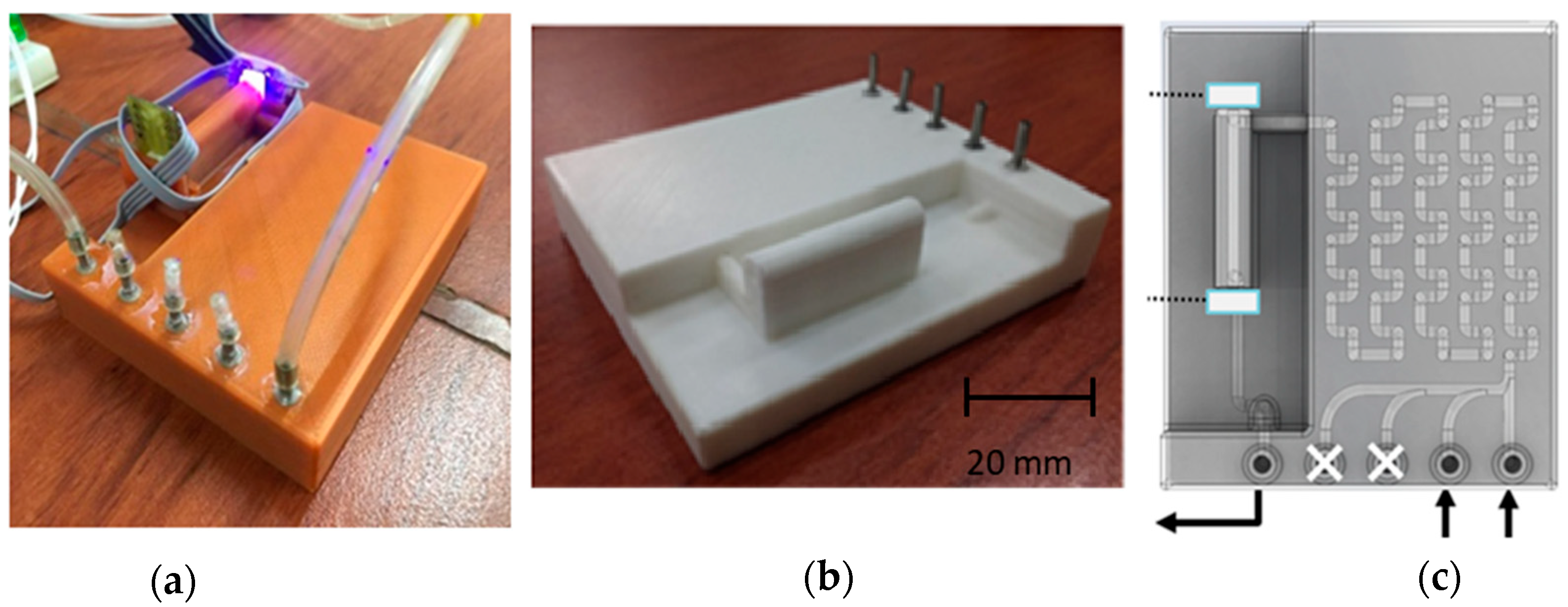

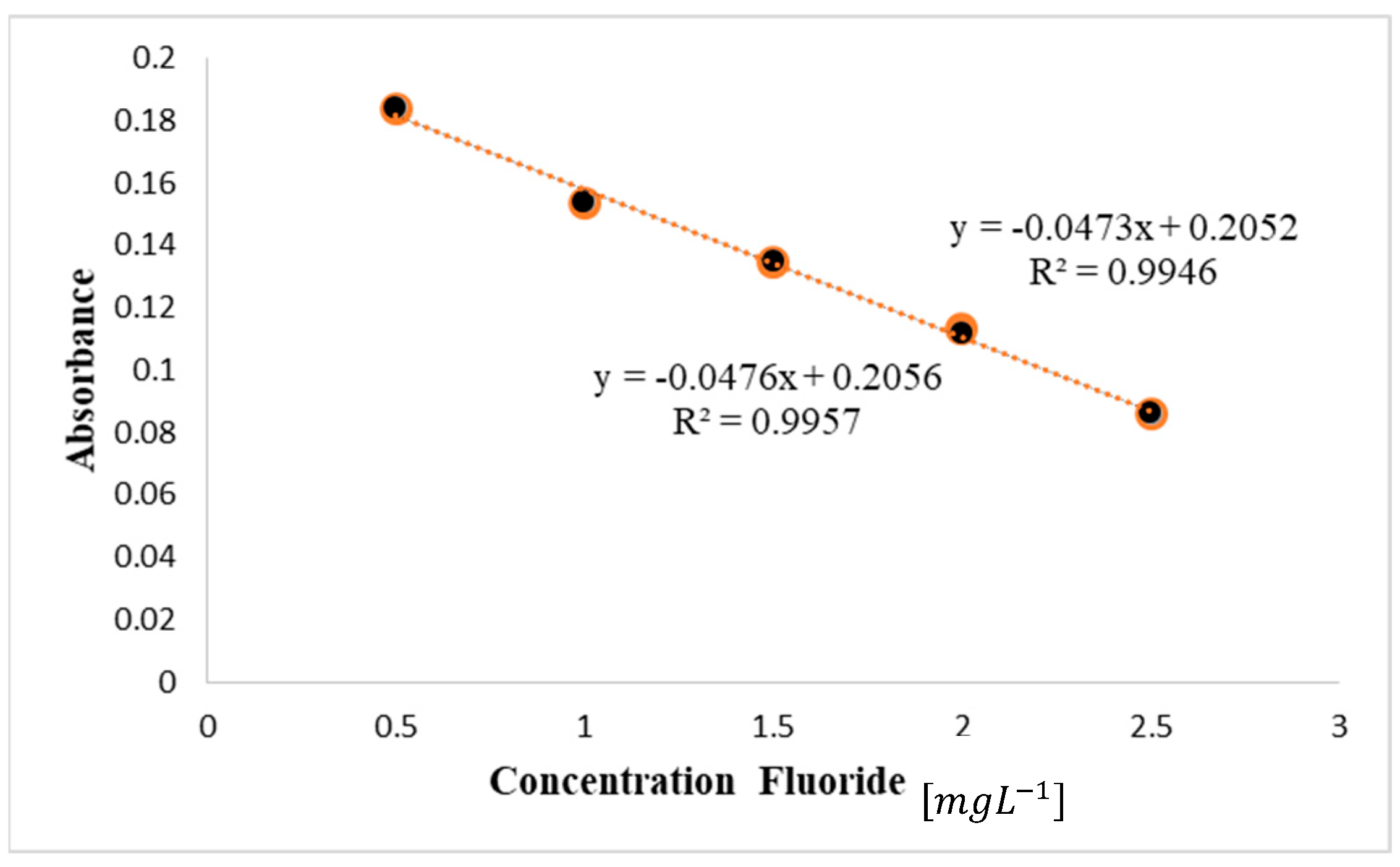
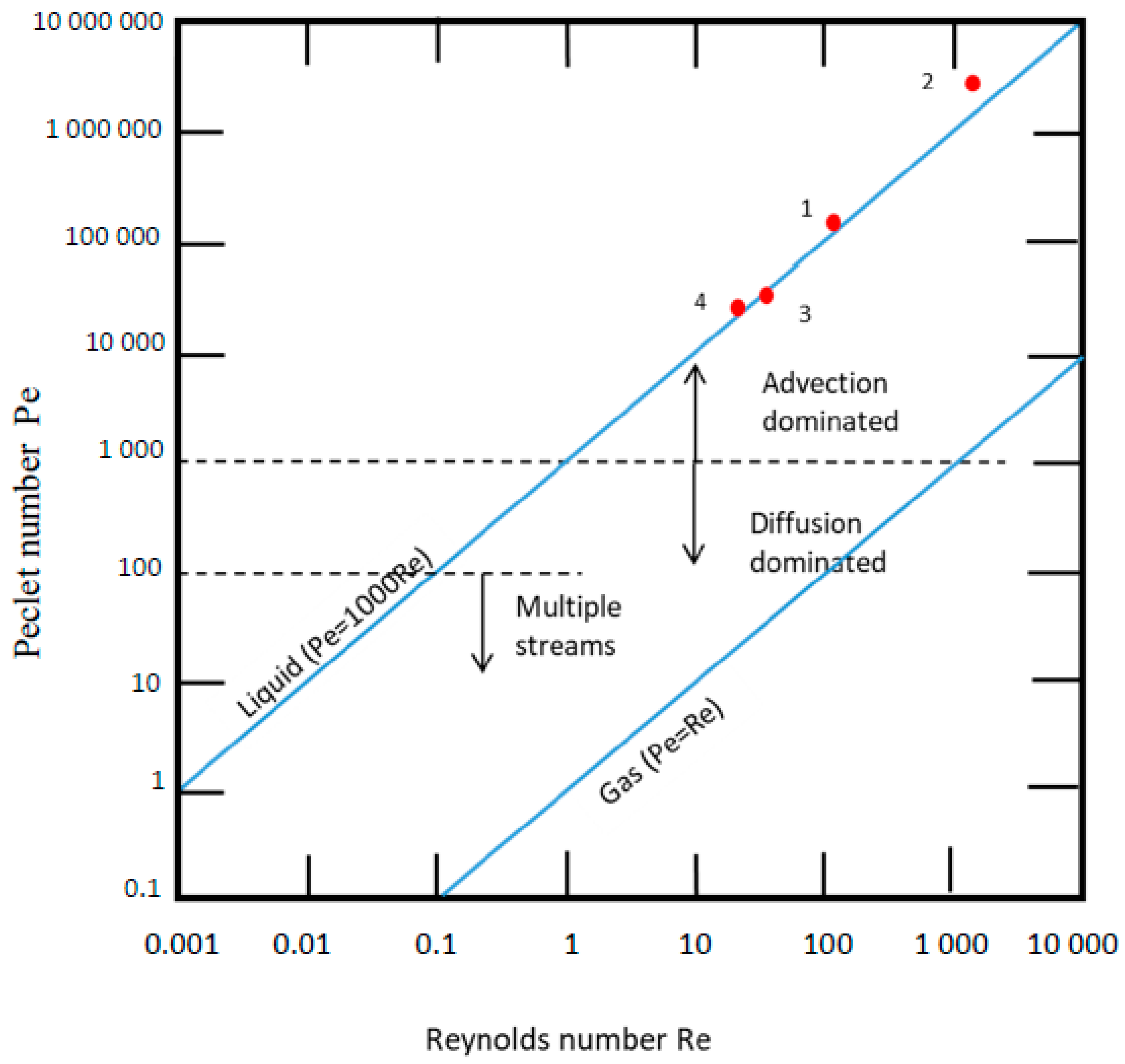

| No. | Type | Materials | Number of Nozzles | Channel Width (µm) | Channel Height (µm) | Typical Velocity (mm s−1) | Re | Pe | tad (s) | Analite/Sample | RMSE |
|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 2D serpentine planar 90°-shaped | Ceramics | 4 | 873 | 850 | 33.69 | 101.07 | 101,000 | 8 | KMnO4 | 0.005 |
| 2 | 3D Serpentine L-shaped | Ceramics | 5 | 873 | 431 | 66.44 | 1787 | 1.7 × 106 | 9 | Co | 0.002 * |
| 3 | 3D Serpentine circular | PLA | 5 | 2000 | 2000 | 25.6 | 49.7 | 49708 | 23.43 | MB MR | 0.021 0.061 |
| 4 | 3D Serpentine circular | PP | 5 | 2000 | 2000 | 25.6 | 32.20 | 32201 | 23.43 | F | 0.012 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Camarillo-Escobedo, R.M.; Flores, J.L.; Camarillo-Escobedo, J.M.; Hernandez-Campos, E.; Garcia-Muñoz, L.H. Design and Evaluation of Micromixers Fabricated with Alternative Technologies and Materials for Microanalytical Applications In Situ. Chemosensors 2025, 13, 191. https://doi.org/10.3390/chemosensors13050191
Camarillo-Escobedo RM, Flores JL, Camarillo-Escobedo JM, Hernandez-Campos E, Garcia-Muñoz LH. Design and Evaluation of Micromixers Fabricated with Alternative Technologies and Materials for Microanalytical Applications In Situ. Chemosensors. 2025; 13(5):191. https://doi.org/10.3390/chemosensors13050191
Chicago/Turabian StyleCamarillo-Escobedo, Rosa M., Jorge L. Flores, Juana M. Camarillo-Escobedo, Elizabeth Hernandez-Campos, and Luis H. Garcia-Muñoz. 2025. "Design and Evaluation of Micromixers Fabricated with Alternative Technologies and Materials for Microanalytical Applications In Situ" Chemosensors 13, no. 5: 191. https://doi.org/10.3390/chemosensors13050191
APA StyleCamarillo-Escobedo, R. M., Flores, J. L., Camarillo-Escobedo, J. M., Hernandez-Campos, E., & Garcia-Muñoz, L. H. (2025). Design and Evaluation of Micromixers Fabricated with Alternative Technologies and Materials for Microanalytical Applications In Situ. Chemosensors, 13(5), 191. https://doi.org/10.3390/chemosensors13050191






