Optimal Rotor Design of Synchronous Reluctance Machines Considering the Effect of Current Angle
Abstract
1. Introduction
2. Design Optimization of SynRMs
2.1. Hybrid PSOGWO Technique
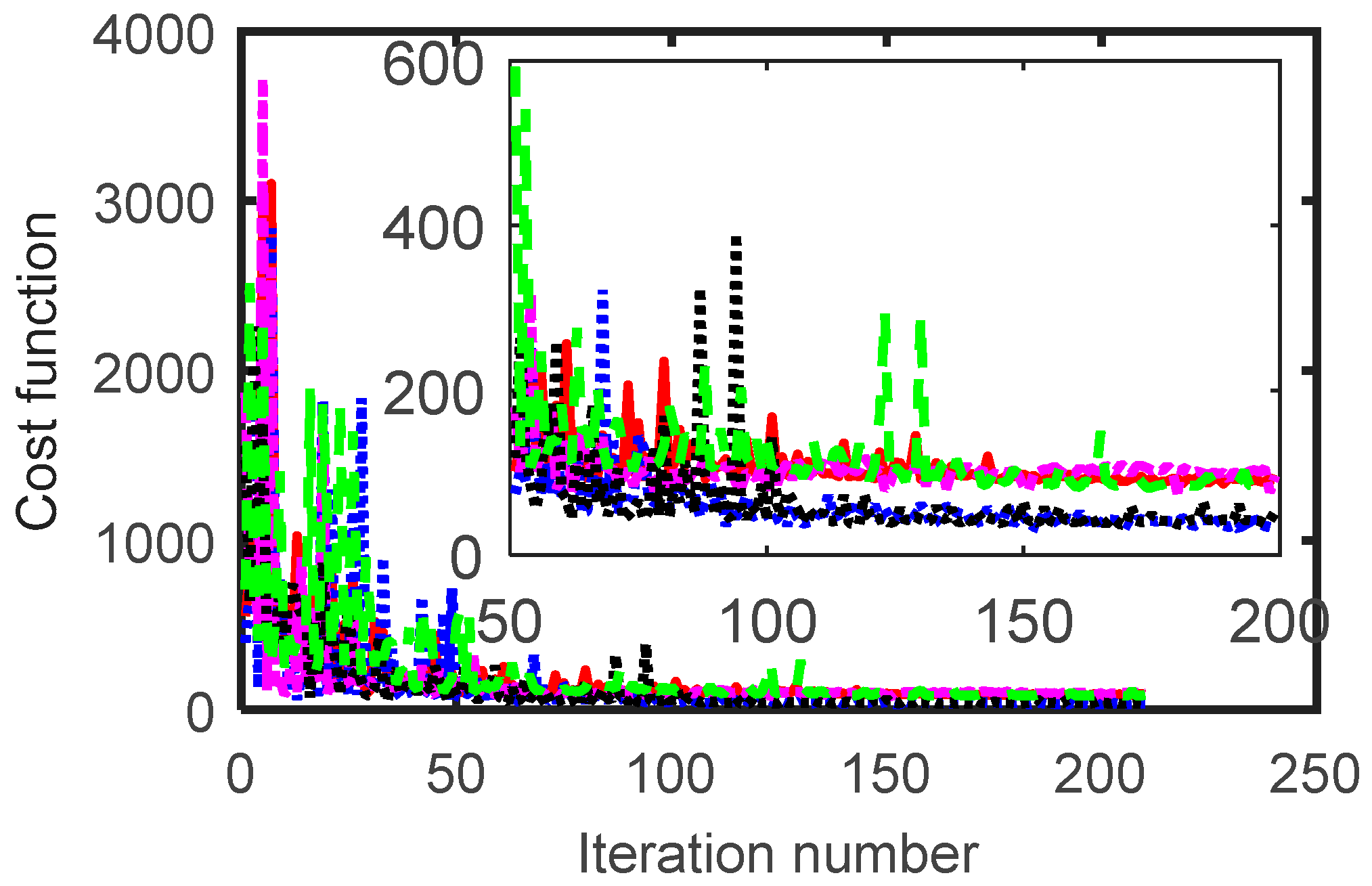
2.2. Optimization Process
3. Performance Analysis of SynRM
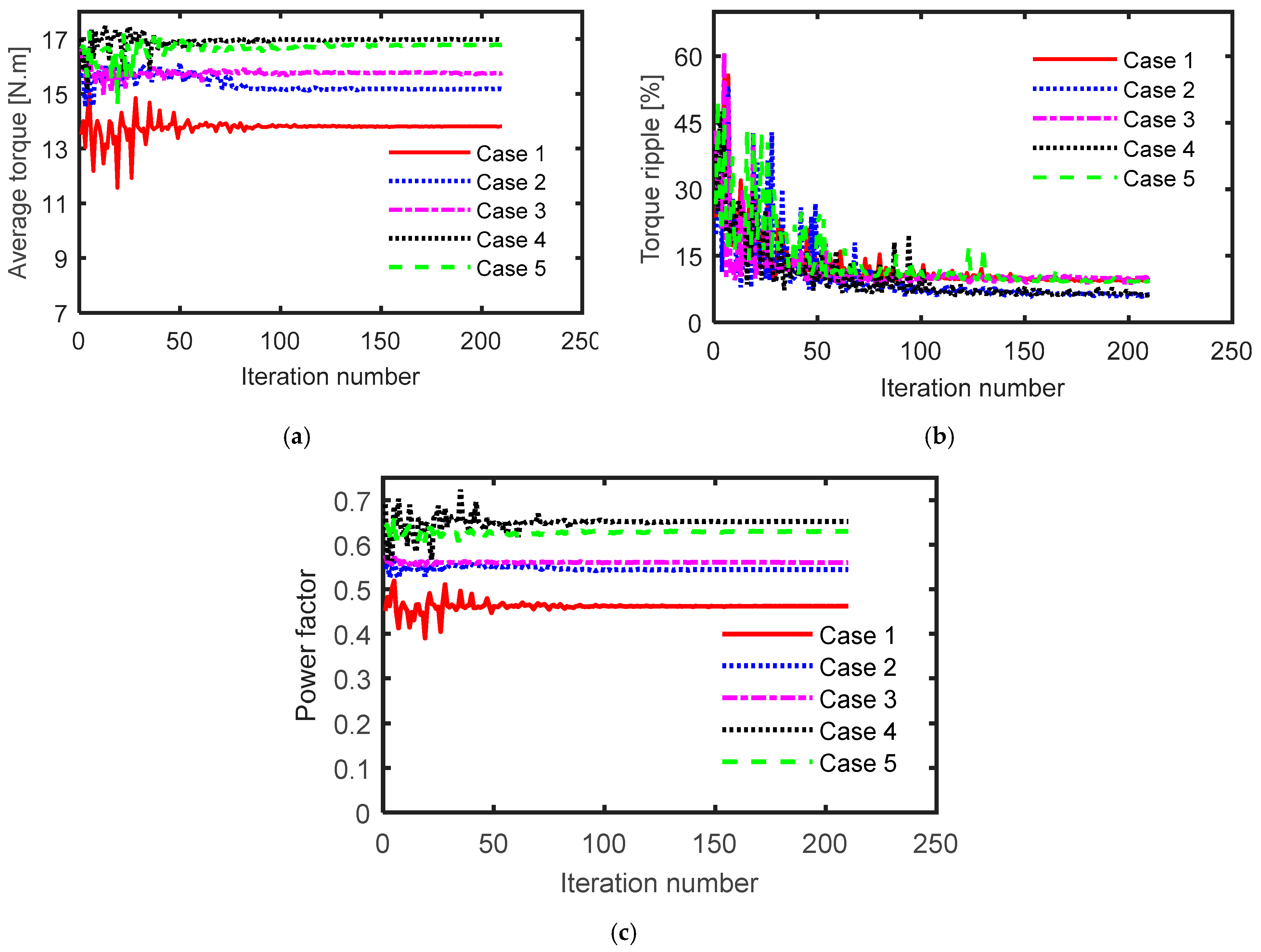
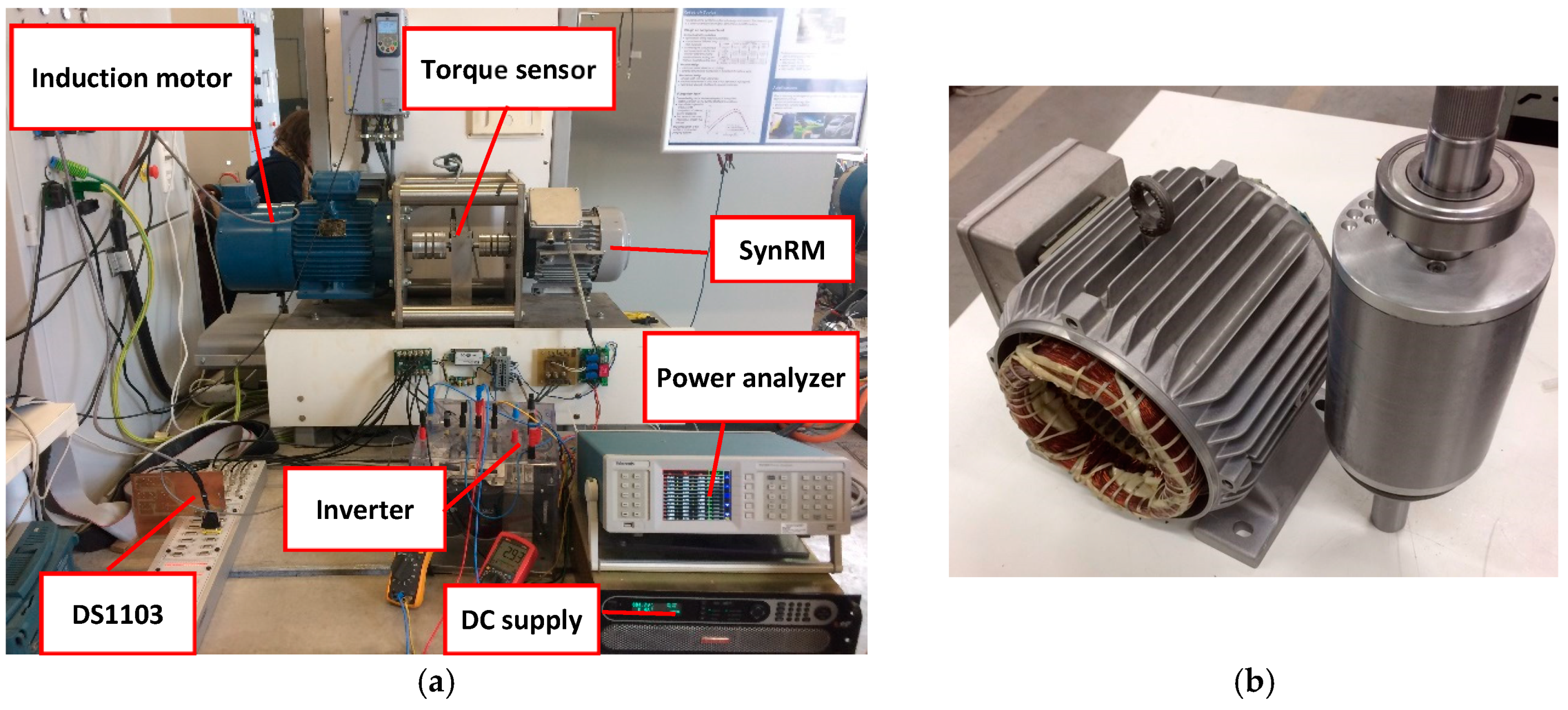
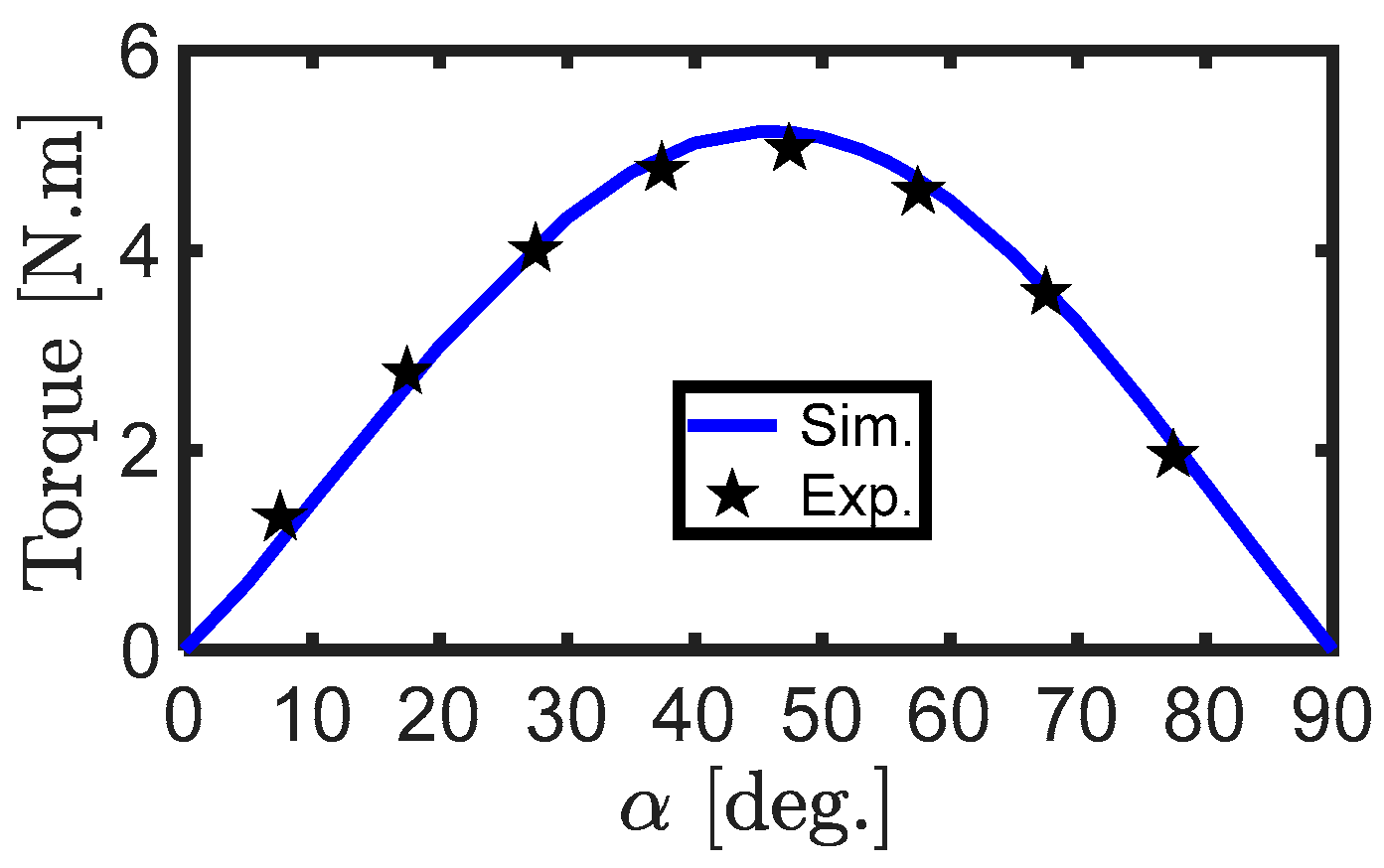
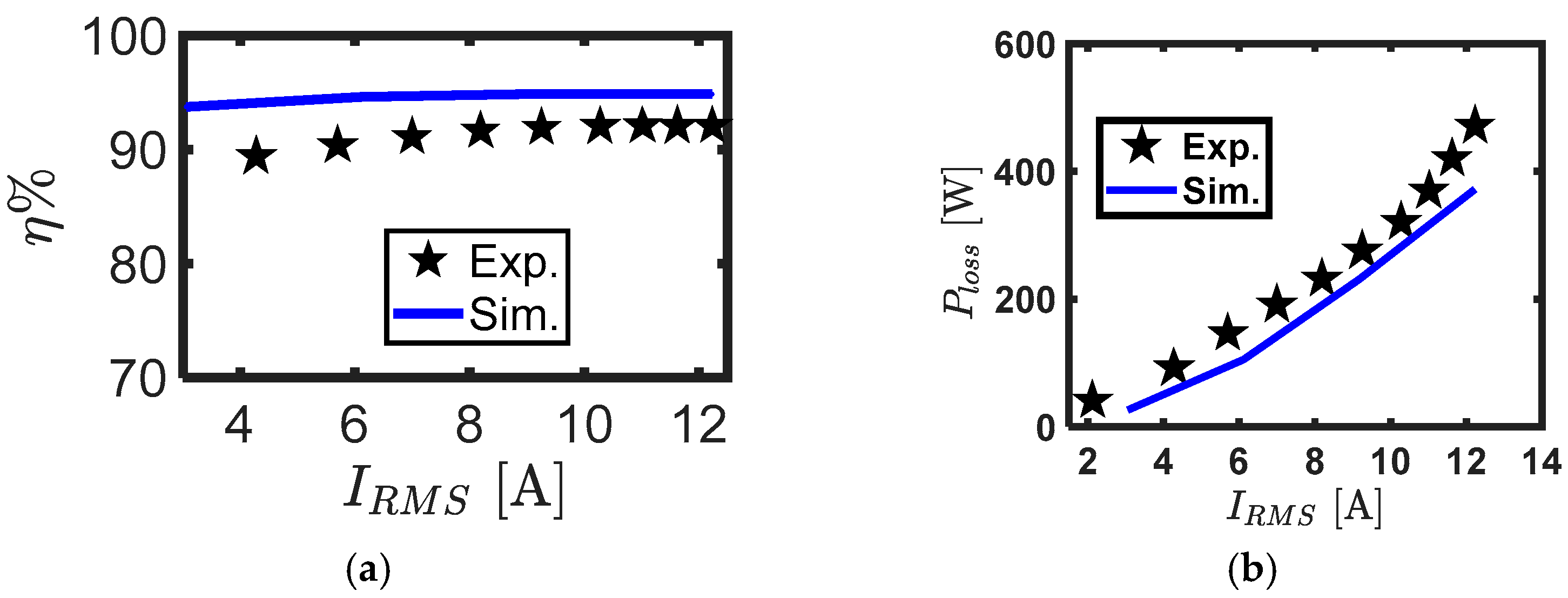
4. Experimental Results
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Bacco, G.; Bianchi, N.; Mahmoud, H. A Nonlinear Analytical Model for the Rapid Prediction of the Torque of Synchronous Reluctance Machines. IEEE Trans. Energy Convers. 2018, 33, 1539–1546. [Google Scholar] [CrossRef]
- Tawfiq, K.B.; Ibrahim, M.N.; El-Kholy, E.E.; Sergeant, P. Refurbishing three-phase synchronous reluctance machines to multiphase machines. Electr. Eng. 2020. [Google Scholar] [CrossRef]
- Ibrahim, M.N.; Sergeant, P.; Rashad, E.E.M. Combined Star-Delta Windings to Improve Synchronous Reluctance Motor Performance. IEEE Trans. Energy Convers. 2016, 31, 1479–1487. [Google Scholar] [CrossRef]
- Bianchi, N.; Bolognani, S.; Bon, D.; Pré, M.D. Torque Harmonic Compensation in a Synchronous Reluctance Motor. IEEE Trans. Energy Convers. 2008, 23, 466–473. [Google Scholar] [CrossRef]
- Tawfiq, K.B.; Ibrahim, M.N.; El-Kholy, E.E.; Sergeant, P. Performance Improvement of Existing Three Phase Synchronous Reluctance Machine: Stator Upgrading to 5-Phase With Combined Star-Pentagon Winding. IEEE Access 2020, 8, 143569–143583. [Google Scholar] [CrossRef]
- Ibrahim, M.N.F.; Sergeant, P.; Rashad, E.M. Relevance of Including Saturation and Position Dependence in the Inductances for Accurate Dynamic Modeling and Control of SynRMs. IEEE Trans. Ind. Appl. 2017, 53, 151–160. [Google Scholar] [CrossRef]
- Vagati, A. Synchronous Reluctance Drives: A New Alternative (Tutorial Course Notes); IEEE Press: Piscataway, NJ, USA, 1994; Chapter 6; pp. 1–27. [Google Scholar]
- Ibrahim, M.N.; Sergeant, P.; Rashad, E.M. Synchronous Reluctance Motor Performance Based on Different Electrical Steel Grades. IEEE Trans. Magn. 2015, 51, 1–4. [Google Scholar] [CrossRef]
- Zhao, W.; Zhao, F.; Lipo, T.A.; Kwon, B.-I. Optimal Design of a Novel V-Type Interior Permanent Magnet Motor with Assisted Barriers for the Improvement of Torque Characteristics. IEEE Trans. Magn. 2014, 50, 1–4. [Google Scholar] [CrossRef]
- Davoli, M.; Bianchini, C.; Torreggiani, A.; Immovilli, F. A design method to reduce pulsating torque in PM assisted synchronous reluctance machines with asymmetry of rotor barriers. In Proceedings of the IECON 2016—42nd Annual Conference of the IEEE Industrial Electronics Society, Florence, Italy, 24–26 October 2016; pp. 1566–1571. [Google Scholar]
- Baek, J.; Bonthu, S.; Choi, S. Design of five-phase permanent magnet assisted synchronous reluctance machine for low output torque ripple applications. IET Electr. Power Appl. 2016, 10, 339–346. [Google Scholar] [CrossRef]
- Babetto, C.; Bacco, G.; Bianchi, N. Synchronous Reluctance Machine Optimization for High-Speed Applications. IEEE Trans. Energy Convers. 2018, 33, 1266–1273. [Google Scholar] [CrossRef]
- Howard, E.; Kamper, M.J. Weighted Factor Multiobjective Design Optimization of a Reluctance Synchronous Machine. IEEE Trans. Ind. Appl. 2016, 52, 2269–2279. [Google Scholar] [CrossRef]
- Cupertino, F.; Pellegrino, G.; Armando, E.; Gerada, C. A SyR and IPM machine design methodology assisted by optimization algorithms. In Proceedings of the 2012 IEEE Energy Conversion Congress and Exposition (ECCE), Raleigh, NC, USA, 15–20 September 2012; pp. 3686–3691. [Google Scholar] [CrossRef]
- Pellegrino, G.; Cupertino, F.; Gerada, C. Automatic Design of Synchronous Reluctance Motors Focusing on Barrier Shape Optimization. IEEE Trans. Ind. Appl. 2015, 51, 1465–1474. [Google Scholar] [CrossRef]
- Howard, E.; Kamper, M.J.; Gerber, S. Asymmetric Flux Barrier and Skew Design Optimization of Reluctance Synchronous Machines. IEEE Trans. Ind. Appl. 2015, 51, 3751–3760. [Google Scholar] [CrossRef]
- Cupertino, F.; Pellegrino, G.; Gerada, C. Design of Synchronous Reluctance Motors With Multiobjective Optimization Algorithms. IEEE Trans. Ind. Appl. 2014, 50, 3617–3627. [Google Scholar] [CrossRef]
- Vagati, A.; Pastorelli, M.A.; Francheschini, G.; Petrache, S.C. Design of low-torque-ripple synchronous reluctance motors. IEEE Trans. Ind. Appl. 1998, 34, 758–765. [Google Scholar] [CrossRef]
- Muteba, M.; Twala, B.; Nicolae, D.V. Torque ripple minimization in synchronous reluctance motor using a sinusoidal rotor lamination shape. In Proceedings of the 2016 XXII International Conference on Electrical Machines (ICEM), Lausanne, Switzerland, 4–7 September 2016; pp. 606–611. [Google Scholar]
- Arafat, A.; Choi, S. Active current harmonic suppression for torque ripple minimization at open phase faults in a five-phase PMa-SynRM. IEEE Trans. Ind. Electron. 2019, 66, 922–931. [Google Scholar] [CrossRef]
- Bianchi, N.; Degano, M.; Fornasiero, E. Sensitivity analysis of torque ripple reduction of synchronous reluctance and interior PM motors. IEEE Trans. Ind. Appl. 2015, 51, 187–195. [Google Scholar] [CrossRef]
- Donaghy-Spargo, C.M. Electromagnetic–mechanical design of synchronous reluctance rotors with fine features. IEEE Trans. Magn. 2017, 53, 1–8. [Google Scholar] [CrossRef]
- Bao, Y.; Degano, M.; Wang, S.; Chuan, L.; Zhang, H.; Xu, Z.; Gerada, C. A Novel Concept of Ribless Synchronous Reluctance Motor for Enhanced Torque Capability. IEEE Trans. Ind. Electron. 2020, 67, 2553–2563. [Google Scholar] [CrossRef]
- Taghavi, S.; Pillay, P. A novel grain-oriented lamination rotor core assembly for a synchronous reluctance traction motor with a reduced torque ripple algorithm. IEEE Trans. Ind. Appl. 2016, 52, 3729–3738. [Google Scholar] [CrossRef]
- Moghaddam, R.R. Synchronous Reluctance Machine (SynRM) in Variable Speed Drives (VSD) Applications. Ph.D. Thesis, KTH Royal Institute of Technology, Stockholm, Sweden, 2011. [Google Scholar]
- Ibrahim, M.N.; Sergeant, P.; Rashad, E. Simple Design Approach for Low Torque Ripple and High Output Torque Synchronous Reluctance Motors. Energies 2016, 9, 942. [Google Scholar] [CrossRef]
- Guo, F.; Brown, I.P. Simultaneous Magnetic and Structural Topology Optimization of Synchronous Reluctance Machine Rotors. IEEE Trans. Magn. 2020, 56, 1–12. [Google Scholar] [CrossRef]
- Uberti, F.; Frosini, L.; Szabo, L. An Optimization Procedure for a Synchronous Reluctance Machine with Fluid Shaped Flux Barriers. In Proceedings of the 2020 International Conference on Electrical Machines (ICEM), Gothenburg, Sweden, 23–26 August 2020; pp. 389–395. [Google Scholar] [CrossRef]
- Lolova, I.; Barta, J.; Bramerdorfer, G.; Silber, S. Topology optimization of line-start synchronous reluctance machine. In Proceedings of the 2020 19th International Conference on Mechatronics—Mechatronika (ME), Prague, Czech Republic, 2–4 December 2020; pp. 1–7. [Google Scholar] [CrossRef]
- Eberhart, R.; Kennedy, J. A new optimizer using particle swarm theory. In Proceedings of the Sixth International Symposium on Micro Machine and Human Science, MHS’95, Nagoya, Japan, 4–6 October 1995; pp. 39–43. [Google Scholar]
- Abdalla, O.; Rezk, H.; Ahmed, E.M. Wind driven optimization algorithm based global MPPT for PV system under non-uniform solar irradiance. Sol. Energy 2019, 180, 429–444. [Google Scholar] [CrossRef]
- Mohamed, A.; Diab, A.A.Z.; Rezk, H. Partial shading mitigation of PV systems via different meta-heuristic techniques. Renew. Energy 2019, 130, 1159–1175. [Google Scholar] [CrossRef]
- Şenel, F.A.; Gökçe, F.; Yüksel, A.S.; Yiğit, T. A novel hybrid PSO–GWO algorithm for optimization problems. Eng. Comput. 2019, 35, 1359–1373. [Google Scholar] [CrossRef]
- Wang, K.; Zhu, Z.Q. Torque ripple reduction of synchronous reluctance machines optimal slot/pole and flux-barrier layer number combinations. Int. J. Comput. Math. Electr. Electron. Eng. 2015, 34, 3–17. [Google Scholar] [CrossRef]
- Palmieri, M.; Perta, M.; Cupertino, F.; Pellegrino, G. Effect of the numbers of slots and barriers on the optimal design of synchronous reluctance machines. In Proceedings of the 2014 International Conference on Optimization of Electrical and Electronic Equipment (OPTlM), Brasov, Romania, 22–24 May 2014. [Google Scholar]
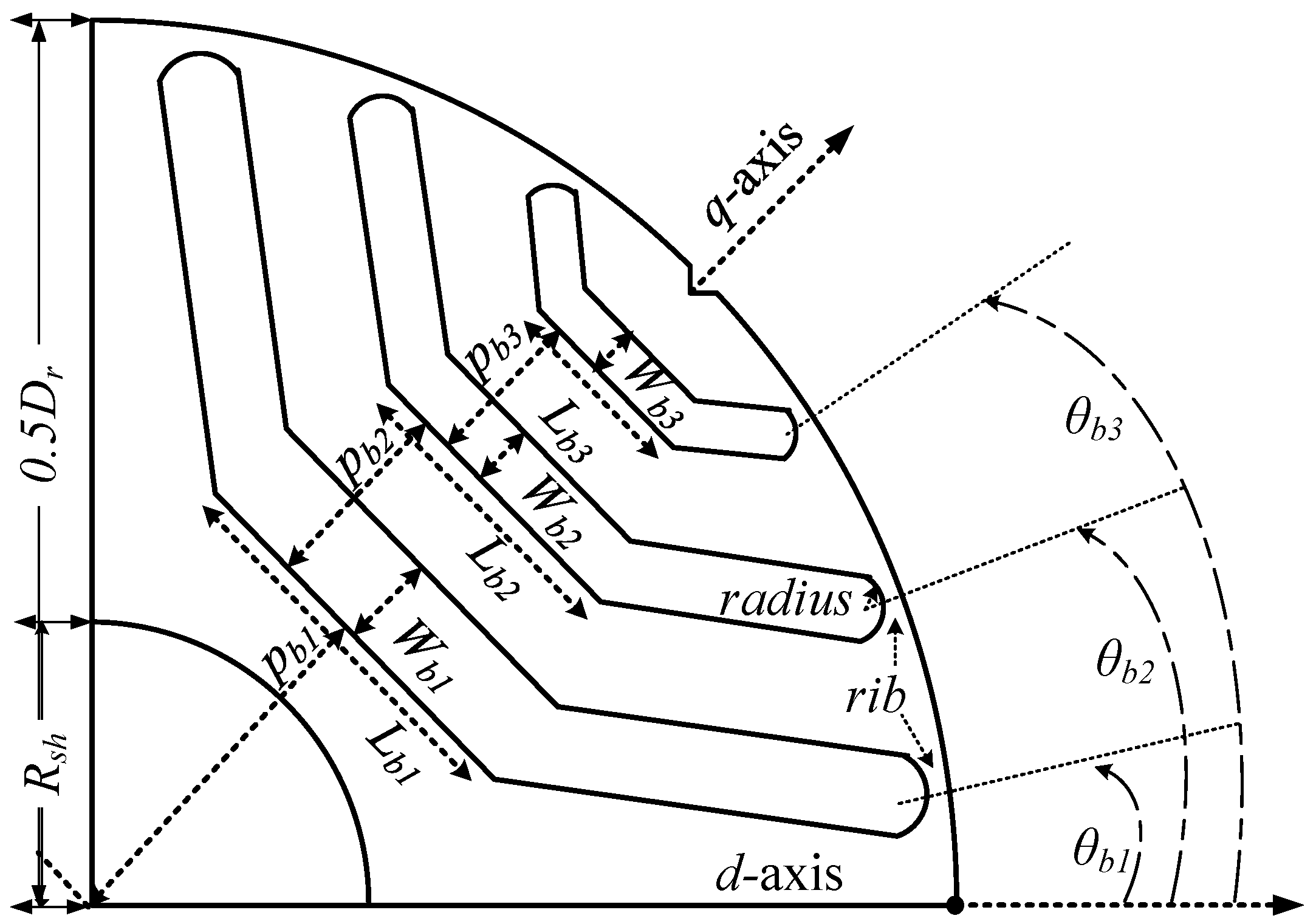
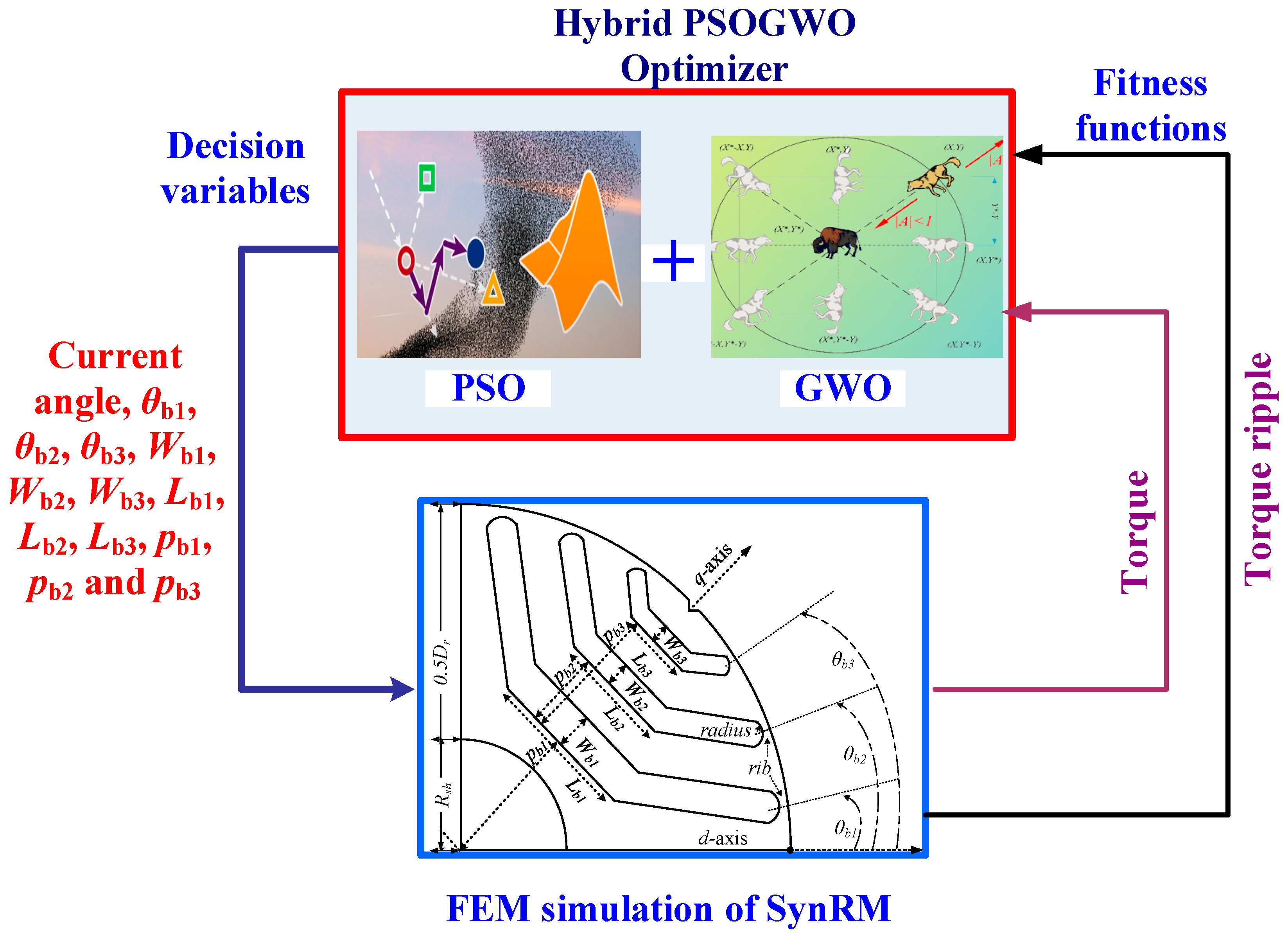






| Parameter | Value | Parameter | Value |
|---|---|---|---|
| Stator inner diameter | 110 mm | Air gap length | 0.3 mm |
| Stator outer diameter | 180 mm | Slots | 36 |
| Rotor outer diameter | 109.4 mm | poles | 4 |
| Shaft diameter | 35 mm | Rated frequency | 100 Hz |
| Axial length | 140 mm | Rated power | 5.5 kW |
| Rotor flux barriers per pole | 3 | Number of phases | 3 |
| Stator/Rotor steel | M270-50A/M330-50A | Rms rated current | 12.3 A |
| Variable | Lower Limit | Upper Limit |
|---|---|---|
| 5° | 9.3° | |
| 15° | 20° | |
| 25° | 30° | |
| Wb1 | 6 mm | 8.3 mm |
| Wb2 | 5 mm | 6.5 mm |
| Wb3 | 3 mm | 4 mm |
| Lb1 | 20 mm | 30 mm |
| Lb2 | 20 mm | 25 mm |
| Lb3 | 10 mm | 16 mm |
| pb1 | 20 mm | 23 mm |
| pb2 | 9 mm | 13.6 mm |
| pb3 | 8 mm | 11.8 mm |
| radius1,2,3 | 25% of Wb1,2,3 | |
| Case Number | Range of Current Angle |
|---|---|
| Case 1 | 30°: 40° |
| Case 2 | 40°: 45° |
| Case 3 | 45° |
| Case 4 | 45°: 65° |
| Case 5 | 50°: 55° |
| Case 1 | Case 2 | Case 3 | Case 4 | Case 5 | |
|---|---|---|---|---|---|
| Current angle range [Deg.] | 30°: 40° | 40°: 45° | 45° | 45°: 65° | 50°: 55° |
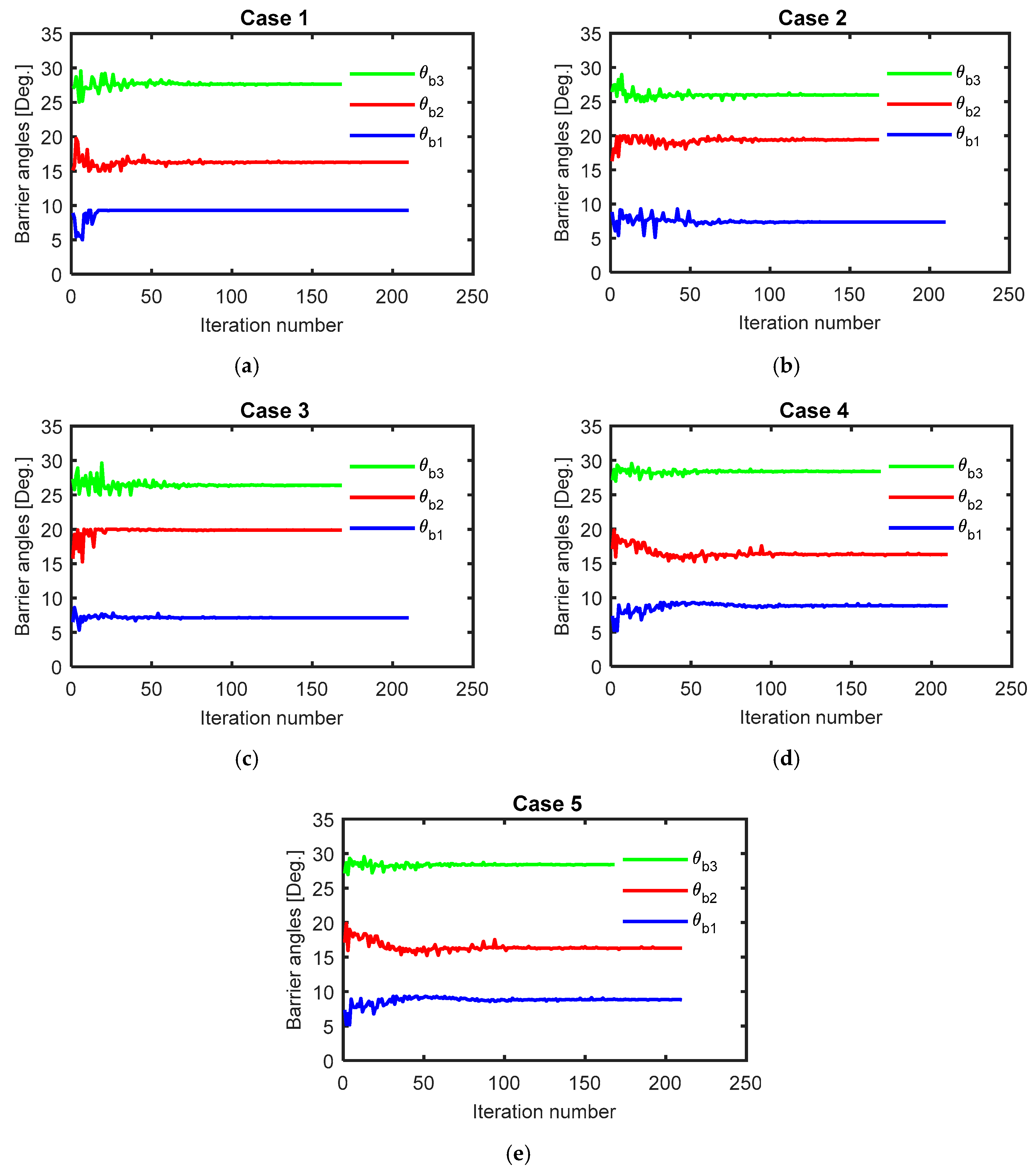
| Optimal angles [Deg.] , | 9.3°, 16.27° and 27.65° | 7.36°, 19.42° and 25.98° | 7.14°, 19.89° and 26.39° | 8.82°, 16.3° and 28.39° | 7.84°, 18.66° and 25.69° |
| Optimal widths [mm] Wb1, Wb2, Wb3 | 8.3, 5.8 and 3.425 | 7.7, 6.49 and 3.44 | 6.76, 5.05 and 3.49 | 8.3, 5.8 and 4 | 6.71, 6.5 and 3 |
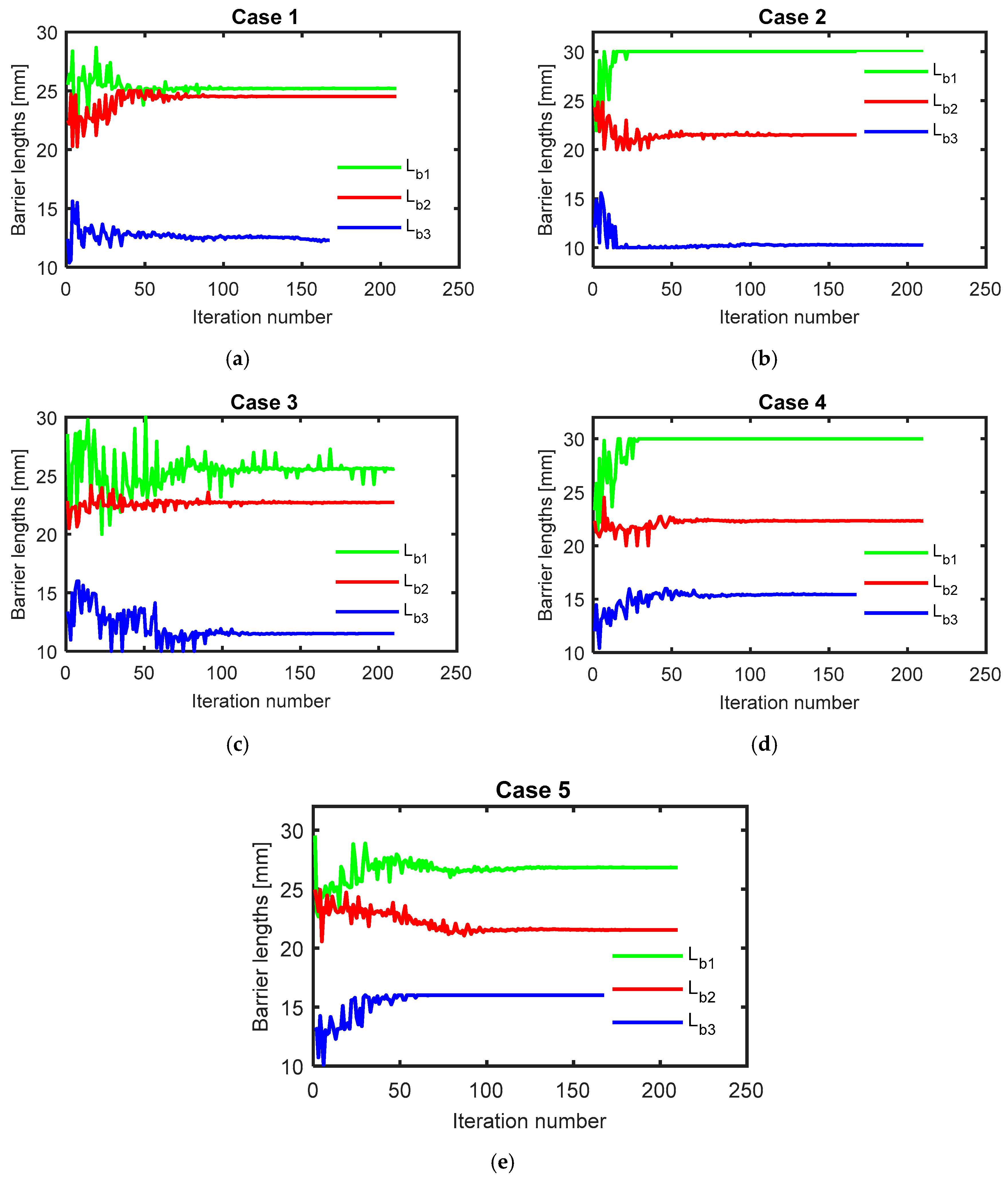
| Optimal lengths [mm] Lb1, Lb2, Lb3 | 25.2, 24.52 and 12.38 | 30, 21.5 and 10.26 | 25.6, 22.7 and 11.5 | 30, 22.32 and 15.41 | 26.82, 21.5 and 16 |
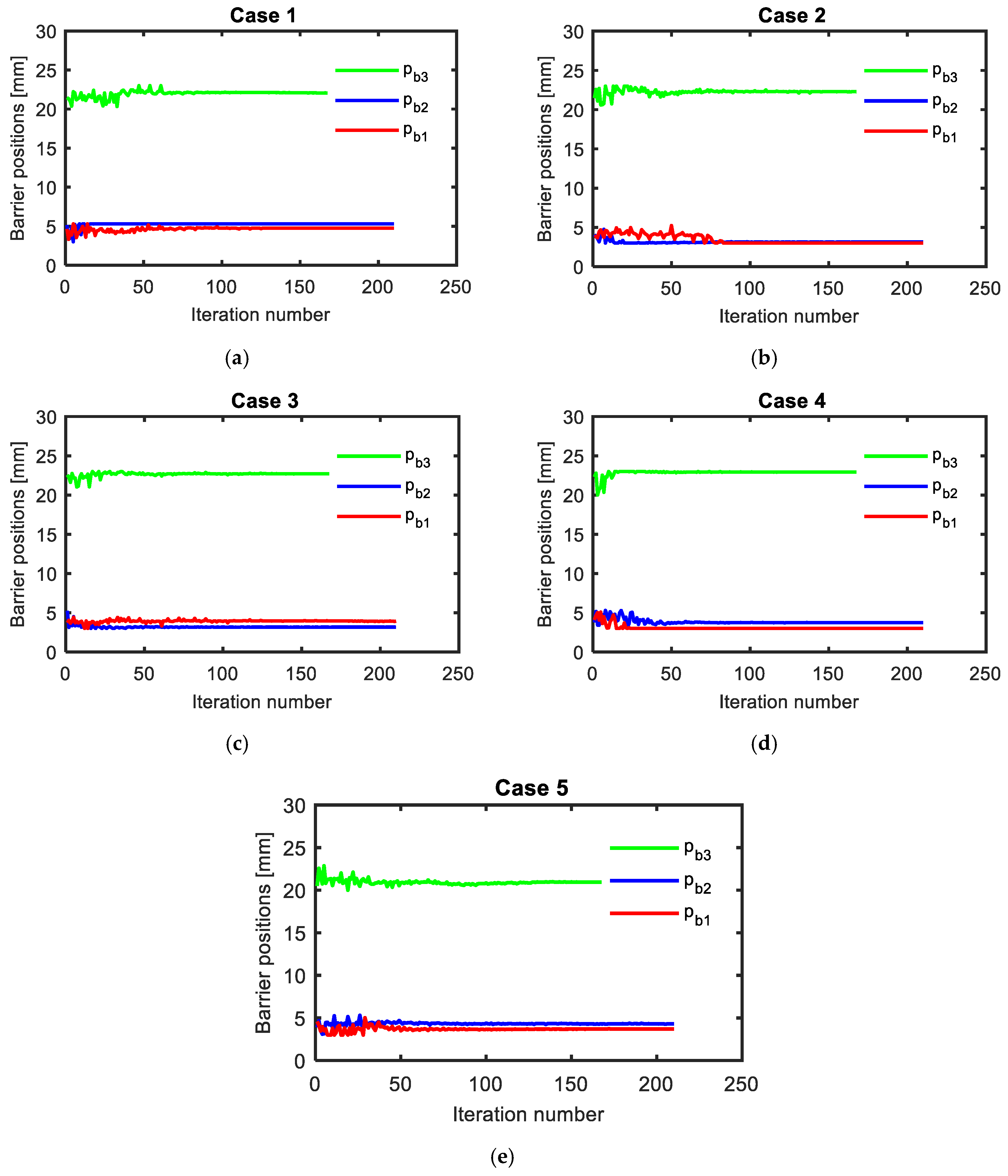
| Optimal positions [mm] pb1, pb2, pb3 | 22.06, 5.3 and 4.75 | 22.29, 3.13 and 3 | 22.71, 3.9 and 3.17 | 22.93, 3.74 and 3 | 20.95, 4.3 and 3.7 |
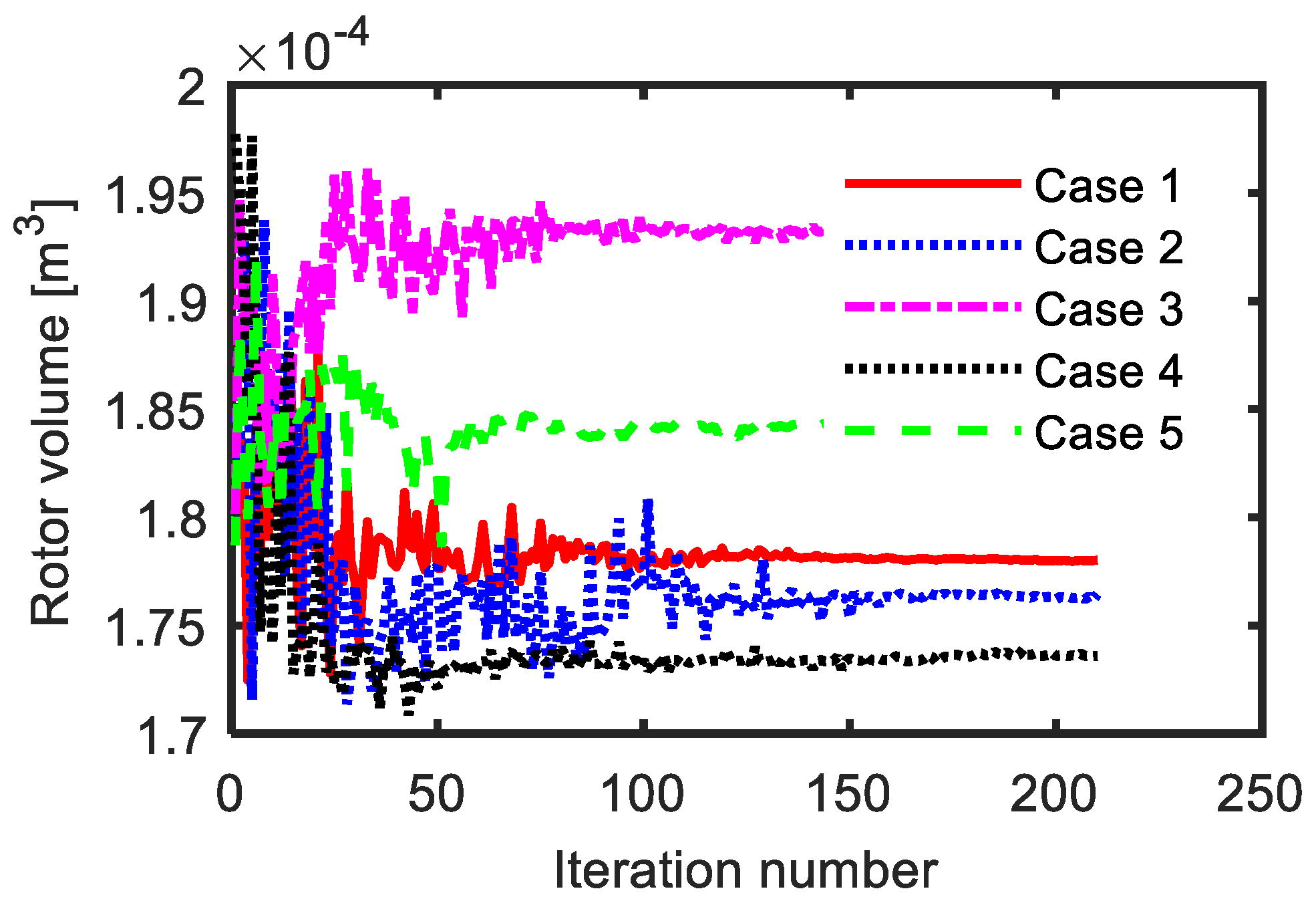
| Rotor iron volume [m3] | 1.780 × 10−4 | 1.763 × 10−4 | 1.932 × 10−4 | 1.736 × 10−4 | 1.842 × 10−4 |
| Case 1 | Case 2 | Case 3 | Case 4 | Case 5 | |
|---|---|---|---|---|---|
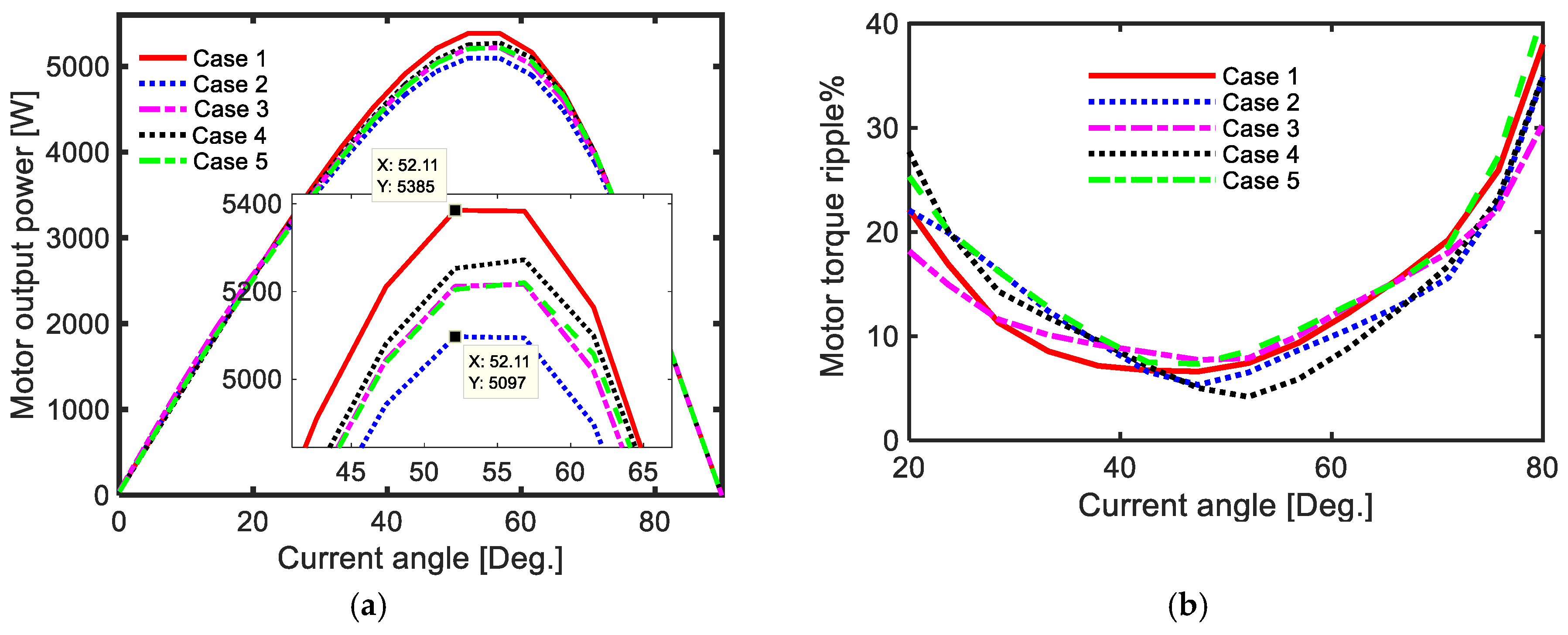
| Optimal current angle | 52.11° | 52.11° | 52.11° | 56.8° | 56.8° |
| Output power at optimal current angle [W] | 5385 | 5097 | 5212 | 5273 | 5221 |
| Torque ripple at optimal current angle [%] | 7.4 | 6.5 | 7.9 | 5.85 | 10.58 |
| Power factor at optimal current angle | 0.6297 | 0.6185 | 0.6182 | 0.6628 | 0.6555 |
| Saliency ratio at optimal current angle [%] | 5.36 | 4.84 | 4.8 | 5.25 | 5.06 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rezk, H.; Tawfiq, K.B.; Sergeant, P.; Ibrahim, M.N. Optimal Rotor Design of Synchronous Reluctance Machines Considering the Effect of Current Angle. Mathematics 2021, 9, 344. https://doi.org/10.3390/math9040344
Rezk H, Tawfiq KB, Sergeant P, Ibrahim MN. Optimal Rotor Design of Synchronous Reluctance Machines Considering the Effect of Current Angle. Mathematics. 2021; 9(4):344. https://doi.org/10.3390/math9040344
Chicago/Turabian StyleRezk, Hegazy, Kotb B. Tawfiq, Peter Sergeant, and Mohamed N. Ibrahim. 2021. "Optimal Rotor Design of Synchronous Reluctance Machines Considering the Effect of Current Angle" Mathematics 9, no. 4: 344. https://doi.org/10.3390/math9040344
APA StyleRezk, H., Tawfiq, K. B., Sergeant, P., & Ibrahim, M. N. (2021). Optimal Rotor Design of Synchronous Reluctance Machines Considering the Effect of Current Angle. Mathematics, 9(4), 344. https://doi.org/10.3390/math9040344









