The Integral Mittag-Leffler, Whittaker and Wright Functions
Abstract
1. Introduction
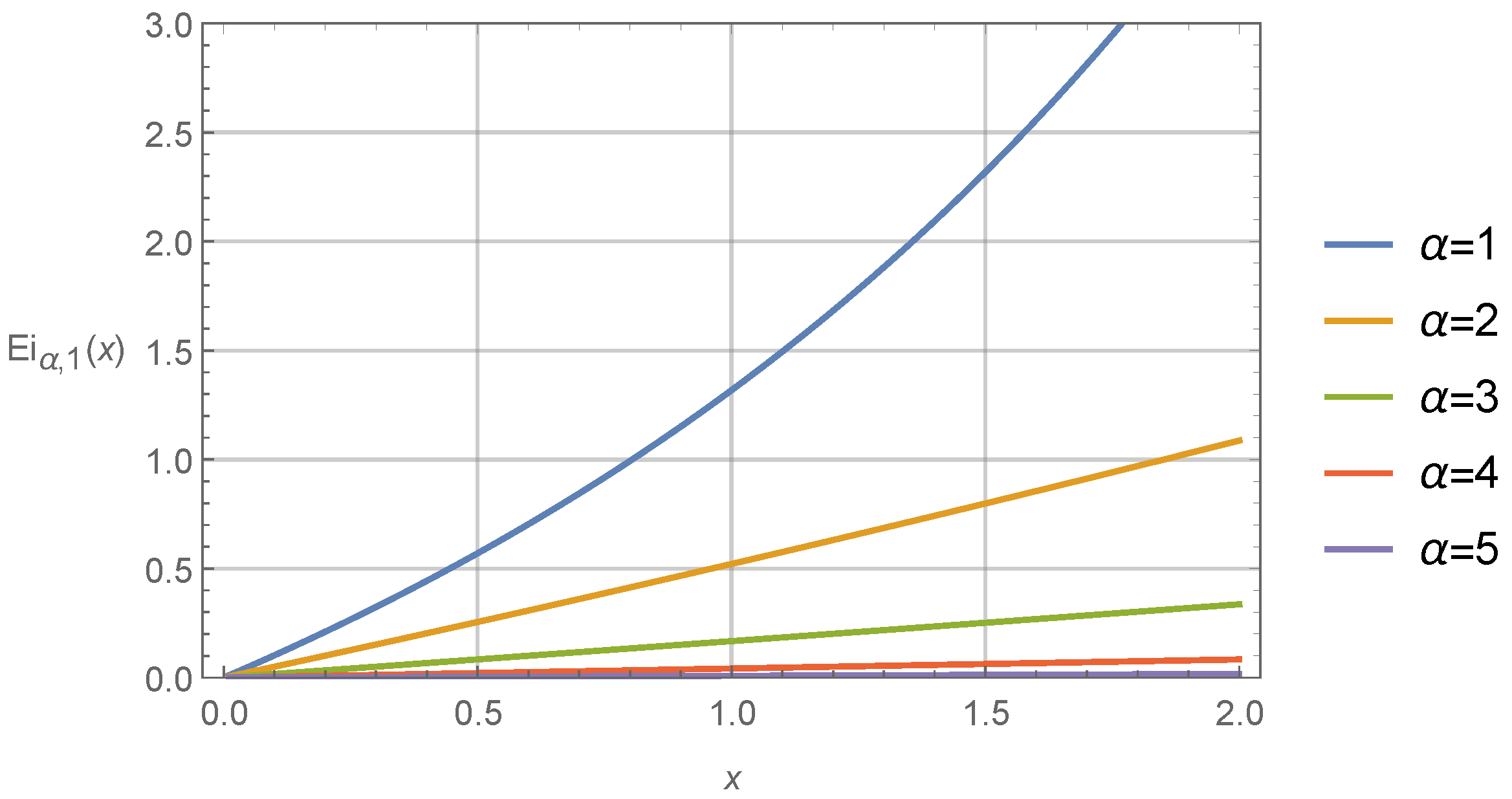
2. The Integral Mittag-Leffler Functions
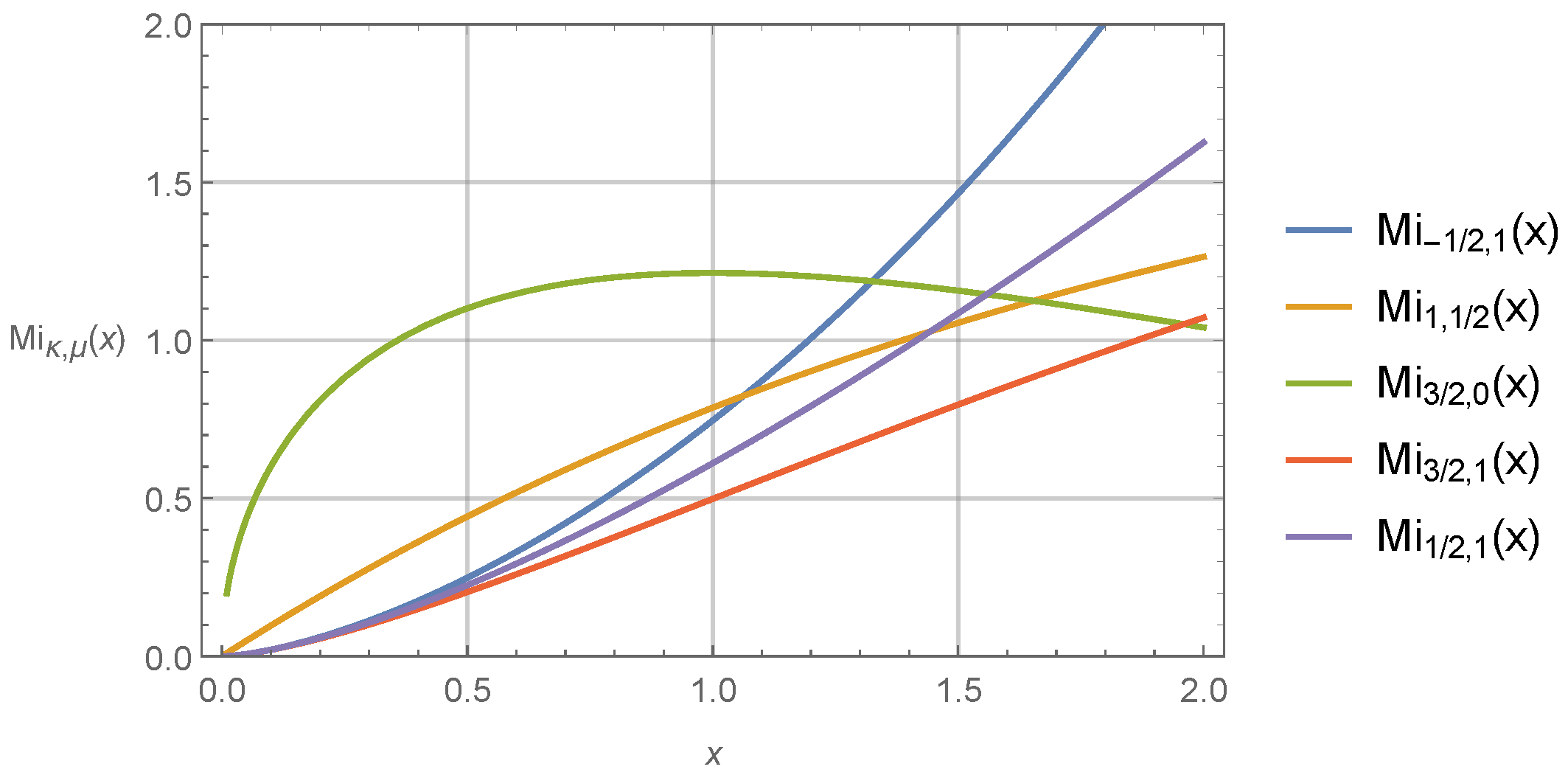
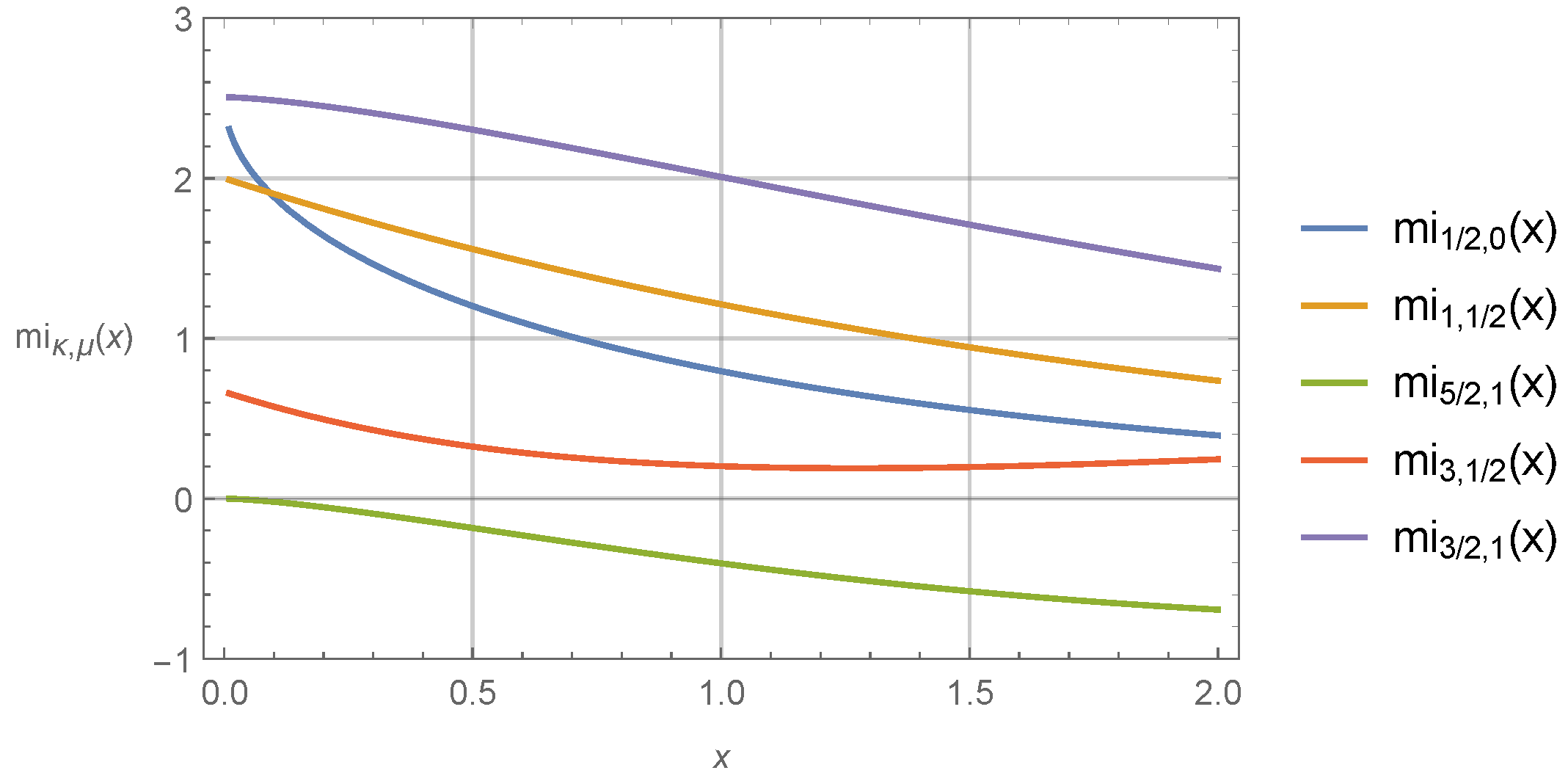
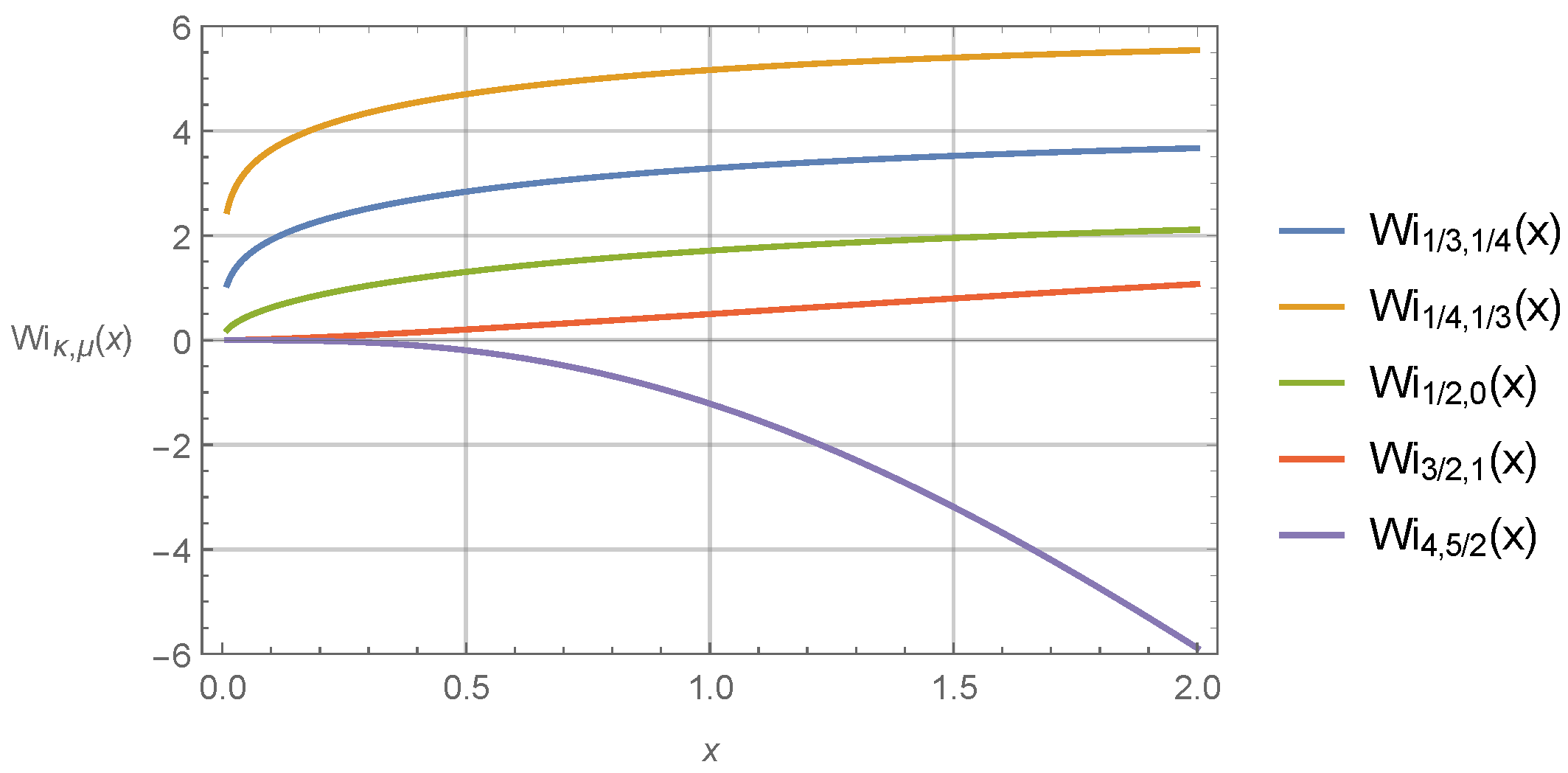
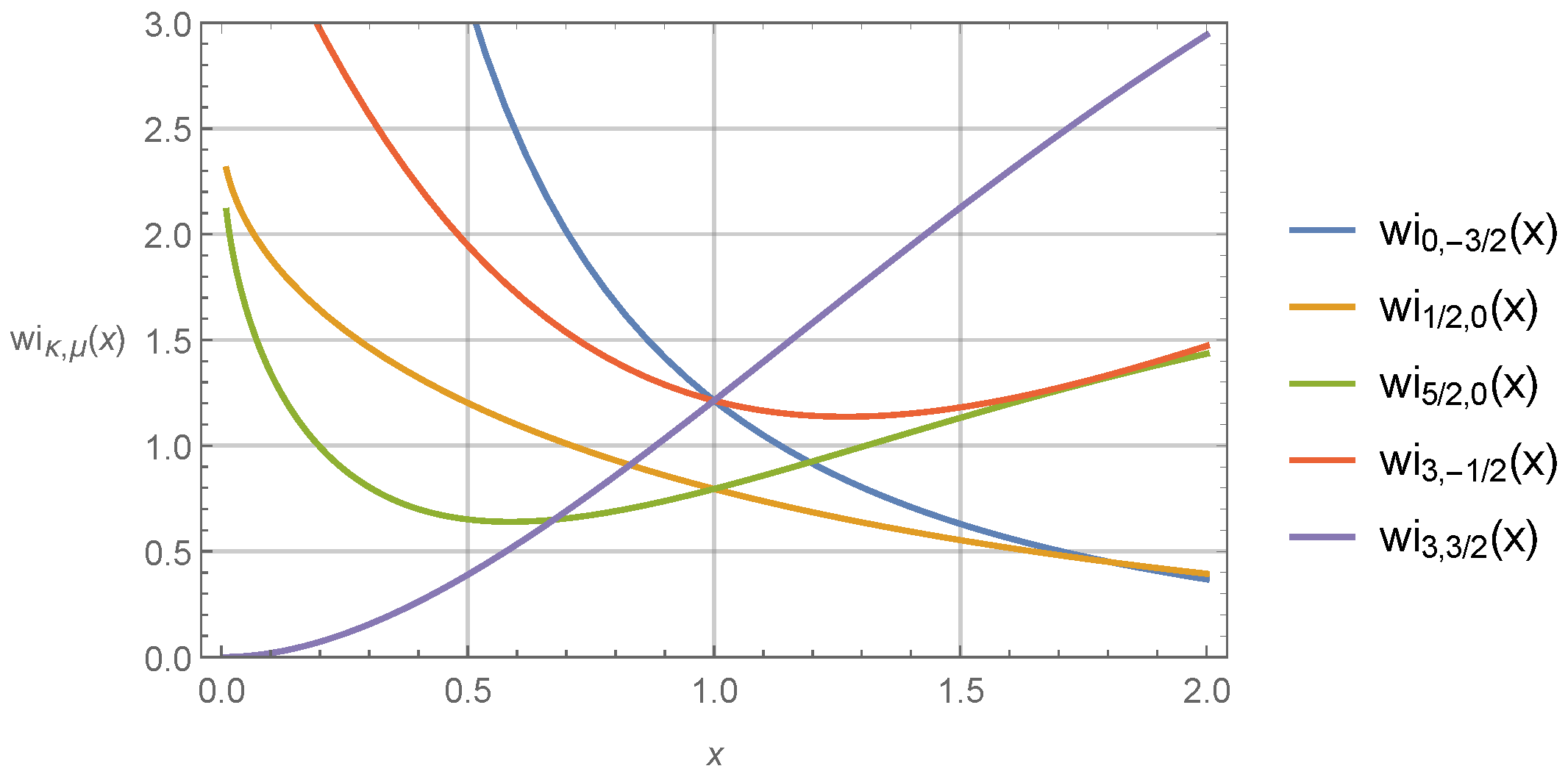
3. The Integral Whittaker Functions
4. The Integral Wright Functions
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Representations of the One- and Two-Parameter Mittag-Leffler Functions and Their Laplace Transforms
| 1 | ||
| 2 | ||
| 3 | ||
| 4 | ||
| 1 | ||
| 1 | 1 | |
| 1 | ||
| 1 | 2 | |
| 1 | ||
| 2 | ||
| 2 | ||
| 2 | 1 | |
| 2 | 2 | |
| 2 | 3 | |
| 2 | 4 | |
| 2 |
| 3 | 1 | |
| 3 | 2 | |
| 3 | 3 | |
| 3 | ||
| 4 | 1 | |
| 4 | 2 | |
| 4 | 3 | |
| 4 | 4 | |
| 4 | ||
| 5 | 1 | |
| 5 | 2 | |
| 5 | 3 | |
| 5 | 4 | |
| 5 | 5 | |
| 5 |
| 1 | ||
| 1 | ||
| 1 | ||
| 1 | 2 | |
| 1 | ||
| 1 | ||
| 2 | ||
| 2 | ||
| 2 | 1 | |
| 2 | 2 | |
| 2 | 3 | |
| 2 | 4 | |
| 2 |
| 3 | 1 | |
| 3 | 2 | |
| 3 | 3 | |
| 3 | ||
| 4 | 1 | |
| 4 | 2 | |
| 4 | 3 | |
| 4 | 4 | |
| 4 | ||
| 5 | 1 | |
| 5 | 2 | |
| 5 | 3 | |
| 5 | 4 | |
| 5 | 5 | |
| 5 |
Appendix B. Representations of the Whittaker Functions and Their Laplace Transforms
| 0 | ||
| 0 | ||
| 1 | ||
| 2 | ||
| 3 | ||
| 0 | ||
| 0 | ||
| 0 | ||
| 1 | ||
| 2 | ||
| 0 | ||
| 0 | ||
| 0 | ||
| 0 | ||
| 0 | ||
| 0 |
| 0 | ||
| 0 | 1 | |
| 0 | ||
| 0 | 2 | |
| 0 | ||
| 0 | ||
| 0 | ||
| 0 | ||
| 0 | ||
| 1 | ||
| 1 | 0 | |
| 1 | ||
| 1 | 1 | |
| 0 | ||
| 1 | ||
| 2 | 0 | |
| 2 | ||
| 2 | 1 | |
| 2 | ||
| 2 | 2 | |
| 0 | ||
| 1 | ||
| 2 | ||
| 3 | ||
| 3 | 1 | |
| 3 | ||
| 3 | ||
| 0 | ||
| 4 | ||
| 4 |
| 0 | ||
| 0 | ||
| 1 | ||
| 0 | ||
| 0 | ||
| 0 | ||
| 1 | ||
| 0 | 1 | |
| 0 | 2 | |
| 0 |
| 0 | ||
| 0 | ||
| 0 | ||
| 1 | ||
| 1 | 0 | |
| 1 | ||
| 1 | 1 | |
| 0 | ||
| 1 | ||
| 2 | 0 | |
| 2 | ||
| 2 | 1 | |
| 2 |
| 1 | ||
| 2 | ||
| 3 | ||
| 3 | ||
| 3 | ||
| 0 | ||
| 4 | ||
| 4 |
| 0 | ||
| 0 | ||
| 1 | ||
| 0 | ||
| 1 | ||
| 2 | ||
| 3 | ||
| 0 | ||
| 0 | ||
| 0 | ||
| 0 | ||
| 0 | ||
| 1 | ||
| 1 | ||
| 0 | ||
| 1 | ||
| 2 | ||
| 2 | ||
| 2 | ||
| 2 | ||
| 0 | ||
| 1 | ||
| 2 | ||
| 3 | ||
| 3 | ||
| 3 | ||
| 3 | ||
| 0 | ||
| 4 | ||
| 4 |
| 0 | ||
| 1 | ||
| 0 | ||
| 0 | 1 | |
| 0 | ||
| 1 | ||
| 0 | ||
| 1 | ||
| 2 | ||
| 2 | ||
| 0 | ||
| 1 | ||
| 2 | ||
| 3 | ||
| 3 | ||
| 3 | ||
| 0 | ||
| 4 | ||
| 4 |
Appendix C. Representations of the Wright Functions
| 0 | ||
| 1 | ||
| 0 | ||
| 0 | ||
| 0 | 1 | |
| 0 | ||
| 1 | ||
| 1 | ||
| 1 | ||
| 1 | 0 | |
| 1 | ||
| 1 | 1 | |
| 1 | ||
| 1 |
| 2 | ||
| 3 | ||
| 4 | ||
| 5 |
References
- Erdélyi, A.; Magnus, W.; Oberhettinger, F.; Tricomi, F. Higher Transcendental Functions; McGraw-Hill: New York, NY, USA, 1953; Volume 1. [Google Scholar]
- Erdélyi, A.; Magnus, W.; Oberhettinger, F.; Tricomi, F. Tables of Integral Transforms; McGraw-Hill: New York, NY, USA, 1954. [Google Scholar]
- Abramowitz, M.; Stegun, I. Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables; U.S. National Bureau of Standards: Washington, DC, USA, 1964; Volume 55.
- Magnus, W.; Oberhettinger, F.; Soni, R. Formulas and Theorems for the Special Functions of Mathematical Physics, 3rd ed.; Springer: Berlin, Germany, 1966. [Google Scholar]
- Olver, F.; Lozier, D.; Boisvert, R.; Clark, C. NIST Handbook of Mathematical Functions; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Roberts, G.; Kaufman, H. Table of Laplace Transforms; WB Saunders Co.: Philadelphia, PA, USA, 1966. [Google Scholar]
- Oberhettinger, F.; Badii, L. Tables of Laplace Transforms; Springer: Berlin, Germany, 1970. [Google Scholar]
- Apelblat, A. Laplace Transforms and Their Applications; Nova Science Publishers Inc.: New York, NY, USA, 2012. [Google Scholar]
- Van der Pol, B. On the operational solution of linear differential equations and an investigation of the properties of these solutions. Lond. Edinb. Dublin Philos. Mag. J. Sci. 1929, 8, 861–898. [Google Scholar] [CrossRef]
- Van der Pol, B.; Bremmer, H. Operational Calculus: Based on the Two-Sided Laplace Integral; Taylor & Francis: Abingdon, UK, 1987; Volume 327. [Google Scholar]
- Humbert, P. Bessel-integral functions. Proc. Edinb. Math. Soc. 1933, 3, 276–285. [Google Scholar] [CrossRef][Green Version]
- Apelblat, A.; Kravitsky, N. Integral representations of derivatives and integrals with respect to the order of the Bessel functions Jν(t), Iν(t), the Anger function Jν(t) and the integral Bessel function Jiν(t). IMA J. Appl. Math. 1985, 34, 187–210. [Google Scholar] [CrossRef]
- Apelblat, A. Bessel and Related Functions. Mathematical Operations with Respect to the Order; Theoretical Aspects; Walter de Gruyter GmbH: Berlin, Germany, 2020; Volume 1. [Google Scholar]
- Gorenflo, R.; Kilbas, A.; Mainardi, F.; Rogosin, S. Mittag-Leffler Functions, Related Topics and Applications; Springer: Berlin, Germany, 2014; Volume 2. [Google Scholar]
- Whittaker, E. An expression of certain known functions as generalized hypergeometric functions. Bull. Am. Math. Soc. 1903, 10, 125–134. [Google Scholar] [CrossRef]
- Prudnikov, A.; Brychkov, Y.; Marichev, O. Integrals and Series: More Specific Function; CRC Press: Boca Raton, FL, USA, 1986; Volume 3. [Google Scholar]
- Wright, E. On the coefficients of power series having exponential singularities. J. Lond. Math. Soc. 1933, 1, 71–79. [Google Scholar] [CrossRef]
- Wright, E. The generalized Bessel function of order greater than one. Q. J. Math. 1940, 1, 36–48. [Google Scholar] [CrossRef]
- Gorenflo, R.; Luchko, Y.; Mainardi, F. Analytical properties and applications of the Wright function. Fract. Calc. Appl. Anal. 1999, 2, 383–414. [Google Scholar]
- Mainardi, F. Fractional Calculus and Waves in Linear Viscoelasticity: An Introduction to Mathematical Models; World Scientific: Singapore, 2010. [Google Scholar]
- Apelblat, A. Differentiation of the Mittag-Leffler functions with respect to parameters in the Laplace transform approach. Mathematics 2020, 8, 657. [Google Scholar] [CrossRef]

| 1 | ||
| 2 | ||
| 3 | ||
| 4 | ||
| 1 | ||
| 1 | ||
| 1 | ||
| 1 | 1 | |
| 1 | ||
| 1 |
| 1 | ||
| 2 | ||
| 2 | ||
| 2 | ||
| 2 | ||
| 2 | 1 | |
| 2 | 2 | |
| 2 | 3 | |
| 2 | 4 | |
| 2 | ||
| 3 | 1 | |
| 3 | ||
| 4 | 1 | |
| 4 | ||
| 5 | 1 | |
| 5 |
| 1 | ||
| 1 | ||
| 1 | ||
| 1 | ||
| 1 | 1 | |
| 1 | ||
| 1 | ||
| 1 | ||
| 2 | ||
| 2 | ||
| 2 | ||
| 2 | ||
| 2 | ||
| 2 | 1 | |
| 2 | 2 | |
| 2 | 3 | |
| 2 | 4 | |
| 2 |
| 3 | ||
| 3 | ||
| 3 | ||
| 3 | ||
| 3 | 1 | |
| 3 | 3 | |
| 3 | ||
| 4 | 1 | |
| 4 | 4 | |
| 4 | ||
| 5 | 1 | |
| 5 |
| 0 | ||
| 0 | ||
| 1 | ||
| 2 | ||
| 3 | ||
| 0 | ||
| 1 | ||
| 2 | ||
| 0 | ||
| 0 | ||
| 0 | ||
| 0 | ||
| 0 | ||
| 0 | ||
| 0 | ||
| 0 | 1 | |
| 0 | ||
| 0 | 2 | |
| 0 |
| 0 | ||
| 1 | ||
| 1 | 0 | |
| 1 | ||
| 1 | 1 | |
| 0 | ||
| 1 | ||
| 2 | ||
| 2 | ||
| 2 |
| 0 | ||
| 1 | ||
| 1 | ||
| 2 | ||
| 2 | ||
| 1 | ||
| 2 | ||
| 3 | ||
| 3 | ||
| 4 | ||
| 4 |
| 0 | ||
| 0 | ||
| 1 | ||
| 1 | ||
| 0 | ||
| 1 | ||
| 2 | ||
| 2 | ||
| 2 | ||
| 0 | ||
| 3 | ||
| 3 | ||
| 4 | ||
| 4 | ||
| 4 | ||
| 4 |
| 1 | ||
| 2 | ||
| 3 | ||
| 0 | ||
| 0 | ||
| 0 | ||
| 0 | ||
| 1 | ||
| 1 | ||
| 1 | ||
| 0 | ||
| 1 | ||
| 2 | ||
| 2 | ||
| 0 | ||
| 1 | ||
| 3 | ||
| 3 | ||
| 3 | ||
| 3 | ||
| 4 | ||
| 4 | ||
| 4 |
| 0 | ||
| 0 | ||
| 0 | ||
| 1 | ||
| 2 | ||
| 1 | ||
| 3 | ||
| 1 | 0 | |
| 1 | ||
| 1 | ||
| 1 | 1 | |
| 1 | ||
| 1 |
| 2 | ||
| 2 | ||
| 2 | ||
| 2 | 1 | |
| 2 | 2 | |
| 2 | ||
| 3 | 1 | |
| 3 | ||
| 4 | ||
| 5 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Apelblat, A.; González-Santander, J.L. The Integral Mittag-Leffler, Whittaker and Wright Functions. Mathematics 2021, 9, 3255. https://doi.org/10.3390/math9243255
Apelblat A, González-Santander JL. The Integral Mittag-Leffler, Whittaker and Wright Functions. Mathematics. 2021; 9(24):3255. https://doi.org/10.3390/math9243255
Chicago/Turabian StyleApelblat, Alexander, and Juan Luis González-Santander. 2021. "The Integral Mittag-Leffler, Whittaker and Wright Functions" Mathematics 9, no. 24: 3255. https://doi.org/10.3390/math9243255
APA StyleApelblat, A., & González-Santander, J. L. (2021). The Integral Mittag-Leffler, Whittaker and Wright Functions. Mathematics, 9(24), 3255. https://doi.org/10.3390/math9243255







