Panic Spreading Model with Different Emotions under Emergency
Abstract
:1. Introduction
2. Level-Headed Group–Impatient Group Nonlinear SIRS Panic Spreading Model
- (I)
- Drawing on the divisions in personality by Jung [32], the groups were divided into a level-headed group and an impatient group. Level-headed individuals have meticulous thinking and will calm down in the face of difficulty. On the contrary, impatient individuals are reckless and adventurous, and are vulnerable to the emotional influence of others. The level-headed group and the impatient group are both classified into three categories: susceptible, infected, and recovered, denoted by S1, I1, and R1 and S2, I2, and R2 at time t, respectively.
- (II)
- Several traditional infection rates have been widely used in various fields, namely the bilinear infection rate, , and the standard infection rate, . A characteristic of these two infection rates is that the number of infected individuals increases linearly, which is obviously limited and overly idealistic for academic research. Whether it is the level-headed group or the impatient group, the panic emotion tends to show a nonlinear relationship with the group through the process of spreading, which is more in line with real situations. S and I in the infection rate are considered as a function of time t. The nonlinear infection rate is [33]
- (III)
- Emotion spreads not only within groups but also between different groups to susceptible people. Based on the characteristics of each group, we consider that the impatient group is affected by panic contagion, not only within the group but also occurring through cross infection from the level-headed group. Furthermore, in the level-headed group, panic is contagious from infected individuals to susceptible individuals.
- (IV)
- Susceptible individuals in the level-headed group and the impatient group can become recovered individuals; however, they do not have permanent immunity and may become susceptible individuals again with a certain probability.
- (i)
- If and , is unique in the panic-free equilibrium.
- (ii)
- If and , two equilibria exist, the panic-free equilibrium, , and the panic-permanence equilibrium, , respectively.
3. Stability Analysis of Level-Headed Group–Impatient Group SIRS Panic Spreading Model
3.1. Locally Stable Analysis of the Model
3.2. Global Stability Analysis of Level-Headed Sub-Model
3.3. Global Stability of the Model
- (i)
- If and , the panic-free equilibrium, , is globally asymptotically stable.
- (ii)
- If and , the panic-permanence equilibrium, , is globally asymptotically stable.
4. Numerical Simulations and Analysis
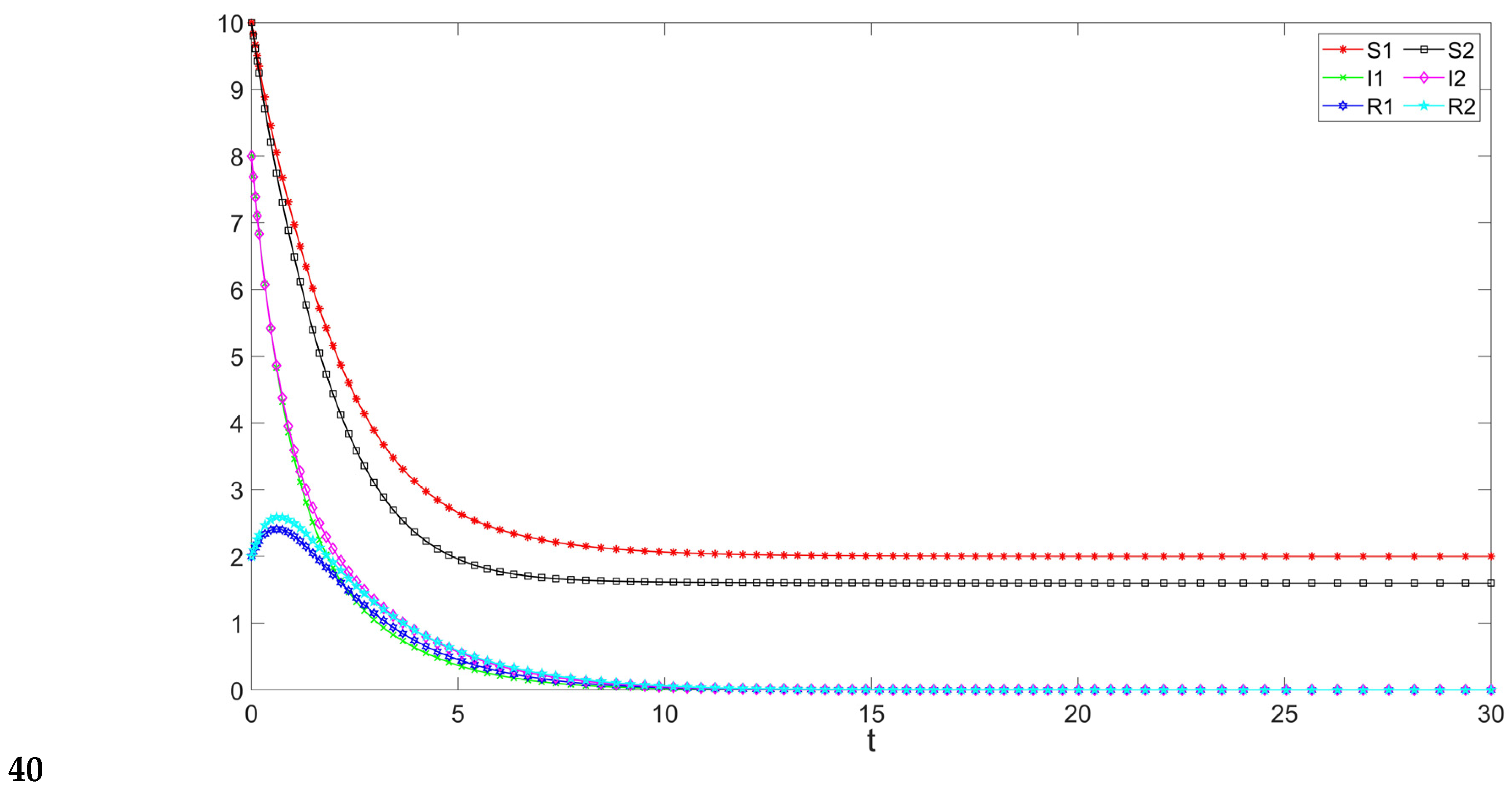
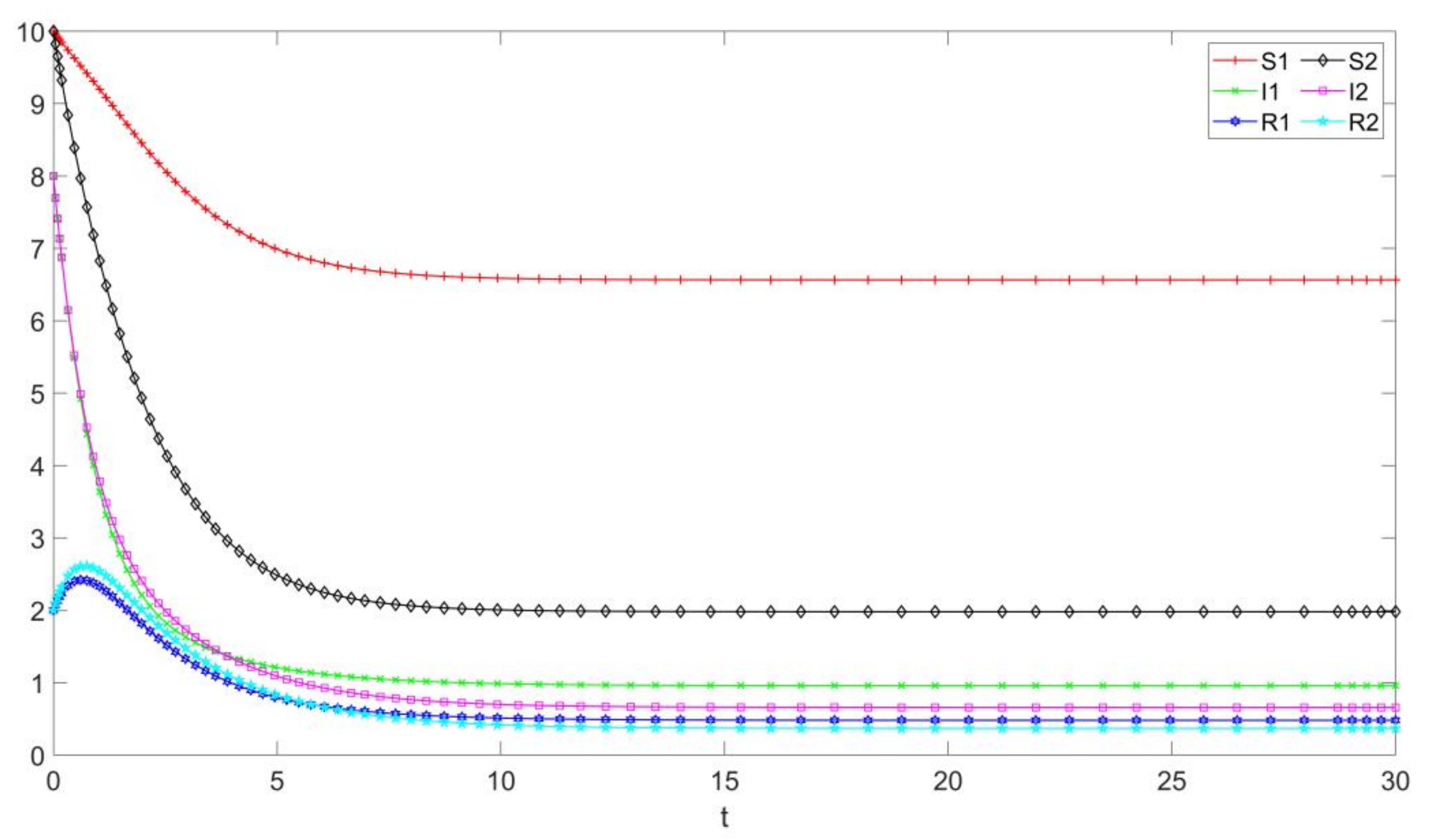
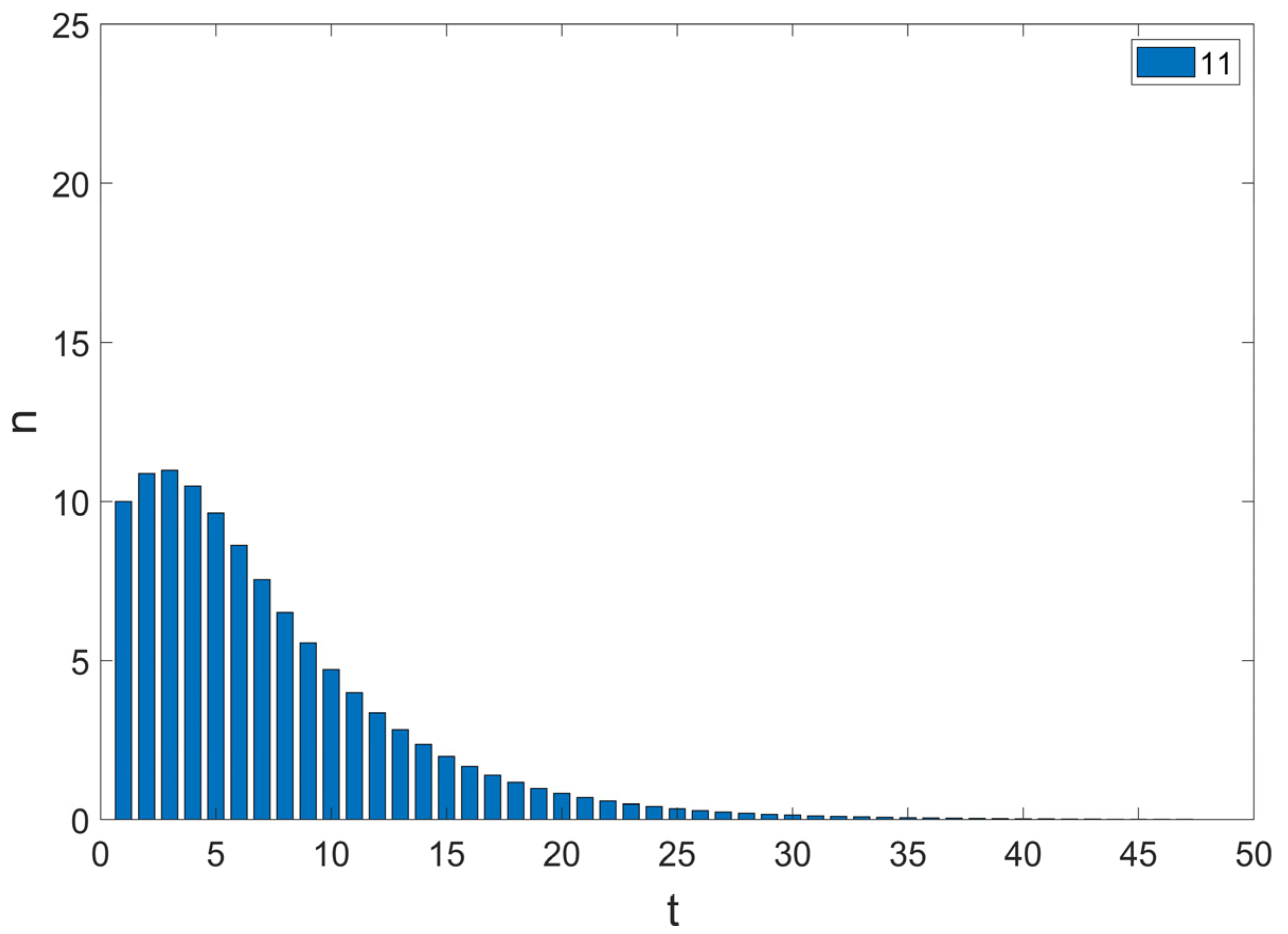
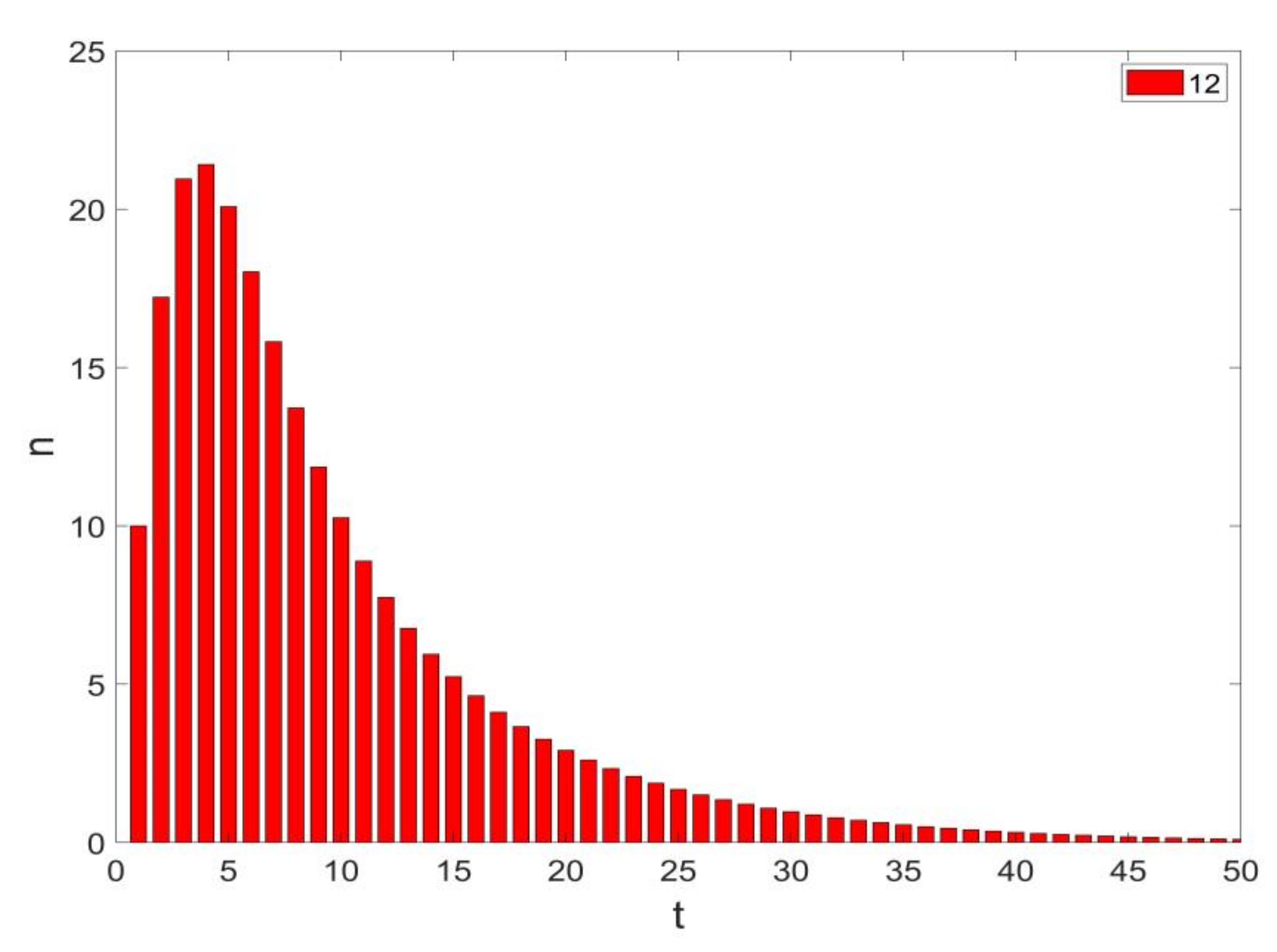
4.1. Simulation of Equilibrium Stability
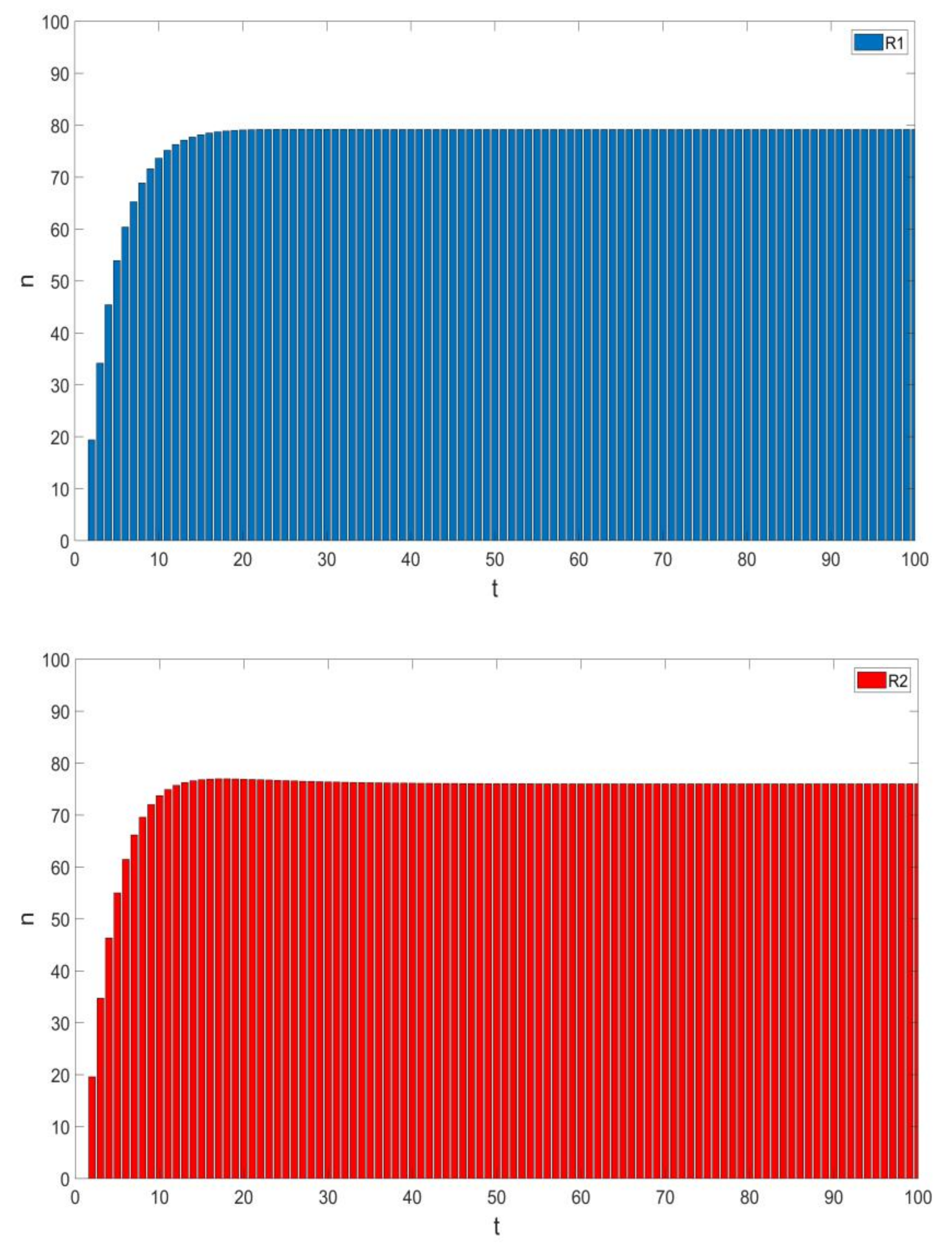
4.2. Analysis of Numerical Simulation
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
Appendix A.1. Proof of Theorem 2
Appendix A.2. Proof of Theorem 4
References
- Ross, W.; Gorod, A.; Ulieru, M. A Socio-Physical Approach to Systemic Risk Reduction in Emergency Response and Prepar-edness. IEEE Trans. Syst. Man Cybern. Syst. 2015, 45, 1125–1137. [Google Scholar]
- Mao, Y.; Li, Z.; Li, Y.; He, W. Emotion-based diversity crowd behavior simulation in public emergency. Vis. Comput. 2019, 35, 1725–1739. [Google Scholar] [CrossRef]
- Low, D.J. Following the crowd. Nature 2000, 407, 465–466. [Google Scholar] [CrossRef]
- Meier, S.T.; Bützler, J.; Schlick, C.M. The influence of information presented on digital escape route signage on decision-making under mentally and emotionally strenuous conditions. In Proceedings of 19th Triennial Congress of the IEA, Melbourne, Australia, 9–14 August 2015; Volume 1, p. 1. [Google Scholar]
- Che, X.; Niu, Y.; Shui, B.; Fu, J.; Fei, G.; Goswami, P.; Zhang, Y. A novel simulation framework based on information asymmetry to evaluate evacuation plan. Vis. Comput. 2015, 31, 853–861. [Google Scholar] [CrossRef]
- Fu, L.; Song, W.; Lo, S.M. A fuzzy-theory-based method for studying the effect of information transmission on nonlinear crowd dispersion dynamics. Commun. Nonlinear Sci. Numer. Simul. 2017, 42, 682–698. [Google Scholar] [CrossRef]
- Bon, G.L. The Crowd, A Study of the Popular Mind. Am. J. Sociol. 1897, 2, 734–735. [Google Scholar]
- Durupınar, F.; Gudukbay, U.; Aman, A.; Badler, N.I.; Durupinar, F. Psychological Parameters for Crowd Simulation: From Audiences to Mobs. IEEE Trans. Vis. Comput. Graph. 2015, 22, 2145–2159. [Google Scholar] [CrossRef]
- Olson, K.R. A Literature Review of Social Mood. J. Behav. Finance 2006, 7, 193–203. [Google Scholar] [CrossRef]
- Quarantelli, E.L. The Sociology of Panic. Int. Encycl. Soc. Behav. Sci. 2001, 11020–11023. [Google Scholar]
- Bosse, T.; Duell, R.; Memon, Z.A.; Treur, J.; van der Wal, C.N. Multi-Agent Model for Emotion Contagion Spirals Integrated within a Supporting Ambient Agent Model; Springer: Berlin/Heidelberg, Germany, 2009; pp. 48–67. [Google Scholar]
- Ta, X.H.; Gaudou, B.; Longin, D.; Ho, T.V. Emotional contagion model for group evacuation simulation. Informatica 2017, 41, 169–182. [Google Scholar]
- Li, C.; Lv, P.; Manocha, D.; Wang, H.; Li, Y.; Zhou, B.; Xu, M. ACSEE: Antagonistic Crowd Simulation Model with Emotional Contagion and Evolutionary Game Theory. IEEE Trans. Affect. Comput. 2019, 99, 1. [Google Scholar] [CrossRef]
- Liu, Z.; Zhang, T.; Lan, Q. An Extended SISa Model for Sentiment Contagion. Discret. Dyn. Nat. Soc. 2014, 2014, 1–7. [Google Scholar] [CrossRef]
- Bosse, T.; Duell, R.; Memon, Z.; Treur, J.; van der Wal, N. Agent-Based Modeling of Emotion Contagion in Groups. Cogn. Comput. 2014, 7, 111–136. [Google Scholar] [CrossRef] [Green Version]
- Mao, Y.; Du, X.; Li, Y.; He, W. An emotion based simulation framework for complex evacuation scenarios. Graph. Model. 2019, 102, 1–9. [Google Scholar] [CrossRef]
- Xu, T.; Shi, D.; Chen, J.; Li, T.; Lin, P.; Ma, J. Dynamics of emotional contagion in dense pedestrian crowds. Phys. Lett. A 2020, 384, 126080. [Google Scholar] [CrossRef]
- Liu, Z.; Liu, T.; Ma, M.; Hsu, H.-H.; Ni, Z.; Chai, Y.J. A perception-based emotion contagion model in crowd emergent evacuation simulation. Comput. Animat. Virtual Worlds 2018, 29, e1817. [Google Scholar] [CrossRef]
- Liu, T.; Liu, Z.; Chai, Y.J.; Wang, J.; Lin, X.; Huang, P. Simulating evacuation crowd with emotion and personality. Artif. Life Robot. 2018, 24, 59–67. [Google Scholar] [CrossRef]
- Kermack, W.O.; Mckendrick, A.G.A. A Contribution to the Mathematical Theory of Epidemics. Proceedings of The Royal Society A Mathematical Physical and Engineering Sciences 1927, 115, 700–721. [Google Scholar]
- Anderson, R.M.; May, R.M. Infectious Diseases of Human: Dynamics and Control; Oxford University Press (OUP): Oxford, UK, 1992. [Google Scholar]
- Hethcote, H.W.; Driessche, P.V.D. An SIS epidemic model with variable population size and a delay. J. Math. Biol. 1995, 34, 177–194. [Google Scholar] [CrossRef]
- Beretta, E.; Takeuchi, Y. Global stability of an SIR epidemic model with time delays. J. Math. Biol. 1995, 33, 250–260. [Google Scholar] [CrossRef]
- Hill, A.L.; Rand, D.; Nowak, M.A.; Christakis, N.A. Emotions as infectious diseases in a large social network: The SISa model. Proc. R. Soc. B Boil. Sci. 2010, 277, 3827–3835. [Google Scholar] [CrossRef]
- Zhao, H.; Jiang, J.; Xu, R.; Ye, Y. SIRS Model of Passengers’ Panic Propagation under Self-Organization Circumstance in the Subway Emergency. Math. Probl. Eng. 2014, 2014, 1–12. [Google Scholar] [CrossRef] [Green Version]
- Lv, P.; Zhang, Z.; Li, C.; Guo, Y.; Zhou, B.; Xu, M. Crowd Behavior Evolution with Emotional Contagion in Political Rallies. IEEE Trans. Comput. Soc. Syst. 2019, 6, 377–386. [Google Scholar] [CrossRef]
- Fu, L.; Song, W.; Lv, W.; Lo, S. Simulation of emotional contagion using modifed sir model: A cellular automaton approach. Phys. A 2014, 405, 380–391. [Google Scholar]
- Ni, X.; Zhou, H.; Chen, W. Addition of an Emotionally Stable Node in the SOSa-SPSa Model for Group Emotional Contagion of Panic in Public Health Emergency: Implications for Epidemic Emergency Responses. Int. J. Environ. Res. Public Health 2020, 17, 5044. [Google Scholar] [CrossRef]
- Hossain, M.; Pal, N.; Samanta, S. Impact of fear on an eco-epidemiological model. Chaos Solitons Fractals 2020, 134, 109718. [Google Scholar] [CrossRef]
- Fu, L.; Song, W.; Lv, W.; Liu, X.; Lo, S. Multi-grid simulation of counter flow pedestrian dynamics with emotion propagation. Simul. Model. Pr. Theory 2016, 60, 1–14. [Google Scholar] [CrossRef]
- Cornes, F.; Frank, G.; Dorso, C. Fear propagation and the evacuation dynamics. Simul. Model. Pr. Theory 2019, 95, 112–133. [Google Scholar] [CrossRef]
- Jung, C.G. Collected works of C.G. Jung. In Psychology and Alchemy; Princeton University Press: Princeton, NJ, USA, 1980; Volume 12. [Google Scholar]
- Xiao, D.; Ruan, S. Global analysis of an epidemic model with nonmonotone incidence rate. Math. Biosci. 2007, 208, 419–429. [Google Scholar] [CrossRef]
- Driessche, P.V.D.; Watmough, J. Reproduction numbers and sub-threshold endemic equilibria for compartmental models of disease transmission. Math. Biosci. 2002, 180, 29–48. [Google Scholar] [CrossRef]
- Cruz, V.-D.-L. On the global stability of SIS, SIR and SIRS epidemic models with standard incidence. Chaos Solitons Fractals 2011, 44, 1106–1110. [Google Scholar]
- Tang, Q.; Teng, Z.; Abdurahman, X. A New Lyapunov Function for SIRS Epidemic Models. Bull. Malays. Math. Sci. Soc. 2017, 40, 237–258. [Google Scholar] [CrossRef]
- Lasalle, J.P. The Stability of Dynamical Systems; SIAM: Philadelphia, PA, USA, 1976; pp. 1096–1105. [Google Scholar]
- Li, Y.; Liu, H.; Zheng, X.; Han, Y.; Li, L. A Top–Bottom Clustering Algorithm Based on Crowd Trajectories for Small Group Classification. IEEE Access 2019, 7, 29679–29698. [Google Scholar] [CrossRef]
- Huang, K.; Zheng, X.; Yang, Y.; Wang, T. Behavioral evolution in evacuation crowd based on heterogeneous rationality of small groups. Appl. Math. Comput. 2015, 266, 501–506. [Google Scholar] [CrossRef]
- Zhou, R.; Ou, Y.; Tang, W.; Wang, Q.; Yu, B. An Emergency Evacuation Behavior Simulation Method Combines Personality Traits and Emotion Contagion. IEEE Access 2020, 8, 66693–66706. [Google Scholar] [CrossRef]
- Hébert-Dufresne, L.; Scarpino, S.V.; Young, J.-G. Macroscopic patterns of interacting contagions are indistinguishable from social reinforcement. Nat. Phys. 2020, 16, 426–431. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lv, R.; Li, H.; Sun, Q. Panic Spreading Model with Different Emotions under Emergency. Mathematics 2021, 9, 3190. https://doi.org/10.3390/math9243190
Lv R, Li H, Sun Q. Panic Spreading Model with Different Emotions under Emergency. Mathematics. 2021; 9(24):3190. https://doi.org/10.3390/math9243190
Chicago/Turabian StyleLv, Rongjian, Hua Li, and Qiubai Sun. 2021. "Panic Spreading Model with Different Emotions under Emergency" Mathematics 9, no. 24: 3190. https://doi.org/10.3390/math9243190
APA StyleLv, R., Li, H., & Sun, Q. (2021). Panic Spreading Model with Different Emotions under Emergency. Mathematics, 9(24), 3190. https://doi.org/10.3390/math9243190





