Continuous Stability TS Fuzzy Systems Novel Frame Controlled by a Discrete Approach and Based on SOS Methodology
Abstract
:1. Introduction
2. Materials and Methods
2.1. Takagi Sugeno Continuous Systems
2.1.1. Notations
- .
2.1.2. Quadratic Stabilization Conditions
2.2. Continuous Stability Conditions from the Sum of Square Approach
2.2.1. Definition
2.2.2. Stability Conditions
2.3. Discrete Stabilization Conditions from the Euler Method
- First step: Using the Euler method, the discrete model corresponding to the continuous model (1) is obtained. Several models were tried, in this case, by progressively increasing the Euler approximation order .
- Second step: By applying Theorem 3 on the previously-obtained discretized model, the discrete gains and are determined, satisfying the Euler discrete stabilization model.
- Third step: By saving the previously-obtained gains and , a new continuous control law, expressed by (20), will be applied to the continuous TS model (1).
- Fourth step: By applying the discrete controller, the closed loop continuous TS fuzzy model is expressed by Equation (21):
3. Simulation Results and Discussion
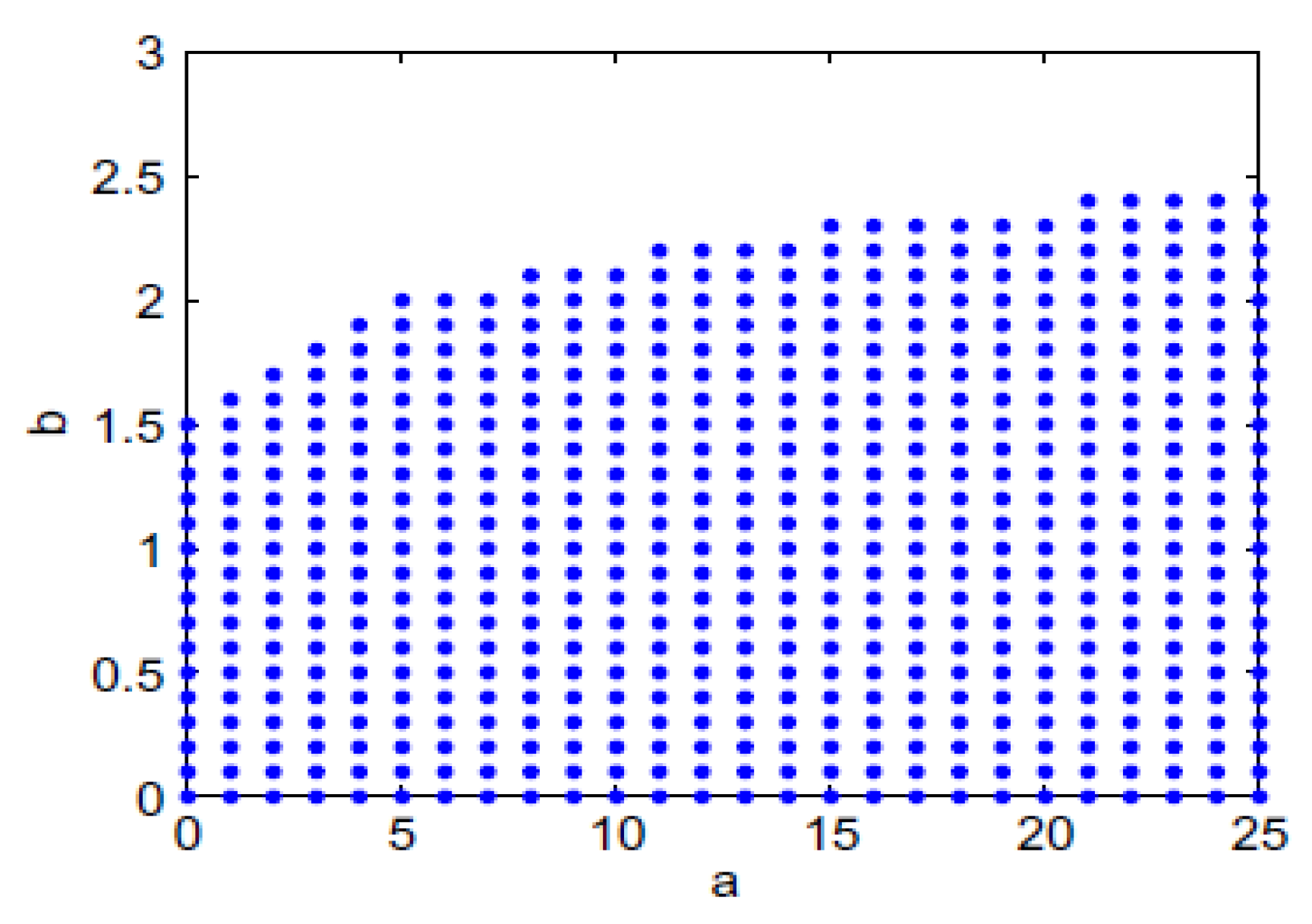
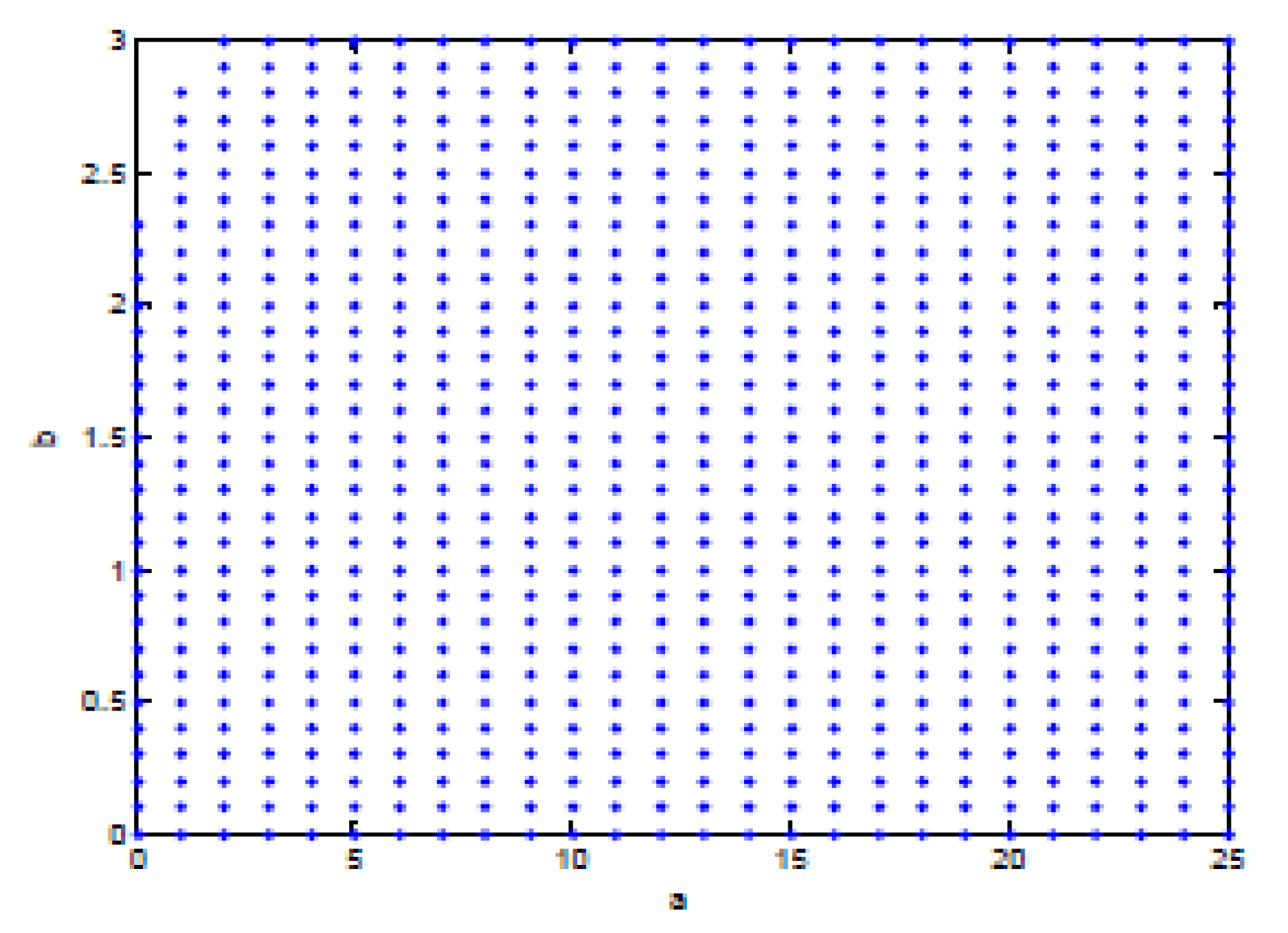
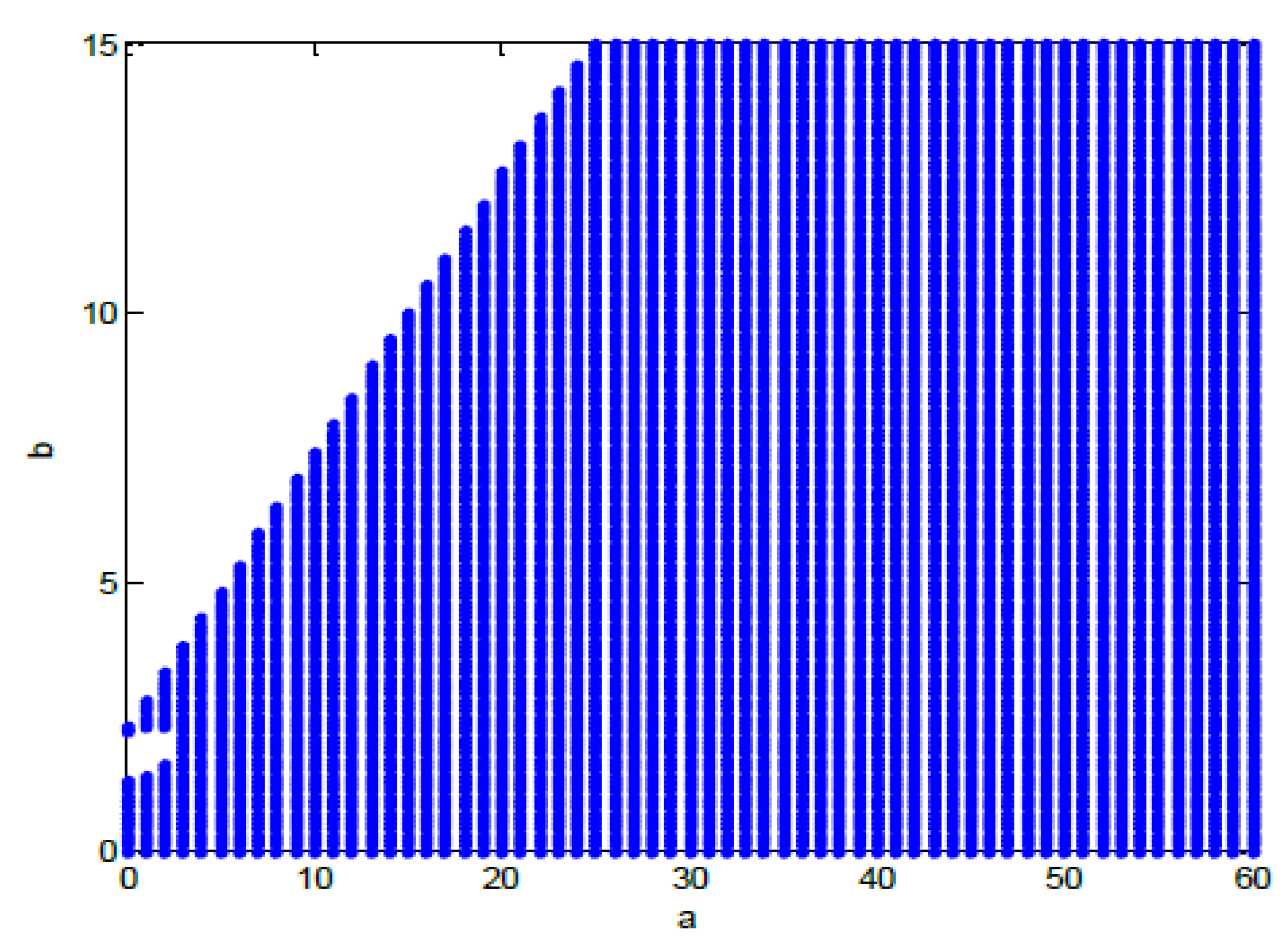
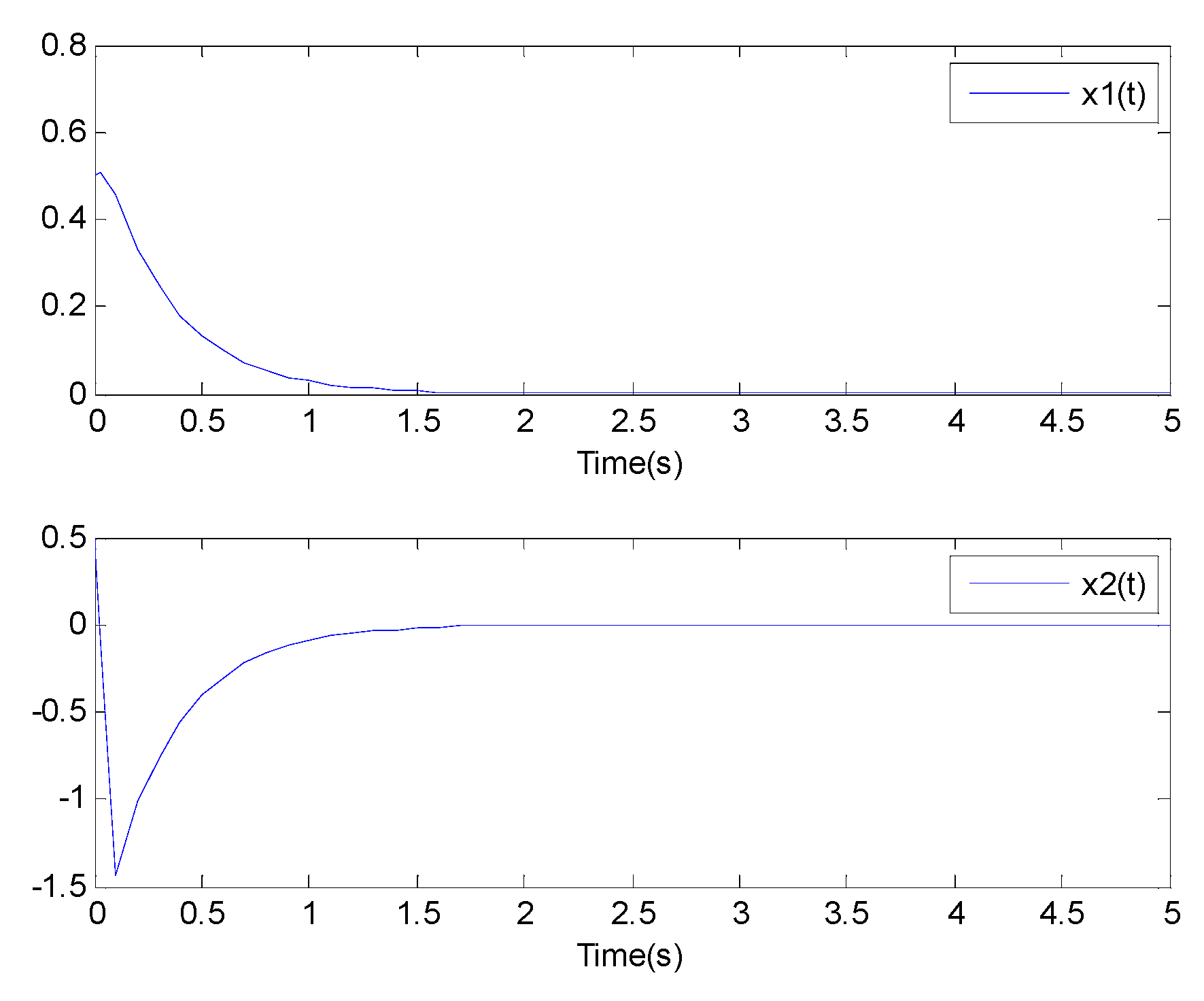
3.1. Example 1
- The Lyapunov Polynomials function of the first-order are:
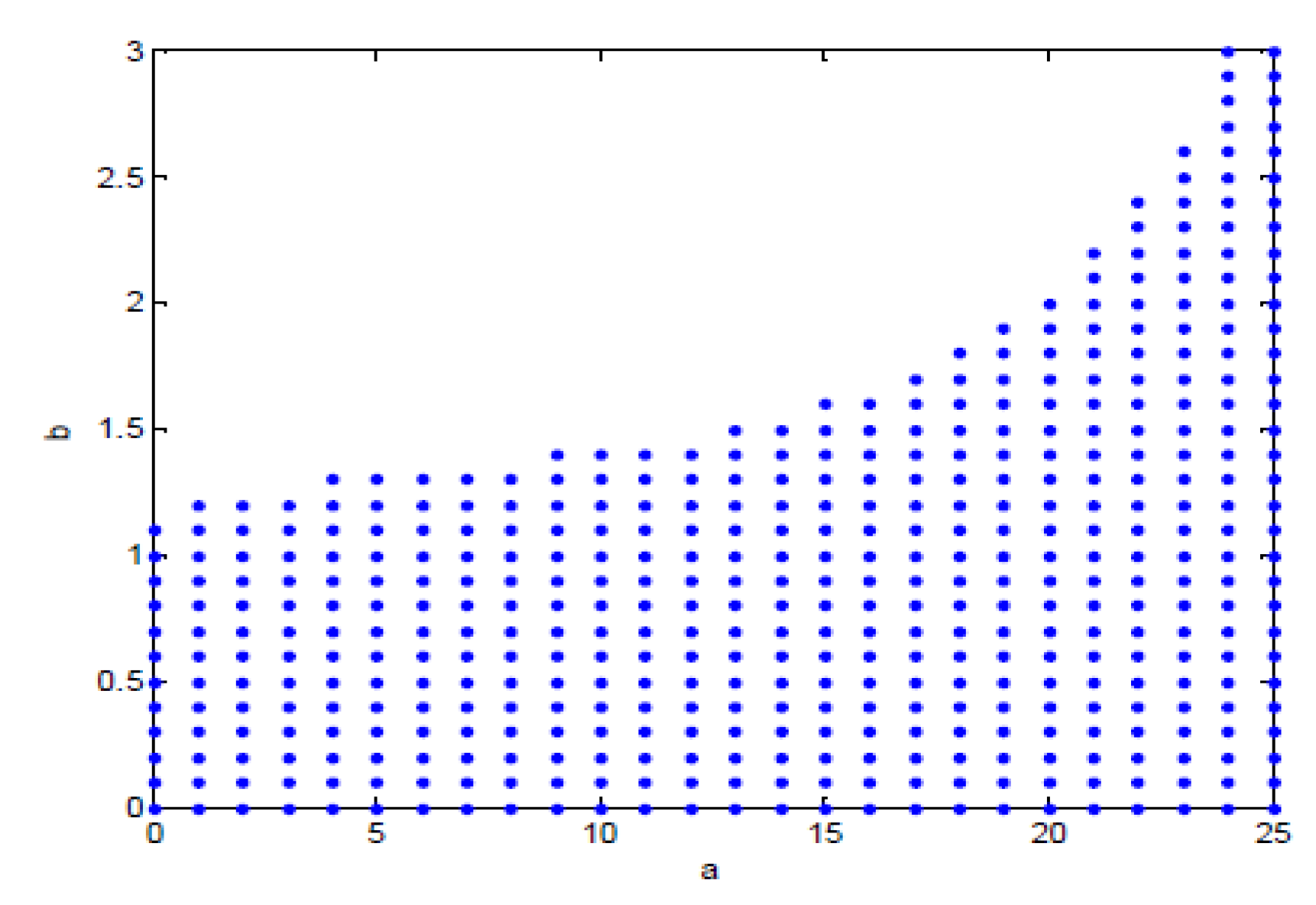
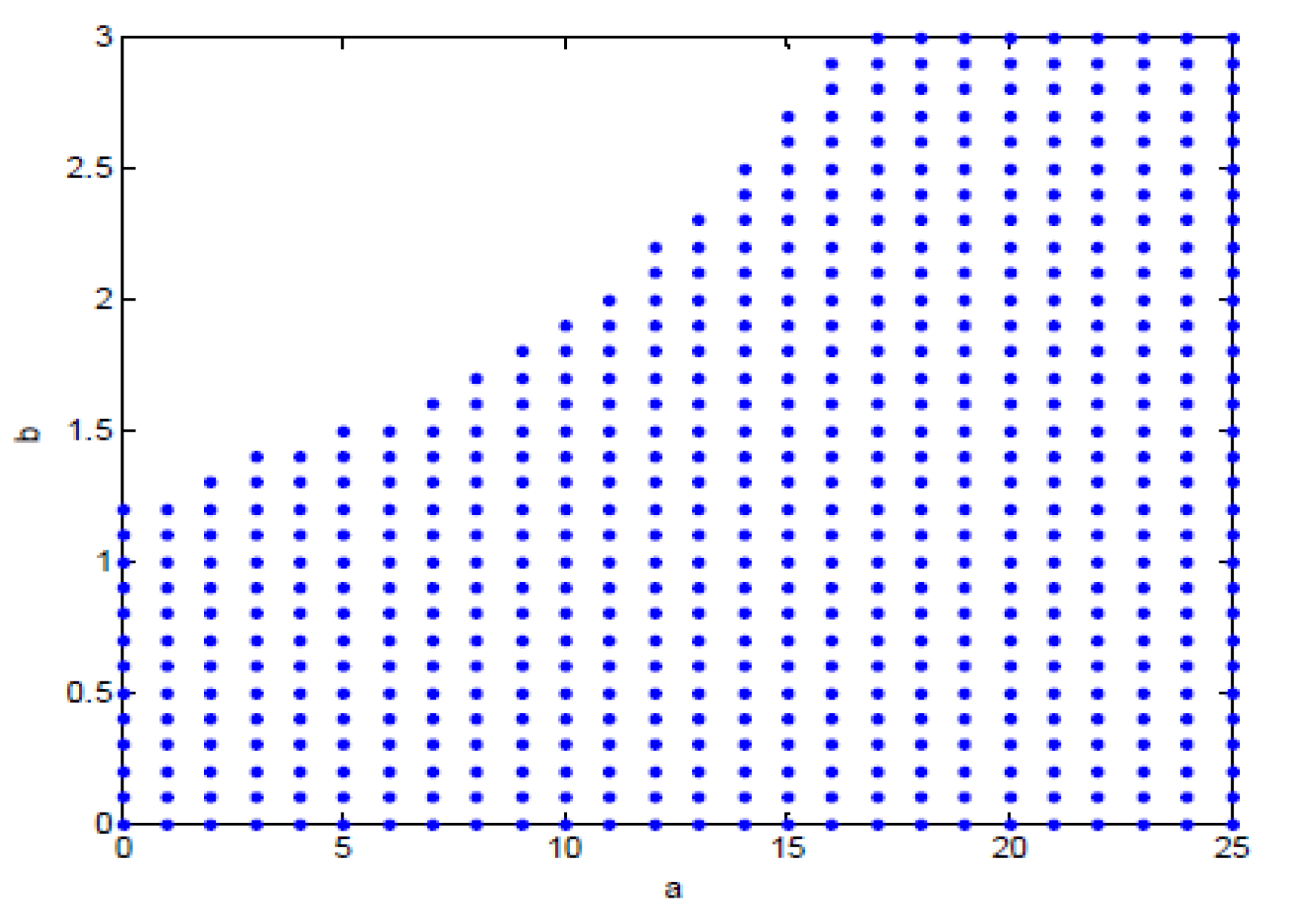
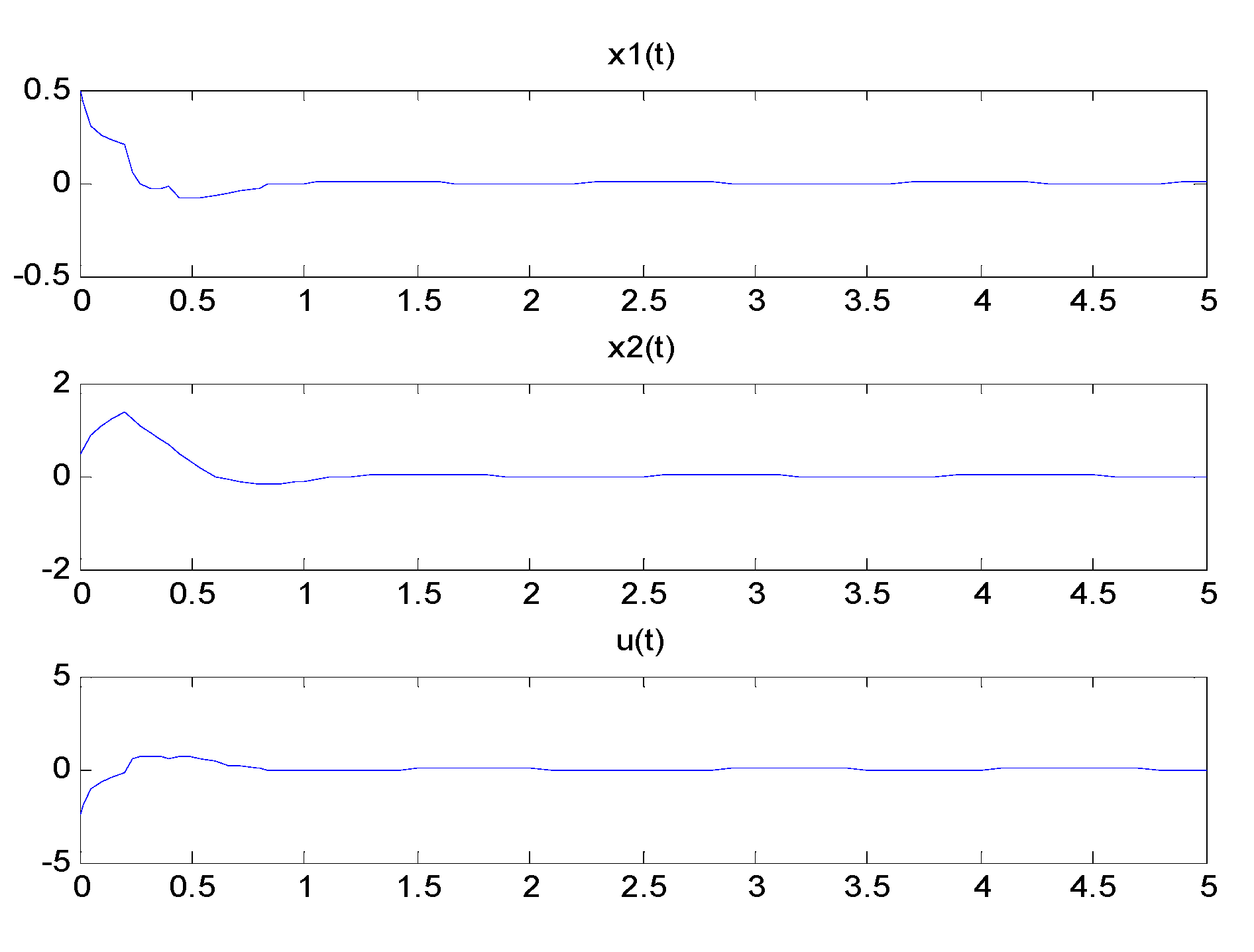
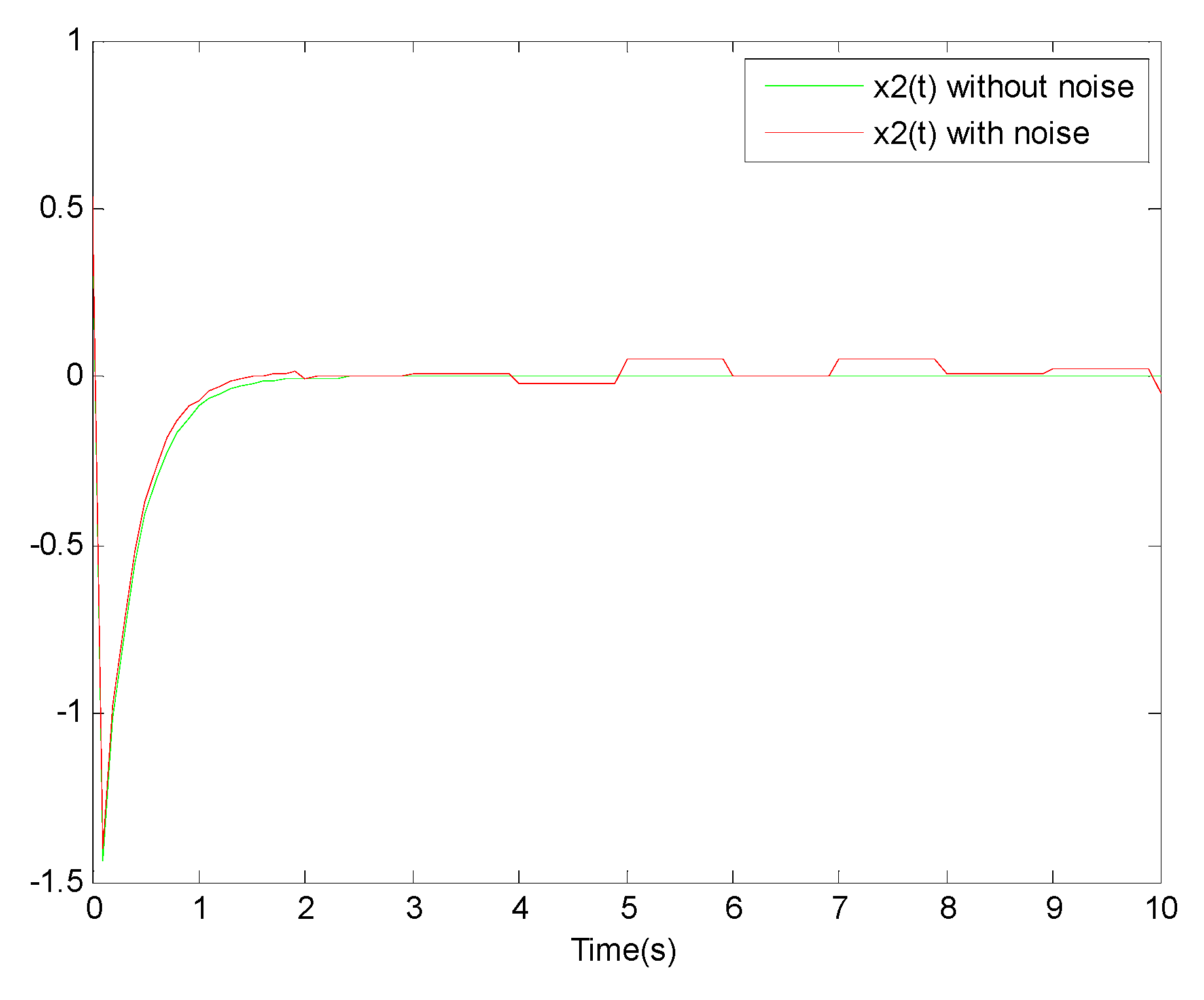
3.2. Example 2
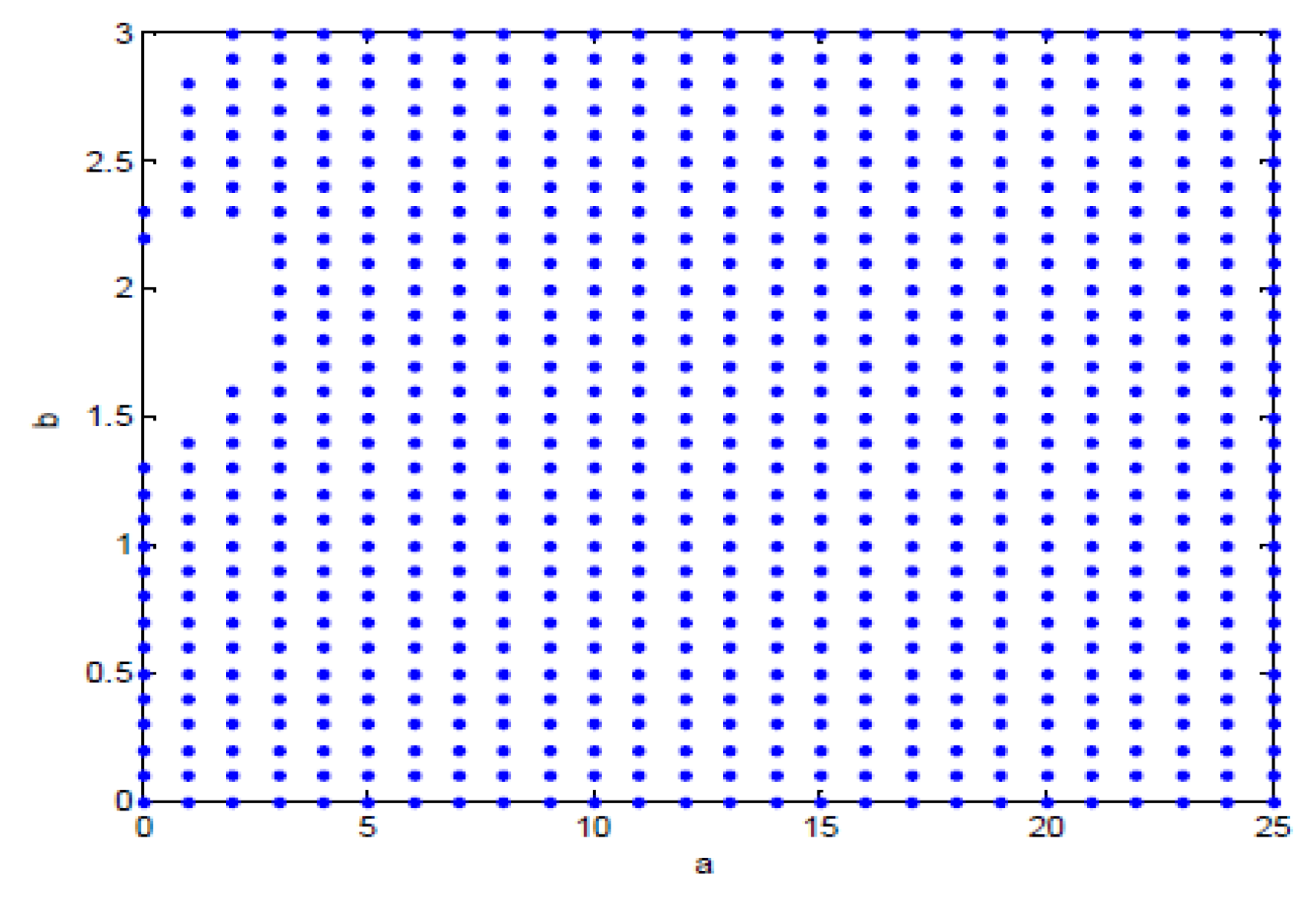
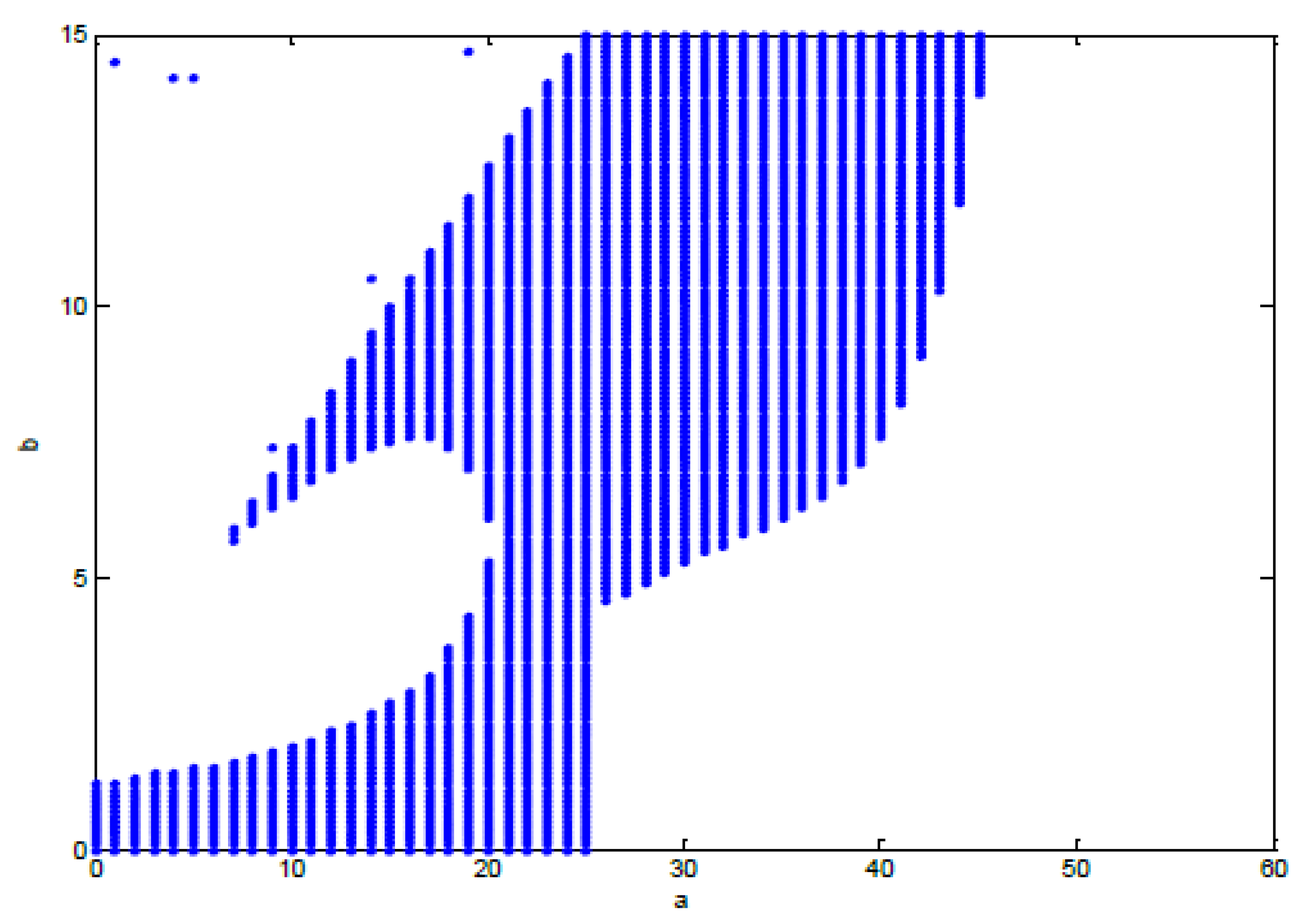
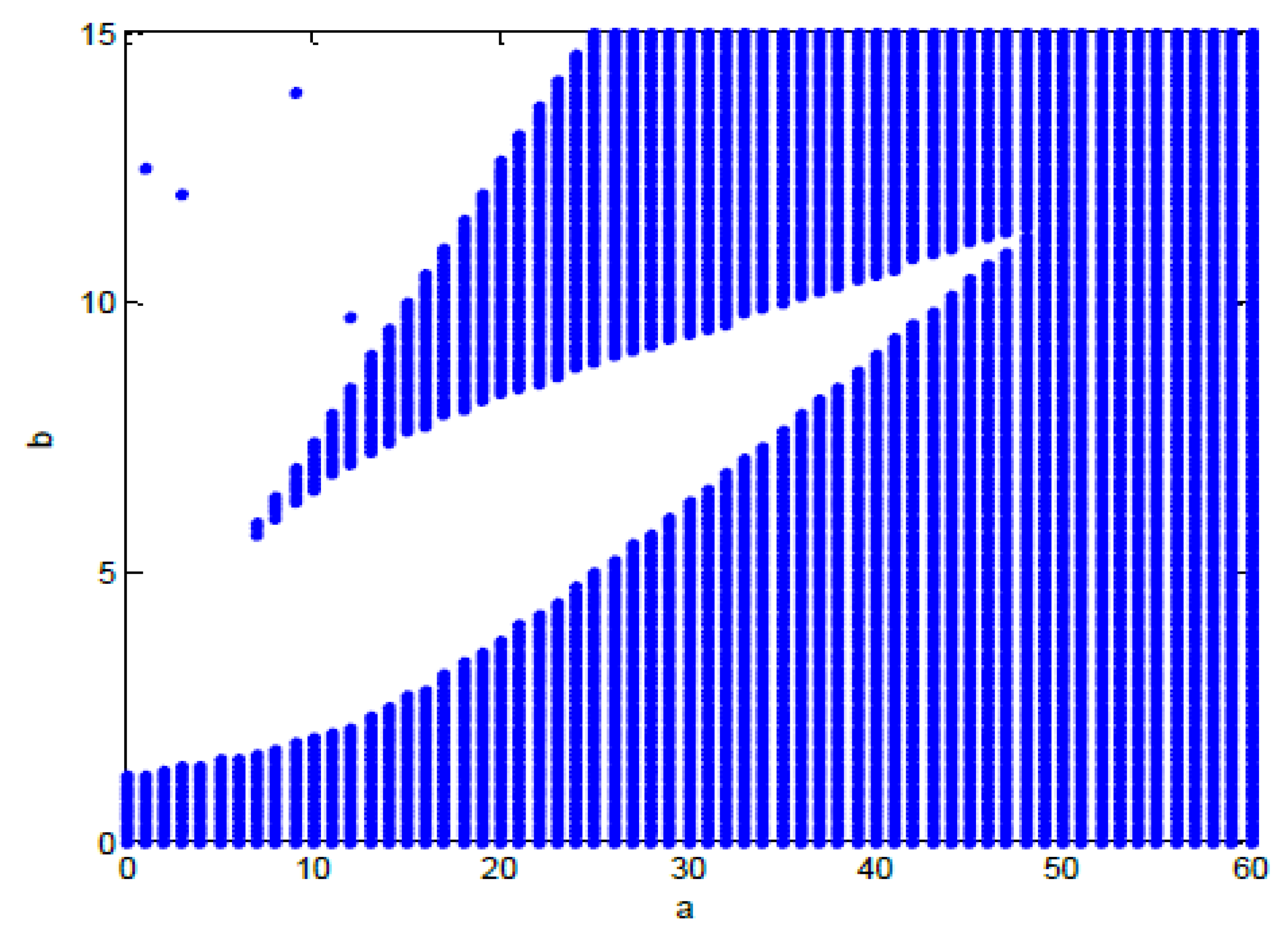
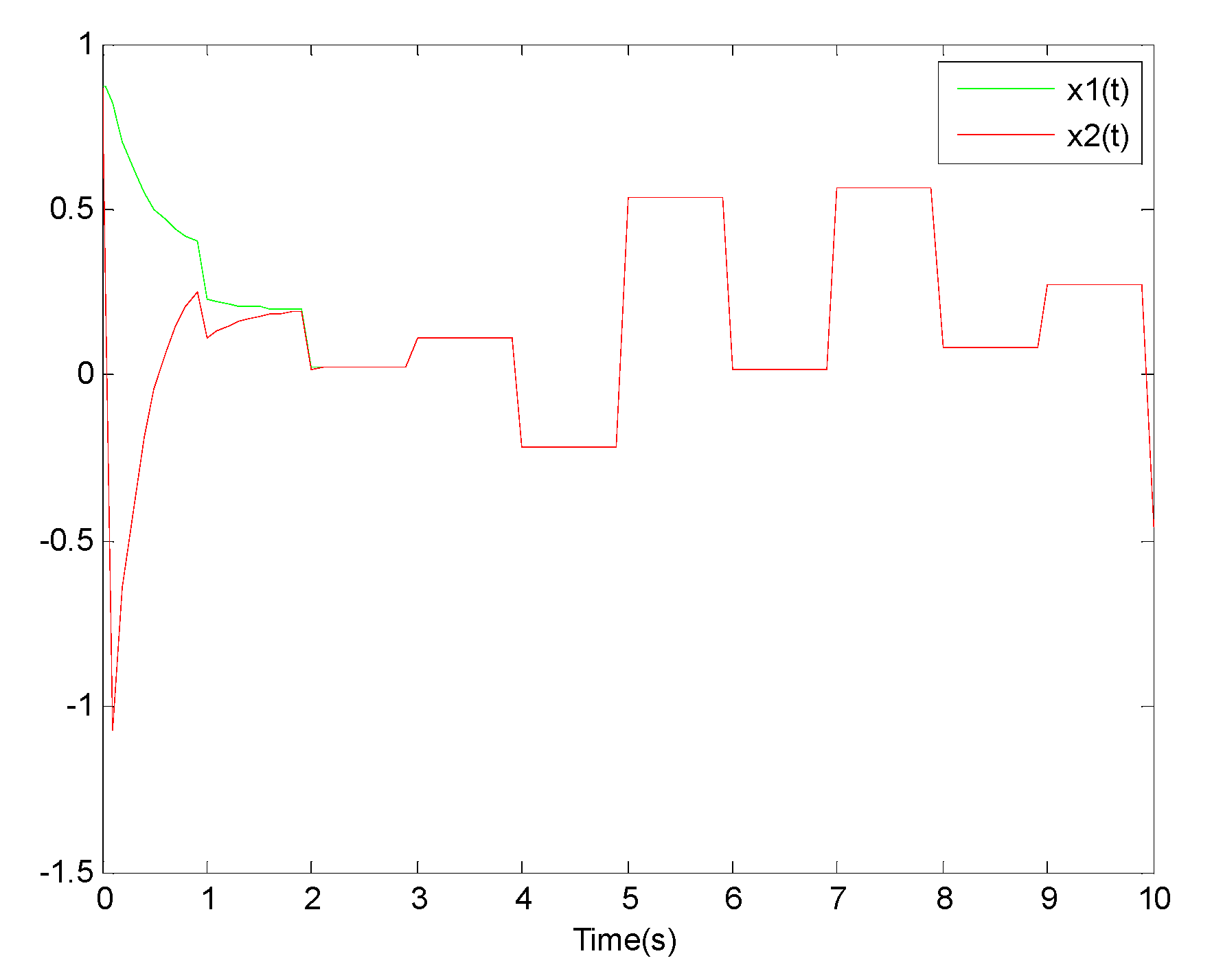
3.3. Example 3
- cart mass ,
- pendulum mass ,
- g gravity constant ,
- length of the pendulum .
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Takagi, G.T.; Sugeno, M. Fuzzy identification of systems and its applications to modeling and control. IEEE Trans. SMC 1985, 15, 116–132. [Google Scholar] [CrossRef]
- Tuan, H.D.; Apkarian, P.; Narikiyo, T.; Yamamoto, Y. Parameterized linear matrix inequality techniques in Fuzzy control system design. IEEE Trans. Fuzzy Syst. 2001, 19, 324–332. [Google Scholar] [CrossRef] [Green Version]
- Tanaka, K.; Hori, T.; Wang, H.O. A multiple Lyapunov function approach to stabilization of fuzzy control systems. IEEE Trans. Fuzzy Syst. 2003, 11, 582–589. [Google Scholar] [CrossRef]
- Guerra, T.M.; Vermeiren, L. LMI-based relaxed nonquadratic stabilization conditions for nonlinear systems in the Takagi-Sugeno’s form. Automatica 2004, 40, 823–829. [Google Scholar] [CrossRef]
- Tanaka, K.; Wang, H.O. Fuzzy Control Systems Design and Analysis. A Linear Matrix Inequality Approach; John Wiley and Sons: Hoboken, NJ, USA, 2001. [Google Scholar]
- Ding, B.C.; Sun, H.X.; Yang, P. Further studies on LMI-based relaxed stabilization conditions for nonlinear systems in Takagi-Sugeno’s form. Automatica 2006, 42, 503–508. [Google Scholar] [CrossRef]
- Salah, R.B.; Kahouli, O.; Hadjabdallah, H.A. Nonlinear Takagi-Sugeno fuzzy logic control for single machine power system. Int. J. Adv. Manuf. Technol. 2017, 90, 575–590. [Google Scholar] [CrossRef]
- Chen, Y.J.; Ohtake, H.; Tanaka, K.; Wang, W.J.; Wang, H.O. Relaxed Stabilization Criterion for T-S Fuzzy Systems by Minimum-type Piecewise Lyapunov Function Based Switching Fuzzy Controller. IEEE Trans. Fuzzy Syst. 2012, 20, 1166–1173. [Google Scholar] [CrossRef]
- Pan, J.T.; Guerral, T.M.; Fei, S.M.; Jaadari, A. Non Quadratic Stabilization of Continuous TS Fuzzy Models: LMI Solution for a Local Approach. IEEE Trans. Fuzzy Syst. 2012, 20, 1063–6706. [Google Scholar] [CrossRef]
- Chang, X.H.; Yang, G.H. Relaxed stabilization conditions for continuous-time Takagi-Sugeno fuzzy control systems. Inf. Sci. 2010, 180, 3273–3287. [Google Scholar] [CrossRef]
- Bernal, M.; Husek, P.; Kucera, V. Nonquadratic stabilization of continuous-time systems in the Takagi-Sugeno form. Kybernetika 2006, 42, 665–672. [Google Scholar]
- Vafamand, N. Global non-quadratic Lyapunov-based stabilization of T-S fuzzy systems: A descriptor approach. J. Vib. Control 2020, 26, 1765–1778. [Google Scholar] [CrossRef]
- Cao, Y.; Frank, P.M.; Hadjili, M.L. Stability analysis and synthesis of nonlinear time-delay systems via linear Takagi-Sugeno fuzzy models. Fuzzy Sets Syst. 2001, 124, 213–229. [Google Scholar] [CrossRef]
- Cao, Y.; Frank, P.M.; Hadjili, M.L. Improved delay-dependent robust stabilization conditions of uncertain T-S fuzzy systems with time-varying delay. Fuzzy Sets Syst. 2008, 159, 2713–2729. [Google Scholar]
- Ellouze, A.; Lauber, J.F.; Delmotte Chtourou, M.; Ksantini, M. Non quadratic Lyapunov function for continuous TS fuzzy models through their discretized forms. Fuzzy Sets Syst. 2014, 253, 64–81. [Google Scholar] [CrossRef]
- Ellouze, A.; Lauber, J.; Delmotte, F.; Chtourou, M.; Ksantini, M. On the stabilization of continuous fuzzy models using their discretized forms. In Proceedings of the 20th Mediterranean Conference on Control & Automation (MED), Barcelona, Spain, 3–6 July 2012; pp. 3–6. [Google Scholar]
- Prajna, S.; Papachristodoulou, A.; Wu, F. Nonlinear control synthesis by sum of squares optimization: A Lyapunov-based approach. In Proceedings of the Asian Control Conference (ASCC), Melbourne, Australia, 20–23 July 2004; pp. 157–165. [Google Scholar]
- Tanaka, K.; Yoshida, H.; Othake, H.; Wang, H.O. A sum-of-squares approach to modeling and control of nonlinear dynamical systems with polynomial fuzzy systems. IEEE Trans. Fuzzy Syst. 2009, 17, 911–922. [Google Scholar] [CrossRef]
- Guelton, K.; Manamanni, N.; Koumba-Emianiwe, D.L.; Chinh, C.D. SOS stability conditions for nonlinear systems based on a polynomial fuzzy Lyapunov function. In Proceedings of the 18th IFAC World Congress, Milano, Italy, 29 August–3 September 2011; Volume 18, pp. 12777–12782. [Google Scholar]
- Lam, H.K.; Li, H. Output-feedback tracking control for polynomial fuzzy-model-based control systems. IEEE Trans. Ind. Electron. 2013, 60, 5830–5840. [Google Scholar] [CrossRef]
- Lam, H.K.; Wu, L.; Lam, J. Two-step stability analysis for general polynomial-fuzzy-model-based control systems. IEEE Trans. Fuzzy Syst. 2015, 23, 511–524. [Google Scholar] [CrossRef] [Green Version]
- Rakhshan, M.; Vafamand, N.; Mardani, M.M. Polynomial control design for polynomial systems: A non-iterative sum of squares approach. Trans. Inst. Meas. Control 2018, 41, 1993–2004. [Google Scholar] [CrossRef]
- Zhao, D.Y.; He, Y.; Du, X. Relaxed Sum-of-Squares Based Stabilization Conditions for Polynomial Fuzzy-Model-Based Control Systems. IEEE Trans. Fuzzy Syst. 2019, 27, 1767–1778. [Google Scholar] [CrossRef]
- Chaibi, R.; Hmamed, A.; Tissir, E.H.; Tadeo, F. Control of Discrete 2-D Takagi-Sugeno Systems Via a Sum-Of-Squares Approach. J. Control Autom. Electr. Syst. 2019, 30, 137–147. [Google Scholar] [CrossRef]
- Wang, H.O.; Tanaka, K.; Griffin, M. An approach to fuzzy control of non linear systems: Stability and design issues. IEEE Trans. Fuzzy Syst. 1996, 4, 14–23. [Google Scholar] [CrossRef]
- Prajna, S.; Papachristodoulou, A.; Parrilo, P.A. Introducing SOSTOOLS: A general purpose sum of squares programming solver. In Proceedings of the IEEE Conference on Decision and Control, Las Vegas, NV, USA, 12–14 December 2002. [Google Scholar]
- Nesic, D.; Teel, A.R.; Kokotovic, P.V. Sufficient conditions for stabilization of sampled-data nonlinear systems via discrete-time approximations. Syst. Contr. Lett. 1999, 38, 259–270. [Google Scholar] [CrossRef] [Green Version]
- Mozelli, L.A.; Palhares, R.M.; Avellar, G.S.C. A systematic approach to improve multiple Lyapunov function stability and stabilization conditions for fuzzy systems. Inf. Sci. 2009, 179, 1149–1162. [Google Scholar] [CrossRef]
- Bernal, M.; Guerra, T.M.; Jaadari, A. Non quadratic stabilization of Takagi Sugeno models: A local point of view. In Proceedings of the International Conference on Fuzzy Systems, Luxembourg, 11–14 July 2010. [Google Scholar]
- Lee, D.H.; Park, J.B.; Joo, Y.H. Fuzzy Lyapunov function approach to estimating the domain of attraction for continuous-time Takagi-Sugeno fuzzy systems. Inf. Sci. 2012, 185, 230–248. [Google Scholar] [CrossRef]
- Huijun, G.; Tongwen, C. Stabilization of Nonlinear Systems Under Variable Sampling: A Fuzzy Control Approach. IEEE Trans. Fuzzy Syst. 2007, 185, 972–983. [Google Scholar]







Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ellouze, A.; Kahouli, O.; Ksantini, M.; Rebhi, A.; Hnaien, N.; Delmotte, F. Continuous Stability TS Fuzzy Systems Novel Frame Controlled by a Discrete Approach and Based on SOS Methodology. Mathematics 2021, 9, 3129. https://doi.org/10.3390/math9233129
Ellouze A, Kahouli O, Ksantini M, Rebhi A, Hnaien N, Delmotte F. Continuous Stability TS Fuzzy Systems Novel Frame Controlled by a Discrete Approach and Based on SOS Methodology. Mathematics. 2021; 9(23):3129. https://doi.org/10.3390/math9233129
Chicago/Turabian StyleEllouze, Ameni, Omar Kahouli, Mohamed Ksantini, Ali Rebhi, Nidhal Hnaien, and François Delmotte. 2021. "Continuous Stability TS Fuzzy Systems Novel Frame Controlled by a Discrete Approach and Based on SOS Methodology" Mathematics 9, no. 23: 3129. https://doi.org/10.3390/math9233129
APA StyleEllouze, A., Kahouli, O., Ksantini, M., Rebhi, A., Hnaien, N., & Delmotte, F. (2021). Continuous Stability TS Fuzzy Systems Novel Frame Controlled by a Discrete Approach and Based on SOS Methodology. Mathematics, 9(23), 3129. https://doi.org/10.3390/math9233129






