A New Attractive Method in Solving Families of Fractional Differential Equations by a New Transform

Abstract

In this paper, we use the ARA transform to solve families of fractional differential equations. New formulas about the ARA transform are presented and implemented in solving some applications. New results related to the ARA integral transform of the Riemann-Liouville fractional integral and the Caputo fractional derivative are obtained and the last one is implemented to create series solutions for the target equations. The procedure proposed in this article is mainly based on some theorems of particular solutions and the expansion coefficients of binomial series. In order to achieve the accuracy and simplicity of the new method, some numerical examples are considered and solved. We obtain the solutions of some families of fractional differential equations in a series form and we show how these solutions lead to some important results that include generalizations of some classical methods.

1. Introduction

Fractional calculus is a field of mathematics that studies the theory and applications of integral and derivatives of a non-integer order. It becomes a vibrant area for mathematicians and scientists in their research because of its popularity and importance in modeling and describing many phenomena in many areas including quantum mechanics, plasma physics, electromagnetic theory and other different areas of science; see the books of Podlubny [1], Miller and Ross [2] and Oldham [3] and the papers by Friedrich [4], Chen and Moore [5] and Ahmad and Sivasundaram [6].

During the last decade, researchers have been interested in establishing and refining new methods to solve fractional differential equations such as the Adomian decomposition method [7], the iteration method [8,9], residual power series [10], the finite-difference method [11,12], the Laplace transform method [12,13,14,15] and the Homotopy analysis method [16]. Some of these methods give the solution in a series form which converges to the exact solution and other methods reduce the given equation into a simple one or system of equations [17,18,19,20].

In order to produce this paper, we deal with the ARA transform [21], which is a general form of the Laplace transform. In addition, the ARA transform is applicable for some functions which can’t be defined in the case of Laplace transform.

Some important theorems related to the Laplace transform have been generalized and constructed to the ARA transform.

New general formulas are established throughout applying the ARA transform on both Riemann-Liouville fractional integral and Caputo fractional derivative. The last one is implemented to solve and construct series solutions of some families of fractional differential equations.

The article is organized as follows. In Section 2, we introduce some basic definitions of fractional calculus and the ARA transform. Some properties of the ARA transform that are needed in our work also are illustrated in Section 2. In Section 3, the ARA transform is implemented to obtain new formulas by applying the ARA transform on the Riemann-Liouville fractional integral and the Caputo fractional derivative.

In Section 4, solutions of some families of fractional differential equations are introduced and discussed with some applications.

2. Definitions and Properties

In this section, we present some basic definitions and properties of fractional calculus theory and the ARA transform that are needed to construct the new formulas about the ARA solution of the fractional differential equations.

Definition 1

[1]. The Riemann-Liouville fractional integral of order $\alpha >0$ of the function $g\left(t\right)$ is defined by:

$${\displaystyle I_t^{\alpha }}g\left(t\right)=\frac{1}{\Gamma \left(\alpha \right)}{\displaystyle \underset{a}{\overset{t}{\int }}}{\left(t-\tau \right)}^{\alpha -1}g\left(\tau \right)d\tau .$$

Definition 2

[1]. The Caputo fractional derivative of order $\alpha >0$ of the function $g\left(t\right)$ is defined by:

$${\displaystyle D_t^{\alpha }} g\left(t\right)=\frac{1}{\Gamma \left(m-\alpha \right)}{\displaystyle \underset{0}{\overset{t}{\int }}}\frac{g^{\left(m\right)}\left(\tau \right)}{{\left(t-\tau \right)}^{\alpha +1-m}}d\tau , m-1<\alpha <m.$$

Recently, Saadeh et al. [21] introduced a new integral transform, called the ARA transform, which is applied to solve ordinary and partial differential equations.

Definition 3

[21]. The ARA transform of order n of the continuous function $g:\left(0,\infty \right)\to ℝ$, is defined by:

$${\mathcal{G}}_n\left[g\left(t\right)\right]\left(s\right)=G\left(n,s\right)=s{\displaystyle \underset{0}{\overset{\infty }{\int }}}{t}^{n-1}{e}^{-st}g\left(t\right)dt, s>0.$$

Definition 4.

The binomial formula is written as:

$${\left(x+y\right)}^n={\displaystyle \sum _{k=0}^n}{\displaystyle C_k^n} x^{n-k}{y}^k,$$

where

$${\displaystyle C_k^n}=\left(\begin{array}{c}n\\ k\end{array}\right)=\frac{n!}{\left(n-k\right)!k!}=\frac{n\left(n-1\right)\dots \left(n-k-1\right)}{k!}.$$

Definition 5.

The Mittag-Leffler function (cf. [22,23]) is defined by:

$$E_{\gamma ,\lambda }\left(z\right)={\displaystyle \sum _{j=0}^{\infty }}\frac{z^j}{\Gamma \left(\gamma j+\lambda \right)}, \left(z,\gamma ,\lambda \in ℂ, ℝ\left(\gamma \right)>0\right).$$

In the following we introduce some basic properties of the ARA transform [21] that are essential in our research.

Property 1.

(Existence of the ARA Transform)

If$g\left(t\right)$is a piecewise continuous function on every finite interval$0\le t\le \beta$and satisfies:

$$\left|t^{n-1}g\left(t\right)\right|\le K{e}^{\beta t},$$

then the ARA transform exists for all$s>\beta$.

Property 2.

(Linearity Property)

Let$u\left(t\right)$ and $v\left(t\right)$ be two functions in which the ARA transform exists. Then

$${\mathcal{G}}_n\left[\alpha u\left(t\right)+\beta v\left(t\right)\right]\left(s\right)=\alpha {\mathcal{G}}_n\left[u\left(t\right)\right]\left(s\right)+\beta {\mathcal{G}}_n\left[v\left(t\right)\right]\left(s\right),$$

where α and β are nonzero constants.

Property 3.

(Shifting in n—Domain)

$${\mathcal{G}}_n\left[t^{m}g\left(t\right)\right]\left(s\right)={\mathcal{G}}_{n+m}\left[g\left(t\right)\right]\left(s\right).$$

Property 4.

(The ARA Transform for Derivatives)

$${\mathcal{G}}_n\left[g^{\left(m\right)}\left(t\right)\right]\left(s\right)={\left(-1\right)}^{n-1}s\frac{d^{n-1}}{d{s}^{n-1}}\left( \frac{{\mathcal{G}}_1\left[g^{\left(m\right)}\left(t\right)\right]\left(s\right)}{s}\right).$$

Property 5.

(Convolution)

$${\mathcal{G}}_n\left[f\left(t\right) \ast h\left(t\right)\right]\left(s\right)={\left(-1\right)}^{n-1}s{\displaystyle \sum _{j=0}^{n-1}}{\displaystyle C_j^{n-1}}{\left(\frac{{\mathcal{G}}_1\left[f\left(t\right)\right]\left(s\right)}{s}\right)}^{\left(j\right)}·{\left(\frac{{\mathcal{G}}_1\left[h\left(t\right)\right]\left(s\right)}{s}\right)}^{\left(n-1-j\right)}$$

Property 6.

The ARA transform of$t^{\alpha }$is given by:

$${\mathcal{G}}_n\left[t^{\alpha }\right]\left(s\right)=\frac{\Gamma \left(\alpha +n\right)}{s^{\alpha +n-1}}.$$

In the following arguments, we introduce the dualities between the ARA transform and some well-known transforms.

• Duality to Laplace transform [24]

  $$\mathcal{L}\left[g\left(t\right)\right]\left(s\right)=G\left(s\right)=\frac{{\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)}{s}$$

  and

  $$\mathcal{L}\left[t^{n-1} g\left(t\right)\right]\left(s\right)=\frac{{\mathcal{G}}_n\left[g\left(t\right)\right]\left(s\right)}{s}.$$
• Duality to Sumudu transform [25]

  $$S\left[g\left(t\right)\right]\left(u\right)=G\left(u\right)=\frac{1}{u}{\displaystyle \underset{0}{\overset{\infty }{\int }}}\exp \left(-\frac{t}{u}\right)g\left(t\right)dt.$$
  Putting $=\frac{1}{s}$, we get

  $$S\left[g\left(t\right)\right]\left(\frac{1}{s}\right)=G\left(\frac{1}{s}\right)={\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right).$$
• Duality to Shehu transform [26]

  $$\mathbb{S}\left[g\left(t\right)\right]\left(s\right)=V\left(s,u\right)={\displaystyle \underset{0}{\overset{\infty }{\int }}}\exp \left(-\frac{st}{u}\right)g\left(t\right)dt.$$
  Putting = 1, we get

  $$sV\left(s,1\right)={\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)$$

  and

  $$s \mathbb{S}\left[t^{n-1} g\left(t\right)\right]\left(s,1\right)={\mathcal{G}}_n\left[g\left(t\right)\right]\left(s\right).$$

3. The ARA Transform of Fractional Operators

In this section we present two basic theorems about the application of ARA transform on the Riemann-Liouville fractional integral and the Caputo fractional derivative and construct new formulas to solve the target equations.

Theorem 1.

The ARA transform of the Riemann-Liouville fractional integral of order α > 0 of the function g(t) is given by:

$${\mathcal{G}}_n\left[{\displaystyle I_t^{\alpha }}g\left(t\right)\right]\left(s\right)={\left(-1\right)}^{n-1}s{\displaystyle \sum _{j=0}^{n-1}}{\displaystyle C_j^{n-1}}{\left(\frac{{\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)}{s}\right)}^{\left(j\right)}{\left(s^{-\alpha } \right)}^{\left(n-1-j\right)},$$

where${\displaystyle C_j^{n-1}}$is the binomial coefficient.

Proof of Theorem 1.

The Riemann-Liouville fractional integral of the function g(t) can be written as

$${\displaystyle I_t^{\alpha }}g\left(t\right)=\frac{1}{\Gamma \left(\alpha \right)}\left(g\left(t\right) \ast t^{\alpha -1}\right).$$

(1)

Applying the ARA transform on both sides of Equation (1), to get

$${\mathcal{G}}_n\left[{\displaystyle I_t^{\alpha }}g\left(t\right)\right]\left(s\right)=\frac{1}{\Gamma \left(\alpha \right)}{\mathcal{G}}_n\left[g\left(t\right) \ast t^{\alpha -1}\right]\left(s\right).$$

Using Property 5, we have

$$\begin{array}{ll}\hfill {\mathcal{G}}_n\left[{\displaystyle I_t^{\alpha }}g\left(t\right)\right]\left(s\right)& =\frac{1}{\Gamma \left(\alpha \right)}\left({\left(-1\right)}^{n-1}s{\displaystyle {\displaystyle \sum }_{j=0}^{n-1}}{\displaystyle C_j^{n-1}}{\left(\frac{{\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)}{s}\right)}^{\left(j\right)} {\left(\frac{\Gamma \left(\alpha \right)}{s^{\alpha }}\right)}^{\left(n-1-j\right)}\right)\\ & ={\left(-1\right)}^{n-1}s{\displaystyle \sum _{j=0}^{n-1}}{\displaystyle C_j^{n-1}}{\left(\frac{{\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)}{s}\right)}^{\left(j\right)} {\left(s^{-\alpha }\right)}^{\left(n-1-j\right)}.\end{array}$$

□

Theorem 2.

The ARA transform of the Caputo fractional derivative of order α > 0 of the function g(t) is given by:

$${\mathcal{G}}_n\left[{\displaystyle D_t^{\alpha }}g\left(t\right)\right]\left(s\right)=\frac{1}{\Gamma \left(m-\alpha \right)}{\displaystyle \sum _{j=1}^n}\left(\begin{array}{c}n-1\\ j-1\end{array}\right)\frac{\Gamma \left(n+m-j-\alpha \right)}{s^{n+m-j-\alpha }}{\mathcal{G}}_j\left[g^{\left(m\right)}\left(t\right)\right]\left(s\right),$$

for m − 1 < α ≤ m.

Proof of Theorem 2.

Applying the ARA transform on the Caputo fractional derivative of the function g(t), we have:

$$\begin{array}{ll}\hfill {\mathcal{G}}_n\left[{\displaystyle D_t^{\alpha }}g\left(t\right)\right]\left(s\right)& =s{\displaystyle \underset{0}{\overset{\infty }{\int }}}{e}^{-st}{t}^{n-1}\left({\displaystyle D_t^{\alpha }}g\left(t\right)\right)dt\\ & =s{\displaystyle \underset{0}{\overset{\infty }{\int }}}{e}^{-st}{t}^{n-1}\left(\frac{1}{\Gamma \left(m-\alpha \right)}{\displaystyle \underset{0}{\overset{t}{{\displaystyle \int }}}}\frac{g^{\left(m\right)}\left(\zeta \right)}{{\left(t-\zeta \right)}^{\alpha -m+1}}d\zeta \right)dt.\end{array}$$

Changing the order of integration, we get

$$\begin{array}{ll}\hfill {\mathcal{G}}_n\left[{\displaystyle D_t^{\alpha }}g\left(t\right)\right]\left(s\right)& =\frac{s}{\Gamma \left(m-\alpha \right)}{\displaystyle \underset{0}{\overset{\infty }{\int }}}{\displaystyle \underset{\zeta }{\overset{\infty }{\int }}}\frac{e^{-st}{t}^{n-1}{g}^{\left(m\right)}\left(\zeta \right)}{{\left(t-\zeta \right)}^{\alpha -m+1}}dt d\zeta \\ & =\frac{s}{\Gamma \left(m-\alpha \right)}{\displaystyle \underset{0}{\overset{\infty }{\int }}}{g}^{\left(m\right)}\left(\zeta \right){\displaystyle \underset{\zeta }{\overset{\infty }{\int }}}\frac{e^{-st}{t}^{n-1}}{{\left(t-\zeta \right)}^{\alpha -m+1}}dt d\zeta .\end{array}$$

(2)

Letting $u=t-\zeta$ in Equation (2) leads to

$$\begin{array}{ll}\hfill {\mathcal{G}}_n\left[{\displaystyle D_t^{\alpha }}g\left(t\right)\right]\left(s\right)& =\frac{s}{\Gamma \left(m-\alpha \right)}{\displaystyle \underset{0}{\overset{\infty }{\int }}}{g}^{\left(m\right)}\left(\zeta \right){\displaystyle \underset{0}{\overset{\infty }{\int }}}\frac{e^{-s\left(u+\zeta \right)}{\left(u+\zeta \right)}^{n-1}}{u^{\alpha -m+1}}du d\zeta \\ & =\frac{s}{\Gamma \left(m-\alpha \right)}{\displaystyle \underset{0}{\overset{\infty }{\int }}}{g}^{\left(m\right)}\left(\zeta \right)e^{-s\zeta }{\displaystyle \underset{0}{\overset{\infty }{\int }}}{e}^{-su} {\left(u+\zeta \right)}^{n-1} u^{m-\alpha -1}dud\zeta \end{array}$$

(3)

Using the binomial formula, Equation (3) can be written as

$${\mathcal{G}}_n\left[{\displaystyle D_t^{\alpha }}g\left(t\right)\right]\left(s\right)=\frac{s}{\Gamma \left(m-\alpha \right)}{\displaystyle \underset{0}{\overset{\infty }{\int }}}{g}^{\left(m\right)}\left(\zeta \right)e^{-s\zeta }{\displaystyle \underset{0}{\overset{\infty }{\int }}}{e}^{-su}{\displaystyle \sum _{j=0}^{n-1}}\left(\begin{array}{c}n-1\\ j\end{array}\right)u^{n+m-\alpha -j-2} {\zeta }^{j}du d\zeta$$

Thus,

$${\mathcal{G}}_n\left[{\displaystyle D_t^{\alpha }}g\left(t\right)\right]\left(s\right)=\frac{1}{\Gamma \left(m-\alpha \right)}{\displaystyle \underset{0}{\overset{\infty }{\int }}}{g}^{\left(m\right)}\left(\zeta \right)e^{-s\zeta }s{\displaystyle \underset{0}{\overset{\infty }{\int }}}{e}^{-su}{\displaystyle \sum _{j=1}^n}\left(\begin{array}{c}n-1\\ j-1\end{array}\right)u^{n+m-\alpha -j-1} {\zeta }^{j-1}du d\zeta$$

From the definition of the ARA transform of order one ${\mathcal{G}}_1\left[ \right]$, we obtain

$${\mathcal{G}}_n\left[{\displaystyle D_t^{\alpha }}g\left(t\right)\right]\left(s\right)=\frac{1}{\Gamma \left(m-\alpha \right)}{\displaystyle \underset{0}{\overset{\infty }{\int }}}{g}^{\left(m\right)}\left(\zeta \right)e^{-s\zeta }{\mathcal{G}}_1\left[{\displaystyle {\displaystyle \sum }_{j=1}^n}\left(\begin{array}{c}n-1\\ j-1\end{array}\right)u^{n+m-\alpha -j-1} {\zeta }^{j-1}\right]d\zeta .$$

Property 6 and the definition of the ARA transform yield

$$\begin{array}{cc}\hfill {\mathcal{G}}_n\left[{\displaystyle D_t^{\alpha }}g\left(t\right)\right]\left(s\right)& =\frac{1}{\Gamma \left(m-\alpha \right)}{\displaystyle \underset{0}{\overset{\infty }{\int }}}{e}^{-s\zeta }{g}^{\left(m\right)}\left(\zeta \right) {\displaystyle {\displaystyle \sum _{j=1}^n}}\left(\begin{array}{c}n-1\\ j-1\end{array}\right)\frac{\Gamma \left(n+m-j-\alpha \right)}{s^{n+m-j-\alpha -1}} {\zeta }^{j-1}d\zeta \hfill \\ & =\frac{1}{\Gamma \left(m-\alpha \right)}{\displaystyle {\displaystyle \sum _{j=1}^n}}\left(\begin{array}{c}n-1\\ j-1\end{array}\right)\frac{\Gamma \left(n+m-j-\alpha \right)}{s^{n+m-j-\alpha -1}} {\displaystyle \underset{0}{\overset{\infty }{\int }}}{e}^{-s\zeta }{\zeta }^{j-1} g^{\left(m\right)}\left(\zeta \right) d\zeta \hfill \\ & =\frac{1}{\Gamma \left(m-\alpha \right)}{\displaystyle {\displaystyle \sum _{j=1}^n}}\left(\begin{array}{c}n-1\\ j-1\end{array}\right)\frac{\Gamma \left(n+m-j-\alpha \right)}{s^{n+m-j-\alpha }}{\mathcal{G}}_j\left[g^{\left(m\right)}\left(t\right)\right]\left(s\right).\hfill \end{array}$$

This completes the proof. □

Remark 1.

(Special Cases of Theorem 2)

$${\mathcal{G}}_1\left[{\displaystyle D_t^{\alpha }}g\left(t\right)\right]\left(s\right)=\frac{1}{s^{m-\alpha }}{\mathcal{G}}_1\left[g^{\left(m\right)}\left(t\right)\right]\left(s\right), m-1<\alpha \le m.$$

(4)

$$\begin{array}{ll}\hfill {\mathcal{G}}_2\left[{\displaystyle D_t^{\alpha }}g\left(t\right)\right]\left(s\right)=& \frac{m-\alpha }{s^{m+1-\alpha }}{\mathcal{G}}_1\left[g^{\left(m\right)}\left(t\right)\right]\left(s\right)+\frac{1}{s^{m-\alpha }}{\mathcal{G}}_2\left[g^{\left(m\right)}\left(t\right)\right]\left(s\right),\\ & m-1<\alpha \le m.\end{array}$$

(5)

4. Solutions of Families of Fractional Differential Equations

Throughout this section, we derive three basic theorems to construct series solutions of fractional differential equations of the first and second order. Mathematica software is used to compute and simplify the results.

Theorem 3.

The solution of the fractional differential equation

$${\displaystyle D_t^{\alpha }}g\left(t\right)+a g^{\prime }\left(t\right)+b g\left(t\right)=0, 1<\alpha \le 2$$

(6)

where$a,b\in ℝ,$ $g\left(t\right)$is a piecewise continuous function in every finite interval$0\le t\le \beta$in which the ARA transform exists, with initial conditions

$$g\left(0\right)=c_0\mathit{and}{g}^{\prime }\left(0\right)=c_1$$

(7)

is given by

$$g\left(t\right)={\displaystyle \sum _{j=0}^{\infty }}{\displaystyle \sum _{i=0}^{\infty }}\frac{{\left(-1\right)}^{j+i}\Gamma \left(j+i+1\right)a^j{b}^i}{\Gamma \left(\left(\alpha -1\right)j+\alpha i+1\right)} \frac{t^{\left(\alpha -1\right)j+\alpha i}}{j!i!}\left(c_0\left(a{t}^{\alpha -1}+1\right)+\frac{c_1 t}{\left(\alpha -1\right)j+\alpha i+1}\right).$$

Proof of Theorem 3.

Applying the ARA transform of second order ${\mathcal{G}}_2\left[ \right]$ on both sides of Equation (6), we get:

$${\mathcal{G}}_2\left[{\displaystyle D_t^{\alpha }}g\left(t\right)\right]\left(s\right)+a{\mathcal{G}}_2\left[ g^{\prime }\left(t\right)\right]\left(s\right)+b {\mathcal{G}}_2\left[g\left(t\right)\right]\left(s\right)=0$$

Using Equation (5) with m = 2,

$$\frac{2-\alpha }{s^{3-\alpha }} {\mathcal{G}}_1\left[g^{″}\left(t\right)\right]\left(s\right)-\frac{1}{s^{1-\alpha }}\frac{d}{ds}\left(s{\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)\right)+\frac{g\left(0\right)}{s^{1-\alpha }}+a{\mathcal{G}}_2\left[ g^{\prime }\left(t\right)\right]\left(s\right)+b{\mathcal{G}}_2\left[g\left(t\right)\right]\left(s\right)=0.$$

Using Property 4 with n = 2 for m = 1 and m = 0 respectively, and the initial conditions in (7)

$$\begin{array}{ll}\hfill \frac{2-\alpha }{s^{3-\alpha }} {\mathcal{G}}_1\left[g^{″}\left(t\right)\right]\left(s\right)& -\frac{1}{s^{1-\alpha }}\frac{d}{ds}\left(s{\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)\right)+\frac{c_0}{s^{1-\alpha }}-a s\frac{d}{ds}{\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)\\ & -bs \frac{d}{ds}\left(\frac{{\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)}{s}\right)=0.\end{array}$$

Again, using Property 4 with n = 1 and m = 2, and the initial conditions in (7)

$$\begin{array}{ll}\hfill \frac{2-\alpha }{s^{3-\alpha }}& \left(s^2{\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)-c_0{s}^2-c_{1}s\right)-\frac{1}{s^{1-\alpha }}\frac{d}{ds}\left(s{\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)\right)+\frac{c_0}{s^{1-\alpha }}\\ & -a s\frac{d}{ds}{\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)-bs \frac{d}{ds}\left(\frac{{\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)}{s}\right)=0\end{array}$$

(8)

After simple computations, Equation (8) becomes

$$\begin{array}{ll}\hfill {\displaystyle {\mathcal{G}}_1^{\prime }}\left[g\left(t\right)\right]\left(s\right)\left(-\frac{s}{s^{1-\alpha }}-a s-b\right)& +{\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)\left(\frac{2-\alpha }{s^{3-\alpha }}{s}^2-\frac{1}{s^{1-\alpha }}+\frac{b}{s}\right)\\ & +c_0\left(-s^2\frac{2-\alpha }{s^{3-\alpha }}+\frac{1}{s^{1-\alpha }}\right)+c_1\left(-s\frac{2-\alpha }{s^{3-\alpha }}\right)=0\end{array}$$

$$\begin{array}{ll}\hfill {\displaystyle {\mathcal{G}}_1^{\prime }}\left[g\left(t\right)\right]\left(s\right)\left(-s^{\alpha }-a s-b\right)& +{\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)\left(\left(2-\alpha \right)s^{\alpha -1}-s^{\alpha -1}+b s^{-1}\right)\\ & +c_0\left(\alpha -1\right)s^{\alpha -1}+c_1\left(\alpha -2\right)s^{\alpha -2}=0\end{array}$$

$$\begin{gathered} {\displaystyle {\mathcal{G}}_1^{\prime }}\left[g\left(t\right)\right]\left(s\right)+{\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)\left(\frac{\left(2-\alpha \right)s^{\alpha -1}-s^{\alpha -1}+b s^{-1}}{-s^{\alpha }-a s-b}\right) \\ +\frac{c_0\left(\alpha -1\right)s^{\alpha -1}+c_1\left(\alpha -2\right)s^{\alpha -2}}{-s^{\alpha }-a s-b}=0 \end{gathered}$$

$${\displaystyle {\mathcal{G}}_1^{\prime }}\left[g\left(t\right)\right]\left(s\right)-{\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)\left(\frac{\left(1-\alpha \right)s^{\alpha }+b }{s^{\alpha +1}+a s^2+sb}\right)-\frac{c_0\left(\alpha -1\right)s^{\alpha }+c_1\left(\alpha -2\right)s^{\alpha -1}}{s^{\alpha +1}+a s^2+bs}=0.$$

(9)

Solving the ordinary differential Equation (9), we get:

$${\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)=\frac{c_0\left(s^{\alpha }+a s\right)}{s^{\alpha }+a s+b}+\frac{c_1 s^{\alpha -1}}{s^{\alpha }+a s+b}.$$

(10)

Now expand the term $\frac{1}{s^{\alpha }+a s+b}$ in the following form

$$\begin{array}{cc}\hfill \frac{1}{s^{\alpha }+a s+b}& =\frac{s^{-1}}{s^{\alpha -1}+a+b{s}^{-1}}=\frac{s^{-1}}{\left(s^{\alpha -1}+a\right)\left(1+\frac{b{s}^{-1}}{s^{\alpha -1}+a}\right)}=\frac{s^{-1}}{s^{\alpha -1}+a}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}{\left(\frac{-b{s}^{-1}}{s^{\alpha -1}+a}\right)}^i={\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}\frac{{\left(-b\right)}^i{s}^{-i-1}}{{\left(s^{\alpha -1}+a\right)}^{i+1}}\hfill \\ & ={\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}\frac{{\left(-b\right)}^i{s}^{-i-1}{s}^{-\alpha i-\alpha +i+1} }{{\left(1+a s^{1-\alpha }\right)}^{i+1}}={\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}\frac{{\left(-b\right)}^i{s}^{-\alpha i-\alpha } }{{\left(1+a s^{1-\alpha }\right)}^{i+1}}={\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}{\left(-b\right)}^i{s}^{-\alpha i-\alpha }{\left(\frac{1 }{1+a s^{1-\alpha }}\right)}^{i+1}\hfill \\ & ={\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}{\left(-b\right)}^i{s}^{-\alpha i-\alpha }{\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}\left(\begin{array}{c}j+i\\ j\end{array}\right){\left(-a s^{1-\alpha }\right)}^j={\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}{\left(-1\right)}^{j+i}\left(\begin{array}{c}j+i\\ j\end{array}\right)a^j{b}^i s^{\left(1-\alpha \right)j-\alpha i-\alpha }\hfill \end{array}$$

Thus, Equation (10) can be written as

$$\begin{array}{cc}\hfill {\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)& =c_0\left(s^{\alpha }+a s\right){\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}{\left(-1\right)}^{j+i}\left(\begin{array}{c}j+i\\ j\end{array}\right)a^j{b}^i s^{\left(1-\alpha \right)j-\alpha i-\alpha }\hfill \\ & +c_1 s^{\alpha -1}{\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}{\left(-1\right)}^{j+i}\left(\begin{array}{c}j+i\\ j\end{array}\right)a^j{b}^i s^{\left(1-\alpha \right)j-\alpha i-\alpha }\hfill \\ & =c_0{\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}{\left(-1\right)}^{j+i}\left(\begin{array}{c}j+i\\ j\end{array}\right)a^j{b}^i s^{\left(1-\alpha \right)j-\alpha i}\hfill \\ & +a{c}_0 {\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}{\left(-1\right)}^{j+i}\left(\begin{array}{c}j+i\\ j\end{array}\right)a^j{b}^i s^{\left(1-\alpha \right)j-\alpha i-\alpha +1}\hfill \\ & +c_1 {\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}{\left(-1\right)}^{j+i}\left(\begin{array}{c}j+i\\ j\end{array}\right)a^j{b}^i s^{\left(1-\alpha \right)j-\alpha i-1}\hfill \end{array}$$

(11)

Applying the inverse ARA transform of order 1 ${\displaystyle {\mathcal{G}}_1^{-1}}\left[ \right]$ on Equation (11), we have

$$\begin{array}{cc}\hfill g\left(t\right)& =c_0 {\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}{\left(-1\right)}^{j+i}\left(\begin{array}{c}j+i\\ j\end{array}\right)a^j{b}^i{\displaystyle {\mathcal{G}}_1^{-1}}\left[s^{\left(\alpha -2\right)j-2i}\right]\hfill \\ & +a c_0 {\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}{\left(-1\right)}^{j+i}\left(\begin{array}{c}j+i\\ j\end{array}\right)a^j{b}^i{\displaystyle {\mathcal{G}}_1^{-1}}\left[s^{\left(\alpha -2\right)j-2i+\alpha -2}\right]\hfill \\ & +c_1{\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}{\left(-1\right)}^{j+i}\left(\begin{array}{c}j+i\\ j\end{array}\right)a^j{b}^i{\displaystyle {\mathcal{G}}_1^{-1}}\left[s^{\left(\alpha -2\right)j-2i-1}\right]\hfill \\ & =c_0\left(a{t}^{\alpha -1}+1\right){\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}\frac{{\left(-1\right)}^{j+i}\Gamma \left(j+i+1\right)a^j{b}^i}{\Gamma \left(\left(\alpha -1\right)j+\alpha i+1\right)} \frac{t^{\left(\alpha -1\right)j+\alpha i}}{j!i!}\hfill \\ & +c_1 t{\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}\frac{{\left(-1\right)}^{j+i}\Gamma \left(j+i+1\right)a^j{b}^i}{\Gamma \left(\left(\alpha -1\right)j+\alpha i+2\right)} \frac{t^{\left(\alpha -1\right)j+\alpha i}}{j!i!}\hfill \end{array}$$

$$g\left(t\right)={\displaystyle \sum _{j=0}^{\infty }}{\displaystyle \sum _{i=0}^{\infty }}\frac{{\left(-1\right)}^{j+i}\Gamma \left(j+i+1\right)a^j{b}^i}{\Gamma \left(\left(\alpha -1\right)j+\alpha i+1\right)} \frac{t^{\left(\alpha -1\right)j+\alpha i}}{j!i!}\left(c_0\left(a{t}^{\alpha -1}+1\right)+\frac{c_1 t}{\left(\alpha -1\right)j+\alpha i+1}\right).$$

□

Example 1.

The fractional differential equation

$${\displaystyle D_t^{\frac{3}{2}}}g\left(t\right)+2g^{\prime }\left(t\right)+g\left(t\right)=0$$

with initial conditions g(0) = 1 and g′(0) = 0, has the following solution

$$g\left(t\right)={\displaystyle \sum _{j=0}^{\infty }}{\displaystyle \sum _{i=0}^{\infty }}\frac{{\left(-1\right)}^{j+i}\Gamma \left(j+i+1\right)2^j{t}^{\frac{1}{2}j+\frac{3}{2}i}}{j!i!\Gamma \left(\frac{1}{2}j+\frac{3}{2}i+1\right)} \left(2t^{\frac{1}{2}}+1\right).$$

Putting α = 0 in Theorem 3 we get the following results.

Corollary 1.

The solution of the fractional differential equation

$${\displaystyle D_t^{\alpha }}g\left(t\right)+bg\left(t\right)=0, 1<\alpha \le 2,$$

where$b\in ℝ$with the initial conditions$g\left(0\right)=c_0$and$g^{\prime }\left(0\right)=c_1$is

$$\begin{array}{ll}\hfill g\left(t\right)& ={\displaystyle \sum _{i=0}^{\infty }}\frac{{\left(-1\right)}^i{b}^i{t}^{\alpha i}}{\Gamma \left(\alpha i+1\right)} \left(c_0+\frac{c_1 t}{\alpha i+1}\right)=c_0{\displaystyle \sum _{i=0}^{\infty }}\frac{{\left(-1\right)}^i{b}^i{t}^{\alpha i}}{\Gamma \left(\alpha i+1\right)}+c_1 t{\displaystyle \sum _{i=0}^{\infty }}\frac{{\left(-1\right)}^i{b}^i{t}^{\alpha i}}{\Gamma \left(\alpha i+2\right)}\\ & =c_0{E}_{\alpha ,1}\left(-b{t}^{\alpha }\right)+c_1 t{E}_{\alpha ,2}\left(-b{t}^{\alpha }\right).\end{array}$$

Example 2.

The fractional differential equation

$${\displaystyle D_t^{\alpha }}g\left(t\right)+g\left(t\right)=0, 1<\alpha \le 2,$$

(12)

with initial conditions$g\left(0\right)=1$and$g^{\prime }\left(0\right)=1$, has the following solution

$$\begin{array}{ll}\hfill g\left(t\right)& ={\displaystyle \sum _{i=0}^{\infty }}\frac{{\left(-1\right)}^i{t}^{\alpha i}}{\Gamma \left(\alpha i+1\right)}+t{\displaystyle \sum _{i=0}^{\infty }}\frac{{\left(-1\right)}^i{t}^{\alpha i}}{\Gamma \left(\alpha i+2\right)}\\ & =E_{\alpha ,1}\left(-t^{\alpha }\right)+t{E}_{\alpha ,2}\left(-t^{\alpha }\right).\end{array}$$

(13)

The exact solution of Equation (12) can be obtained at α = 2 as the following

$$g\left(t\right)=\mathit{cos}t+\mathit{sin}t.$$

(14)

Table 1. The absolute error at α = 2 for Example 2.

t	Absolute Error
0	0
0.5	0
1	2.22045 × 10−16
1.5	2.22045 × 10−16
2	1.11022 × 10−16
2.5	1.11022 × 10−16
3	8.88178 × 10−16
3.5	2.22045 × 10−16
4	2.22045 × 10−16

shows the absolute error between the exact solution (14) and the solution obtained in Equation (13) with α = 2.

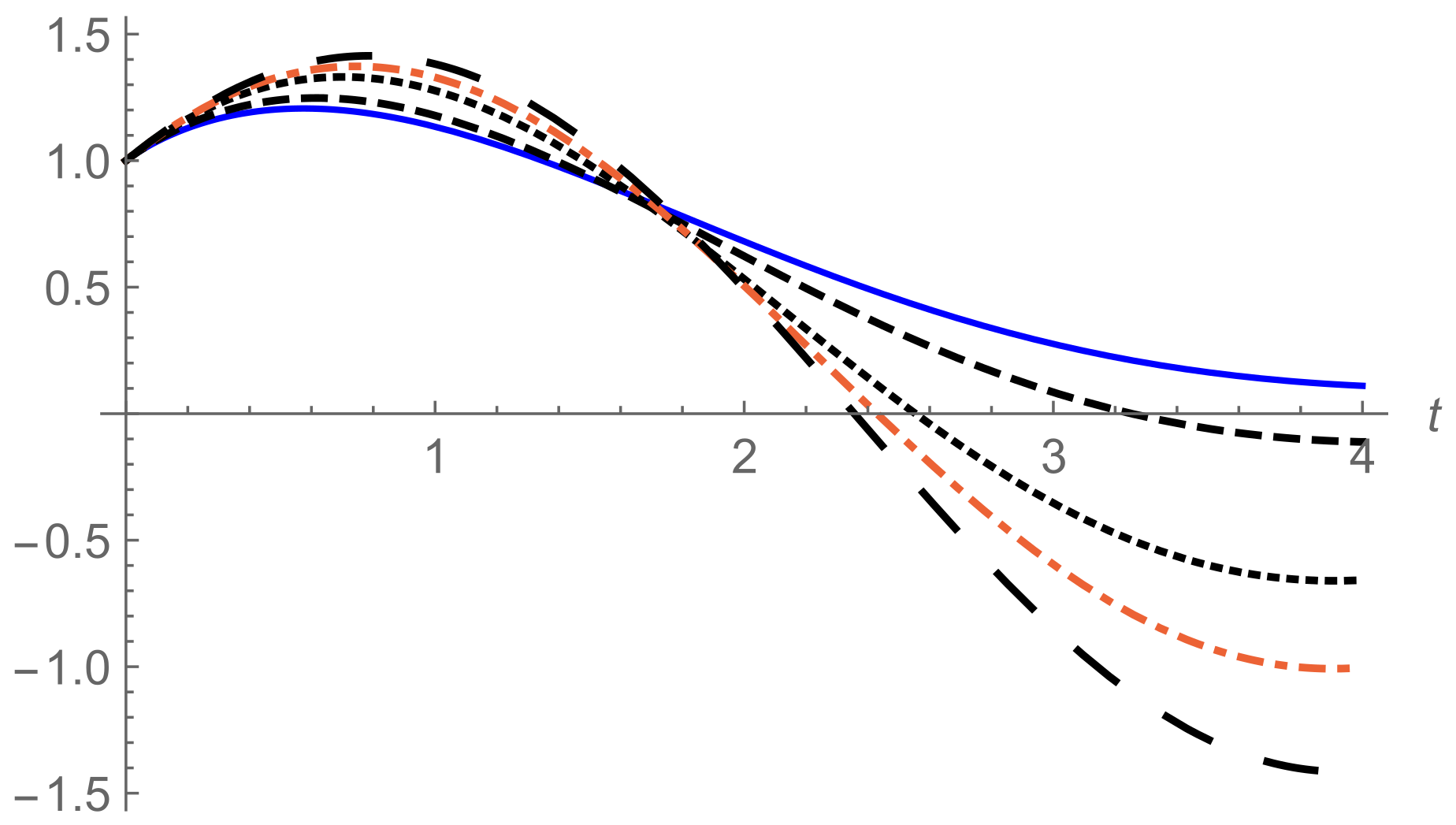
Figure 1 illustrates the solution behavior of the fractional differential Equation (12) at various values of α. Blue line: exact solution at α = 2, dashed line at α = 1.9, dotted line at α = 1.8, dash. dotted line at α = 1.6, large dash line at α = 1.5.

Corollary 2.

The solution of the harmonic vibration equation [27]

$${\displaystyle D_t^{\alpha }}g\left(t\right)+w^{2}g\left(t\right)=0, 1<\alpha \le 2,$$

with the initial conditions $g\left(0\right)=c_0$and $g^{\prime }\left(0\right)=c_1$ is given by

$$g\left(t\right)=c_0{E}_{\alpha ,1}\left(-w^2{t}^{\alpha }\right)+c_1 t{E}_{\alpha ,2}\left(-w^2{t}^{\alpha }\right).$$

Theorem 4.

The solution of the fractional differential equation

$$g^{″}\left(t\right)+a {\displaystyle D_t^{\alpha }}g\left(t\right)+b g\left(t\right)=0, 1<\alpha \le 2,$$

(15)

where$a,b\in ℝ,$ $g\left(t\right)$is a piecewise continuous function in every finite interval$0\le t\le \beta$in which the ARA transform exists, with initial conditions

$$g\left(0\right)=c_0\mathit{and}{g}^{\prime }\left(0\right)=c_1$$

(16)

is given by

$$\begin{array}{ll}\hfill g\left(t\right)& ={\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}\frac{{\left(-1\right)}^{j+i}\Gamma \left(j+i+1\right)a^j{b}^i}{\Gamma \left(\left(2-\alpha \right)j+2i+1\right)}\frac{t^{\left(2-\alpha \right)j+2i}}{j!i!}\left(c_0+\frac{c_{1}t}{\left(2-\alpha \right)j+2i+1}\right)\\ & + {\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}\frac{{\left(-1\right)}^{j+i}\Gamma \left(j+i+1\right)a^j{b}^i}{\Gamma \left(\left(2-\alpha \right)j+2i-\alpha +3\right)}\frac{t^{\left(2-\alpha \right)j+2i-\alpha +2}}{j!i!}\\ & \left(a{c}_0+\frac{a c_{1}t}{\left(2-\alpha \right)j+2i-\alpha +3}\right).\end{array}$$

Proof of Theorem 4.

Applying the ARA integral transform of second order ${\mathcal{G}}_2\left[ \right]$ on both sides of Equation (15), we get

$${\mathcal{G}}_2\left[g^{″}\left(t\right)\right]\left(s\right)+a{\mathcal{G}}_2\left[{\displaystyle D_t^{\alpha }}g\left(t\right)\right]\left(s\right)+b {\mathcal{G}}_2\left[g\left(t\right)\right]\left(s\right)=0.$$

Using Equation (5) with m = 2,

$$\begin{array}{ll}\hfill {\mathcal{G}}_2\left[g^{″}\left(t\right)\right]\left(s\right)& +a\left(\frac{2-\alpha }{s^{3-\alpha }} {\mathcal{G}}_1\left[g^{″}\left(t\right)\right]\left(s\right)+\frac{g\left(0\right)}{s^{1-\alpha }}-\frac{{\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)}{s^{1-\alpha }}-s^{\alpha }{\displaystyle {\mathcal{G}}_1^{\prime }}\left[g\left(t\right)\right]\left(s\right)\right)\\ & +b {\mathcal{G}}_2\left[g\left(t\right)\right]\left(s\right)=0\end{array}$$

Using Property 4 with n = 2 for m = 2 and m = 0 respectively, and the initial conditions in (16), we get

$$\begin{array}{ll}\hfill c_{0}s-s^2{\displaystyle {\mathcal{G}}_1^{\prime }}\left[g\left(t\right)\right]\left(s\right)& -s{\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)\\ & +a(\frac{2-\alpha }{s^{3-\alpha }} {\mathcal{G}}_1\left[g^{″}\left(t\right)\right]\left(s\right)+\frac{c_0}{s^{1-\alpha }}-\frac{{\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)}{s^{1-\alpha }}\\ & -s^{\alpha }{\displaystyle {\mathcal{G}}_1^{\prime }}\left[g\left(t\right)\right]\left(s\right))+bs\frac{d}{ds}\left(\frac{{\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)}{s}\right)=0\end{array}$$

Again, using Property 4 with n = 1 and m = 2, and the initial conditions in (16),

$$\begin{gathered} c_{0}s-s^2{\displaystyle {\mathcal{G}}_1^{\prime }}\left[g\left(t\right)\right]\left(s\right)-s{\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)+a\left(\frac{2-\alpha }{s^{3-\alpha }} \left(s^2{\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)-s^2{c}_0-s c_1\right)+ \\ \frac{g\left(0\right)}{s^{1-\alpha }}-\frac{{\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)}{s^{1-\alpha }}-s^{\alpha }{\displaystyle {\mathcal{G}}_1^{\prime }}\left[g\left(t\right)\right]\left(s\right)\right)+bs\frac{d}{ds}\left(\frac{{\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)}{s}\right)=0. \end{gathered}$$

(17)

After simple computations, Equation (17) can be written as

$$\begin{array}{ll}\hfill {\displaystyle {\mathcal{G}}_1^{\prime }}\left[g\left(t\right)\right]\left(s\right)& +{\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)\left(\frac{s-a s^{\alpha -1}+a \alpha s^{\alpha -1}-\frac{b}{s}}{s^2+a s^{\alpha }+b}\right)\\ & +\frac{c_0\left(-s+a s^{\alpha -1}-a \alpha s^{\alpha -1}\right)+c_1\left(2a s^{\alpha -2}-a \alpha s^{\alpha -2}\right)}{s^2+a s^{\alpha }+b}=0\end{array}$$

(18)

Solving the ordinary differential Equation (18), we get

$${\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)=c_0\frac{s^2+a s^{\alpha }}{s^2+a s^{\alpha }+b}+c_1\frac{ s+a s^{\alpha -1}}{s^2+a s^{\alpha }+b}.$$

(19)

Now, to get our target, we expand the term $\frac{1}{s^2+a s^{\alpha }+b}$ as follows

$$\begin{array}{cc}\hfill \frac{1}{s^2+a s^{\alpha }+b}& =\frac{s^{-\alpha }}{s^{2-\alpha }+a+b{s}^{-\alpha }}=\frac{s^{-\alpha }}{\left(s^{2-\alpha }+a\right)\left(1+\frac{b{s}^{-\alpha }}{s^{2-\alpha }+a}\right)}=\frac{s^{-\alpha }}{s^{2-\alpha }+a}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}{\left(\frac{-b{s}^{-\alpha }}{s^{2-\alpha }+a}\right)}^i\hfill \\ & ={\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}\frac{{\left(-b\right)}^i{s}^{-\alpha i-\alpha }}{{\left(s^{2-\alpha }+a\right)}^{i+1}}={\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}\frac{{\left(-b\right)}^i{s}^{-\alpha i-\alpha } }{{\left(1+a s^{\alpha -2}\right)}^{i+1}{s}^{\left(2-\alpha \right)\left(i+1\right)}}={\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}\frac{{\left(-b\right)}^i{s}^{-\alpha i-\alpha } }{{\left(1+a s^{\alpha -2}\right)}^{i+1}{s}^{2i+2-\alpha i-\alpha }}\hfill \\ & ={\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}\frac{{\left(-b\right)}^i{s}^{-2i-2} }{{\left(1+a s^{\alpha -2}\right)}^{i+1}}={\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}{\left(-b\right)}^i{s}^{-2i-2}{\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}\left(\begin{array}{c}j+i\\ j\end{array}\right){\left(-a s^{\alpha -2}\right)}^j\hfill \\ & ={\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}{\left(-1\right)}^{j+i}\left(\begin{array}{c}j+i\\ j\end{array}\right)a^j{b}^i s^{\left(\alpha -2\right)j-2i-2}\hfill \end{array}$$

Thus, Equation (19) can be written as

$$\begin{array}{cc}\hfill {\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)& =c_0 s^2{\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}{\left(-1\right)}^{j+i}\left(\begin{array}{c}j+i\\ j\end{array}\right)a^j{b}^i s^{\left(\alpha -2\right)j-2i-2}\hfill \\ & +a c_0 s^{\alpha }{\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}{\left(-1\right)}^{j+i}\left(\begin{array}{c}j+i\\ j\end{array}\right)a^j{b}^i s^{\left(\alpha -2\right)j-2i-2}\hfill \\ & +c_{1}s{\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}{\left(-1\right)}^{j+i}\left(\begin{array}{c}j+i\\ j\end{array}\right)a^j{b}^i s^{\left(\alpha -2\right)j-2i-2}\hfill \\ & +a c_1 s^{\alpha -1}{\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}{\left(-1\right)}^{j+i}\left(\begin{array}{c}j+i\\ j\end{array}\right)a^j{b}^i s^{\left(\alpha -2\right)j-2i-2}\hfill \end{array}$$

$$\begin{array}{cc}\hfill {\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)& =c_0 {\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}{\left(-1\right)}^{j+i}\left(\begin{array}{c}j+i\\ j\end{array}\right)a^j{b}^i s^{\left(\alpha -2\right)j-2i}\hfill \\ & +a c_0 {\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}{\left(-1\right)}^{j+i}\left(\begin{array}{c}j+i\\ j\end{array}\right)a^j{b}^i s^{\left(\alpha -2\right)j-2i+\alpha -2}\hfill \\ & +c_1{\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}{\left(-1\right)}^{j+i}\left(\begin{array}{c}j+i\\ j\end{array}\right)a^j{b}^i s^{j\left(\alpha -2\right)-2i-1}\hfill \\ & +a c_1{\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}{\left(-1\right)}^{j+i}\left(\begin{array}{c}j+i\\ j\end{array}\right)a^j{b}^i s^{\left(\alpha -2\right)j-2i+\alpha -1}\hfill \end{array}$$

(20)

Applying the inverse ARA transform ${\displaystyle {\mathcal{G}}_1^{-1}}\left[\right]$ on Equation (20), we have

$$\begin{array}{cc}\hfill g\left(t\right)& =c_0 {\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}{\left(-1\right)}^{j+i}\left(\begin{array}{c}j+i\\ j\end{array}\right)a^j{b}^i{\displaystyle {\mathcal{G}}_1^{-1}}\left[s^{\left(\alpha -2\right)j-2i}\right]\hfill \\ & +a c_0 {\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}{\left(-1\right)}^{j+i}\left(\begin{array}{c}j+i\\ j\end{array}\right)a^j{b}^i{\displaystyle {\mathcal{G}}_1^{-1}}\left[s^{\left(\alpha -2\right)j-2i+\alpha -2}\right]\hfill \\ & +c_1{\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}{\left(-1\right)}^{j+i}\left(\begin{array}{c}j+i\\ j\end{array}\right)a^j{b}^i{\displaystyle {\mathcal{G}}_1^{-1}}\left[s^{\left(\alpha -2\right)j-2i-1}\right]\hfill \\ & +a c_1{\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}{\left(-1\right)}^{j+i}\left(\begin{array}{c}j+i\\ j\end{array}\right)a^j{b}^i{\displaystyle {\mathcal{G}}_1^{-1}}\left[s^{\left(\alpha -2\right)j-2i+\alpha -1}\right]\hfill \end{array}$$

$$\begin{array}{cc}\hfill g\left(t\right)& =c_0{\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}\frac{{\left(-1\right)}^{j+i}\Gamma \left(j+i+1\right)a^j{b}^i}{\Gamma \left(\left(2-\alpha \right)j+2i+1\right)}\frac{t^{\left(2-\alpha \right)j+2i}}{j!i!}\hfill \\ & +a{c}_0 {\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}\frac{{\left(-1\right)}^{j+i}\Gamma \left(j+i+1\right)a^j{b}^i}{\Gamma \left(\left(2-\alpha \right)j+2i-\alpha +3\right)}\frac{t^{\left(2-\alpha \right)j+2i-\alpha +2}}{j!i!}\hfill \\ & +c_1 {\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}\frac{{\left(-1\right)}^{j+i}\Gamma \left(j+i+1\right)a^j{b}^i}{\Gamma \left(\left(2-\alpha \right)j+2i+2\right)}\frac{t^{\left(2-\alpha \right)j+2i+1}}{j!i!}\hfill \\ & +a c_1{\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}\frac{{\left(-1\right)}^{j+i}\Gamma \left(j+i+1\right)a^j{b}^i}{\Gamma \left(\left(2-\alpha \right)j+2i-\alpha +4\right)}\frac{t^{\left(2-\alpha \right)j+2i-\alpha +3}}{j!i!}\hfill \\ & ={\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}\frac{{\left(-1\right)}^{j+i}\Gamma \left(j+i+1\right)a^j{b}^i}{\Gamma \left(\left(2-\alpha \right)j+2i+1\right)}\frac{t^{\left(2-\alpha \right)j+2i}}{j!i!}\left(c_0+\frac{c_{1}t}{\left(2-\alpha \right)j+2i+1}\right)\hfill \\ & +{\displaystyle {\displaystyle \sum _{j=0}^{\infty }}}{\displaystyle {\displaystyle \sum _{i=0}^{\infty }}}\frac{{\left(-1\right)}^{j+i}\Gamma \left(j+i+1\right)a^j{b}^i}{\Gamma \left(\left(2-\alpha \right)j+2i-\alpha +3\right)}\frac{t^{\left(2-\alpha \right)j+2i-\alpha +2}}{j!i!}\left(a{c}_0+\frac{a c_{1}t}{\left(2-\alpha \right)j+2i-\alpha +3}\right)\hfill \end{array}$$

□

Example 3.

The fractional differential equation

$$g^{″}\left(t\right)+2{\displaystyle D_t^{\frac{3}{2}}}g\left(t\right)+2g\left(t\right)=0,$$

with initial conditions$g\left(0\right)=1$and$g^{\prime }\left(0\right)=0$has the following series solution

$$g\left(t\right)={\displaystyle \sum _{j=0}^{\infty }}{\displaystyle \sum _{i=0}^{\infty }}\frac{{\left(-1\right)}^{j+i}\Gamma \left(j+i+1\right)2^j{t}^{\frac{1}{2}j+2i}}{j!i!\Gamma \left(\frac{1}{2}j+2i+1\right)}+2{\displaystyle \sum _{j=0}^{\infty }}{\displaystyle \sum _{i=0}^{\infty }}\frac{{\left(-1\right)}^{j+i}\Gamma \left(j+i+1\right)2^j{t}^{\frac{1}{2}j+2i+\frac{1}{2}}}{j!i!\Gamma \left(\frac{1}{2}j+2i+\frac{3}{2}\right)}.$$

Putting $b=0$ in Theorem 2 we get the following result.

Corollary 3.

The solution of the fractional differential equation of the form:

$$g^{″}\left(t\right)+a{\displaystyle D_t^{\alpha }}g\left(t\right)=0, 1<\alpha \le 2$$

where$a\in ℝ$, with initial conditions$g\left(0\right)=c_0$and$g^{\prime }\left(0\right)=c_1$is

$$\begin{array}{ll}\hfill g\left(t\right)& ={\displaystyle \sum _{j=0}^{\infty }}\frac{{\left(-1\right)}^j{a}^j{t}^{\left(2-\alpha \right)j}}{\Gamma \left(\left(2-\alpha \right)j+1\right)}\left(c_0+\frac{c_{1}t}{\left(2-\alpha \right)j+1}\right)\\ & +{\displaystyle \sum _{j=0}^{\infty }}\frac{{\left(-1\right)}^j{a}^j{t}^{\left(2-\alpha \right)j-\alpha +2}}{\Gamma \left(\left(2-\alpha \right)j-\alpha +3\right)}\left(a{c}_0+\frac{a{c}_{1}t}{\left(2-\alpha \right)j-\alpha +3}\right).\end{array}$$

Theorem 5.

The solution of the fractional differential equation of the form

$${\displaystyle D_t^{\alpha }}g\left(t\right)-b g\left(t\right)=0, 0<\alpha \le 1,$$

(21)

where$b\in ℝ,$ $g\left(t\right)$is a piecewise continuous function in every finite interval$0\le t\le \beta$in which the ARA transform exists, with initial condition

$$g\left(0\right)=c_0$$

(22)

is:

$$g\left(t\right)=c_0{\displaystyle \sum _{i=0}^{\infty }}\frac{{\left(b{t}^{\alpha }\right)}^i}{\Gamma \left(\alpha i+1\right)}.$$

Proof of Theorem 5.

Applying the ARA transform of the second order ${\mathcal{G}}_2\left[ \right]$ on both sides of Equation (21) we get

$${\mathcal{G}}_2\left[{\displaystyle D_t^{\alpha }}g\left(t\right)\right]\left(s\right)-b {\mathcal{G}}_2\left[g\left(t\right)\right]\left(s\right)=0.$$

Using Equation (5) of Remark 1 with $m=1$ and the initial condition (22)

$$s^{\alpha -1}\left(1-\alpha \right){\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)-c_0{s}^{\alpha -1}\left(1-\alpha \right)-s^{\alpha }{\displaystyle {\mathcal{G}}_1^{\prime }}\left[g\left(t\right)\right]\left(s\right)-b {\mathcal{G}}_2\left[g\left(t\right)\right]\left(s\right)=0$$

Again, using Equation (4) with $n=2$ and $m=0$ we have

$$s^{\alpha -1}\left(1-\alpha \right){\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)-c_0{s}^{\alpha -1}\left(1-\alpha \right)-s^{\alpha }{\displaystyle {\mathcal{G}}_1^{\prime }}\left[g\left(t\right)\right]\left(s\right)-b\frac{{\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)}{s}+b{\displaystyle {\mathcal{G}}_1^{\prime }}\left[g\left(t\right)\right]\left(s\right)=0$$

$${\displaystyle {\mathcal{G}}_1^{\prime }}\left[g\left(t\right)\right]\left(s\right)\left(b-s^{\alpha }\right)+{\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)\left(s^{\alpha -1}\left(1-\alpha \right)-\frac{b}{s}\right)-c_0{s}^{\alpha -1}\left(1-\alpha \right)=0$$

$${\displaystyle {\mathcal{G}}_1^{\prime }}\left[g\left(t\right)\right]\left(s\right)+\left(\frac{s^{\alpha -1}\left(1-\alpha \right)-\frac{b}{s}}{b-s^{\alpha }}\right){\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)-\frac{c_0{s}^{\alpha -1}\left(1-\alpha \right)}{b-s^{\alpha }}=0.$$

(23)

Solving the ordinary differential Equation (23), we get

$${\mathcal{G}}_1\left[g\left(t\right)\right]\left(s\right)=\frac{c_0{s}^{\alpha }}{s^{\alpha }-b}=c_0{\displaystyle \sum _{i=0}^{\infty }}{\left(b{s}^{-\alpha }\right)}^i=c_0{\displaystyle \sum _{i=0}^{\infty }}{b}^i{s}^{-\alpha i}.$$

(24)

To get our result we apply the inverse ARA transform of order 1, ${\displaystyle {\mathcal{G}}_1^{-1}}\left[ \right]$ of both sides of Equation (24).

$$g\left(t\right)=c_0{\displaystyle \sum _{i=0}^{\infty }}\frac{{\left(b{t}^{\alpha }\right)}^i}{\Gamma \left(\alpha i+1\right)}=c_0{E}_{\alpha ,1}\left(b{t}^{\alpha }\right).$$

□

5. Conclusions

In this work a new technique has been developed for solving families of fractional differential equations. We introduced new formulas using the ARA transform to achieve series solutions of differential equations with fractional order. A formula of the ARA transform for Caputo fractional derivative was established and implemented to solve examples and obtain new results. Three main theorems are presented, including the series solution of some fractional differential equations of first and second orders. In future work, we intend to solve partial differential equations and fractional integral equations using new formulas combined with the ARA transform.