Nonlinear Analysis of Tropical Waves and Cyclogenesis Excited by Pressure Disturbance in Atmosphere
Abstract
:1. Introduction
2. Constructing Nonlinear Mathematical Model
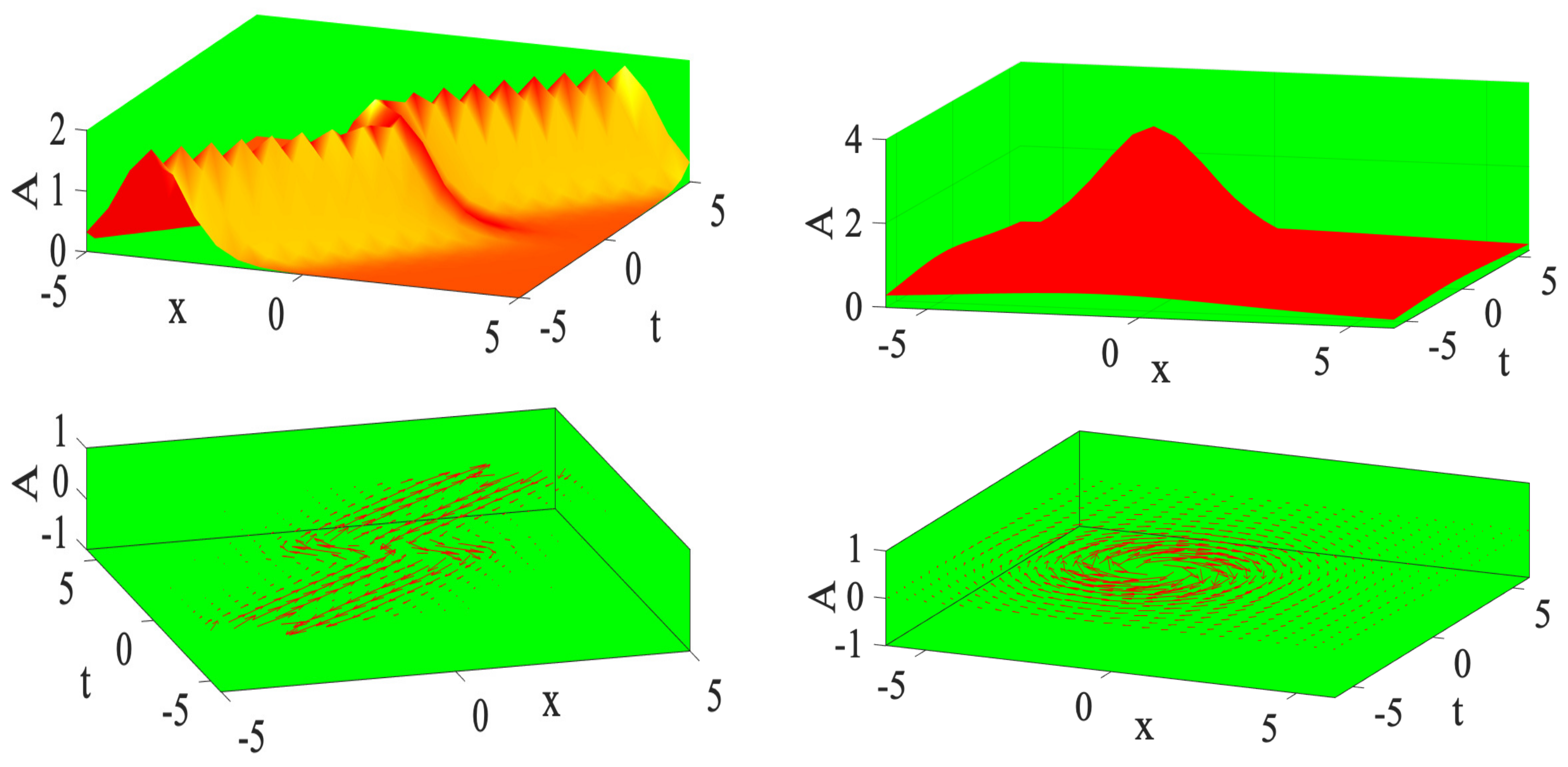
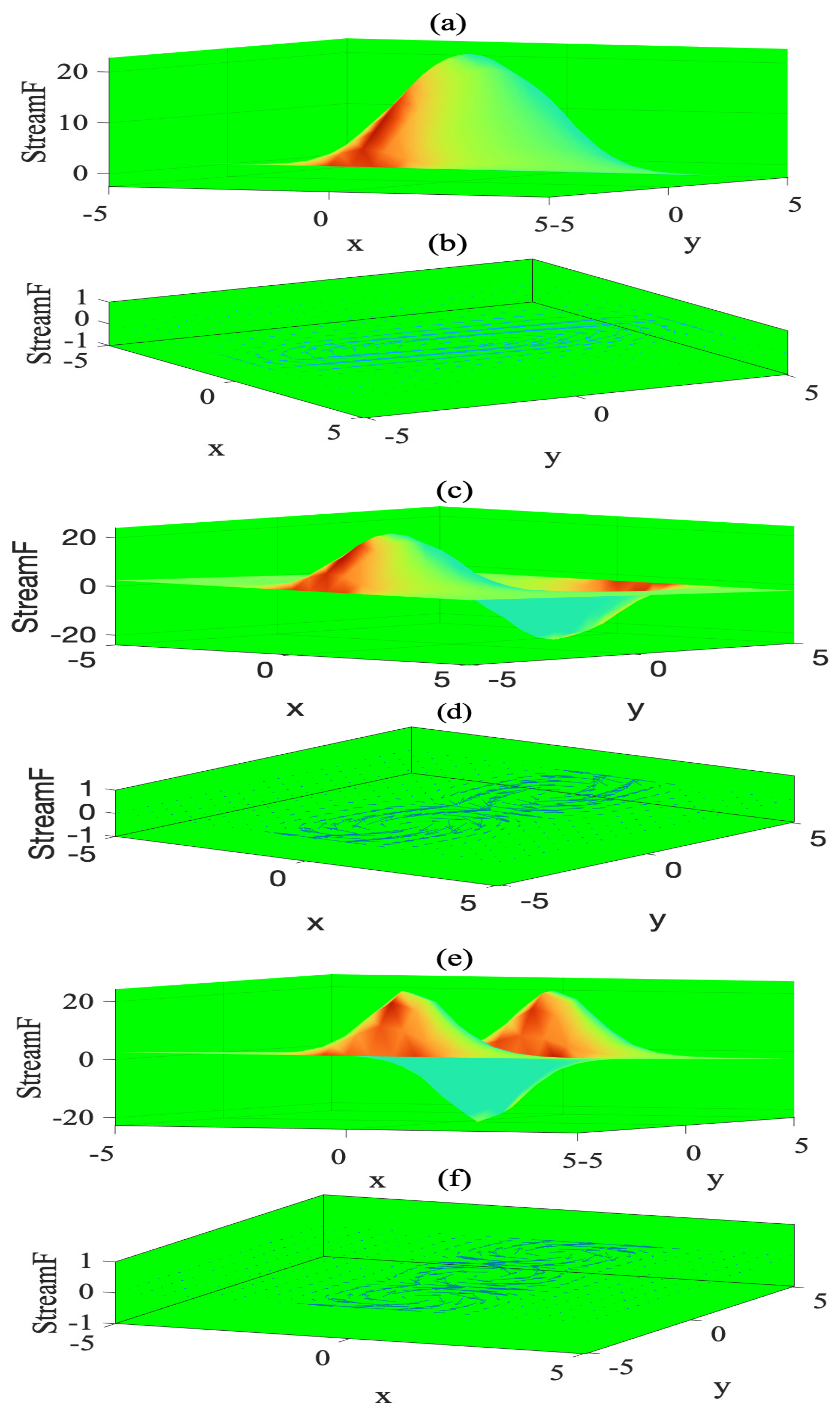
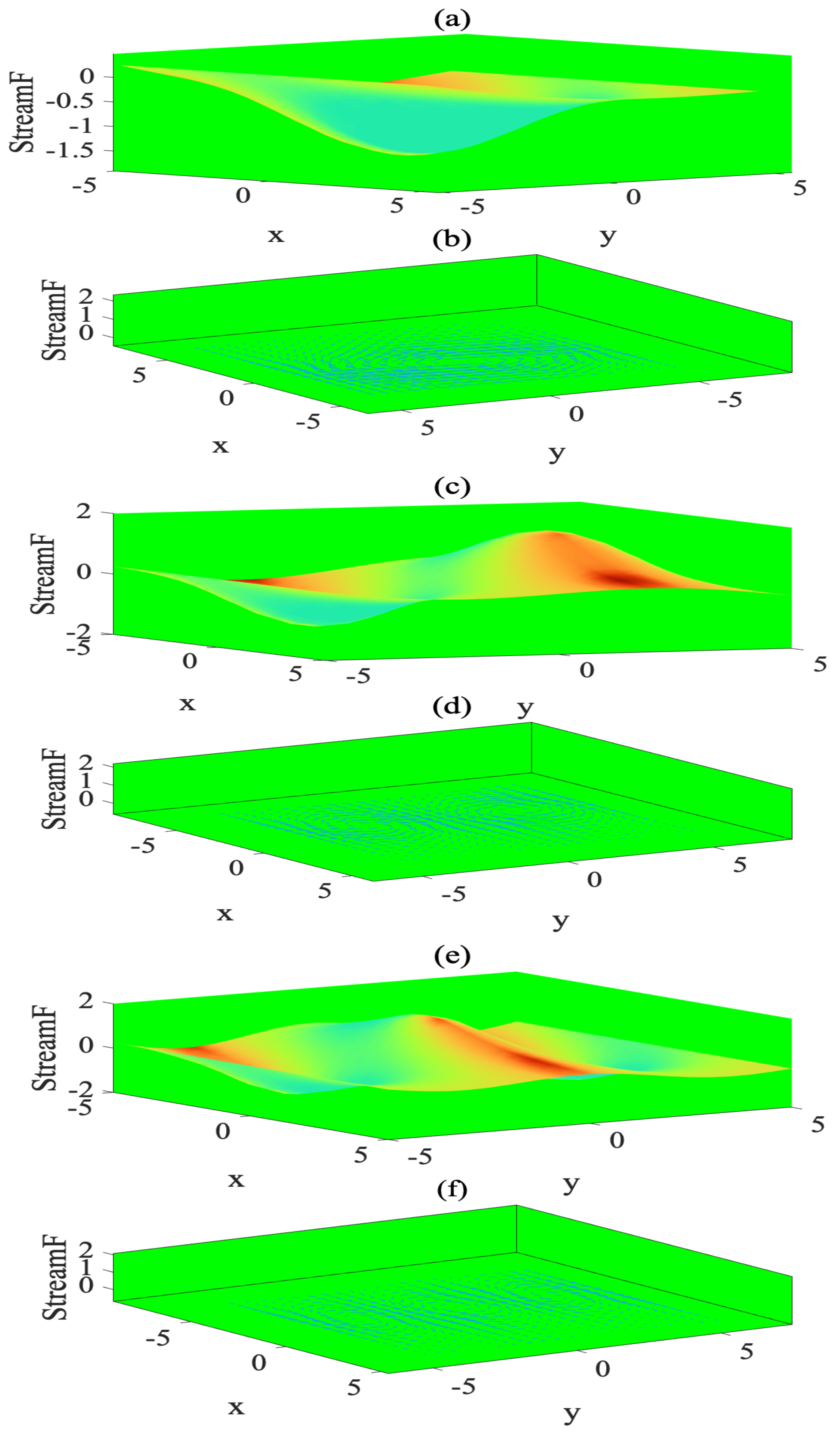
3. Solutions of the Forced KdV Equation
4. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hirota, R. Exact n-soliton solutions of the wave equation of long waves in shallow water and in nonlinear lattices. J. Math. Phys. 1973, 14, 810–814. [Google Scholar] [CrossRef]
- Hirota, R. The Direct Method in Soliton Theory; Cambridge University Press: Cambridge, UK, 2004. [Google Scholar]
- Ablowitz, M.J.; Clarkson, P.A. Solitons, Nonlinear Evolution Equations and Inverse Scattering Transform; Cambridge University Press: Cambridge, UK, 1991. [Google Scholar]
- Ma, W. Exact one-periodic and two-periodic wave solutions to Hirota bilinear equations in (2 + 1) dimensions. Mod. Phys. Lett. A 2009, 24, 1677–1888. [Google Scholar] [CrossRef] [Green Version]
- Ma, W. Bilinear equations and resonant solutions characterized by bell polynomials. Rep. Math. Phys. 2013, 72, 41–56. [Google Scholar] [CrossRef]
- Ma, W.X. N-soliton solutions and the hirota conditions in (2 + 1)-dimensions. Opt. Quantum Electron. 2020, 52, 511–512. [Google Scholar] [CrossRef]
- Chen, F.; Zhang, H.Q. Rogue waves on the periodic background in the higher-order modified Korteweg-de Vries equation. Mod. Phys. Lett. B 2021, 35, 2150081. [Google Scholar] [CrossRef]
- Gao, X.; Zhang, H.Q. Rogue waves for the Hirota equation on the Jacobi elliptic cn-function background. Nonlinear Dyn. 2020, 101, 1159–1168. [Google Scholar] [CrossRef]
- Chen, J.B.; Pelinovsky, D.E. Rogue periodic waves of the modified KdV equation. Nonlinearity 2018, 31, 1955–1980. [Google Scholar] [CrossRef] [Green Version]
- Zhao, J.; Guo, B. Analytic solutions to forced KdV equation. Commun. Theor. Phys. 2009, 52, 279–283. [Google Scholar]
- Salas, A.H. Computing solutions to a forced kdv equation. Nonlinear Anal. Real World Appl. 2011, 12, 1314–1320. [Google Scholar] [CrossRef]
- Gandarias, M.L.; Bruzon, M.S. Some conservation laws for a forced KdV equation. Nonlinear Anal. Real World Appl. 2012, 13, 2692–2700. [Google Scholar] [CrossRef]
- Ali, R.; Saha, A.; Chatterjee, P. Analytical electron acoustic solitary wave solution for the forced KdV equation in super thermal plasmas. Phys. Plasmas 2017, 24, 122106. [Google Scholar] [CrossRef]
- Amiya, D.; Uttam, K.M. Integrability, bilinearization, solitons and exact three wave solutions for a forced Korteweg-de Vries equation. Commun. Nonlinear Sci. Numer. Simul. 2021, 102, 105936. [Google Scholar]
- Mandi, L.; Mondal, K.K.; Chatterjee, P. Analytical solitary wave solution of the dust ion acoustic waves for the damped forced modified Korteweg-de Vries equation in q-nonextensive plasmas. Eur. Phys. J. Spec. Top. 2019, 228, 2753–2768. [Google Scholar] [CrossRef]
- Muller, C.J.; Romps, D.M. Acceleration of tropical cyclogenesis by self-aggregation feedbacks. Proc. Natl. Acad. Sci. USA 2018, 115, 2930–2935. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Emanuel, K. 100 years of progress in tropical cyclone research. A Century of Progress in Atmospheric and Related Sciences: Celebrating the American Meteorological Society Centennial. Meteorol. Monogr. Am. Meteorol. Soc. 2018, 59, 15.1–15.68. [Google Scholar]
- Davis, C.A. The formation of moist vortices and tropical cyclones in idealized simulations. J. Atmos. Sci. 2015, 72, 3499–3516. [Google Scholar] [CrossRef]
- Frank, W.M.; Roundy, P.E. The role of tropical waves in tropical cyclogenesis. Mon. Weather Rev. 2006, 134, 2397–2417. [Google Scholar] [CrossRef]
- Ritchie, E.; Holland, G. Large-scale patterns associated with tropical cyclogenesis in the western Pacific. Mon. Weather Rev. 1999, 127, 2027–2043. [Google Scholar] [CrossRef]
- Narenpitak, P.; Bretherton, C.S.; Khairoutdinov, M.F. The role of multiscale interaction in tropical cyclogenesis and its predictability in near-global aquaplanet cloud-resolving simulations. J. Atmos. Sci. 2020, 55, 3177–3196. [Google Scholar] [CrossRef]
- Yuan, P.; Qi, J.; Li, Z.; An, H. General M-lumps, T-breathers, and hybrid solutions to (2 + 1)-dimensional generalized KDKK equation. Chin. Phys. B 2021, 30, 040503. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Z.-L.; Liu, J.-Q. Nonlinear Analysis of Tropical Waves and Cyclogenesis Excited by Pressure Disturbance in Atmosphere. Mathematics 2021, 9, 3038. https://doi.org/10.3390/math9233038
Li Z-L, Liu J-Q. Nonlinear Analysis of Tropical Waves and Cyclogenesis Excited by Pressure Disturbance in Atmosphere. Mathematics. 2021; 9(23):3038. https://doi.org/10.3390/math9233038
Chicago/Turabian StyleLi, Zi-Liang, and Jin-Qing Liu. 2021. "Nonlinear Analysis of Tropical Waves and Cyclogenesis Excited by Pressure Disturbance in Atmosphere" Mathematics 9, no. 23: 3038. https://doi.org/10.3390/math9233038
APA StyleLi, Z.-L., & Liu, J.-Q. (2021). Nonlinear Analysis of Tropical Waves and Cyclogenesis Excited by Pressure Disturbance in Atmosphere. Mathematics, 9(23), 3038. https://doi.org/10.3390/math9233038





