Mathematical Analysis of Biodegradation Model under Nonlocal Operator in Caputo Sense
Abstract
:1. Introduction and Motivation
- and denote the soluble substrate, heterotroph (particulate biomass), soluble micropollutants, and particulate micropollutants, respectively.
- and represent the substrate concentration in the feed, the flow rate through bioreactor, and the volume of the bioreactor, respectively.
- and denote the recycle concentration factor, heterotroph concentration, and the recycle ratio, respectively.
- and denote the decay coefficient of particulate biomass, soluble micropollutants concentration, and the total suspended solids, respectively.
- represent the biological removal rate and residence time.
- and represent the Henry coefficient of micropollutant, the flow rate of aeration, and the concentration of particulate micropollutants in the feed.
2. Mathematical Analysis
2.1. Theoretical Analysis
2.1.1. Steady States and Its Stability
- The proposed model has two steady states. The first one is the washout branch (), when . The washout branch is given byThe second one is the no-washout branch (), when The no-washout branch is given by:whereNow, we present the local stability (LS) of the steady states. Consider the Jacobian matrix as:
- For the LS of the washout solution branch, consider the eigenvalues of at the washout branch as:Since The eigenvalue whenIt follows that whenOtherwise, for , the WSS is LS if is enough low:
- For an LS of no-washout solution branch, from the second equation of model (8), we haveFor the no-washout branch soFor the above equation, the characteristic polynomail of is as follows:whereThe no-washout solution is of interest when all solution are positive. So, in these cases, the coefficients of are positive. From the above equations, we see that , , and To prove that considerThus so no-washout solution branch is LS.
2.1.2. Applications of Fixed Point Theory to the Existence of Solution
2.1.3. Ulam–Hyers Stability
- for
- for
2.2. Computational Analysis
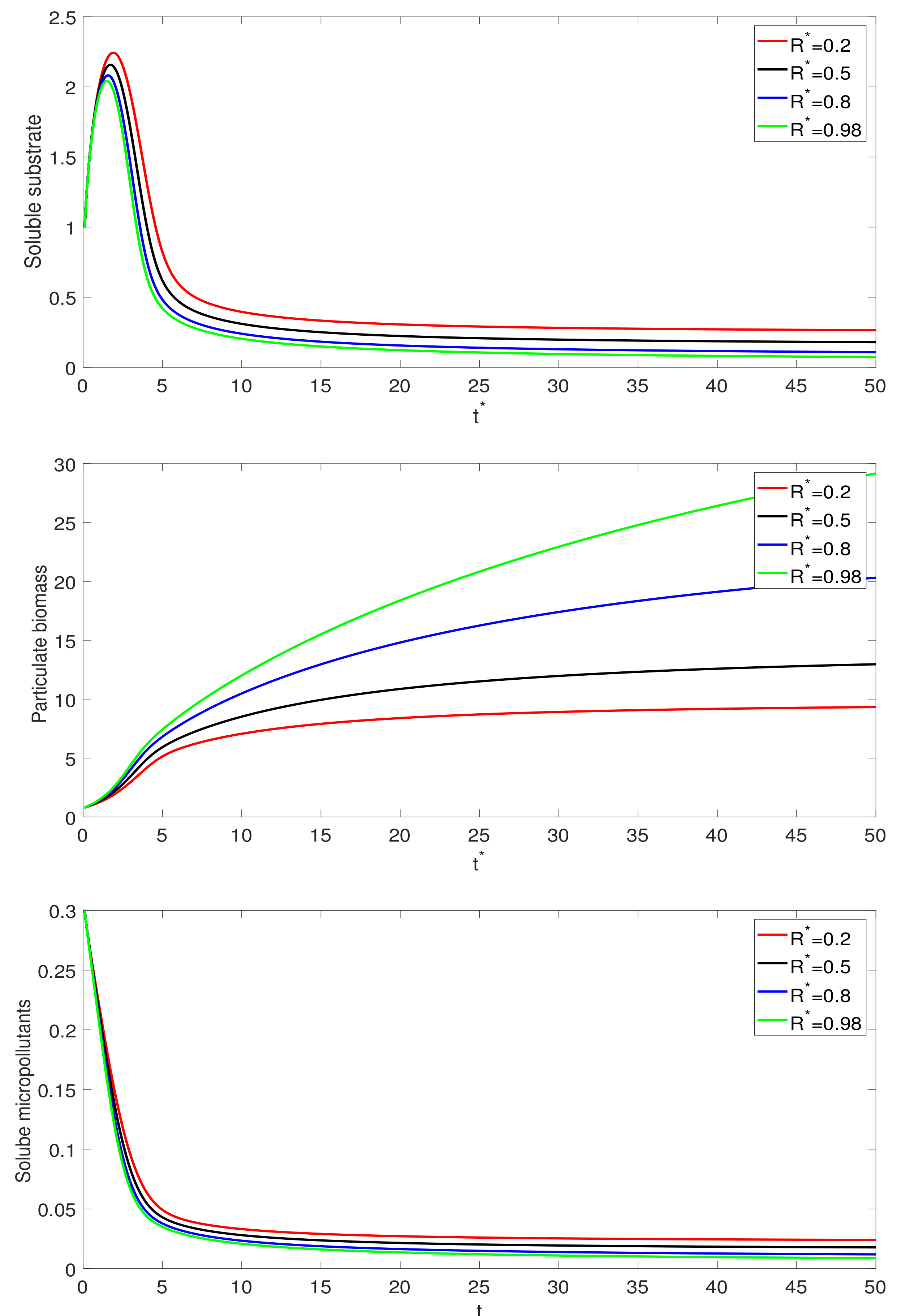
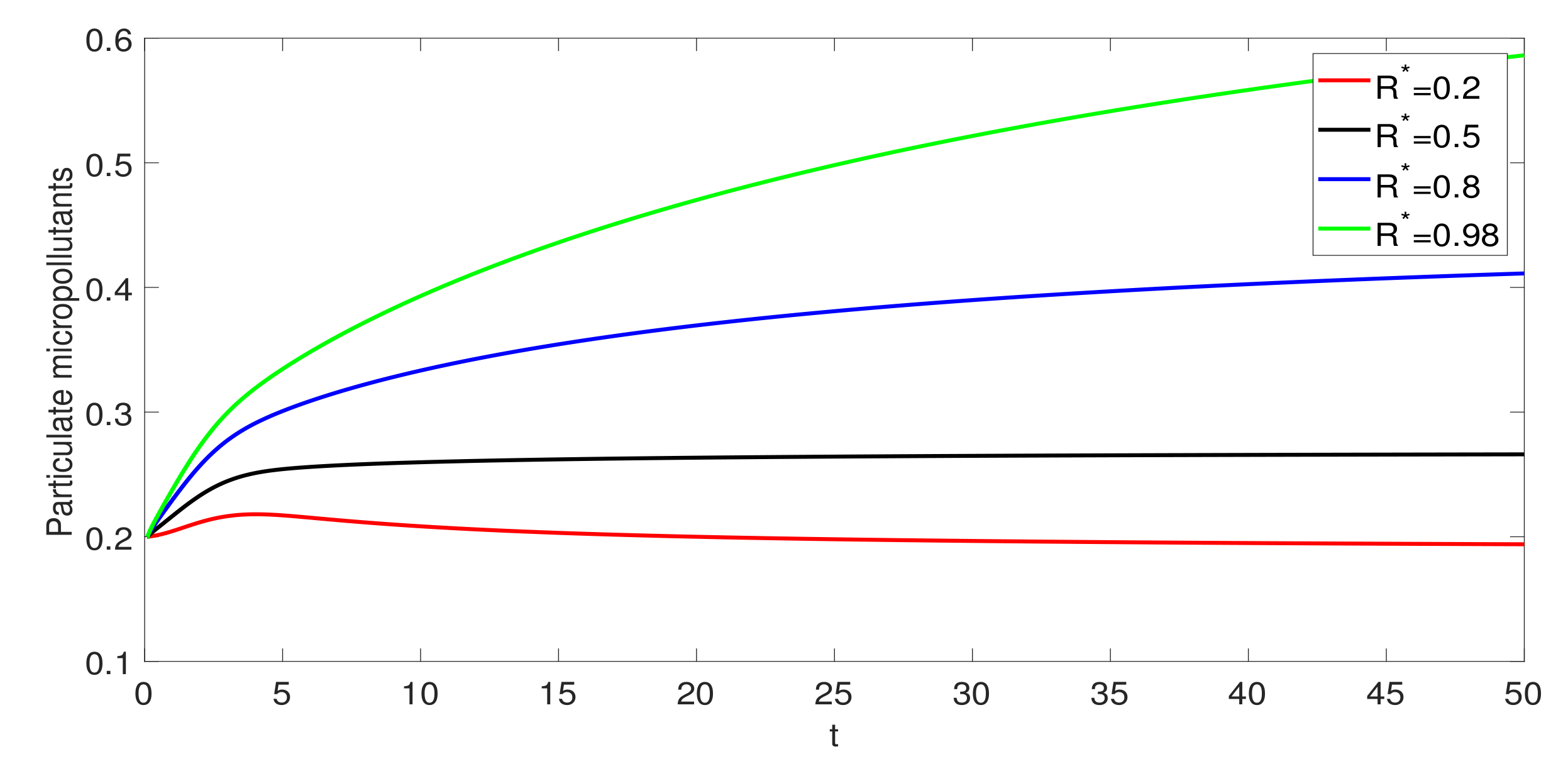
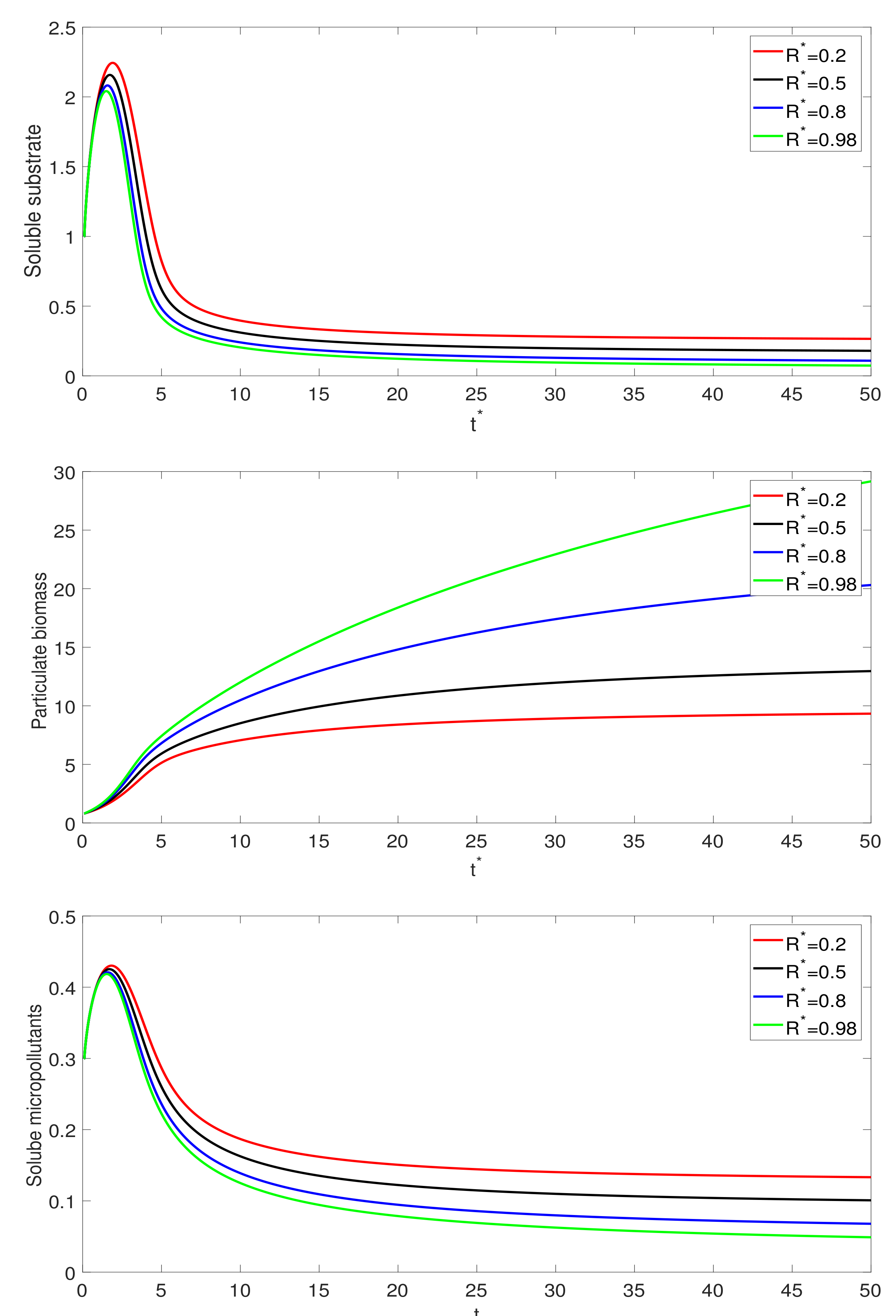
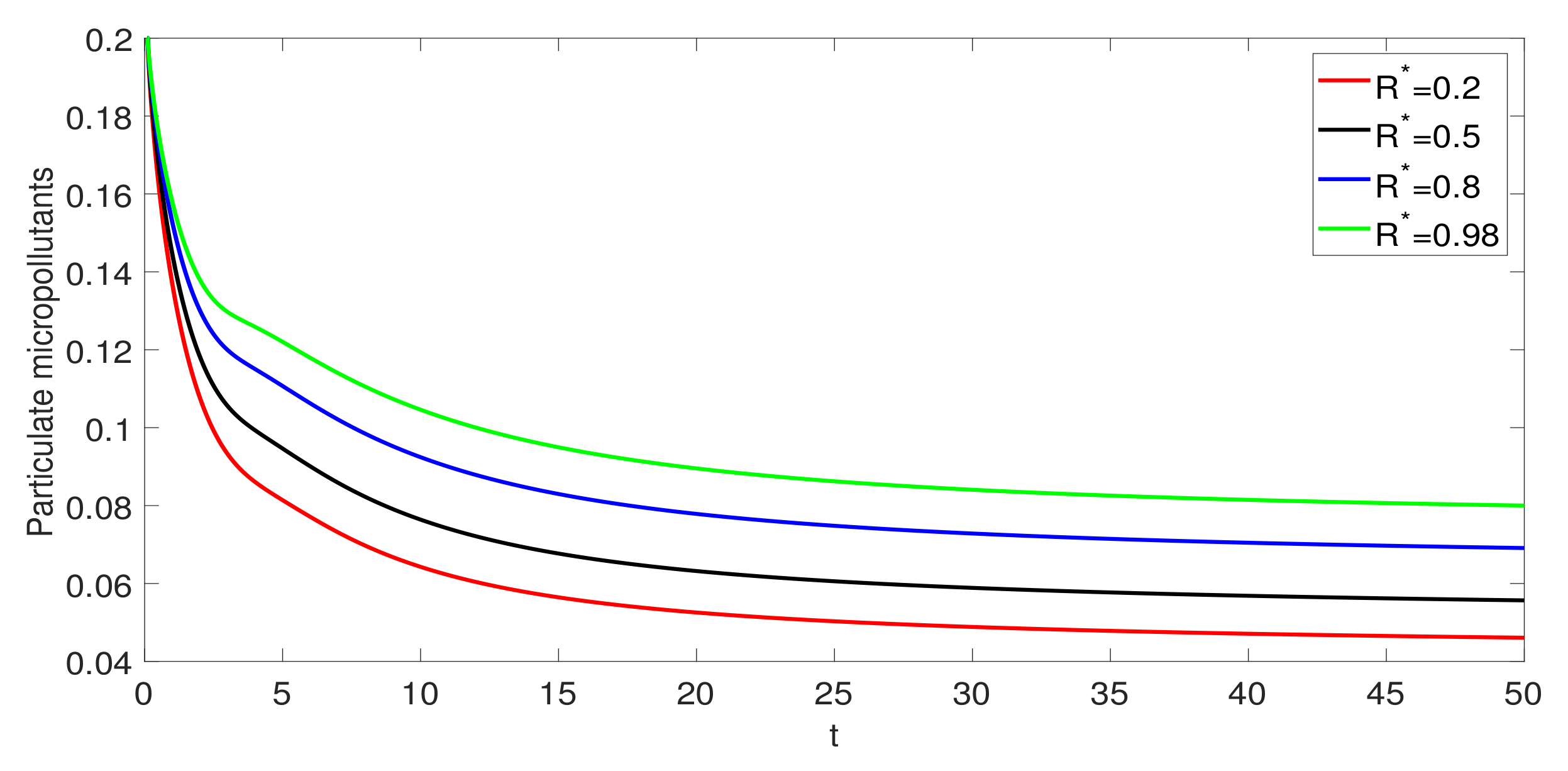
3. Numerical Simulations
4. Conclusions
- Using more generalized nonolocal operators with different kernels.
- Involving fuzziness and uncertainty in the model.
- Controllability and chaotic behaviour of the model.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hai, F.I.; Nghiem, L.D.; Khan, S.J.; Yamamoto, W.E.P.K. Wastewater Reuse: Removal of Emerging Trace Organic Contaminants in: Membrane Biological Reactors: Theory, Modeling, Design, Management and Applications to Wastewater Reuse; Hai, F.I., Yamamoto, K., Lee, C., Eds.; IWA: London, UK, 2014; pp. 165–205. [Google Scholar]
- Luo, Y.; Guo, W.; Ngo, H.H.; Nghiem, L.D.; Hai, F.I.; Zhang, J.; Liang, S.; Wang, X.C. A review on the occurrence of micropollutants in the aquatic environment and their fate and removal during wastewater treatment. Sci. Total Environ. 2014, 473–474, 619–641. [Google Scholar] [CrossRef] [PubMed]
- Pomies, M.; Choubert, J.-M.; Wisniewski, C.; Coquery, M. Modelling of micropollutant removal in biological wastewater treatments: A review. Sci. Total Environ. 2013, 443, 733–748. [Google Scholar] [CrossRef] [PubMed]
- Delgadillo-Mirquez, L.; Lardon, L.; Steyer, J.-P.; Patureau, D. A new dynamic model for bioavailability and cometabolism of micropollutants during anaerobic digestion. Water Res. 2011, 45, 4511–4521. [Google Scholar] [CrossRef] [PubMed]
- Criddle, C.S. The kinetics of cometabolism. Biotechnol. Bioeng. 1993, 41, 1048–1056. [Google Scholar] [CrossRef]
- Li, B.; Stenstrom, M.K. A sensitivity and model reduction analysis of one-dimensional secondary settling tank models under wet-weather flow and sludge bulking conditions. Chem. Eng. J. 2016, 288, 813–823. [Google Scholar] [CrossRef] [Green Version]
- Torfs, E.; Maere, T.; Burger, R.; Diehl, S.; Nopens, I. Impact on sludge inventory and control strategies using the benchmark simulation model no. 1 with the Burger–Diehl settler model. Water Sci. Technol. 2015, 71, 1524–1535. [Google Scholar] [CrossRef] [Green Version]
- Torfs, E.; Mart, M.C.; Locatelli, F.; Balemans, S.; Burger, R.; Diehl, S.; Laurent, J.; Vanrolleghem, P.; Francois, P.; Nopens, I. Concentration-driven models revisited: Towards a unified framework to model settling tanks in water resource recovery facilities. Water Sci. Technol. 2017, 75, 539–551. [Google Scholar] [CrossRef] [Green Version]
- Xu, G.; Yin, F.; Xu, Y.; Yu, H.-Q. A force-based mechanistic model for describing activated sludge settling process. Water Res. 2017, 127, 118–126. [Google Scholar] [CrossRef]
- Nelson, M.; Alqahtani, R.; Hai, F. Mathematical modelling of the removal of organic micropollutants in the activated sludge process: A linear biodegradation model. Anziam J. 2018, 60, 191–229. [Google Scholar] [CrossRef] [Green Version]
- Ahmad, S.; Ullah, A.; Akgül, A.; la Sen, M.D. A Novel Homotopy Perturbation Method with Applications to Nonlinear Fractional Order KdV and Burger Equation with Exponential-Decay Kernel. J. Funct. Spaces 2021, 2021, 8770488. [Google Scholar]
- Ullah, A.; Abdeljawad, T.; Ahmad, S.; Shah, K. Study of a fractional-order epidemic model of childhood diseases. J. Funct. Spaces 2020, 2020, 5895310. [Google Scholar] [CrossRef]
- Kilbas, A.; Srivastava, H.H.; Trujillo, J.J. Theory and Applications of Fractional Differential Equations; Elsevier: Amsterdam, The Netherlands, 2006. [Google Scholar]
- Caputo, M.; Fabrizio, M. A new definition of fractional derivative without singular kernel. Prog. Fract. Differ. Appl. 2015, 2015, 1–13. [Google Scholar]
- Ahmad, S.; Ullah, A.; Akgül, A.; DelaSen, M.A. study of fractional order Ambartsumian equation involving exponential decay kernel. AIMS Math. 2021, 6, 9981–9997. [Google Scholar] [CrossRef]
- Atangana, A.; Baleanu, D. New fractional derivatives with non-local and non-singular kernel: Theory and application to heat transfer model. Therm. Sci. 2016, 20, 763–769. [Google Scholar] [CrossRef] [Green Version]
- Ahmad, S.; Ullah, A.; Arfan, M.; Shah, K. On analysis of the fractional mathematical model of rotavirus epidemic with the effects of breastfeeding and vaccination under Atangana-Baleanu (AB) derivative. Chaos Solitons Fractals 2020, 140, 110233. [Google Scholar] [CrossRef]
- Ikram, M.D.; Asjad, M.I.; Akgül, A.; Baleanu, D. Effects of hybrid nanofluid on novel fractional model of heat transfer flow between two parallel plates. Alex. Eng. J. 2021, 60, 3593–3604. [Google Scholar] [CrossRef]
- Wongcharoen, A.; Ntouyas, S.K.; Tariboon, J. Boundary Value Problems for Hilfer Fractional Differential Inclusions with Nonlocal Integral Boundary Conditions. Mathematics 2020, 8, 1905. [Google Scholar] [CrossRef]
- Abdo, M.S.; Saeed, A.M.; Panchal, S.K. Panchal, Existence and Ulam–Hyers–Mittag–Leffler stability results of Ψ-Hilfer nonlocal Cauchy problem. Rend. Circ. Mat. 2021, 2, 57–77. [Google Scholar]
- Liu, K.; Feckan, M.; Wang, J. Hyers–Ulam Stability and Existence of Solutions to the Generalized Liouville–Caputo Fractional Differential Equations. Symmetry 2020, 12, 955. [Google Scholar] [CrossRef]
- Ahmed, I.; Kumam, P.; Abubakar, J.; Borisut, P.; Sitthithakerngkiet, K. Solutions for impulsive fractional pantograph differential equation via generalized anti-periodic boundary condition. Adv. Diff. Equ. 2020, 2020, 1–15. [Google Scholar]
- Hinze, M.; Schmidt, A.; Leine, R.I. Numerical solution of fractional-order ordinary differential equations using the reformulated infinite state representation. Fract. Calc. Appl. Anal. 2019, 22, 1321–1350. [Google Scholar] [CrossRef]
- Alqahtani, R.T.; Ahmad, S.; Akgül, A. Dynamical Analysis of Bio-Ethanol Production Model under Generalized Nonlocal Operator in Caputo Sense. Mathematics 2021, 9, 2370. [Google Scholar] [CrossRef]
- Qureshi, S.; Yusuf, A.; Shaikh, A.A.; Inc, M.; Baleanu, D. Fractional modeling of blood ethanol concentration system with real data application. Chaos 2019, 29, 013143. [Google Scholar] [CrossRef]
- Panjwani, S.; Tejaswini, E.S.S.; Rao, A.S. Fractional Order Model-Based Design of Controllers for Improved Operation of Wastewater Treatment Plants. Trans. Indian Natl. Acad. Eng. 2020, 5, 719–726. [Google Scholar] [CrossRef]
- Barbu, M.; Ceanga, E. Fractional order controllers for urban wastewater treatment systems. In Proceedings of the 23rd Mediterranean Conference on Control and Automation (MED), Malaga, Spain, 16–19 June 2015; pp. 1174–1179. [Google Scholar]
- El-Seddik, M.M. Modified Fractional-Order Activated Sludge Model (MFASM) for Aerobic Microbial Growth in Wastewater. Inorg. Chem. Ind. J. 2017, 12, 1–8. [Google Scholar]
- Sidorov, N.; Sidorov, D.; Sinitysn, A. Toward General Theory of Differential-Operator and Kinetic Models; World Scientific Series on Nonlinear Science Series A; Chua, L., Ed.; World Scientific: Singapore; London, UK, 2020. [Google Scholar]
- Vainberg, M.M.; Trenogin, V.A. Theory of Branching of Solutions of Non-linear Equations, Noordhoff. 1974. Available online: https://www.abebooks.com/Theory-Branching-Solutions-Nonlinear-Equations-Monographs/22683271746/bd (accessed on 2 October 2021).
- Trenogin, V.A.; Sidorov, N.A. An investigation of the bifurcation points and nontrivial branches of the solutions of nonlinear equations. Differ. Integral Equ. 1972, 1, 216–247. [Google Scholar]
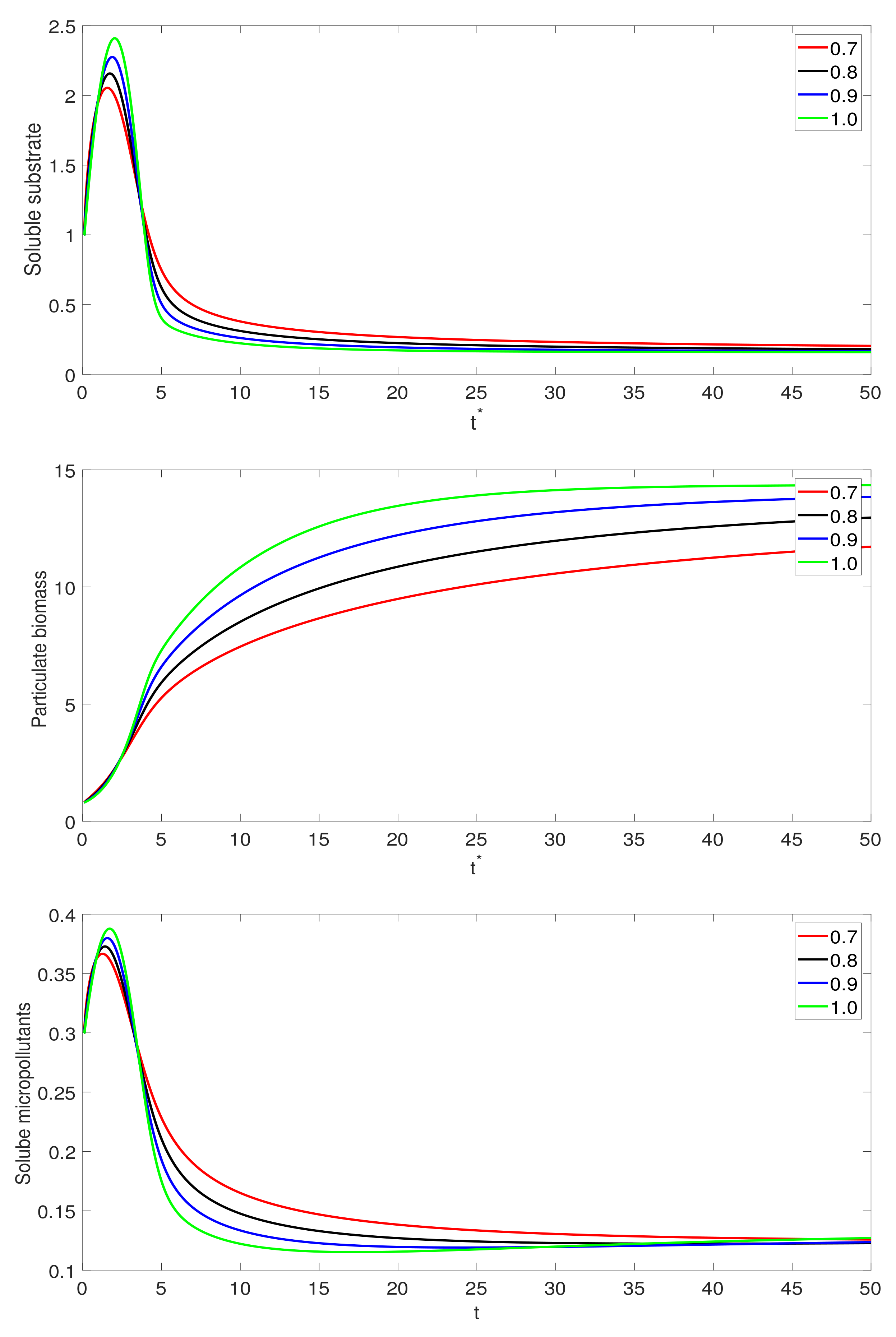
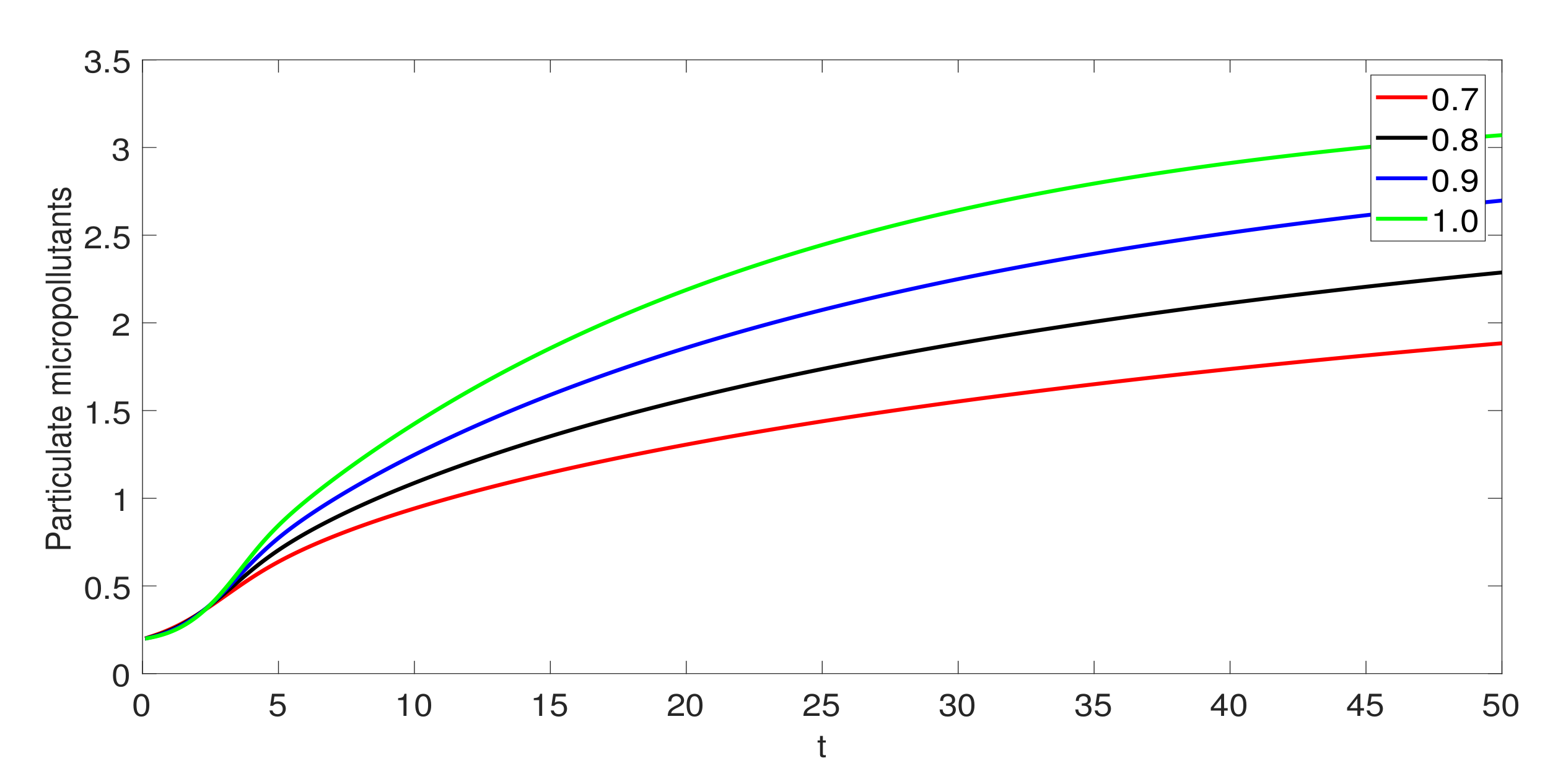
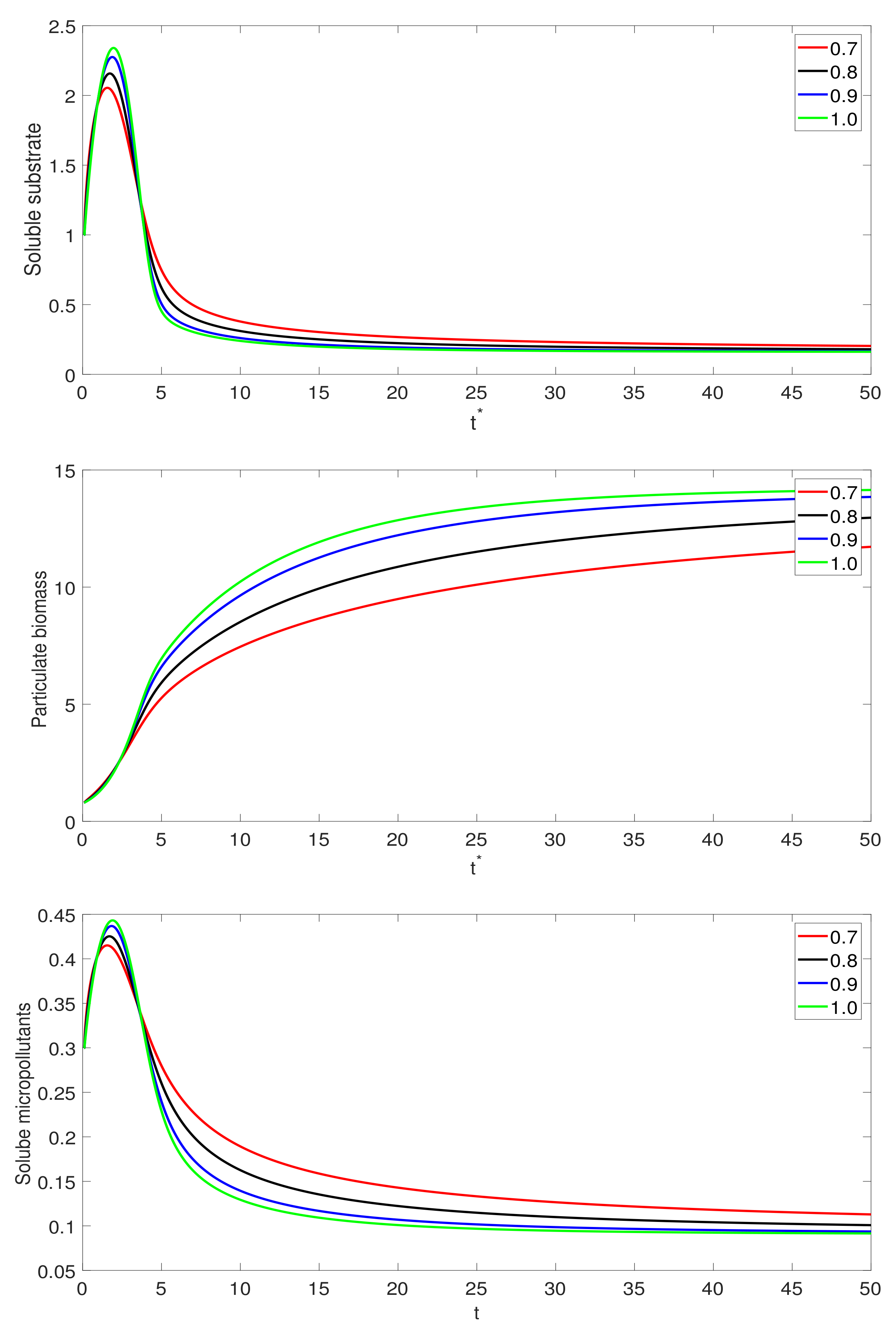
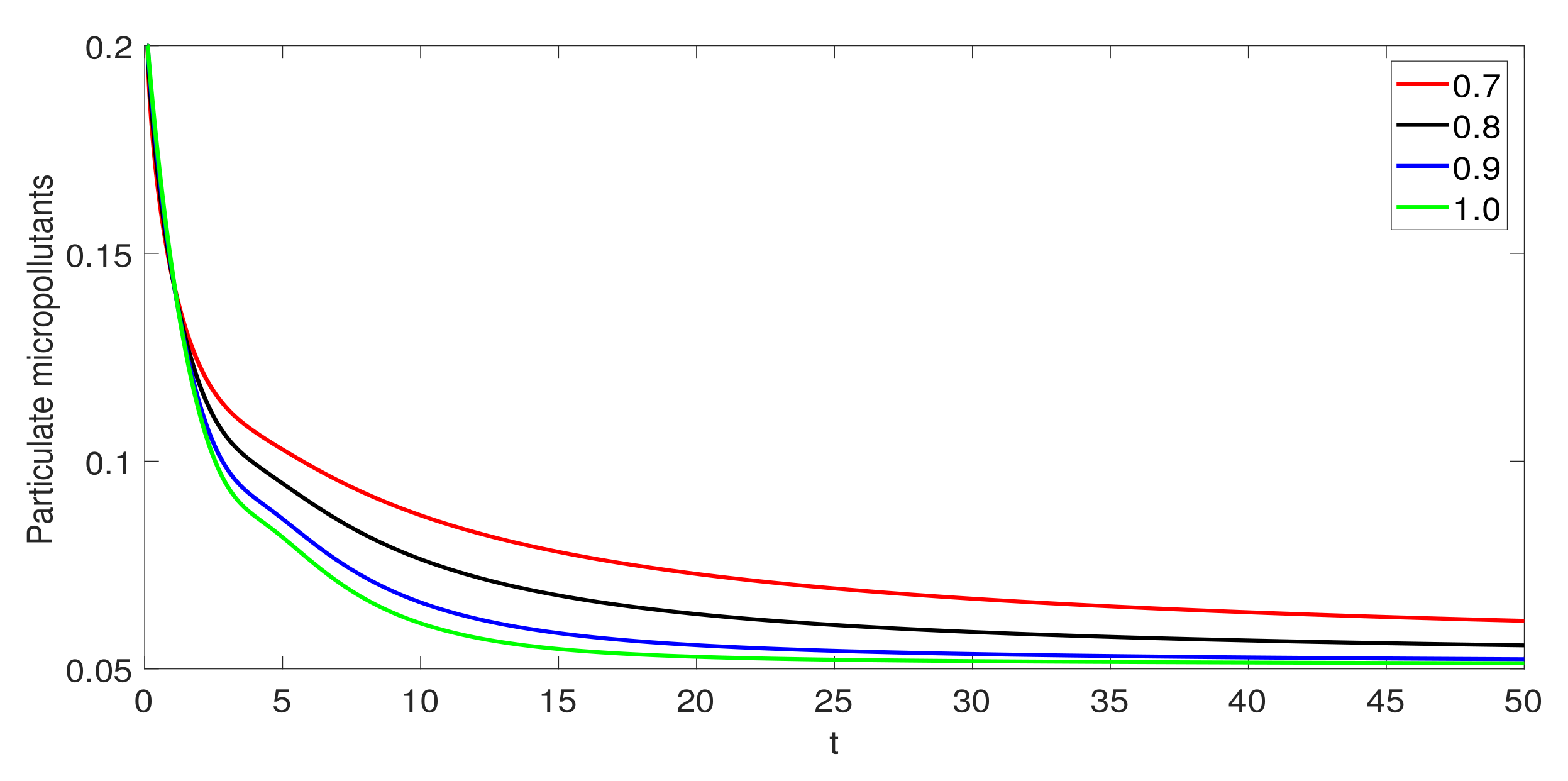
| Compounds | |||
|---|---|---|---|
| Highly biodegradable | |||
| Erythromycin (ERY) | |||
| Ibuprofen (IBP) | |||
| Roxithromycin(ROX) | |||
| Naproxen(NPX) | |||
| Slowly biodegradable | |||
| Trimethoprim(TMP) | |||
| Sulfamethoxazole(SMX) | |||
| Fluoxetine(FLX) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alqahtani, R.T.; Ahmad, S.; Akgül, A. Mathematical Analysis of Biodegradation Model under Nonlocal Operator in Caputo Sense. Mathematics 2021, 9, 2787. https://doi.org/10.3390/math9212787
Alqahtani RT, Ahmad S, Akgül A. Mathematical Analysis of Biodegradation Model under Nonlocal Operator in Caputo Sense. Mathematics. 2021; 9(21):2787. https://doi.org/10.3390/math9212787
Chicago/Turabian StyleAlqahtani, Rubayyi T., Shabir Ahmad, and Ali Akgül. 2021. "Mathematical Analysis of Biodegradation Model under Nonlocal Operator in Caputo Sense" Mathematics 9, no. 21: 2787. https://doi.org/10.3390/math9212787
APA StyleAlqahtani, R. T., Ahmad, S., & Akgül, A. (2021). Mathematical Analysis of Biodegradation Model under Nonlocal Operator in Caputo Sense. Mathematics, 9(21), 2787. https://doi.org/10.3390/math9212787








