An Efficient Discrete Model to Approximate the Solutions of a Nonlinear Double-Fractional Two-Component Gross–Pitaevskii-Type System
Abstract
:1. Introduction
2. Numerical Algorithm
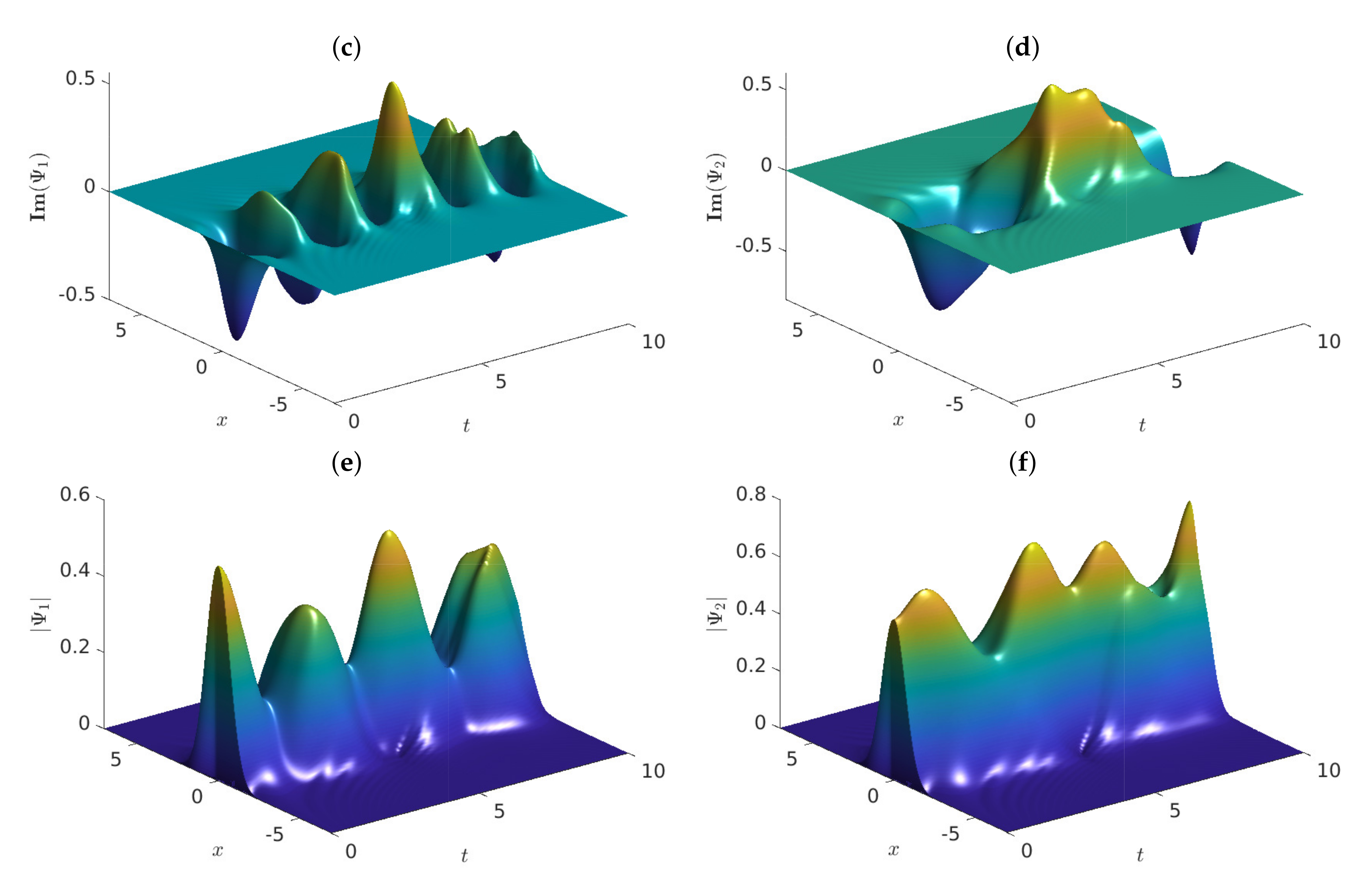
3. Computational Properties
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Podlubny, I. Fractional Differential Equations: An Introduction to Fractional Derivatives, Fractional Differential Equations, to Methods of Their Solution and Some of Their Applications; Elsevier: Amsterdam, The Netherlands, 1998; Volume 198. [Google Scholar]
- Saeed, A.; Bilal, M.; Gul, T.; Kumam, P.; Khan, A.; Sohail, M.M. Fractional Order Stagnation Point Flow of the Hybrid Nanofluid towards a Stretching Sheet. Sci. Rep. 2021, 11, 20429. [Google Scholar] [CrossRef] [PubMed]
- Gul, T.; Alghamdi, W.; Khan, I.; Ali, I. New similarity variable to transform the fluid flow from PDEs into fractional-order ODEs: Numerical study. Phys. Scr. 2021, 96, 084009. [Google Scholar] [CrossRef]
- Serna-Reyes, A.J.; Macías-Díaz, J. Theoretical analysis of a conservative finite-difference scheme to solve a Riesz space-fractional Gross–Pitaevskii system. J. Comput. Appl. Math. 2021, 113413. [Google Scholar] [CrossRef]
- Macías-Díaz, J. An explicit dissipation-preserving method for Riesz space-fractional nonlinear wave equations in multiple dimensions. Commun. Nonlinear Sci. Numer. Simul. 2018, 59, 67–87. [Google Scholar] [CrossRef]
- Wang, P.; Huang, C. An energy conservative difference scheme for the nonlinear fractional Schrödinger equations. J. Comput. Phys. 2015, 293, 238–251. [Google Scholar] [CrossRef]
- Xing, Z.; Wen, L. A conservative difference scheme for the Riesz space-fractional sine-Gordon equation. Adv. Differ. Equ. 2018, 2018, 238. [Google Scholar] [CrossRef] [Green Version]
- Ying, Y.; Lian, Y.; Tang, S.; Liu, W.K. High-order central difference scheme for Caputo fractional derivative. Comput. Methods Appl. Mech. Eng. 2017, 317, 42–54. [Google Scholar] [CrossRef]
- Cen, Z.; Huang, J.; Xu, A.; Le, A. A modified integral discretization scheme for a two-point boundary value problem with a Caputo fractional derivative. J. Comput. Appl. Math. 2020, 367, 112465. [Google Scholar] [CrossRef]
- Bhalekar, S.; Daftardar-Gejji, V. A predictor-corrector scheme for solving nonlinear delay differential equations of fractional order. J. Fract. Calc. Appl. 2011, 1, 1–9. [Google Scholar]
- Mendes, E.M.; Salgado, G.H.; Aguirre, L.A. Numerical solution of Caputo fractional differential equations with infinity memory effect at initial condition. Commun. Nonlinear Sci. Numer. Simul. 2019, 69, 237–247. [Google Scholar] [CrossRef]
- Yan, Y.; Sun, Z.Z.; Zhang, J. Fast evaluation of the Caputo fractional derivative and its applications to fractional diffusion equations: A second-order scheme. Commun. Comput. Phys. 2017, 22, 1028–1048. [Google Scholar] [CrossRef]
- Das, P.; Rana, S.; Ramos, H. Homotopy perturbation method for solving Caputo-type fractional-order Volterra–Fredholm integro-differential equations. Comput. Math. Methods 2019, 1, e1047. [Google Scholar] [CrossRef] [Green Version]
- Strauss, W.; Vazquez, L. Numerical solution of a nonlinear Klein-Gordon equation. J. Comput. Phys. 1978, 28, 271–278. [Google Scholar] [CrossRef]
- Ben-Yu, G.; Pascual, P.J.; Rodriguez, M.J.; Vázquez, L. Numerical solution of the sine-Gordon equation. Appl. Math. Comput. 1986, 18, 1–14. [Google Scholar] [CrossRef]
- Fei, Z.; Vázquez, L. Two energy conserving numerical schemes for the sine-Gordon equation. Appl. Math. Comput. 1991, 45, 17–30. [Google Scholar] [CrossRef]
- Tang, Y.F.; Vázquez, L.; Zhang, F.; Pérez-García, V. Symplectic methods for the nonlinear Schrödinger equation. Comput. Math. Appl. 1996, 32, 73–83. [Google Scholar] [CrossRef] [Green Version]
- Furihata, D. Fast and structure-preserving schemes for PDEs based on discrete variational derivative method. Geom. Numer. Integr. Its Appl. 2017, 74, 19–22. [Google Scholar]
- Furihata, D.; Matsuo, T. Discrete Variational Derivative Method: A Structure-Preserving Numerical Method for Partial Differential Equations; CRC Press: New York, NY, USA, 2010. [Google Scholar]
- Matsuo, T. Dissipative/conservative Galerkin method using discrete partial derivatives for nonlinear evolution equations. J. Comput. Appl. Math. 2008, 218, 506–521. [Google Scholar] [CrossRef]
- Li, X.G.; Li, S.C. High-order numerical methods with mass and energy conservation for spin–orbit-coupled Bose–Einstein condensates. Int. J. Comput. Math. 2021, 98, 738–757. [Google Scholar] [CrossRef]
- Zhai, L.; Wang, J. High-order conservative scheme for the coupled space fractional nonlinear Schrödinger equations. Int. J. Comput. Math. 2021, 1–22. [Google Scholar] [CrossRef]
- Fu, Y.; Shi, Y.; Zhao, Y. Explicit high-order structure-preserving algorithms for the two-dimensional fractional nonlinear Schrödinger equation. Int. J. Comput. Math. 2021, 1–28. [Google Scholar] [CrossRef]
- Macías-Díaz, J.E. On the solution of a Riesz space-fractional nonlinear wave equation through an efficient and energy-invariant scheme. Int. J. Comput. Math. 2019, 96, 337–361. [Google Scholar] [CrossRef]
- Serna-Reyes, A.J.; Macías-Díaz, J.E.; Reguera, N. A Convergent Three-Step Numerical Method to Solve a Double-Fractional Two-Component Bose–Einstein Condensate. Mathematics 2021, 9, 1412. [Google Scholar] [CrossRef]
- Ertik, H.; Şirin, H.; Demirhan, D.; Büyükkiliç, F. Fractional mathematical investigation of Bose–Einstein condensation in dilute 87Rb, 23Na and 7Li atomic gases. Int. J. Mod. Phys. B 2012, 26, 1250096. [Google Scholar] [CrossRef]
- Perez-Garcia, V.M.; Michinel, H.; Cirac, J.; Lewenstein, M.; Zoller, P. Dynamics of Bose-Einstein condensates: Variational solutions of the Gross–Pitaevskii equations. Phys. Rev. A 1997, 56, 1424. [Google Scholar] [CrossRef]
- Ortigueira, M.D. Riesz potential operators and inverses via fractional centred derivatives. Int. J. Math. Math. Sci. 2006, 2006, 048391. [Google Scholar] [CrossRef]
- Wang, X.; Liu, F.; Chen, X. Novel second-order accurate implicit numerical methods for the Riesz space distributed-order advection-dispersion equations. Adv. Math. Phys. 2015, 2015, 590435. [Google Scholar] [CrossRef]
- Desplanques, J. Théoreme d’algébre. J. Math. Spec. 1887, 9, 12–13. [Google Scholar]
- Pen-Yu, K. Numerical methods for incompressible viscous flow. Sci. Sin. 1977, 20, 287–304. [Google Scholar]
- Mickens, R.E. Nonstandard Finite Difference Schemes: Methodology And Applications, 1st ed.; World Scientific: New York, NY, USA, 2020. [Google Scholar]
- Xiao, A.; Wang, C.; Wang, J. Conservative linearly-implicit difference scheme for a class of modified Zakharov systems with high-order space fractional quantum correction. Appl. Numer. Math. 2019, 146, 379–399. [Google Scholar] [CrossRef]
- Wang, Y.; Li, Q.; Mei, L. A linear, symmetric and energy-conservative scheme for the space-fractional Klein–Gordon–Schrödinger equations. Appl. Math. Lett. 2019, 95, 104–113. [Google Scholar] [CrossRef]



| (a) Time | ||||||
|---|---|---|---|---|---|---|
| − | − | − | ||||
| (b) Space | ||||||
| − | − | − | ||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Macías-Díaz, J.E.; Reguera, N.; Serna-Reyes, A.J. An Efficient Discrete Model to Approximate the Solutions of a Nonlinear Double-Fractional Two-Component Gross–Pitaevskii-Type System. Mathematics 2021, 9, 2727. https://doi.org/10.3390/math9212727
Macías-Díaz JE, Reguera N, Serna-Reyes AJ. An Efficient Discrete Model to Approximate the Solutions of a Nonlinear Double-Fractional Two-Component Gross–Pitaevskii-Type System. Mathematics. 2021; 9(21):2727. https://doi.org/10.3390/math9212727
Chicago/Turabian StyleMacías-Díaz, Jorge E., Nuria Reguera, and Adán J. Serna-Reyes. 2021. "An Efficient Discrete Model to Approximate the Solutions of a Nonlinear Double-Fractional Two-Component Gross–Pitaevskii-Type System" Mathematics 9, no. 21: 2727. https://doi.org/10.3390/math9212727
APA StyleMacías-Díaz, J. E., Reguera, N., & Serna-Reyes, A. J. (2021). An Efficient Discrete Model to Approximate the Solutions of a Nonlinear Double-Fractional Two-Component Gross–Pitaevskii-Type System. Mathematics, 9(21), 2727. https://doi.org/10.3390/math9212727







