Modeling the Risk of Infectious Diseases Transmitted by Aedes aegypti Using Survival and Aging Statistical Analysis with a Case Study in Colombia
Abstract
:1. Introduction, Case Study, and Description
1.1. Introduction
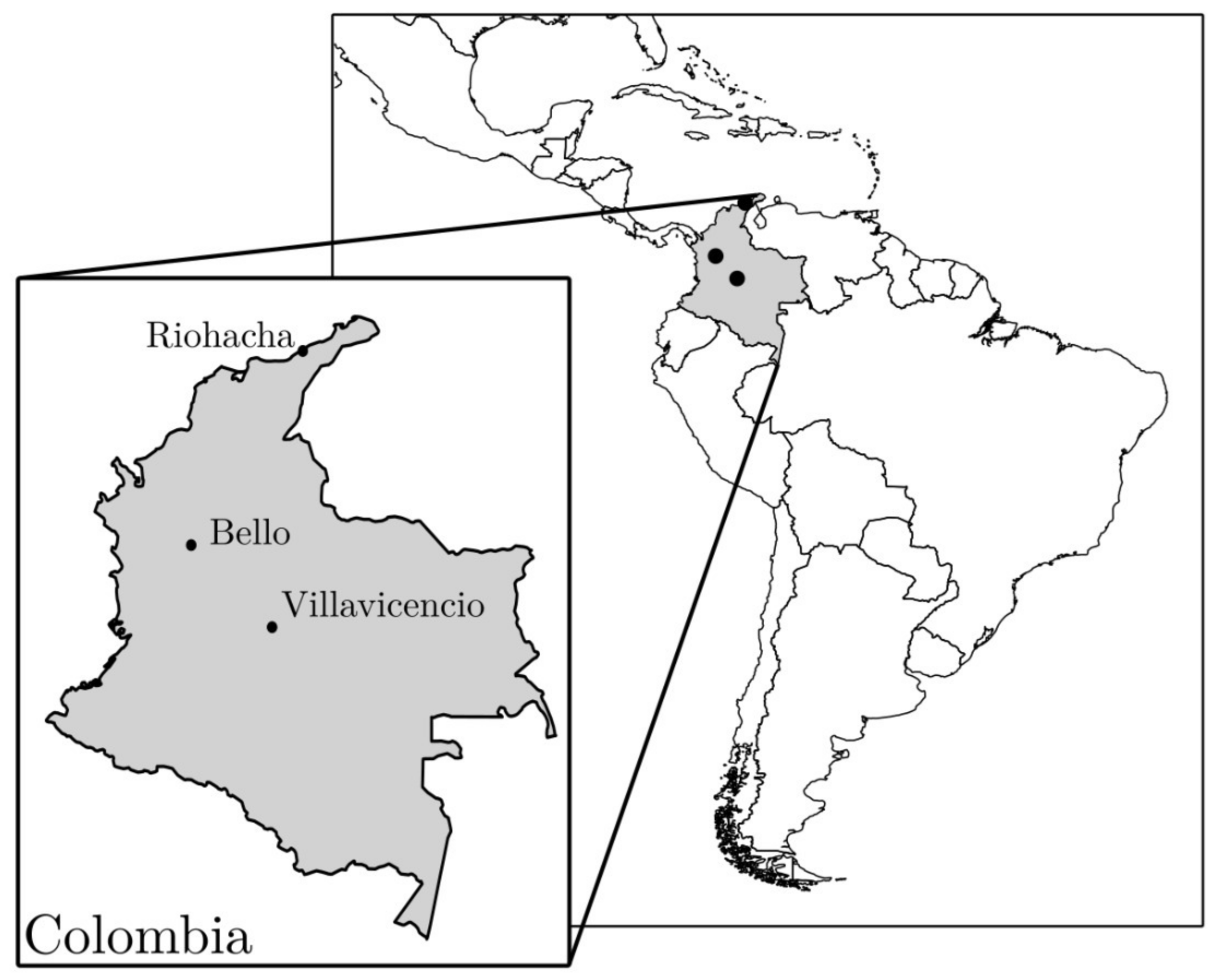
1.2. Definition of the Case Study
- (i)
- Bello city is located in the Andean Mountains (1310 m above mean sea level (m.a.s.l.)), with an average rainfall of 1542 mm and average minimum/maximum temperatures of 17 C/28 C, respectively.
- (ii)
- Riohacha is a city close to the Caribbean Sea (5 m.a.s.l.) and the Riohacha river, with an average rainfall of 546 mm and average minimum/maximum temperatures of 24 C/33 C, respectively.
- (iii)
- Villavicencio city is located in the Eastern Plains at 467 m.a.s.l., with an average rainfall of 4537 mm and average minimum/maximum temperatures of 21 C/30 C, respectively.
- (iv)
- For these three cities, the average humidities are very similar with a value of approximately 77%. In addition, Figure 1 indicates that each study zone is far from the other two zones and all of them present different geographic conditions.
1.3. Objective and Description of the Paper
2. Statistical Methods
2.1. Elements of Reliability Analysis
- (i)
- The survival function leads to the concept of usual stochastic (ST) order in which the random variable is less than the random variable , denoted by , if only if the survival function of is everywhere less than the survival function of .
- (ii)
- The HR function, also named failure rate function, leads to the concept of HR order in which is less than , denoted by , if and only if the HR function of is everywhere less than the HR function of .
- (iii)
- The RH rate function permits us to obtain the concept of RH order in which the random variable is less than the random variable , denoted by , if only if the RH rate function of is everywhere less than the RH rate function of .
- (iv)
- The function above defined leads to the concept of convex (CX) order in which is less than , denoted by , if and only if the function of is everywhere less than the function of and . Since is the mean residual life at time t, then implies that the mean residual life of is less than the mean residual life of .
2.2. Non-Parametric and Parametric Survival Analysis
2.3. Regression Analysis
2.4. Non-Parametric Test
3. Statistical Analysis of the Case Study
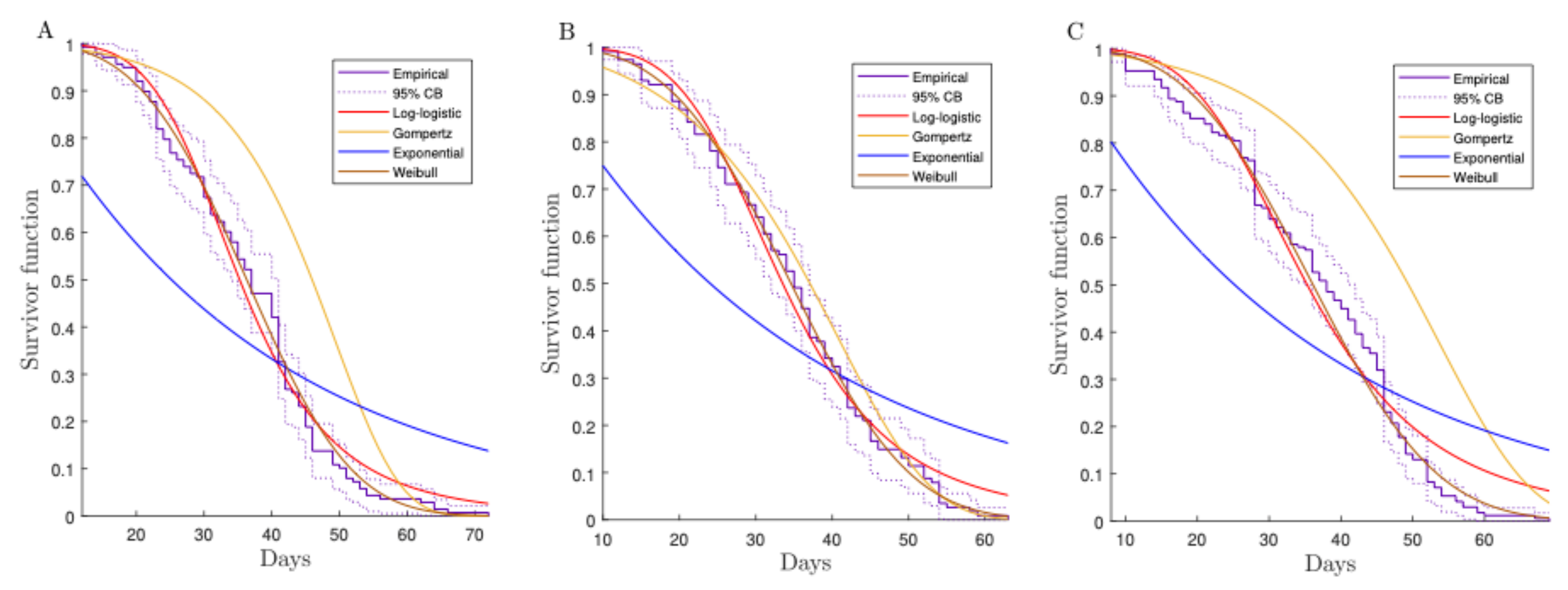
3.1. Survival Analysis of Aedes aegypti Females
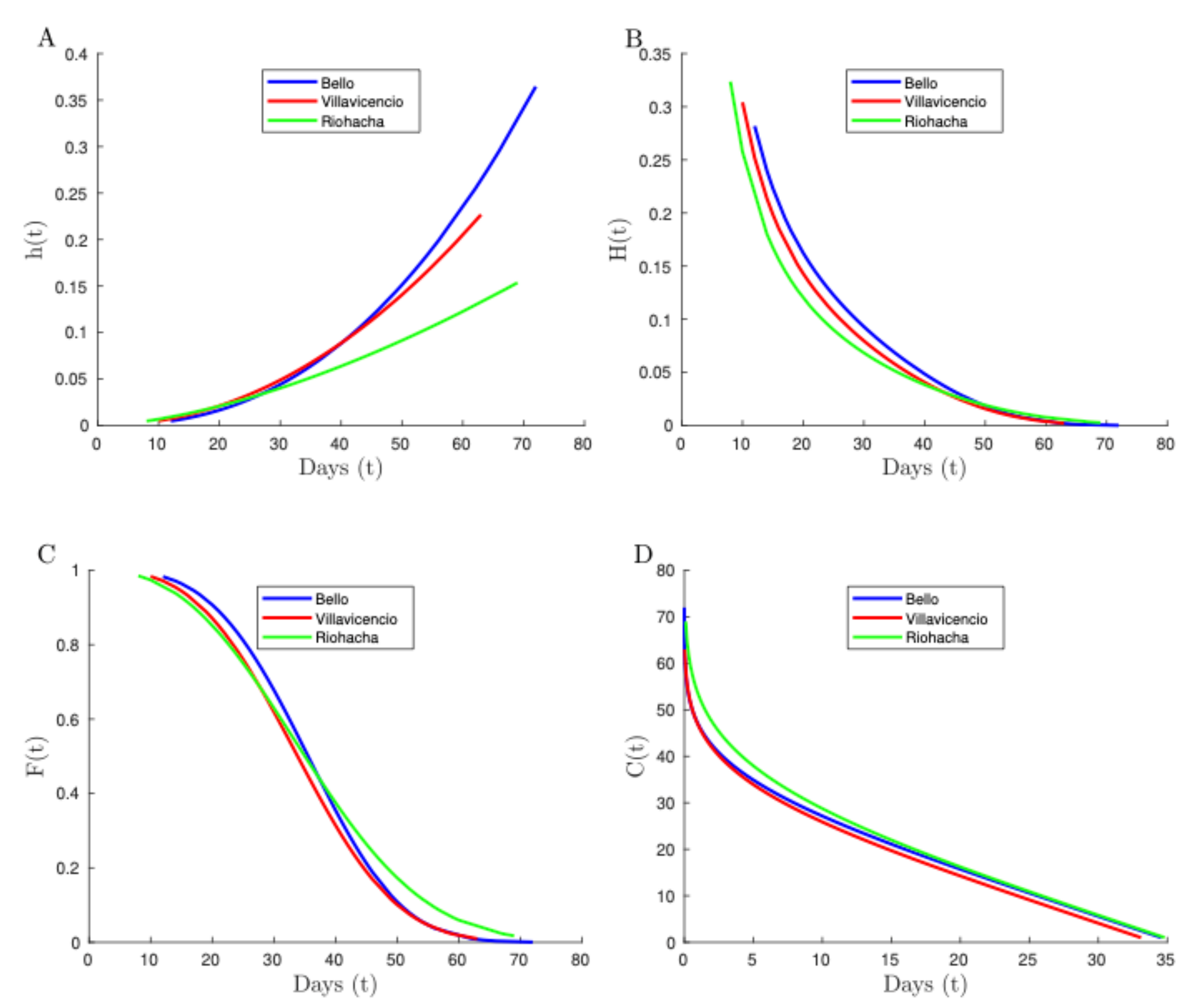
3.2. Aging Speed Analysis of Aedes aegypti Females
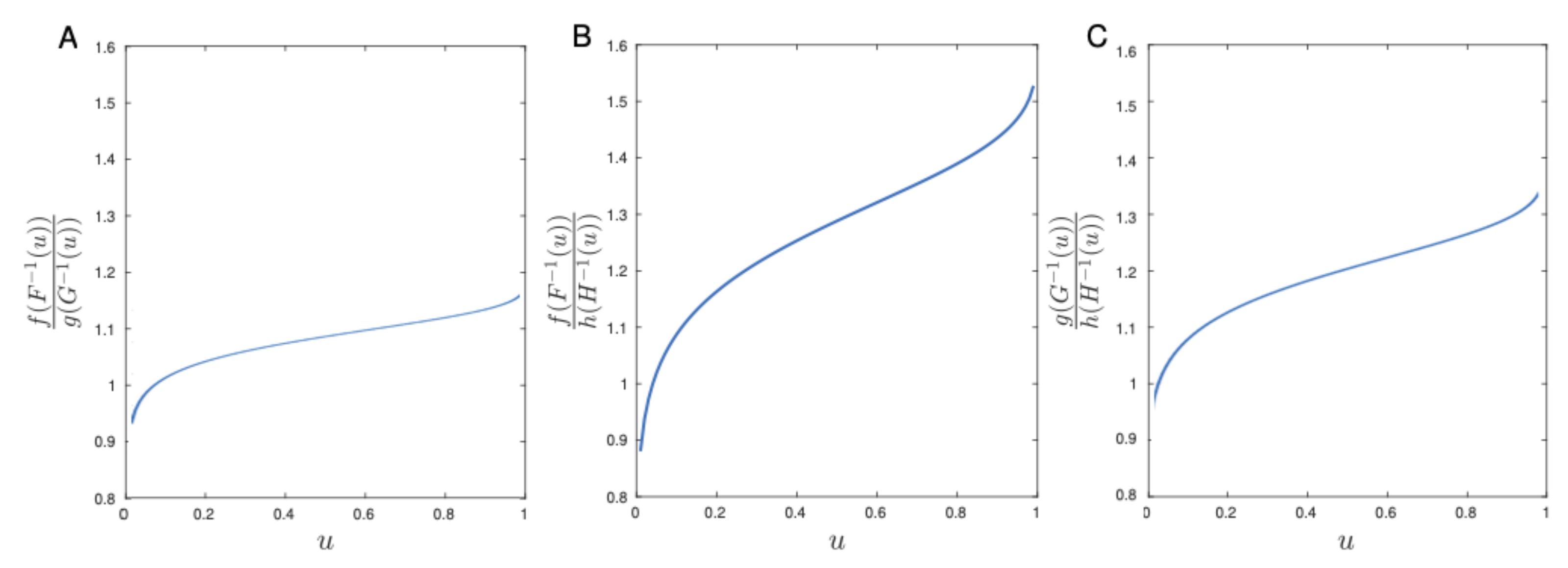
3.3. Survival and Aging Analysis of Aedes aegypti Females
4. Results, Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zafar, Z.U.A.; Ali, N.; Shah, Z.; Zaman, G.; Roy, P.; Deebani, W. Hopf bifurcation and global dynamics of time delayed dengue model. Comput. Methods Programs Biomed. 2020, 195, 105530. [Google Scholar] [CrossRef] [PubMed]
- Brady, O.J.; Gething, P.W.; Bhatt, S.; Messina, J.P.; Brownstein, J.S.; Hoen, A.G.; Hay, S.I. Refining the global spatial limits of dengue virus transmission by evidence-based consensus. PLoS Negl. Trop. Dis. 2012, 6, e1760. [Google Scholar] [CrossRef] [PubMed]
- Jentes, E.S.; Poumerol, G.; Gershman, M.D.; Hill, D.R.; Lemarch, J.; Lewis, R.F.; Monath, T.P. The revised global yellow fever risk map and recommendations for vaccination, 2010: Consensus of the informal who working group on geographic risk for yellow fever. Lancet Infect. Dis. 2011, 11, 622–632. [Google Scholar] [CrossRef]
- Otero, M.; Solari, H.G.; Schweigmann, N. A stochastic population dynamics model for Aedes Aegypti: Formulation and application to a city with temperate climate. Bull. Math. Biol. 2006, 68, 1945–1974. [Google Scholar] [CrossRef]
- Brady, O.J.; Johansson, M.A.; Guerra, C.A.; Bhatt, S.; Golding, N.; Pigott, D.M.; Hay, S.I. Modelling adult Aedes aegypti and Aedes albopictus survival at different temperatures in laboratory and field settings. Parasites Vectors 2013, 6, 351. [Google Scholar] [CrossRef] [Green Version]
- Garrett-Jones, C.; Shidrawi, G. Malaria vectorial capacity of a population of Anopheles Gambiae: An exercise in epidemiological entomology. Bull. World Health Organ. 1969, 40, 531. [Google Scholar]
- Styer, L.M.; Carey, J.R.; Wang, J.L.; Scott, T.W. Mosquitoes do senesce: Departure from the paradigm of constant mortality. Am. J. Trop. Med. Hyg. 2007, 76, 111–117. [Google Scholar] [CrossRef]
- Lunde, T.M.; Bayoh, M.N.; Lindtjørn, B. How malaria models relate temperature to malaria transmission. Parasites Vectors 2013, 6, 20. [Google Scholar] [CrossRef] [Green Version]
- Macdonald, G. Epidemiological basis of malaria control. Bull. World Health Organ. 1956, 15, 613. [Google Scholar]
- Reisen, W.K.; Mahmood, F.; Parveen, T. Anopheles culicifacies giles: A release-recapture experiment with cohorts of known age with implications for malaria epidemiology and genetical control in Pakistan. Trans. R. Soc. Trop. Med. Hyg. 1980, 74, 307–317. [Google Scholar] [CrossRef]
- Smith, D.L.; Battle, K.E.; Hay, S.I.; Barker, C.M.; Scott, T.W.; McKenzie, F.E. Ross, MacDonald, and a theory for the dynamics and control of mosquito-transmitted pathogens. PLoS Pathog. 2012, 8, e1002588. [Google Scholar] [CrossRef] [Green Version]
- Alto, B.W.; Bettinardi, D. Temperature and dengue virus infection in mosquitoes: Independent effects on the immature and adult stages. Am. J. Trop. Med. Hyg. 2013, 88, 497–505. [Google Scholar] [CrossRef]
- Huang, Y.J.S.; Higgs, S.; Vanlandingham, D.L. Arbovirus-mosquito vector-host interactions and the impact on transmission and disease pathogenesis of arboviruses. Front. Microbiol. 2019, 10, 22. [Google Scholar] [CrossRef]
- Yang, H.; Macoris, M.; Galvani, K.; Andrighetti, M.; Wanderley, D. Assessing the effects of temperature on the population of Aedes aegypti, the vector of dengue. Epidemiol. Infect. 2009, 137, 1188–1202. [Google Scholar] [CrossRef] [Green Version]
- Delatte, H.; Gimonneau, G.; Triboire, A.; Fontenille, D. Influence of temperature on immature development, survival, longevity, fecundity, and gonotrophic cycles of Aedes albopictus, vector of chikungunya and dengue in the Indian ocean. J. Med Entomol. 2009, 46, 33–41. [Google Scholar] [CrossRef]
- Degallier, N.; Servain, J.; Lucio, P.S.; Hannart, A.; Dur, B.; de Souza, R.N.; Mota, R.Z. The influence of local environment on the aging and mortality of Aedes aegypti: Case study in Fortaleza-CE, Brazil. J. Vector Ecol. 2012, 37, 428–441. [Google Scholar] [CrossRef]
- Harrington, L.C.; Jones, J.J.; Kitthawee, S.; Sithiprasasna, R.; Edman, J.D.; Scott, T.W. Age-dependent survival of the dengue vector Aedes aegypti (diptera: Culicidae) demonstrated by simultaneous release–recapture of different age cohorts. J. Med. Entomol. 2008, 45, 307–313. [Google Scholar]
- Maciel-de-Freitas, R.; Koella, J.C.; Lourenço-de-Oliveira, R. Lower survival rate, longevity and fecundity of Aedes aegypti (diptera: Culicidae) females orally challenged with dengue virus serotype 2. R. Soc. Trop. Med. Hyg. 2011, 105, 452–458. [Google Scholar] [CrossRef]
- Kochar, S.; Xu, M. Comparisons of parallel systems according to the convex transform order. J. Appl. Probab. 2009, 46, 342–352. [Google Scholar] [CrossRef] [Green Version]
- Harrington, L.C.; Buonaccorsi, J.P.; Edman, J.D.; Costero, A.; Kittayapong, P.; Clark, G.G.; Scott, T.W. Analysis of survival of young and old Aedes aegypti (diptera: Culicidae) from Puerto Rico and Thailand. J. Med. Entomol. 2001, 38, 537–547. [Google Scholar] [CrossRef] [Green Version]
- Muir, L.E.; Kay, B.H. Aedes aegypti survival and dispersal estimated by mark-release-recapture in northern Australia. Am. J. Trop. Med. Hyg. 1998, 58, 277–282. [Google Scholar] [CrossRef] [Green Version]
- David, M.R.; Lourenço-de-Oliveira, R.; Freitas, R.M.D. Container productivity, daily survival rates and dispersal of Aedes aegypti mosquitoes in a high income dengue epidemic neighbourhood of Rio de Janeiro: Presumed influence of differential urban structure on mosquito biology. Memórias Do Inst. Oswaldo Cruz 2009, 104, 927–932. [Google Scholar] [CrossRef] [Green Version]
- Tsuda, Y.; Takagi, M.; Wang, S.; Wang, Z.; Tang, L. Movement of Aedes aegypti (diptera: Culicidae) released in a small isolated village on Hainan Island, China. J. Med Entomol. 2001, 38, 93–98. [Google Scholar] [CrossRef]
- Lee, E.; Go, O. Survival analysis in public health research. Annu. Rev. Public Health 1997, 18, 105–134. [Google Scholar] [CrossRef] [Green Version]
- Catano-Lopez, A.; Rojas-Diaz, D.; Laniado, H.; Arboleda-Sánchez, S.; Puerta-Yepes, M.E.; Lizarralde-Bejarano, D.P. An alternative model to explain the vectorial capacity using as example Aedes aegypti case in dengue transmission. Heliyon 2019, 5, e02577. [Google Scholar] [CrossRef] [Green Version]
- Wilson, D.L. The analysis of survival (mortality) data: Fitting Gompertz, Weibull, and logistic functions. Mech. Aging Dev. 1994, 74, 15–33. [Google Scholar] [CrossRef]
- Styer, L.M.; Minnick, S.L.; Sun, A.K.; Scott, T.W. Mortality and reproductive dynamics of Aedes aegypti (diptera: Culicidae) fed human blood. Vector-Borne Zoonotic Dis. 2007, 7, 86–98. [Google Scholar] [CrossRef] [PubMed]
- Yang, H.M.; Macoris, M.; Galvani, K.C.; Andrighetti, M. Follow up estimation of Aedes aegypti entomological parameters and mathematical modelling. BioSystems 2011, 103, 360–371. [Google Scholar] [CrossRef] [Green Version]
- Takahashi, L.T.; Maidana, N.A.; Ferreira, W.C.; Pulino, P.; Yang, H.M. Mathematical models for the Aedes aegypti dispersal dynamics: Travelling waves by wing and wind. Bull. Math. Biol. 2005, 67, 509–528. [Google Scholar] [CrossRef] [PubMed]
- Lizarralde-Bejarano, D.P.; Arboleda-Sanchez, S.; Puerta-Yepes, M.E. Understanding epidemics from mathematical models: Details of the 2010 dengue epidemic in Bello (Antioquia, Colombia). Appl. Math. Model. 2017, 43, 566–578. [Google Scholar] [CrossRef]
- Mann, H.B.; Whitney, D.R. On a test of whether one of two random variables is stochastically larger than the other. Ann. Math. Stat. 1947, 18, 50–60. [Google Scholar] [CrossRef]
- Clements, A.; Paterson, G. The analysis of mortality and survival rates in wild populations of mosquitoes. J. Appl. Ecol. 1981, 18, 373–399. [Google Scholar] [CrossRef]
- Xue, D. MATLAB Programming: Mathematical Problem Solutions; de Gruyter: New York, NY, USA, 2020. [Google Scholar]
- Marshall, A.W.; Olkin, I. Life Distributions; Springer: New York, NY, USA, 2006. [Google Scholar]
- Wasserman, L. All of Nonparametric Statistics; Springer: New York, NY, USA, 2006. [Google Scholar]
- Lambert, P.C.; Thompson, J.R.; Weston, C.L.; Dickman, P.W. Estimating and modeling the cure fraction in population-based cancer survival analysis. Biostatistics 2006, 8, 576–594. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Sparling, Y.H.; Younes, N.; Lachin, J.M.; Bautista, O.M. Parametric survival models for interval-censored data with time-dependent covariates. Biostatistics 2006, 7, 599–614. [Google Scholar] [CrossRef]
- Wager, T.D.; Keller, M.C.; Lacey, S.C.; Jonides, J. Increased sensitivity in neuroimaging analyses using robust regression. Neuroimage 2005, 26, 99–113. [Google Scholar] [CrossRef]
- Velasco, H.; Laniado, H.; Toro, M.; Leiva, V.; Lio, Y. Robust three-step regression based on comedian and its performance in cell-wise and case-wise outliers. Mathematics 2020, 8, 1259. [Google Scholar] [CrossRef]
- Fritsch, V.; DaMota, B.; Varoquaux, G.; Frouin, V.; Loth, E.; Poline, J.B.; Thirion, B. Robust group-level inference in neuroimaging genetic studies. In Proceedings of the 2013 International Workshop on Pattern Recognition in Neuroimaging, Philadelphia, PA, USA, 22–24 June 2013; pp. 21–24. [Google Scholar]
- Hugo, L.E.; Jeffery, J.A.L.; Trewin, B.J.; Wockner, L.F.; Yen, N.T.; Le, N.H.; Nghia, L.T.; Hine, E.; Ryan, P.A.; Kay, B.H. Adult survival of the dengue mosquito Aedes aegypti varies seasonally in central Vietnam. PLoS Negl. Trop. Dis. 2014, 8, e2669. [Google Scholar] [CrossRef]
- Galar, D.; Kumar, U. Prognosis. In eMaintenance; Elsevier: Amsterdam, The Netherlands, 2017; pp. 311–370. [Google Scholar]
- Farewell, V.T. Mixture models in survival analysis: Are they worth the risk? Can. J. Stat. 1986, 14, 257–262. [Google Scholar] [CrossRef]
- Ryan, S.J.; Ben-Horin, T.; Johnson, L.R. Malaria control and senescence: The importance of accounting for the pace and shape of aging in wild mosquitoes. Ecosphere 2015, 6, art170. [Google Scholar] [CrossRef]
- Liu, Z.; Zhang, Z.; Lai, Z.; Zhou, T.; Jia, Z.; Gu, J.; Wu, K.; Chen, X.G. Temperature increase enhances Aedes albopictus competence to transmit dengue virus. Front. Microbiol. 2017, 8, 2337. [Google Scholar] [CrossRef] [Green Version]
- Leiva, V.; Ruggeri, F.; Saulo, H.; Vivanco, J.F. A methodology based on the Birnbaum-Saunders distribution for reliability analysis applied to nano-materials. Reliab. Eng. Syst. Saf. 2017, 157, 192–201. [Google Scholar] [CrossRef]
- Athayde, E.; Azevedo, A.; Barros, M.; Leiva, V. Failure rate of Birnbaum-Saunders distributions: Shape, change-point, estimation and robustness. Braz. J. Probab. Stat. 2019, 33, 301–328. [Google Scholar] [CrossRef] [Green Version]
- Costa, E.; Santos-Neto, M.; Leiva, V. Optimal sample size for the Birnbaum-Saunders distribution under decision theory with symmetric and asymmetric loss functions. Symmetry 2021, 13, 926. [Google Scholar] [CrossRef]


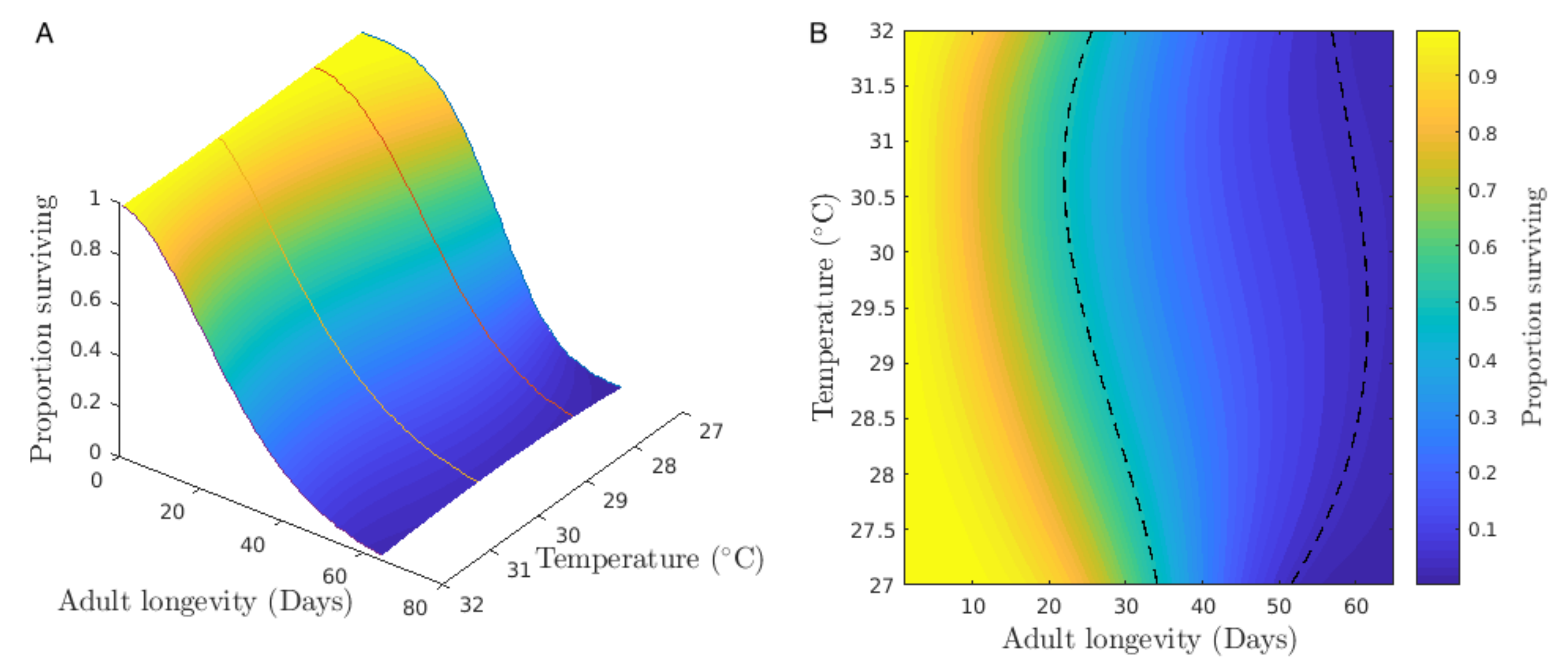
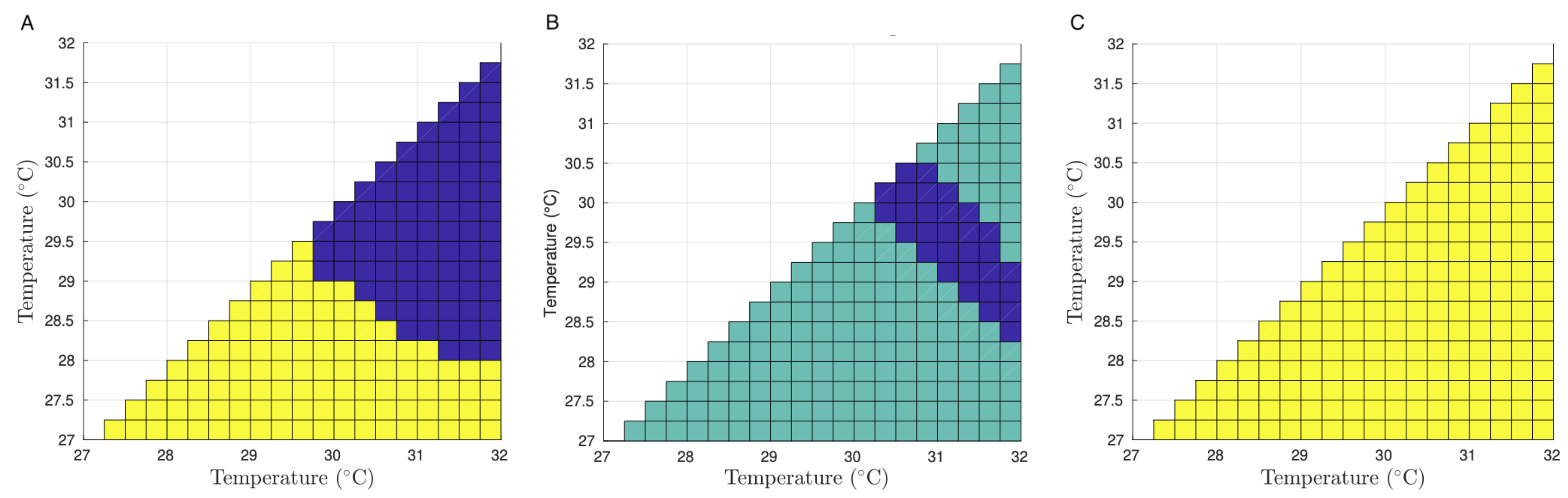
| Statistic | Bello | Villavicencio | Riohacha |
|---|---|---|---|
| Total count | 138 | 114 | 169 |
| Count to 23 C | 77 | 62 | 79 |
| Count to 27 C | 61 | 52 | 90 |
| Minimum | 12.00 | 10.00 | 8.00 |
| 25th percentile | 27.00 | 25.25 | 28.00 |
| Mean | 36.33 | 34.66 | 36.35 |
| Median | 37.00 | 35.00 | 38.00 |
| 75th percentile | 44.00 | 42.00 | 46.00 |
| Maximum | 72.00 | 63.00 | 69.00 |
| Standard deviation | 11.66 | 11.87 | 13.37 |
| Weibull Fitting | |||||
|---|---|---|---|---|---|
| City | CI() | CI() | |||
| Bello | 39.586 | 3.411 | 0.985 | ||
| Riohacha | 40.320 | 2.610 | 0.974 | ||
| Villavicencio | 38.144 | 3.067 | 0.994 | ||
| KS p-Value | |||
|---|---|---|---|
| Distribution | Bello | Villavicencio | Riohacha |
| Exponential | <0.0001 | <0.0001 | <0.0001 |
| Gompertz | <0.0001 | <0.0001 | <0.0001 |
| Log-logistic | |||
| Weibull | 0.5522 | 0.9967 | 0.4169 |
| Cities | Statistic | p-Value | Decision |
|---|---|---|---|
| Bello versus Riohacha | 11 171 | 0.2637 | Distributions are not statistically different at 5% |
| Bello versus Villavicencio | 7276 | 0.1530 | Distributions are not statistically different at 5% |
| Riohacha versus Villavicencio | 8704 | 0.0846 | Distributions are not statistically different at 5% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Velasco, H.; Laniado, H.; Toro, M.; Catano-López, A.; Leiva, V.; Lio, Y. Modeling the Risk of Infectious Diseases Transmitted by Aedes aegypti Using Survival and Aging Statistical Analysis with a Case Study in Colombia. Mathematics 2021, 9, 1488. https://doi.org/10.3390/math9131488
Velasco H, Laniado H, Toro M, Catano-López A, Leiva V, Lio Y. Modeling the Risk of Infectious Diseases Transmitted by Aedes aegypti Using Survival and Aging Statistical Analysis with a Case Study in Colombia. Mathematics. 2021; 9(13):1488. https://doi.org/10.3390/math9131488
Chicago/Turabian StyleVelasco, Henry, Henry Laniado, Mauricio Toro, Alexandra Catano-López, Víctor Leiva, and Yuhlong Lio. 2021. "Modeling the Risk of Infectious Diseases Transmitted by Aedes aegypti Using Survival and Aging Statistical Analysis with a Case Study in Colombia" Mathematics 9, no. 13: 1488. https://doi.org/10.3390/math9131488
APA StyleVelasco, H., Laniado, H., Toro, M., Catano-López, A., Leiva, V., & Lio, Y. (2021). Modeling the Risk of Infectious Diseases Transmitted by Aedes aegypti Using Survival and Aging Statistical Analysis with a Case Study in Colombia. Mathematics, 9(13), 1488. https://doi.org/10.3390/math9131488









