First Solution of Fractional Bioconvection with Power Law Kernel for a Vertical Surface
Abstract
1. Introduction
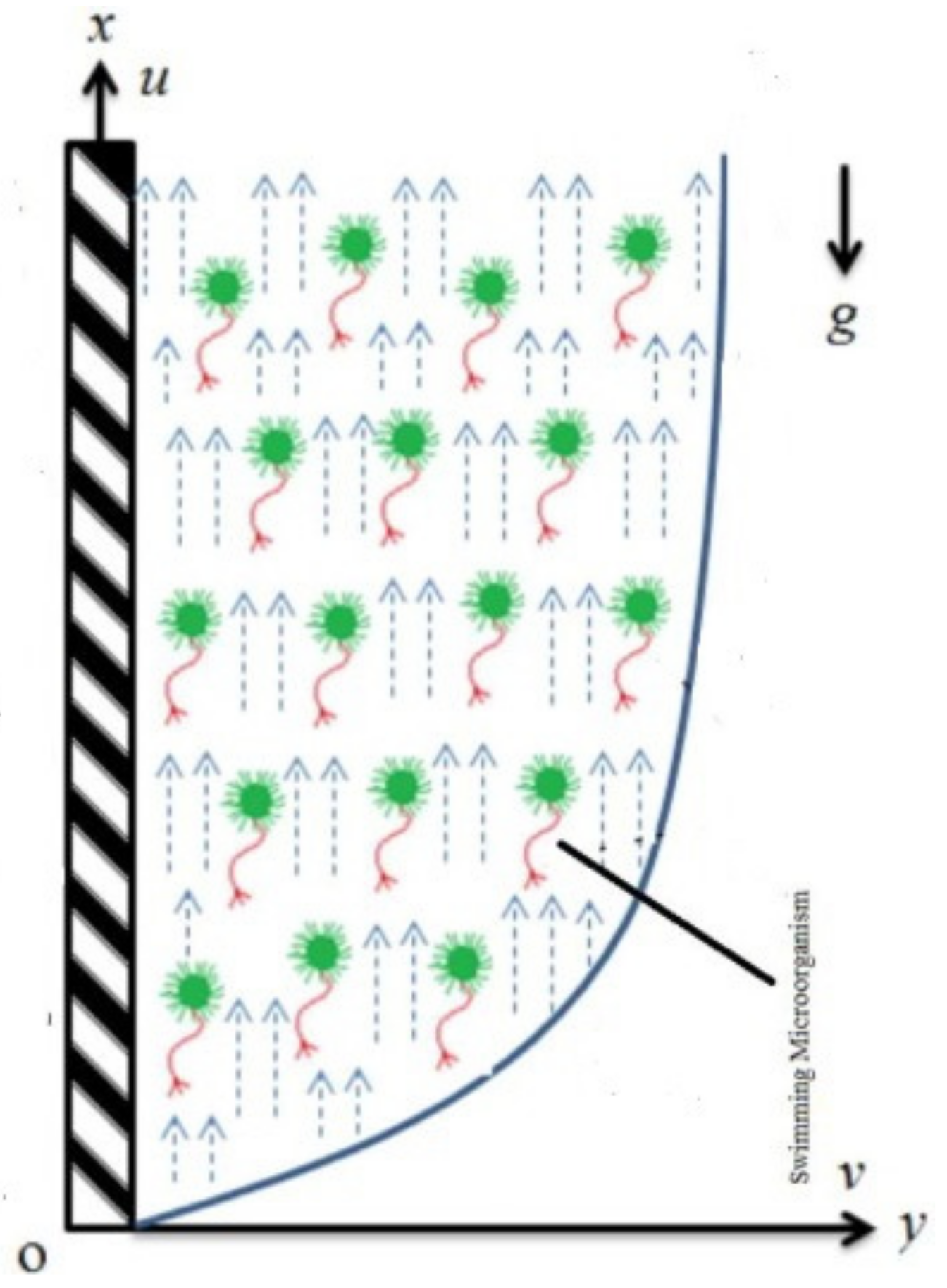
2. Mathematical Formulation
3. The Solution of the Problem with Classical Time Derivative
3.1. The Solution of Bioconvection
3.2. The Solution of Temperature Field
3.3. The Solution of the Velocity Field
3.4. Fractional Modeling
3.5. Solution of the Fractional Model Using Generalized Constitutive Relations
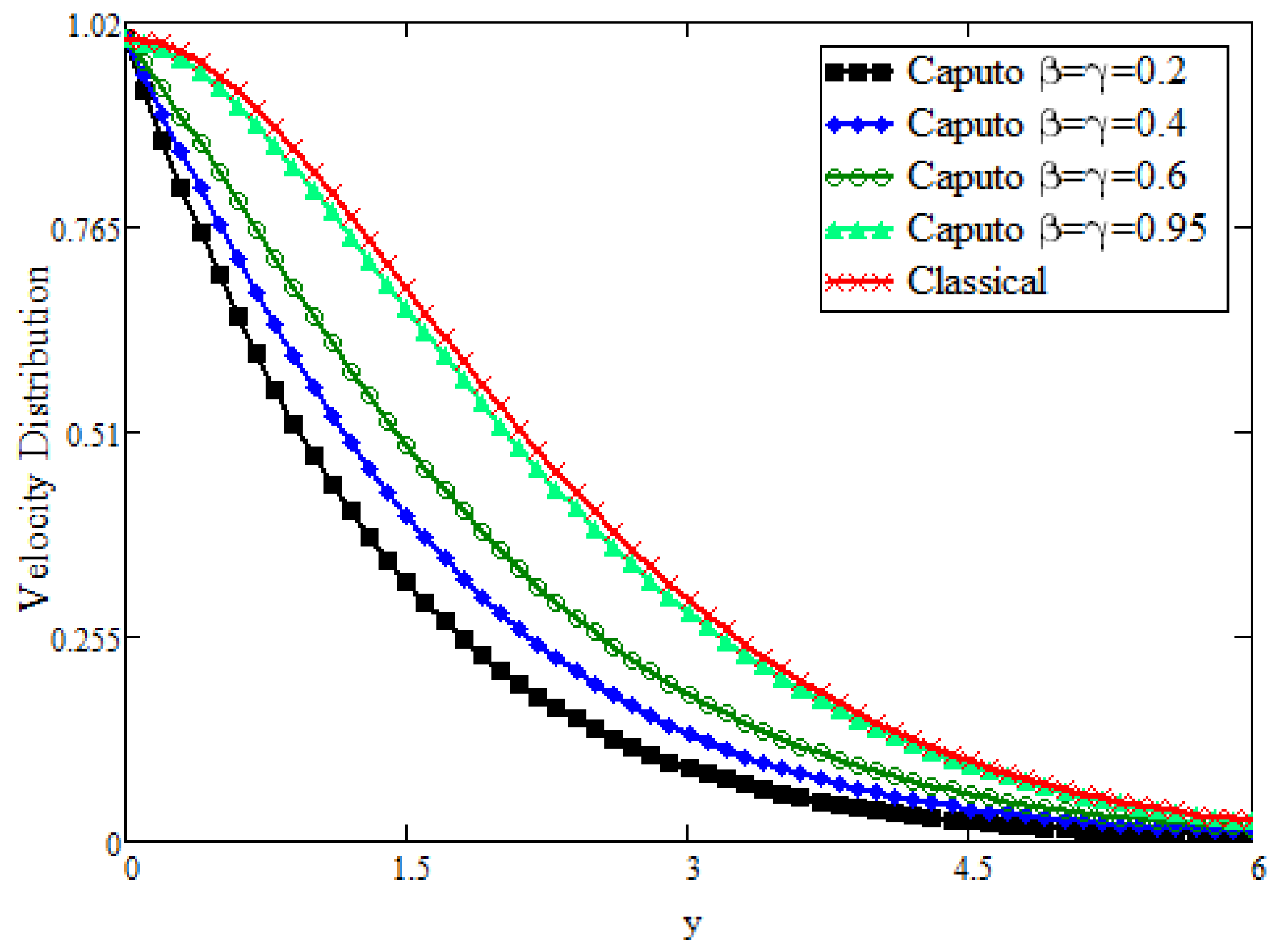
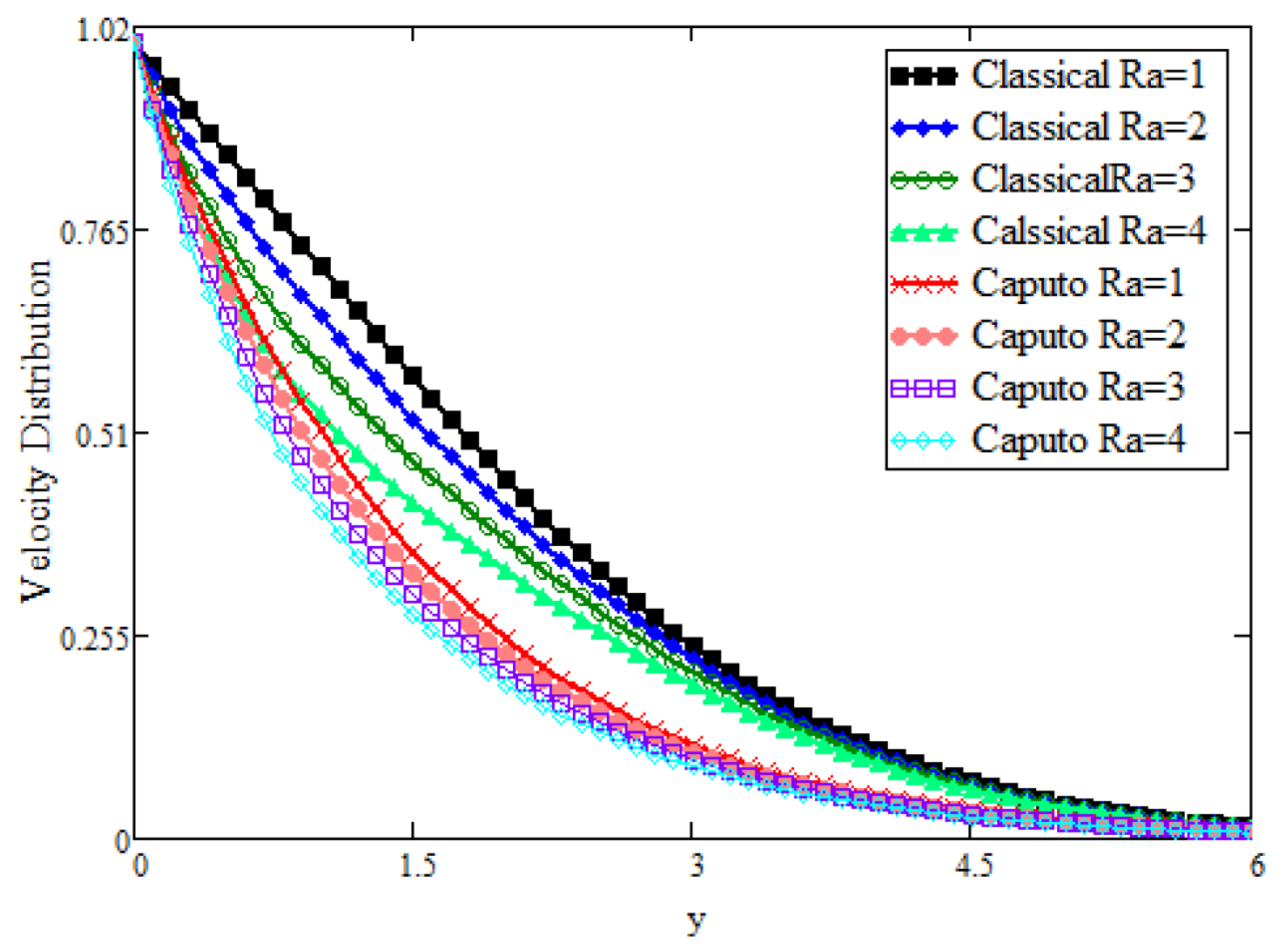
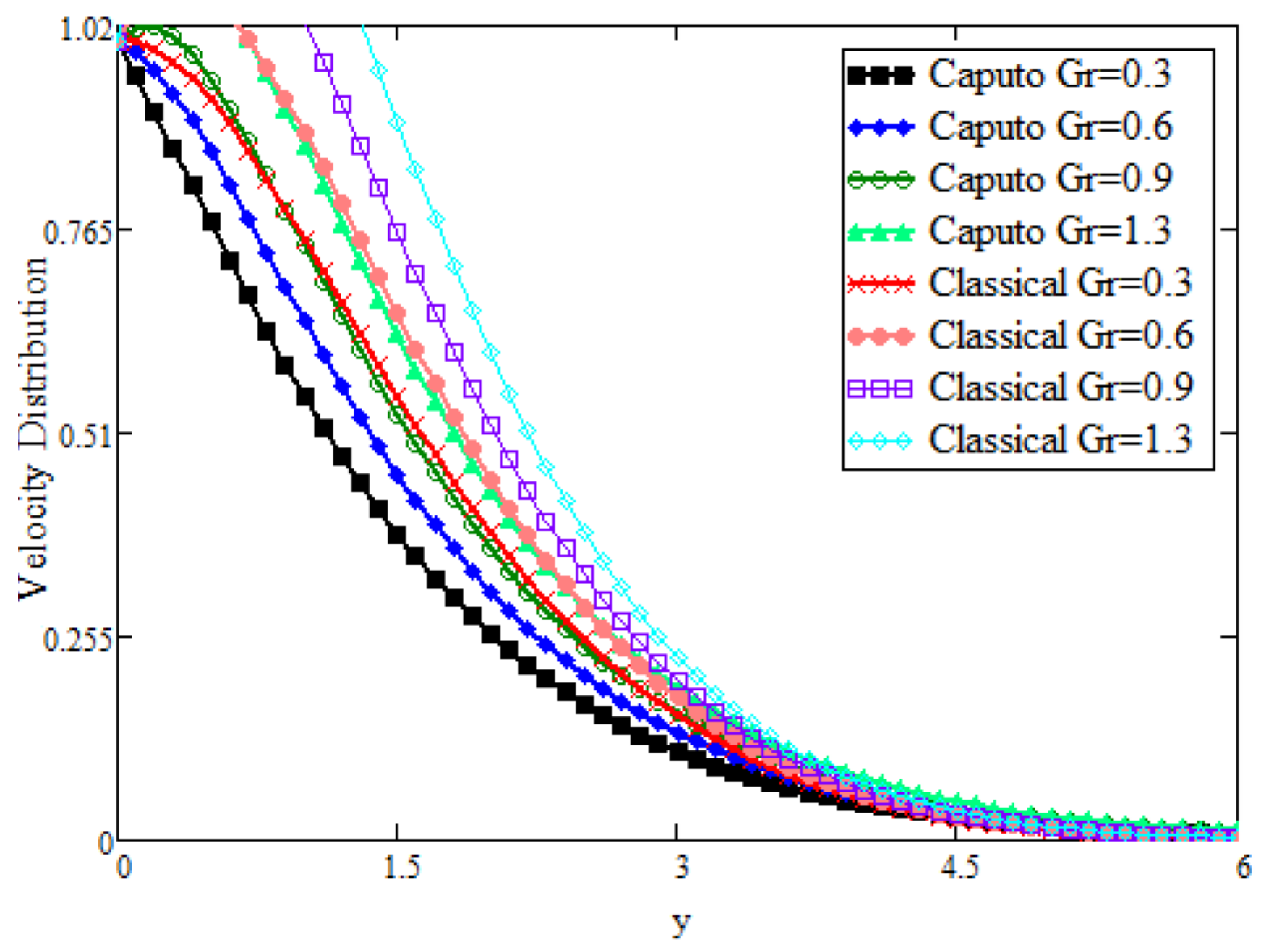
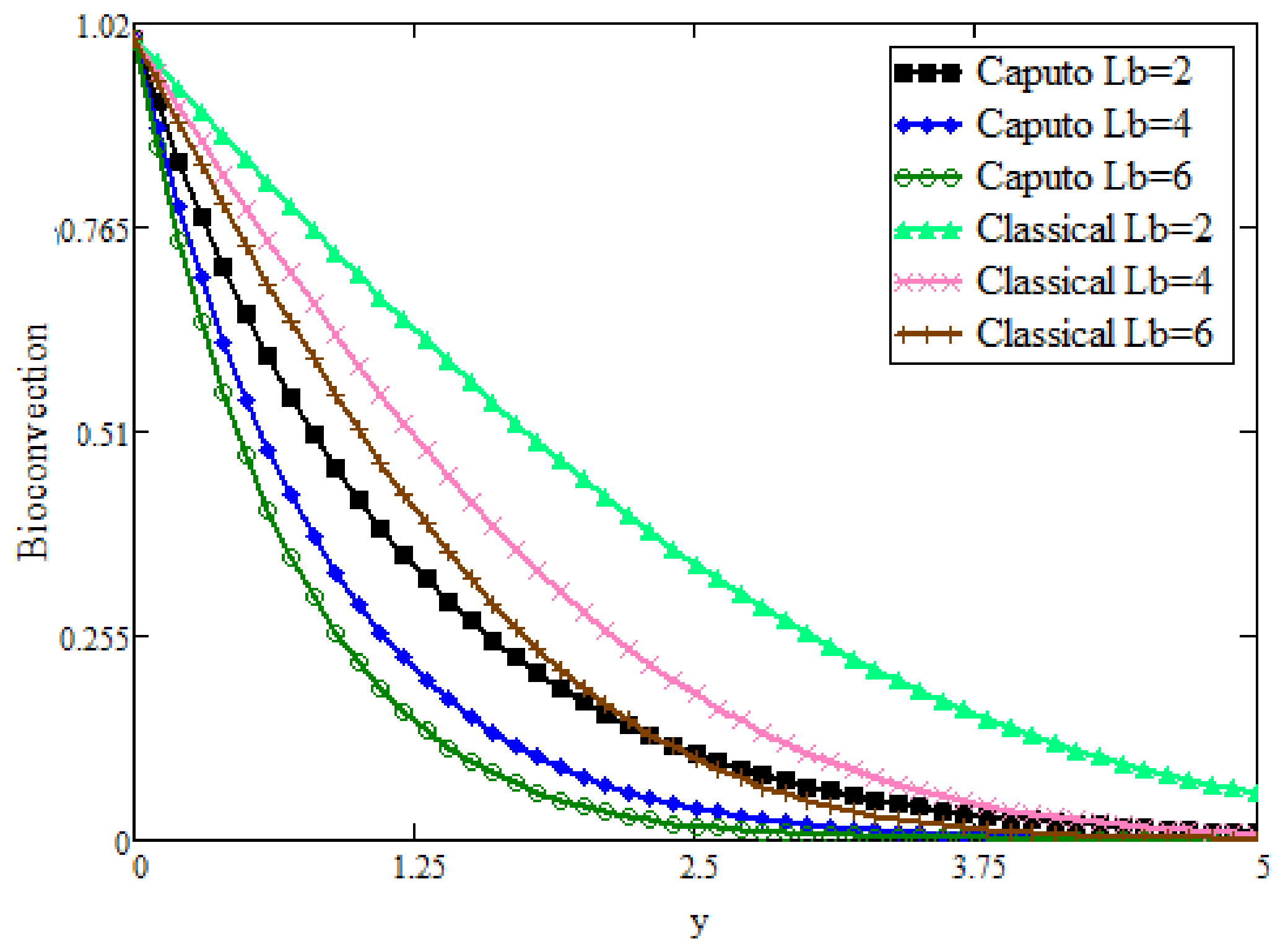
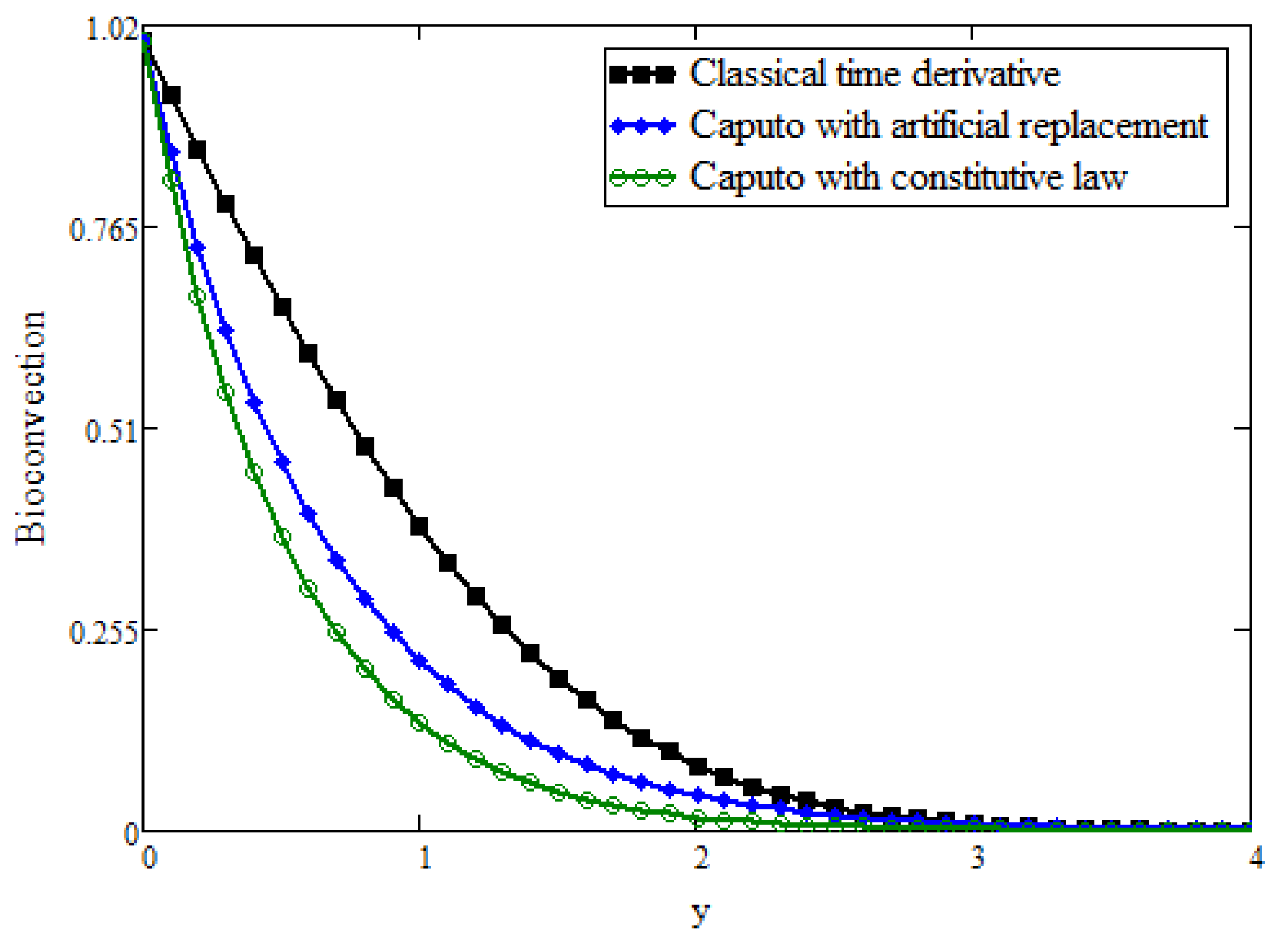
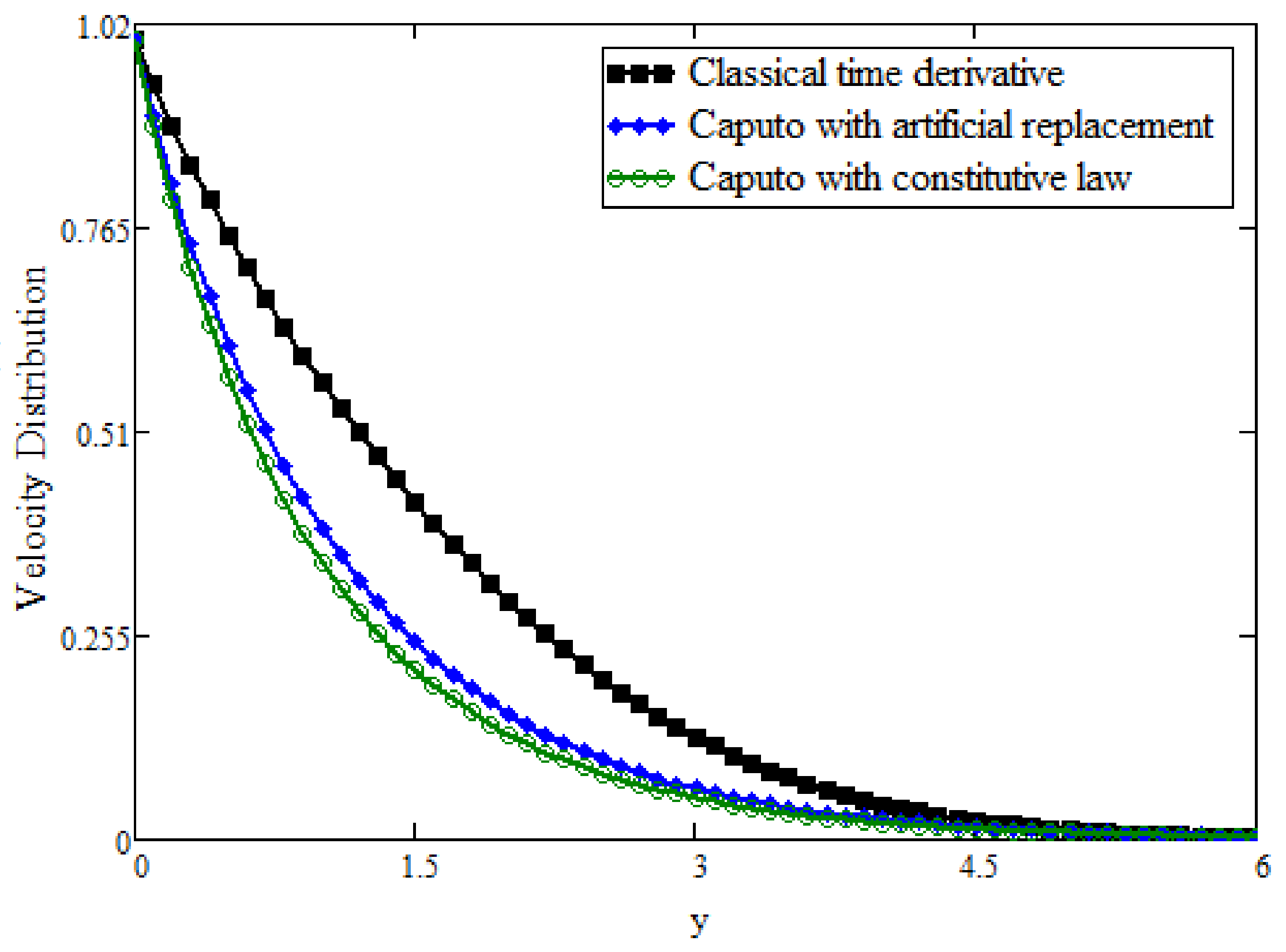
4. Results and Discussion
5. Conclusions
- The fractional parameter can be used to control the boundary layers of the fluid properties like bioconvection, temperature and velocity.
- The fractional approach can be the best fit in some experimental work, where the needed and desired results can be achieved for different values of fractional parameters and time.
- The fractional model obtained with generalized constitutive laws gives better and more accurate results in terms of memory than the fractional approach with artificial replacement.
- The present results are compared with the existing literature in the absence of bioconvection and they are in good agreement.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| Symbol | Name |
| Fluid density | |
| s | Laplace transform |
| Viscosity | |
| Prandtl number | |
| g | Gravitational acceleration |
| Bioconvection Lewis number | |
| T | Fluid temperature |
| Grashof number | |
| Temperature at wall | |
| Bioconvection Rayleigh number | |
| Ambient temperature of the fluid | |
| Gauss’s error function of complimentary | |
| Volumetric coefficient of thermal expansion | |
| The dimensionless nanoparticle volume fraction | |
| Mass density | |
| t | Time (s) |
| Fluid density | |
| Concentration of microorganisms | |
| N | The dimensionless concentration of microorganisms |
| The density of motile microorganisms | |
| Specific heat at constant pressure | |
| k | Thermal conductivity |
| Diffusivity of microorganisms | |
| Dimensionless temperature | |
| u | Velocity |
References
- Platt, J.R. Bioconvection patterns in cultures of free-swimming organisms. Science 1961, 133, 1766–1767. [Google Scholar] [CrossRef] [PubMed]
- Khan, N.S.; Shah, Q.; Sohail, A. Dynamics with Cattaneo-Christov heat and mass flux theory of bioconvection Oldroyd-B nanofluid. Adv. Mech. Eng. 2020, 12, 1–20. [Google Scholar]
- Ramesh, K.; Khan, S.U.; Jameel, M.; Khan, M.I.; Chu, Y.-M.; Kadry, S. Bioconvection assessment in Maxwell nanofluid configured by a Riga surface with nonlinear thermal radiation and activation energy. Surf. Interfaces 2020, 21, 100749. [Google Scholar] [CrossRef]
- Tlili, I.; Ramzan, M.; Nisa, H.U.; Shutaywi, M.; Shah, Z.; Kumam, P. Onset of gyrotactic microorganisms in MHD micropolar nanofluid flow with partial slip and double stratification. J. King Saud Univ. Sci. 2020, 32, 2741–2751. [Google Scholar] [CrossRef]
- Kotha, G.; Kolipaula, V.R.; Rao, M.V.S.; Penki, S.; Chamkha, A.J. Internal heat generation on bioconvection of an MHD nanofluid flow due to gyrotactic microorganisms. Eur. J. Plus 2020, 135, 1–19. [Google Scholar]
- Khan, N.S.; Shah, Q.; Bhaumik, A.; Kumam, P.; Thounthong, P.; Amiri, I. Entropy generation in bioconvection nanofluid flow between two stretchable rotating disks. Sci. Rep. 2020, 10, 4448. [Google Scholar] [CrossRef]
- Shah, Z.; Alzahrani, E.; Jawad, M.; Khan, U. Microstructure and inertial characteristics of MHD suspended SWCNTS and MWCNTS based Maxwell nanofluid flow with bioconvection and entropy generation past a permeable vertical cone. Coatings 2020, 10, 998. [Google Scholar] [CrossRef]
- Chu, Y.-M.; Khan, M.I.; Khan, N.B.; Kadry, S.; Khan, S.U.; Tlili, I.; Nayak, M. Significance of activation energy, bio-convection and magnetohydrodynamic in flow of third grade fluid (non-Newtonian) towards stretched surface: A Buongiorno model analysis. Int. Heat Mass Transf. 2020, 118, 104893. [Google Scholar] [CrossRef]
- Bhatti, M.M.; Marin, M.; Zeeshan, A.; Ellahi, R.; Abdelsalam, S.I. Swimming of motile gyrotactic microorganisms and nanoparticles in blood flow through anisotropically tapered arteries. Front. Phys. 2020, 8, 95. [Google Scholar] [CrossRef]
- Abbasi, A.; Mabood, F.; Farooq, W.; Batool, M. Bioconvective flow of viscoelastic nanofluid over a convective rotating stretching disk. Int. Commun. Heat Mass Transf. 2020, 119, 104921. [Google Scholar] [CrossRef]
- Waqas, H.; Khan, S.A.; Khan, S.U.; Khan, M.I.; Kadry, S.; Chu, Y.M. Falkner-skan time-dependent bioconvrction flow of cross nanofluid with nonlinear thermal radiation, activation energy and melting process. Int. Commun. Heat Mass Transf. 2021, 120, 105028. [Google Scholar] [CrossRef]
- Waqas, H.; Imran, M.; Bhatti, M. Influence of bioconvection on Maxwell nanofluid flow with the swimming of motile microorganisms over a vertical rotating cylinder. Chin. J. Phys. 2020, 68, 558–577. [Google Scholar] [CrossRef]
- Alshomrani, A.S.; Ullah, M.Z.; Baleanu, D. Importance of multiple slips on bioconvection flow of cross nanofluid past a wedge with gyrotactic motile microorganisms. Case Stud. Therm. Eng. 2020, 22, 100798. [Google Scholar] [CrossRef]
- Alshomrani, A.S. Numerical investigation for bio-convection flow of viscoelastic nanofluid with magnetic dipole and motile microorganisms. Arab. J. Sci. Eng. 2021, 46, 5945–5956. [Google Scholar] [CrossRef]
- Ullah, M.Z.; Jang, T. An efficient numerical scheme for analyzing bioconvection in vonk LarmLan flow of third-grade nanofluid with motile microorganisms. Alex. Eng. J. 2020, 59, 2739–2752. [Google Scholar] [CrossRef]
- Naz, R.; Noor, M.; Hayat, T.; Javed, M.; Alsaedi, A. Dynamism of magnetohydrodynamic cross nanofluid with particulars of entropy generation and gyrotactic motile microorganisms. Int. Commun. Heat Mass Transf. 2020, 110, 104431. [Google Scholar] [CrossRef]
- Naz, R.; Tariq, S.; Sohail, M.; Shah, Z. Investigation of entropy generation in stratified MHD Carreau nanofluid with gyrotactic microorganisms under Von Neumann similarity transformations. Eur. Phys. J. Plus 2020, 135, 178. [Google Scholar] [CrossRef]
- Alqarni, M.; Waqas, H.; Imran, M.; Alghamdi, M.; Muhammad, T. Thermal transport of bioconvection flow of micropolar nanofluid with motile microorganisms and velocity slip effects. Phys. Scr. 2020, 96, 015220. [Google Scholar] [CrossRef]
- Khan, S.U.; Waqas, H.; Bhatti, M.; Imran, M. Bioconvection in the rheology of magnetized couple stress nanofluid featuring activation energy and wufs slip. J. Non-Equilib. Thermodyn. 2020, 45, 81–95. [Google Scholar] [CrossRef]
- Sajid, T.; Sagheer, M.; Hussain, S.; Shahzad, F. Impact of double-diffusive convection and motile gyrotactic microorganisms on magnetohydrodynamics bioconvection tangent hyperbolic nanofluid. Open Phys. 2020, 18, 74–88. [Google Scholar] [CrossRef]
- Ali, B.; Hussain, S.; Nie, Y.; Ali, L.; Hassan, S.U. Finite element simulation of bioconvection and Cattaneo-Christov effects on micropolar based nanofluid flow over a vertically stretching sheet. Chin. J. Phys. 2020, 68, 654–670. [Google Scholar] [CrossRef]
- Mahdy, A.; Nabwey, H.A. Microorganisms time-mixed convection nanofluid flow by the stagnation domain of an impulsively rotating sphere due to newtonian heating. Results Phys. 2020, 19, 103347. [Google Scholar] [CrossRef]
- Sharif, H.; Naeem, M.N.; Khadimallah, M.A.; Ayed, H.; Bouzgarrou, S.M.; Naim, A.F.A.; Hussain, S.; Hussain, M.; Iqbal, Z.; Tounsi, A. Energy effects on mhd flow of eyringfs nanofluid containing motile microorganism. Adv. Concr. Constr. 2020, 10, 357–367. [Google Scholar]
- Ansari, M.S.; Otegbeye, O.; Trivedi, M.; Goqo, S. Magnetohydrodynamic bioconvective Casson nanofluid flow: A numerical simulation by paired quasilinearisationh. J. Appl. Comput. Mech. 2020. [Google Scholar] [CrossRef]
- Shah, N.A.; Imran, M.A.; Miraj, F. Exact solutions of time fractional free convection flows of viscous fluid over an isothermal vertical plate with Caputo and Caputo-fabrizio derivatives. J. Prime Res. Math. 2017, 13, 56–74. [Google Scholar]
- Podlubny, I. Fractional Differential Equations: An Introduction to Fractional Derivatives, Fractional Differential Equations, to Methods of Their Solution and Some of Their Applications; Elsevier: Edinburgh, UK, 1998. [Google Scholar]
- Samko, S.G.; Kilbas, A.A.; Marichev Oleg, I. Fractional Integrals and Derivatives; Gordon and Breach Science Publishers: London, UK, 1993. [Google Scholar]
- Kilbas, A. Theory and Applications of Fractional Differential Equations; Elsevier Science Inc.: New York, NY, USA, 2006; ISBN 978-0-444-51832-3. [Google Scholar]
- Magin, R.L. Fractional Calculus in Bioengineering; Begell House Redding: Redding, CT, USA, 2006; Volume 2. [Google Scholar]
- Caputo, M.; Fabrizio, M. Applications of new time and spatial fractional derivatives with exponential kernels. Progr. Fract. Differ. Appl. 2016, 2, 1–11. [Google Scholar] [CrossRef]
- Hilfer, R. Applications of Fractional Calculus in Physics; World Scientific: Singapore, 2000. [Google Scholar]
- Lorenzo, C.F.; Hartley, T.T. Variable order and distributed order fractional operators. Nonlinear Dyn. 2002, 29, 57–98. [Google Scholar] [CrossRef]
- Salahshour, S.; Ahmadian, A.; Senu, N.; Baleanu, D.; Agarwal, P. On analytical solutions of the fractional differential equation with uncertainty: Application to the basset problem. Entropy 2015, 17, 885–902. [Google Scholar] [CrossRef]
- Pakdaman, M.; Ahmadian, A.; Effati, S.; Salahshour, S.; Baleanu, D. Solving differential equations of fractional order using an optimization technique based on training artificial neural network. Appl. Math. Comput. 2017, 293, 81–95. [Google Scholar] [CrossRef]
- Ahmadian, A.; Salahshour, S.; Chan, C.S. Fractional differential systems: A fuzzy solution based on operational matrix of shifted Chebyshev polynomials and its applications. IEEE Trans. Fuzzy Syst. 2016, 25, 218–236. [Google Scholar] [CrossRef]
- Ahmadian, A.; Ismail, F.; Salahshour, S.; Baleanu, D.; Ghaemi, F. Uncertain viscoelastic models with fractional order: A new spectral tau method to study the numerical simulations of the solution. Commun. Nonlinear Sci. Numer. Simul. 2017, 53, 44–64. [Google Scholar] [CrossRef]
- Shahriyar, M.; Ismail, F.; Aghabeigi, S.; Ahmadian, A.; Salahshour, S. An eigenvalueeigenvector method for solving a system of fractional differential equations with uncertainty. Math. Probl. Eng. 2013, 2013, 579761. [Google Scholar] [CrossRef]
- Salahshour, S.; Ahmadian, A.; Abbasbandy, S.; Baleanu, D. M-fractional derivative under interval uncertainty: Theory, properties and applications. Chaos Solitons Fractals 2018, 117, 84–93. [Google Scholar] [CrossRef]
- Basit, A.; Imran, M.A.; Akgul, A. Convective flow of a fractional second grade fluid containing different nanoparticles with Prabhakar fractional derivative subject to non-uniform velocity at the boundary. Math. Meth. Appl. Sci. 2021. [Google Scholar] [CrossRef]
- Butt, A.R.; Abdullah, M.; Raza, N.; Imran, M.A. Influence of non-integer order parameter and Hartmann number on the heat and mass transfer flow of a Jeffery fluid over an oscillating vertical plate via Caputo-Fabrizio time fractional derivatives. Eur. Phys. J. Plus 2017, 132, 414. [Google Scholar] [CrossRef]
- Jamil, M.; Khan, N.A.; Imran, M.A. New exact solutions for an Oldroyd-B Fluid with Fractional Derivatives: Stokes’ first problem. Int. J. Nonlinear Sci. Numer. Simul. 2013, 14, 443–451. [Google Scholar] [CrossRef]
- Asjad, M.I.; Aleem, M.; Ahmadian, A.; Salahshour, S.; Ferrara, M. New trends of fractional modeling and heat and mass transfer investigation of (SWCNTs and MWCNTs)-CMC based nanofluids flow over inclined plate with generalized boundary conditions. Chin. J. Phys. 2020, 66, 497–516. [Google Scholar] [CrossRef]
- Ahmad, M.M.; Imran, M.A.; Aleem, M.; Khan, I. A comparative study and analysis of natural convection flow of MHD non-Newtonian fluid in the presence of heat source and first-order chemical reaction. J. Therm. Anal. Calorim. 2019, 137, 1783–1796. [Google Scholar] [CrossRef]
- Sohail, A.; Vieru, D.; Imran, M.A. Influence of side walls on the oscillating motion of a Maxwell fluid over an infinite plate. Mechanics 2013, 19, 269–276. [Google Scholar] [CrossRef]
- Jarad, F.; Abdeljawad, T. Generalized fractional derivatives and laplace transform. Discret. Contin. Dyn. Syst. S 2020, 13, 709–722. [Google Scholar] [CrossRef]
- Vieru, D.; Fetecau, C.; Fetecau, C. Time-fractional free convection flow near a vertical plate with Newtonian heating and mass diffusion. Therm. Sci. 2015, 19 (Suppl. 1), 85–98. [Google Scholar] [CrossRef]
- Abro, K.A.; Soomro, M.; Atangana, A.; Lomez-Aguilar, J.G. Thermophysical properties of Maxwell nanofluids via fractional derivatives with regular kernel. J. Therm. Anal. Calorim. 2020, 1–11. [Google Scholar] [CrossRef]
- Zhang, Z. A novel covid-19 mathematical model with fractional derivatives: Singular and nonsingular kernels. Chaos Solitons Fractals 2020, 139, 110060. [Google Scholar] [CrossRef]
- Rayal, A.; Verma, S.R. Numerical analysis of pantograph differential equation of the stretched type associated with fractal-fractional derivatives via fractional order Legendre wavelets. Chaos Solitons Fractals 2020, 139, 110076. [Google Scholar] [CrossRef]
- Singh, J.; Kumar, D.; Baleanu, D. On the analysis of chemical kinetics system pertaining to a fractional derivative with Mittag-Leffler type kernel. Chaos Interdiscip. J. Nonlinear Sci. 2017, 27, 103113. [Google Scholar] [CrossRef] [PubMed]
- Ghanbari, B.; Atangana, A. Some new edge detecting techniques based on fractional derivatives with non-local and non-singular kernels. Adv. Differ. Equ. 2020, 2020, 1–19. [Google Scholar] [CrossRef]
- Son, N.T.; Dong, N.P.; Long, H.V.; Khastan, A. Linear quadratic regulator problem governed by granular neutrosophic fractional differential equations. ISA Trans. 2020, 97, 296–316. [Google Scholar] [CrossRef] [PubMed]
- Gambo, Y.; Ameen, R.; Jarad, F.; Abdeljawad, T. Existence and uniqueness of solutions to fractional differential equations in the frame of generalized Caputo fractional derivatives. Adv. Differ. Equ. 2018, 2018, 134. [Google Scholar] [CrossRef]
- Akgul, E.K. Solutions of the linear and nonlinear differential equations within the generalized fractional derivatives. Chaos Interdiscip. J. Nonlinear Sci. 2019, 29, 023108. [Google Scholar] [CrossRef]
- El-Nabulsi, A.R. Complexified quantum field theory and hmass without massh from multidimensional fractional actionlike variational approach with dynamical fractional exponents. Chaos Solitons Fractals 2009, 42, 2384–2398. [Google Scholar] [CrossRef]
- El-Nabulsi, A.; Wu, C. Fractional complexified field theory from saxena-kumbhat fractional integral, fractional derivative of order and dynamical fractional integral exponent. Afr. Diaspora J. Math. New Ser. 2012, 13, 45–61. [Google Scholar]
- Moshrefi-Torbati, M.; Hammond, J. Physical and geometrical interpretation of fractional operators. J. Frankl. Inst. 1998, 335, 1077–1086. [Google Scholar] [CrossRef]
- Cloot, A.; Botha, J. A generalised groundwater flow equation using the concept of noninteger order derivatives. Water Sa 2006, 32, 1–7. [Google Scholar]
- Lima, M.F.; Machado, J.T.; CrisLostomo, M. Experimental signal analysis of robot impacts in a fractional calculus perspective. J. Adv. Comput. Intell. Intell. Inform. 2007, 11, 1079–1085. [Google Scholar] [CrossRef]
- Bhalekar, S.; Daftardar-Gejji, V.; Baleanu, D.; Magin, R. Fractional bloch equation with delay. Comput. Math. Appl. 2011, 61, 1355–1365. [Google Scholar] [CrossRef]
- Odzijewicz, T.; Malinowska, A.B.; Torres, D.F. Fractional calculus of variations in terms of a generalized fractional integral with applications to physics. Abstr. Appl. Anal. 2012, 2012, 871912. [Google Scholar] [CrossRef]
- Malinowska, A.B. Fractional variational calculus for non-differentiable functions. In Fractional Dynamics and Control; Springer: New York, NY, USA, 2012; pp. 97–108. [Google Scholar]
- Kulish, V.V.; Lage, J.L. Application of fractional calculus to fluid mechanics. J. Fluids Eng. 2002, 124, 803–806. [Google Scholar] [CrossRef]
- El-Nabulsi, R.A. Path integral formulation of fractionally perturbed Lagrangian oscillators on fractal. J. Stat. Phys. 2018, 172, 1617–1640. [Google Scholar] [CrossRef]
- Meerschaert, M.M.; Benson, D.A.; Bäumer, B. Multidimensional advection and fractional dispersion. Phys. Rev. E 1999, 59, 5026. [Google Scholar] [CrossRef]
- Raees, A.; Xu, H.; Liao, S.-J. Unsteady mixed nano-bioconvection flow in a horizontal channel with its upper plate expanding or contracting. Int. J. Heat Mass Transf. 2015, 86, 174–182. [Google Scholar] [CrossRef]
- Zhao, Q.; Xu, H.; Tao, L. Unsteady bioconvection squeezing flow in a horizontal channel with chemical reaction and magnetic field effects. Math. Probl. Eng. 2017, 2017, 2541413. [Google Scholar] [CrossRef]
- Latiff, N.A.A.; Uddin, M.J.; Leg, O.A.B.; Ismail, A.I. Unsteady forced bioconvection slip flow of a micropolar nanofluid from a stretching/shrinking sheet. Proc. Inst. Mech. Eng. Part N J. Nanomater. Nanoeng. Nanosyst. 2016, 230, 177–187. [Google Scholar]
- Ali, L.; Liu, X.; Ali, B.; Mujeed, S.; Abdal, S. Finite element simulation of multi-slip effects on unsteady MHD bioconvective micropolar nanofluid flow over a sheet with solutal and thermal convective boundary conditions. Coatings 2019, 9, 842. [Google Scholar] [CrossRef]
- Hristov, J. Derivatives with non-singular kernels from the Caputo-Fabrizio definition and beyond: Appraising analysis with emphasis on diffusion models. Front. Fract. Calc. 2017, 1, 270–342. [Google Scholar]
- Povstenko, Y. Fractional Thermoelasticity; Springer: Cham, Switzerland, 2015. [Google Scholar]
- Ahmed, N.; Vieru, D.; Fetecau, C.; Shah, N.A. Convective flows of generalized time-nonlocal nanofluids through a vertical rectangular channel. Phys. Fluids 2018, 30, 052002. [Google Scholar] [CrossRef]
- Shah, N.A.; Khan, I.; Aleem, M.; Asjad, M.I. Influence of magnetic field on double convection problem of fractional viscous fluid over an exponentially moving vertical plate: New trends of caputo time-fractional derivative model. Adv. Mech. Eng. 2019, 11. [Google Scholar] [CrossRef]





Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Asjad, M.I.; Ur Rehman, S.; Ahmadian, A.; Salahshour, S.; Salimi, M. First Solution of Fractional Bioconvection with Power Law Kernel for a Vertical Surface. Mathematics 2021, 9, 1366. https://doi.org/10.3390/math9121366
Asjad MI, Ur Rehman S, Ahmadian A, Salahshour S, Salimi M. First Solution of Fractional Bioconvection with Power Law Kernel for a Vertical Surface. Mathematics. 2021; 9(12):1366. https://doi.org/10.3390/math9121366
Chicago/Turabian StyleAsjad, Muhammad Imran, Saif Ur Rehman, Ali Ahmadian, Soheil Salahshour, and Mehdi Salimi. 2021. "First Solution of Fractional Bioconvection with Power Law Kernel for a Vertical Surface" Mathematics 9, no. 12: 1366. https://doi.org/10.3390/math9121366
APA StyleAsjad, M. I., Ur Rehman, S., Ahmadian, A., Salahshour, S., & Salimi, M. (2021). First Solution of Fractional Bioconvection with Power Law Kernel for a Vertical Surface. Mathematics, 9(12), 1366. https://doi.org/10.3390/math9121366








