On the Stability of Convection in a Non-Newtonian Vertical Fluid Layer in the Presence of Gold Nanoparticles: Drug Agent for Thermotherapy
Abstract
1. Introduction
2. Mathematical Analysis
2.1. Basic State
2.2. Perturbed State and Linear Stability Analysis
3. Numerical Procedure
4. Results and Discussion
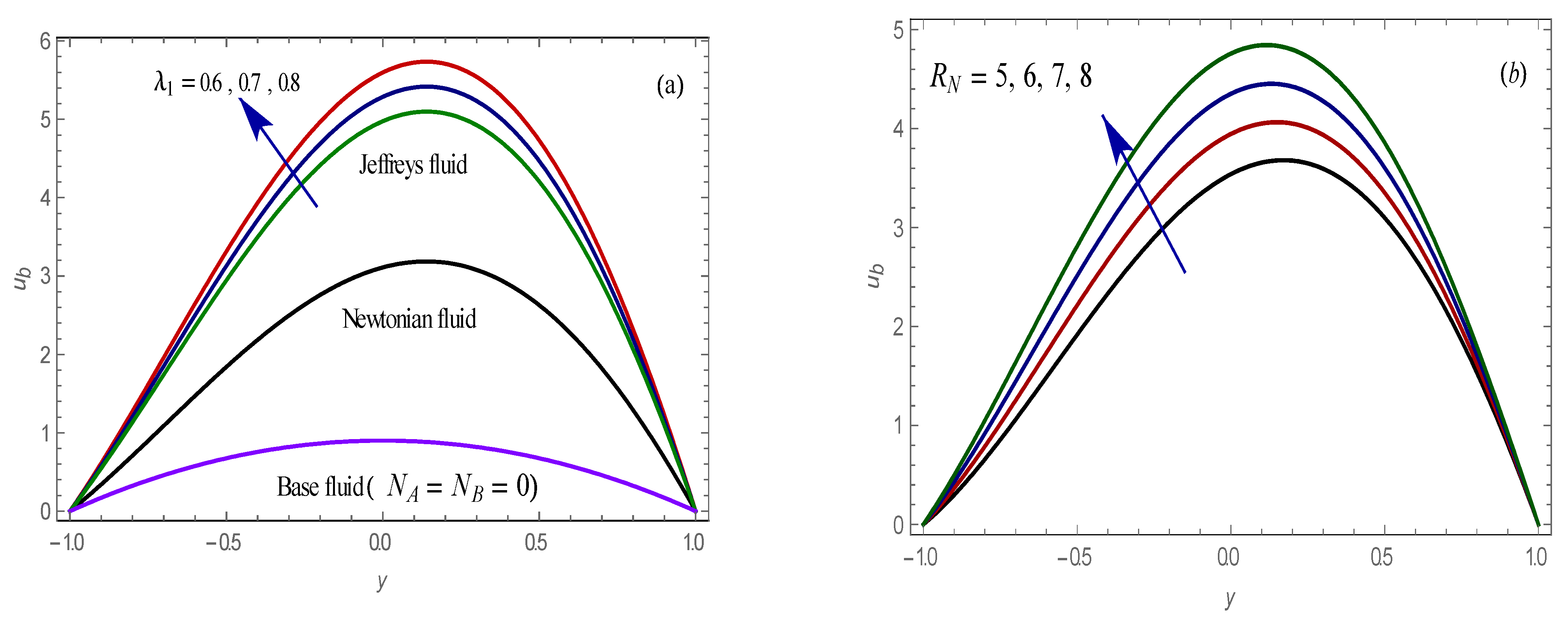
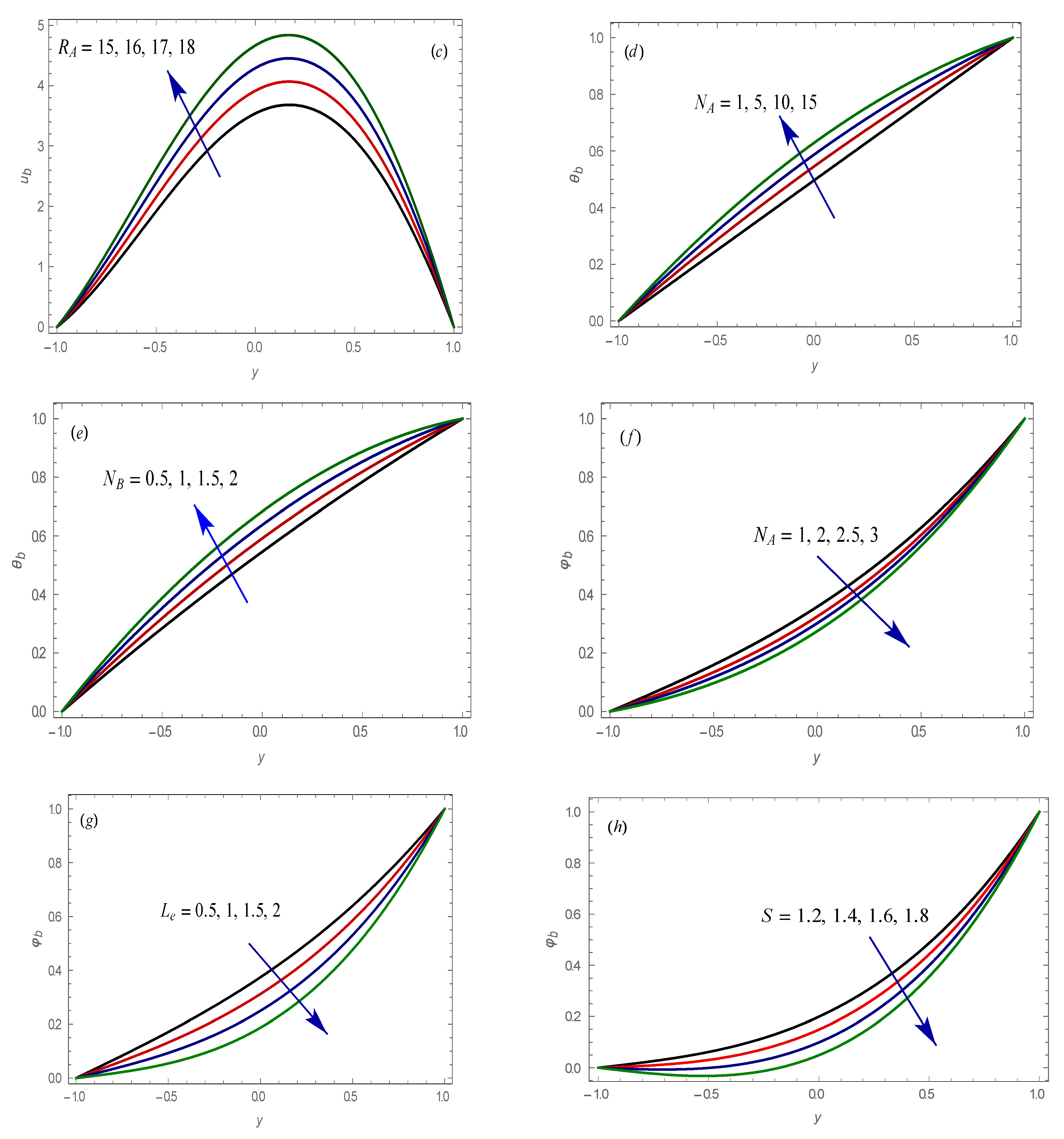
4.1. Base Flow
4.2. Validation of the Code
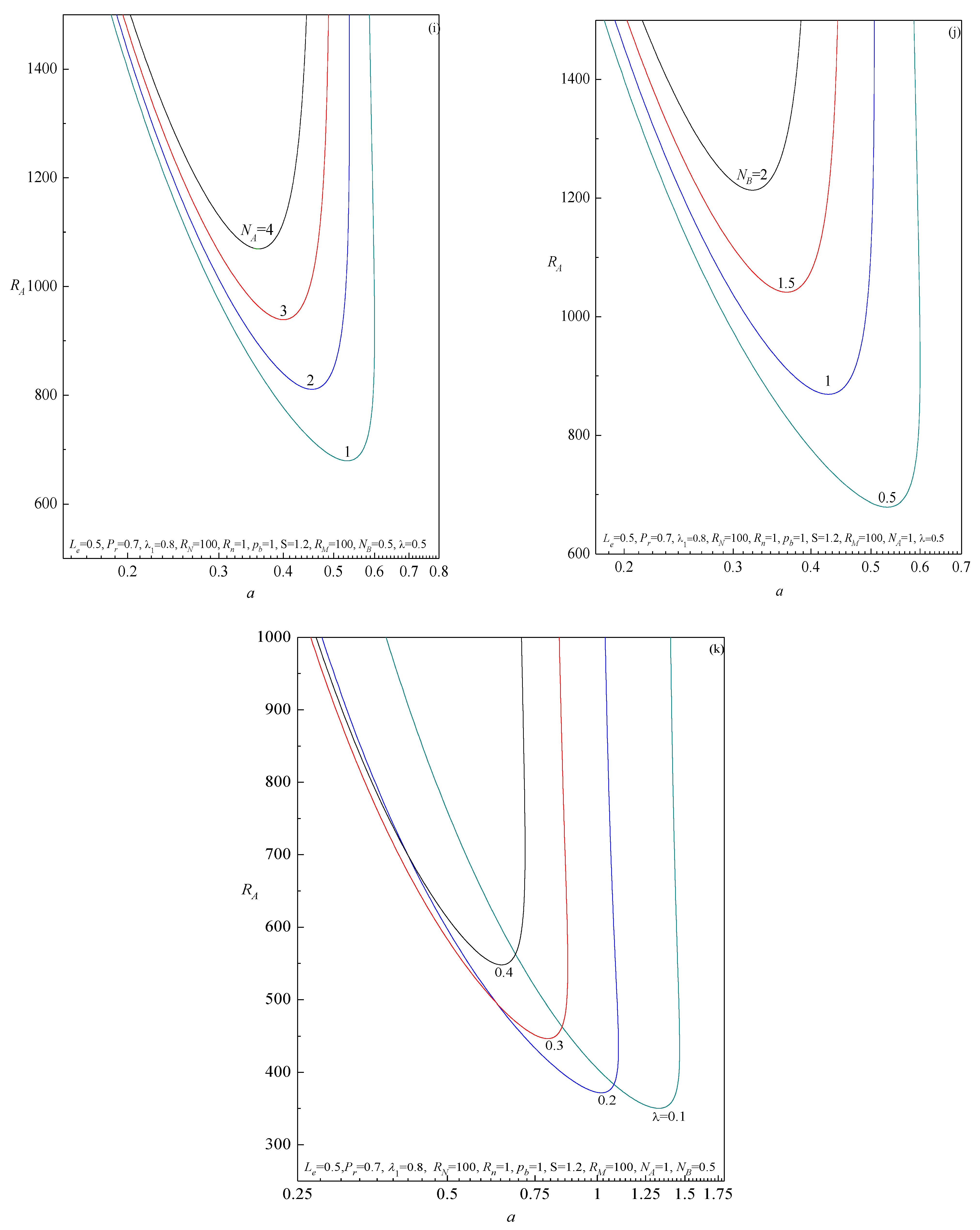
4.3. Neutral Stability Curves
5. Concluding Remarks
- The influences of the basic state parameters on the velocity, temperature and nanoparticles concentration were exhibited graphically.
- The preferred mode of instability is traveling-wave mode irrespective of the values of governing parameters.
- The effect of increasing and, is to hasten the onset of instability.
- The presence of and is to reinforce stability of the system. The density Rayleigh number instills both stabilizing and destabilizing effects on the basic flow.
- Newtonian fluid has a more stabilizing effect than second-grade and the Jeffreys fluids in the presence of gold nanoparticles; the Jeffreys fluid being the least stable.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hussain, F.; Ellahi, R.; Zeeshan, A.; Vafai, K. Modelling study on heated couple stress fluid peristaltically conveying gold nanoparticles through coaxial tubes: A remedy for gland tumors and arthritis. J. Mol. Liq. 2018, 268, 149–155. [Google Scholar] [CrossRef]
- Mekheimer, K.S.; Hasona, W.M.; Abo-Elkhair, R.E.; Zaher, A.Z. Peristaltic blood flow with gold nanoparticles as a third grade nanofluid in catheter: Application of cancer therapy. Phys. Lett. A 2018, 382, 85–93. [Google Scholar] [CrossRef]
- Ebaid, A.; Aljohani, A.F. Homotopy perturbation method for peristaltic motion of gold-blood nanofluid with heat source. Int. J. Numer. Methods Heat Fluid Flow 2020, 30, 3121–3138. [Google Scholar] [CrossRef]
- Abd Elmaboud, Y.; Mekheimer, K.S.; Emam, T.G. Numerical examination of gold nanoparticles as a drug carrier on peristaltic blood flow through physiological vessels: Cancer therapy treatment. BioNanoScience 2019, 9, 952–965. [Google Scholar] [CrossRef]
- Eldabe, N.T.; Ramadan, S.F. Impacts of peristaltic flow of micropolar fluid with nanoparticles through a porous medium under the effects of heat absorption and wall properties: Homotopy perturbation method. Heat Transf. 2020, 49, 889–908. [Google Scholar] [CrossRef]
- Eldabe, N.; Ramadan, S.; Awad, A. Analytical and numerical treatment to study the effects of hall currents with viscous dissipation, heat absorpation and chemical reaction on peristaltic flow of Carreau nanofluid. Therm. Sci. 2021, 25, 181–196. [Google Scholar] [CrossRef]
- Eldabe, N.T.; Kamel, K.A.; Ramadan, S.F.; Saad, R.A. Peristaltic motion of Eyring-Powell nano fluid with couple stresses and heat and mass transfer through a porous media under the effect of magnetic field inside asymmetric vertical channel. J. Adv. Res. Fluid Mech. Therm. Sci. 2020, 68, 58–71. [Google Scholar] [CrossRef]
- Orszag, S.A. Accurate solution of the Orr–Sommerfeld stability equation. J. Fluid Mech. 1971, 50, 689–703. [Google Scholar] [CrossRef]
- Drazin, P.G. Introduction to Hydrodynamic Stability; Cambridge University Press (CUP): Cambridge, UK, 2002; p. 32. [Google Scholar]
- Makinde, O.D. Chebyshev collocation approach to stability of blood flows in a large artery. Afr. J. Biotechnol. 2012, 11, 9881–9887. [Google Scholar] [CrossRef]
- Chimetta, B.P.; de Moraes Franklin, E. Asymptotic and Numerical Solutions of the Orr-Sommerfeld Equation for a Thin Liquid Film on an Inclined Plane. In Proceedings of the IV Journeys in Multiphase Flows (JEM2017), São Paulo, Brazil, 27–31 March 2017. [Google Scholar]
- Lin, J.; Xia, Y.; Bao, F. Hydrodynamic instability of nanofluids in a channel flow. Fluid Dyn. Res. 2014, 46, 055512. [Google Scholar] [CrossRef]
- Xia, Y.; Lin, J.; Bao, F.; Chan, T.L. Flow instability of nanofluids in jet. Appl. Math. Mech. 2015, 36, 141–152. [Google Scholar] [CrossRef]
- Anuar, N.S.; Bachok, N.; Arifin, N.M.; Rosali, H. MHD flow past a nonlinear stretching/shrinking sheet in carbon nanotubes: Stability analysis. Chin. J. Phys. 2020, 65, 436–446. [Google Scholar] [CrossRef]
- Hussain, Z.; Rehman, A.U.; Zeeshan, R.; Sultan, F.; Hamid, T.A.; Ali, M.; Shahzad, M. MHD instability of Hartmann flow of nanoparticles Fe2O3 in water. Appl. Nanosci. 2020, 10, 5149–5165. [Google Scholar] [CrossRef]
- Moatimid, G.M.; Hassan, M.A. Convection instability of non-Newtonian Walter’s nanofluid along a vertical layer. J. Egypt. Math. Soc. 2017, 25, 220–229. [Google Scholar] [CrossRef]
- Mekheimer, K.S.; Shankar, B.M.; Abo-Elkhair, R.E. Effects of Hall current and permeability on the stability of peristaltic flow. SN Appl. Sci. 2019, 1, 1–1610. [Google Scholar] [CrossRef]
- Shankar, B.M.; Kumar, J.; Shivakumara, I.S. Stability of natural convection in a vertical dielectric couple stress fluid layer in the presence of a horizontal AC electric field. Appl. Math. Model. 2016, 40, 5462–5481. [Google Scholar] [CrossRef]
- Shankar, B.M.; Shivakumara, I.S. Magnetohydrodynamic instability of mixed convection in a differentially heated vertical channel. Appl. Math. Comput. 2018, 321, 752–767. [Google Scholar] [CrossRef]
- Shankar, B.M.; Kumar, J.; Shivakumara, I.S.; Kumar, S.B.N. MHD instability of pressure-driven flow of a non-Newtonian fluid. SN Appl. Sci. 2019, 1. [Google Scholar] [CrossRef]
- Hayat, T.; Akram, J.; Alsaedi, A.; Zahir, H. Endoscopy and homogeneous-heterogeneous reactions in MHD radiative peristaltic activity of Ree-Eyring fluid. Results Phys. 2018, 8, 481–488. [Google Scholar] [CrossRef]
- Baranovskii, E.S. Optimal Control for Steady Flows of the Jeffreys Fluids with Slip Boundary Condition. J. Appl. Ind. Math. 2014, 8, 2168–2176. [Google Scholar] [CrossRef]
- Vorotnikov, D.A. On the existence of weak stationary solutions of a boundary value problem in the Jeffreys model of the motion of a viscoelastic medium. Izv. Vyssh. Uchebn. Zaved. Mat. 2004, 9, 13–17. [Google Scholar]
- Moler, C.B.; Stewart, W. An algorithm for generalized matrix eigenvalue problems. SIAM J. Numer. Anal. 1973, I0, 241–256. [Google Scholar] [CrossRef]



| N | Le = 0.5, Pr = 0.7, λ1 = 0.6, RN = 100, Rn = 1, pb = 1, S = 1.2, RM = 100, NA = 1, NB = 0.5, λ = 0.2 | N | Le = 2, Pr = 0.7, λ1 = 0.6, RN = 100, Rn = 1, pb = 1, S = 1.2, RM = 100, NA = 1, NB = 0.5, λ = 0.2 | N | Le = 0.5, Pr = 1, λ1 = 0.6, RN = 100, Rn = 1, pb = 1, S = 1.2, RM = 100, NA = 1, NB = 0.5, λ = 0.2 | ||||||
| ac | RAc | cc | ac | RAc | cc | ac | RAc | cc | |||
| 5 | 1.3926 | 307.6482 | 14.6398 | 5 | 1.4268 | 270.8822 | 10.0793 | 5 | 1.2421 | 407.6655 | 21.5326 |
| 10 | 0.8509 | 523.6534 | 43.4964 | 10 | 0.9427 | 450.7811 | 31.1460 | 10 | 0.6363 | 951.5356 | 77.2332 |
| 15 | 0.8560 | 518.1818 | 42.7893 | 15 | 0.9459 | 447.8552 | 30.8200 | 15 | 0.6406 | 939.3423 | 76.0186 |
| 20 | 0.8560 | 518.1818 | 42.7893 | 20 | 0.9459 | 447.8554 | 30.8200 | 20 | 0.6406 | 939.3429 | 76.0186 |
| N | Le = 0.5, Pr = 0.7, λ1 = 0.9, RN = 100, Rn = 1, pb = 1, S = 1.2, RM = 100, NA = 1, NB = 0.5, λ = 0.2 | N | Le = 0.5, Pr = 0.7, λ1 = 0.6, RN = 500, Rn = 1, pb = 1,S = 1.2, RM = 100, NA = 1, NB = 0.5, λ = 0.2 | N | Le = 0.5, Pr = 0.7, λ1 = 0.6, RN = 100, Rn = 10, pb = 1, S = 1.2, RM = 100, NA = 1, NB = 0.5, λ = 0.2 | ||||||
| ac | RAc | cc | ac | RAc | cc | ac | RAc | cc | |||
| 5 | 1.4921 | 243.9072 | 6.9705 | 5 | 5 | 1.3611 | 310.9509 | 6.2310 | |||
| 10 | 1.1481 | 314.4263 | 19.9220 | 10 | 0.7326 | 964.5307 | 78.7104 | 10 | 1.0310 | 452.4231 | 23.0459 |
| 15 | 1.1503 | 313.5978 | 19.7783 | 15 | 0.7398 | 952.9166 | 77.2331 | 15 | 1.0310 | 452.1279 | 23.0146 |
| 20 | 1.1503 | 313.5976 | 19.7783 | 20 | 0.7398 | 952.9190 | 77.2334 | 20 | 1.0310 | 452.1278 | 23.0146 |
| 25 | 1.1503 | 313.5976 | 19.7783 | 25 | 0.7398 | 952.9190 | 77.2334 | 25 | 1.0310 | 452.1278 | 23.0146 |
| N | Le = 0.5, Pr = 0.7, λ1 = 0.6, RN = 100, Rn = 1, pb = 10, S = 1.2, RM = 100, NA = 1, NB = 0.5, λ = 0.2 | N | Le = 0.5, Pr = 0.7, λ1 = 0.6, RN = 100, Rn = 1, pb = 1, S = 1.8, RM = 100, NA = 1, NB = 0.5, λ = 0.2 | N | Le = 0.5, Pr = 0.7, λ1 = 0.6, RN = 100, Rn = 1, pb = 1, S = 1.2, RM = 1000, NA = 1, NB = 0.5, λ = 0.2 | ||||||
| ac | RAc | cc | ac | RAc | cc | ac | RAc | cc | |||
| 5 | 1.4068 | 304.3978 | 12.0650 | 5 | 1.3983 | 304.2107 | 15.5356 | 5 | 1.2541 | 321.5724 | −191.9384 |
| 10 | 0.8841 | 504.0515 | 38.9607 | 10 | 0.8459 | 522.6236 | 44.6216 | 10 | 0.5626 | 866.0277 | −107.9221 |
| 15 | 0.8892 | 499.2325 | 38.3358 | 15 | 0.8512 | 516.8656 | 43.8780 | 15 | 0.5620 | 860.8959 | −108.6310 |
| 20 | 0.8892 | 499.2324 | 38.3357 | 20 | 0.8512 | 516.8657 | 43.8780 | 20 | 0.5620 | 860.8961 | −108.6310 |
| 25 | 0.8892 | 499.2324 | 38.3357 | 25 | 0.8512 | 516.8657 | 43.8780 | 25 | 0.5620 | 860.8961 | −108.6310 |
| N | Le = 0.5, Pr = 0.7, λ1 = 0.6, RN = 100, Rn = 1, pb = 1, S = 1.2, RM = 100, NA = 4, NB = 0.5, λ = 0.2 | N | Le = 0.5, Pr = 0.7, λ1 = 0.6, RN = 100, Rn = 1, pb = 1, S = 1.2, RM = 100, NA = 1, NB = 2, λ = 0.2 | N | Le = 0.5, Pr = 0.7, λ1 = 0.6, RN = 100, Rn = 1, pb = 1, S = 1.2, RM = 100, NA = 1, NB = 0.5, λ = 0.5 | ||||||
| ac | RAc | cc | ac | RAc | cc | ac | RAc | cc | |||
| 5 | 1.4197 | 319.2867 | 54.0587 | 5 | 1.4211 | 314.4287 | 49.6449 | 5 | 1.1724 | 313.0687 | 8.2348 |
| 10 | 0.6824 | 624.9957 | 102.1356 | 10 | 0.6690 | 631.3779 | 111.7336 | 10 | 0.4296 | 1115.9144 | 64.6064 |
| 15 | 0.6910 | 610.2450 | 99.7242 | 15 | 0.6788 | 615.2087 | 108.4975 | 15 | 0.4349 | 1091.6271 | 62.9311 |
| 20 | 0.6910 | 610.2486 | 99.7248 | 20 | 0.6788 | 615.2125 | 108.4983 | 20 | 0.4349 | 1091.6281 | 62.9311 |
| 25 | 0.6910 | 610.2486 | 99.7248 | 25 | 0.6788 | 615.2125 | 108.4983 | 25 | 0.4349 | 1091.6281 | 62.9311 |
| Newtonian Fluid | Second-Grade Fluid , | Jeffreys Fluid , | |||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 0.5 | 0.7 | 100 | 1 | 1 | 1.2 | 100 | 1 | 0.5 | 0.9940 | 10,392.65 | 864.5781 | 0.9951 | 4642.71 | 567.1697 | 0.7941 | 618.43 | 50.7498 |
| 1 | 0.9573 | 14,132.58 | 1013.3955 | 0.9574 | 6323.88 | 668.7228 | 0.8515 | 571.93 | 39.9794 | ||||||||
| 2 | 0.9356 | 16,907.73 | 1116.3420 | 0.9361 | 7509.17 | 735.4287 | 0.8669 | 545.40 | 38.0532 | ||||||||
| 0.8 | 0.9903 | 12,242.60 | 1004.9593 | 0.9910 | 5473.60 | 661.5412 | 0.7147 | 768.49 | 62.3736 | ||||||||
| 1 | 0.9839 | 16,135.24 | 1293.1352 | 0.9840 | 7220.91 | 855.1587 | 0.5998 | 1140.61 | 86.9350 | ||||||||
| 200 | 0.9958 | 10,327.92 | 857.7339 | 0.9994 | 4575.61 | 556.5940 | 0.7624 | 732.89 | 59.1921 | ||||||||
| 500 | 1.0016 | 10,129.66 | 836.8116 | 1.0136 | 4363.20 | 523.3194 | 0.7051 | 1051.41 | 81.5126 | ||||||||
| 5 | 0.9460 | 15,471.22 | 1062.3798 | 0.9459 | 6956.57 | 703.5628 | 0.8945 | 557.05 | 34.2217 | ||||||||
| 10 | 0.9308 | 17,573.54 | 1138.3208 | 0.9300 | 7933.93 | 757.1569 | 0.9252 | 547.32 | 30.7248 | ||||||||
| 5 | 0.9940 | 10,397.03 | 864.4272 | 0.9950 | 4646.95 | 566.9162 | 0.8037 | 610.80 | 49.0667 | ||||||||
| 10 | 0.9940 | 10,402.49 | 864.2481 | 0.9950 | 4652.24 | 566.6301 | 0.8167 | 600.86 | 46.9125 | ||||||||
| 1.4 | 0.9941 | 10,383.78 | 864.2200 | 0.9954 | 4633.65 | 566.6079 | 0.7930 | 617.82 | 51.0560 | ||||||||
| 1.8 | 0.9943 | 10,366.04 | 863.5012 | 0.9958 | 4615.51 | 565.4802 | 0.7910 | 616.59 | 51.6657 | ||||||||
| 500 | 0.9916 | 10,816.78 | 848.7251 | 0.9903 | 5027.08 | 539.2726 | 0.8512 | 606.53 | −31.9050 | ||||||||
| 1000 | 0.9889 | 11,299.04 | 825.7010 | 0.9898 | 5285.42 | 481.5624 | 0.5889 | 915.59 | −91.8284 | ||||||||
| 2 | 1.0242 | 8002.73 | 760.9271 | 1.0286 | 3507.08 | 491.9218 | 0.7345 | 660.37 | 67.5225 | ||||||||
| 4 | 1.0717 | 5191.24 | 625.8271 | 1.0863 | 2112.73 | 387.4967 | 0.6563 | 717.14 | 106.3018 | ||||||||
| 1 | 1.0469 | 6534.64 | 692.7507 | 1.0517 | 2870.97 | 446.3826 | 0.7163 | 672.46 | 74.3268 | ||||||||
| 2 | 1.1094 | 3549.30 | 536.7460 | 1.1218 | 1505.72 | 334.9832 | 0.6405 | 726.35 | 118.5282 | ||||||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mekheimer, K.S.; Shankar, B.M.; Ramadan, S.F.; Mallik, H.E.; Mohamed, M.S. On the Stability of Convection in a Non-Newtonian Vertical Fluid Layer in the Presence of Gold Nanoparticles: Drug Agent for Thermotherapy. Mathematics 2021, 9, 1302. https://doi.org/10.3390/math9111302
Mekheimer KS, Shankar BM, Ramadan SF, Mallik HE, Mohamed MS. On the Stability of Convection in a Non-Newtonian Vertical Fluid Layer in the Presence of Gold Nanoparticles: Drug Agent for Thermotherapy. Mathematics. 2021; 9(11):1302. https://doi.org/10.3390/math9111302
Chicago/Turabian StyleMekheimer, Khaled S., Bangalore M. Shankar, Shaimaa F. Ramadan, Hosahalli E. Mallik, and Mohamed S. Mohamed. 2021. "On the Stability of Convection in a Non-Newtonian Vertical Fluid Layer in the Presence of Gold Nanoparticles: Drug Agent for Thermotherapy" Mathematics 9, no. 11: 1302. https://doi.org/10.3390/math9111302
APA StyleMekheimer, K. S., Shankar, B. M., Ramadan, S. F., Mallik, H. E., & Mohamed, M. S. (2021). On the Stability of Convection in a Non-Newtonian Vertical Fluid Layer in the Presence of Gold Nanoparticles: Drug Agent for Thermotherapy. Mathematics, 9(11), 1302. https://doi.org/10.3390/math9111302







