A Review of and Some Results for Ollivier–Ricci Network Curvature
Abstract
1. Introduction
Basic Notations and Terminologies
- ▷
- For a node , denotes the set of neighbors of v, and denotes the degree of v.
- ▷
- (or simply ) denote the distance (i.e., number of edges in a shortest path) between the nodes u and v in G.
2. Ollivier–Ricci Curvature: Motivation, Definition and Illustration
- ►
- Let be the set of nodes .
- ►
- Let the probability distributions and be uniform distributions (If the given graph is non-negative node weights then another option is to normalize the restrictions of these node weights to the sub-graph and use them for the distributions and .) and , respectively, over the nodes in and , respectively, i.e.,
- ►
- Remembering that for an edge , we can then define the course Ricci curvature as (cf. [20] (Definition 3)):The measure can easily be extended for graphs with non-negative edge weights; redefine to be minimum total weight over all possible paths between u and v and use the equation:
An Illustration of Computing the Value of Ric For a Two-dimensional Grid
3. Exact and Approximate Computation of Ric
- ▷
- ▷
- Solve an with variables and constraints via standard solvers such as the interior-point method. Alternatively, the can be solved by minimum-cost network flow algorithms by viewing it as a transportation problem, e.g., see [30].
| ⋯ | ⋯ | u | ⋯ | v | |||||||
| ⋯ | ⋯ | 0 | ⋯ | 0 | |||||||
| ⋯ | 0 | ⋯ | ⋯ |
4. Review of Efficient Approximate Computation of Ric via Linear Sketching
There exists a distribution (“q-dimensional sketch”) over linear maps from where and a “post-processing” function such that for any with polynomially-bounded entries, it holds that
5. Discussion
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| Emd | Earth Mover’s Distance |
| Ric | Ricci curvature |
References
- Berger, M. A Panoramic View of Riemannian Geometry; Springer: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Bridson, M.R.; Haefliger, A. Metric Spaces of Non-Positive Curvature; Springer: Berlin/Heidelberg, Germany, 1999. [Google Scholar]
- Schutz, B.F. A First Course in General Relativity; Cambridge University Press: Cambridge, UK, 1990. [Google Scholar]
- Gromov, M. Hyperbolic groups. Essays Group Theory 1987, 8, 75–263. [Google Scholar]
- Benjamini, I. Expanders are not hyperbolic. Isr. J. Math. 1998, 108, 33–36. [Google Scholar] [CrossRef]
- Chalopin, J.; Chepoi, V.; Dragan, F.F.; Ducoffe, G.; Mohammed, A.; Vaxès, Y. Fast approximation and exact computation of negative curvature parameters of graphs. In Proceedings of the 34th International Symposium on Computational Geometry, Budapest, Hungary, 11–14 June 2018. [Google Scholar]
- Chepoi, V.; Dragan, F.F.; Estellon, B.; Habib, M.; Vaxès, Y. Diameters, centers, and approximating trees of δ-hyperbolic geodesic spaces and graphs. In Proceedings of the 24th Annual Symposium on Computational Geometry, College Park, MD, USA, 9–11 June 2008; pp. 59–68. [Google Scholar]
- DasGupta, B.; Janardhanan, M.V.; Yahyanejad, F. How did the shape of your network change? (On detecting network anomalies via non-local curvatures). Algorithmica 2020, 82, 1741–1783. [Google Scholar] [CrossRef]
- DasGupta, B.; Karpinski, M.; Mobasheri, N.; Yahyanejad, F. Effect of Gromov-hyperbolicity Parameter on Cuts and Expansions in Graphs and Some Algorithmic Implications. Algorithmica 2018, 80, 772–800. [Google Scholar] [CrossRef]
- Fournier, H.; Ismail, A.; Vigneron, A. Computing the Gromov hyperbolicity of a discrete metric space. Inf. Process. Lett. 2015, 115, 576–579. [Google Scholar] [CrossRef]
- Albert, R.; DasGupta, B.; Mobasheri, N. Topological implications of negative curvature for biological and social networks. Phys. Rev. E 2014, 89, 032811. [Google Scholar] [CrossRef] [PubMed]
- Jonckheere, E.; Lou, M.; Bonahon, F.; Baryshnikov, Y. Euclidean versus hyperbolic congestion in idealized versus experimental networks. Internet Math. 2011, 7, 1–27. [Google Scholar] [CrossRef]
- Papadopoulos, F.; Krioukov, D.; Boguna, M.; Vahdat, A. Greedy Forwarding in Dynamic Scale-Free Networks Embedded in Hyperbolic Metric Spaces. In Proceedings of the IEEE Conference on Computer Communications, San Diego, CA, USA, 15–19 March 2010; pp. 1–9. [Google Scholar]
- Forman, R. Bochner’s method for cell complexes and combinatorial ricci curvature. Discret. Comput. Geom. 2003, 29, 323–374. [Google Scholar] [CrossRef]
- Samal, A.; Sreejith, R.P.; Gu, J.; Liu, S.; Saucan, E.; Jost, J. Comparative analysis of two discretizations of Ricci curvature for complex networks. Sci. Rep. 2018, 8, 8650. [Google Scholar] [CrossRef] [PubMed]
- Sreejith, R.P.; Jost, J.; Saucan, E.; Samal, A. Systematic evaluation of a new combinatorial curvature for complex networks. Chaos Solitons Fractals 2017, 101, 50–67. [Google Scholar] [CrossRef]
- Sreejith, R.P.; Mohanraj, K.; Jost, J.; Saucan, E.; Samal, A. Forman curvature for complex networks. J. Stat. Mech. Theory Exp. 2016, 2016, 063206. [Google Scholar] [CrossRef]
- Weber, M.; Saucan, E.; Jost, J. Characterizing complex networks with Forman-Ricci curvature and associated geometric flows. J. Complex Netw. 2017, 5, 527–550. [Google Scholar] [CrossRef]
- Ollivier, Y. Ricci curvature of metric spaces. Comptes Rendus Math. 2007, 345, 643–646. [Google Scholar] [CrossRef]
- Ollivier, Y. Ricci curvature of Markov chains on metric spaces. J. Funct. Anal. 2009, 256, 810–864. [Google Scholar] [CrossRef]
- Ollivier, Y. A survey of Ricci curvature for metric spaces and Markov chains. In Probabilistic Approach to Geometry; Kotani, M., Hino, M., Kumagai, T., Eds.; Mathematical Society of Japan: Tokyo, Japan, 2010; pp. 343–381. [Google Scholar]
- Ollivier, Y. A visual introduction to Riemannian curvatures and some discrete generalizations. In Analysis and Geometry of Metric Measure Spaces; Lecture Notes of the 50th Séminaire de Mathématiques Supérieures, Montréal, 2011; Dafni, G., McCann, R.J., Stancu, A., Eds.; American Mathematical Society: Providence, RI, USA, 2013; pp. 197–219. [Google Scholar]
- Chow, B.; Luo, F. Combinatorial Ricci flows on surfaces. J. Differ. Geom. 2003, 63, 97–129. [Google Scholar] [CrossRef]
- Mallows, L. A note on asymptotic joint normality. Ann. Math. Stat. 1972, 43, 508–515. [Google Scholar] [CrossRef]
- Rubner, Y.; Tomasi, C.; Guibas, L.J. A metric for distributions with applications to image databases. In Proceedings of the 6th International Conference on Computer Vision, Bombay, India, 4–7 January 1998; pp. 59–66. [Google Scholar]
- Rubner, Y.; Tomasi, C.; Guibas, L.J. The earth mover’s distance as a metric for image retrieval. Int. J. Comput. Vis. 2000, 40, 99–121. [Google Scholar] [CrossRef]
- Villani, C. Topics in optimal transportation. In Graduate Studies in Mathematics; American Mathematical Society: Providence, RI, USA, 2003; p. 58. [Google Scholar]
- Seidel, R. On the All-Pairs-Shortest-Path Problem in Unweighted Undirected Graphs. J. Comput. Syst. Sci. 1995, 51, 400–403. [Google Scholar] [CrossRef]
- Williams, V.V. Multiplying matrices faster than Coppersmith-Winograd. In Proceedings of the 44th ACM Symposium on Theory of Computing, New York, NY, USA, 20–22 May 2012; pp. 887–898. [Google Scholar]
- Ahuja, R.K.; Magnanti, T.L.; Orlin, J.B. Network Flows: Theory, Algorithms, and Applications; Prentice-Hall, Inc.: Upper Saddle River, NJ, USA, 1993. [Google Scholar]
- Cormode, G.; Garofalakis, M.N.; Haas, P.J.; Jermaine, C. Synopses for massive data: Samples, histogram, wavelets, sketches. Found. Trends Databases 2012, 4, 1–294. [Google Scholar] [CrossRef]
- Andoni, A.; Ba, K.D.; Indyk, P.; Woodruff, D.P. Efficient sketches for earth-mover distance, with applications. In Proceedings of the 50 thAnnual IEEE Symposium on Foundations of Computer Science, Atlanta, GA, USA, 25–27 October 2009; pp. 324–330. [Google Scholar]
- Brody, J.; Liang, H.; Sun, X. Space-efficient approximation scheme for circular earth mover distance. In LATIN 2012; Fernández-Baca, D., Ed.; Lecture Notes in Computer Science; Springer: Berlin/Heidelberg, Germany, 2012; Volume 7256, pp. 97–108. [Google Scholar]
- Indyk, P. A near linear time constant factor approximation for euclidean bichromatic matching (cost). In Proceedings of the 18th annual ACM-SIAM symposium on Discrete algorithms, New Orleans, LA, USA, 7–9 January 2007; pp. 39–42. [Google Scholar]
- Indyk, P.; Price, E. K-median clustering, model-based compressive sensing, and sparse recovery for earth mover distance. In Proceedings of the 43rd Annual ACM Symposium on Theory of Computing, San Jose, CA, USA, 6–8 June 2011; pp. 627–636. [Google Scholar]
- Verbin, E.; Zhang, Q. Rademacher-sketch: A dimensionality-reducing embedding for sum-product norms, with an application to earth-mover distance. In Proceedings of the International Colloquium on Automata, Languages, and Programming, Warwick, UK, 9–13 July 2012; pp. 834–845. [Google Scholar]
- McGregor, A.; Stubbs, D. Sketching earth-mover distance on graph metrics. In International Workshop on Approximation Algorithms for Combinatorial Optimization; Springer: Berlin/Heidelberg, Germany, 2013; pp. 274–286. [Google Scholar]
- Kane, D.M.; Nelson, J.; Porat, E.; Woodruff, D.P. Fast moment estimation in data streams in optimal space. In Proceedings of the 43rd annual ACM symposium on Theory of computing, San Jose, CA, USA, 6–8 June 2011; pp. 745–754. [Google Scholar]
- Clarkson, K.L.; Drineas, P.; Magdon-Ismail, M.; Mahoney, M.W.; Meng, X.; Woodruff, D.P. The fast cauchy transform and faster robust linear regression. In Proceedings of the 24th Annual ACM-SIAM Symposium on Discrete Algorithms, New Orleans, LA, USA, 6–8 January 2013; pp. 466–477. [Google Scholar]
- Kane, D.M.; Nelson, J.; Porat, E.; Woodruff, D.P. Subspace embeddings for the ℓ1-norm with applications. In Proceedings of the 43rd Annual ACM Symposium on Theory of Computing, San Jose, CA, USA, 6–8 June 2011; pp. 755–764. [Google Scholar]
- Bubley, R.; Dyer, M.E. Path coupling: A technique for proving rapid mixing in Markov chains. In Proceedings of the 38th Annual Symposium on Foundations of Computer Science, Miami Beach, FL, USA, 20–22 October 1997; pp. 223–231. [Google Scholar]
- Gerin, L. Random sampling of lattice paths with constraints, via transportation. In Proceedings of the 21st International Meeting on Probabilistic, Combinatorial, and Asymptotic Methods in the Analysis of Algorithms, Vienna, Austria, 28 June–2 July 2010; pp. 317–328. [Google Scholar]
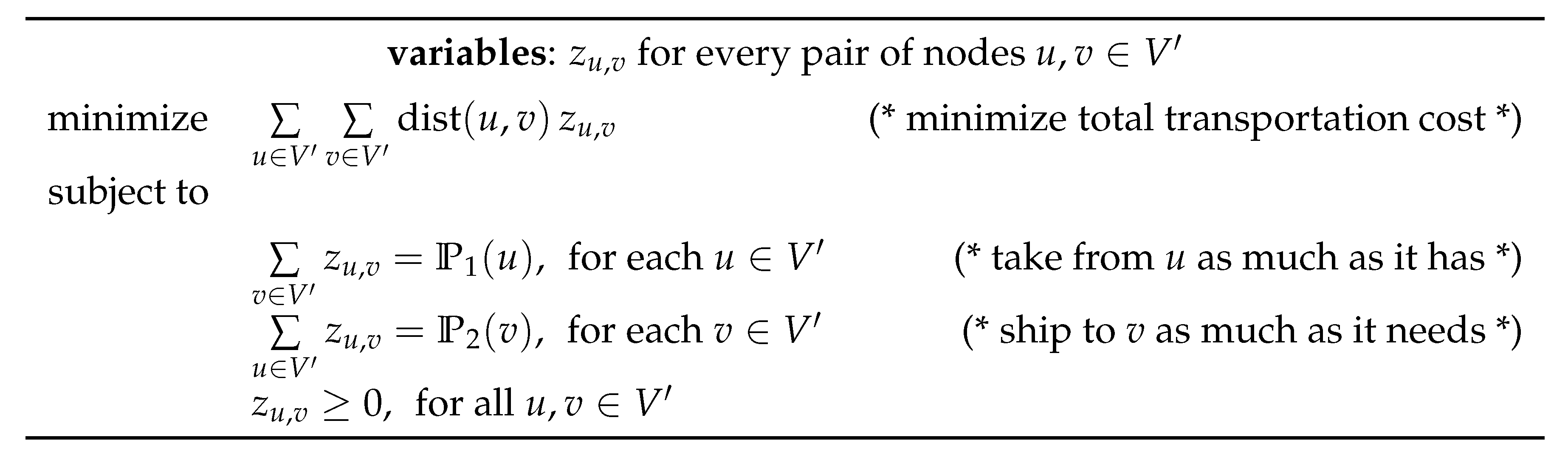


© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Azarhooshang, N.; Sengupta, P.; DasGupta, B. A Review of and Some Results for Ollivier–Ricci Network Curvature. Mathematics 2020, 8, 1416. https://doi.org/10.3390/math8091416
Azarhooshang N, Sengupta P, DasGupta B. A Review of and Some Results for Ollivier–Ricci Network Curvature. Mathematics. 2020; 8(9):1416. https://doi.org/10.3390/math8091416
Chicago/Turabian StyleAzarhooshang, Nazanin, Prithviraj Sengupta, and Bhaskar DasGupta. 2020. "A Review of and Some Results for Ollivier–Ricci Network Curvature" Mathematics 8, no. 9: 1416. https://doi.org/10.3390/math8091416
APA StyleAzarhooshang, N., Sengupta, P., & DasGupta, B. (2020). A Review of and Some Results for Ollivier–Ricci Network Curvature. Mathematics, 8(9), 1416. https://doi.org/10.3390/math8091416




