The Strong Resolving Graph and the Strong Metric Dimension of Cactus Graphs
Abstract
:1. Introduction
1.1. Strong Metric Dimension of Graphs
- (a)
- if and only if .
- (b)
- If , then .
- (c)
- if and only if .
- (d)
- If , then .
- (e)
- If G is a tree with l leaves, .
1.2. Strong Resolving Graph of a Graph
- (a)
- If equals the set of simplicial vertices of G, then . In particular, and for any tree T, .
- (b)
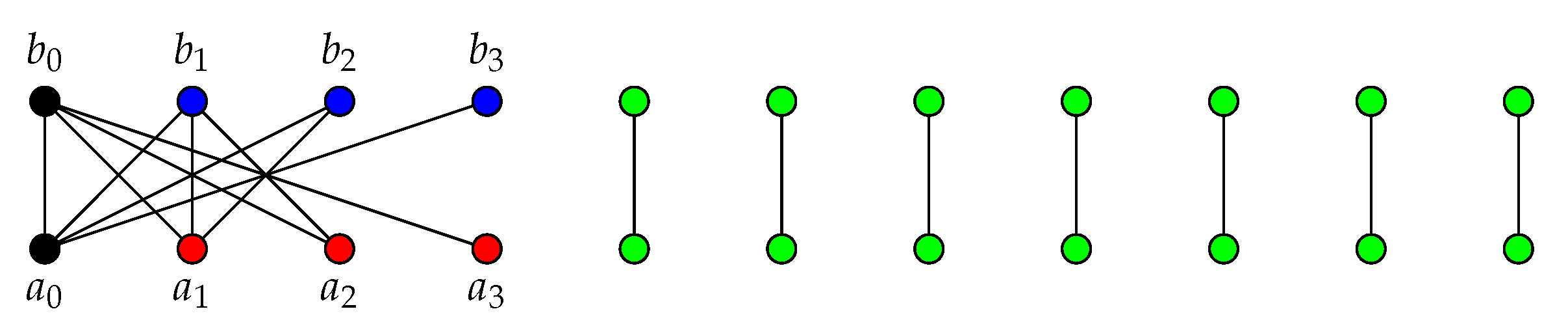
- For any 2-antipodal graph G of order n, . In particular, .
- (c)
- For odd cycles .
- (d)
- For any complete k-partite graph such that , , .
- Realization Problem. Determine which graphs have a given graph as their strong resolving graphs.
- Characterization Problem. Characterize those graphs that are strong resolving graphs of some graphs.
1.3. Strong Metric Dimension of G versus Vertex Cover Number of
2. Cactus Graphs: General Issues
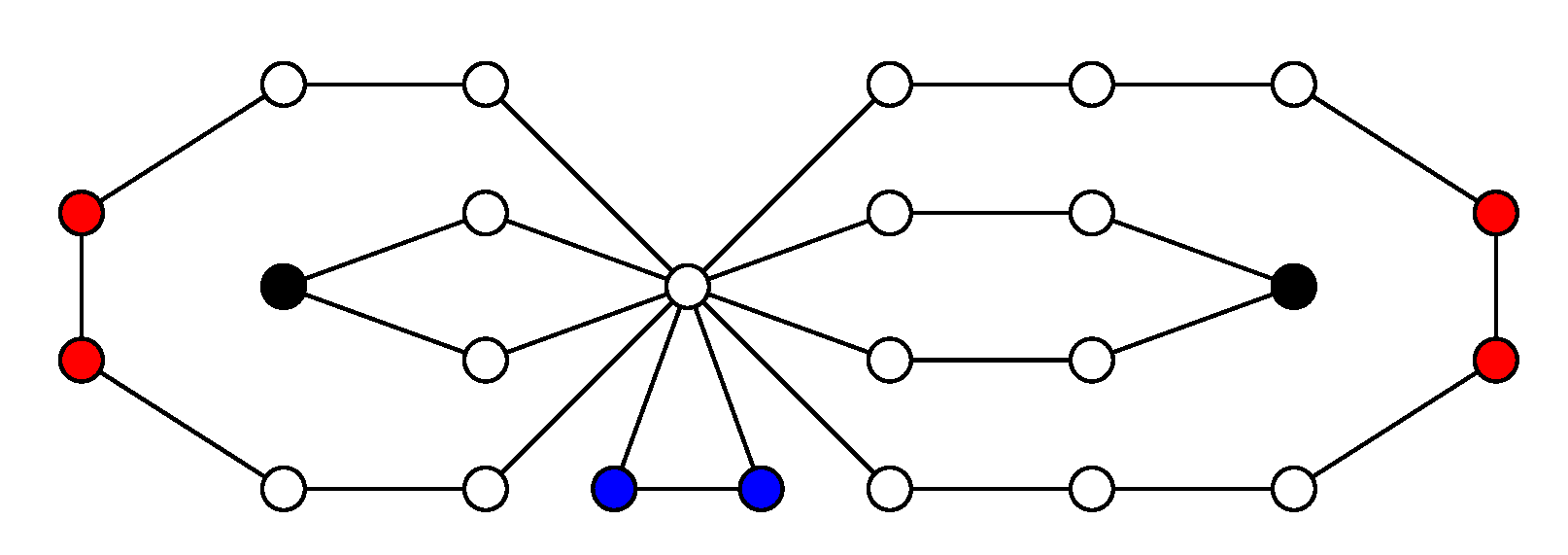
3. Strong Resolving Graphs
3.1. Unicyclic Graphs
- w is a terminal vertex of a vertex u of such that are diametral vertices in .
- w is a diametral vertex with v in and .
for r even.
- The set forms a clique in and each vertex of has at most one neighbor in .
- If are diametral vertices in , then is a connected component of isomorphic to .
- If are diametral vertices in , and , then forms a subgraph of isomorphic to and .
for r odd.
- The set forms a clique in and each vertex of has at most two neighbors in .
- Let and let being diametral vertices with u in .
- –
- If , then is a subgraph of isomorphic to , and for every , .
- –
- If , then is a subgraph of isomorphic to , and for every , for (notice that if , then ).
- –
- If and , then the set form a subgraph (not induced) (Notice that the vertices are adjacent between them in .) of isomorphic to a star graph with central vertex u, , and for every , .
3.2. Bouquet of Cycles
- The set of vertices of each odd cycle , , which are different from w induces a path of order , in , whose leaves are the two vertices that are diametral with w.
- The set of vertices of each cycle , , which are not diametral with w induces a graph isomorphic to the disjoint union of complete graphs in .
- Every three vertices such that , and are pairwise adjacent.
3.3. Chains of Even Cycles
- We begin with straight chains of even cycles, say , satisfying that the last cycle of the straight chain is isomorphic to the first cycle of the straight chain for every .
- Assume that the last cycle of each straight chain is , for every . By the item above, this (in ) is isomorphic to the first cycle of the straight chain with .
- Assume also that the terminal vertices of each straight chain are , for every .
- To construct our chain of even cycles , for every , we identify the last cycle of with the first cycle of (that are isomorphic) as follows. Every vertex of is identified with the vertex for some and every (operations with the subindex of v are done modulo r).
- The terminal vertex of the straight chain is MMD with every vertex of the straight chain .
- The terminal vertex of the straight chain is MMD with every vertex of the straight chain .
- In any cycle of F, any pair of diametral (in the cycle) vertices being not cut nor terminal vertices of F are MMD.
- The set of vertices and , with , forms a component of the graph isomorphic to a bipartite graph .
- In each cycle of F, each pair of diametral vertices in the cycle, not including terminal nor cut vertices, induces a graph isomorphic to in .
4. The Strong Metric Dimension
4.1. Unicyclic Graphs
- (i)
- for every x of .
- (ii)
- There is at most one pair of diametral vertices in each one having one terminal vertex.
4.2. Bouquet of Cycles
4.3. Chains of Even Cycles
5. Concluding Remarks
- Describe the structure of the strong resolving graphs of some classes of cactus graphs, and compute the strong metric dimension of the graphs in such families.
- Apply the results concerning the descriptions of the strong resolving graphs of the graphs given in the work to other problems, like for instance computing the strong partition dimension (see [4]) of such graphs.
- Continue the lines of this study for other more general families that cactus graphs. This could include for instance, planar graphs or chordal graphs.
Funding
Conflicts of Interest
References
- Kelenc, A.; Tratnik, N.; Yero, I.G. Uniquely identifying the edges of a graph: The edge metric dimension. Discret. Appl. Math. 2018, 251, 204–220. [Google Scholar] [CrossRef] [Green Version]
- Kelenc, A.; Kuziak, D.; Taranenko, A.; Yero, I.G. Mixed metric dimension of graph. Appl. Math. Comput. 2017, 314, 429–438. [Google Scholar]
- Trujillo-Rasúa, R.; Yero, I.G. k-metric antidimension: A privacy measure for social graphs. Inform. Sci. 2016, 328, 403–417. [Google Scholar] [CrossRef] [Green Version]
- Yero, I.G. On the strong partition dimension of graphs. Electron. J. Combin. 2014, 21, P3.14. [Google Scholar]
- Gil-Pons, R.; Ramírez-Cruz, Y.; Trujillo-Rasua, R.; Yero, I.G. Distance-based vertex identification in graphs: The outer multiset dimension. Appl. Math. Comput. 2019, 363, 124612. [Google Scholar] [CrossRef]
- Simanjuntak, R.; Siagian, P.; Vetrik, T. The multiset dimension of graphs. arXiv 2019, arXiv:1711.00225v2. [Google Scholar]
- Oellermann, O.R.; Peters-Fransen, J. The strong metric dimension of graphs and digraphs. Discret. Appl. Math. 2007, 155, 356–364. [Google Scholar] [CrossRef] [Green Version]
- Kuziak, D. Strong Resolvability in Product Graphs. Ph.D. Thesis, Universitat Rovira i Virgili, Catalonia, Spain, 2014. [Google Scholar]
- Kuziak, D.; Puertas, M.L.; Rodríguez-Velázquez, J.A.; Yero, I.G. Strong resolving graphs: The realization and the characterization problems. Discret. Appl. Math. 2018, 236, 270–287. [Google Scholar] [CrossRef] [Green Version]
- Lenin, R. A short note on: There is no graph G with GSR ≅ Kr,s, r, s ≥ 2. Discrete Appl. Math. 2019, 265, 204–205. [Google Scholar] [CrossRef]
- Kuziak, D.; Yero, I.G.; Rodríguez-Velázquez, J.A. On the strong metric dimension of corona product graphs and join graphs. Discrete Appl. Math. 2013, 161, 1022–1027. [Google Scholar] [CrossRef] [Green Version]
- Kuziak, D.; Yero, I.G.; Rodríguez-Velázquez, J.A. Strong metric dimension of rooted product graphs. Int. J. Comput. Math. 2016, 93, 1265–1280. [Google Scholar] [CrossRef] [Green Version]
- Yi, E. On strong metric dimension of graphs and their complements. Acta Math. Sin. (Engl. Ser.) 2013, 29, 1479–1492. [Google Scholar] [CrossRef]
- Brešar, B.; Klavžar, S.; Tepeh Horvat, A. On the geodetic number and related metric sets in Cartesian product graphs. Discret. Math. 2008, 308, 5555–5561. [Google Scholar] [CrossRef] [Green Version]
- Cáceres, J.; Puertas, M.L.; Hernando, C.; Mora, M.; Pelayo, I.M.; Seara, C. Searching for geodetic boundary vertex sets. Electron. Notes Discret. Math. 2005, 19, 25–31. [Google Scholar] [CrossRef]
- Rodríguez-Velázquez, J.A.; Yero, I.G.; Kuziak, D.; Oellermann, O.R. On the strong metric dimension of Cartesian and direct products of graphs. Discret. Math. 2014, 335, 8–19. [Google Scholar] [CrossRef]
- Kuziak, D.; Yero, I.G. Further new results on strong resolving partitions for graphs. Open Math. 2020. to appear. [Google Scholar] [CrossRef]



© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kuziak, D. The Strong Resolving Graph and the Strong Metric Dimension of Cactus Graphs. Mathematics 2020, 8, 1266. https://doi.org/10.3390/math8081266
Kuziak D. The Strong Resolving Graph and the Strong Metric Dimension of Cactus Graphs. Mathematics. 2020; 8(8):1266. https://doi.org/10.3390/math8081266
Chicago/Turabian StyleKuziak, Dorota. 2020. "The Strong Resolving Graph and the Strong Metric Dimension of Cactus Graphs" Mathematics 8, no. 8: 1266. https://doi.org/10.3390/math8081266
APA StyleKuziak, D. (2020). The Strong Resolving Graph and the Strong Metric Dimension of Cactus Graphs. Mathematics, 8(8), 1266. https://doi.org/10.3390/math8081266




