Turbulence as a Network of Fourier Modes
Abstract
:1. Introduction
2. Fourier Space Formulation
- a finite number of nodes in space (the grid in the above example);
- a list of node to pair couplings to be determined by the triadic interaction condition;
- the coefficient of interaction for each of these couplings; and
- a set of field variables (e.g., ) to evolve on each node of the network using Equation (5).
2.1. Energy Transfer
2.2. Network Reduction
2.3. Transfer Rates
3. Spectral Reduction
3.1. Phase Dynamics: Synchronization vs. Random Phase
3.2. Beyond Spectral Reduction
4. Examples of Network Models
4.1. Nested Polyhedra Models
4.2. Predator–Prey Models
4.3. Food Webs
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Frisch, U. Turbulence: The Legacy of A. N. Kolmogorov; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Albert, R.; Barabási, A.L. Statistical mechanics of complex networks. Rev. Mod. Phys. 2002, 74, 47–97. [Google Scholar] [CrossRef] [Green Version]
- Watts, D.J.; Strogatz, S.H. Collective dynamics of small-world networks. Nature 1998, 393, 440–442. [Google Scholar] [CrossRef] [PubMed]
- Barabási, A.L.; Albert, R. Emergence of Scaling in Random Networks. Science 1999, 286, 509–512. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Arenas, A.; Díaz-Guilera, A.; Kurths, J.; Moreno, Y.; Zhou, C. Synchronization in complex networks. Phys. Rep. 2008, 469, 93–153. [Google Scholar] [CrossRef] [Green Version]
- Sipos, M.; Goldenfeld, N. Directed percolation describes lifetime and growth of turbulent puffs and slugs. Phys. Rev. E 2011, 84, 035304. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kaneko, K. Overview of coupled map lattices. Chaos Interdiscip. J. Nonlinear Sci. 1992, 2, 279–282. [Google Scholar] [CrossRef] [PubMed]
- Taira, K.; Nair, A.G.; Brunton, S.L. Network structure of two-dimensional decaying isotropic turbulence. J. Fluid Mech. 2016, 795, R2. [Google Scholar] [CrossRef] [Green Version]
- Kartashova, E. (Ed.) Nonlinear Resonance Analysis. In Nonlinear Resonance Analysis; Cambridge University Press: Cambridge, UK, 2010; Volume 1. [Google Scholar]
- Newell, A. (Ed.) Nonlinear Wave Motion. Lectures in Applied Mathematics; Number 15; American Mathematical Society: Providence, RI, USA, 1974. [Google Scholar]
- Zakharov, V.E.; Kuznetsov, E.A. Hamiltonian formalism for nonlinear waves. Physics-Uspekhi 1997, 40, 1087. [Google Scholar] [CrossRef]
- Newell, A.C.; Rumpf, B. Wave Turbulence. Annu. Rev. Fluid Mech. 2011, 43, 59–78. [Google Scholar] [CrossRef] [Green Version]
- Thorpe, S.A. On wave interactions in a stratified fluid. J. Fluid Mech. 1966, 24, 737–751. [Google Scholar] [CrossRef]
- Kim, W.; West, B.J. Chaotic properties of internal wave triad interactions. Phys. Fluids 1997, 9, 632–647. [Google Scholar] [CrossRef]
- Zakharov, V.E.; Korotkevich, A.O.; Pushkarev, A.; Resio, D. Coexistence of Weak and Strong Wave Turbulence in a Swell Propagation. Phys. Rev. Lett. 2007, 99, 164501. [Google Scholar] [CrossRef] [Green Version]
- Meyrand, R.; Kiyani, K.H.; Gürcan, Ö.D.; Galtier, S. Coexistence of Weak and Strong Wave Turbulence in Incompressible Hall Magnetohydrodynamics. Phys. Rev. X 2018, 8, 031066. [Google Scholar] [CrossRef] [Green Version]
- Furuichi, N.; Hibiya, T.; Niwa, Y. Bispectral Analysis of Energy Transfer within the Two-Dimensional Oceanic Internal Wave Field. J. Phys. Oceanogr. 2005, 35, 2104–2109. [Google Scholar] [CrossRef]
- Falcon, E.; Laroche, C.; Fauve, S. Observation of Gravity-Capillary Wave Turbulence. Phys. Rev. Lett. 2007, 98, 094503. [Google Scholar] [CrossRef] [Green Version]
- Connaughton, C.; Newell, A.C.; Pomeau, Y. Non-stationary spectra of local wave turbulence. Phys. D Nonlinear Phenom. 2003, 184, 64–85, Complexity and Nonlinearity in Physical Systems—A Special Issue to Honor Alan Newell. [Google Scholar] [CrossRef] [Green Version]
- Buchhave, P.; Velte, C.M. Dynamic triad interactions and evolving turbulence spectra. arXiv 2019, arXiv:1906.04756. [Google Scholar]
- Kartashova, E.A. Weakly nonlinear theory of finite-size effects in resonators. Phys. Rev. Lett. 1994, 72, 2013–2016. [Google Scholar] [CrossRef]
- Pires, C.A.L.; Perdig ao, R.A.P. Non-Gaussian interaction information: Estimation, optimization and diagnostic application of triadic wave resonance. Nonlinear Process. Geophys. 2015, 22, 87–108. [Google Scholar] [CrossRef] [Green Version]
- Lesieur, M. Turbulence in Fluids, 3rd ed.; Kluwer: Dordrecht, The Netherlands, 1997. [Google Scholar]
- Bowman, J.C.; Shadwick, B.A.; Morrison, P.J. Spectral Reduction: A Statistical Description of Turbulence. Phys. Rev. Lett. 1999, 83, 5491–5494. [Google Scholar] [CrossRef] [Green Version]
- Bowman, J.C.; Roberts, M. Pseudospectral reduction of incompressible two-dimensional turbulence. Commun. Nonlinear Sci. Numer. Simul. 2012, 17, 2008–2013. [Google Scholar] [CrossRef]
- Waleffe, F. Inertial transfers in the helical decomposition. Phys. Fluids A Fluid Dyn. 1993, 5, 677–685. [Google Scholar] [CrossRef]
- Biferale, L. Shell models of energy cascade in turbulence. Ann. Rev. Fluid Mech. 2003, 35, 441–468. [Google Scholar] [CrossRef] [Green Version]
- Ohkitani, K.; Yamada, M. Temporal Intermittency in the Energy Cascade Process and Local Lyapunov Analysis in Fully-Developed Model Turbulence. Prog. Theor. Phys. 1989, 81, 329–341. [Google Scholar] [CrossRef] [Green Version]
- L’vov, V.S.; Podivilov, E.; Pomyalov, A.; Procaccia, I.; Vandembroucq, D. Improved shell model of turbulence. Phys. Rev. E 1998, 58, 1811–1822. [Google Scholar] [CrossRef] [Green Version]
- Gürcan, Ö.D.; Xu, S.; Morel, P. Spiral chain models of two-dimensional turbulence. Phys. Rev. E 2019, 100, 043113. [Google Scholar] [CrossRef] [Green Version]
- Newman, M.E.J.; Watts, D.J. Scaling and percolation in the small-world network model. Phys. Rev. E 1999, 60, 7332–7342. [Google Scholar] [CrossRef] [Green Version]
- Gürcan, Ö.D. Nested polyhedra model of turbulence. Phys. Rev. E 2017, 95, 063102. [Google Scholar] [CrossRef] [Green Version]
- Gürcan, Ö.D. Nested polyhedra model of isotropic magnetohydrodynamic turbulence. Phys. Rev. E 2018, 97, 063111. [Google Scholar] [CrossRef] [Green Version]
- Gürcan, Ö.D. nestp3d. 2017. Available online: https://github.com/gurcani/nestp3d (accessed on 26 March 2020).

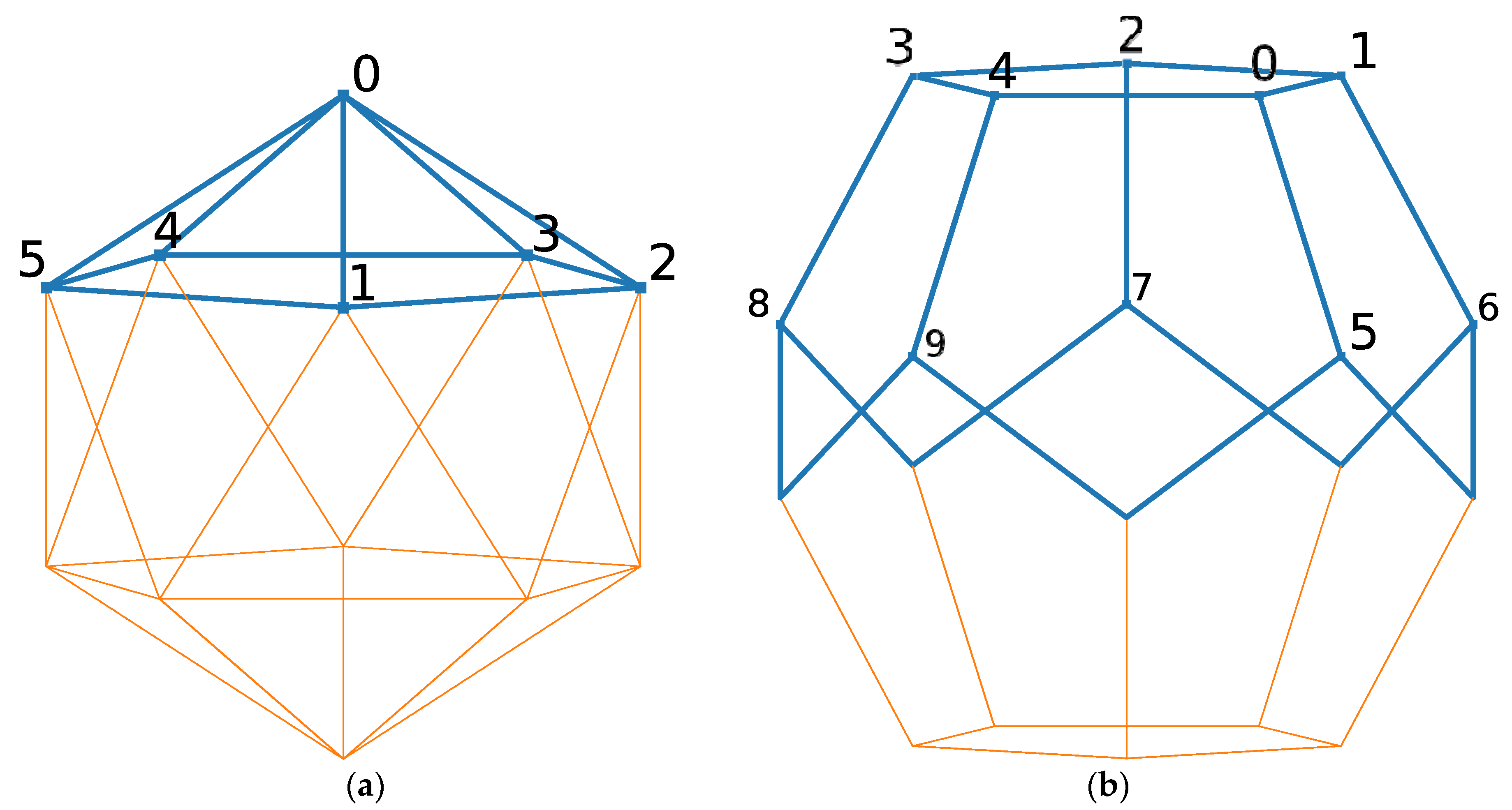
| 0 | |||
| 1 | |||
| 2 | |||
| 3 | |||
| 4 | |||
| 5 |
| 0 | |||
| 1 | |||
| 2 | |||
| 3 | |||
| 4 | |||
| 5 | |||
| 6 | |||
| 7 | |||
| 8 | |||
| 9 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gürcan, Ö.D.; Li, Y.; Morel, P. Turbulence as a Network of Fourier Modes. Mathematics 2020, 8, 530. https://doi.org/10.3390/math8040530
Gürcan ÖD, Li Y, Morel P. Turbulence as a Network of Fourier Modes. Mathematics. 2020; 8(4):530. https://doi.org/10.3390/math8040530
Chicago/Turabian StyleGürcan, Özgür. D., Yang Li, and Pierre Morel. 2020. "Turbulence as a Network of Fourier Modes" Mathematics 8, no. 4: 530. https://doi.org/10.3390/math8040530
APA StyleGürcan, Ö. D., Li, Y., & Morel, P. (2020). Turbulence as a Network of Fourier Modes. Mathematics, 8(4), 530. https://doi.org/10.3390/math8040530




