Analytical Wave Solutions for Foam and KdV-Burgers Equations Using Extended Homogeneous Balance Method
Abstract
:1. Introduction
2. Proposed Analytical Method
3. Applied Examples
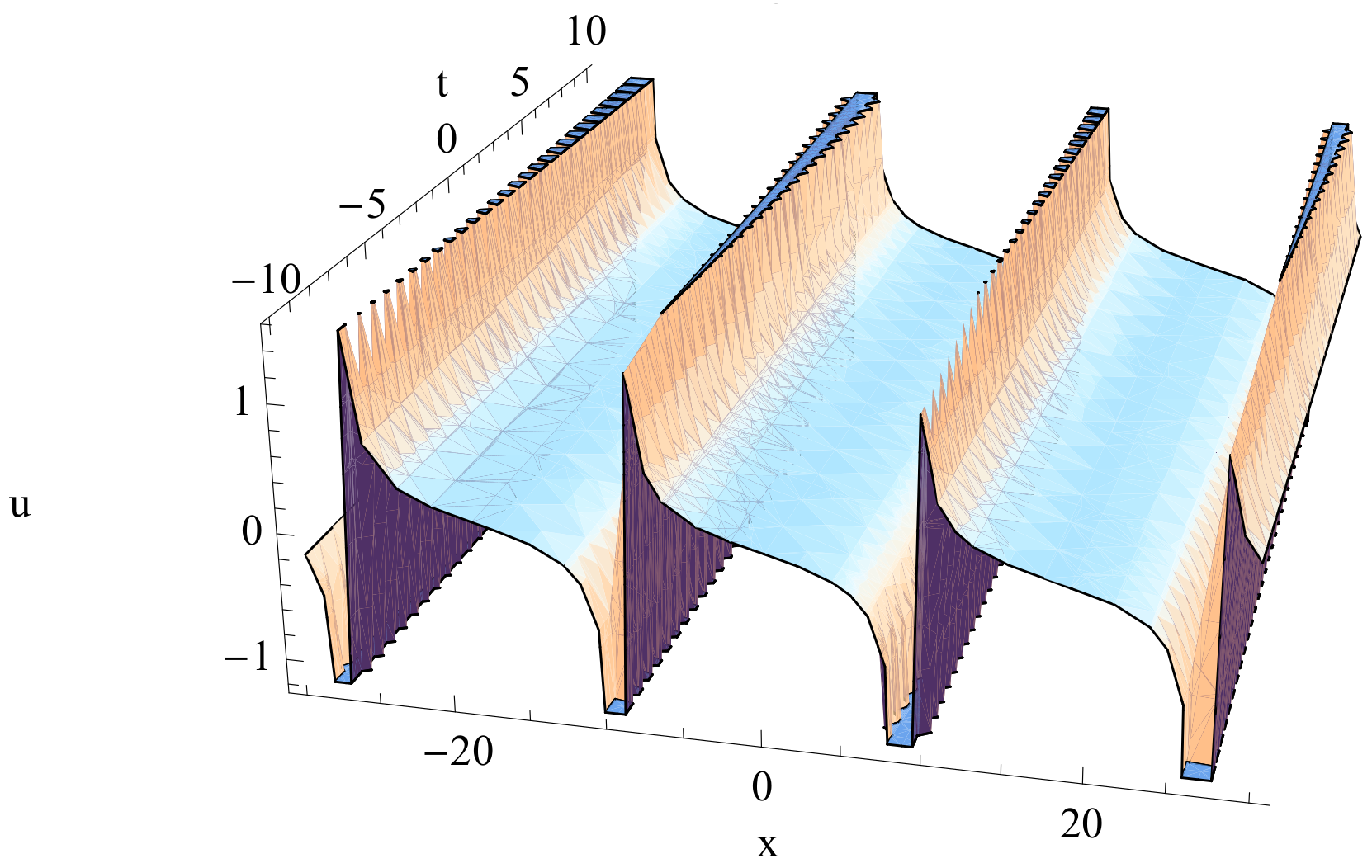
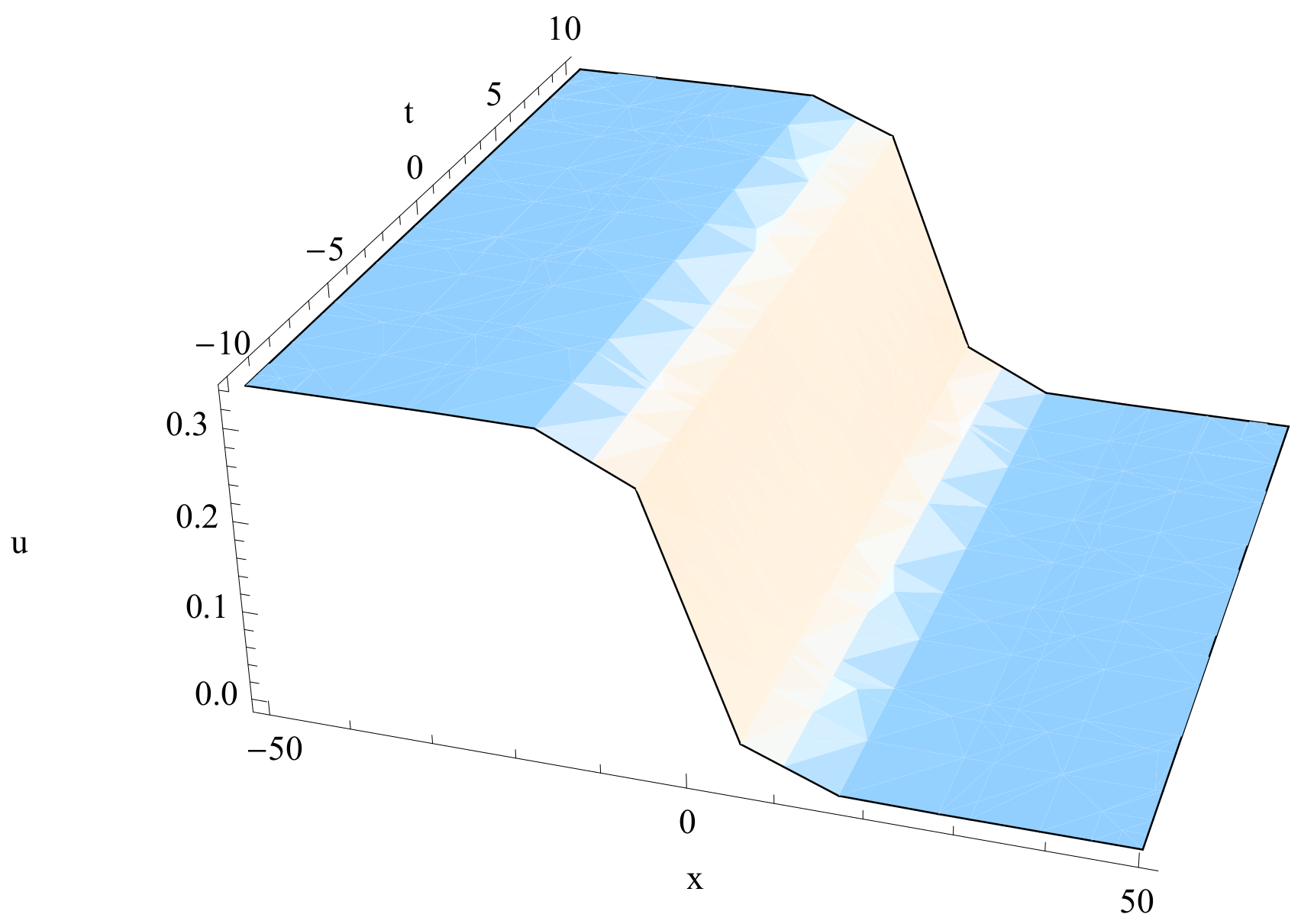
3.1. Example 1. Foam Drainage Equation
3.2. Example 2. Korteweg-de Vries Burgerss
4. Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Moslem, W.M.; Sabry, R.; Abdelsalam, U.M.; Kourakis, I.; Shukla, P.K. Solitary and blow-up electrostatic excitations in rotating magnetized electron-positron-ion plasmas. N. J. Phys. 2009, 11, 033028. [Google Scholar] [CrossRef]
- Abdelsalam, U.M.; Allehiany, F.M.; Moslem, W.M.; El-Labany, S.K. Nonlinear structures for extended Korteweg-de Vries equation in multicomponent plasma. Pramana J. Phys. 2016, 86, 581–597. [Google Scholar] [CrossRef]
- Abdelsalam, U.M.; Zobaer, M.S. Exact Traveling Wave Solutions of KdV Equation for DAWs in Superthermal Plasma. Rev. Mex. Astron. Astrofsica 2018, 54, 363–373. [Google Scholar]
- Abdelsalam, U.M. Traveling wave solutions for shallow water equations. J. Ocean. Eng. Sci. 2017, 2, 28–33. [Google Scholar] [CrossRef]
- Baskonus, H.M.; Sulaiman, T.A.; Bulut, H. On the new wave behavior to the Klein-Gordon-Zakharov equations in plasma physics. Indian J. Phys. 2019, 93, 393–399. [Google Scholar] [CrossRef]
- Drazin, P.G.; Johnson, R.S. Solitons: An Introduction; Cambridge University Press: Cambridge, UK, 1989. [Google Scholar]
- Vakhnenko, V.O.; Parkes, E.J.; Morrison, A.J. A Backlund transformation and the inverse scattering transform method for the generalised Vakhnenko equation. Chaos Solitons Fractals 2003, 17, 683–692. [Google Scholar] [CrossRef]
- Hirota, R. Direct method of finding exact solutions of nonlinear evolution equations. In Backlund Transformations; Bullough, R., Caudrey, P., Eds.; Springer: Berlin, Germany, 1980; pp. 1157–1175. [Google Scholar]
- Lu, D.; Hong, B.; Tian, L. Backlund Transformation and N-soliton-like Solutions to the Combined KdV-Burgers Equation with Variable Coefficients. Int. J. Nonlinear Sci. 2006, 10, 3–10. [Google Scholar]
- Malfliet, W. Solitary wave solutions of nonlinear wave equations. Am. J. Phys. 1992, 60, 650–654. [Google Scholar] [CrossRef]
- Gao, Y.T.; Tian, B. Generalized tanh method with symbolic computation and generalized shallow-water wave-equation. Comput. Math. Appl. 1997, 33, 115–118. [Google Scholar] [CrossRef]
- Fan, E. Extended tanh-function method and its applications to nonlinear equations. Phys. Lett. A 2000, 277, 212–218. [Google Scholar] [CrossRef]
- Abdelsalam, U.M. Exact travelling solutions of two coupled (2 + 1)-Dimensional Equations. J. Egypt. Math. Soc. 2017, 25, 125–128. [Google Scholar] [CrossRef]
- El-wakil, S.A.; Madkour, M.A.; Abdou, M.A. Application of Exp-function method for nonlinear evolution equations with variable coefficients. Phys. Lett. A 2007, 369, 62–69. [Google Scholar] [CrossRef]
- Wang, M.L.; Li, X.; Zhang, J. The (GG)-expansion method and travelling wave solutions of nonlinear evolution equations in mathematical physics. Phys. Lett. A. 2008, 372, 417. [Google Scholar] [CrossRef]
- Abdelsalam, U.M.; Zobaer, M.S. Exact Traveling Wave Solutions of Further Modified Korteweg-De Vries Equation in Multicomponent Plasma. Iran. J. Sci. Technol. Trans. Sci. 2018, 42, 2175–2182. [Google Scholar] [CrossRef]
- Abdelsalam, U.M.; Selim, M. Ion acoustic waves in a degenerate multicomponent magnetoplasma. J. Plasma Phys. 2013, 79, 163. [Google Scholar] [CrossRef]
- Sabry, R.; Zahran, M.A.; Fan, E. A new generalized expansion method and its application in finding explicit exact solutions for a generalized variable coefficients KdV equation. Phys. Lett. A 2004, 326, 326–393. [Google Scholar] [CrossRef]
- Moslem, W.M.; Abdelsalam, U.M.; Sabry, R.; Shukla, P.K. Electrostatic structures associated with dusty electronegative magnetoplasmas. N. J. Phys. 2010, 12, 73010. [Google Scholar] [CrossRef]
- Moussa, M.H.M.; El-Shiekh, R.M. Similarity reduction and similarity solutions of Zabolotskay- Khoklov equation with dissipative term via symmetry method. Phys. A 2006, 371, 325–335. [Google Scholar] [CrossRef]
- Wang, M.L. Solitrary wave solution for variant Boussinesq equation. Phys. Lett. A 1995, 199, 169–172. [Google Scholar] [CrossRef]
- Wang, M.L. Applicatian of homogeneous balance method to exact solutions of nonlinear equation in mathematical physics. Phys. Lett. A 1996, 216, 67–75. [Google Scholar] [CrossRef]
- Fan, E.G.; Zhang, H.Q. New exact solutions to a system of coupled KdV equations. Phys. Lett. A 1998, 245, 389–392. [Google Scholar] [CrossRef]
- Yang, L.; Zhu, Z.; Wang, Y. Exact Solutions of Nonlinear Equations. Phys. Lett. 1999, 260, 55–59. [Google Scholar] [CrossRef]
- Fan, E.G. Two new applications of the homogeneous balance method. Phys. Lett. A 2000, 265, 353–357. [Google Scholar] [CrossRef]
- Verbist, G.; Weaire, D.; Kraynik, A.M. The foam drainage equation. J. Phys. Condens. Matter 1996, 8, 3715. [Google Scholar] [CrossRef]
- Meng, P.; Yin, W. The Travelling Wave Solutions of KdV-Burgers Equations. In Proceedings of the International Conference on Management Science and Innovative Education (MSIE2015), Xi’an, China, 12–13 December 2015. [Google Scholar]
- Senthilkumaran, M.; Selvam, S.K. Solitary wave solution of the variable coefficient KdV-Burgers equation. Bull. Soc. Math. Banja Luka 2017, 7, 395–401. [Google Scholar]
- Chukkol, Y.B.; Mohamad, M.N.; Muminov, M.I. Exact Solutions to the KDV-Burgers Equation with Forcing Term Using Tanh-Coth Method. Aip Conf. Proc. 2017, 1870, 040024. [Google Scholar]
- Zaghbeer, S.K.; Salah, H.H.; Sheta, N.H.; El-Shewy, E.K.; Elgarayhi, A. Dust acoustic shock waves in dusty plasma of opposite polarity with non-extensive electron and ion distributions. J. Plasma Phys. 2014, 80, 517–528. [Google Scholar] [CrossRef]
- Nakamura, Y.; Bailung, H.; Shukla, P.K. Coulomb Dissociation of 19C and its Halo Structure. Phys. Rev. Lett. 1999, 83, 1602–1605. [Google Scholar] [CrossRef]
- Masood, W.; Rizvi, H.; Jehan, N.; Siddiq, M. Dissipative cylindrical fast magnetoacoustic waves in planetary magnetospheres. Astrophys. Space Sci. 2011, 335, 405–413. [Google Scholar] [CrossRef]
- Zayed, E.M.E.; Al-Nowehy, A.G. Exact solutions for nonlinear foam drainage equation. Indian J. Phys. 2017, 91, 209–218. [Google Scholar] [CrossRef]
- Abdelsalam, U.M.; Allehiany, F.M.; Moslem, W.M. Nonlinear Waves in GaAs Semiconductor. Acta Phys. Pol. A 2016, 129, 472–477. [Google Scholar] [CrossRef]
- Abdelsalam, U.M. Dust-ion-acoustic solitary waves in a dense pair-ion plasma. Phys. B 2010, 405, 3914–3918. [Google Scholar] [CrossRef]
- Abdelsalam, U.M. Solitary and freak waves in superthermal plasma with ion jet. J. Plasma Phys. 2013, 79, 287–294. [Google Scholar] [CrossRef]
- Abdelsalam, U.M.; Allehiany, F.M. Different Nonlinear Solutions of KP Equation in Dusty Plasmas. Arab. J. Sci. Eng. 2018, 43, 399–406. [Google Scholar] [CrossRef]


© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abdelsalam, U.M.; Ghazal, M.G.M. Analytical Wave Solutions for Foam and KdV-Burgers Equations Using Extended Homogeneous Balance Method. Mathematics 2019, 7, 729. https://doi.org/10.3390/math7080729
Abdelsalam UM, Ghazal MGM. Analytical Wave Solutions for Foam and KdV-Burgers Equations Using Extended Homogeneous Balance Method. Mathematics. 2019; 7(8):729. https://doi.org/10.3390/math7080729
Chicago/Turabian StyleAbdelsalam, U.M., and M. G. M. Ghazal. 2019. "Analytical Wave Solutions for Foam and KdV-Burgers Equations Using Extended Homogeneous Balance Method" Mathematics 7, no. 8: 729. https://doi.org/10.3390/math7080729
APA StyleAbdelsalam, U. M., & Ghazal, M. G. M. (2019). Analytical Wave Solutions for Foam and KdV-Burgers Equations Using Extended Homogeneous Balance Method. Mathematics, 7(8), 729. https://doi.org/10.3390/math7080729




