A Theoretical Rigid Body Model of Vibrating Screen for Spring Failure Diagnosis
Abstract
1. Introduction
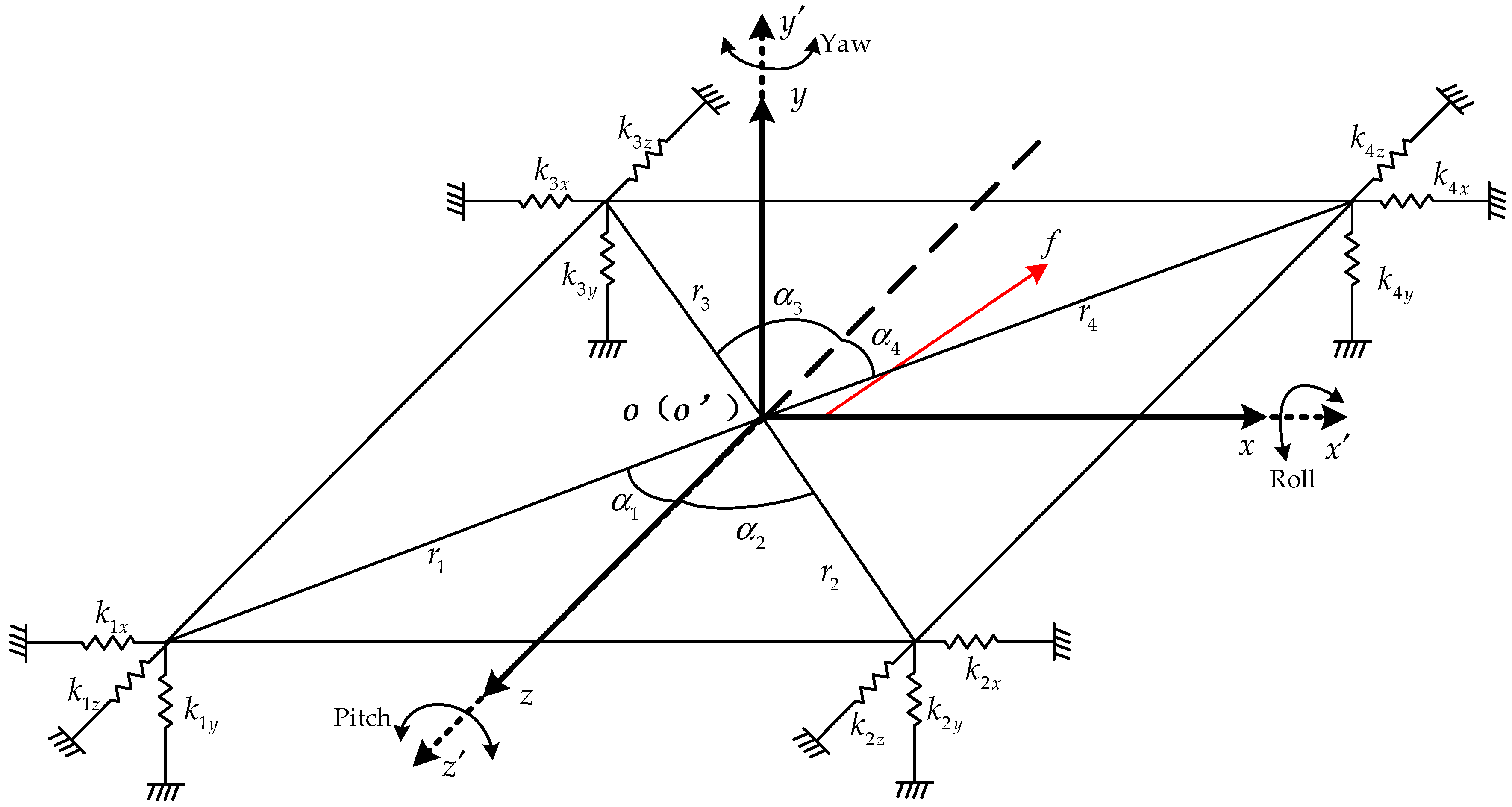
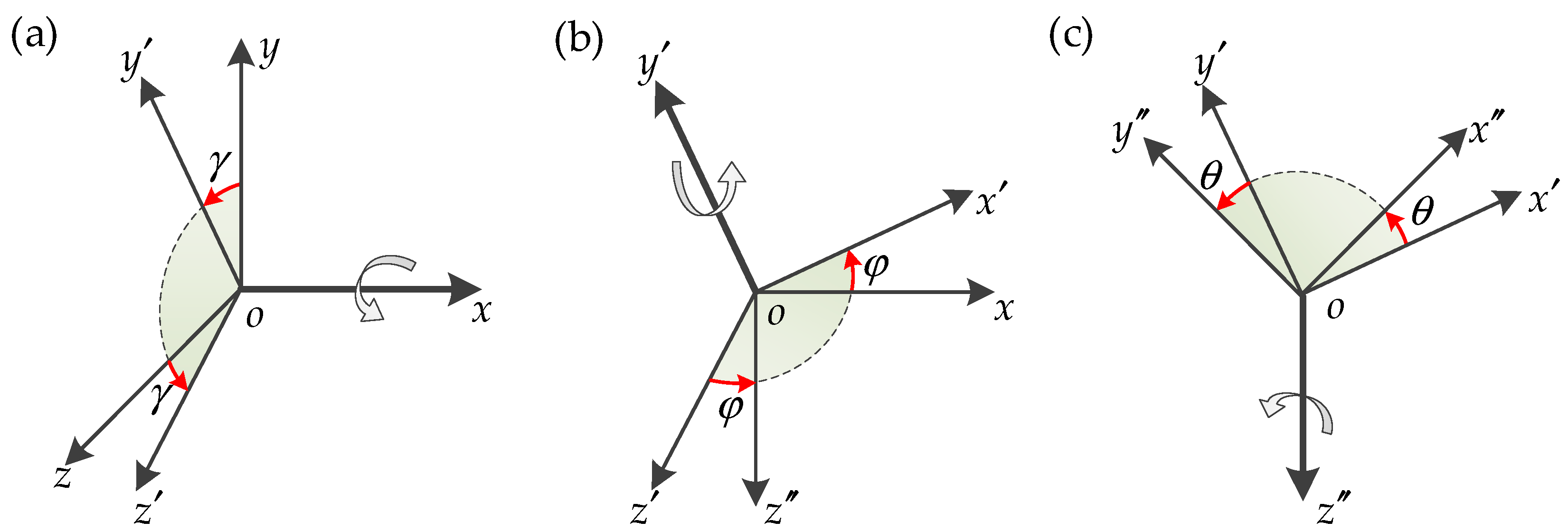
2. Theoretical Rigid Body Model
2.1. The Model
2.2. System Potential Energy
2.3. System Kinetic Energy
2.4. System Force Vector
2.5. Dynamic Equation
3. Simulations
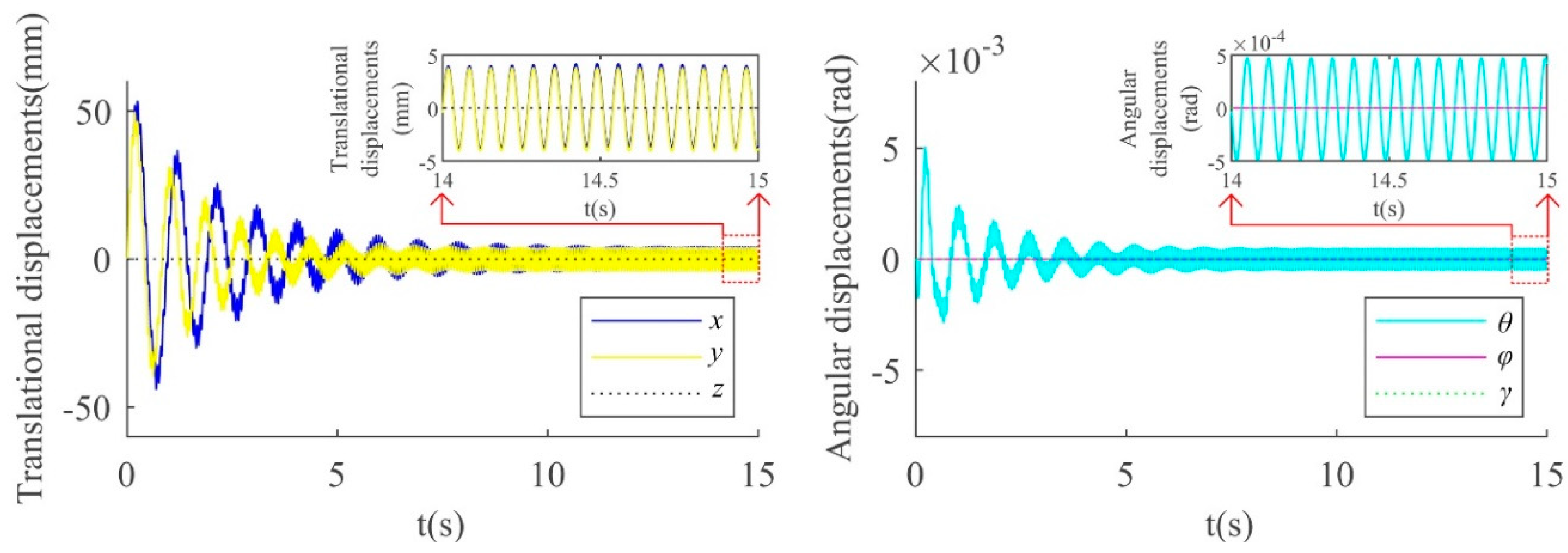
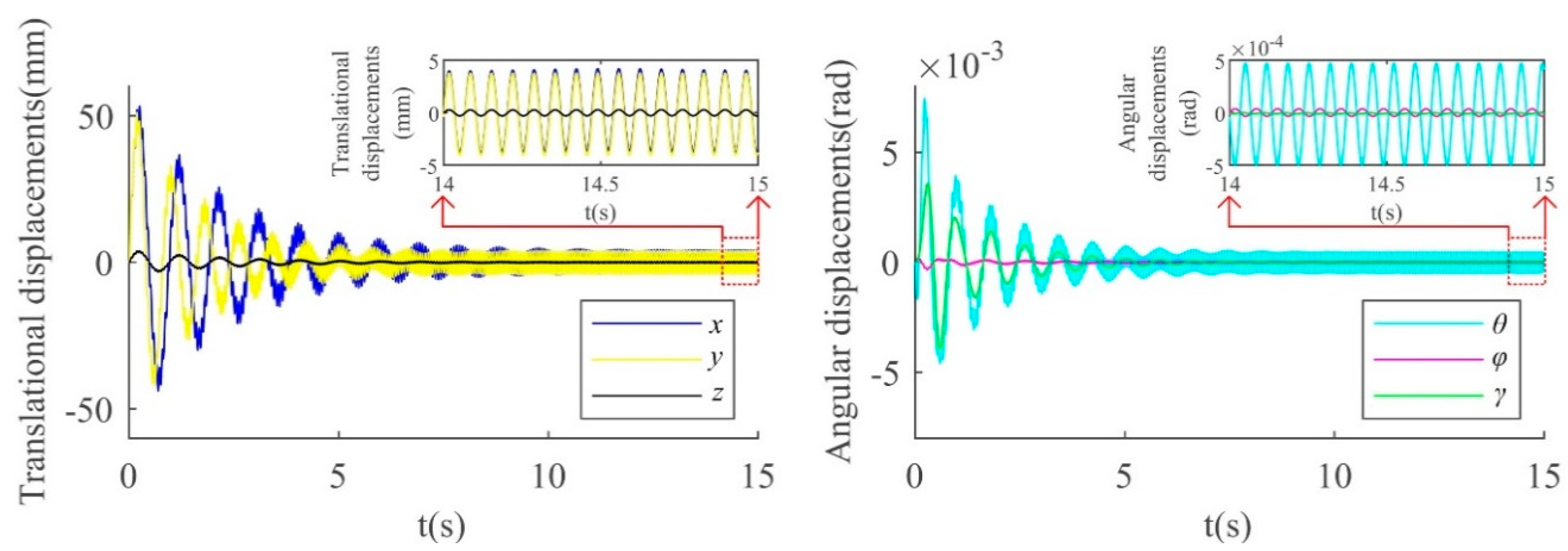
3.1. Numerical Simulations Results
3.2. Spring Failure Simulations Results
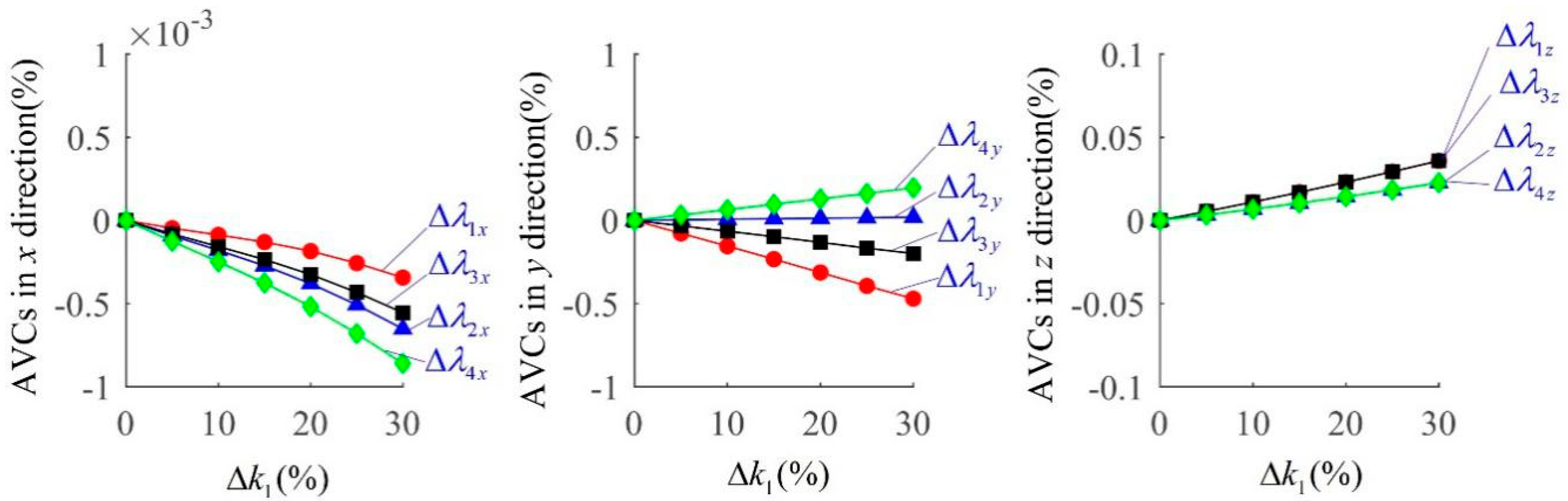
3.2.1. Single Spring Failure Simulations Results
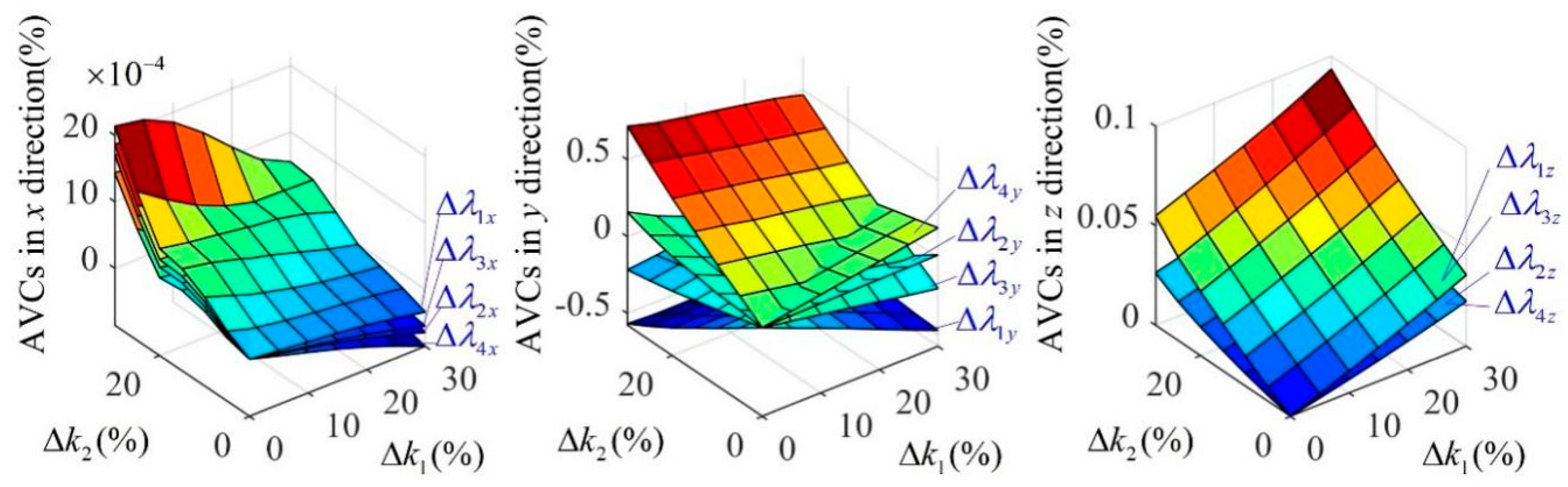
3.2.2. Double Spring Failures Simulations Results
3.3. Discussion
- In the case of spring failure, the amplitudes of all points in the x direction will decrease, while all amplitudes increase in the z direction. In the y direction, the amplitudes of points 2 and 4 increase, while the amplitudes of points 1 and 3 decrease.
- In the case of spring failure, all amplitudes will increase in both the x and z directions. In the y direction, the amplitudes of points 1 and 3 increase, while the amplitudes of points 2 and 4 decrease.
- In the case of spring and spring failure, all amplitudes will increase in the z direction. In the other directions, the change rules of all amplitudes are indeterminate.
- In the case of spring and spring failure, all amplitudes will decrease in the x direction. In the y direction, the amplitudes of points 3 and 4 increase, while the change rules of the amplitudes of points 1 and 2 are indeterminate. In the z direction, the change rules of all amplitudes are indeterminate.
- In the case of spring and spring failure, the change rules of all amplitudes are indeterminate in all directions.
- In the case of spring and spring failure, all amplitudes will increase in the x direction. In the y direction, the amplitudes of points 1 and 3 increase, while the amplitudes of points 2 and 4 decrease. In the z direction, the change rules of all amplitudes are indeterminate.
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| Spring of the elastic support point 1 in y direction | |
| Spring of the elastic support point 2 in y direction | |
| Spring of the elastic support point 3 in y direction | |
| Spring of the elastic support point 4 in y direction | |
| Spring stiffness of the elastic support point 1 in x direction | |
| Spring stiffness of the elastic support point 1 in y direction | |
| Spring stiffness of the elastic support point 1 in z direction | |
| Spring stiffness of the elastic support point 2 in x direction | |
| Spring stiffness of the elastic support point 2 in y direction | |
| Spring stiffness of the elastic support point 2 in z direction | |
| Spring stiffness of the elastic support point 3 in x direction | |
| Spring stiffness of the elastic support point 3 in y direction | |
| Spring stiffness of the elastic support point 3 in z direction | |
| Spring stiffness of the elastic support point 4 in x direction | |
| Spring stiffness of the elastic support point 4 in y direction | |
| Spring stiffness of the elastic support point 4 in z direction | |
| i | Elastic support points number |
| o | Origin of the inertial frame |
| x | x-axis |
| x | Translation displacement of the rigid body in x direction |
| x | Coordinate of the mass center in the inertial frame |
| xd | Coordinate of one point of the rigid body in the inertial frame |
| Displacement column vector | |
| Velocity column vector | |
| Acceleration column vector | |
| y | y-axis |
| y | Translation displacement of the rigid body in y direction |
| y | Coordinate of the mass center in the inertial frame |
| yd | Coordinate of one point of the rigid body in the inertial frame |
| z | z-axis |
| z | Translation displacement of the rigid body in z direction |
| z | Coordinate of the mass center in the inertial frame |
| zd | Coordinate of one point of the rigid body in the inertial frame |
| o’ | Origin of the body frame |
| x′ | x′-axis |
| x′ | Coordinate of the mass center in the body frame |
| y′ | y′-axis |
| y′ | Coordinate of the mass center in the body frame |
| z′ | z′-axis |
| z′ | Coordinate of the mass center in the body frame |
| Distance between the mass center of the rigid body and the elastic support point 1 | |
| Distance between the mass center of the rigid body and the elastic support point 2 | |
| Distance between the mass center of the rigid body and the elastic support point 3 | |
| Distance between the mass center of the rigid body and the elastic support point 4 | |
| Angle between and z-axis | |
| Angle between and z-axis | |
| Angle between and z-axis | |
| Angle between and z-axis | |
| m | Mass of rigid body |
| Moment of inertia of the rigid body rotation around ox-axis | |
| Moment of inertia of the rigid body rotation around oy-axis | |
| Moment of inertia of the rigid body rotation around oz-axis | |
| Angular of the rigid body rotation around ox-axis | |
| Angular of the rigid body rotation around oy-axis | |
| Angular of the rigid body rotation around oz-axis | |
| f | Exciting force |
| F | System force vector |
| Angle between exciting force vector and o’x′-axis | |
| Angle between exciting force vector and o’y′-axis | |
| Angle between exciting force vector and o’z′-axis | |
| Rotation matrix of the rigid body rotation around ox-axis | |
| Rotation matrix of the rigid body rotation around oy-axis | |
| Rotation matrix of the rigid body rotation around oz-axis | |
| Rotation matrix of the rigid body in the sequence of oz-oy-ox | |
| U | System potential energy |
| E | System kinetic energy |
| Mass matrix | |
| Stiffness matrix | |
| C | Damping matrix |
| Circular frequency of exciting force | |
| Angular velocity of the rigid body rotation around ox-axis | |
| Angular velocity of the rigid body rotation around oy-axis | |
| Angular velocity of the rigid body rotation around oz-axis | |
| Steady state solution of the forced vibration system | |
| Steady state solution of the forced vibration system | |
| Steady state solution of the forced vibration system | |
| Steady state solution of the forced vibration system | |
| Steady state solution of the forced vibration system | |
| Steady state solution of the forced vibration system | |
| tm | Total time of simulation |
| dt | Time step of simulation |
| Spring stiffness variation coefficient of spring k1 | |
| Spring stiffness variation coefficient of spring k2 | |
| Spring stiffness variation coefficient of spring k3 | |
| Spring stiffness variation coefficient of spring k4 | |
| Spring stiffness variation coefficient of spring k1 | |
| Spring stiffness variation coefficient of spring k2 | |
| Spring stiffness variation coefficient of spring k3 | |
| Spring stiffness variation coefficient of spring k4 | |
| Amplitude variation coefficient of elastic support point 1 in x direction | |
| Amplitude variation coefficient of elastic support point 1 in y direction | |
| Amplitude variation coefficient of elastic support point 1 in z direction | |
| Amplitude variation coefficient of elastic support point 2 in x direction | |
| Amplitude variation coefficient of elastic support point 2 in y direction | |
| Amplitude variation coefficient of elastic support point 2 in z direction | |
| Amplitude variation coefficient of elastic support point 3 in x direction | |
| Amplitude variation coefficient of elastic support point 3 in y direction | |
| Amplitude variation coefficient of elastic support point 3 in z direction | |
| Amplitude variation coefficient of elastic support point 4 in x direction | |
| Amplitude variation coefficient of elastic support point 4 in y direction | |
| Amplitude variation coefficient of elastic support point 4 in z direction |
References
- Liu, C.S.; Zhang, S.M.; Zhou, H.P.; Li, J.; Xia, Y.F.; Peng, L.P.; Wang, H. Dynamic analysis and simulation of four-axis forced synchronizing banana vibrating screen of variable linear trajectory. J. Cent. South Univ. 2012, 19, 1530–1536. [Google Scholar] [CrossRef]
- Wang, H.; Liu, C.S.; Peng, L.P.; Jiang, X.W.; Ji, L.Q. Dynamic analysis of elastic screen surface with multiple attached substructures and experimental validation. J. Cent. South Univ. 2012, 19, 2910–2917. [Google Scholar] [CrossRef]
- Song, B.C.; Liu, C.S.; Peng, L.P.; Li, J. Dynamic analysis of new type elastic screen surface with multi degree of freedom and experimental validation. J. Cent. South Univ. 2015, 22, 1334–1341. [Google Scholar] [CrossRef]
- Zhang, X.L.; Zhao, C.Y.; Wen, B.C. Theoretical and experimental study on synchronization of the two homodromy exciters in a non-resonant vibrating system. Shock Vib. 2013, 20, 327–340. [Google Scholar] [CrossRef]
- Zhang, Z.R.; Wang, Y.Y.; Fan, Z.M. Similarity Analysis between Scale Model and Prototype of Large Vibrating Screen. Shock Vib. 2015. [Google Scholar] [CrossRef]
- Dong, K.J.; Wang, B.; Yu, A.B. Modeling of Particle Flow and Sieving Behavior on a Vibrating Screen: From Discrete Particle Simulation to Process Performance Prediction. Ind. Eng. Chem. Res. 2013, 52, 11333–11343. [Google Scholar] [CrossRef]
- Makinde, O.A.; Ramatsetse, B.I.; Mpofu, K. Review of vibrating screen development trends: Linking the past and the future in mining machinery industries. Int. J. Miner. Process. 2015, 145, 17–22. [Google Scholar] [CrossRef]
- Peng, L.; Liu, C.; Wang, H. Health identification for damping springs of large vibrating screen based on stiffness identification. J. China Coal Soc. 2016, 41, 1568–1574. [Google Scholar]
- Jiang, H.S.; Zhao, Y.M.; Duan, C.L.; Yang, X.L.; Liu, C.S.; Wu, J.D.; Qiao, J.P.; Diao, H.R. Kinematics of variable-amplitude screen and analysis of particle behavior during the process of coal screening. Powder Technol. 2017, 306, 88–95. [Google Scholar] [CrossRef]
- Peng, L.P.; Liu, C.S.; Song, B.C.; Wu, J.D.; Wang, S. Improvement for design of beam structures in large vibrating screen considering bending and random vibration. J. Cent. South Univ. 2015, 22, 3380–3388. [Google Scholar] [CrossRef]
- Zhang, Z.R. Strain modal analysis and fatigue residual life prediction of vibrating screen beam. J. Meas. Eng. 2016, 4, 217–223. [Google Scholar] [CrossRef]
- Li, Z.; Tong, X. Modeling and parameter optimization for vibrating screens based on AFSA-SimpleMKL. Chin. J. Eng. Des. 2016, 23, 181–187. [Google Scholar]
- Xiao, J.; Tong, X. Characteristics and efficiency of a new vibrating screen with a swing trace. Particuology 2013, 11, 601–606. [Google Scholar] [CrossRef]
- Baragetti, S. Innovative structural solution for heavy loaded vibrating screens. Miner. Eng. 2015, 84, 15–26. [Google Scholar] [CrossRef]
- Baragetti, S.; Villa, F. A dynamic optimization theoretical method for heavy loaded vibrating screens. Nonlinear Dyn. 2014, 78, 609–627. [Google Scholar] [CrossRef]
- Peng, L.P.; Fang, R.X.; Fen, H.H.; Zhang, L.; Ma, W.D.; He, X.D. A more accurate dynamic model for dual-side excitation large vibrating screens. J. Vibroeng. 2018, 20, 858–871. [Google Scholar]
- Du, C.L.; Gao, K.D.; Li, J.P.; Jiang, H. Dynamics Behavior Research on Variable Linear Vibration Screen with Flexible Screen Face. Adv. Mech. Eng. 2014, 12. [Google Scholar] [CrossRef]
- Trumic, M.; Magdalinovic, N. New model of screening kinetics. Miner. Eng. 2011, 24, 42–49. [Google Scholar] [CrossRef]
- Dong, K.J.; Yu, A.B.; Brake, I. DEM simulation of particle flow on a multi-deck banana screen. Miner. Eng. 2009, 22, 910–920. [Google Scholar] [CrossRef]
- Rodriguez, C.G.; Moncada, M.A.; Dufeu, E.E.; Razeto, M.I. Nonlinear Model of Vibrating Screen to Determine Permissible Spring Deterioration for Proper Separation. Shock Vib. 2016. [Google Scholar] [CrossRef]
- Peng, L.P.; Liu, C.S.; Wu, J.D.; Wang, S. Stiffness identification of four-point-elastic-support rigid plate. J. Cent. South Univ. 2015, 22, 159–167. [Google Scholar] [CrossRef]
- Baranowski, L. Equations of motion of a spin-stabilized projectile for flight stability testing. J. Theor. Appl. Mech. 2013, 51, 235–246. [Google Scholar]
- Bai, L.; Dong, Z.F.; Ge, X.S. The closed-loop kinematics modeling and numerical calculation of the parallel hexapod robot in space. Adv. Mech. Eng. 2017, 9, 15. [Google Scholar] [CrossRef]
- Bai, L.; Ma, L.H.; Dong, Z.F.; Ge, X.S. Kinematics, Dynamics, and Optimal Control of Pneumatic Hexapod Robot. Math. Probl. Eng. 2017. [Google Scholar] [CrossRef]


| Parameters | m/kg | Jx/(kg·m2) | Jy/(kg·m2) | Jz/(kg·m2) |
|---|---|---|---|---|
| value | 18,900 | 35,200 | 30,650 | 32,600 |
| parameters | /(N/m) | /(N/m) | /(N/m) | /(N/m) |
| value | 353,010 | 470,680 | 353,010 | 470,680 |
| parameters | /(N/m) | /(N/m) | /(N/m) | /(N/m) |
| value | 931,800 | 1,242,400 | 931,800 | 1,242,400 |
| parameters | /(N/m) | /(N/m) | /(N/m) | /(N/m) |
| value | 353,010 | 470,680 | 353,010 | 470,680 |
| parameters | /(N/m) | /(N/m) | /(N/m) | /(N/m) |
| value | 353,010 | 470,680 | 353,010 | 470,680 |
| parameters | /(m) | /(m) | /(m) | /(m) |
| value | 3 | 3 | 3 | 3 |
| parameters | /(°) | /(°) | /(°) | /(°) |
| value | 53.13 | 53.13 | 53.13 | 53.13 |
| parameters | /(°) | /(°) | /(°) | /(rad/s) |
| value | 45 | 45 | 90 | 93.12 |
| parameters | f/(N) | tm/(s) | dt/(s) | - |
| value | 1,800,000 | 20 | 1/10,240 | - |
| Failures Type | ||||
|---|---|---|---|---|
| Single spring failure | × 1 | √ 2 | √ | √ |
| √ | × | √ | √ | |
| Double spring failure | × | × | √ | √ |
| × | √ | × | √ | |
| × | √ | √ | × | |
| √ | × | √ | × |
| Stiffness Variation Coefficient | Amplitude Variation Coefficient | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| − 1 | − | − | − | − | + 2 | − | + | + | + | + | + | |
| + | + | + | + | + | − | + | − | + | + | + | + | |
| ± 3 | ± | ± | ± | ± | ± | ± | ± | + | + | + | + | |
| − | − | − | − | ± | ± | + | + | ± | ± | ± | ± | |
| ± | ± | ± | ± | ± | ± | ± | ± | ± | ± | ± | ± | |
| + | + | + | + | + | − | + | − | ± | ± | ± | ± | |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Y.; Suo, S.; Meng, G.; Shang, D.; Bai, L.; Shi, J. A Theoretical Rigid Body Model of Vibrating Screen for Spring Failure Diagnosis. Mathematics 2019, 7, 246. https://doi.org/10.3390/math7030246
Liu Y, Suo S, Meng G, Shang D, Bai L, Shi J. A Theoretical Rigid Body Model of Vibrating Screen for Spring Failure Diagnosis. Mathematics. 2019; 7(3):246. https://doi.org/10.3390/math7030246
Chicago/Turabian StyleLiu, Yue, Shuangfu Suo, Guoying Meng, Deyong Shang, Long Bai, and Jianwen Shi. 2019. "A Theoretical Rigid Body Model of Vibrating Screen for Spring Failure Diagnosis" Mathematics 7, no. 3: 246. https://doi.org/10.3390/math7030246
APA StyleLiu, Y., Suo, S., Meng, G., Shang, D., Bai, L., & Shi, J. (2019). A Theoretical Rigid Body Model of Vibrating Screen for Spring Failure Diagnosis. Mathematics, 7(3), 246. https://doi.org/10.3390/math7030246






