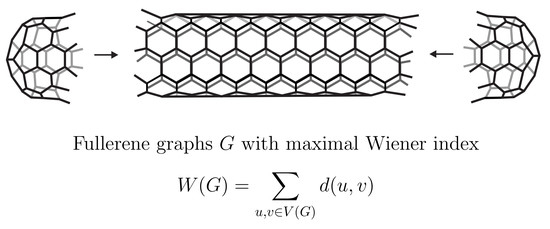
On the Wiener Complexity and the Wiener Index of Fullerene Graphs
Abstract
1. Introduction
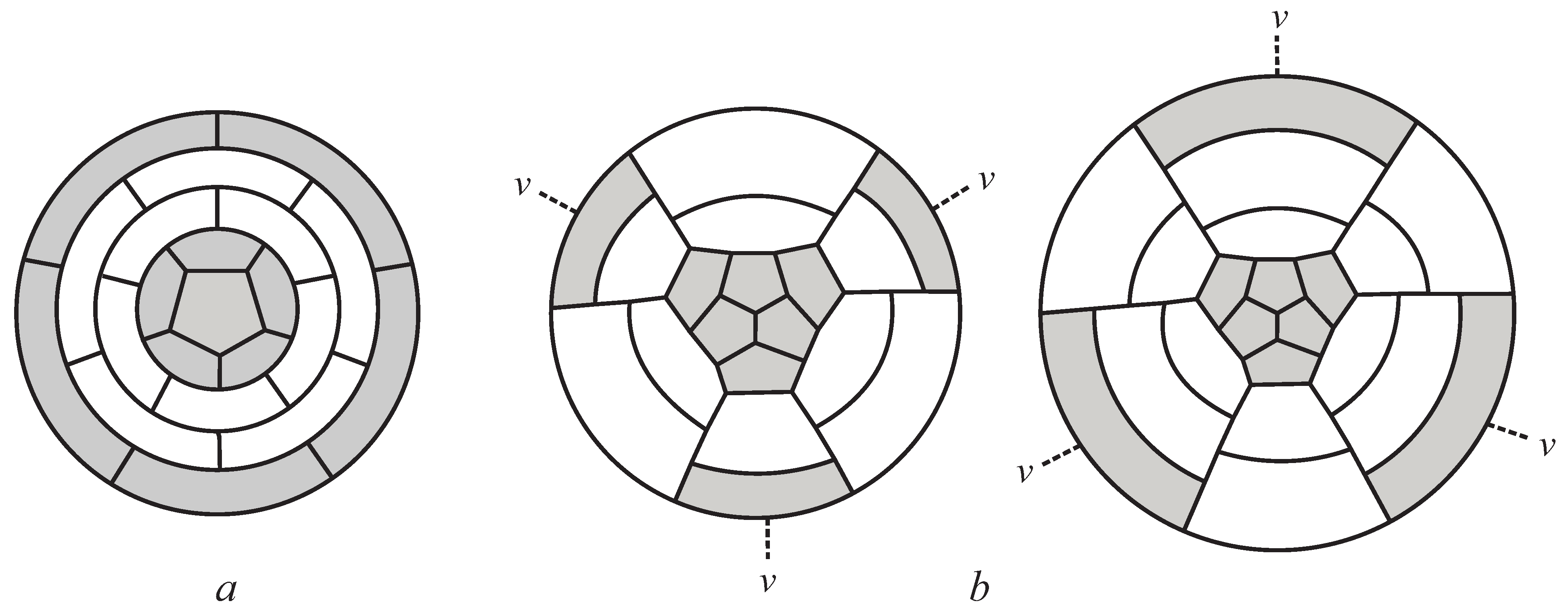
2. Wiener Complexity of Fullerene Graphs
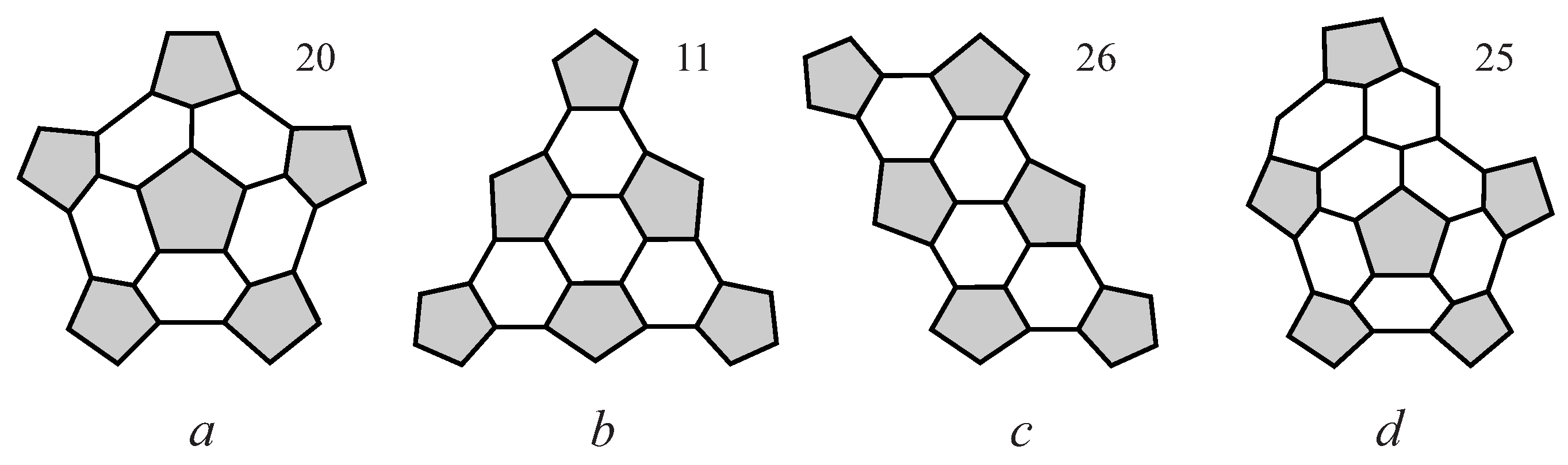
3. Graphs with the Maximal Wiener Complexity
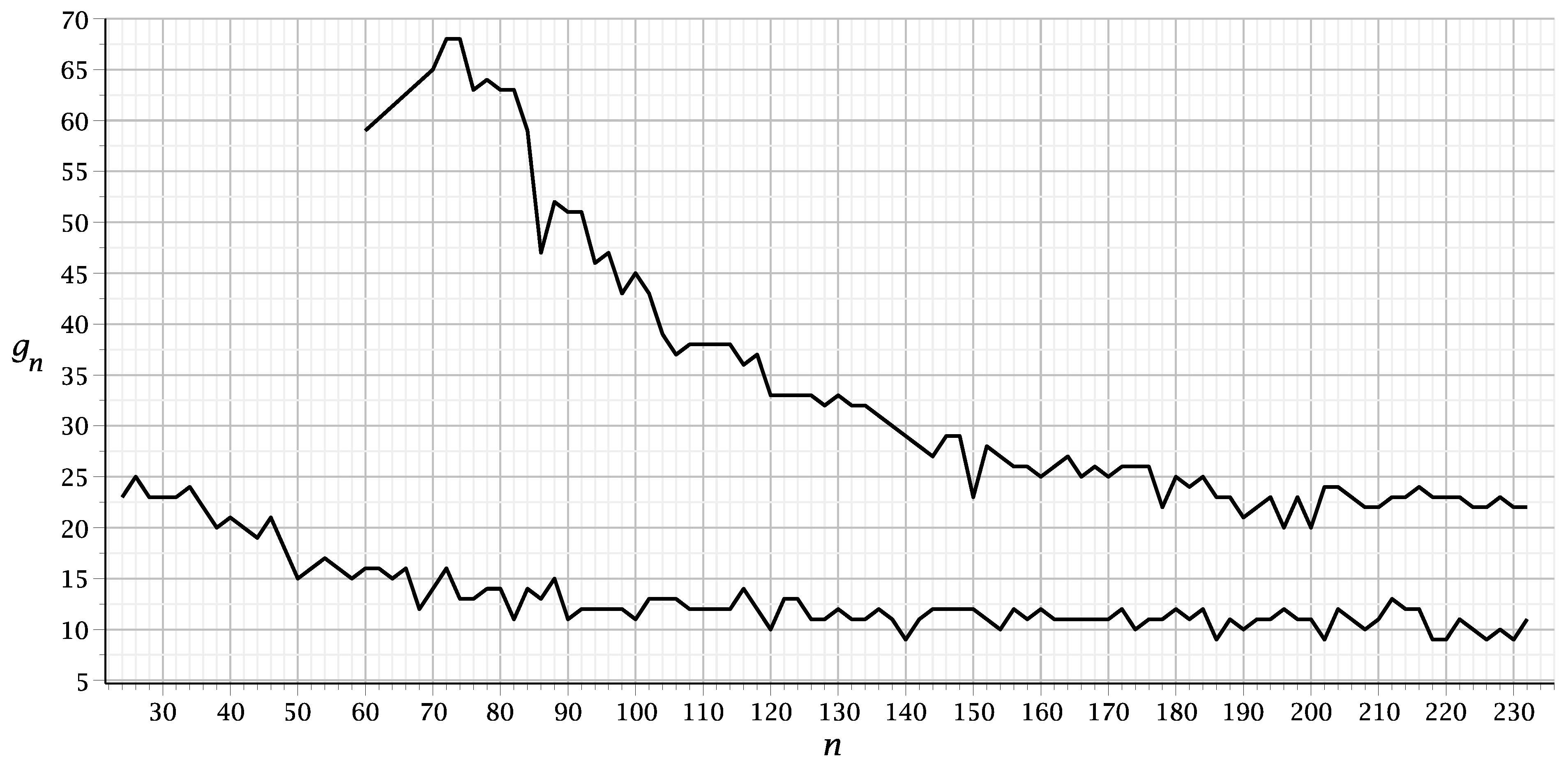
- Values of the maximal Wiener complexity of all fullerene graphs do not decrease except for one case: and . For IPR fullerene graphs, we have four exceptions (see pairs ; ; , and ).
- Wiener indices of fullerene and IPR fullerene graphs with maximal () are not maximal except graphs of order with ().
- Several fullerene or IPR fullerene graphs of fixed n may have the maximal complexity .
- Almost all fullerene graphs with fixed have distinct Wiener indices except for two graphs of order 46 with and and two IPR fullerene graphs of order 204 with and 185,306.
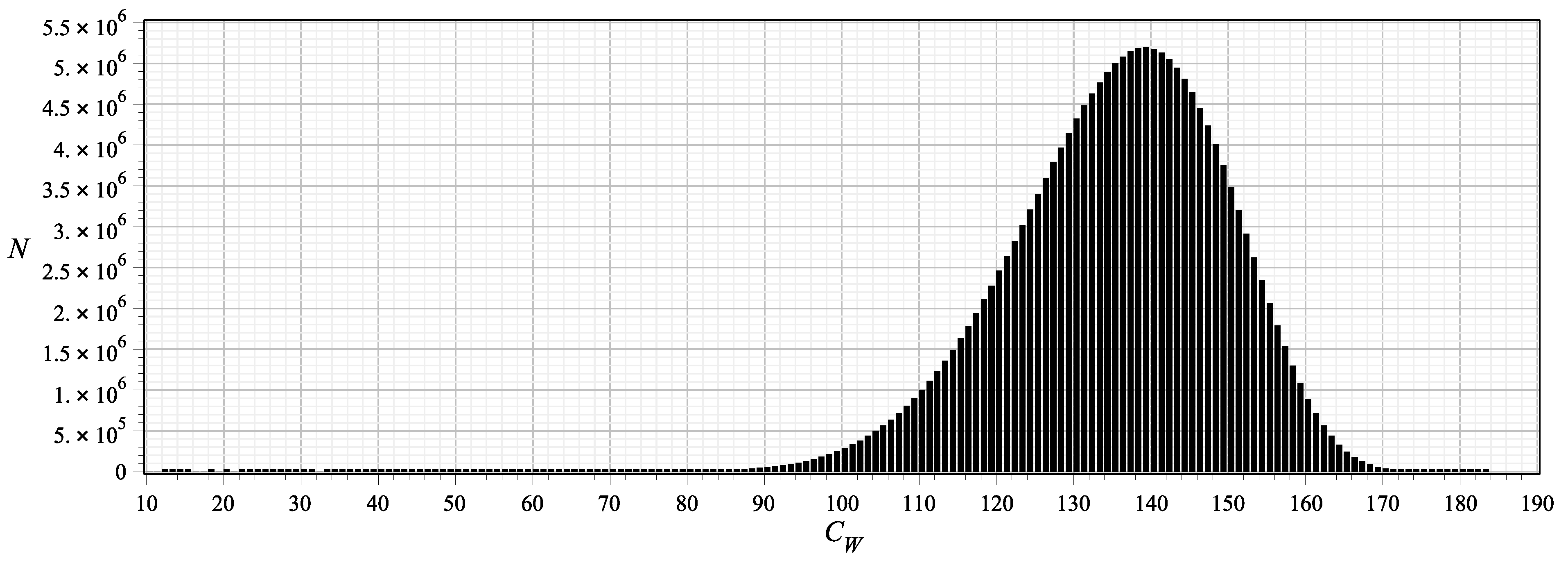
- Given n, only one fullerene graph has the maximal Wiener index while there are pairs of IPR fullerene graphs with the same maximal W (see graphs of order 132, 186, 204, 222, and 258).
- The diameter D of graphs with fixed is less than or equal to the diameter of graphs with a maximal Wiener index for all fullerene graphs (with seven exceptions for IPR fullerene graphs of order 90, 92, 96, 100, 102, 116, and 124).
- Fullerene graphs with the maximal Wiener index have the maximal diameter among all fullerenes. The values of the Wiener complexity of these graphs can vary greatly. This can be partially explained by the appearance of symmetries in graphs with .
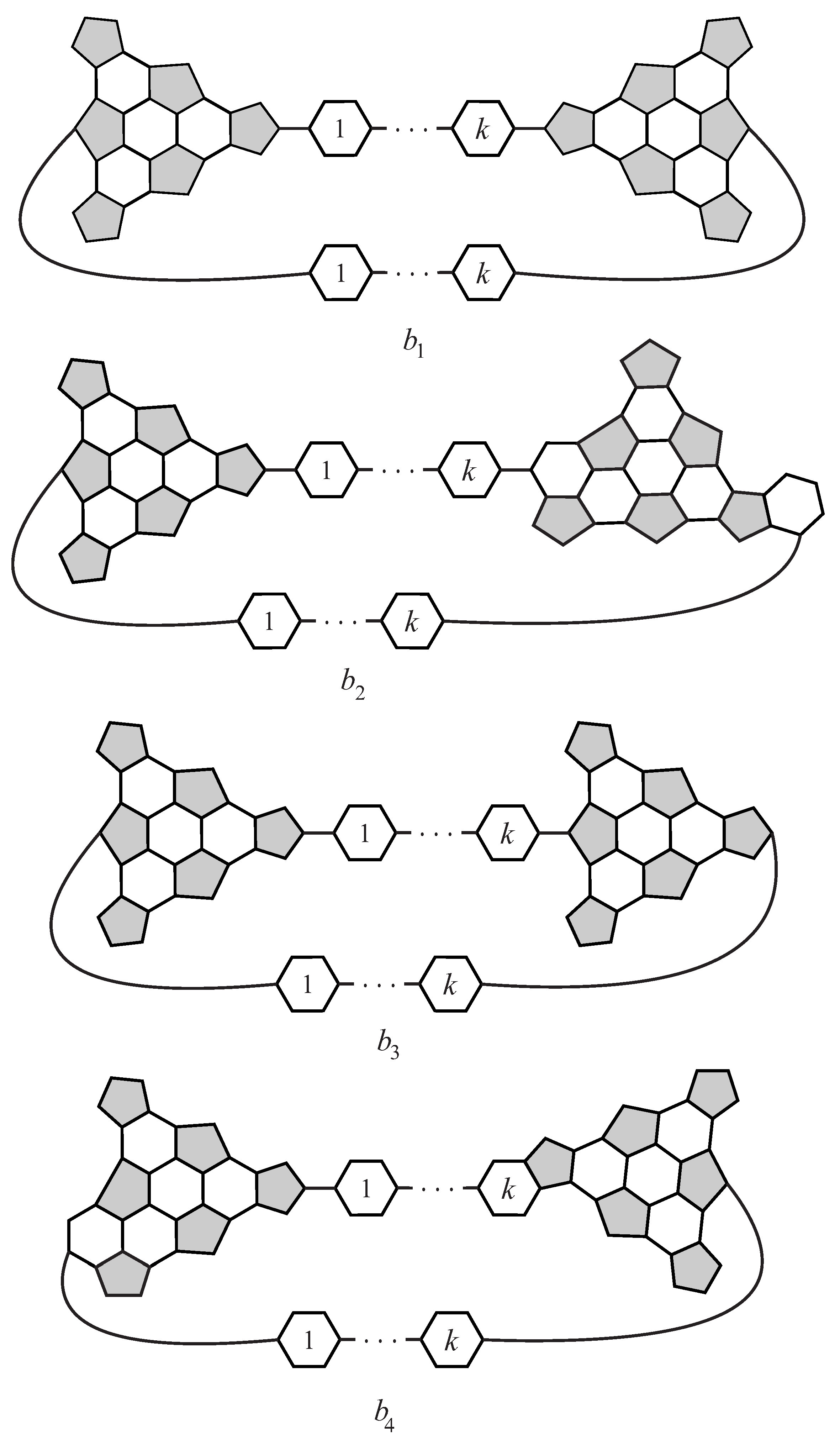
4. Fullerene Graphs with the Maximal Wiener Index
- (a)
- Let be a nanotubical fullerene graph of family . Then, for ,
- (b)
- Let be a nanotubical fullerene graph of family . Then, for ,
- (a)
- Let be a nanotubical fullerene graph of family . Then, and for ,
- (b)
- Let be a nanotubical fullerene graph of family . Then, and for ,
5. IPR Fullerene Graphs with the Maximal Wiener Index
- (a)
- Let be a nanotubical IPR fullerene graph of family . It has vertices, . Then, and
- (b)
- Let be a nanotubical IPR fullerene graph of family . It has vertices, . Then, and
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Ashrafi, A.R.; Diudea, M.V. Distance, Symmetry, and Topology in Carbon Nanomaterials; Carbon Materials: Chemistry and Physics Book 9; Springer: Cham, Switzerland, 2016. [Google Scholar]
- Cataldo, F.; Graovac, A.; Ori, O. The Mathematics and Topology of Fullerenes; Carbon Materials: Chemistry and Physics Book 4; Springer: Berlin, Germany, 2011. [Google Scholar]
- Brinkmann, G.; Dress, A.W.M. A constructive enumeration of fullerenes. J. Algorithams 1997, 309, 345–358. [Google Scholar] [CrossRef]
- Fowler, P.W.; Manolopoulos, D.E. An Atlas of Fullerenes; Clarendon Press: Oxford, UK, 1995. [Google Scholar]
- Goedgebeur, J.; McKay, B.D. Recursive generation of IPR fullerenes. J. Math. Chem. 2015, 53, 1702–1724. [Google Scholar] [CrossRef]
- Goedgebeur, J.; McKay, B.D. Fullerenes with distant pentagons. MATCH Commun. Math. Comput. Chem. 2015, 74, 659–672. [Google Scholar]
- Alizadeh, Y.; Andova, V.; Klavžar, S.; Škrekovski, R. Wiener dimension: Fundamental properties and (5,0)-nanotubical fullerenes. MATCH Commun. Math. Comput. Chem. 2014, 72, 279–294. [Google Scholar]
- Andova, V.; Orlić, D.; Škrekovski, R. Leapfrog fullerenes and Wiener index. Appl. Math. Comput. 2017, 309, 281–288. [Google Scholar] [CrossRef]
- Bašić, N.; Brinkmann, G.; Fowler, P.W.; Pisanski, T.; Van Cleemput, N. Sizes of pentagonal clusters in fullerenes. J. Math. Chem. 2017, 55, 1669–1682. [Google Scholar] [CrossRef]
- Erokhovets, N. Construction of fullerenes and Pogorelov polytopes with 5-, 6- and one 7-gonal face. Symmetry 2018, 10, 67. [Google Scholar] [CrossRef]
- Fowler, P.W. Resistance distances in fullerene graphs. Croat. Chem. Acta 2002, 10, 401–408. [Google Scholar]
- Fowler, P.W.; Caporossi, G.; Hansen, P. Distance matrices, Wiener indices, and related invariants of fullerenes. J. Phys. Chem. A. 2001, 105, 6232–6242. [Google Scholar] [CrossRef]
- Ghorbani, M. Computing Wiener index of C24n fullerenes. J. Comput. Theor. Nanosci. 2015, 12, 1847–1851. [Google Scholar] [CrossRef]
- Ghorbani, M.; Dehmer, M.; Rajabi-Parsa, M.; Mowshowitz, A.; Emmert-Streib, F. On properties of distance-based entropies on fullerene graphs. Entropy 2019, 12, 482. [Google Scholar] [CrossRef]
- Ghorbani, M.; Ghorbani, T. Computing the Wiener index of an infinite class of fullerenes. Studia Ubb Chemia 2013, 58, 43–50. [Google Scholar]
- Ghorbani, M.; Songhori, M. Computing Wiener index of C12n fullerenes. Ars Combinatoria 2017, 130, 175–180. [Google Scholar]
- Ghosh, T.; Mondal, S.; Mondal, S.; Mandal, B. Distance numbers and Wiener indices of IPR fullerenes with formula C10(n−2) (n ≥ 8) in analytical forms. Chem. Phys. Lett. 2018, 701, 72–80. [Google Scholar] [CrossRef]
- Graovac, A.; Ori, O.; Faghani, M.; Ashrafi, A. Distance property of fullerenes. Iranian J. Math. Chem. 2011, 2, 99–107. [Google Scholar]
- Hua, H.; Faghani, M.; Ashrafi, A. The Wiener and Wiener polarity indices of a class of fullerenes with exactly 12n carbon atoms. MATCH Commun. Math. Comput. Chem. 2014, 71, 361–372. [Google Scholar]
- Iranmanesh, A.; Alizadeh, Y.; Mirzaie, S. Computing Wiener polynomial, Wiener index and hyper Wiener index of C80 fullerene by GAP program. Fuller. Nanotub. Car. Nanostruct. 2009, 17, 560–566. [Google Scholar] [CrossRef]
- Ori, O.; D’Mello, M. A topological study of the structure of the C76 fullerene. Chem. Phys. Lett. 1992, 197, 49–54. [Google Scholar] [CrossRef]
- Sabirov, D.S.; Ori, O.; László, I. Isomers of the C84 fullerene: A theoretical consideration within energetic, structural, and topological approaches. Fuller. Nanotub. Carbon Nanostruct. 2018, 26, 100–110. [Google Scholar] [CrossRef]
- Sabirov, D.S.; Ōsawa, E. Information entropy of fullerenes. J. Chem. Inf. Model. 2015, 55, 1576–1584. [Google Scholar] [CrossRef]
- Vukičević, D.; Cataldo, F.; Ori, O.; Craovac, A. Topological efficiency of C66 fullerene. Chem. Phys. Lett. 2011, 501, 442–445. [Google Scholar] [CrossRef]
- Brinkmann, G.; Nathusius, U.; Palser, A.H.R. A constructive enumeration of nanotube caps. Discrete Appl. Math. 2002, 116, 55–71. [Google Scholar] [CrossRef]
- Brinkmann, G.; Fowler, P.W.; Manolopoulos, D.E.; Palser, A.H.R. A census of nanotube caps. Chem. Phys. Lett. 1999, 315, 335–347. [Google Scholar] [CrossRef]
- Buchstaber, V.M.; Erokhovets, N.Y. Finite sets of operations sufficient to construct any fullerene from C20. Struct. Chem. 2017, 28, 225–234. [Google Scholar] [CrossRef]
- Andova, V.; Kardoš, F.; Škrekovski, R. Mathematical aspects of fullerenes. Ars Math. Contemp. 2016, 11, 353–379. [Google Scholar]
- Schwerdtfeger, P.; Wirz, L.N.; Avery, J. The topology of fullerenes. WIREs Comput. Mol. Sci. 2015, 5, 96–145. [Google Scholar] [CrossRef]
- Sharafdini, R.; Reti, T. On the transmission-based graph topological indices. Kragujevac J. Math. 2020, 44, 41–63. [Google Scholar]
- Wiener, H. Structural determination of paraffin boiling points. J. Am. Chem. Soc. 1947, 69, 17–20. [Google Scholar] [CrossRef]
- Hosoya, H. Topological index. A newly proposed quantity characterizing the topological nature of structural isomers of saturated hydrocarbons. Bull. Chem. Soc. Jpn. 1971, 4, 2332–2339. [Google Scholar] [CrossRef]
- Dehmer, M.; Emmert-Streib, F. Quantitative Graph Theory: Mathematical Foundations and Applications; Discrete Mathematics and Its Applications; Chapman and Hall/CRC: London, UK, 2014. [Google Scholar]
- Dobrynin, A.A.; Entringer, R.; Gutman, I. Wiener index for trees: Theory and applications. Acta Appl. Math. 2001, 340, 211–249. [Google Scholar] [CrossRef]
- Dobrynin, A.A.; Gutman, I.; Klavžar, S.; Žigert, P. Wiener index of hexagonal systems. Acta Appl. Math. 2002, 72, 247–294. [Google Scholar] [CrossRef]
- Gutman, I.; Furtula, B. Distance in Molecular Graphs—Theory; Mathematical Chemistry Monographs, 12; University of Kragujevac: Kragujevac, Serbia, 2012. [Google Scholar]
- Gutman, I.; Furtula, B. Distance in Molecular Graphs—Applications; Mathematical Chemistry Monographs, 13; University of Kragujevac: Kragujevac, Serbia, 2012. [Google Scholar]
- Gutman, I.; Polansky, O.E. Mathematical Concepts in Organic Chemistry; Springer: Berlin, Germany, 1986. [Google Scholar]
- Todeschini, R.; Consonni, V. Handbook of Molecular Descriptors; Wiley–VCH: Weinheim, Germany, 2000. [Google Scholar]
- Trinajstić, N. Chemical Graph Theory, 2nd ed.; CRC Press: Boca Raton, FL, USA, 1992. [Google Scholar]
- Knor, M.; Škrekovski, R.; Tepeh, A. Mathematical aspects of Wiener index. Ars Mathematica Contemporanea 2016, 11, 327–352. [Google Scholar] [CrossRef]
- Ashrafi, A.R. Wiener index of nanotubes, toroidal fullerenes and nanostars. In The Mathematics and Topology of Fullerenes; Cataldo, F., Graovac, A., Ori, O., Eds.; Carbon Materials: Chemistry and Physics Book 4; Springer: Berlin, Germany, 2011; pp. 21–38. [Google Scholar]
- Alizadeh, Y.; Klavžar, S. Complexity of topological indices: The case of connective eccentric index. MATCH Commun. Math. Comput. Chem. 2016, 76, 659–667. [Google Scholar]
- Alizadeh, Y.; Klavžar, S. On graphs whose Wiener complexity equals their order and on Wiener index of asymmetric graphs. Appl. Math. Comput. 2018, 328, 113–118. [Google Scholar] [CrossRef]
- Klavžar, S.; Jemilet, D.A.; Rajasingh, I.; Manuel, P.; Parthiban, N. General transmission lemma and Wiener complexity of triangular grids. Appl. Math. Comput. 2018, 338, 115–122. [Google Scholar] [CrossRef]
- Dobrynin, A.A. On 2-connected transmission irregular graphs. J. Appl. Ind. Math. 2018, 28, 642–647. [Google Scholar] [CrossRef]
- Dobrynin, A.A. Infinite family of 2-connected transmission irregular graphs. Appl. Math. Comput. 2019, 340, 1–4. [Google Scholar] [CrossRef]
- Dobrynin, A.A. Infinite family of transmission irregular trees of even order. Discrete Math. 2019, 340, 74–77. [Google Scholar] [CrossRef]
- Dobrynin, A.A. Infinite family of 3-connected cubic transmission irregular graphs. Discrete Appl. Math. 2019, 340, 151–157. [Google Scholar] [CrossRef]



| n | W | D | D | t | n | W | D | D | t | |||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 20 | 1 | 500 | 5 | 500 | 1 | 5 | 84 | 70 | 19,939 | 13 | 21,754 | 21 | 15 | |||
| 24 | 2 | 804 | 5 | 804 | 2 | 5 | 20,076 | 13 | ||||||||
| 26 | 2 | 987 | 6 | 987 | 2 | 6 | b | 86 | 73 | 21,404 | 13 | 23,467 | 8 | 16 | b | |
| 28 | 5 | 1198 | 6 | 1198 | 5 | 6 | 21,521 | 13 | ||||||||
| 30 | 7 | 1431 | 6 | 1435 | 3 | 6 | a | 21,593 | 13 | |||||||
| 32 | 9 | 1688 | 6 | 1696 | 3 | 7 | b | 88 | 73 | 22,359 | 13 | 24,714 | 21 | 16 | ||
| 34 | 10 | 1973 | 7 | 1978 | 10 | 7 | 22,421 | 13 | ||||||||
| 1978 | 7 | 22,604 | 13 | |||||||||||||
| 36 | 14 | 2288 | 7 | 2298 | 8 | 7 | 22,616 | 13 | ||||||||
| 38 | 18 | 2627 | 7 | 2651 | 4 | 8 | b | 22,619 | 13 | |||||||
| 40 | 19 | 3001 | 7 | 3035 | 4 | 8 | a | 22,750 | 14 | |||||||
| 42 | 22 | 3397 | 8 | 3415 | 19 | 8 | 22,939 | 14 | ||||||||
| 44 | 25 | 3830 | 8 | 3888 | 4 | 9 | b | 90 | 79 | 23,923 | 14 | 27,155 | 9 | 17 | a | |
| 46 | 25 | 4285 | 8 | 4322 | 19 | 9 | 92 | 80 | 25,731 | 15 | 28,256 | 8 | 17 | b | ||
| 4289 | 8 | 94 | 82 | 26,793 | 14 | 28,910 | 44 | 17 | ||||||||
| 4289 | 8 | 96 | 84 | 28,274 | 14 | 31,418 | 24 | 17 | ||||||||
| 4291 | 8 | 28,317 | 15 | |||||||||||||
| 48 | 30 | 4795 | 9 | 4858 | 12 | 9 | 98 | 86 | 30,068 | 15 | 33,651 | 9 | 18 | b | ||
| 50 | 35 | 5310 | 9 | 5455 | 5 | 9 | a | 100 | 89 | 31,196 | 15 | 36,580 | 10 | 19 | a | |
| 52 | 36 | 5876 | 9 | 5994 | 13 | 10 | 102 | 89 | 32984 | 15 | 36,206 | 47 | 18 | |||
| 54 | 37 | 6475 | 9 | 6558 | 22 | 10 | 33,070 | 15 | ||||||||
| 56 | 40 | 7114 | 10 | 7352 | 5 | 11 | b | 33,226 | 15 | |||||||
| 58 | 43 | 7782 | 10 | 7910 | 25 | 11 | 33,505 | 15 | ||||||||
| 7822 | 10 | 104 | 91 | 34,402 | 15 | 39,688 | 9 | 19 | b | |||||||
| 60 | 44 | 8437 | 10 | 8880 | 6 | 11 | a | 34,529 | 15 | |||||||
| 8466 | 10 | 36,801 | 17 | |||||||||||||
| 8490 | 10 | 106 | 93 | 36,648 | 16 | 40,278 | 47 | 19 | ||||||||
| 62 | 46 | 9202 | 10 | 9651 | 6 | 12 | b | 36,664 | 16 | |||||||
| 9220 | 11 | 37,594 | 17 | |||||||||||||
| 9250 | 11 | 108 | 96 | 38,033 | 15 | 43,578 | 27 | 19 | ||||||||
| 64 | 49 | 9988 | 11 | 10,410 | 15 | 12 | 110 | 98 | 40,154 | 16 | 48,005 | 11 | 21 | a | ||
| 9993 | 11 | 41,419 | 17 | |||||||||||||
| 10,003 | 11 | 112 | 100 | 41,940 | 17 | 48,234 | 27 | 20 | ||||||||
| 10,013 | 11 | 114 | 102 | 43,885 | 16 | 49,318 | 52 | 20 | ||||||||
| 10,016 | 11 | 116 | 102 | 45,437 | 16 | 53,832 | 10 | 21 | b | |||||||
| 66 | 50 | 10,814 | 11 | 11,126 | 30 | 12 | 46,632 | 17 | ||||||||
| 10,842 | 11 | 46,798 | 17 | |||||||||||||
| 68 | 56 | 11,714 | 11 | 12,376 | 6 | 13 | b | 47,927 | 18 | |||||||
| 70 | 56 | 12,589 | 11 | 13,505 | 7 | 13 | a | 118 | 106 | 47,059 | 15 | 54,310 | 50 | 21 | ||
| 72 | 56 | 13,407 | 11 | 14,298 | 18 | 13 | 47,489 | 16 | ||||||||
| 13,448 | 11 | 47,697 | 16 | |||||||||||||
| 13,453 | 11 | 120 | 110 | 49,143 | 16 | 61,630 | 12 | 23 | a | |||||||
| 13,567 | 12 | 49,606 | 17 | |||||||||||||
| 13,578 | 12 | 122 | 109 | 51,344 | 16 | 62,011 | 11 | 22 | b | |||||||
| 13,766 | 12 | 51,456 | 16 | |||||||||||||
| 74 | 61 | 14,521 | 12 | 15,563 | 7 | 14 | b | 51,933 | 17 | |||||||
| 76 | 63 | 15,867 | 13 | 16,554 | 18 | 14 | 52,974 | 17 | ||||||||
| 78 | 64 | 16,834 | 13 | 17,398 | 37 | 14 | 53,070 | 17 | ||||||||
| 16,877 | 13 | 124 | 111 | 54,105 | 17 | 64,170 | 30 | 22 | ||||||||
| 80 | 66 | 17,727 | 13 | 19,530 | 8 | 15 | a | 55,050 | 18 | |||||||
| 17,832 | 13 | 55,789 | 18 | |||||||||||||
| 82 | 71 | 19,075 | 13 | 19,918 | 38 | 15 | 57,358 | 19 | ||||||||
| 57,473 | 19 | |||||||||||||||
| 126 | 115 | 57,238 | 18 | 65,286 | 57 | 22 | 186 | 177 | 167,300 | 26 | 198,046 | 82 | 32 | |||
| 128 | 117 | 60,434 | 18 | 70,976 | 11 | 23 | b | 188 | 177 | 154,868 | 21 | 211,776 | 16 | 33 | b | |
| 130 | 118 | 63,736 | 19 | 77,655 | 13 | 25 | a | 190 | 180 | 169,849 | 24 | 235,405 | 19 | 37 | a | |
| 63,922 | 19 | 192 | 181 | 163,370 | 22 | 222,778 | 48 | 33 | ||||||||
| 65,396 | 20 | 194 | 183 | 187,947 | 27 | 231,763 | 17 | 34 | b | |||||||
| 132 | 121 | 62,917 | 17 | 76,538 | 33 | 23 | 191,290 | 27 | ||||||||
| 134 | 123 | 64,935 | 17 | 80,763 | 12 | 24 | b | 196 | 184 | 174,774 | 23 | 236,394 | 48 | 34 | ||
| 65,225 | 17 | 178,192 | 24 | |||||||||||||
| 68,161 | 19 | 178,529 | 25 | |||||||||||||
| 136 | 124 | 69,838 | 19 | 83,274 | 33 | 24 | 179,284 | 25 | ||||||||
| 138 | 127 | 72,311 | 19 | 84,398 | 62 | 24 | 184,011 | 24 | ||||||||
| 73,771 | 19 | 198 | 187 | 177,296 | 23 | 237,198 | 87 | 34 | ||||||||
| 140 | 131 | 73,644 | 19 | 96,280 | 14 | 27 | a | 189,530 | 25 | |||||||
| 142 | 131 | 79,852 | 20 | 91,518 | 56 | 25 | 200 | 189 | 180,683 | 23 | 273,830 | 20 | 39 | a | ||
| 144 | 132 | 77,934 | 18 | 97,914 | 36 | 25 | 192,365 | 25 | ||||||||
| 78,924 | 18 | 202 | 193 | 210,388 | 28 | 251,318 | 71 | 35 | ||||||||
| 146 | 134 | 86,095 | 20 | 102,947 | 13 | 26 | b | 204 | 192 | 189,450 | 22 | 265,274 | 51 | 35 | ||
| 86,287 | 21 | 206 | 195 | 221,909 | 28 | 275,427 | 18 | 36 | b | |||||||
| 87,298 | 21 | 221,995 | 28 | |||||||||||||
| 87,442 | 21 | 222,097 | 28 | |||||||||||||
| 148 | 136 | 86,432 | 20 | 105,834 | 36 | 26 | 208 | 198 | 201,644 | 23 | 280,554 | 51 | 36 | |||
| 150 | 138 | 87,886 | 19 | 117,705 | 15 | 29 | a | 210 | 199 | 238,572 | 29 | 316,255 | 21 | 41 | a | |
| 88,860 | 19 | 212 | 199 | 207,617 | 23 | 299,176 | 18 | 37 | b | |||||||
| 92,732 | 21 | 207,975 | 23 | |||||||||||||
| 93,898 | 21 | 209,707 | 23 | |||||||||||||
| 152 | 141 | 92,988 | 20 | 115,416 | 13 | 27 | b | 211,942 | 24 | |||||||
| 154 | 144 | 97,359 | 21 | 115,270 | 59 | 27 | 215,779 | 24 | ||||||||
| 156 | 144 | 95,579 | 19 | 122,938 | 39 | 27 | 228,285 | 26 | ||||||||
| 96,997 | 19 | 228,507 | 26 | |||||||||||||
| 98,864 | 21 | 228,922 | 26 | |||||||||||||
| 158 | 147 | 100,055 | 19 | 128,851 | 14 | 28 | b | 214 | 202 | 226,652 | 25 | 297,030 | 74 | 37 | ||
| 160 | 148 | 103,952 | 20 | 142,130 | 16 | 31 | a | 247,352 | 29 | |||||||
| 108,170 | 22 | 250,978 | 29 | |||||||||||||
| 162 | 151 | 104,909 | 19 | 133,206 | 72 | 28 | 216 | 204 | 220,131 | 23 | 312,858 | 54 | 37 | |||
| 116,278 | 23 | 226,928 | 25 | |||||||||||||
| 164 | 153 | 110,088 | 20 | 143,288 | 14 | 29 | b | 240,920 | 27 | |||||||
| 166 | 155 | 117,531 | 21 | 142,838 | 62 | 29 | 270,770 | 33 | ||||||||
| 119,485 | 23 | 218 | 209 | 260,324 | 30 | 324,251 | 19 | 38 | b | |||||||
| 168 | 157 | 114,316 | 18 | 151,898 | 42 | 29 | 220 | 211 | 233,711 | 25 | 362,880 | 22 | 43 | a | ||
| 126,632 | 23 | 222 | 211 | 240,755 | 26 | 330,406 | 97 | 38 | ||||||||
| 170 | 159 | 123,193 | 22 | 169,755 | 17 | 33 | a | 273,605 | 30 | |||||||
| 130,548 | 24 | 273,701 | 30 | |||||||||||||
| 172 | 160 | 129,708 | 22 | 162,474 | 42 | 30 | 273,813 | 30 | ||||||||
| 174 | 164 | 131,354 | 23 | 163,478 | 77 | 30 | 224 | 214 | 248,633 | 27 | 350,688 | 19 | 39 | b | ||
| 176 | 165 | 130,105 | 20 | 175,312 | 15 | 31 | b | 226 | 217 | 288,789 | 31 | 347,998 | 77 | 39 | ||
| 178 | 167 | 141,743 | 23 | 174,510 | 65 | 31 | 228 | 218 | 258,913 | 25 | 365,818 | 57 | 39 | |||
| 150,696 | 25 | 230 | 221 | 269,286 | 27 | 413,905 | 23 | 45 | a | |||||||
| 180 | 168 | 139,697 | 21 | 200,780 | 18 | 35 | a | 295,719 | 31 | |||||||
| 182 | 171 | 144,410 | 21 | 192,971 | 16 | 32 | b | 232 | 221 | 292,719 | 28 | 384,714 | 57 | 40 | ||
| 184 | 172 | 146,581 | 22 | 197,130 | 45 | 32 | ||||||||||
| 147,054 | 21 | |||||||||||||||
| 153,615 | 23 | |||||||||||||||
| 154,923 | 23 |
| n | W | D | D | t | n | W | D | D | t | |||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 60 | 1 | 8340 | 9 | 8340 | 1 | 9 | 156 | 130 | 94,028 | 17 | 95,340 | 37 | 18 | c | ||
| 70 | 5 | 12,375 | 10 | 12,375 | 5 | 10 | 158 | 132 | 97,061 | 17 | 98,238 | 72 | 18 | d | ||
| 72 | 4 | 13,284 | 10 | 13,284 | 5 | 10 | 160 | 135 | 100,031 | 17 | 103,500 | 9 | 19 | |||
| 74 | 6 | 14,275 | 10 | 14,275 | 6 | 10 | 162 | 136 | 103,213 | 17 | 104,799 | 72 | 18 | d | ||
| 76 | 13 | 15,248 | 10 | 15,294 | 4 | 10 | 103,842 | 18 | ||||||||
| 78 | 14 | 16,305 | 11 | 16,365 | 8 | 11 | 164 | 137 | 106,920 | 18 | 108,598 | 40 | 19 | c | ||
| 80 | 17 | 17,412 | 11 | 17,600 | 1 | 11 | 166 | 141 | 109,926 | 18 | 111,660 | 77 | 18 | d | ||
| 82 | 19 | 18,533 | 11 | 18,633 | 7 | 11 | 168 | 142 | 113,094 | 17 | 116,100 | 18 | 19 | |||
| 84 | 25 | 19,664 | 11 | 19,734 | 10 | 11 | 113,725 | 18 | ||||||||
| 86 | 39 | 20,864 | 11 | 21,007 | 14 | 11 | 170 | 145 | 116,472 | 17 | 121,575 | 10 | 20 | |||
| 88 | 36 | 22,097 | 11 | 22,244 | 14 | 11 | 116,713 | 18 | ||||||||
| 90 | 39 | 23,406 | 12 | 23,546 | 15 | 11 | 117,141 | 19 | ||||||||
| 23,445 | 12 | 172 | 146 | 120,328 | 18 | 123,010 | 41 | 20 | c | |||||||
| 92 | 41 | 24,761 | 12 | 24,890 | 13 | 11 | 120,379 | 18 | ||||||||
| 94 | 48 | 26,111 | 12 | 26,262 | 21 | 12 | 120,404 | 18 | ||||||||
| 96 | 49 | 27,589 | 12 | 27,738 | 6 | 11 | 120,859 | 18 | ||||||||
| 98 | 55 | 29,016 | 12 | 29,164 | 24 | 12 | 174 | 148 | 123,729 | 18 | 126,254 | 78 | 19 | d | ||
| 100 | 55 | 30,567 | 12 | 30,770 | 10 | 13 | 123,765 | 18 | ||||||||
| 30,590 | 12 | 123,767 | 18 | |||||||||||||
| 30,551 | 13 | 124,309 | 19 | |||||||||||||
| 102 | 59 | 32,133 | 13 | 32,275 | 28 | 12 | 176 | 150 | 127,275 | 18 | 130,684 | 44 | 20 | c | ||
| 104 | 65 | 33,677 | 12 | 33,946 | 7 | 13 | 127,354 | 18 | ||||||||
| 106 | 69 | 35,406 | 13 | 35,547 | 32 | 13 | 127,583 | 18 | ||||||||
| 108 | 70 | 37,097 | 13 | 37,296 | 26 | 13 | 178 | 156 | 131,619 | 19 | 134,008 | 82 | 20 | d | ||
| 110 | 72 | 38,849 | 13 | 39,055 | 7 | 14 | 180 | 155 | 135,137 | 19 | 141,540 | 10 | 21 | |||
| 112 | 74 | 40,689 | 13 | 40,878 | 10 | 13 | 135,577 | 19 | ||||||||
| 114 | 76 | 42,494 | 13 | 42,753 | 17 | 13 | 182 | 158 | 138,427 | 19 | 142,031 | 78 | 20 | d | ||
| 42,500 | 13 | 184 | 159 | 142,077 | 18 | 146,918 | 45 | 21 | c | |||||||
| 116 | 80 | 44,426 | 14 | 44,616 | 9 | 13 | 142,114 | 18 | ||||||||
| 44,434 | 13 | 186 | 163 | 146,825 | 19 | 151,887 | 20 | 21 | ||||||||
| 118 | 81 | 46,334 | 13 | 46,629 | 33 | 13 | 147,381 | 20 | 151,887 | 26 | 21 | |||||
| 120 | 87 | 48,386 | 14 | 48,820 | 7 | 15 | 188 | 165 | 150,899 | 20 | 155,512 | 46 | 21 | c | ||
| 122 | 89 | 50,445 | 14 | 50,691 | 26 | 14 | 190 | 169 | 154,949 | 20 | 163,615 | 11 | 22 | |||
| 50,473 | 14 | 192 | 170 | 158,798 | 19 | 164,434 | 47 | 22 | c | |||||||
| 124 | 91 | 52,593 | 14 | 52,830 | 11 | 13 | 194 | 171 | 162,674 | 19 | 168,005 | 88 | 21 | d | ||
| 52,635 | 14 | 162,770 | 19 | |||||||||||||
| 126 | 93 | 54,737 | 14 | 54,950 | 52 | 14 | 196 | 176 | 167,808 | 20 | 173,708 | 46 | 22 | c | ||
| 128 | 96 | 57,026 | 15 | 57,240 | 32 | 15 | c | 198 | 175 | 171,657 | 20 | 177,339 | 92 | 22 | d | |
| 130 | 97 | 59,208 | 14 | 60,095 | 8 | 16 | 200 | 180 | 176,651 | 20 | 187,780 | 11 | 23 | |||
| 59,221 | 15 | 202 | 178 | 180,593 | 20 | 186,984 | 94 | 22 | d | |||||||
| 59,328 | 15 | 180,818 | 21 | |||||||||||||
| 132 | 100 | 61,579 | 15 | 62,097 | 14 | 16 | 181,279 | 21 | ||||||||
| 61,609 | 15 | 62,097 | 20 | 16 | 182,321 | 20 | ||||||||||
| 134 | 102 | 63,929 | 15 | 64,270 | 57 | 16 | d | 204 | 180 | 185,306 | 20 | 194,376 | 22 | 23 | ||
| 64,087 | 15 | 185,306 | 20 | 194,376 | 28 | 23 | ||||||||||
| 136 | 105 | 66,439 | 16 | 66,880 | 33 | 16 | c | 185,410 | 21 | |||||||
| 138 | 108 | 68,859 | 15 | 69,285 | 57 | 16 | d | 206 | 183 | 188,946 | 20 | 196,937 | 92 | 22 | d | |
| 68,865 | 15 | 208 | 186 | 196,192 | 21 | 203,528 | 50 | 23 | c | |||||||
| 68,911 | 16 | 210 | 188 | 199,403 | 21 | 214,255 | 12 | 24 | ||||||||
| 69,075 | 16 | 199,484 | 21 | |||||||||||||
| 140 | 111 | 71,543 | 16 | 72,860 | 8 | 17 | 212 | 189 | 203,781 | 20 | 214,178 | 51 | 24 | c | ||
| 142 | 114 | 74,058 | 16 | 74,558 | 64 | 16 | d | 204,706 | 21 | |||||||
| 144 | 117 | 76,661 | 16 | 77,480 | 34 | 17 | c | 205,041 | 21 | |||||||
| 146 | 117 | 79,350 | 16 | 80,068 | 65 | 17 | d | 214 | 191 | 209,844 | 21 | 217,915 | 95 | 23 | d | |
| 148 | 119 | 82,141 | 16 | 83,160 | 35 | 17 | c | 209,858 | 21 | |||||||
| 150 | 127 | 85,249 | 17 | 87,335 | 9 | 18 | 209,885 | 21 | ||||||||
| 152 | 124 | 88,004 | 17 | 89,106 | 38 | 18 | c | 210,112 | 21 | |||||||
| 154 | 127 | 90,792 | 16 | 91,921 | 68 | 18 | d | 216 | 192 | 213,004 | 20 | 225,212 | 51 | 24 | c | |
| 90,969 | 17 | 213,920 | 21 | |||||||||||||
| 91,173 | 17 | |||||||||||||||
| 218 | 195 | 218,701 | 21 | 228,952 | 102 | 23 | d | 244 | 223 | 294,943 | 23 | 313,078 | 58 | 27 | c | |
| 219,051 | 21 | 246 | 225 | 298,839 | 23 | 316,562 | 117 | 26 | d | |||||||
| 219,302 | 21 | 248 | 228 | 310,038 | 25 | 327,240 | 60 | 27 | c | |||||||
| 219,702 | 22 | 250 | 228 | 313,548 | 24 | 344,535 | 14 | 28 | ||||||||
| 220 | 197 | 223,863 | 21 | 243,020 | 12 | 25 | 252 | 231 | 323,180 | 25 | 341,826 | 63 | 28 | c | ||
| 224,835 | 21 | 328,878 | 26 | |||||||||||||
| 226,742 | 22 | 254 | 233 | 324,097 | 23 | 345,088 | 121 | 26 | d | |||||||
| 222 | 199 | 230,922 | 22 | 244,209 | 24 | 25 | 256 | 235 | 328,374 | 23 | 356,860 | 63 | 28 | c | ||
| 244,209 | 28 | 25 | 328,471 | 23 | ||||||||||||
| 224 | 202 | 235,105 | 21 | 248,358 | 55 | 25 | c | 329,926 | 24 | |||||||
| 226 | 204 | 237,981 | 21 | 252,089 | 101 | 24 | d | 258 | 237 | 336,694 | 24 | 368,499 | 30 | 29 | ||
| 228 | 205 | 246,587 | 22 | 260,504 | 53 | 25 | c | 368,499 | 26 | 29 | ||||||
| 230 | 208 | 252,526 | 23 | 274,295 | 13 | 26 | 260 | 240 | 348,484 | 25 | 383,700 | 14 | 29 | |||
| 232 | 210 | 260,165 | 23 | 273,042 | 57 | 26 | c | 262 | 242 | 349,765 | 24 | 374,298 | 123 | 27 | d | |
| 262,089 | 23 | 264 | 242 | 357,069 | 24 | 388,198 | 65 | 29 | c | |||||||
| 234 | 213 | 262,982 | 23 | 276,696 | 107 | 25 | d | 358,221 | 24 | |||||||
| 236 | 215 | 267,862 | 22 | 285,996 | 58 | 26 | c | 360,379 | 24 | |||||||
| 238 | 216 | 275,410 | 23 | 289,583 | 111 | 25 | d | 362,260 | 25 | |||||||
| 276,644 | 23 | 266 | 246 | 364,584 | 25 | 391,052 | 127 | 28 | d | |||||||
| 240 | 219 | 282,476 | 23 | 308,060 | 13 | 27 | 268 | 247 | 380,916 | 26 | 404,536 | 67 | 29 | c | ||
| 242 | 222 | 292,194 | 25 | 302,885 | 114 | 26 | d | 270 | 250 | 375,313 | 24 | 425,775 | 15 | 30 |
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | |
| N | 9 | 8 | 27 | 66 | 45 | 42 | 9 | 1 |
| 0 | 1 | 2 | 3 | 4 | 5 | |
| N | 23 | 56 | 50 | 47 | 26 | 5 |
| Family | Family | ||||
| n | D | n | D | ||
| Family | Family | ||||
| n | D | n | D | ||
| n | 150 | 186 | 222 | 258 | 132 | 168 | 204 | 240 | 276 |
| 2 | 3 | 4 | 5 | ||||||
| 2 | 3 | 4 | 5 | ||||||
| 2 | 3 | 4 | 5 | 6 | |||||
| 2 | 3 | 4 | 5 | 6 | |||||
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dobrynin, A.A.; Vesnin, A.Y. On the Wiener Complexity and the Wiener Index of Fullerene Graphs. Mathematics 2019, 7, 1071. https://doi.org/10.3390/math7111071
Dobrynin AA, Vesnin AY. On the Wiener Complexity and the Wiener Index of Fullerene Graphs. Mathematics. 2019; 7(11):1071. https://doi.org/10.3390/math7111071
Chicago/Turabian StyleDobrynin, Andrey A., and Andrei Yu Vesnin. 2019. "On the Wiener Complexity and the Wiener Index of Fullerene Graphs" Mathematics 7, no. 11: 1071. https://doi.org/10.3390/math7111071
APA StyleDobrynin, A. A., & Vesnin, A. Y. (2019). On the Wiener Complexity and the Wiener Index of Fullerene Graphs. Mathematics, 7(11), 1071. https://doi.org/10.3390/math7111071