1. Introduction
The coupling problem of structure and fluid interaction is a classic problem interested in various engineering applications such as vehicle dynamics, aircraft dynamics, and storage tanks [
1,
2,
3,
4,
5]. The storage tanks usually built from strong materials such as steels (for drums), stainless steels, concrete, aluminum alloys, fiber-reinforced plastics (for fiber drum), and Timber which can bear large elastic vibrational energy. These tanks are exposed to dead and live loads such as snow, wind (vortex oscillation), seismic, buckling, and earth pressures. When the structure is assumed to have the many mass vibration system, modal analysis is a useful tool for the design of yield shear force of the convective mass vibration, damage to the structure (cumulative plastic strain energy), and water pressure imposed on the tank. That analysis needs the natural frequency of the maximum number of vibration modes that influence seismic responses.
One of the first analytical methods proposed to solve that problem is the Rayleigh–Ritz method [
6]. The application of the method of Rayleigh–Ritz on the structure and fluid interaction with weak coupling [
5] and strong coupling [
7] was investigated by Amabili [
7]. Various methods are applied to find the solution of the coupled fluid-structure interaction such as artificial spring method [
8], finite element formulation [
9], Galerkin method [
10], time-marching technique [
11], collocation method [
12], Rayleigh quotients method [
13], boundary elements method [
14], boundary integral equation method [
15]. Various numerical methods such as finite element, collocation, Rayleigh, boundary elements, and boundary integral method are the approximation to the exact solution. In the Rayleigh–Ritz method, the approximations to eigenvalue equations are found [
5]. The finite element method analytical solution of boundary value problems is used to get a system of algebraic equations. In the Galerkin method [
10] which is the popular method of finite element formulation [
9], the differential equation is converted from a continuous operator problem to a discrete problem. In the collocation method, the idea is to choose a finite-dimensional space of proposed polynomial solutions and several domain points that satisfy the given equation at the collocation points [
12]. In the boundary element method [
14] the integral equations are solved by Green’s function elements connecting pairs of source and field patches defined by the mesh form a matrix. The boundary element method [
15] is often more efficient than other methods [
2] when there is a small surface to volume ratio. Conceptually, it works by constructing the surface mesh. However, for many problems boundary element methods are significantly less efficient than volume-discretization methods (finite element method, finite difference method, finite volume method). The added complexity of the boundary integral equation method could be overwhelmed by Compression techniques. Computational time and the storage requirements by boundary element formulations grow according to the square of the problem size while the finite element matrices are typically grown linearly with the problem size.
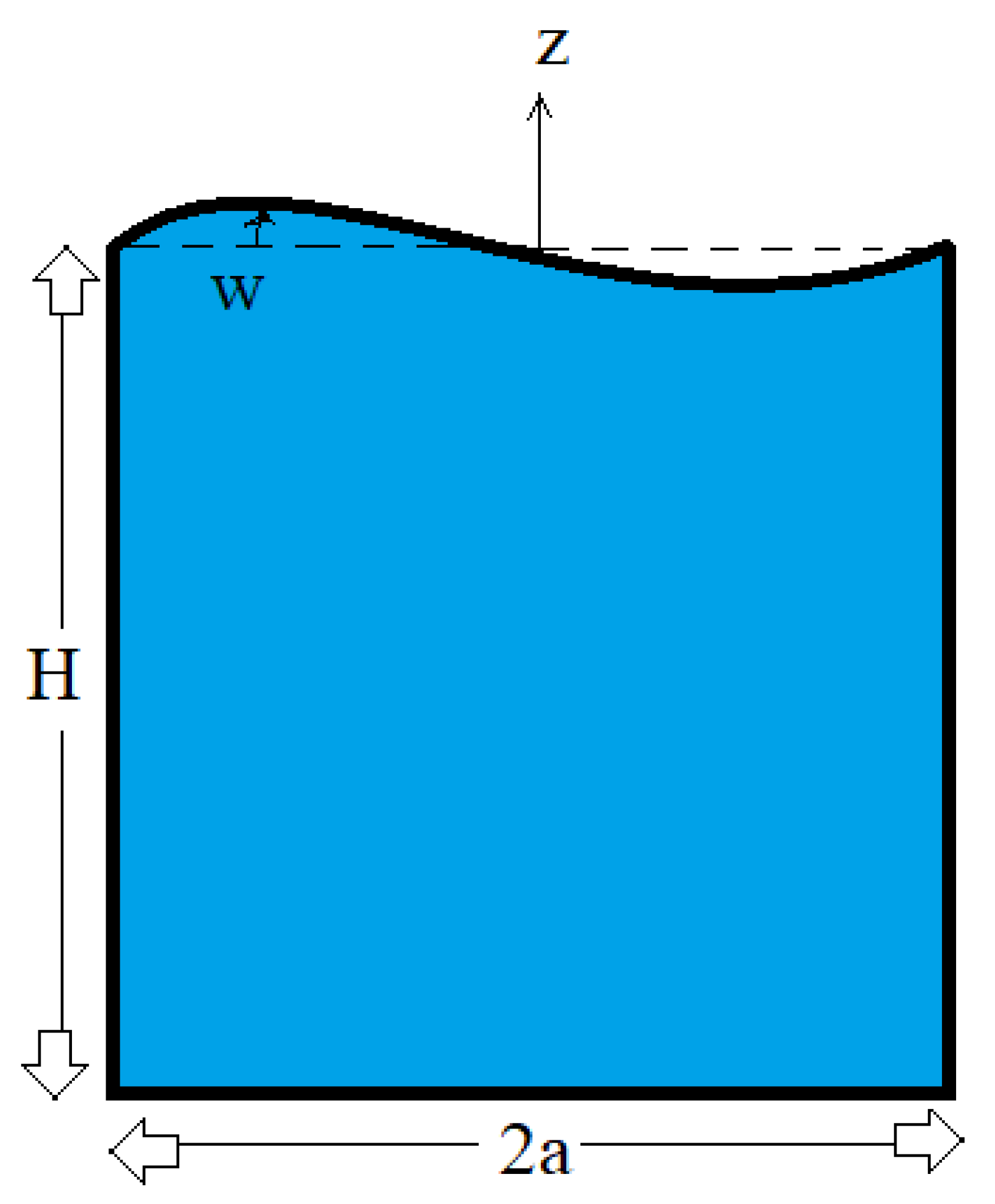
The fluid-structure coupling problem considered for various fluids such as dense fluids [
16], the frictionless liquid in zero gravity [
17], and compressible fluid [
18], filled with non-viscous liquid [
19] and various filling ratios for fluid-filled [
19] and partially filled [
20] were discussed in the literature. One of the most interesting geometries is a circular cylinder container. A drum (also called barrels in common usage) used in the shipping of dangerous powders and liquids, with 208 liters volume, has an 880 millimeters tall and 610 millimeters diameter (
). Similar geometry is used for a barrel of crude oil (72 cm × 43 cm).
On the other hand, the types of solid structures connected with fluid were composed of cylindrical baffles [
21], annular cylindrical tanks [
22], ring-stiffeners and flexible bottom [
23], annular baffle [
24], vertical baffle [
25], submerged components [
26], internal bodies [
27,
28], two identical plates [
29], immersed plate in a container [
30]. As well the elastic structure bonding the fluids were initially bent cylindrical shells [
31] and composite shells with orthogonal stiffeners [
32]. Based on the size the containers are flexible and rigid. The design of a fluid-structure coupling problem in a cylindrical tank is an interesting research theme with possible engineering practical applications on stiffeners. Drums have chimes or rims at ends (chines). Most steel drums have reinforcing rolling hoops or rings of thickened metal or plastic. Amabili [
23] investigated the effect of ring-stiffeners and flexible bottom on sloshing modes in cylindrical containers and drums.
Amabili [
33] addresses a research theme in the area of fluid–solid interaction, focusing the analytical solution of an interesting problem of coupled vibration of a fluid-filled cylindrical rigid tank with an elastic top cover. The two kinds of drums are barrels (welded top) and the open top. Vibrations of a circular flexible membrane or elastic plates resting on a sloshing liquid is a class of problem which first studied by Amabili [
33], for annular plate cover by Kim and Lee [
34] and fully covered case by Bauer [
35]. Bauer [
35] makes use of the Fourier–Bessel series expansion to analytically derive the solution of the addressed problem, and perform a parametric study on the natural frequency of the coupled fluid-structure interaction in clamped boundary condition and for varying characteristic values of the system.
Considering all of the above, there is no research performed on the analytical solution of rigid tank coupling with elastic cover with different support conditions. The analytical solution is not easy to use by engineers but is the exact solution of the problem and has the benefits of a minimum number of parameters and accuracy over the other methods such as finite element, collocation, Rayleigh, boundary elements, and boundary integral method. The aim of the current study is finding the analytical solution of a fluid-filled cylindrical container with rigid sides and bottom and elastic circular plate over the top surface. In the next section mathematical model of the system in the fluid and solid parts is presented.
5. Results and Discussion
The analytical solutions which are obtained in the previous section, are investigated numerically for the practical case. The numerical results in the current section have been derived by developing code inside the MATLAB software. The natural frequencies are presented in
unit where
. Usual material properties of the water tank storage are given in
Table 5. The values are not fixed and are averaged from various references. For example, the stone Poisson ratio is reported between 0.2 and 0.3 in various references but the value of 0.25 is chosen for the table. The last two rows of the
Table 5 calculated for the special case of
h = 1 mm and
a = 1 m. As shown for that values the first parameter
is in order of
and second parameter
is in order of
. The geometrical and physical properties of the fluid material and solid material are presented in
Table 6. Relative errors of computing axi-symmetric modes of the clamped cover plates are presented in
Table 7.
Table 7 presents the relative error (
) versus mode number and aspect ratios where the solution with ten expansion terms is considered as the exact frequency (
M = 10). The performance of the method is comparable with the Table 4 in Amabili [
33] where the convergence of the solution was discussed. As shown the use of the current method causes the precise results in most cases by three terms in fluid expansion series with three terms. Table 4 in Amabili [
33] used 10 terms for the solid motion (in
Table 7 just one term is used for solid deflection) and 50 terms for liquid potential (As seen in
Table 7 the
M = 3 matches the exact solution). Assessment of the non-dimensional angular velocity (
) versus height ratio of the tank (
) for the boundary condition of the clamped solid at the three axi-symmetric modes with minimum values (
and
) against the values presented in Tables 1 and 2 of Amabili [
33] are presented in
Table 8. As shown a good agreement seen among the current study results and those of Bauer ([
35]) and Amabili ([
33]) for axi-symmetric modes of the clamped cover plates (for
and
modes). Results were found by one-term expansion of the solid plate deflection and 4 terms for the liquid potential.
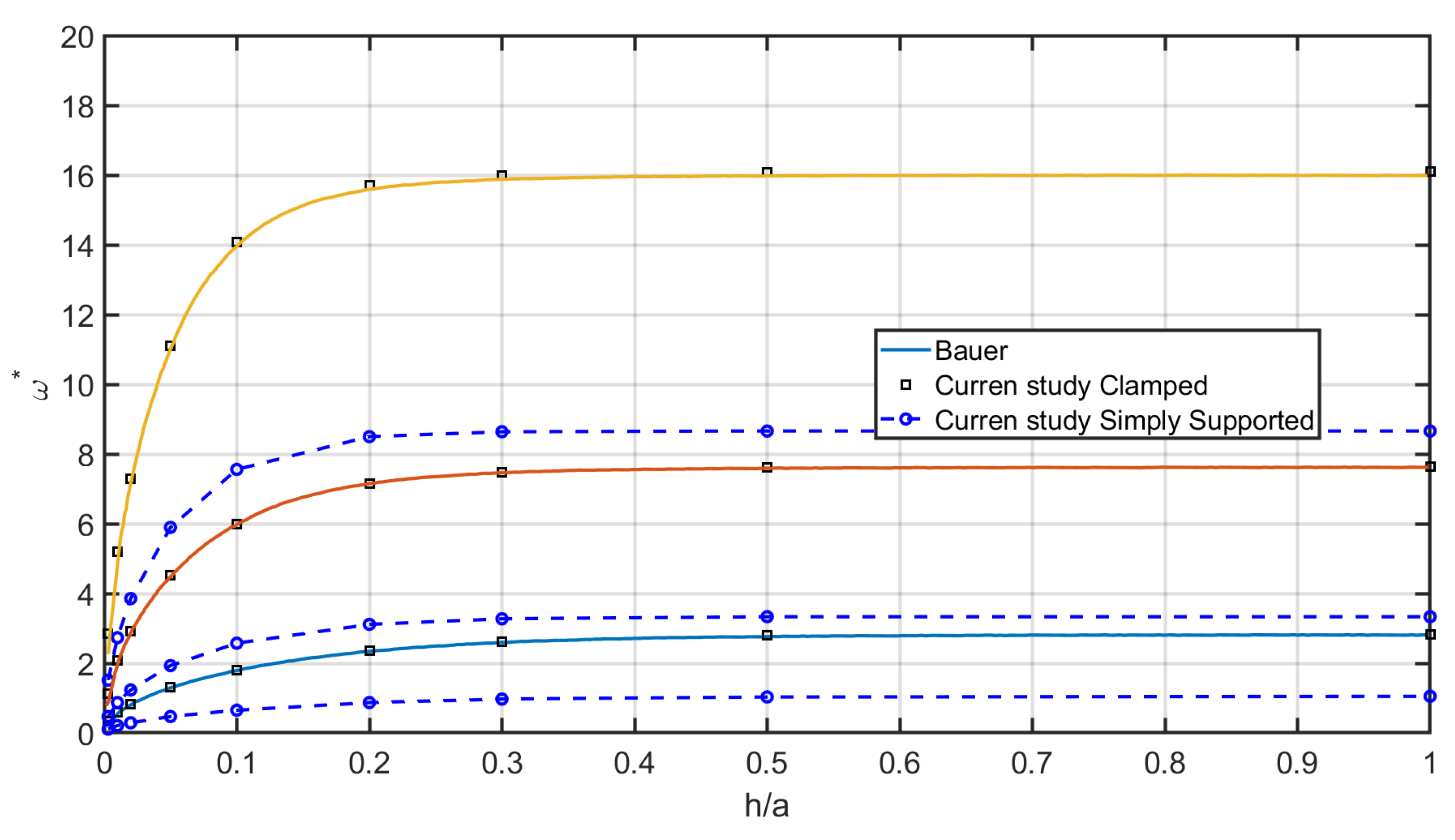
The non-dimensional fluid-structure frequencies for simply supported boundary condition (presented by the dashed line in
Figure 3) and clamped boundary condition (presented by solid line in
Figure 3) at
,
, and axi-symmetric mode
is plotted in
Figure 3. As shown, the agreement of results of the current study with non-dimensional fluid-structure frequencies for clamped plate obtained by Bauer ([
35]) is perfect. As exposed in all cases the clamped boundary condition caused higher frequencies than the simply supported plate.
5.1. Clamped Plate
Assessment of the present results and the Bauer ([
35]) results for other figures in plate cover case has been performed in
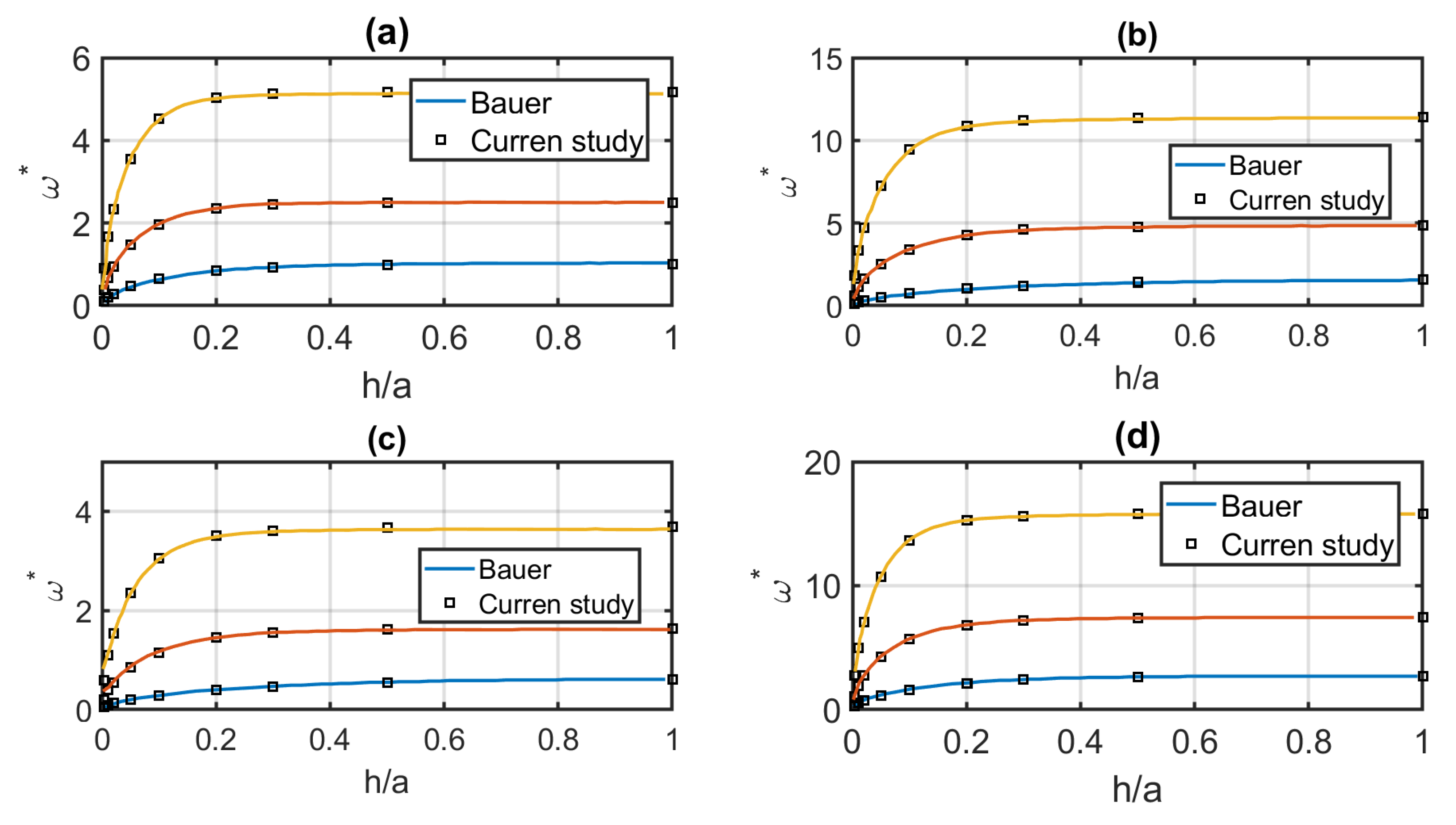
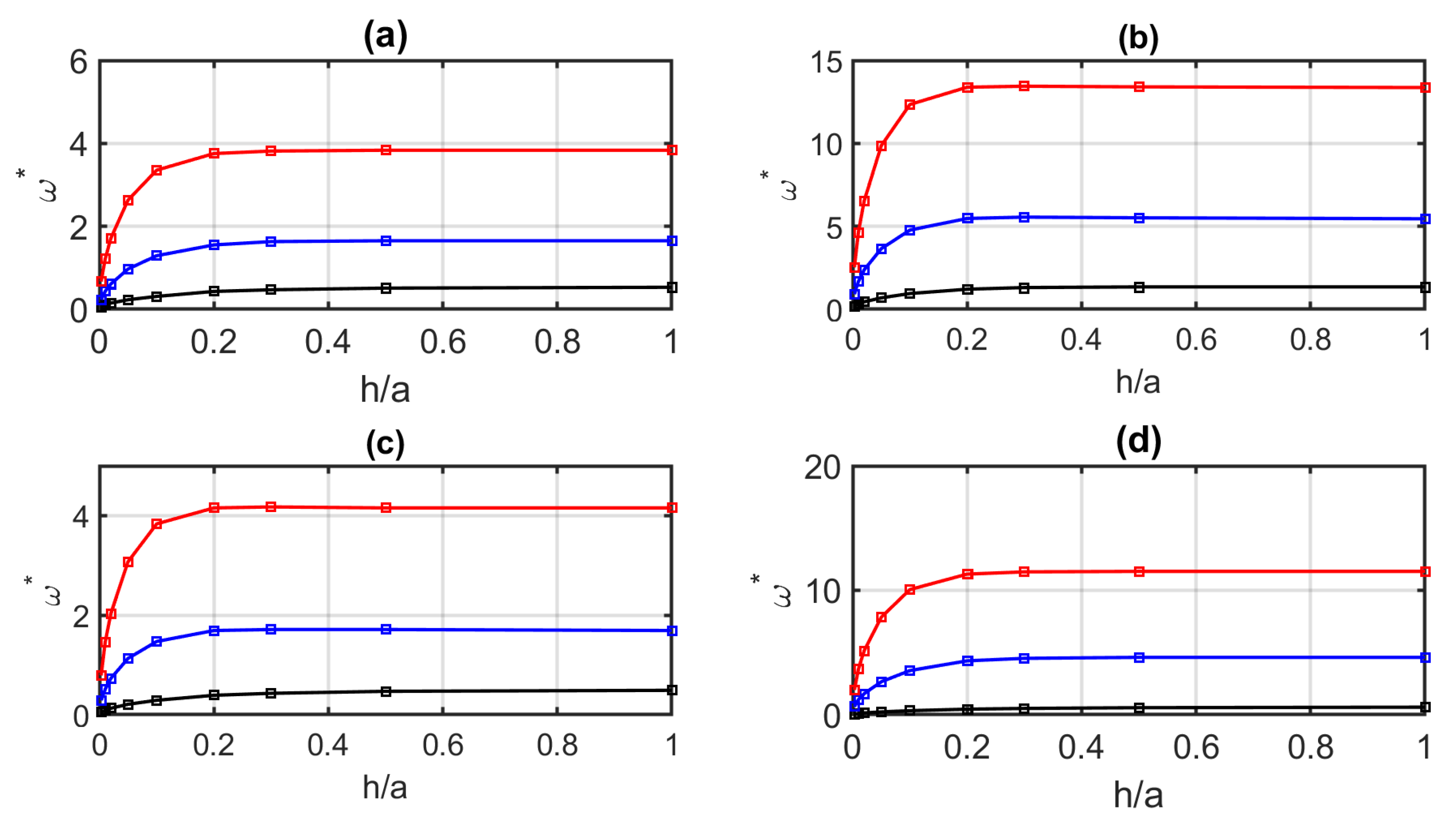
Figure 4. The dominant modes of the structure i.e., the first three mode shapes are illustrated in
Figure 4. The non-dimensional fluid-structure frequencies for clamped plate
,
, and axi-symmetric mode
,
,
, and axi-symmetric mode
,
,
, and axi-symmetric mode
,
,
, and axi-symmetric mode
is plotted in
Figure 4. As shown, the agreement of results of the current study with non-dimensional fluid-structure frequencies for clamped plate obtained by Bauer ([
35]) are matching. As for the common storage tank the aspect ratio (
) is above the unity the parameter study of the natural frequencies as the function of the elastic parameter (
) and mass ratio (
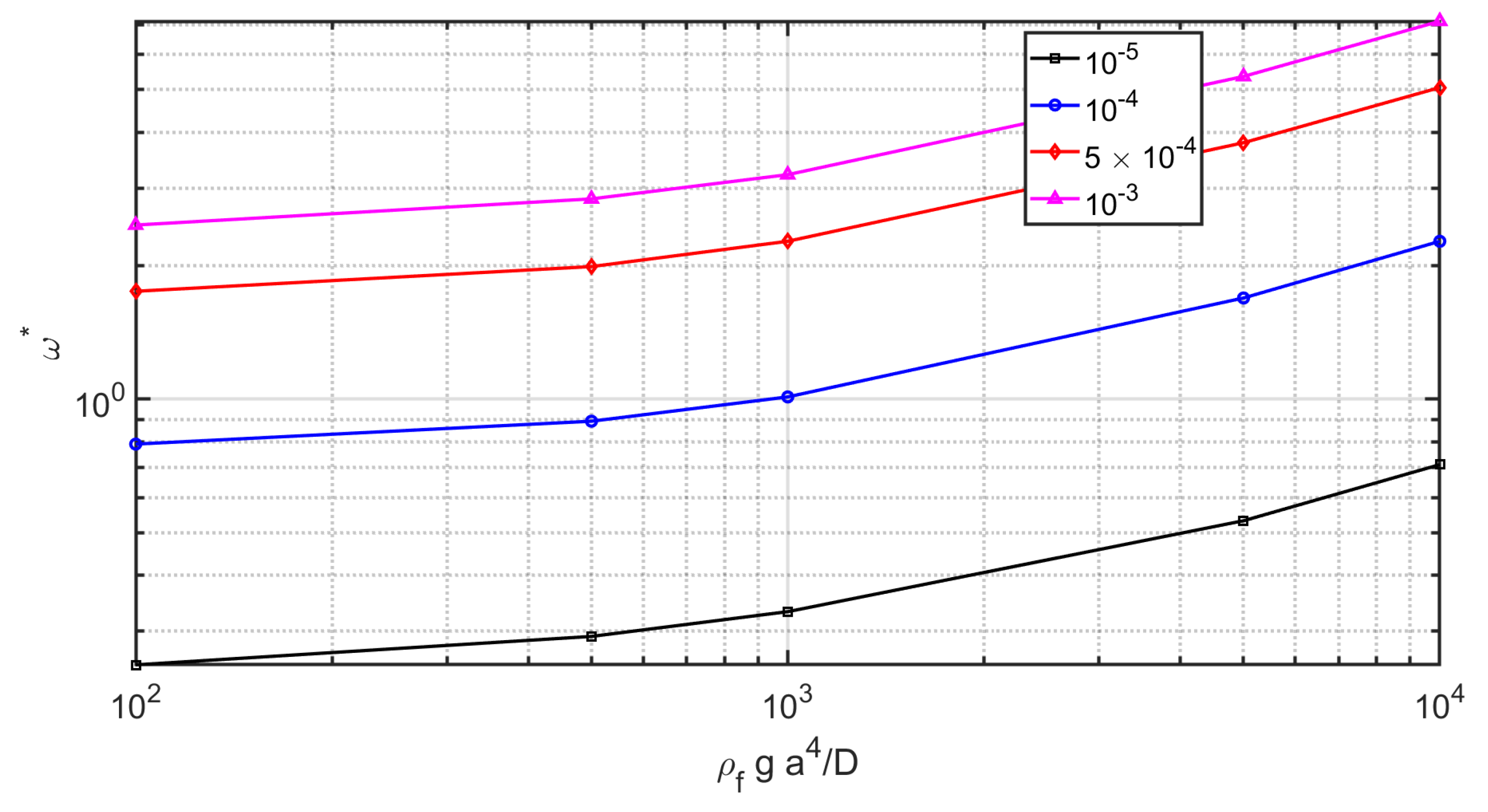
) is useful for engineering design. For the first mode natural frequency at high aspect ratios, the natural frequency grows monotonically with
. As shown by the increase of the elastic parameter (
) and the mass ratio (
) the non-dimensional circular frequency (
) is increased. The sensitivity analysis of the results show that the mass ratio (
) is the most important parameter on the detect of natural frequency. The non-dimensional fluid-structure frequencies for clamped plate versus
and axi-symmetric mode
for various
is plotted in
Figure 5. As shown by the increase of the elastic parameter (
) and mass ratio (
) is useful for engineering design. As shown by the increase of elastic parameter (
) and mass ratio (
) the non-dimensional circular frequency (
) is increased. The non-dimensional fluid-structure frequencies for clamped plate versus
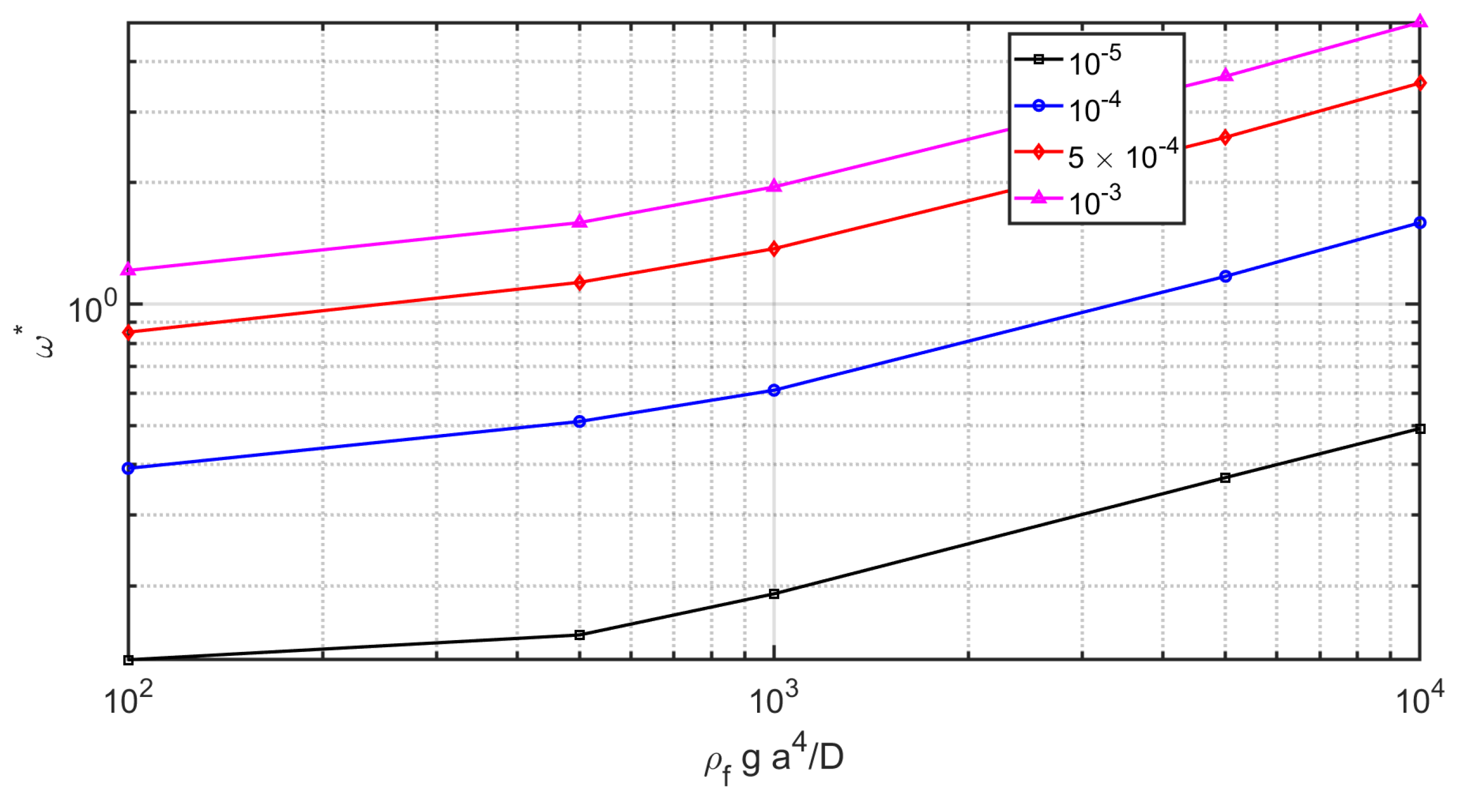
and axi-symmetric mode
for various
is plotted in
Figure 6. As illustrated by an increase of the elastic parameter (
) and mass ratio (
) is useful for engineering design. As shown by the increase of elastic parameter (
) and mass ratio (
) the non-dimensional circular frequency (
) is increased. The non-dimensional fluid-structure frequencies for clamped plate versus
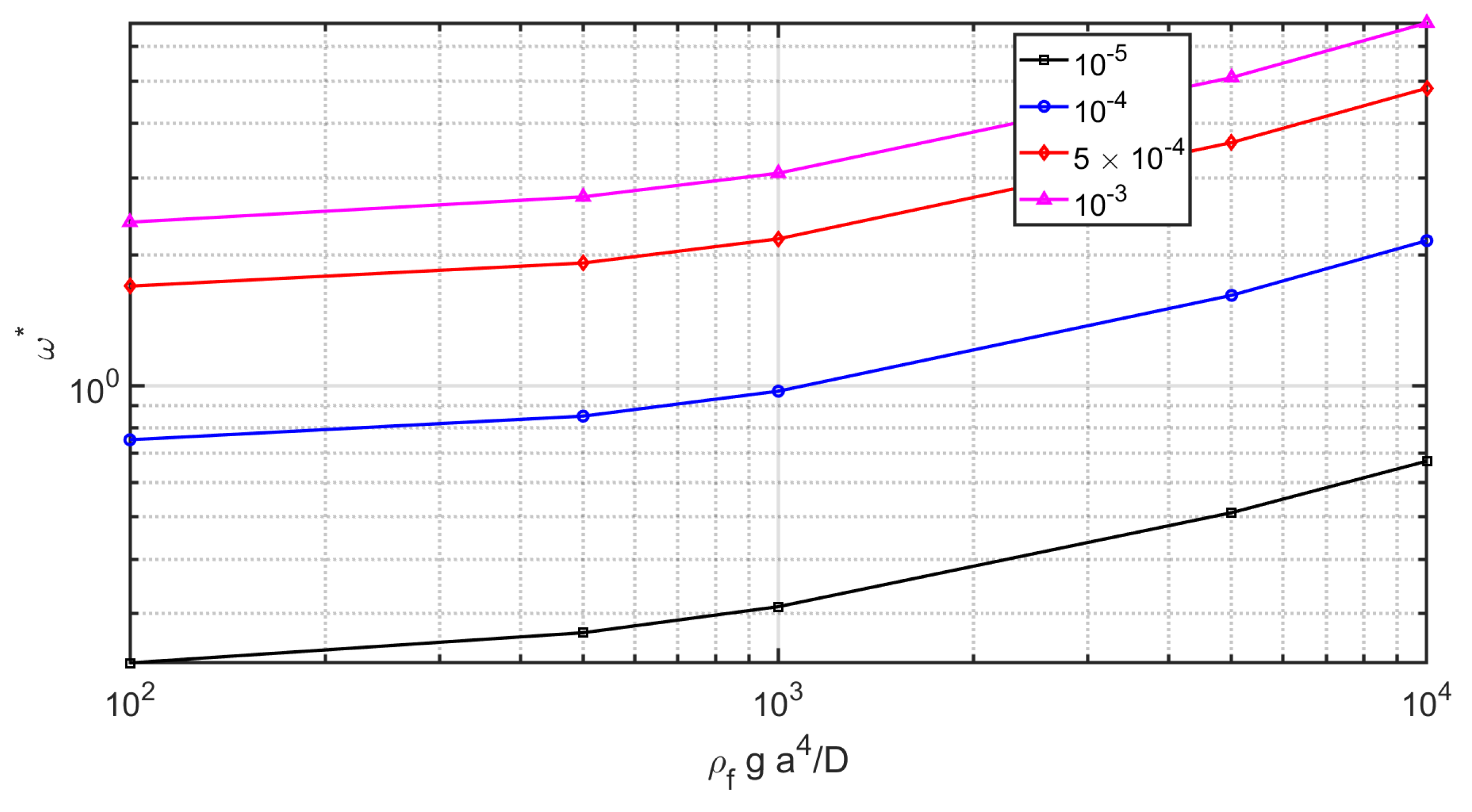
and axi-symmetric mode
for various
is plotted in
Figure 7. As illustrated by an increase of the elastic parameter (
) and mass ratio (
) is useful for engineering design. As shown by the increase of elastic parameter (
) and mass ratio (
) the non-dimensional circular frequency (
) is increased.
5.2. Simply Supported Plate
The modal analysis is required to find the inherent dynamic properties of the any domain in terms of its natural frequencies. The non-dimensional fluid-structure frequencies for simply supported plate
,
, and axi-symmetric mode
,
,
, and axi-symmetric mode
,
,
, and axi-symmetric mode
,
,
, and axi-symmetric mode
is plotted in
Figure 8. The dominant modes of the structure i.e., the first three mode shapes are illustrated in
Figure 8. As illustrated in
Figure 8, the natural frequencies are increased by an increase of aspect ratio. The comparison of the
Figure 8 and
Figure 4 is not simple such as the results of zero modes
Figure 3 for clamped plate and simply supported case. As exposed in some cases the clamped boundary condition caused higher frequencies than the simply supported plate and in some cases it was vice versa.
The non-dimensional fluid-structure frequencies for clamped plate versus
and axi-symmetric mode
for various
is plotted in
Figure 9. As shown the parameter study of the natural frequencies as the function of the elastic parameter (
) and mass ratio (
) presents a linear relation between the parameters in comparison with the nonlinear case was shown for clamped case in
Figure 5. As shown by the increase of elastic parameter (
) and mass ratio (
) the non-dimensional circular frequency (
) is increased. The sensitivity analysis of the results show that even the mass ratio (
) is the most important parameter on the detect of natural frequency, the elastic parameter (
) is more important than the nonlinear case was shown for clamped case in
Figure 5. The non-dimensional fluid-structure frequencies for clamped plate versus
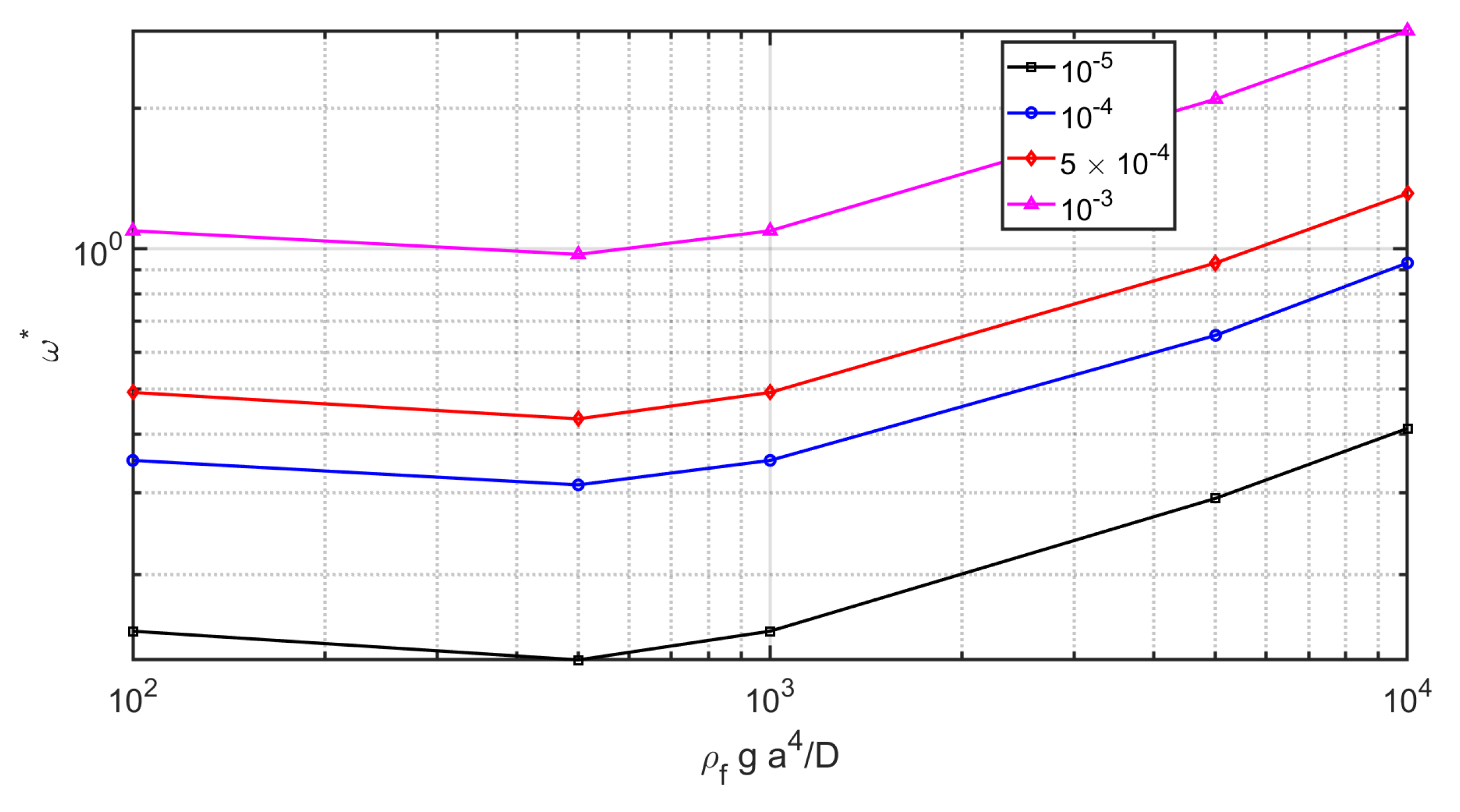
and axi-symmetric mode (
) for various
is plotted in
Figure 10. In this case in contract with linear behavior of the clamped case in
Figure 5 a parabola shape is seen. It means that there are some optimal values of elastic parameter (
) where the natural angular velocity of the axi-symmetric mode (
) is minimized. This frequency is important in horizontal movement of the sloshing tanks. The non-dimensional fluid-structure frequencies for clamped plate versus
and axi-symmetric mode
for various
is plotted in
Figure 11. The fundamental angular velocity of the axi-symmetric mode (
) shows a parabola shape same as the fundamental angular velocity of the axi-symmetric mode (
).