Family of Enneper Minimal Surfaces
Abstract
:1. Introduction
2. The Family of Enneper Minimal Surfaces
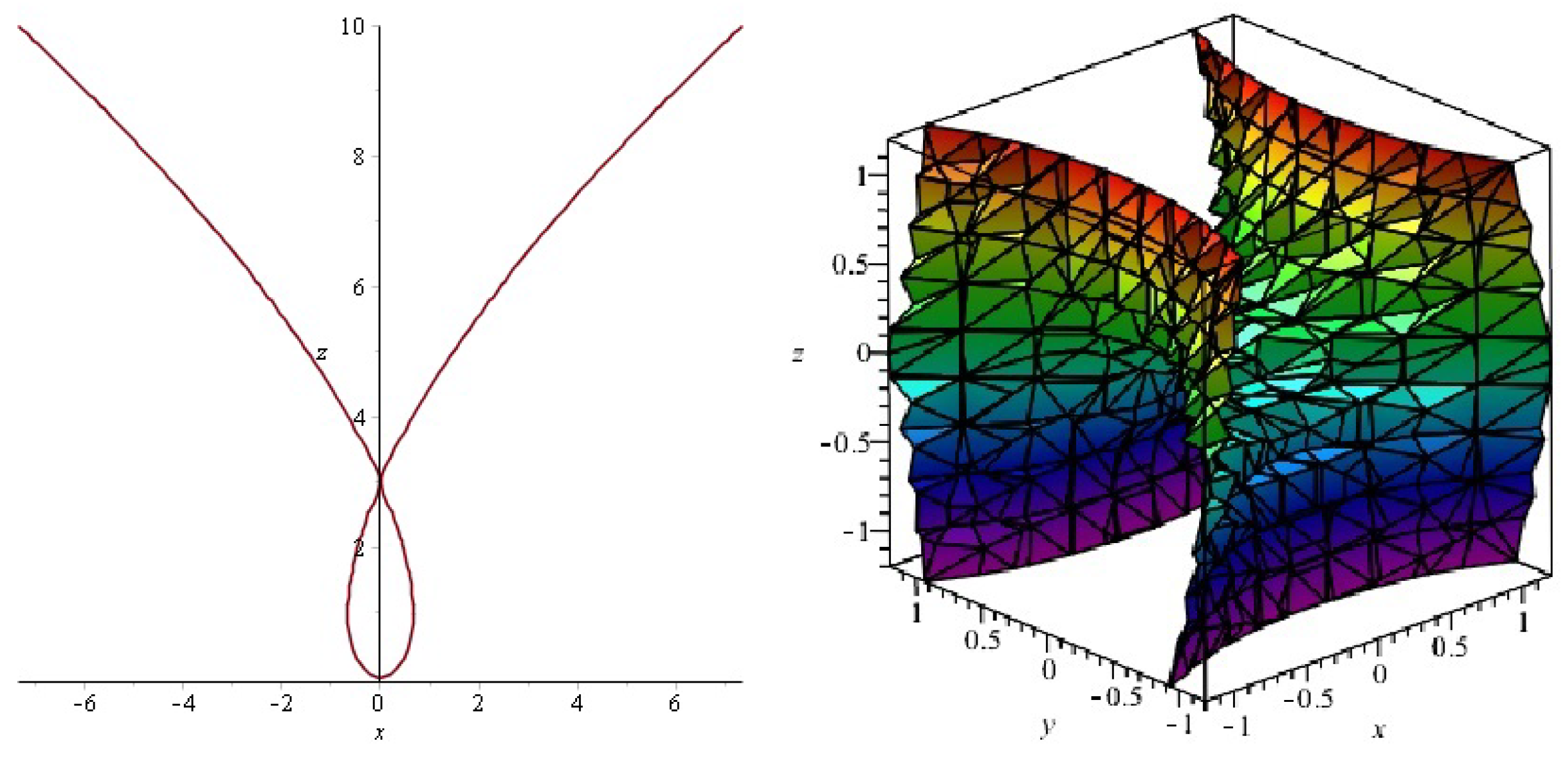
2.1. Algebraic Equation of Enneper Minimal Surface
2.2. Algebraic Equation of Enneper Minimal Surface
2.3. Algebraic Equation of Enneper Minimal Surface
3. Integral Free Form
4. Conclusions
Funding
Conflicts of Interest
References
- Bour, E. Théorie de la déformation des surfaces. J. l’Êcole Imperiale Polytech. 1862, 22, 1–148. [Google Scholar]
- Demoulin, A. Sur les surfaces minima applicables sur des surfaces de revolution ou sur des surfaces spirales. Darboux Bull. 1897, 2, 244–252. [Google Scholar]
- Haag, J. Note sur les surfaces minima applicables sur une surface de revolution. Darboux Bull. 1906, 30, 75–94. [Google Scholar]
- Nitsche, J.C.C. Lectures on Minimal Surfaces. Volume 1. Introduction, Fundamentals, Geometry and Basic Boundary Value Problems; Translated from the German by Feinberg, J.M.; With a German Foreword; Cambridge University Press: Cambridge, UK, 1989. [Google Scholar]
- Osserman, R. A Survey of Minimal Surfaces; Van Nostrand Reinhold Co.: New York, NY, USA; London, UK; Melbourne, Australia, 1969. [Google Scholar]
- Rado, T. On the Problem of Plateau, 1st ed.; Springer: New York, NY, USA, 1933; Subharmonic Functions Reprint; Springer: New York, NY, USA; Heidelberg, Germany, 1971. [Google Scholar]
- Ribaucour, A. Etude des elassoides ou surfaces a courbure moyenne nulle. Mem. Cour. et Mem. Sav. Etr. Acad. Roy. Sci. Belg. Bruxelles. 1882, 44, 236. [Google Scholar]
- Schwarz, H.A. Miscellen aus dem Gebiete der Minimalflachen. J. für die reine und Angew. Math. (Crelle’s J.) 1875, 80, 280–300. [Google Scholar]
- Weierstrass, K. Untersuchungen über die flächen, deren mittlere Krümmung überall gleich null ist. Preuss Akad. Wiss. 1866, III, 219–220. [Google Scholar]
- Whittemore, J.K. Minimal surfaces applicable to surfaces of revolution. Ann. Math. 1917, 19, 1–20. [Google Scholar] [CrossRef]
- Enneper, A. Untersuchungen über einige Punkte aus der allgemeinen Theorie der Flächen. Math. Ann. 1870, 2, 58–623. [Google Scholar] [CrossRef]
- Enneper, A. Analytisch geometrische Untersuchungen. Gott. Nachr. 1868, 258–277, 421–443. [Google Scholar]
- Henneberg, L. Uber die evoluten der ebenen algebraischen kurven. Vierteljahresschr. Naturforsch. Ges. Zur. 1876, 21, 71–72. [Google Scholar]
- Henneberg, L. Determination of the lowest genus of the algebraic minimum surfaces. Br. Ann. 1878, IX/2, 54–57. [Google Scholar]
- Weierstrass, K. Mathematische Werke; Mayer & Muller: Berlin, Germany, 1903; Volume 3. [Google Scholar]
- Barbosa, J.L.M.; Colares, A.G. Minimal Surfaces in ℝ3; Lecture Notes in Mathematics 1195; Springer: Berlin, Germany, 1986. [Google Scholar]
- Barbosa, J.L.M.; Do Carmo, M.P. On regular algebraic surfaces of with constant mean curvature. J. Differ. Geom. 2016, 102, 173–178. [Google Scholar] [CrossRef]
- Cheshkova, M.A. On the geometry of Enneper’s surface. (Russian) Differentsialnaya Geom. Mnogoobraz 2007, 38, 139–142. [Google Scholar]
- Dumitru, D. Minimal surfaces that generalize the Enneper’s surface. Novi Sad J. Math. 2010, 40, 17–22. [Google Scholar]
- Fernandez, I.; Lopez, F.J. On the uniqueness of the helicoid and Enneper’s surface in the Lorentz-Minkowski space . Trans. Am. Math. Soc. 2011, 363, 4603–4650. [Google Scholar] [CrossRef]
- Kaya, S.; López, R. The Björling problem and Weierstrass-Enneper representation of maximal surfaces in Lorentz-Minkowski space. In Differential Geometry in Lorentz-Minkowski Space; University Granada: Granada, Spain, 2017; pp. 43–59. [Google Scholar]
- Kokubu, M. On a construction of higher codimensional minimal surfaces based on Enneper’s surface and the catenoid. (Japanese) Minimal surfaces and related topics (Kyoto, 1999). Sūrikaisekikenkyūsho Kōkyūroku 1999, 1113, 65–84. [Google Scholar]
- Odehnal, B. On algebraic minimal surfaces. KoG 2016, 20, 61–78. [Google Scholar]
- Velickovic, V. Visualization of Enneper’s surface by line graphics. Filomat 2017, 31, 387–405. [Google Scholar] [CrossRef]
- Dierkes, U.; Hildebrandt, S.; Sauvigny, F. Minimal Surfaces, 2nd ed.; Springer: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- Gray, A.; Abbena, E.; Salamon, S. Modern Differential Geometry of Curves and Surfaces with Mathematica®, 3rd ed.; Studies in Advanced Mathematics; Chapman & Hall/CRC: Boca Raton, FL, USA, 2006. [Google Scholar]

| Surface | Algebraic Function | |||
|---|---|---|---|---|
| 9 | 3 | |||
| 25 | 5 | |||
| 49 | 7 | |||
| ⋮ | ⋮ | ⋮ | ⋮ | ⋮ |
© 2018 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Güler, E. Family of Enneper Minimal Surfaces. Mathematics 2018, 6, 281. https://doi.org/10.3390/math6120281
Güler E. Family of Enneper Minimal Surfaces. Mathematics. 2018; 6(12):281. https://doi.org/10.3390/math6120281
Chicago/Turabian StyleGüler, Erhan. 2018. "Family of Enneper Minimal Surfaces" Mathematics 6, no. 12: 281. https://doi.org/10.3390/math6120281
APA StyleGüler, E. (2018). Family of Enneper Minimal Surfaces. Mathematics, 6(12), 281. https://doi.org/10.3390/math6120281





