Thermophysical Properties and Expectation Values for Pöschl–Teller-like Pseudo-Harmonic Oscillator
Abstract
1. Introduction
2. Bound State Solutions of the PTPO
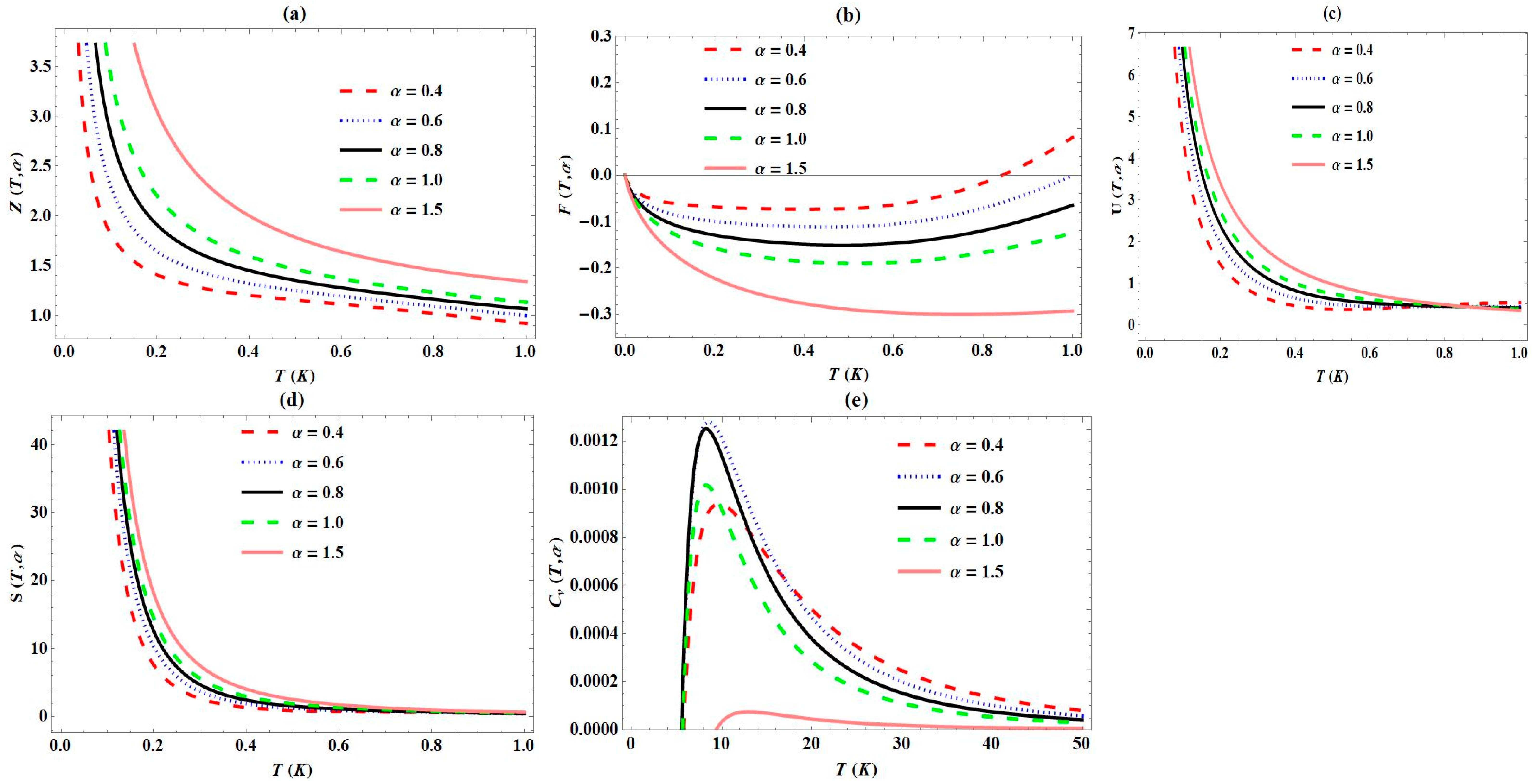
3. Thermodynamic Functions for the PTPO
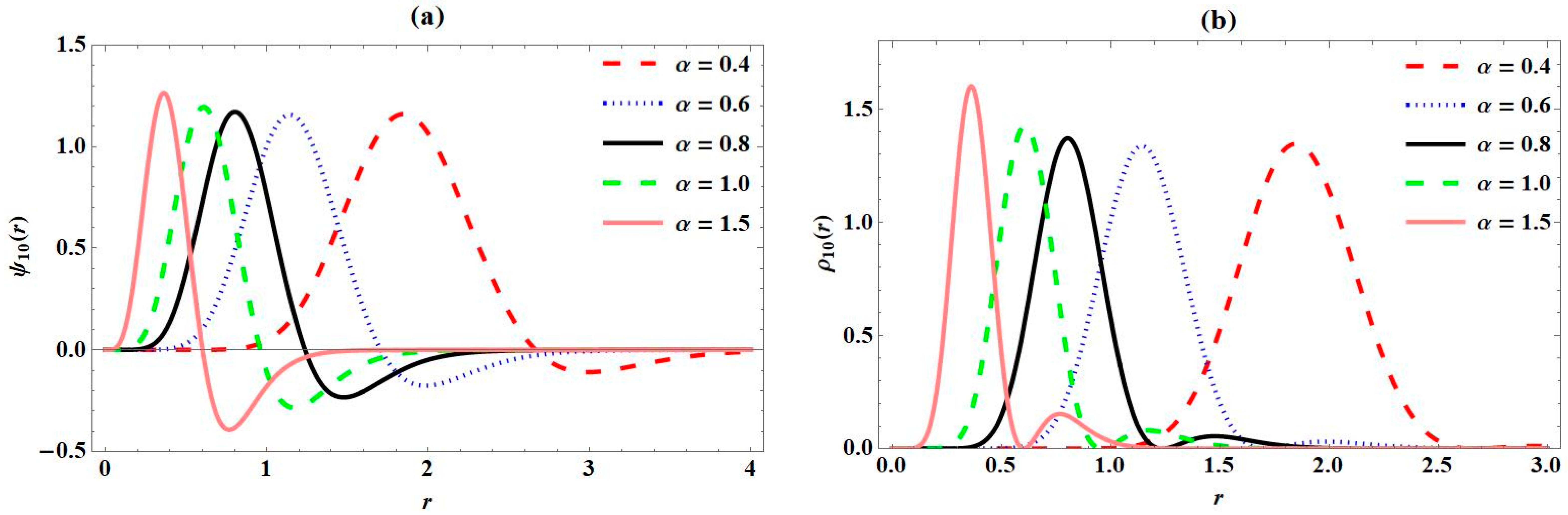
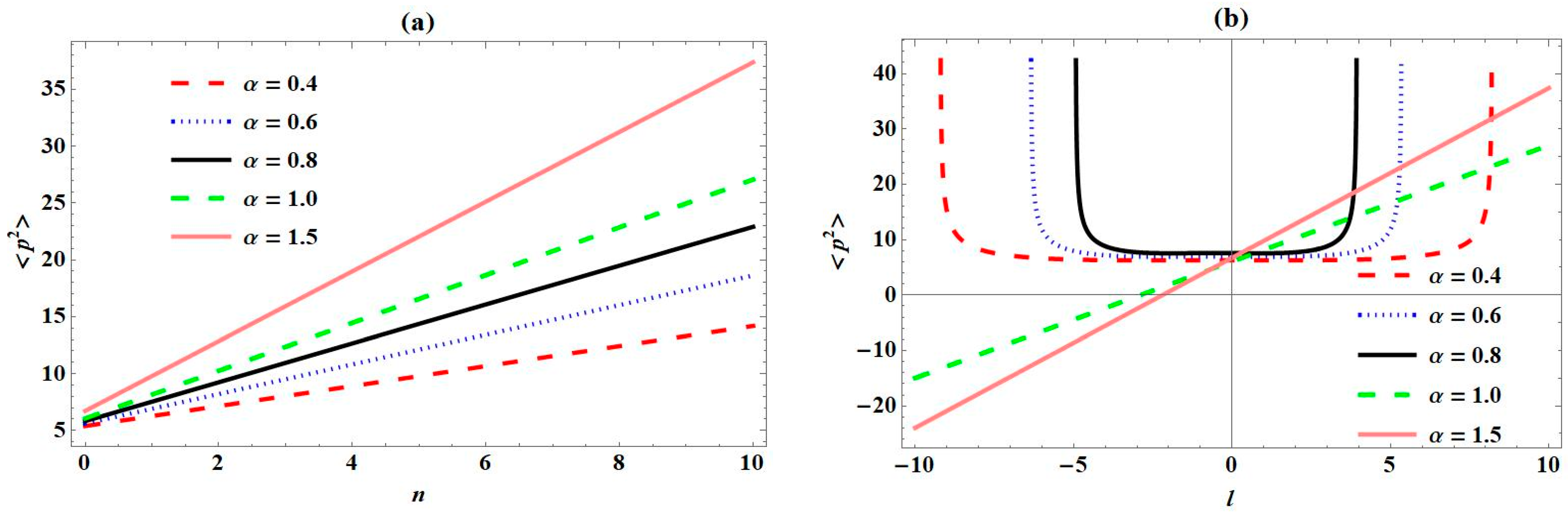
4. Expectation Values of the PTPO
5. Results and Discussion
6. Concluding Remarks
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
Review of Nikiforov–Uvarov Functional Analysis (NUFA) Method
References
- Gertrud, P.; Teller, E. Bemerkungen zur Quantenmechanik des anharmonischen Oszillators. Z. Für Physik 1933, 83, 143–151. [Google Scholar]
- Zhang, M.C.; Wang, Z.B. Bound states of the Klein-Gordon equation and Dirac equation with the Manning-Rosen scalar and vector potentials. Acta Phys Sin. 2006, 55, 0525. [Google Scholar] [CrossRef]
- Liu, X.Y.; Wei, G.F.; Cao, X.W.; Bai, H.G. Spin symmetry for Dirac equation with the trigonometric Pöschl-Teller potential. Int. J. Theor. Phys. 2010, 49, 343–348. [Google Scholar] [CrossRef]
- Hamzavi, M.; Rajabi, A.A. Exact S-wave solution of the trigonometric pöschl-teller potential. Int. J. Quantum Chem. 2012, 112, 1592–1597. [Google Scholar] [CrossRef]
- Saregar, A.; Suparmi, A.; Cari, C.; Yuliani, H. Analysis of Energy Spectra and Wave Function of Trigonometric Poschl-Teller plus Rosen-Morse Non-Central Potential Using Supersymmetric Quantum Mechanics Approach. Res. Inven. Int. J. Eng. Sci. 2013, 2, 14–26. [Google Scholar]
- Vélez, M.; Sicard, A.; Ospina, J. Pöschl-Teller potentials based solution to Hilbert’s tenth problem. Ingeníeria Y Cienc. 2006, 2, 43–57. [Google Scholar]
- Chen, C.Y.; You, Y.; Lu, F.L.; Dong, S.H. The position–momentum uncertainty relations for a Pöschl–Teller type potential and its squeezed phenomena. Phys. Lett. A 2013, 377, 1070–1075. [Google Scholar] [CrossRef]
- Yahya, W.Y.; Oyewumi, K.J.; Assoc, J. Arab Univ. Basic Appl. Sci. 2016, 21, 53. [Google Scholar]
- Büyükiliç, F.; Egˇrifes, H.; Demirhan, D. Solution of the Schrödinger equation for two different molecular potentials by the Nikiforov-Uvarov method. Theor. Chem. Acc. 1998, 98, 192–196. [Google Scholar] [CrossRef]
- Arai, A. Exactly solvable supersymmetric quantum mechanics. J. Math. Anal. Appl. 1991, 158, 63–79. [Google Scholar] [CrossRef]
- Yang, Q.B. A Nearly Discrete Method for Acoustic and Elastic Wave Equations in Anisotropic Media. Acta Photonica Sin. 2003, 32, 882. [Google Scholar] [CrossRef]
- Zhao, X.Q.; Jia, C.S.; Yang, Q.B. Bound states of relativistic particles in the generalized symmetrical double-well potential. Phys. Lett. A 2005, 337, 189–196. [Google Scholar] [CrossRef]
- Wei, G.F.; Dong, S.H. Spin symmetry in the relativistic symmetrical well potential including a proper approximation to the spin–orbit coupling term. Phys. Scr. 2010, 81, 035009. [Google Scholar] [CrossRef]
- Wei, G.F.; Dong, S.H. Algebraic approach to pseudospin symmetry for the Dirac equation with scalar and vector modified Pöschl-Teller potentials. Europhys. Lett. 2009, 87, 40004. [Google Scholar] [CrossRef]
- Candemir, N. Klein–Gordon particles in symmetrical well potential. Appl. Math. Comput. 2016, 274, 531–538. [Google Scholar] [CrossRef]
- Durmus, A. Approximate treatment of the Dirac equation with hyperbolic potential function. Few-Body Syst. 2018, 59, 7. [Google Scholar] [CrossRef]
- Okorie, U.S.; Ikot, A.N.; Edet, C.O.; Rampho, G.J.; Sever, R.; Akpan, I.O. Solutions of the Klein Gordon equation with generalized hyperbolic potential in D-dimensions. J. Phys. Commun. 2019, 3, 095015. [Google Scholar] [CrossRef]
- Hammou, A.A.B.; Chabab, M.; El Batoul, A.; Hamzavi, M.; Lahbas, A.; Moumene, I.; Oulne, M. Bohr Hamiltonian with trigonometric Pöschl-Teller potential in-unstable and-stable pictures. Eur. Phys. J. Plus 2019, 134, 577. [Google Scholar] [CrossRef]
- Eyube, E.S.; Bitrus, B.M.; Jabil, Y.Y. Thermodynamic relations and ro-vibrational energy levels of the improved Pöschl–Teller oscillator for diatomic molecules. J. Phys. B At. Mol. Opt. Phys. 2021, 54, 155102. [Google Scholar] [CrossRef]
- Omugbe, E.; Osafile, O.E.; Okon, I.B. Approximate eigensolutions, thermodynamic properties and expectation values of a mixed hyperbolic Pöschl–Teller potential (MHPTP). Eur. Phys. J. Plus 2021, 136, 1–18. [Google Scholar] [CrossRef]
- Ahmed, F.; Bouzenada, A.; Moreira, A.R.P. Global monopole effects on exact s-state solution under trigonometric Pöschl–Teller potential. Mol. Phys. 2024, 123, e2365420. [Google Scholar] [CrossRef]
- Hachama, M.; Diaf, A. Ro-vibrational relativistic states for the q-deformed hyperbolic barrier potential. Eur. Phys. J. Plus 2024, 139, 501. [Google Scholar] [CrossRef]
- Ikot, A.N.; Okorie, U.S.; Amadi, P.O.; Edet, C.O.; Rampho, G.J.; Sever, R. The Nikiforov–Uvarov-Functional Analysis (NUFA) Method: A new approach for solving exponential-type potentials. Few-Body Syst. 2021, 62, 9. [Google Scholar] [CrossRef]
- Boumali, A. The statistical properties of q-deformed Morse potential for some diatomic molecules via Euler–MacLaurin method in one dimension. J. Math. Chem. 2018, 56, 1656–1666. [Google Scholar] [CrossRef]
- Arfken, G. Mathematical Methods for Physicists, 3rd ed.; Academic Press: San Diego, CA, USA, 1985. [Google Scholar]
- Chabi, K.; Boumali, A. Thermal properties of three-dimensional Morse potential for some diatomic molecules via Euler-Maclaurin approximation. Rev. Mex. De Física 2020, 66, 110–120. [Google Scholar] [CrossRef]
- Feynman, R.P. Forces in molecules. Phys. Rev. 1939, 56, 340. [Google Scholar] [CrossRef]
- Bader, R.F.; Jones, G.A. The Hellmann–Feynman theorem and chemical binding. Can. J. Chem. 1961, 39, 1253–1265. [Google Scholar] [CrossRef]
- Okon, I.B.; Popoola, O.; Isonguyo, C.N. Approximate Solutions of Schrodinger Equation with Some Diatomic Molecular Interactions Using Nikiforov-Uvarov Method. Adv. High Energy Phys. 2017, 2017, 9671816. [Google Scholar] [CrossRef]
- Politzer, P.; Murray, J.S. Electronegativity—A perspective. J. Mol. Mod. 2018, 24, 214. [Google Scholar] [CrossRef]
- Okon, I.B.; Onate, C.A.; Omugbe, E.; Okorie, U.S.; Antia, A.D.; Onyeaju, M.C.; Li, C.W.; Araujo, J. Approximate Solutions, Thermal Properties, and Superstatistics Solutions to Schrödinger Equation. Adv. High Energy Phys. 2022, 2022, 5178247. [Google Scholar] [CrossRef]
- Griffiths, D.J.; Schroeter, D.F. Introduction to Quantum Mechanics; Cambridge University Press: Navi Mumbai, India, 2018. [Google Scholar]
- You, Y.; Lu, F.L.; Sun, D.S.; Chen, C.Y.; Dong, S.H. Solutions of the second Pöschl–Teller potential solved by an improved scheme to the centrifugal term. Few-Body Syst. 2013, 54, 2125–2132. [Google Scholar] [CrossRef]
- Ikhdair, S.M.; Falaye, B.J. Approximate analytical solutions to relativistic and nonrelativistic Pöschl–Teller potential with its thermodynamic properties. Chem. Phys. 2013, 421, 84–95. [Google Scholar] [CrossRef]
- Gradstein, I.S.; Ryzhik, I.M. Tables of Integrals, Series, and Products; Jeffrey, A., Zwillinger, D., Eds.; Academic Press: Burlington, MA, USA, 2007. [Google Scholar]
- Khordad, R.; Ghanbari, A. Analytical calculations of thermodynamic functions of lithium dimer using modified Tietz and Badawi-Bessis-Bessis potentials. Comput. Theor. Chem. 2019, 1155, 1–8. [Google Scholar] [CrossRef]
- Jia, C.-S.; You, X.-T.; Liu, J.-Y.; Zhang, L.-H.; Peng, X.-L.; Wang, Y.-T.; Wei, L.-S. Prediction of enthalpy for the gases Cl2, Br2, and gaseous BBr. Chem. Phys. Lett. 2019, 717, 16–20. [Google Scholar] [CrossRef]
- Edet, C.O.; Ikot, A.N. Analysis of the impact of external fields on the energy spectra and thermo-magnetic properties of N2,I2, CO, NO and HCl diatomic molecules. Mol. Phys. 2021, 119, e1957170. [Google Scholar] [CrossRef]
- Oyewumi, K.J.; Sen, K.D. Exact solutions of the Schrödinger equation for the pseudoharmonic potential: An application to some diatomic molecules. J. Math. Chem. 2012, 50, 1039–1059. [Google Scholar] [CrossRef]
- Okon, I.B.; Onate, C.A.; Horchani, R.; Popoola, O.O.; Omugbe, E.; William, E.S.; Okorie, U.S.; Inyang, E.P.; Isonguyo, C.N.; Udoh, M.E.; et al. Thermomagnetic properties and its effects on Fisher entropy with Schioberg plus Manning-Rosen potential (SPMRP) using Nikiforov-Uvarov functional analysis (NUFA) and supersymmetric quantum mechanics (SUSYQM) methods. Sci. Rep. 2023, 13, 8193. [Google Scholar] [CrossRef]
- Osobonye, G.T.; Adekanmbi, M.; Ikot, A.N.; Okorie, U.S.; Rampho, G.J. Thermal properties of anharmonic Eckart potential model using Euler–MacLaurin formula. Pramana 2021, 95, 98. [Google Scholar] [CrossRef]
- Gabriel, T.O.; Okorie, U.S.; Amadi, P.O.; Ikot, A.N. Statistical analysis and information theory of screened Kratzer–Hellmann potential model. Can. J. Phys. 2021, 99, 583–591. [Google Scholar]
- Udoh, M.E.; Amadi, P.O.; Okorie, U.S.; Antia, A.D.; Obagboye, L.F.; Horchani, R.; Sulaiman, N.; Ikot, A.N. Thermal properties and quantum information theory with the shifted Morse potential. Pramana 2022, 96, 222. [Google Scholar] [CrossRef]
- Bang, J.Y.; Berger, M.S. Possible Equilibra of Interacting Dark Energy Models. Phys. Rev. D 2016, 74, 125012. [Google Scholar] [CrossRef]
- Tawfik, A.; Diab, A. Generalized uncertainty principle: Approaches and applications. Int. J. Mod. Phys. D 2014, 23, 1430025. [Google Scholar] [CrossRef]
- Lütfüoğlu, B.C.; Kříž, J. A comparative interpretation of the thermodynamic functions of a relativistic bound state problem proposed with an attractive or a repulsive surface effect. Eur. Phys. J. Plus 2019, 134, 60. [Google Scholar] [CrossRef]
- Kurnia, P.L.; Cari, C.; Suparmi, A.; Harjana, H. Topological effects on relativistic energy spectra of diatomic molecules under the magnetic field with Kratzer potential and thermodynamic-optical properties. Int. J. Theor. Phys. 2023, 62, 246. [Google Scholar]
- Arnold, F.N.; Uvarov, V.B. Special Functions of Mathematical Physics; Birkhäuser: Basel, Switzerland, 1988; Volume 205. [Google Scholar]
- Tezcan, C.; Sever, R. A general approach for the exact solution of the Schrödinger equation. Int. J. Theor. Phys. 2009, 48, 337–350. [Google Scholar] [CrossRef]
- Falaye, B.J.; Oyewumi, K.J.; Ikhdair, S.M.; Hamzavi, M. Eigensolution techniques, their applications and Fisher’s information entropy of the Tietz–Wei diatomic molecular model. Phys. Scr. 2014, 89, 115204. [Google Scholar] [CrossRef]
- Berkdemir, C. Application of the Nikiforov-Uvarov Method in Quantum Mechanics. In Theoretical Concepts of Quantum Mechanics; IntechOpen: Rijeka, Croatia, 2012; p. 225. [Google Scholar]







| 0 | 0 | 3.0382889813530650 | 2.7612745086722557 | 2.4601481315084320 | 2.1336412887922727 | 1.1985044876545956 |
| 1 | 2.9924166626459120 | 2.6566390026811020 | 2.2737958248621326 | 1.8452354421828350 | 0.5944726399460798 | |
| 2 | 2.9068167714563744 | 2.4780925049321136 | 1.9964924264969070 | 1.5000000000000000 | 1.1497607801534002 | |
| 3 | 2.7928975961425966 | 2.2812784126785110 | 1.8165394840887785 | 1.8354992977529632 | ||
| 1 | 0 | 1.9847657274104495 | 1.0542242954323715 | 0.0115311176543198 | −1.1471351176121427 | −4.5747994832098655 |
| 1 | 1.9313831933120111 | 0.9251171955286521 | −0.2301321373601714 | −1.5373899437517178 | −5.4625526154883115 | |
| 2 | 1.8319204788037373 | 0.7056813154308346 | −0.5872411475095305 | −2.0000000000000000 | −4.647077882440767 | |
| 3 | 1.6998463786642457 | 0.4649778465014194 | −0.8175109605572253 | −1.550501638576419 | ||
| 2 | 0 | 0.7712424734678338 | −1.0128259178075147 | −3.0770858961997956 | −5.4279115240165580 | −12.598103454074327 |
| 1 | 0.7103497239781102 | −1.1664046116237996 | −3.3740600995824774 | −5.9200153296862705 | −13.769577870922696 | |
| 2 | 0.5970241861511010 | −1.4267298740704444 | −3.8109747215159686 | −6.5000000000000000 | −12.693916545034938 | |
| 3 | 0.4467951611858947 | −1.7113227196756720 | −4.0915614052032260 | −5.936502574905804 | ||
| 3 | 0 | −0.6022807804747821 | −3.4398761310474004 | −6.805702910053910 | −10.708687930420972 | −22.871407424938788 |
| 1 | −0.6706837453557899 | −3.6179264187762500 | −7.157988061804781 | −11.302640715620822 | −24.326603126357085 | |
| 2 | −0.7978721065015355 | −3.9191410635717236 | −7.674708295522407 | −12.000000000000000 | −22.990755207629107 | |
| 3 | −0.9662560562924556 | −4.2476232858527645 | −8.005611849849230 | −11.322503511235187 |
| 0 | 0 | −2.4217296080178654 | −2.5169257808803454 | −2.6091008871653200 | −2.6977493752543310 | −2.9015617560869633 |
| 1 | −2.4732809489109004 | −2.6357624382315863 | −2.8257252577768930 | −3.0456121603719937 | −3.757733527950010 | |
| 2 | −2.5791484918679210 | −2.8914721621098330 | −3.3347945020408565 | −4.00000000000000000 | −24.91647286716896 | |
| 3 | −2.7470208050165708 | −3.3505714915840574 | −4.5735437660042250 | −11.544003745317530 | ||
| 1 | 0 | −2.8197444841018613 | −3.1102875926013236 | −3.3935654277180560 | −3.667891875399663 | −4.306055522440531 |
| 1 | −2.8766565361825890 | −3.2473390679567370 | −3.6538039289877178 | −4.102153247962742 | −5.472019242235725 | |
| 2 | −2.9939291697600910 | −3.5451538149532453 | −4.2776035436229200 | −5.333333333333333 | −36.91647286716898 | |
| 3 | −3.1808817206538830 | −4.0877256317847990 | −5.8384548300715770 | −15.54400374531753 | ||
| 2 | 0 | −3.2177593601858567 | −3.7036494043223027 | −4.1780299682707920 | −4.6380343755449940 | −5.710549288794097 |
| 1 | −3.2800321234542780 | −3.8589156976818875 | −4.4818826001985430 | −5.1586943355534910 | −7.186304956521438 | |
| 2 | −3.4087098476522613 | −4.1988354677966570 | −5.2204125852049840 | −6.6666666666666660 | −48.9164728671690 | |
| 3 | −3.6147426362911950 | −4.8248797719855405 | −7.1033658941389280 | −19.544003745317532 | ||
| 3 | 0 | −3.6157742362698526 | −4.2970112160432810 | −4.9624945088235290 | −5.6081768756903270 | −7.115043055147664 |
| 1 | −3.6834077107259670 | −4.4704923274070385 | −5.3099612714093680 | −6.2152354231442395 | −8.900590670807153 | |
| 2 | −3.8234905255444310 | −4.8525171206400690 | −6.1632216267870470 | −8.00000000000000000 | −60.91647286716902 | |
| 3 | −4.0486035519285070 | −5.5620339121862820 | −8.3682769582062800 | −23.544003745317532 |
| 0 | 0 | −1.9806049710096139 | −2.0626399113861380 | −2.1441105892234704 | −2.2246211251235324 | −2.4193494316019120 |
| 1 | −1.9450253712445547 | −1.9818148277613330 | −2.0016670942147754 | −2.0072026807275030 | −1.9771398364036774 | |
| 2 | −1.8801772647564379 | −1.8480213812400481 | −1.7896385274134197 | −1.7142857142857142 | −1.3907019005301633 | |
| 3 | −1.795332419453038 | −1.6905050061018225 | −1.5550515326872110 | −1.3511234415883917 | ||
| 1 | 0 | −2.3061203545180775 | −2.5489044504700025 | −2.7887689604437744 | −3.0246211251235327 | −3.5904295191401516 |
| 1 | −2.2622460055318125 | −2.4416558269049413 | −2.5882555543001886 | −2.7035133045502950 | −2.8791150700070722 | |
| 2 | −2.182548843163820 | −2.2658077555337230 | −2.2956029530403605 | −2.2857142857142856 | −2.060476587153300 | |
| 3 | −2.078884901456372 | −2.0624304425260687 | −1.9851341971435874 | −1.819288030372914 | ||
| 2 | 0 | −2.6316357380265414 | −3.0351689895538680 | −3.4334273316640790 | −3.8246211251235325 | −4.7615096066783910 |
| 1 | −2.579466639819070 | −2.9014968260485500 | −3.1748440143856020 | −3.3998239283730860 | −3.781090303610467 | |
| 2 | −2.4849204215712013 | −2.6835941298273975 | −2.8015673786673010 | −2.8571428571428568 | −2.730251273776437 | |
| 3 | −2.3624373834597066 | −2.434355878950315 | −2.4152168615999634 | −2.2874526191574365 | ||
| 3 | 0 | −2.9571511215350053 | −3.5214335286377323 | −4.0780857028843830 | −4.6246211251235320 | −5.9325896942166320 |
| 1 | −2.8966872741063283 | −3.3613378251921584 | −3.7614324744710150 | −4.0961345521958780 | −4.6830655372138610 | |
| 2 | −2.7872919999785832 | −3.1013805041210727 | −3.3075318042942420 | −3.4285714285714284 | −3.4000259603995744 | |
| 3 | −2.6459898654630410 | −2.8062813153745610 | −2.8452995260563396 | −2.7556172079419587 |
| 0 | 0 | 5.392637064532287 | 5.610885647959551 | 5.825266771000526 | 6.0346810629396300 | 6.5305859034898310 |
| 1 | 5.390819005777733 | 5.608484679873585 | 5.828225899099056 | 6.0564161814632490 | 6.7234432825555240 | |
| 2 | 5.399414389002578 | 5.663504233969904 | 6.019252293160986 | 6.5714285714285710 | 27.002525717964204 | |
| 3 | 5.440019434196128 | 5.886329000736791 | 6.906121065035042 | 13.570688907700118 | ||
| 1 | 0 | 6.2789250158789780 | 6.933644268306327 | 7.576718868383717 | 8.2048235630849630 | 9.6916998011507580 |
| 1 | 6.2700255444803075 | 6.909822808314149 | 7.536187260438002 | 8.1574232047881840 | 9.7906918472463320 | |
| 2 | 6.2677524345058200 | 6.9438654482538285 | 7.721007973183461 | 8.7619047619047610 | 40.007187747898925 | |
| 3 | 6.2992090728384430 | 7.181371295573902 | 8.816156125786959 | 18.272935790876904 | ||
| 2 | 0 | 7.165212967225670 | 8.256402888653103 | 9.3281709657669100 | 10.374966063230294 | 12.852813698811683 |
| 1 | 7.149232083182883 | 8.211160936754710 | 9.2441486217769470 | 10.258430228113120 | 12.857940411937136 | |
| 2 | 7.136090480009064 | 8.224226662537754 | 9.4227636532059350 | 10.952380952380950 | 53.011849777833650 | |
| 3 | 7.158398711480756 | 8.476413590411012 | 10.726191186538873 | 22.975182674053688 | ||
| 3 | 0 | 8.051500918572362 | 9.579161508999878 | 11.079623063150104 | 12.545108563375626 | 16.013927596472612 |
| 1 | 8.028438621885460 | 9.512499065195275 | 10.952109983115891 | 12.359437251438056 | 15.925188976627942 | |
| 2 | 8.004428525512306 | 9.504587876821677 | 11.124519333228410 | 13.142857142857142 | 66.016511807768380 | |
| 3 | 8.017588350123070 | 9.771455885248123 | 12.636226247290791 | 27.677429557230470 |
| 0 | 0 | −0.0938689414669593 | −0.22025963719668412 | −0.40781129392054327 | −0.6626856666860445 | −1.6336824520195394 |
| 1 | −0.0896478710619404 | −0.19858092270314473 | −0.33912277598585960 | −0.4959993136350861 | −0.8151322429728834 | |
| 2 | −0.0816368880640371 | −0.15915551889661860 | −0.21697291522126957 | −0.1904761904761904 | 7.77913768709192800 | |
| 3 | −0.0703727054224678 | −0.10325661160328456 | −0.00977182208612346 | 1.24843890342539270 | ||
| 1 | 0 | −0.1092964421187299 | −0.27218554552852110 | −0.5304257550520821 | −0.9009952499951558 | −2.4244623881174716 |
| 1 | −0.1042688394776759 | −0.24465770476548520 | −0.4385026916173705 | −0.6680644442813570 | −1.1869972379195597 | |
| 2 | −0.0947657962595031 | −0.19513616709887538 | −0.27831523365313710 | −0.2539682539682538 | 11.5256411646409040 | |
| 3 | −0.0814872795657395 | −0.12597394174760437 | −0.01247442787831298 | 1.68102327569979800 | ||
| 2 | 0 | −0.1247239427705005 | −0.32411145386035800 | −0.6530402161836210 | −1.1393048333042672 | −3.2152423242154042 |
| 1 | −0.1188898078934116 | −0.29073448682782566 | −0.5378826072488814 | −0.8401295749276280 | −1.5588622328662356 | |
| 2 | −0.1078947044549691 | −0.23111681530113210 | −0.3396575520850047 | −0.3174603174603172 | 15.2721446421898830 | |
| 3 | −0.0926018537090113 | −0.14869127189192421 | −0.0151770336705025 | 2.11360764797420400 | ||
| 3 | 0 | −0.1401514434222710 | −0.37603736219219497 | −0.7756546773151598 | −1.3776144166133786 | −4.006022260313336 |
| 1 | −0.1335107763091471 | −0.33681126889016616 | −0.6372625228803923 | −1.0121947055738990 | −1.9307272278129117 | |
| 2 | −0.1210236126504351 | −0.26709746350338880 | −0.4009998705168723 | −0.3809523809523807 | 19.0186481197388630 | |
| 3 | −0.1037164278522830 | −0.17140860203624403 | −0.0178796394626920 | 2.54619202024860950 |
| 0 | 0 | −13.05950884447239 | −14.122182740920914 | −15.198708700192133 | −16.280057067404530 | −18.948898302406720 |
| 1 | −13.333009946016865 | −14.782633254608099 | −16.46896513111365 | −18.445494770538190 | −25.264908246129167 | |
| 2 | −13.925891478365946 | −16.375864832515152 | −20.072969453630076 | −26.285714285714280 | −672.80769939668710 | |
| 3 | −14.943846565431238 | −19.722566139953166 | −31.585446944261477 | −156.66008357702924 | ||
| 1 | 0 | −17.704964179613940 | −21.56562773922445 | −25.71209120728605 | −30.094405686127047 | −41.732997450581010 |
| 1 | −18.036709964561076 | −22.438537558097067 | −27.53575062177556 | −33.463000094528496 | −53.57485418293236 | |
| 2 | −18.765206842501797 | −24.617071084399086 | −33.02741106643039 | −46.730158730158720 | −1476.9242609870455 | |
| 3 | −20.037038994368896 | −29.355475516281047 | −51.472729315265994 | −284.0345823713373 | ||
| 2 | 0 | −23.055931093015474 | −30.578821640405003 | −38.97337784412765 | −48.119449246374835 | −73.396626126752080 |
| 1 | −23.449710890869806 | −31.68617783503507 | −41.43118886159145 | −52.920105909437865 | −92.401080912961130 | |
| 2 | −24.324861892944440 | −34.532174605862444 | −49.190713962608356 | −73.015873015873000 | −2593.1527112958383 | |
| 3 | −25.875769029961447 | −40.897676471357414 | −76.1920606484738 | −449.02705623105976 | ||
| 3 | 0 | −29.112409584676993 | −41.16176444446256 | −54.9825686107169 | −70.355187748147880 | −113.93978433091996 |
| 1 | −29.572012724943054 | −42.5255540854221 | −58.1552798505613 | −76.816812215266280 | −141.74358843621556 | |
| 2 | −30.604856629693880 | −46.12117539690524 | −68.56287814216397 | −105.14285714285712 | −4021.4930503230650 | |
| 3 | −32.460036672208886 | −54.34916900518228 | −105.74344094388493 | −651.63750515619640 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alrebdi, H.I.; Okorie, U.S.; Horchani, R.; Rampho, G.J.; Ikot, A.N. Thermophysical Properties and Expectation Values for Pöschl–Teller-like Pseudo-Harmonic Oscillator. Mathematics 2025, 13, 1524. https://doi.org/10.3390/math13091524
Alrebdi HI, Okorie US, Horchani R, Rampho GJ, Ikot AN. Thermophysical Properties and Expectation Values for Pöschl–Teller-like Pseudo-Harmonic Oscillator. Mathematics. 2025; 13(9):1524. https://doi.org/10.3390/math13091524
Chicago/Turabian StyleAlrebdi, Haifa I., Uduakobong S. Okorie, Ridha Horchani, Gaotsiwe J. Rampho, and Akpan N. Ikot. 2025. "Thermophysical Properties and Expectation Values for Pöschl–Teller-like Pseudo-Harmonic Oscillator" Mathematics 13, no. 9: 1524. https://doi.org/10.3390/math13091524
APA StyleAlrebdi, H. I., Okorie, U. S., Horchani, R., Rampho, G. J., & Ikot, A. N. (2025). Thermophysical Properties and Expectation Values for Pöschl–Teller-like Pseudo-Harmonic Oscillator. Mathematics, 13(9), 1524. https://doi.org/10.3390/math13091524





