Analysis of MCP-Distributed Jammers and 3D Beam-Width Variations for UAV-Assisted C-V2X Millimeter-Wave Communications
Abstract
1. Introduction
1.1. Related Works
1.2. Motivation and Objectives
- The work presented in [11] considers a C-V2X network that evaluates the association probability, coverage probability, and rate performance of the V-N. However, our investigated system considers a UAV-assisted C-V2X network that leverages UAVs and MBSs by considering a millimeter-wave antenna and evaluates association, coverage, and SE of the V-N.
- The work presented in [16] considers a UAV-assisted cellular network that leverages LOS UAVs, NLOS UAVs, and MBSs. However, the work does not consider vehicular communications in the presence of jammers. Our method evaluates UAV-assisted C-V2X communications, exploiting jamming and millimeter-wave antennas.
- The work presented in [26] considers UAV-assisted C-V2X communications and evaluates bandwidth efficiency. However, the work does not consider jamming interference as well as 3D beamforming millimeter-wave antennas. Our analysis considers millimeter-wave antennas for UAV-assisted C-V2X communications by exploiting clustered jamming and evaluates association probability, coverage probability, and SE of the network. Also, our setup investigates the effect of 3D antenna beam-width variations on the system’s efficiency.
- A framework for a UAV-assisted C-V2X network, which considers jammers and beam variations, is presented.
- The performance of V2V, V2M, V2L, and V2N connections, considering clustered jamming, is evaluated in terms of coverage and SE by varying network parameters such as the number of V-Ns, MBSs, and LAPs.
- Analytical equations for the association and coverage of V2V, V2M, V2L, and V2N connections, along with the clustered jamming devices, are derived.
- The outcomes demonstrate that the effectiveness of UAV-assisted C-V2X transmissions is severely degraded in a network that is exploited by jamming and beam variations.
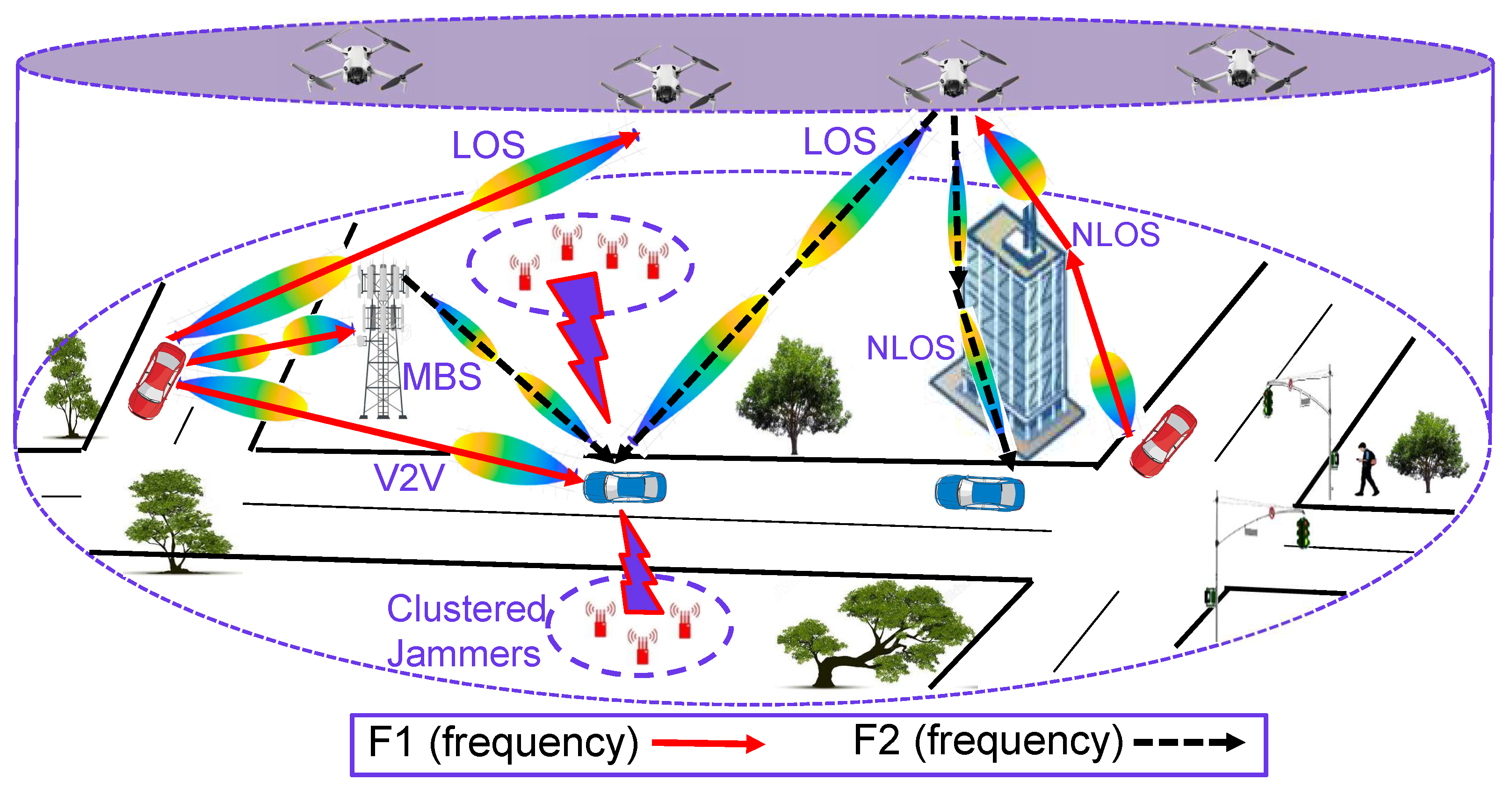
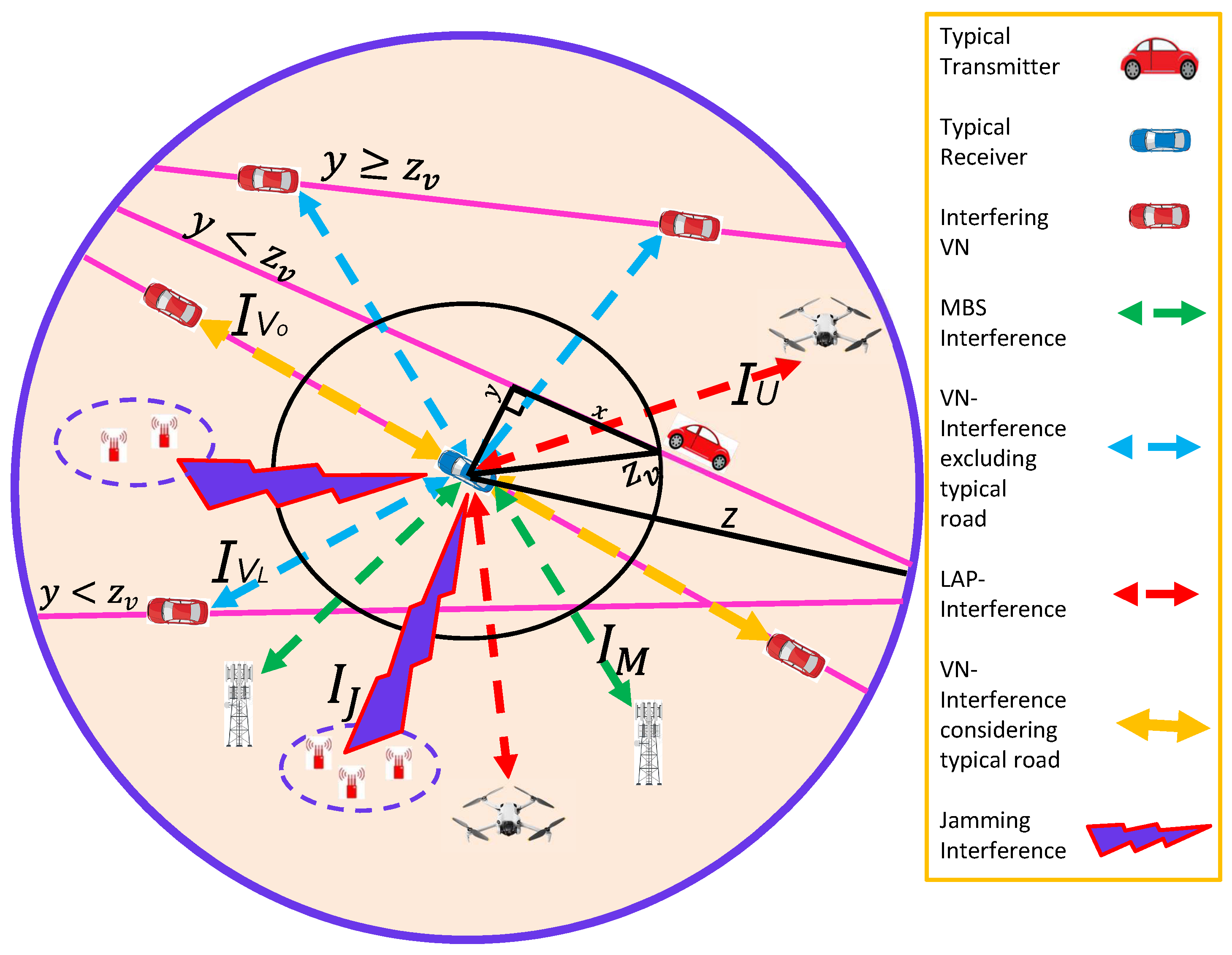
2. System Model
3. Distance Distribution
4. Typical Vehicle’s Association
4.1. Probability of V2V Association
4.2. Probability of V2M Association
4.3. Probability of V2L Association
4.4. Probability of V2N Association
5. Interference Analysis
5.1. V-Ns Interference
5.2. MBSs Interference
5.3. LOS-LAPs Interference
5.4. NLOS-LAPs Interference
5.5. Jammers Interference
6. Performance Metrics
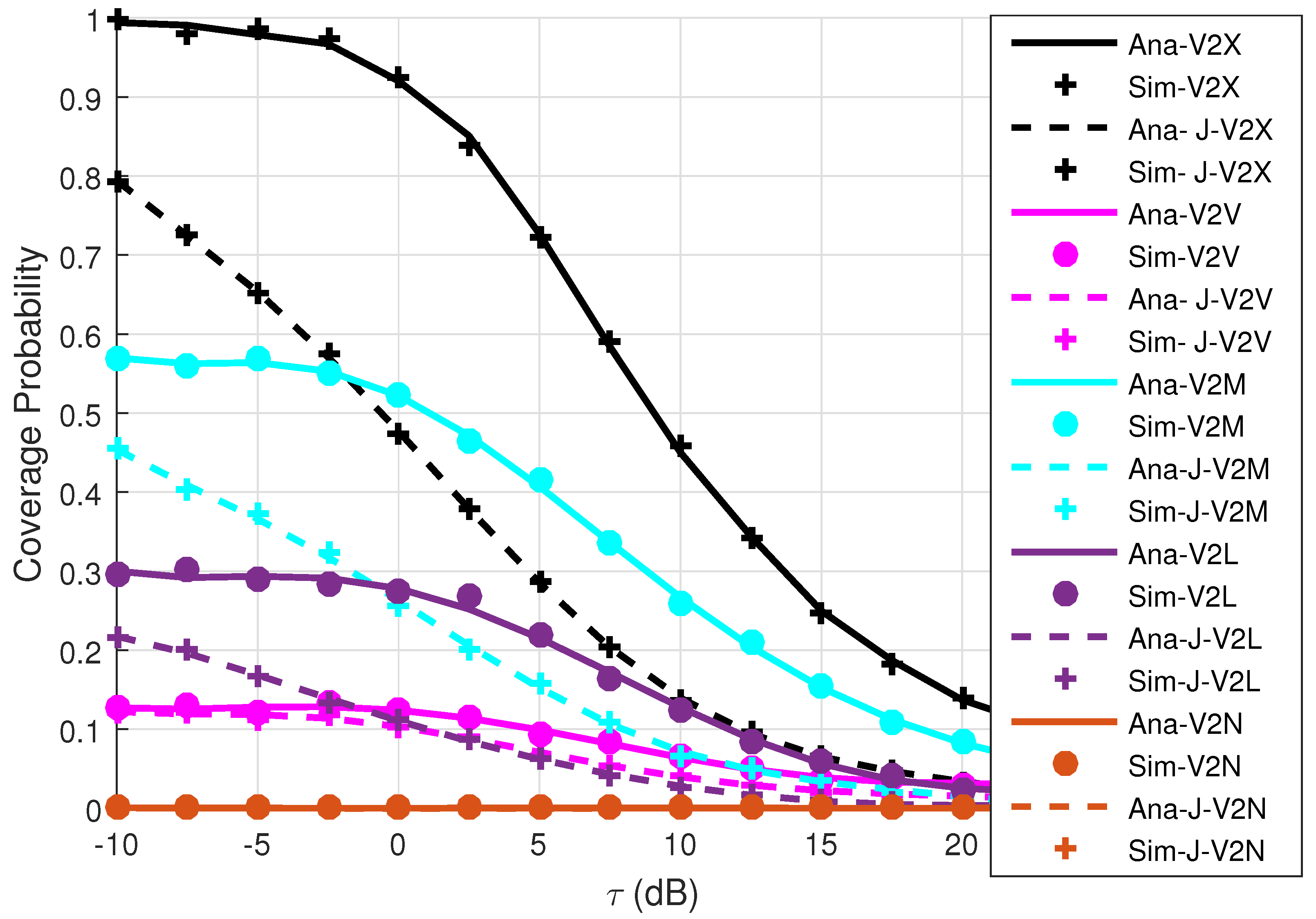
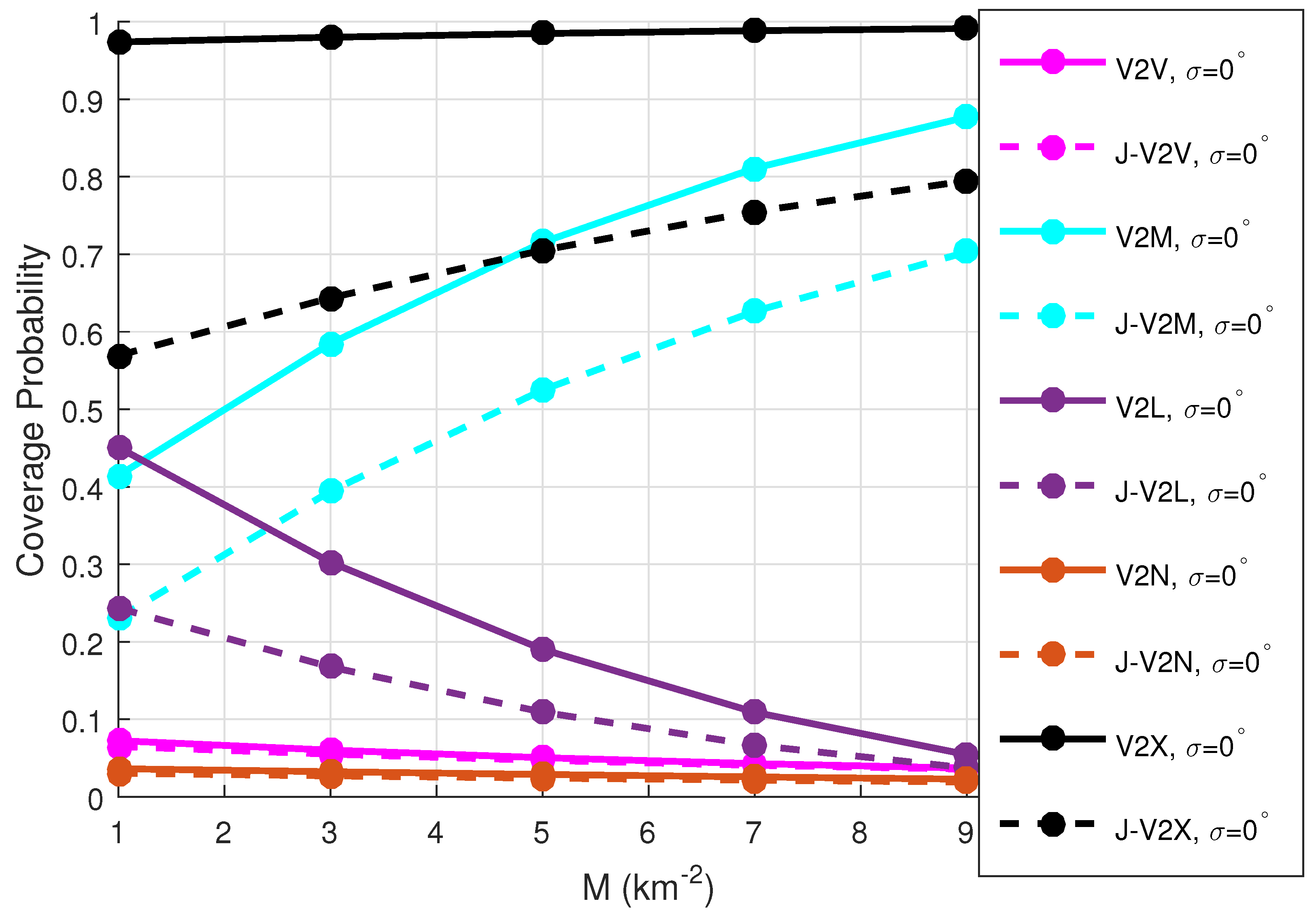
6.1. Coverage Probability
6.2. Spectrum Efficiency
7. Mitigating Jamming Interference
8. Network Setup, Results, and Discussion
8.1. Simulation Setup and Limitations
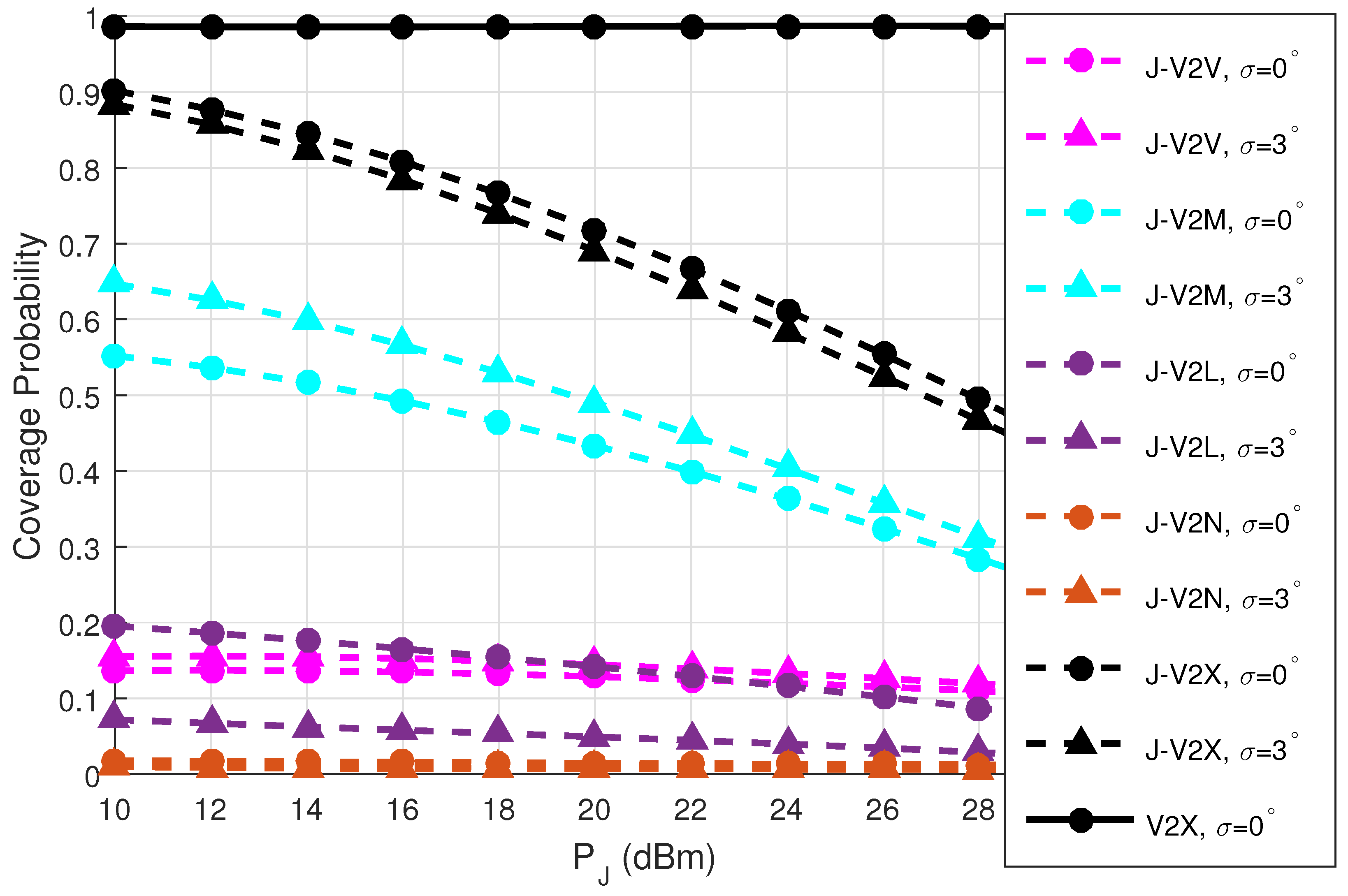
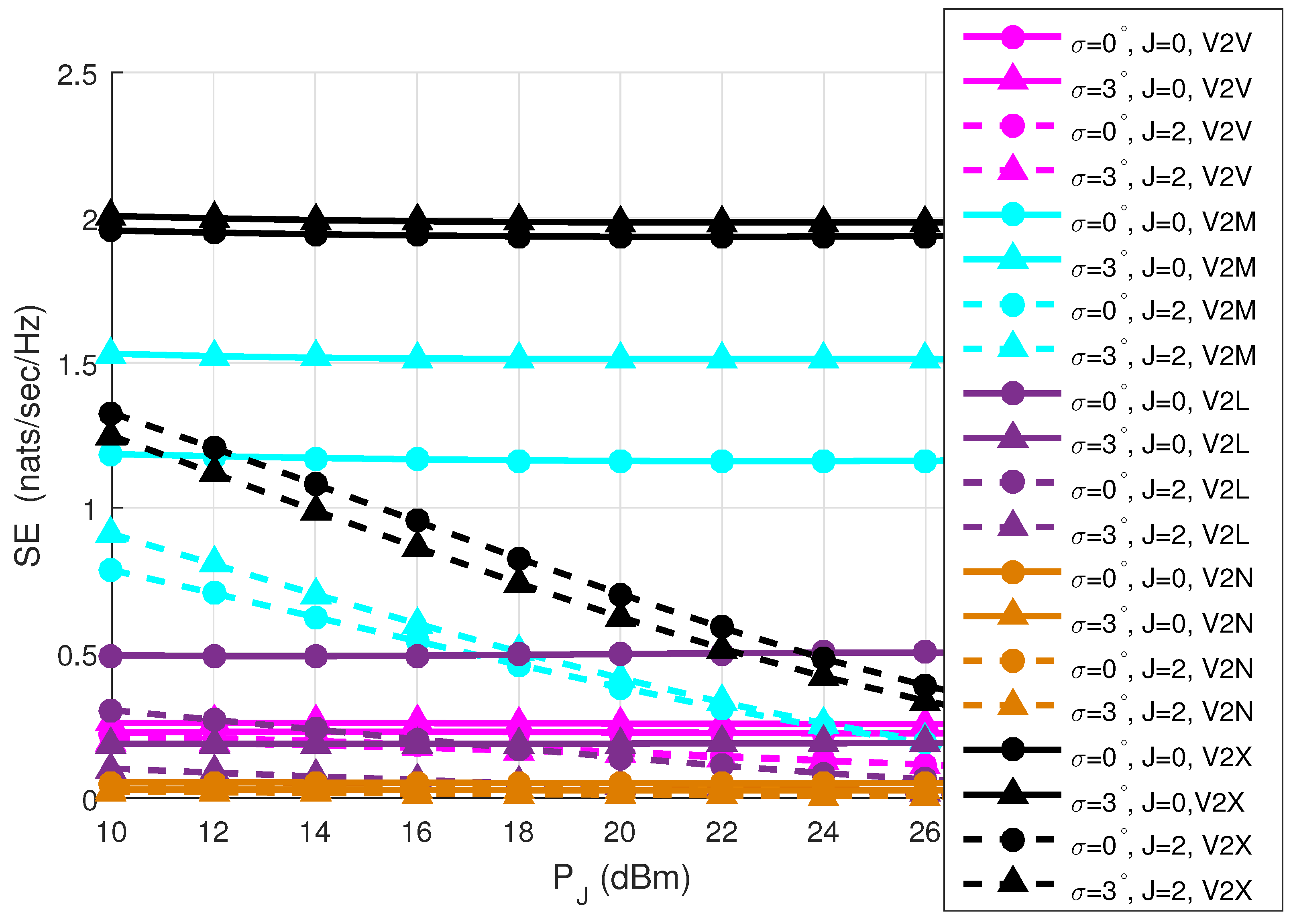
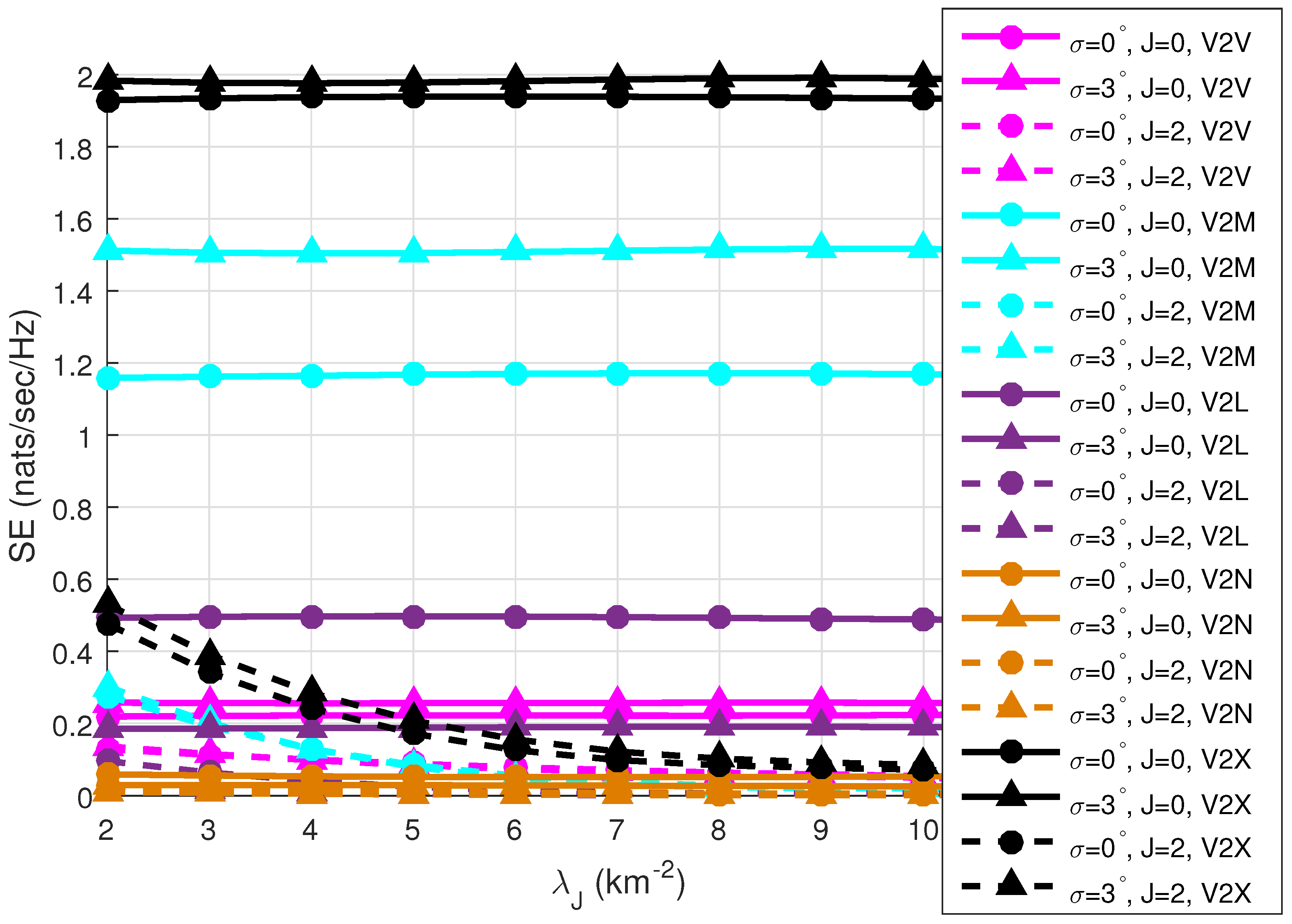
8.2. Results and Discussion
9. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. Derivation of (13)
Appendix B. Derivation of (40)
Appendix C. Derivation of (56)
References
- Arif, M.; Kim, W.; Qureshi, S. Interference characterization in cellular-assisted vehicular communications with Jamming. IEEE Access 2022, 10, 42469–42480. [Google Scholar] [CrossRef]
- Ganti, R.K.; Haenggi, M. Interference and outage in clustered wireless Ad Hoc networks. IEEE Trans. Inf. Theory 2009, 55, 4067–4086. [Google Scholar] [CrossRef]
- Wang, Y.; Zhu, Q. Modeling and analysis of small cells based on clustered stochastic geometry. IEEE Commun. Lett. 2017, 21, 576–579. [Google Scholar] [CrossRef]
- Chetlur, V.V.; Dhillon, H.S. Coverage analysis of a vehicular network modeled as Cox process driven by Poisson line process. IEEE Trans. Wirel. Commun. 2018, 17, 4401–4416. [Google Scholar] [CrossRef]
- Chetlur, V.V.; Dhillon, H.S. On the load distribution of vehicular users modeled by a Poisson line Cox process. IEEE Wirel. Commun. Lett. 2020, 9, 2121–2125. [Google Scholar] [CrossRef]
- Guha, S. Cellular-Assisted Vehicular Communications: A Stochastic Geometric Approach. Ph.D. Thesis, Virginia Tech, Blacksburg, VA, USA, 2016. [Google Scholar]
- Petrov, V.; Moltchanov, D.; Andreev, S.; Heath, R.W. Analysis of intelligent vehicular relaying in urban 5G+ millimeter-wave cellular deployments. In Proceedings of the 2019 IEEE Global Communications Conference (GLOBECOM), Waikoloa, HI, USA, 9–13 December 2019; pp. 1–6. [Google Scholar]
- Khabbaz, M.; Antoun, J.; Assi, C. Modeling and performance analysis of UAV-assisted vehicular networks. IEEE Trans. Veh. Technol. 2019, 68, 8384–8396. [Google Scholar] [CrossRef]
- Jang, D.; Song, H.L.; Ko, Y.C.; Kim, H.J. Distributed Beam Tracking for Vehicular Communications via UAV-Assisted Cellular Network. IEEE Trans. Veh. Technol. 2022, 72, 589–600. [Google Scholar] [CrossRef]
- Choi, C.S.; Baccelli, F. An analytical framework for coverage in cellular networks leveraging vehicles. IEEE Trans. Commun. 2018, 66, 4950–4964. [Google Scholar] [CrossRef]
- Sial, M.N.; Deng, Y.; Ahmed, J.; Nallanathan, A.; Dohler, M. Stochastic geometry modeling of cellular V2X communication over shared channels. IEEE Trans. Veh. Technol. 2019, 68, 11873–11887. [Google Scholar] [CrossRef]
- 3GPP. 3GPP TR36.885. Study on LTE-Based V2X Services (Release 14); Technical Specification Group Services System; 3GPP: Sophia Antipolis, France, 2016. [Google Scholar]
- Haroon, M.S.; Chae, S.H.; Jeon, S.W. Outage analysis for multi-radio heterogeneous networks in the presence of aerial jammers. ICT Express 2023, 9, 1026–1031. [Google Scholar] [CrossRef]
- Qasim, M.; Haroon, M.S.; Imran, M.; Muhammad, F.; Kim, S. 5G cellular networks: Coverage analysis in the presence of inter-cell interference and intentional jammers. Electronics 2020, 9, 1538. [Google Scholar] [CrossRef]
- Pandey, K.; Perumalla, K.R.; Gupta, A.K.; Dhillon, H.S. Fundamentals of vehicular communication networks with vehicle platoons. IEEE Trans. Wirel. Commun. 2023, 22, 8634–8649. [Google Scholar] [CrossRef]
- Peng, J.; Tang, W.; Zhang, H. Directional antennas modeling and coverage analysis of UAV-assisted networks. IEEE Wirel. Commun. Lett. 2022, 11, 2175–2179. [Google Scholar] [CrossRef]
- de Amorim, R.M.; Wigard, J.; Kovacs, I.Z.; Sorensen, T.B.; Mogensen, P.E. Enabling cellular communication for aerial vehicles: Providing reliability for future applications. IEEE Veh. Technol. Mag. 2020, 15, 129–135. [Google Scholar] [CrossRef]
- Cao, Y.; Xu, S.; Liu, J.; Kato, N. Toward smart and secure V2X communication in 5G and beyond: A UAV-enabled aerial intelligent reflecting surface solution. IEEE Veh. Technol. Mag. 2022, 17, 66–73. [Google Scholar] [CrossRef]
- Arif, M.; Kim, W. Analysis of U-V2X communications with non-Clustered and Clustered jamming in the presence of fluctuating UAV beam width. Mathematics 2023, 11, 3434. [Google Scholar] [CrossRef]
- Arif, M.; Hasna, M.O. Analysis of fluctuations of antenna pattern in U-V2X communications. Phys. Commun. 2023, 58, 102066. [Google Scholar] [CrossRef]
- Dabiri, M.T.; Hasna, M. Pointing Error Modeling of mmWave to THz High-Directional Antenna Arrays. IEEE Wirel. Commun. Lett. 2022, 11, 2435–2439. [Google Scholar] [CrossRef]
- Arif, M.; Kim, W. Analysis of Fluctuating Antenna Beamwidth in UAV-Assisted Cellular Networks. Mathematics 2023, 11, 4706. [Google Scholar] [CrossRef]
- Dabiri, M.T.; Hasna, M. 3D Uplink Channel Modeling of UAV-based mmWave Fronthaul Links for Future Small Cell Networks. IEEE Trans. Veh. Technol. 2022, 72, 1400–1413. [Google Scholar] [CrossRef]
- Arif, M.; Kim, W. Efficiency of UAV-assisted cellular networks under jamming scenarios. Veh. Commun. 2024, 49, 100833. [Google Scholar] [CrossRef]
- Arif, M.; Shakoor, A. Clustered jamming and antenna beam-width fluctuations for UAV-assisted cellular networks. Comput. Netw. 2024, 240, 110171. [Google Scholar] [CrossRef]
- Xu, Y.H.; Li, J.H.; Zhou, W.; Chen, C. Learning-empowered resource allocation for air slicing in UAV-assisted cellular V2X communications. IEEE Syst. J. 2022, 17, 1008–1011. [Google Scholar] [CrossRef]
- Chang, B.; Yan, X.; Zhang, L.; Chen, Z.; Li, L.; Imran, M.A. Joint communication and control for mmWave/THz beam alignment in V2X networks. IEEE Internet Things J. 2022, 9, 11203–11213. [Google Scholar] [CrossRef]
- Dabiri, M.T.; Sadough, S.M.S.; Ansari, I.S. Tractable optical channel modeling between UAVs. IEEE Trans. Veh. Technol. 2019, 68, 11543–11550. [Google Scholar] [CrossRef]
- Alzenad, M.; Yanikomeroglu, H. Coverage and rate analysis for vertical heterogeneous networks (VHetNets). IEEE Trans. Wirel. Commun. 2019, 18, 5643–5657. [Google Scholar] [CrossRef]
- Balanis, C.A. Antenna Theory: Analysis and Design; John Wiley & Sons: Hoboken, NJ, USA, 2015. [Google Scholar]
- 3GPP. 3GPP TR 38.901 V14.1.1. Study on Channel Model for Frequencies from 0.5 to 100 GHz (Release 17); Technical Specification Group Radio Access Network; 3GPP: Sophia Antipolis, France, 2017. [Google Scholar]
- Althunibat, S.; Dabiri, M.T.; Hasna, M.O.; Qaraqe, K. Secrecy analysis of directional mmWave UAV-based links under hovering fluctuations. IEEE Open J. Commun. Soc. 2023, 4, 3030–3039. [Google Scholar] [CrossRef]
- Andrews, J.G.; Baccelli, F.; Ganti, R.K. A tractable approach to coverage and rate in cellular networks. IEEE Trans. Commun. 2011, 59, 3122–3134. [Google Scholar] [CrossRef]
- Andrews, J.G.; Gupta, A.K.; Dhillon, H.S. A primer on cellular network analysis using stochastic geometry. arXiv 2016, arXiv:1604.03183. [Google Scholar] [CrossRef]
- Alzenad, M.; Yanikomeroglu, H. Coverage and rate analysis for unmanned aerial vehicle base stations with LoS/NLoS propagation. In Proceedings of the IEEE Globecom Workshops (IGCW), Abu Dhabi, United Arab Emirates, 9–13 December 2018; pp. 1–7. [Google Scholar] [CrossRef]
- Olver, F.W.; Lozier, D.W.; Boisvert, R.F.; Clark, C.W. NIST Handbook of Mathematical Functions Hardback and CD-ROM; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Twardokus, G.; Rahbari, H. Vehicle-to-nothing? In securing C-V2X against protocol-aware DoS attacks. In Proceedings of the IEEE INFOCOM 2022-IEEE Conference on Computer Communications, London, UK, 2–5 May 2022; pp. 1629–1638. [Google Scholar]
- Giang, A.T.; Tran, H.T.; Le, H.T.; Doan, N.Q.; Nguyen, M.H. Jamming attack in vehicular networks: Adaptively probabilistic channel surfing scheme. Wirel. Commun. Mob. Comput. 2022, 2022, 3884761. [Google Scholar] [CrossRef]
- Xiao, L.; Dai, H.; Ning, P. Jamming-resistant collaborative broadcast using uncoordinated frequency hopping. IEEE Trans. Inf. Forensics Secur. 2011, 7, 297–309. [Google Scholar] [CrossRef]
- Cheung, C.; Rawashdeh, S.; Mohammadi, A. Jam Mitigation for Autonomous Convoys via Behavior-Based Robotics. Appl. Sci. 2022, 12, 9863. [Google Scholar] [CrossRef]
- Yan, Q.; Zeng, H.; Jiang, T.; Li, M.; Lou, W.; Hou, Y.T. Jamming resilient communication using MIMO interference cancellation. IEEE Trans. Inf. Forensics Secur. 2016, 11, 1486–1499. [Google Scholar] [CrossRef]
- Pelechrinis, K.; Broustis, I.; Krishnamurthy, S.V.; Gkantsidis, C. A measurement-driven anti-jamming system for 802.11 networks. IEEE/ACM Trans. Netw. 2011, 19, 1208–1222. [Google Scholar] [CrossRef]
- Pelechrinis, K.; Broustis, I.; Krishnamurthy, S.V.; Gkantsidis, C. Ares: An anti-jamming reinforcement system for 802. In 11 networks. In Proceedings of the 5th International Conference on Emerging Networking Experiments and Technologies, Rome, Italy, 1–4 December 2009; pp. 181–192. [Google Scholar]




| Work | Main Focus of the System Model | Basic Outcome | Limitation |
|---|---|---|---|
| [4] | C-V2X network | Association and coverage probability derivation | Evaluation of millimeter wave connectivity and SE |
| [5] | C-V2X network | Probability of association of a V-N and user load on the base station | Evaluation of millimeter waves communications and SE |
| [10] | C-V2X network | Probability of association and coverage of a V-N | Analysis of UAVs employing millimeter waves communications and SE analysis. |
| [27] | C-V2X network | Analysis of millimeter waves and beam alignment closed-form analysis | Probability of association, coverage analysis, and SE analysis |
| [7] | V2X network | Analysis of millimeter waves and beam tracking | Vibrating beam width and coverage analysis |
| [6] | C-V2X network | probability of association, coverage analysis, and SE analysis | UAV-assisted C-V2X analysis |
| [11] | C-V2X network | Probability of association and coverage of a V-N | Analysis of millimeter waves, UAV-assisted C-V2X, and SE analysis |
| [1] | C-V2X network | Analysis of jamming, millimeter waves, probability of association, coverage analysis, and SE analysis | UAV-assisted V2X analysis |
| [21,23,28] | UAV-assisted network | Analysis of millimeter waves, vibrating beam-width modeling, and coverage analysis | U-V2X network and SE analysis |
| [9] | U-V2X network | Analysis of millimeter waves and beam-tracking | Beam-width vibrations, coverage analysis, and SE analysis |
| [8] | U-V2X network | Packet delay computation | Vibrating beam width, coverage, and SE analysis |
| [13] | Cellular network with aerial jammers | Analysis of jamming, millimeter waves analysis, probability of association analysis, and coverage analysis | UAV-assisted V2X analysis |
| [16] | UAV-assisted network | Probability of association and coverage analysis | Analysis of jamming signals, vibrating beam width, and SE analysis |
| [19] | U-V2X network | Analysis of jamming, millimeter waves, probability of association, coverage derivation, and SE derivation | UAV-assisted V2X analysis |
| [26] | UAV-assisted C-V2X network | Band-width efficiency analysis | Analysis of jamming signals, beam-width vibrations, probability of association, and coverage analysis |
| This work | UAV-assisted C-V2X network and clustered jamming | Analysis of clustered jammers, beam-width vibrations, association probability, coverage probability, and SE | – |
| Network Parameter | Value | Network Parameter | Value |
|---|---|---|---|
| 3/km2 | 3 | ||
| 3/km | 3 | ||
| 3/km2 | 4 | ||
| 6/km2 | 2.5 | ||
| −5 dB | 1 | ||
| 80 m | 20 | ||
| 12.08 | 20 | ||
| 0.21 | 0.001 | ||
| 0° | 10 MHz | ||
| 5 dB | 1 dB | ||
| 1 dB | 1 | ||
| 60 GHz | c | 0.3 G m/s | |
| 100 m | 2/km2 | ||
| J | 2 | 23 dBm | |
| 3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Arif, M.; Kim, W.; Iqbal, A.; Kim, S.W. Analysis of MCP-Distributed Jammers and 3D Beam-Width Variations for UAV-Assisted C-V2X Millimeter-Wave Communications. Mathematics 2025, 13, 1665. https://doi.org/10.3390/math13101665
Arif M, Kim W, Iqbal A, Kim SW. Analysis of MCP-Distributed Jammers and 3D Beam-Width Variations for UAV-Assisted C-V2X Millimeter-Wave Communications. Mathematics. 2025; 13(10):1665. https://doi.org/10.3390/math13101665
Chicago/Turabian StyleArif, Mohammad, Wooseong Kim, Adeel Iqbal, and Sung Won Kim. 2025. "Analysis of MCP-Distributed Jammers and 3D Beam-Width Variations for UAV-Assisted C-V2X Millimeter-Wave Communications" Mathematics 13, no. 10: 1665. https://doi.org/10.3390/math13101665
APA StyleArif, M., Kim, W., Iqbal, A., & Kim, S. W. (2025). Analysis of MCP-Distributed Jammers and 3D Beam-Width Variations for UAV-Assisted C-V2X Millimeter-Wave Communications. Mathematics, 13(10), 1665. https://doi.org/10.3390/math13101665







