The Explicit Form of the Unitary Representation of the Poincaré Group for Vector-Valued Wave Functions (Massive and Massless), with Applications to Photon Localization and Position Operators
Abstract
1. Introduction
2. Massive Vector Field
2.1. The Tangent Bundle
2.2. The Action of the Lorentz Group
2.3. Riemannian Metric on
2.4. The Hilbert Space and Unitary Representation
The Longitudinal-Transversal Split
2.5. The Limit
2.6. The Poincaré Group Lie Algebra
3. An Application of the Explicit Form: Photon Polarization Vectors Boosted to the Speed of Light
3.1. Action of Pure Boosts
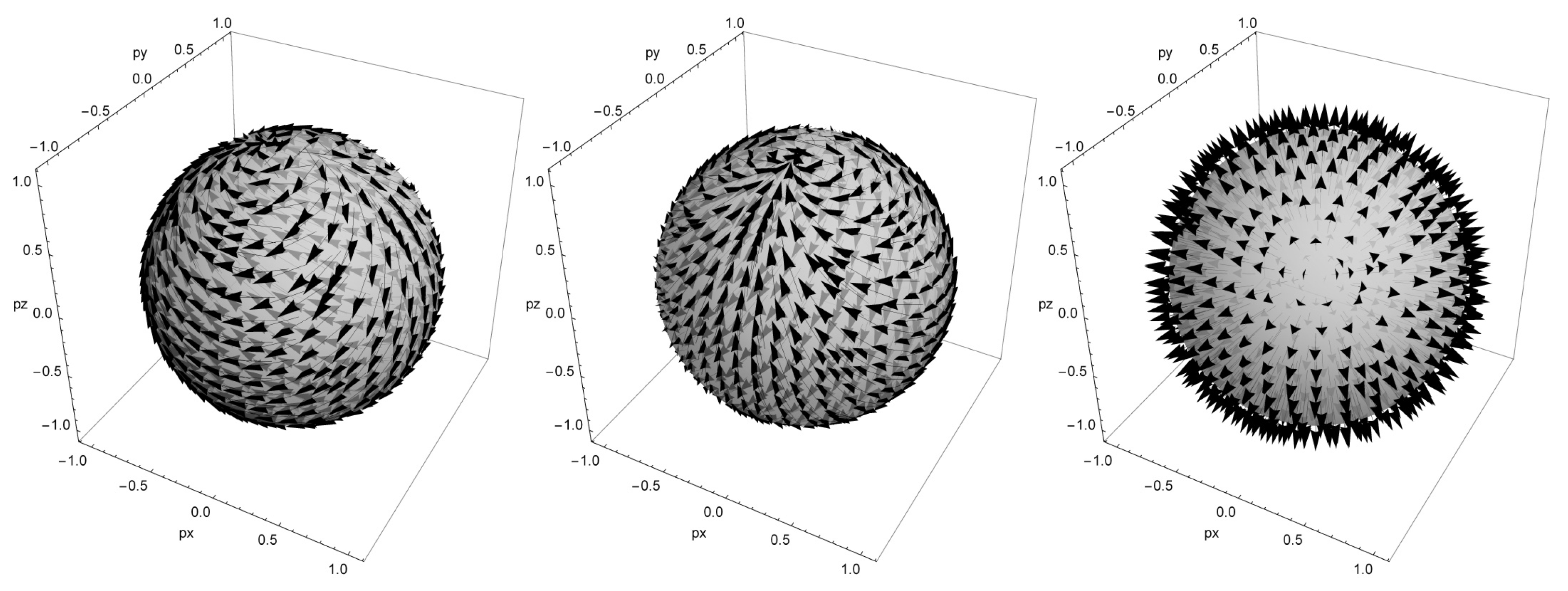
3.2. The Polarization Basis
Lorentz-Boost Eigenmodes
3.3. The Teleparallel Connection
3.3.1. Stereographic Coordinates on the Light Cone
3.3.2. The Connection Coefficients
4. Photon Position Operator with Commuting Components and Axial Symmetry
4.1. Position Operators as Covariant Derivatives
4.2. The Pryce Connection and Operator—Geometric Construction
4.2.1. The Pryce Connection Is Metric Semi-Symmetric
4.2.2. The Difference between the Teleparallel and Pryce Connections
4.2.3. The Pryce Operator
4.3. The Hawton–Baylis Operator
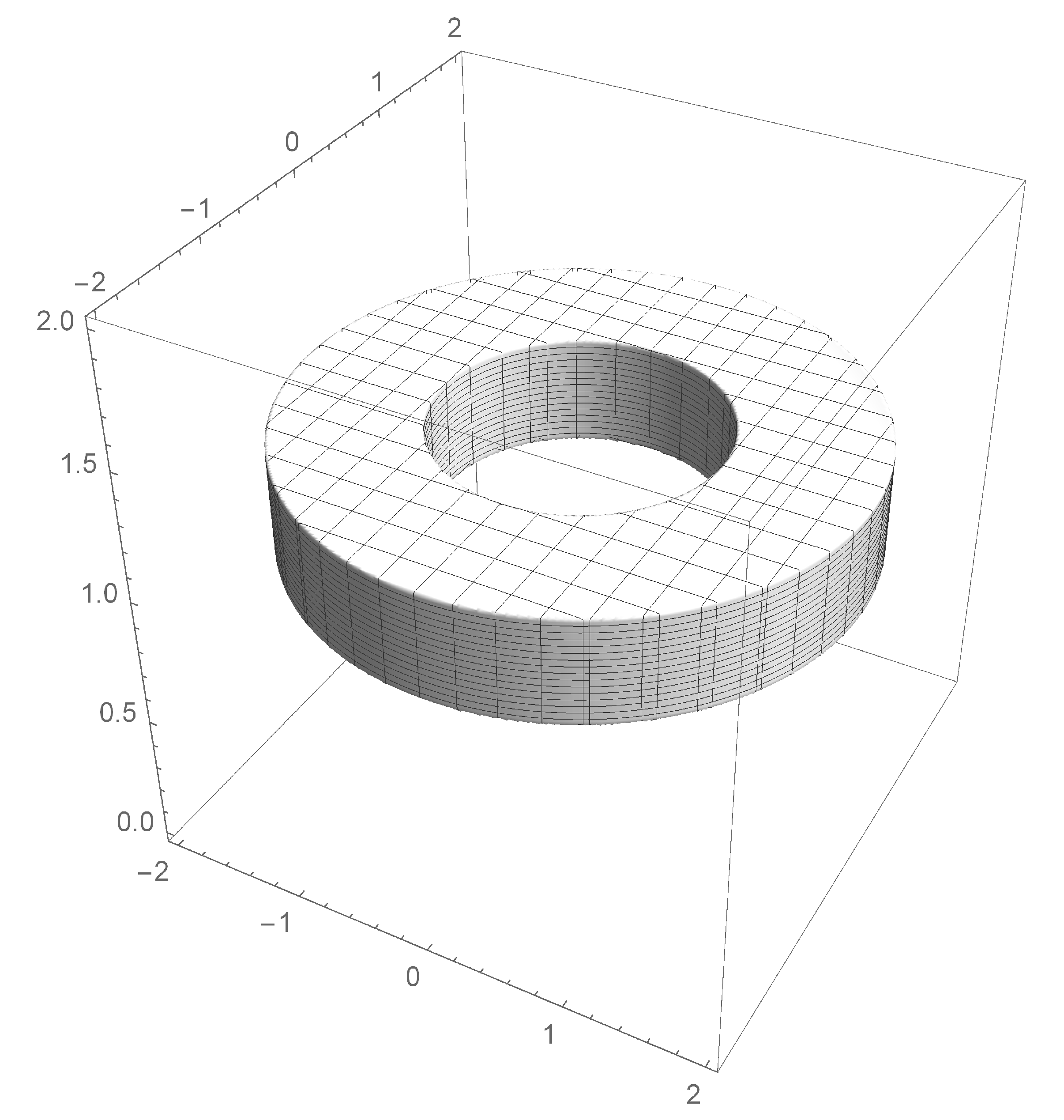
4.3.1. Photon States Localized on Circles
Loop States
Amrein’s Washer Photon States
4.4. POV Measure Photon Localization
5. Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Correction Statement
References
- Buchholz, D.; Cioli, F.; Ruzzi, G.; Vesselli, E. The universal C*-algebra of the electromagnetic field II. Topological charges and spacelike linear fields. Lett. Math. Phys. 2017, 107, 201–222. [Google Scholar] [CrossRef]
- Varadarajan, V.S. Geometry of Quantum Theory, 2nd ed.; Springer: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- Wightman, A.S. On the Localizability of Quantum Mechanical Systems. Rev. Mod. Phys. 1962, 34, 845–872. [Google Scholar] [CrossRef]
- Newton, T.D.; Wigner, E.P. Localized States for Elementary Systems. Rev. Mod. Phys. 1949, 21, 400–406. [Google Scholar] [CrossRef]
- Jauch, J.M.; Piron, C. Generalized Localizability. Helv. Phys. Acta 1962, 40, 539–570. [Google Scholar]
- Amrein, A.O. Localizability of Particles of Mass Zero. Helv. Phys. Acta 1969, 42, 149–190. [Google Scholar]
- Bialynicki-Birula, I.; Bialynicka-Birula, Z. Why photons cannot be sharply localized. Phys. Rev. A 2009, 79, 032112. [Google Scholar] [CrossRef]
- Mourad, J. Optimal photon localization. Phys. Lett. 1993, 182, 319–322. [Google Scholar] [CrossRef]
- Kraus, K. Position Observables of the Photon. In The Uncertainty Principle and Foundations of Quantum Mechanics; Price, W.C., Chissic, S.S., Eds.; John Wiley & Sons: London, UK; New York, NY, USA; Sydney, Australia; Toronto, ON, Canada, 1977; pp. 293–320. [Google Scholar]
- Skagerstam, B.S. Localization of Massless Spinning Particles and the Berry Phase. In On Klauder’s Path: A Field Trip; World Scientific: Singapore, 1994; pp. 209–222. [Google Scholar]
- Jackiv, R. Dirac’s Magnetic Monopole (Again). arXiv 2002, arXiv:hep-th/0212058. [Google Scholar]
- Jadczyk, A. Magnetic Charge Quantization and Generalized Imprimitivity Systems. Int. J. Theor. Phys. 1975, 14, 183–192. [Google Scholar] [CrossRef]
- Ciao, R.; Wu, Y.-S. Manifestations of Berry’s Topological Phase for the Photon. Phys. Rev. Lett. 1986, 57, 933–936. [Google Scholar]
- Bialynicki-Birula, I.; Bialynicka-Birula, Z. Berry’s phase in the relativistic theory of spinning particles. Phys. Rev. D 1987, 35, 2383–2387. [Google Scholar] [CrossRef]
- Hawton, M. Photon position operator with commuting components. Phys. Rev. A 1999, 59, 954–959. [Google Scholar] [CrossRef]
- Staruszkiewicz, A. On Affine Properties of the Light Cone and their Application in the Quantum Electrodynamics. Acta Phys. Pol. B 1973, 4, 57–63. [Google Scholar]
- Staruszkiewicz, A. On Parallel Displacement Within the Light Cone and its Application in the Electrodynamics of Charges Moving with the Velocity of Light. Int. J. Theor. Phys. 1973, 8, 247–252. [Google Scholar] [CrossRef]
- Hawton, M.; Baylis, W.E. Photon position operators and localized bases. Phys. Rev. A 2001, 64, 012101. [Google Scholar] [CrossRef][Green Version]
- Dobrski, M.; Przanowski, M.; Tosiek, J.; Turrubiates, F.J. The geometrical interpretation of the photon position operator. Phys. Rev. A 2021, 104, 042206. [Google Scholar] [CrossRef]
- Dobrski, M.; Przanowski, M.; Tosiek, J.; Turrubiates, F.J. Construction of a photon position operator with commuting components from natural axioms. Phys. Rev. A 2023, 107, 042208. [Google Scholar] [CrossRef]
- Hehl, F.W.; Itin, Y.; Obukhov, Y.N. On Kottler’s path: Origin and evolution of the premetric program in gravity and in electrodynamics. Int. J. Mod. Phys. D 2016, 25, 1640016. [Google Scholar] [CrossRef]
- Bialynicki-Birula, I. Photon Wave Function. arXiv 2005, arXiv:quant-ph/0508202v1. [Google Scholar]
- Keller, O. On the theory of spacial localization of photons. Phys. Rep. 2005, 411, 1–232. [Google Scholar] [CrossRef]
- Keller, O. Light the Physics of the Photon; CRC Press: Boca Raton, FL, USA, 2014. [Google Scholar]
- Ryder, L.H. Quantum Field Theory, 2nd ed.; Cambridge University Press: Cambridge, UK, 1966. [Google Scholar]
- Bialynicki-Birula, I.; Bialynicka-Birula, Z. The role of Riemann-Silberstein vector in classical and quantum theories of electrodynamics. J. Phys. A Math. Theor. 2013, 46, 053001. [Google Scholar] [CrossRef]
- Bliokh, K.Y. Lorentz-boost eigenmodes. Phys. Rev. A 2018, 98, 012143. [Google Scholar] [CrossRef]
- Hermann, R. Vector Bundles in Mathematical Physics, Volume I; W.A. Benjamin Inc.: New York, NY, USA, 1970. [Google Scholar]
- Greub, G.; Halpern, S.; Vanstone, R. Connections, Curvature, and Cohomology, Volume II; Academic Press: Cambridge, MA, USA, 1973. [Google Scholar]
- Crainic, N. Vector Bundles and Connections. Lecture Notes. 2016. Available online: http://www.staff.science.uu.nl/~crain101/DG-2016/chapter1.pdf (accessed on 24 December 2023).
- Jadczyk, A.; Jancewicz, B. Maximal Localizability of Photons. Bull. Acad. Polon. Sci. Ser. Math. Astr. Phys. 1973, 21, 477–483. [Google Scholar]
- Yano, K.; Imai, T. On Semisymmetric Metric Connections in a Sasakian Manifold. Kodai Math. Sem. Rep. 1977, 28, 150–158. [Google Scholar] [CrossRef]
- Yilmaz, H.B.; Zengin, F.O.; Uysal, S.A. On a Semi-Symmetric Metric Connection with a Special Condition on a Riemannian Manifold. Eur. J. Pure Appl. Math. 2011, 4, 152–161. [Google Scholar]
- Bacry, H. Localizability and Space in Quantum Physics; Springer: Berlin/Heidelberg, Germany, 1988. [Google Scholar]
- Hawton, M. Photon Position eigenvectors, Wigner’s little group, and Berry’s phase. J. Math. Phys. 2019, 60, 052104. [Google Scholar] [CrossRef]
- Shishkina, E.; Sitnik, S. Transmutations, Singular and Fractional Differential Equations with Applications to Mathematical Physics; Academic Press: Cambridge, MA, USA, 2020. [Google Scholar]
- Koczan, G.M. Physical unambiguity of the definition of the photon position operator and its special eigenstates. arXiv 2023, arXiv:2309.12125v2. [Google Scholar]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jadczyk, A. The Explicit Form of the Unitary Representation of the Poincaré Group for Vector-Valued Wave Functions (Massive and Massless), with Applications to Photon Localization and Position Operators. Mathematics 2024, 12, 1140. https://doi.org/10.3390/math12081140
Jadczyk A. The Explicit Form of the Unitary Representation of the Poincaré Group for Vector-Valued Wave Functions (Massive and Massless), with Applications to Photon Localization and Position Operators. Mathematics. 2024; 12(8):1140. https://doi.org/10.3390/math12081140
Chicago/Turabian StyleJadczyk, Arkadiusz. 2024. "The Explicit Form of the Unitary Representation of the Poincaré Group for Vector-Valued Wave Functions (Massive and Massless), with Applications to Photon Localization and Position Operators" Mathematics 12, no. 8: 1140. https://doi.org/10.3390/math12081140
APA StyleJadczyk, A. (2024). The Explicit Form of the Unitary Representation of the Poincaré Group for Vector-Valued Wave Functions (Massive and Massless), with Applications to Photon Localization and Position Operators. Mathematics, 12(8), 1140. https://doi.org/10.3390/math12081140





