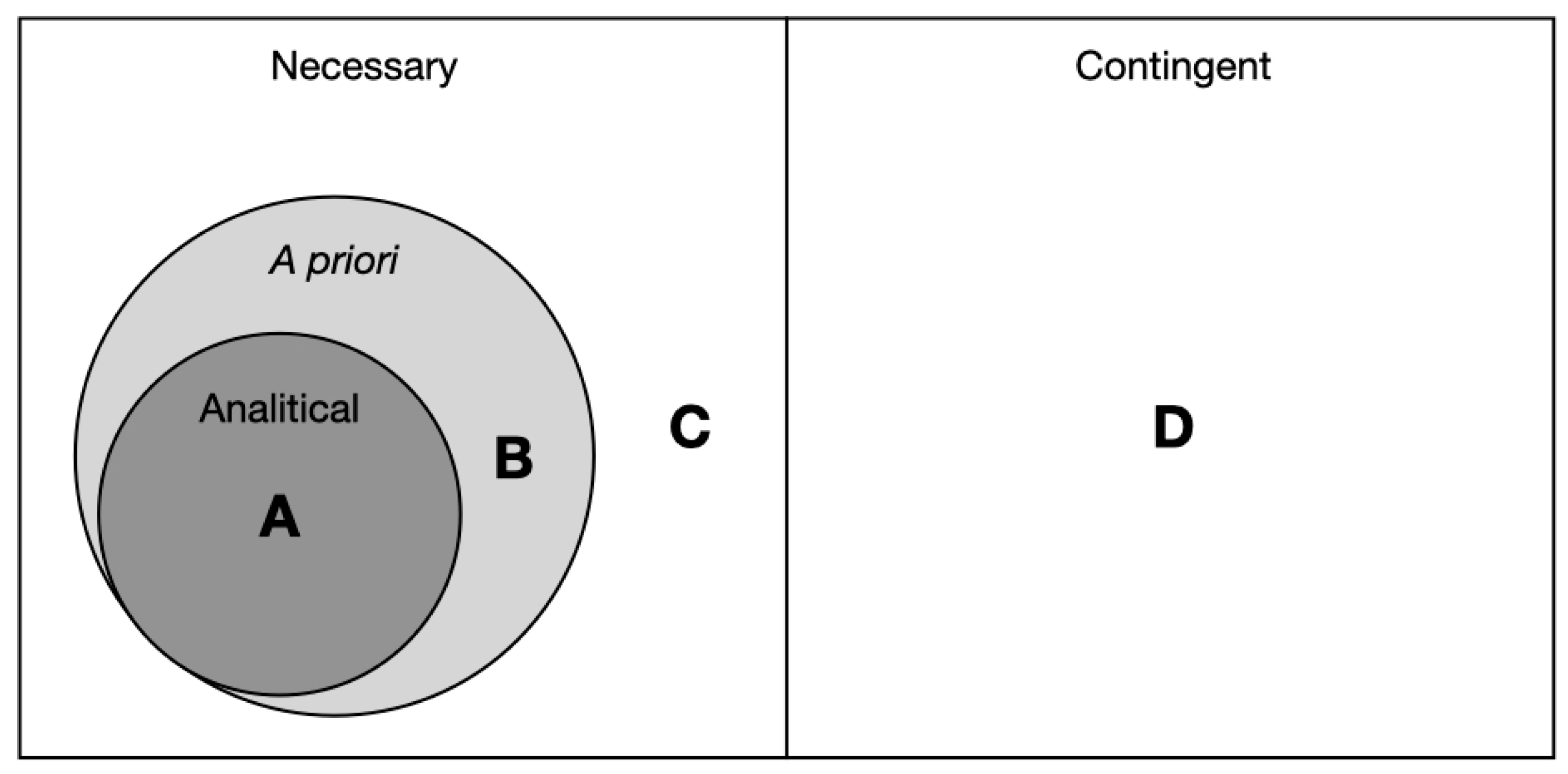
3.1. What Is Indubitably a Priori
The debate about whether the human mind is a tabula rasa at birth has been ongoing since Aristotle (De Anima), through the Stoics, Avicenna, and John Locke [
17] who gave the debate a modern tone. What is difficult to dispute is that there is a neuro-physiological a priori mechanism that allows for the integration of sensory data into a meaningful experiential whole. Otherwise, sensory experience would remain a fragmented field of unrelated elements. This conclusion has implications for our understanding of the possible nature of mathematical knowledge, as it opens up the possibility that it is as a priori as that mechanism, or that it is its derivative. In other words, mathematical knowledge could be biologically necessary. This mechanism is pre-experiential from a personal perspective. Even if we accept that the organization of our primitive sensory data would be impossible without a pre-experiential ability to organize it (that is, a priori principles of thinking by which we systematize unconnected sensory experiences into meaningful wholes), is what is pre-experiential, strictly speaking, knowledge? Does this presumed ‘knowledge’ in the form of thinking principles meet the criteria of the classical three-part definition of knowledge [
18], or some of its alternative formulations? It is difficult to say. If kidneys are not a priori knowledge, while filtering blood as a organ/mechanism, why would it be a biological mechanism that processes our neurophysiological responses to stimuli, that is, integrates experience? Information about its existence is a posteriori knowledge, but its existence is not. Thinking principles, as some have called this mechanism, are strictly not knowledge, because we neither believe in them nor do we do so justifiably. They simply are. In the preverbal stage, when we are already organizing primitive experience, we do not have concepts whose analysis would lead us to conclude about such principles, so they are not only not knowledge by the classical epistemological definition, but they are only pre-experiential and not a priori. Although knowledge can exist without the existence of concepts (for example, a seagull knows that when it drops a shell on a hard surface, it will break), the concepts of analytic and synthetic relate to the relationship between knowledge, language, and experience, not just knowledge and experience. Therefore, the first problem is how to view the principles of thinking in the context of the analytical–synthetic distinction, if they are presupposed. It is not disputed that young turtles when they hatch go towards the sea, but is it because they know that the sea is there? Or is it an instinctive genetically based impulse to which they submit without any belief or justification? We can treat that impulse as their collective knowledge, biologically encoded through generations, but accepting the validity of such individual knowledge is debatable. The past shows that many species have become extinct by following inherited instinctive patterns, which means that the sea may or may not have been there. What turtles actually possess is some structural property of their neuro-physiological apparatus, and at the same time, the structural property of their nucleic material, which stores and transmits this information. Therefore, we propose that what is “a priori”, serving to organize primitive personal experience, be understood as an inherent dispositional property of our biological organism, as a result of biological evolution, as something that is but is not knowledge; we propose that this be reduced to biological structure and function. Otherwise, we will end up in claims that existence is knowledge, and that spiders and turtles can believe. Therefore, the existence of a priori principles of thinking cannot be taken as a convincing proof of the a priori nature of mathematical knowledge, or of any knowledge at all—not even its possibilities.
3.2. Law of Identity: Description or Stipulation?
The Law of Identity represents the foundation of Aristotle’s bivalent logic and the basis of mathematics. In the history of the philosophy of mathematics, there have been several attempts to prove the necessity of the Law of Identity, among which are probably the most well-known proofs given by Ruth Barcan Marcus [
19]), including the simplified proof published five years later by Fitch [
20], and Kripke’s contribution to the proof [
21], which are collectively referred to as the Barcan–Kripke proof. The Barcan–Kripke proof of the necessity of the Law of Identity requires only the validity of Leibniz’s Law (Leibniz’s Law is a bi-conditional that claims the following: Necessarily, for anything,
x, and anything,
y,
x is identical to
y if and only if for any property
x has,
y has,
and for any property
y has,
x has. Because this is a
bi-conditional, it is comprised of two conditional statements (i) and (ii): (i) If
x is identical to
y, then for any property
x has,
y has and for any property
y has,
x has. (ii) If for any property
x has,
y has, and for any property
y has,
x has, then
x is identical to
y), and the reflexivity. The formulation of this proof, translated into ordinary language from the formal statements of modal logic in which it was originally presented, and taken from Lowe (2002) [
22], is as follows:
- (1)
For any object x, it is necessarily the case that x is identical to x (reflexivity).
- (2)
For any objects x and y, if x is identical to y, then whatever is true of x is true of y (Leibniz’s Law).
- (3)
a is identical with b (assumption).
- (4)
It is necessarily the case that a is identical with a (from (1)).
- (5)
It is true of a that a is necessarily identical with a (from (4)).
- (6)
If a is identical with b, then whatever is true of a is also true of b (from (2)).
- (7)
Whatever is true of a is also true of b (from (3) and (6)).
- (8)
It is true of b that it is necessarily identical with a (from (5) and (7)).
- (9)
It is necessarily the case that a is identical with b (from (8)).
- (10)
If a is identical with b, then it is necessarily the case that a is identical with b.
Although simple and without unnecessary assumptions, the Barcan–Kripke proof has been criticized. Lowe [
22], while ultimately not rejecting the proof, notes that the transition from (4) to (5) assumes what it is trying to prove by stating that
a is necessarily identical to
a, rather than
a being necessarily identical to
itself. Replacing premises (5), (8), and (9) to reflect this revision results in (9) becoming “It is necessarily the case that
b is identical with
b,” which renders the truth of the conclusion questionable ([
22], p. 87). The other argument against this proof is repeated in various forms in the literature and can be summarized as an objection that Kripke’s argument is circular because it only works for proper names or object constants construed as rigid designators in Kripke’s sense (In Kripke’s sense, a rigid designator is a term or expression that designates or refers to the same object in all possible worlds in which that object exists. This means that the referent of a rigid designator is necessarily the same entity, regardless of the specific circumstances or context in which it is referred to. For example, Kripke argues that proper names are rigid designators because they refer to the same individual in all possible worlds. So, if we consider the name “Joe Biden,” this name designates the same individual, Joe Biden, in every possible world in which he exists. Even if Joe Biden had not become President of the United States or had pursued a different career path, he would still be the same person referred to by the name “Joe Biden”. Kripke contrasts rigid designators with non-rigid or “accidental” designators, which are terms or expressions that may refer to different objects in different possible worlds. For example, the term “the current president of the United States” is a non-rigid designator, because it refers to different individuals in different possible worlds depending on who happens to hold that position in each world) (e.g., [
23]. Leibniz’s Law, as previously stated, is biconditional and says nothing about the nature of
a and
b. Therefore, Dale [
23] notes that “Kripke’s formalization of the argument for the necessity of identity overlooks identity relations involving non-rigidly designated relata, such as those expressed by means of definite descriptors, from which necessary identities cannot generally be inferred”.
To successfully challenge the necessity of the Law of Identity would mean automatically challenging the necessity of all its derivatives, all axioms and theorems that presuppose it. If it is anywhere possible to destroy the idea of unconditional necessity of mathematical knowledge with one move, then it is in the Law of Identity. In
Section 3.1, we illustrated the way in which the assumption of the a priori nature of knowledge leads to a problem. Now, we could examine the reasons that led to certain knowledge being transferred to the domain of a priori. Let’s take, for example, the Law of Identity, A = A. If it is purely conventional, the argument could go as follows: We first stipulated a relationship with certain properties, including universality, which we denote with the symbol ‘=’, and which by definition expresses that each A is in this relationship with itself. Imagine that we adopted: “Let ‘=’ be a relationship that we will call identity, and in which each A is with itself”. Then, we stated that each A is in that relationship with itself. This argument is circular because it is imposed by the previous definition, and it represents a version of petitio principii. Furthermore, there is still a lack of evidence that such a relationship has any existential meaning. Each A is identical to itself because it has been axiomatized that each A is necessarily identical to itself, but this is a matter of decision and convention. Since we don’t know how to prove the claim here and now, we have transferred it to the realm of a priori. The essence of the meaning of a priori in this maneuver is to express “believe without proof”. If we had constituted this relationship in another way, then each A could not be identical to itself. The question arises: where does the need come from to constitute a supposedly universal relationship in such a way, and why does the assumption that it is valid have numerous positive experiential verifications? No a priori, but properties of this world provide such a solid foundation for the claim that identity is universal. Furthermore, note that A = A has the form of a definition ([
24]). The question arises: what is this definition, logical, factual, or conventional, and how do we arrive at the knowledge of its necessity? Confirming the idea that it is a logical definition would have to reduce the Law under investigation to one of the logical axioms, which is a problem because we are currently investigating a logical axiom, so it cannot be adopted as a conclusion without being presupposed as a premise. So logically, it is unproven (which is the nature of an axiom). In general, it is pointless to logically prove logical axioms, and therefore the validation of the law of identity and other logical axioms is not within logic; but where is it, then? A trivial answer would be that it is self-evident, or a priori. In the same way, we could, by convention, axiomatize the reflexivity of any other relation, for example, A ≠ A. Its necessity, therefore, exists only to the extent that we axiomatize it, but isn’t that artificial, stipulated necessity? We do not believe that we would be happy to know that the basic foundation of mathematics is necessary simply because we agreed to consider it necessary. If the law of identity was a factual, descriptive definition, a highly confirmed generalization from experience, it would certainly not have the strength of necessity, but rather would be just a very likely, inductively created law, in the range of other experiential generalizations and scientific laws, which it actually is. A favorite refuge from this problem is the realm of the a priori. Transferring axiomatized claims to the realm of the a priori is a sure mask for unprovability. The law of identity is, they say, ‘a priori’. In order for this law to be a priori, it would first be necessary to prove such a problematic claim as the existence of a priori laws, second, we would all have to recognize the a priori necessity of this law. The author of these lines does not recognize such an emotion towards the law of identity in the world, in himself.
Therefore, the law of identity can only be a highly confirmed generalization from experience, it is certain but not necessary. Perhaps a comparison with ethics is striking. In ethics, the fundamental problem is how to derive ought from is. How did we derive must from is in the case of the law of identity, if we dare not derive ought from is, or cannot derive it from isn’t? How do we claim the necessity of the law of identity, when it is obviously just a generalization from experience to which general importance has been attributed by convention? The conclusion is that the law of identity is in the world, but not necessarily. It may be necessary in this world, because if it did not exist, it would be a different world. Or, it might not exist at all. The principle of universality, though, says that it is necessary in all worlds, which seems to be a strong statement.
At the end of this section, it might be appropriate to also point out a syntax inconsistency in mathematics. Namely, the relationship “=” is used in two very different ways. Take the following two statements as an example:
Thus, does the symbol “=” in these two statements have the same meaning? No. While the first represents a comparison operator, conveying identity, and can be truthfully evaluated as accurate (at least conditionally, by convention), the second symbol “=” represents something entirely different. It can represent either a comparison operator or an assignment operator. For example, if X already exists and “=” represents a comparison operator, then this statement can be truthfully evaluated. If X exists and “=” represents an assignment operator, then X takes on a new value. If X has no value and “=” represents an assignment operator, then X is constituted by this statement. In the case where X has no value and “=” represents a comparison operator, the statement represents nonsense. This syntactic confusion in mathematics has been successfully resolved in programming languages where different symbols have been adopted for comparison and assignment operators.
3.3. Principium Contradictionis
Problems similar to those related to the law of identity also apply to the other three basic principles of reasoning. For example, the second principle of reasoning, the principle of non-contradiction (principle of contradictionis), is often formulated as: “Two opposing statements cannot be true in the same sense at the same time,” or formally, statements “A = B” and “A ≠ B” exclude each other.
Aristotle observed in his Metaphysics that the principle of non-contradiction has a special status, namely, that it cannot be rejected without prior acceptance. Philosophers later concluded from this that it was a priori and necessary. However, this conclusion is not entirely correct. Let’s consider two statements:
P: The principle of non-contradiction is
Q: If the principle of non-contradiction is, it is necessarily (cannot not be)
Did Aristotle assert P, or P => Q? Namely, what interests us is only P, not P => Q, and therefore the right question is not: “Can the law of non-contradiction be rejected, but not accepted?”, but rather: “Can the law of non-contradiction not be accepted, but not accepted?”. The premise, not the conclusion, is problematic here. The impossibility of rejection here resembles a Constitution in which one of the members stipulates that this Constitution cannot be changed; in a similar way, the principle of non-contradiction appears to stipulate its own existence, once it exists—but does it exist?
Although the world is obviously asymmetrical in terms of providing evidence of the validity of the principle of non-contradiction, and any alternatives, there are still rare examples that can show that this principle is not necessary. For example, in Leszek Kołakowski’s Philosophical Essays [
25], although in a different context, the author states that the three notions, consistent inconsistency, inconsistent inconsistency, and consistent inconsistency, resist the application of the principle of non-contradiction in a specific way. Kołakowski argues that consistent inconsistency cannot truly be consistent inconsistency because it excludes the principle of inconsistency from the realm of the inconsistent, and therefore, it cannot be consistent. Hence, in order for inconsistency to be consistent, it must be inconsistent! Formally expressed, this observation leads to two expressions that virtually opposes the principle of non-contradiction:
1: Consistent inconsistency ≠ Consistent inconsistency
2: Inconsistent inconsistency = Consistent inconsistency
Further, examples of questioning and some other axioms of logic can also be found, for example, the principle of the exclusion of the third (principium ehclusi tertii sine medii). Take the concept of “totality”. Totality as a concept represents both one and many. It is simultaneously A and non-A. Proponents of dialectical logic believe that the concept of “totality” is at a higher evolutionary level than non-veridical Cartesian dualism. The entire classical formal–logical system is an idealization of the world, its necessity is artificially stipulated and as such may not be, and its practical value is highly confirmed. This is an axiomatized, closed system, which is absolutely consistent in its self-sufficiency. Its internal properties are not problematic, they are stipulated, conventionally necessary, and what is problematic is the connection to the world. This connection actually means an answer to the question: why is the axiomatized system of binary Aristotelian logic privileged in some way? Are the rules of logic necessary in the world, just as they are “necessary” in the axiomatized logical system? Is it an idealization, just as a mathematical cube is a nonexistent idealization, to which reality corresponds more or less approximately?
The above-mentioned weaknesses directly affect one of the most common methods of proof, reductio ad absurdum. Namely, if the necessity of the law of identity is challenged, then contradictions also lose their destructive power and no longer have the force of necessity. All mathematical theorems proven by reduction to contradiction become only as necessary as the law of identity itself. This means they become only contingent, a posteriori, and nothing more.
3.5. On the Origin and Content of Mathematical and Logical Knowledge
Criticisms of realism are not as devastating as they appeared at first glance, as seem in the previous section. So, where does mathematical knowledge come from? In our opinion, mathematical and logical knowledge is nothing but a highly formalized reflection, grounded in the physical laws of conservation. It is a picture of the physics of this nature. It relates to this world. Its necessity is conditional, that is, mathematical knowledge is just as much as the world is. It is a statement about the contingent; even if it is a necessary statement about the contingent, its necessity is limited by the contingency of the subject of the statement. This is a conditional necessity, and a conditional necessity is not necessity. The law of identity is a generalization of the observation that I am, that I am in a series of moments t
0 … t
n, that I am sustained in time, that the objects of my perceptions are sustained, that they last, that they are not multiplied, that they do not arise from nothing, that they do not disappear (but only transform). The whole world of conservation laws tells us about identity from the first moment—(matter and) energy, momentum, angular momentum, electrification are sustained. The world is sustained. The world is. The world is the world. A is A. The parallelism we proclaim here may seem counterintuitive, but it can be approached through a thought experiment. Let us imagine an extremely simplified version of our world (a world in which all conservation laws hold): an elastic ball bouncing off the walls of a closed rectangular container (
Figure 3a). Although simplified, this representation of the world contains almost all conservation laws, and by observing it, the same laws of conservation could be inductively derived as in the case of our world. The conservation of mass and energy, momentum, and other conservation laws implies a series of regularities in the motion of this ball, including its inertial properties, the identity of the incident and outgoing angles, regularity in the evolution of its spatiotemporal coordinates, etc. In this experimental model-world, deriving the necessity of the law of identity would be just as justified or expected as in the real world. However, if we were to initiate a series of sequential suspensions of conservation laws, for example, remove momentum conservation, then energy conservation, etc., it would lead to seemingly “irregular” (compared to the rules of the real world to which we are accustomed) motion of the ball; it would begin bouncing at different angles, accelerating and decelerating, disappearing and reappearing, changing shape, size, and the number of copies of the ball (
Figure 3b). Complete degradation of the laws of conservation would lead to a situation where the result of the model-world evolution becomes absolute chaos. All continuities and regularities would be eliminated, and all physical laws, in addition to the laws of conservation, would no longer apply. This suggests that the laws of conservation are a prerequisite for the possibility of physical laws, but this topic goes beyond the scope of this study. In order to consistently apply the suspension of the laws of conservation, we would also have to apply it to the observer–world system, not just the world, because we too are subject to its rules in this world. Even the observer would become temporary, losing the continuity of their physical existence and consciousness, including the consciousness of the experiment itself (in an idealistic interpretation, the elimination of consciousness would actually eliminate the model-world) (
Figure 3c). Everything would disappear. The question is: would the law of identity still hold true in this new, chaotic, and unstable world? What would it refer to? It would not apply to any element in the model-world, even the model-world taken as a whole, because there would no longer be A and A, either in the sense of two separate objects or in the sense of one object observed continuously at different points in time. In the absence of introspective self-perception of the continuity of the observer’s consciousness, the law of identity could not even abstractly reflect that. Binary logic and its necessity would become pure convention, an abstract game of symbols devoid of any ontology. Binary logic would not contain in itself the power of its own applicability to this model-world, as apparently it is not its intrinsic property—it draws that power from the regularities which ultimately have their origin in the laws of conservation. In a completely different experiment, where we would not degrade the laws of conservation in the model-world, but instead replace them with alternatives of a completely different nature, say the rule that in successive moments of time the shape of a ball always changes to that of a cube, writing A = A would no longer have the same meaning.
Therefore, mathematics (and logic) is a reflection of the world. This world. So, the question of the necessity of mathematics reduces to the question of the possibility of another world, a world in which there would be no laws of conservation. The problem is that that world in which there would be no laws of conservation would also be—unsustainable. It could be temporary, sporadic, localized, but it could not be in every sense. Would we be able to show its temporariness in a temporarily sustainable world? Hardly. It could look as sustainable as an unconditionally sustainable world; and that could or could not be.
3.6. Implications for Number Theory
Given that our study advocates a realistic view of the ontology of logic and mathematics, it would be appropriate to address some of the problems that a realistic interpretation encounters in the field of number theory, especially with numbers beyond natural numbers. Realistic ontology of mathematics and logic have implications for the theory of complex numbers, quaternions, and other number types beyond natural numbers, which are often considered to be purely abstract and theoretical, suggesting a non-realistic ontology of mathematics. The answer could be that physicalistic interpretations of the ontology of complex and other numbers beyond natural (or real) numbers are not obvious, but not impossible. For example, in the case of complex numbers, one could argue that the concept of a complex number was developed to explain certain physical phenomena, such as electrical circuits and electromagnetic waves. The use of complex numbers in physics suggests that there may be a connection between the mathematical structure of complex numbers and the behavior of physical systems. In the past, the use of complex numbers in physical theories often caused discomfort because it was intuitively believed to undermine the realistic character of theoretical constructs and to be difficult to interpret physical correlates of complex numbers. A paradigmatic example of a physical theory that uses complex numbers is quantum mechanics. In his letter to Lorenzo in 1926, one of the founders of quantum mechanics, Schrödinger [
27] writes: “What is unpleasant here, and indeed directly to be objected to, is the use of complex numbers.
Ψ [wave function] is surely fundamentally a real function”, clearly demonstrating his own spontaneous realistic standpoint in the interpretation of quantum mechanics formalisms. One response could be that complex numbers are used out of convenience, not out of necessity [
28]. The debate on whether complex numbers can be replaced by real numbers in the Hilbert space formulation of quantum theory without limiting its predictions has been ongoing until recently. In a recent study, it has been shown that quantum theory without complex numbers can be experimentally falsified [
27], refuting the results of some other studies that claimed the opposite (e.g., [
29]). This key study has provided a direct connection between the ontology of complex numbers and experimental evidence, confirming the existence of empirical correlates of complex numbers, or the incompleteness of a mathematical apparatus that would be devoid of complex numbers in describing physical reality. Therefore, although the physicalist interpretation of the ontology of numbers beyond natural numbers is not obvious, and not without challenges, it is possible, and lately, it is experienceing its experimental verification.