Analytical Approximations of Well Function by Solving the Governing Differential Equation Representing Unsteady Groundwater Flow in a Confined Aquifer
Abstract
1. Introduction
2. Brief Overview of Homotopy-Based Methods
2.1. Homotopy Analysis Method
2.2. Homotopy Perturbation Method
2.3. Optimal Homotopy Asymptotic Method
3. Governing Equation and Solution Methodologies
3.1. Theis Solution
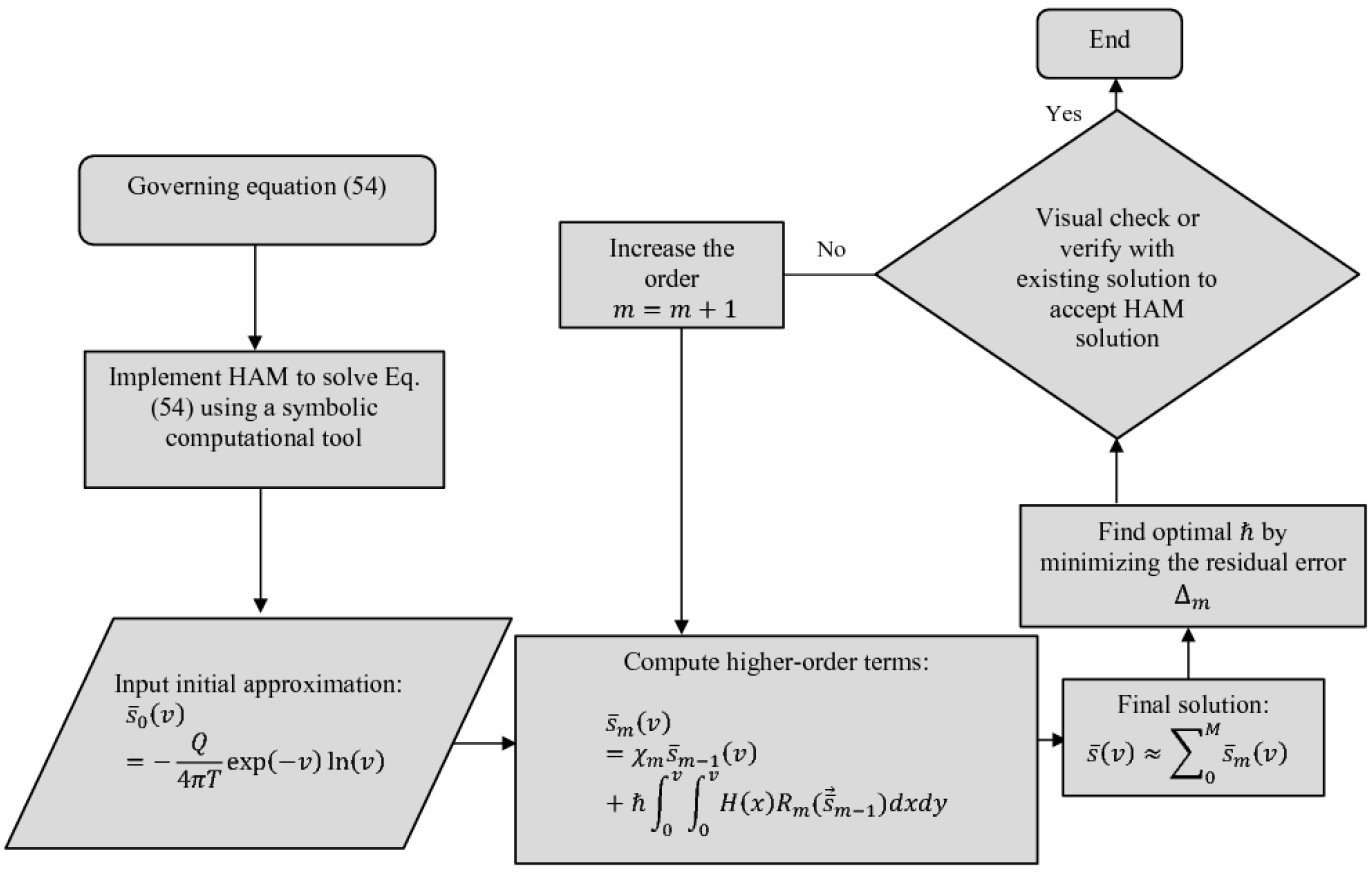
3.2. Homotopy-Based Solutions
3.2.1. HAM-Based Solution
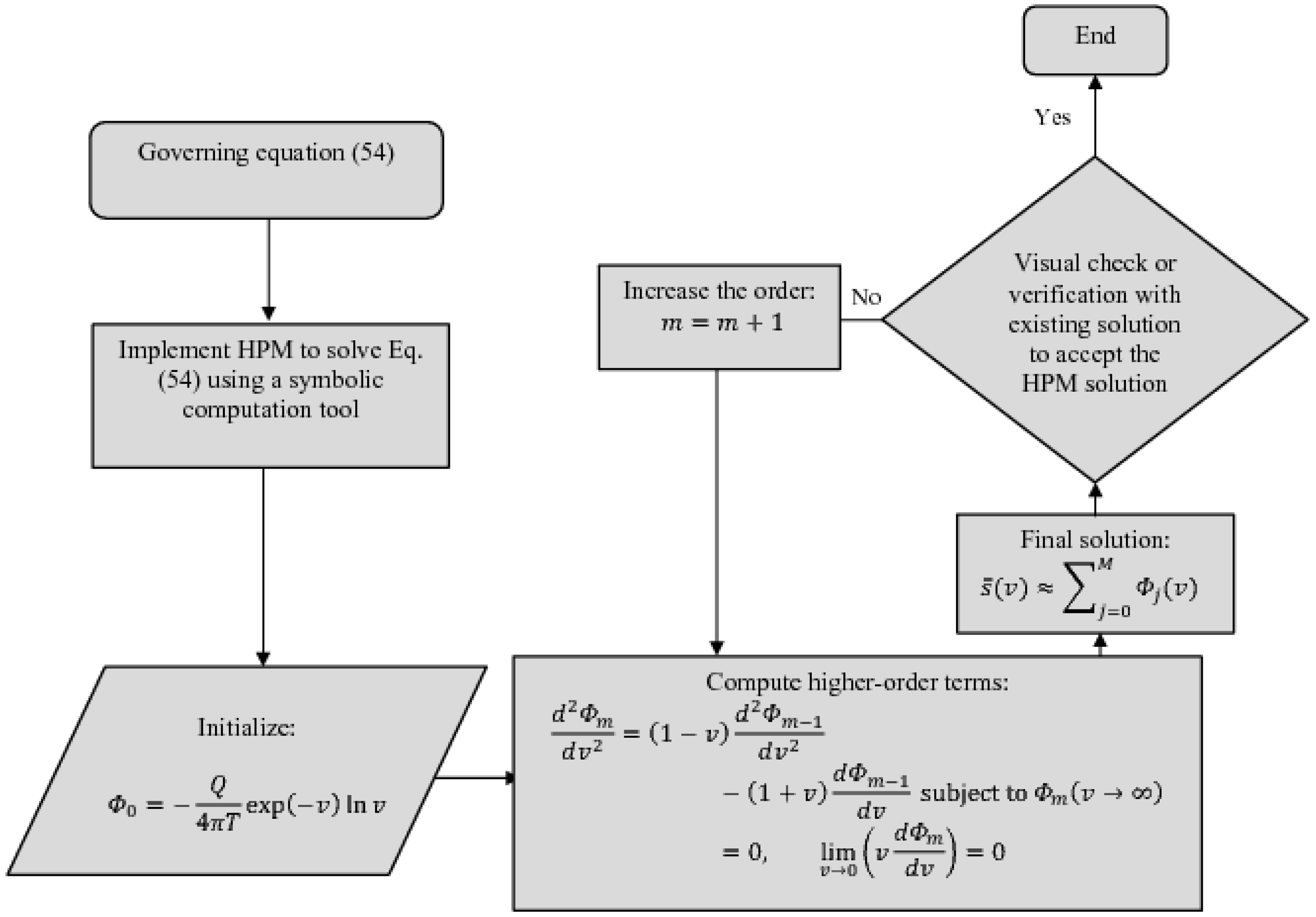
3.2.2. HPM-Based Solution
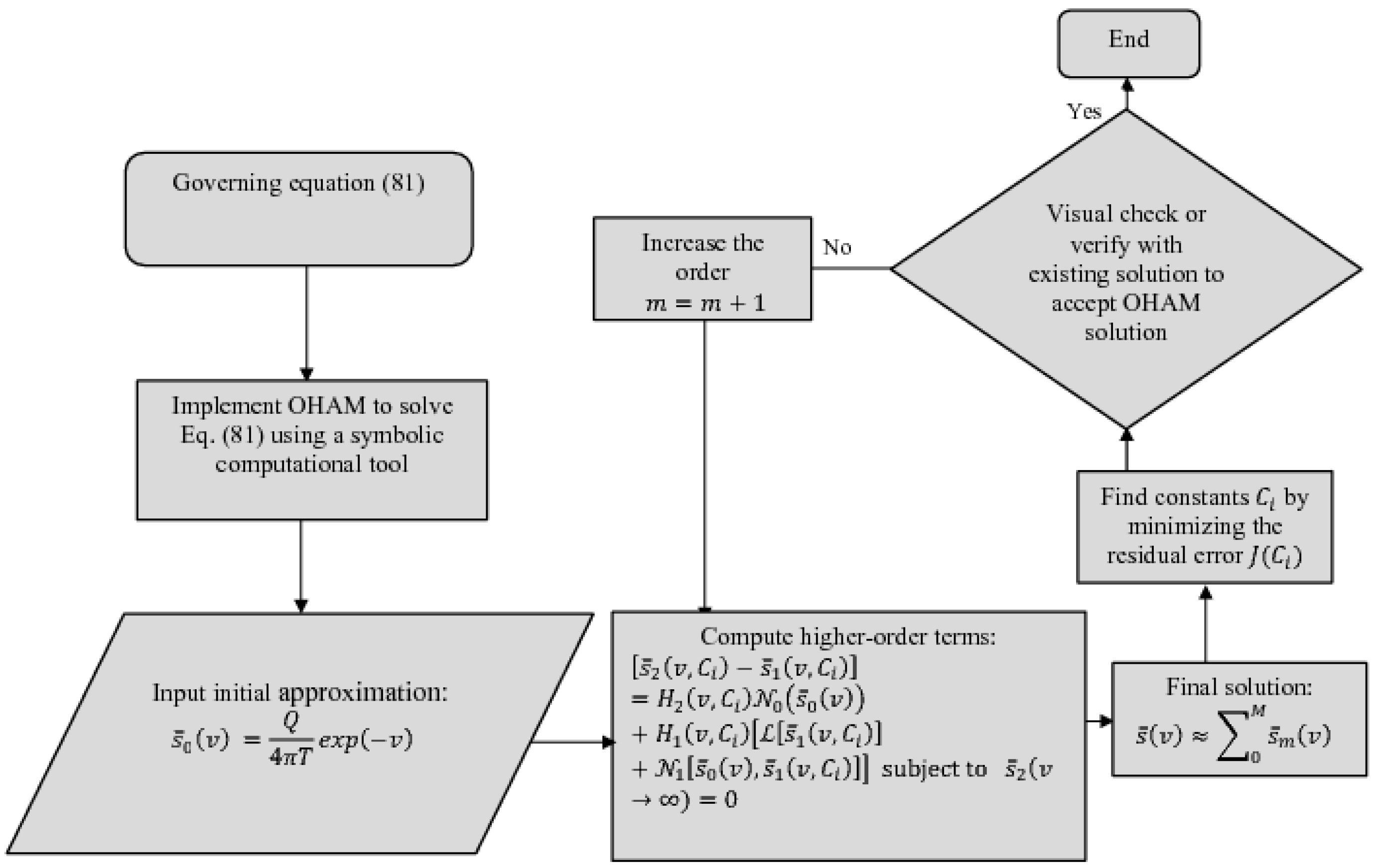
3.2.3. OHAM-Based Solution
4. Results and Discussion
4.1. Validation of the Well Function’s Approximations
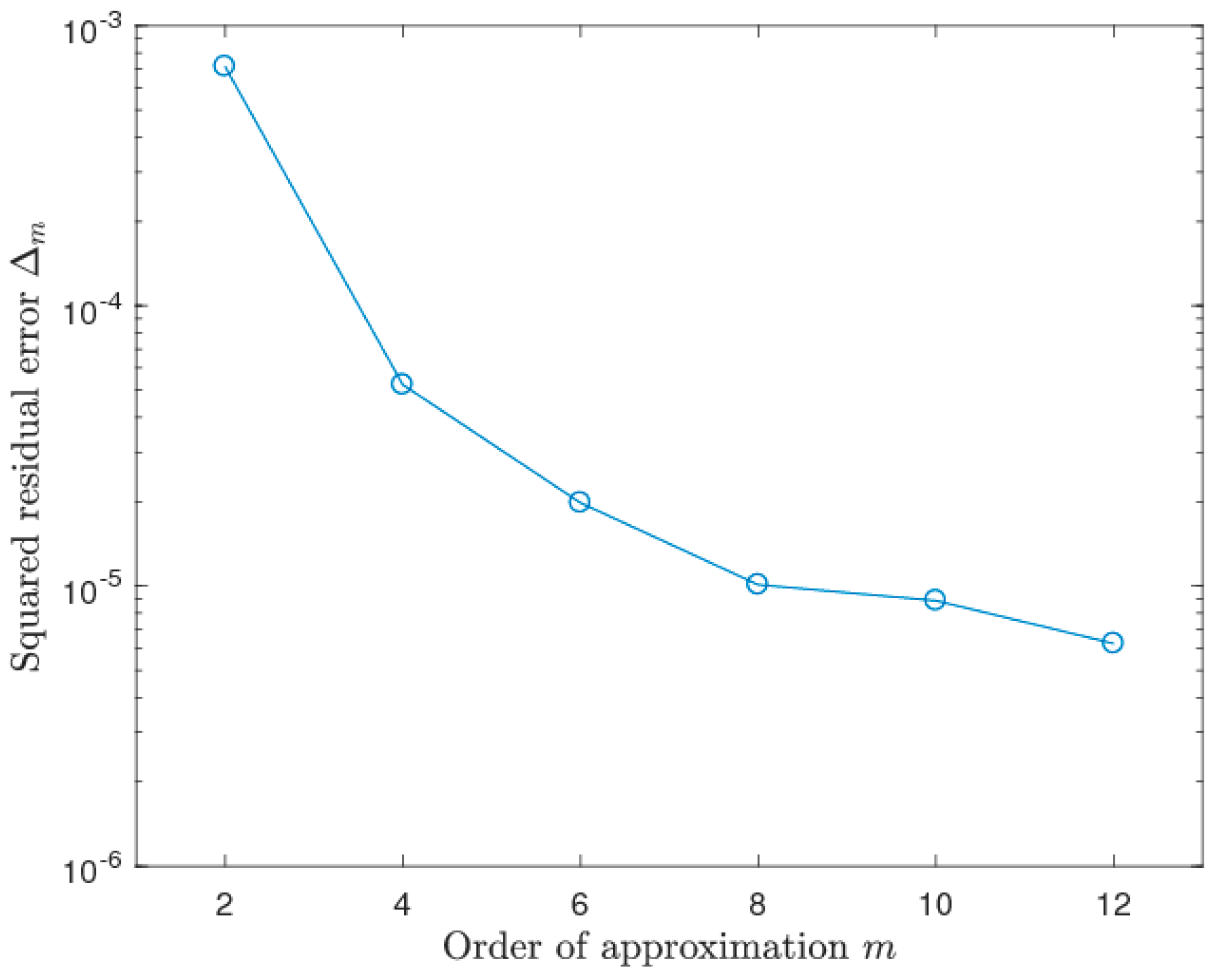
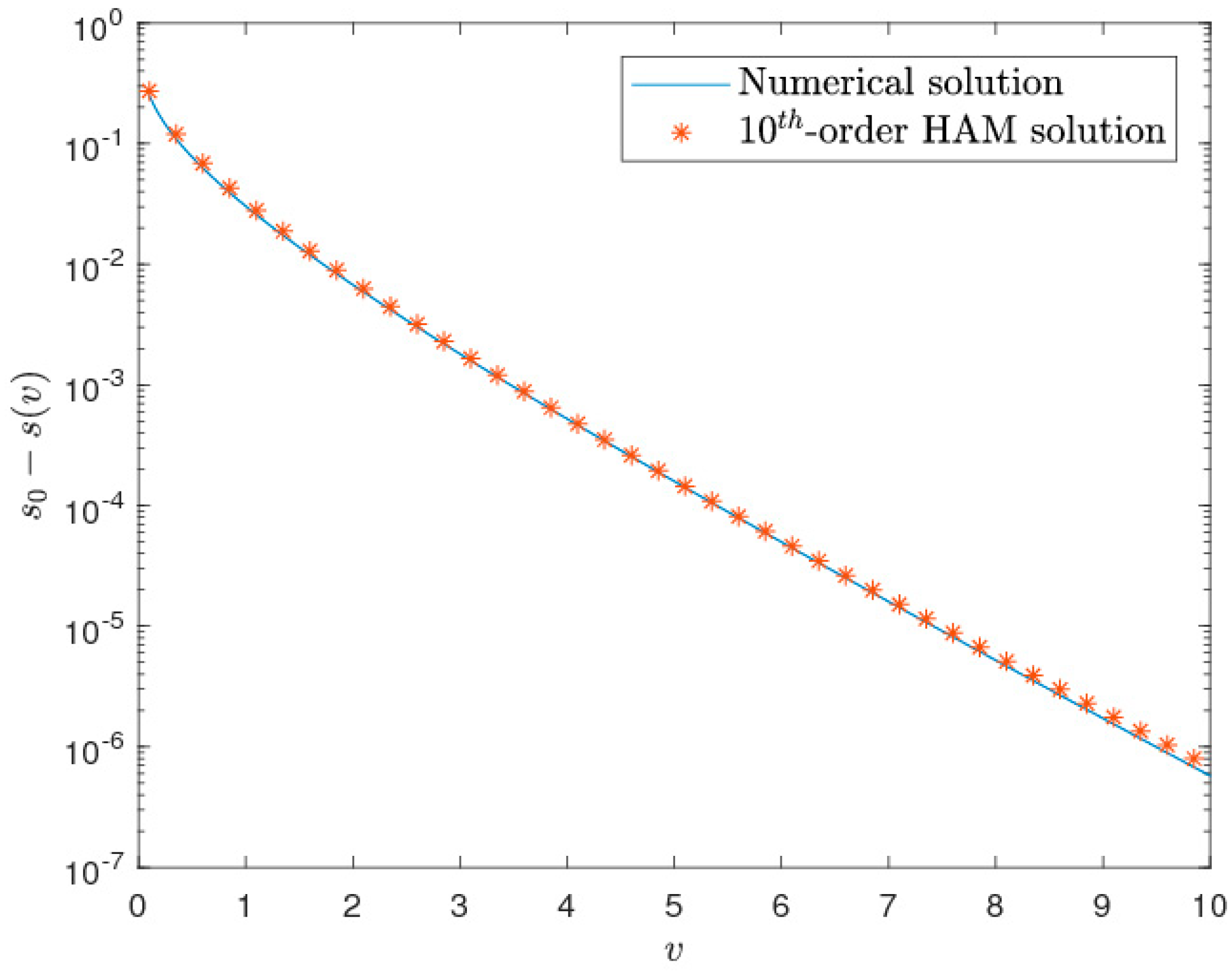
4.2. Numerical Convergence and Validation of the HAM-Based Solution
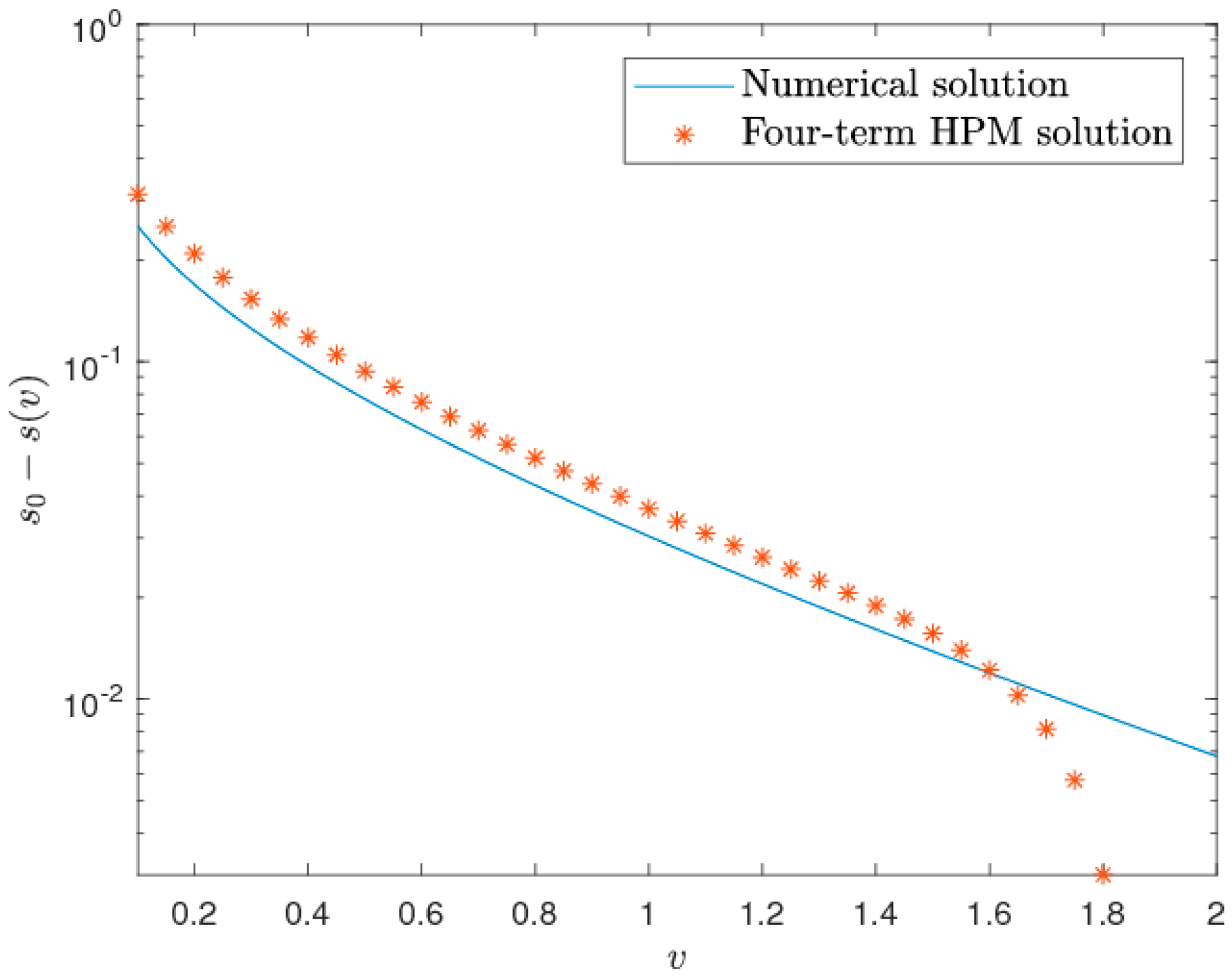
4.3. Validation of HPM-Based Solution
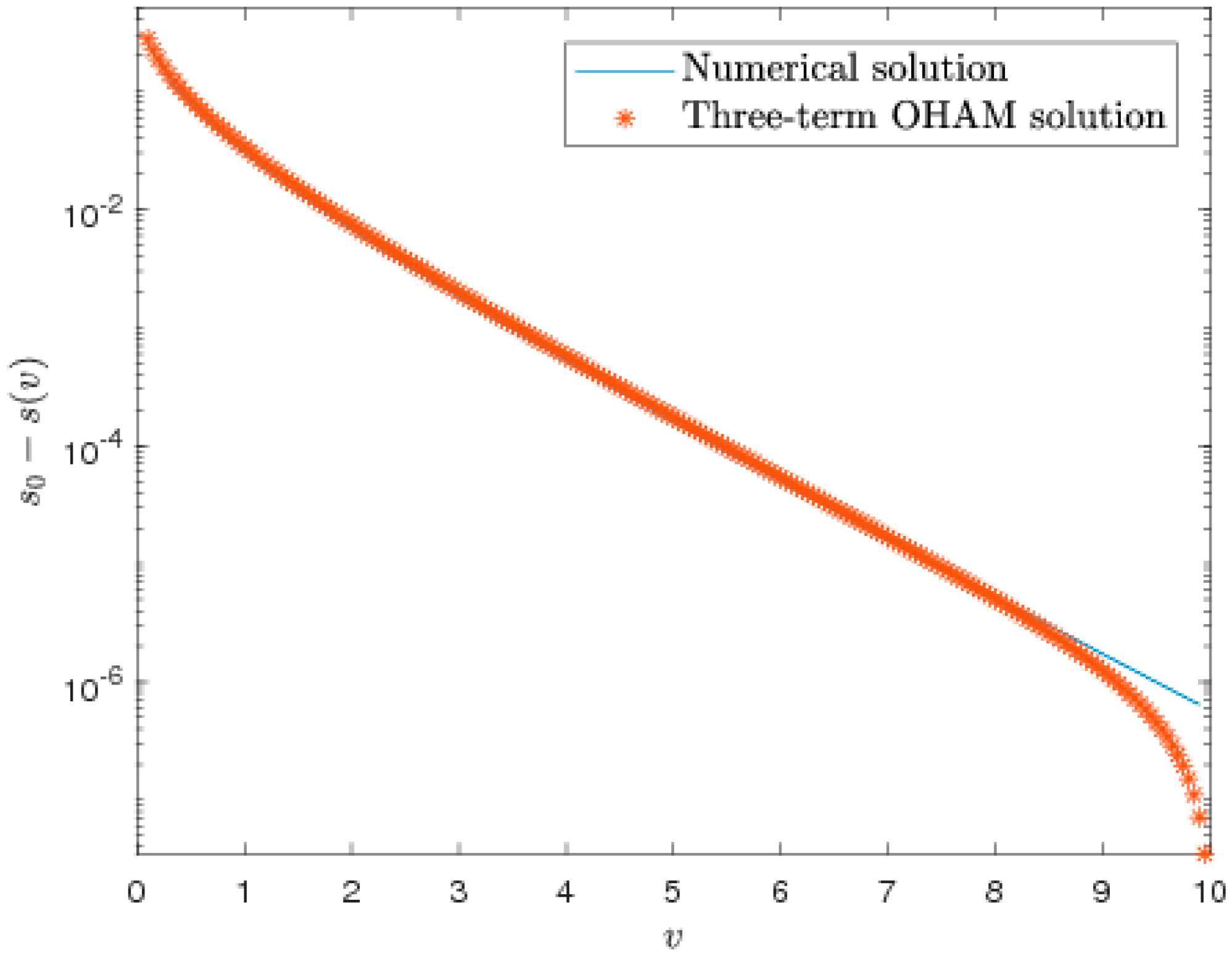
4.4. Validation of OHAM-Based Solution
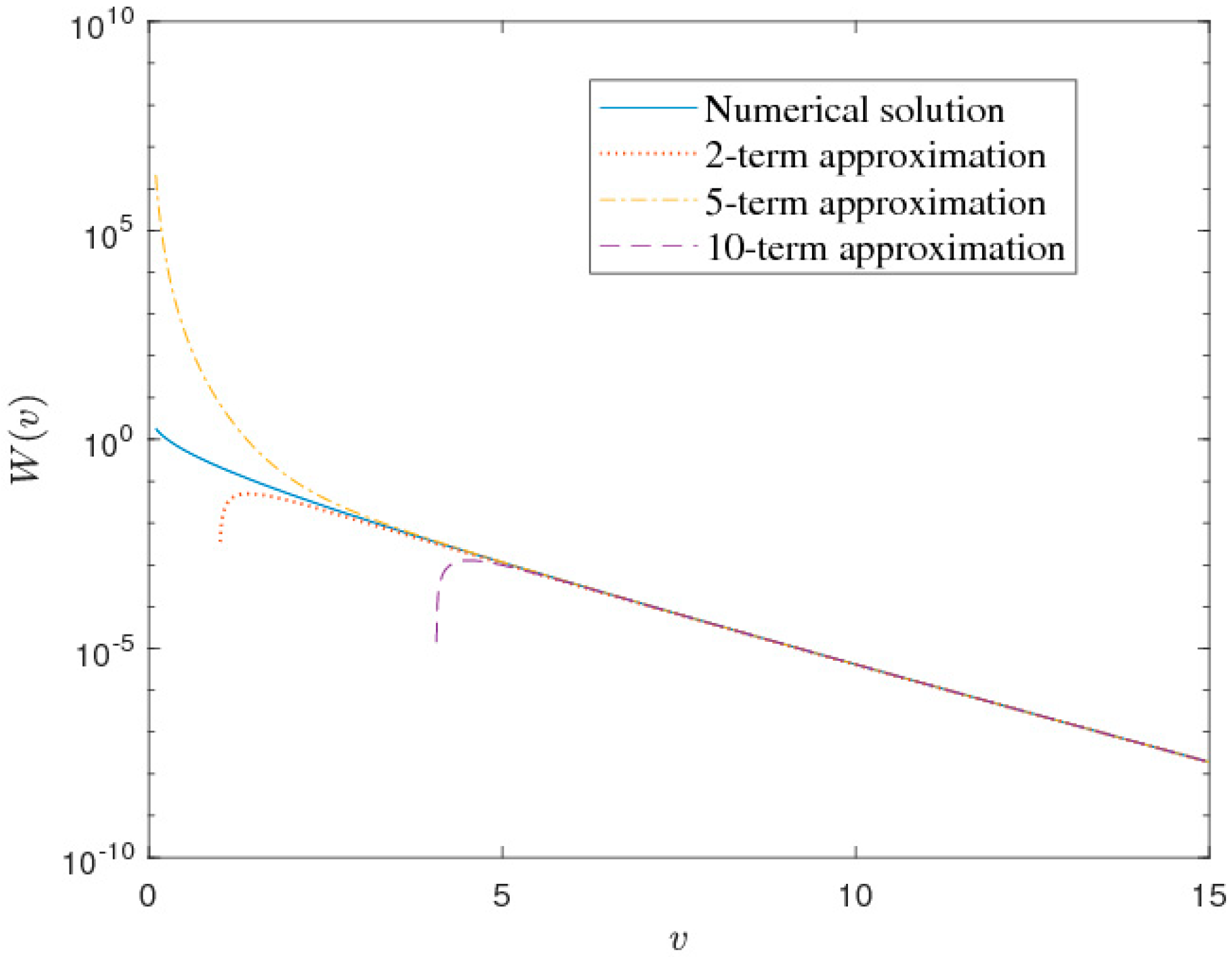
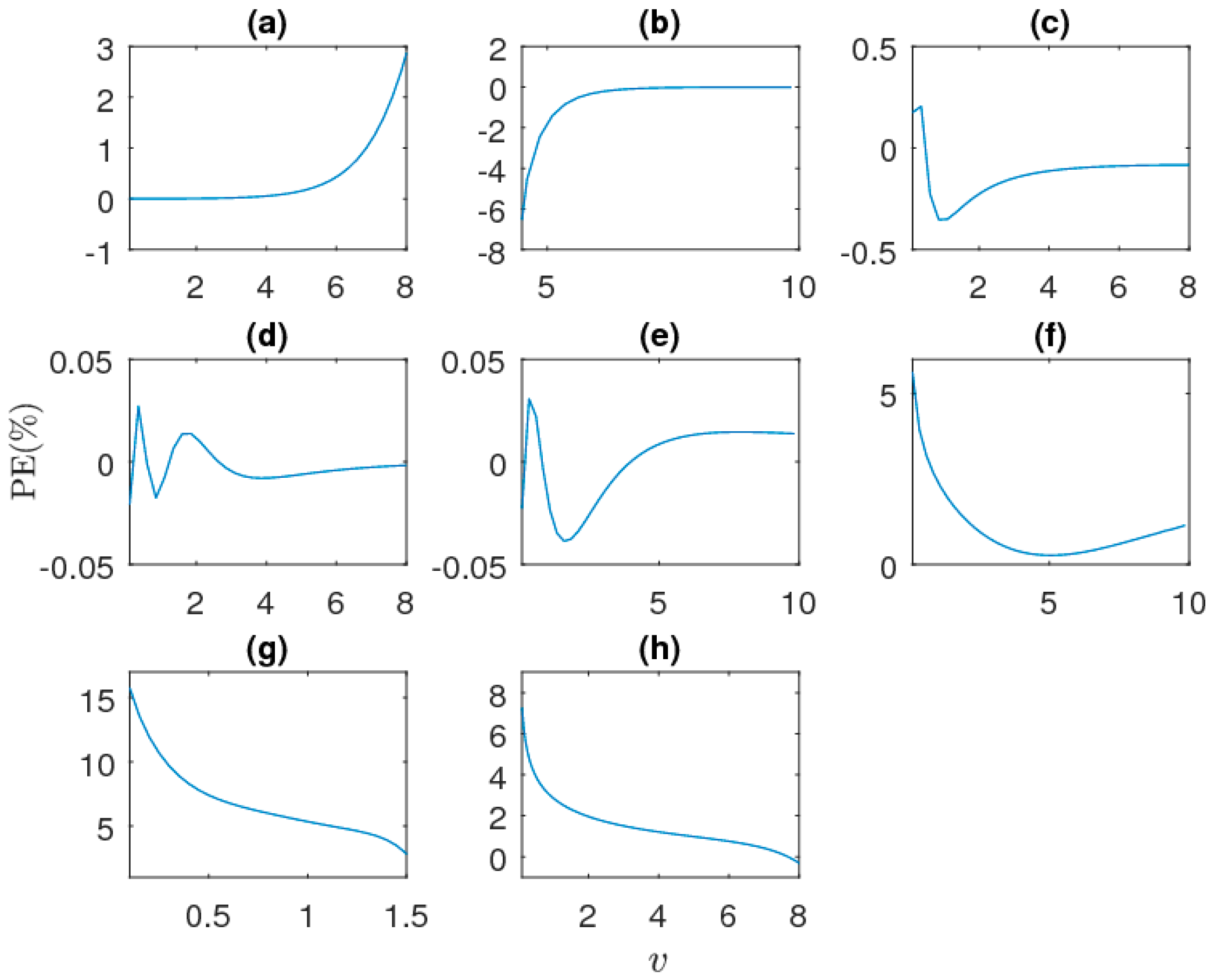
4.5. Comparison between Different Approximations
5. Concluding Remarks
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. Convergence Theorems
Appendix A.1. Convergence Theorem of HAM-Based Solution
Appendix A.2. Convergence Theorem of OHAM-Based Solution
References
- Theis, C.V. The relation between the lowering of the piezometric surface and the rate and duration of discharge of a well using ground-water storage. Eos Trans. Am. Geophys. Union 1935, 16, 519–524. [Google Scholar] [CrossRef]
- Chiccoli, C.; Lorenzutta, S.; Maino, G. Recent results for generalized exponential integrals. Comput. Math. Appl. 1990, 19, 21–29. [Google Scholar] [CrossRef]
- Stankiewicz, A. Tables of the integro-exponential functions. Acta Astron. 1968, 18, 289. [Google Scholar]
- Jacob, C.E. On the flow of water in an elastic artesian aquifer. Eos Trans. Am. Geophys. Union 1940, 21, 574–586. [Google Scholar] [CrossRef]
- Masina, E. Useful review on the Exponential-Integral special function. arXiv 2019, arXiv:1907.12373. [Google Scholar]
- Allen, E.E. Analytical approximations. Math. Comput. 1954, 8, 240–241. [Google Scholar] [CrossRef]
- Cody, W.J.; Thacher, H.C. Rational Chebyshev approximations for the exponential integral E1 (x). Math. Comput. 1968, 22, 641–649. [Google Scholar]
- Abramowitz, M.; Stegun, I.A. (Eds.) Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables; US Government Printing Office: Washington, DC, USA, 1970; Volume 55.
- Srivastava, R. Implications of using approximate expressions for well function. J. Irrig. Drain. Eng. 1995, 121, 459–462. [Google Scholar] [CrossRef]
- Srivastava, R.; Guzman-Guzman, A. Practical approximations of the well function. Groundwater 1998, 36, 844–848. [Google Scholar] [CrossRef]
- Tseng, P.H.; Lee, T.C. Numerical evaluation of exponential integral: Theis well function approximation. J. Hydrol. 1998, 205, 38–51. [Google Scholar] [CrossRef]
- Swamee, P.K.; Ojha, C.S.P. Pump test analysis of confined aquifer. J. Irrig. Drain. Eng. 1990, 116, 99–106. [Google Scholar] [CrossRef]
- Barry, D.A.; Parlange, J.Y.; Li, L. Approximation for the exponential integral (Theis well function). J. Hydrol. 2000, 227, 287–291. [Google Scholar] [CrossRef]
- Vatankhah, A.R. Full-Range Solution for the Theis Well Function. J. Hydrol. Eng. 2014, 19, 649–653. [Google Scholar] [CrossRef]
- Liao, S.J. The Proposed Homotopy Analysis Technique for the Solution of Nonlinear Problems. Ph.D. Thesis, Shanghai Jiao Tong University, Shanghai, China, 1992. [Google Scholar]
- Liao, S. Beyond Perturbation: Introduction to the Homotopy Analysis Method; Chapman and Hall/CRC: Boca Raton, FL, USA, 2003. [Google Scholar]
- Liao, S. Homotopy Analysis Method in Nonlinear Differential Equations; Higher Education Press: Beijing, China, 2012; pp. 153–165. [Google Scholar]
- He, J.H. Homotopy perturbation technique. Comput. Methods Appl. Mech. Eng. 1999, 178, 257–262. [Google Scholar] [CrossRef]
- Marinca, V.; Herişanu, N. Application of optimal homotopy asymptotic method for solving nonlinear equations arising in heat transfer. Int. Commun. Heat Mass Transf. 2008, 35, 710–715. [Google Scholar] [CrossRef]
- Marinca, V.; Herisanu, N. Optimal homotopy asymptotic method. In The Optimal Homotopy Asymptotic Method; Springer: Cham, Switzerland, 2015; pp. 9–22. [Google Scholar]
- Walton, W.C. Groundwater resource evaluation. In McGraw-Hill Series in Water Resources and Environmental Engineering (USA) Eng.; McGraw-Hill: New York, NY, USA, 1970. [Google Scholar]
- Berndt, B.C. Ramanujan’s Notebook Part IV; Springer: New York, NY, USA, 1994. [Google Scholar]
- Vajravelu, K.; Van Gorder, R. Nonlinear Flow Phenomena and Homotopy Analysis; Springer: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- Shampine, L.F. Vectorized adaptive quadrature in MATLAB. J. Comput. Appl. Math. 2008, 211, 131–140. [Google Scholar] [CrossRef]
- Zhao, Y.; Liao, S. User Guide to BVPh 2.0; School of Naval Architecture, Ocean and Civil Engineering: Shanghai, China, 2002; Volume 40. [Google Scholar]



| Computational Time (s) | ||
|---|---|---|
| 2 | 7.15 10−4 | 0.212 |
| 4 | 5.23 10−5 | 1.135 |
| 6 | 1.98 10−5 | 2.481 |
| 8 | 1.01 10−5 | 5.074 |
| 10 | 8.86 10−6 | 6.843 |
| 12 | 6.23 10−6 | 10.149 |
| Numerical Solution | HAM-Based Approximation | |||
|---|---|---|---|---|
| 4th Order | 7th Order | 10th Order | ||
| 0.1 | 2.523 10−1 | 3.009 10−1 | 2.821 10−1 | 2.726 10−1 |
| 1 | 3.036 10−2 | 3.995 10−2 | 3.548 10−2 | 3.305 10−2 |
| 2 | 6.768 10−3 | 9.302 10−3 | 8.011 10−3 | 7.228 10−3 |
| 3 | 1.806 10−3 | 2.560 10−3 | 2.146 10−3 | 1.886 10−3 |
| 4 | 5.230 10−4 | 7.603 10−4 | 6.213 10−4 | 5.377 10−4 |
| 5 | 1.589 10−4 | 2.359 10−4 | 1.881 10−4 | 1.627 10−4 |
| 6 | 4.983 10−5 | 7.530 10−5 | 5.870 10−5 | 5.150 10−5 |
| 7 | 1.598 10−5 | 2.453 10−5 | 1.871 10−5 | 1.689 10−5 |
| 8 | 5.213 10−6 | 8.111 10−6 | 6.066 10−6 | 5.688 10−6 |
| 9 | 1.723 10−6 | 2.713 10−6 | 1.993 10−6 | 1.955 10−6 |
| 10 | 5.753 10−7 | 9.160 10−7 | 6.617 10−7 | 6.814 10−7 |
| Numerical Solution | Four Terms of the HPM-Based Approximation | |
|---|---|---|
| 0.1 | 2.523 10−1 | 3.135 10−1 |
| 0.3 | 1.253 10−1 | 1.533 10−1 |
| 0.5 | 7.747 10−2 | 9.362 10−2 |
| 0.7 | 5.173 10−2 | 6.246 10−2 |
| 0.9 | 3.601 10−2 | 4.346 10−2 |
| 1.1 | 2.574 10−2 | 3.097 10−2 |
| 1.3 | 1.875 10−2 | 2.237 10−2 |
| 1.5 | 1.384 10−2 | 1.563 10−2 |
| 1.7 | 1.033 10−2 | 8.139 10−3 |
| 2.0 | 6.768 10−3 | 1.298 10−2 |
| Numerical Solution | Three Terms of OHAM-Based Approximation | |
|---|---|---|
| 0.1 | 2.523 10−1 | 2.466 10−1 |
| 1 | 3.036 10−2 | 3.143 10−2 |
| 2 | 6.768 10−3 | 6.539 10−3 |
| 3 | 1.806 10−3 | 2.112 10−3 |
| 4 | 5.230 10−4 | 8.502 10−4 |
| 5 | 1.589 10−4 | 3.066 10−4 |
| 6 | 4.983 10−5 | 1.000 10−4 |
| 7 | 1.598 10−5 | 3.013 10−5 |
| 8 | 5.213 10−6 | 8.461 10−6 |
| 9 | 1.723 10−6 | 2.283 10−6 |
| 10 | 5.753 10−7 | 5.978 10−7 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kumbhakar, M.; Singh, V.P. Analytical Approximations of Well Function by Solving the Governing Differential Equation Representing Unsteady Groundwater Flow in a Confined Aquifer. Mathematics 2023, 11, 1652. https://doi.org/10.3390/math11071652
Kumbhakar M, Singh VP. Analytical Approximations of Well Function by Solving the Governing Differential Equation Representing Unsteady Groundwater Flow in a Confined Aquifer. Mathematics. 2023; 11(7):1652. https://doi.org/10.3390/math11071652
Chicago/Turabian StyleKumbhakar, Manotosh, and Vijay P. Singh. 2023. "Analytical Approximations of Well Function by Solving the Governing Differential Equation Representing Unsteady Groundwater Flow in a Confined Aquifer" Mathematics 11, no. 7: 1652. https://doi.org/10.3390/math11071652
APA StyleKumbhakar, M., & Singh, V. P. (2023). Analytical Approximations of Well Function by Solving the Governing Differential Equation Representing Unsteady Groundwater Flow in a Confined Aquifer. Mathematics, 11(7), 1652. https://doi.org/10.3390/math11071652







