Notes on the Localization of Generalized Hexagonal Cellular Networks
Abstract
1. Introduction
2. Certain Important Results
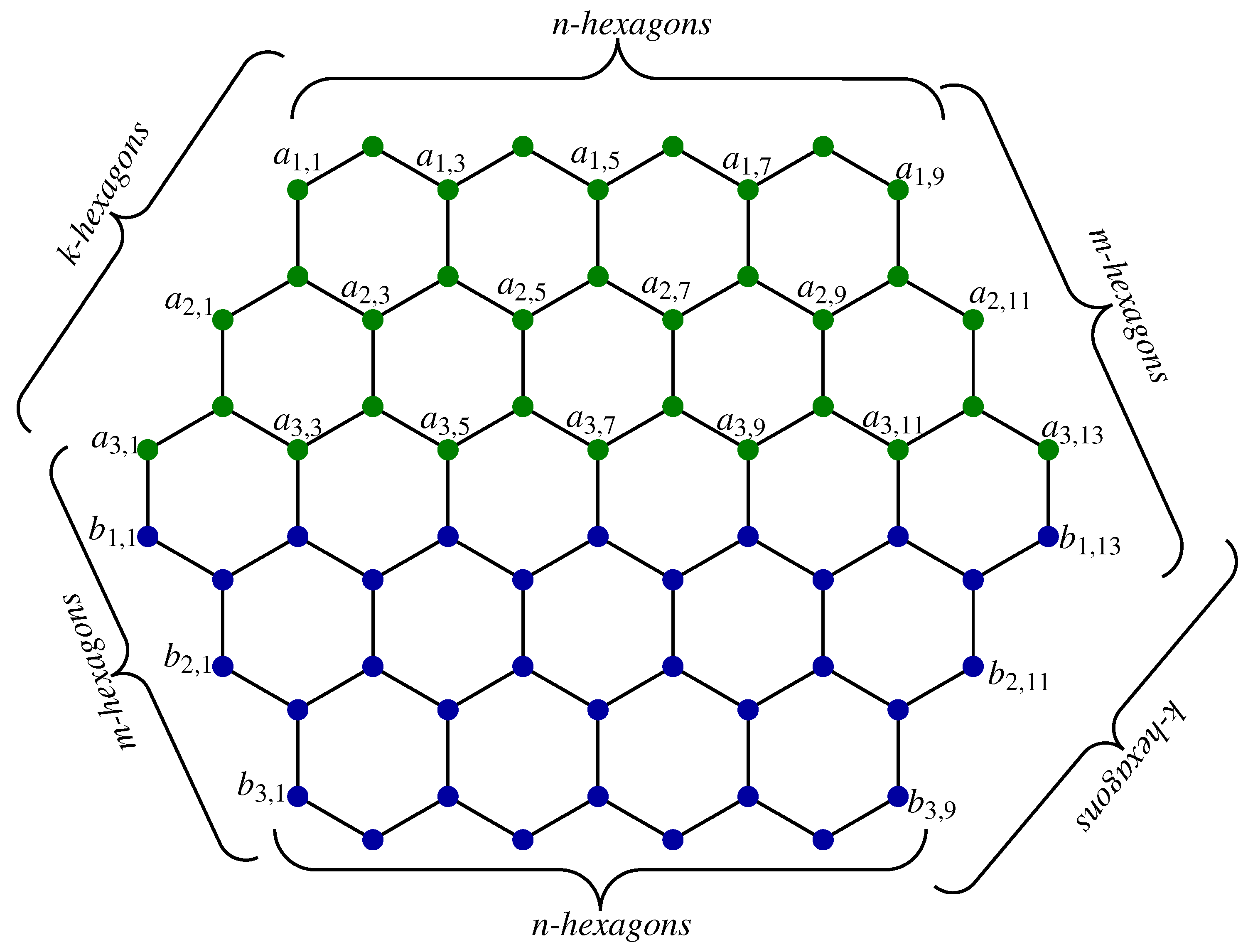
3. Construction
4. Conclusions and Comparison with the Existing Research Work
Author Contributions
Funding
Conflicts of Interest
References
- Nadeem, M.F.; Azeem, M.; Khalil, A. The locating number of hexagonal Möbius ladder network. J. Appl. Math. Comput. 2020, 66, 149–165. [Google Scholar] [CrossRef]
- Hauptmann, M.; Schmied, R.; Viehmann, C. Approximation complexity of metric dimension problem. J. Discret. Algorithms 2012, 14, 214–222. [Google Scholar] [CrossRef][Green Version]
- Lewis, H.; Garey, M.; Johnson, D. Computers and intractability. A guide to the theory of NP-completeness. J. Symb. Log. 1983, 48, 498–500. [Google Scholar] [CrossRef]
- Johnson, M.A. Browsable Structure-Activity Datasets, Advances in Molecular Similarity; JAI Press: Greenwich, CT, USA, 1998; pp. 153–170. [Google Scholar]
- Johnson, M.A. Structure-activity maps for visualizing the graph variables arising in drug design. J. Biopharm. Stat. 1993, 3, 203–236. [Google Scholar] [CrossRef] [PubMed]
- Slater, P. Leaves of trees. In Proceeding of the 6th Southeastern Conference on Combinatorics, Graph Theory, and Computing, Congressus Numerantium, Boca Raton, FL, USA, 17–20 February 1975; Volume 14, pp. 549–559. [Google Scholar]
- Harary, F.; Melter, R.A. On the metric dimension of a graph. Ars Comb. 1976, 2, 191–195. [Google Scholar]
- Chartrand, G.; Salehi, E.; Zhang, P. The partition dimension of graph. Aequationes Math. 2000, 59, 45–54. [Google Scholar] [CrossRef]
- Chartrand, G.; Eroh, L.; Johnson, M.A.O.; Ortrud, R. Resolvability in graphs and the metric dimension of a graph. Discret. Appl. Math. 2000, 105, 99–113. [Google Scholar] [CrossRef]
- Hernando, C.; Mora, M.; Slater, P.; Wood, D. Fault-tolerant metric dimension of graphs. Convexity Discret. Struct. 2008, 5, 81–85. [Google Scholar]
- Shang, Y. Local natural connectivity in complex networks. Chin. Phys. Lett. 2011, 28, 068903. [Google Scholar] [CrossRef]
- Shang, Y. Localized recovery of complex networks against failure. Sci. Rep. 2016, 6, 30521. [Google Scholar]
- Nadeem, M.F.; Hassan, M.; Azeem, M.; Khan, S.U.D.; Shaik, M.R.; Sharaf, M.A.F.; Abdelgawad, A.; Awwad, E.M. Application of Resolvability Technique to Investigate the Different Polyphenyl Structures for Polymer Industry. J. Chem. 2021, 2021, 6633227. [Google Scholar] [CrossRef]
- Ahmad, A.; Koam, A.N.; Siddiqui, M.; Azeem, M. Resolvability of the starphene structure and applications in electronics. Ain Shams Eng. J. 2021, 13, 101587. [Google Scholar] [CrossRef]
- Khuller, S.; Raghavachari, B.; Rosenfeld, A. Landmarks in graphs. Discret. Appl. Math. 1996, 70, 217–229. [Google Scholar] [CrossRef]
- Sebö, A.; Tannier, E. On metric generators of graphs. Math. Oper. Res. 2004, 29, 383–393. [Google Scholar] [CrossRef]
- Ahmad, A.; Bača, M.; Sultan, S. Computing the metric dimension of Kayak Paddles graph and Cycles with chord. Proyecc. J. Math. 2020, 39, 287–300. [Google Scholar] [CrossRef]
- Imran, S.; Siddiqui, M.K.; Hussain, M. Computing the upper bounds for the metric dimension Of cellulose network. Appl. Math. E-Notes 2019, 19, 585–605. [Google Scholar]
- Koam, A.N.A.; Ahmad, A.; Abdelhag, M.E.; Azeem, M. Metric and Fault-Tolerant Metric Dimension of Hollow Coronoid. IEEE Access 2021, 9, 81527–81534. [Google Scholar] [CrossRef]
- Azeem, M.; Nadeem, M.F. Metric-based resolvability of polycyclic aromatic hydrocarbons. Eur. Phys. J. Plus 2021, 136, 395. [Google Scholar] [CrossRef]
- Ahmad, A.; Baca, M.; Sultan, S. On Metric Dimension and Minimal doubly resolving sets of Harary graph. Acta Math. Univ. Comen. 2020, 89, 123–129. [Google Scholar]
- Imran, M.; Siddiqui, M.K.; Naeem, R. On the Metric Dimension of Generalized Petersen Multigraphs. IEEE Access 2018, 6, 74328–74338. [Google Scholar] [CrossRef]
- Ahmad, A.; Imran, M.; Al-Mushayt, O.; Bokhary, S.A.H. On the metric dimension of barcycentric subdivision of Cayley graphs Cay(Zn⊕Zm). Miskolc Math. Notes 2015, 16, 637–646. [Google Scholar]
- Azeem, M.; Imran, M.; Nadeem, M.F. Sharp bounds on partition dimension of hexagonal Möbius ladder. J. King Saud Univ.-Sci. 2022, 34, 101779. [Google Scholar] [CrossRef]
- Bukhari, S.; Jamil, M.K.; Azeem, M.; Swaray, S. Patched Network and its Vertex-Edge Metric-Based Dimension. IEEE Access 2023, 11, 4478–4485. [Google Scholar] [CrossRef]
- Koam, A.N.A.; Ahmad, A. Barycentric subdivision of Cayley graphs with constant edge metric dimension. IEEE Access 2020, 8, 80624–80628. [Google Scholar] [CrossRef]
- Ahmad, Z.; Chaudhary, M.A.; Baig, A.Q.; Zahid, M.A. Fault-tolerant metric dimension of P(n, 2) graph. J. Discret. Math. Sci. Cryptogr. 2021, 24, 647–656. [Google Scholar] [CrossRef]
- Vietz, D.; Wanke, E. The Fault-Tolerant Metric Dimension of Cographs. In Fundamentals of Computation Theory; Springer International Publishing: Berlin/Heidelberg, Germany, 2019; pp. 350–364. [Google Scholar] [CrossRef]
- Sharma, S.K.; Bhat, V.K. Fault-tolerant metric dimension of two-fold heptagonal-nonagonal circular ladder. Discret. Math. Algorithms Appl. 2021, 14, 2150132. [Google Scholar] [CrossRef]
- Saha, L.; Lama, R.; Tiwary, K.; Das, K.; Shang, Y. Fault-tolerant metric dimension of circulant graphs. Mathematics 2022, 10, 124. [Google Scholar] [CrossRef]
- Nadeem, M.F.; Ali, W.; Siddiqui, H.M.A. Locating Number of Biswapped Networks. Int. J. Found. Comput. Sci. 2022, 33, 667–690. [Google Scholar] [CrossRef]
- Deng, W.; Karaliopoulos, M.; Mühlbauer, W.; Zhu, P.; Lu, X.; Plattner, B. k-Fault tolerance of the Internet AS graph. Comput. Netw. 2011, 55, 2492–2503. [Google Scholar] [CrossRef]
- Imran, M.; Ahmad, A.; Azeem, M.; Elahi, K. Metric-Based Resolvability of Quartz Structure. Comput. Mater. Contin. 2021, 71, 2053–2071. [Google Scholar]
- Raza, H.; Hayat, S.; Pan, X.F. On the fault-tolerant metric dimension of certain interconnection networks. J. Appl. Math. Comput. 2019, 60, 517–535. [Google Scholar] [CrossRef]
- Raza, H.; Hayat, S.; Pan, X.F. On the fault-tolerant metric dimension of convex polytopes. Appl. Math. Comput. 2018, 339, 172–185. [Google Scholar] [CrossRef]
- Donald, V.H.M. Advanced Mobile Phone Service: The Cellular Concept. Bell Syst. Tech. J. 1979, 58, 15–41. [Google Scholar] [CrossRef]
- Yang, X.; Fapojuwo, A.O. Performance analysis of hexagonal cellular networks in fading channels. Wirel. Commun. Mob. Comput. 2015, 16, 850–867. [Google Scholar] [CrossRef]
- Garg, V. Wireless Communications and Networking; Elsevier Inc.: Boston, MA, USA, 2007; pp. 47–147. [Google Scholar]
- Jacks, E.; Schumacher, G. NGMN Alliance Performance Evaluation Methodology; NGMN Technical Working Group Steering Committee (TWG-SC): Frankfurt, Germany, 2007; pp. 1–36. [Google Scholar]
- 3GPP TR 36.814 V9.0.0. Further Advancements for E-UTRA Physical Layer Aspect. 2010. Available online: https://www.scirp.org/(S(lz5mqp453edsnp55rrgjct55))/reference/ReferencesPapers.aspx?ReferenceID=996975 (accessed on 3 January 2023).
- Saquib, N.; Hossain, E.; Le, L.B.; Kim, D.I. Interference management in OFDMA femtocell networks: Issues and approaches. IEEE Wirel. Commun. 2012, 19, 86–95. [Google Scholar] [CrossRef]
- Tang, J.; Liu, G.; Pan, Q. A Review on Representative Swarm Intelligence Algorithms for Solving Optimization Problems: Applications and Trends. IEEE/CAA J. Autom. Sin. 2021, 8, 1627–1643. [Google Scholar] [CrossRef]
- Gao, Y.; Wang, G.; Jafar, S.A. Topological Interference Management for Hexagonal Cellular Networks. IEEE Trans. Wirel. Commun. 2015, 14, 2368–2376. [Google Scholar] [CrossRef]
- Alqahtani, Y.; Jamil, M.K.; Alshehri, H.; Ahmad, A.; Azeem, M. Vertex metric resolvability of COVID antiviral drug structures. J. Intell. Fuzzy Syst. 2022, 1–12. [Google Scholar] [CrossRef]
- Melter, R.A.; Tomescu, I. Metric bases in digital geometry. Comput. Vis. Graph. Image Process. 1984, 25, 113–121. [Google Scholar] [CrossRef]
- Chaudhry, M.A.; Javaid, I.; Salman, M. Fault-Tolerant metric and partition dimension of graphs. Utliltas Math. 2010, 83, 187–199. [Google Scholar]
- Abd-El-Barr, M. Topological network design: A survey. J. Netw. Comput. 2009, 32, 501–509. [Google Scholar] [CrossRef]
- Yu, X.; Zhou, L.; Li, X. A novel hybrid localization scheme for deep mine based on wheel graph and chicken swarm optimization. Comput. Netw. 2019, 154, 73–78. [Google Scholar] [CrossRef]
- Aksu, H.; Aksoy, D.; Korpeoglu, I. A study of localization metrics: Evaluation of position errors in wireless sensor networks. Comput. Netw. 2011, 55, 3562–3577. [Google Scholar] [CrossRef]
- Ward, C.B.; Wiegand, N.M. Complexity results on labeled shortest path problems from wireless routing metrics. Comput. Netw. 2010, 54, 208–217. [Google Scholar] [CrossRef]
- Sun, Y.; Yu, X.; Bie, R.; Song, H. Discovering time-dependent shortest path on traffic graph for drivers towards green driving. J. Netw. Comput. 2017, 83, 204–212. [Google Scholar] [CrossRef]
- Chou, C.P.; Li, Y.; Witek, H.A. Zhang–Zhang Polynomials of Various Classes of Benzenoid Systems. MATCH Commun. Math. Comput. Chem. 2012, 68, 31–64. [Google Scholar]
- Manuel, P.; Bharati, R.; Rajasingh, I.; M, C.M. On minimum metric dimension of honeycomb networks. J. Discret. Algorithms 2008, 6, 20–27. [Google Scholar] [CrossRef]
- Shreedhar, K.; Sooryanarayana, B.; Hegde, C.; Kumar, V. Metric dimension of a hexagonal cellular network. Int. J. Math. Sci. Eng. Appl. 2010, 4, 133–148. [Google Scholar]
- Huang, Q.; Khalil, A.; Ali, D.A.; Ahmad, A.; Luo, R.; Azeem, M. Breast cancer chemical structures and their partition resolvability. Math. Biosci. Eng. 2022, 20, 3838–3853. [Google Scholar] [CrossRef]
| 0 | 6 | 1 | 5 | ||
| 2 | 4 | 3 | 3 | ||
| 4 | 2 | 5 | 1 | ||
| 6 | 0 | 2 | 8 | ||
| 2 | 7 | 2 | 6 | ||
| 3 | 5 | 4 | 4 | ||
| 5 | 4 | 6 | 2 | ||
| 7 | 1 | 8 | 2 |
| 3 | 9 | 4 | 8 | ||
| 3 | 7 | 4 | 6 | ||
| 5 | 5 | 6 | 4 | ||
| 7 | 3 | 8 | 4 | ||
| 9 | 3 | 5 | 9 | ||
| 6 | 8 | 5 | 7 | ||
| 6 | 6 | 7 | 5 | ||
| 8 | 6 | 9 | 5 |
| 1 | 5 | 0 | 6 | ||
| 1 | 7 | 2 | 8 | ||
| 3 | 9 | 3 | 3 | ||
| 2 | 4 | 3 | 5 | ||
| 2 | 6 | 3 | 7 | ||
| 4 | 8 | 5 | 9 |
| 5 | 1 | 4 | 2 | ||
| 5 | 3 | 4 | 4 | ||
| 5 | 5 | 4 | 6 | ||
| 5 | 7 | 6 | 8 | ||
| 6 | 0 | 7 | 1 | ||
| 6 | 2 | 7 | 3 | ||
| 6 | 4 | 7 | 5 | ||
| 6 | 6 | 8 | 2 | ||
| 9 | 3 | 8 | 4 | ||
| 9 | 5 | 8 | 6 |
| 0 | 11 | 1 | 10 | ||
| 2 | 9 | 3 | 10 | ||
| 4 | 9 | 5 | 10 | ||
| 6 | 9 | 2 | 10 | ||
| 1 | 9 | 2 | 8 | ||
| 3 | 8 | 4 | 7 | ||
| 5 | 8 | 6 | 7 | ||
| 7 | 8 | 8 | 7 | ||
| 4 | 10 | 3 | 9 | ||
| 4 | 8 | 3 | 7 | ||
| 4 | 8 | 5 | 6 | ||
| 6 | 5 | 7 | 6 | ||
| 8 | 5 | 9 | 6 | ||
| 10 | 5 |
| 5 | 9 | 6 | 8 | ||
| 5 | 7 | 6 | 6 | ||
| 5 | 5 | 6 | 4 | ||
| 7 | 4 | 8 | 3 | ||
| 9 | 4 | 10 | 3 | ||
| 11 | 4 | 7 | 7 | ||
| 8 | 6 | 7 | 5 | ||
| 8 | 4 | 7 | 3 | ||
| 8 | 2 | 9 | 2 | ||
| 10 | 1 | 11 | 2 | ||
| 9 | 5 | 10 | 4 | ||
| 9 | 3 | 10 | 2 | ||
| 9 | 1 | 11 | 1 | ||
| 10 | 0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Azeem, M.; Jamil, M.K.; Shang, Y. Notes on the Localization of Generalized Hexagonal Cellular Networks. Mathematics 2023, 11, 844. https://doi.org/10.3390/math11040844
Azeem M, Jamil MK, Shang Y. Notes on the Localization of Generalized Hexagonal Cellular Networks. Mathematics. 2023; 11(4):844. https://doi.org/10.3390/math11040844
Chicago/Turabian StyleAzeem, Muhammad, Muhammad Kamran Jamil, and Yilun Shang. 2023. "Notes on the Localization of Generalized Hexagonal Cellular Networks" Mathematics 11, no. 4: 844. https://doi.org/10.3390/math11040844
APA StyleAzeem, M., Jamil, M. K., & Shang, Y. (2023). Notes on the Localization of Generalized Hexagonal Cellular Networks. Mathematics, 11(4), 844. https://doi.org/10.3390/math11040844








