New (3+1)-Dimensional Kadomtsev–Petviashvili–Sawada– Kotera–Ramani Equation: Multiple-Soliton and Lump Solutions
Abstract
:1. Introduction
2. Formulation of a New (3+1)-Dimensional KP–SK–R Equation
3. Painlevé Analysis to a Related Equation
Painlevé Analysis
- (i)
- Leading order behavior and coefficients:
- (ii)
- Resonant points:
- (i)
- The principal branch: ;
- (ii)
- The secondary branch: .
- (iii)
- Verifying compatibility conditions
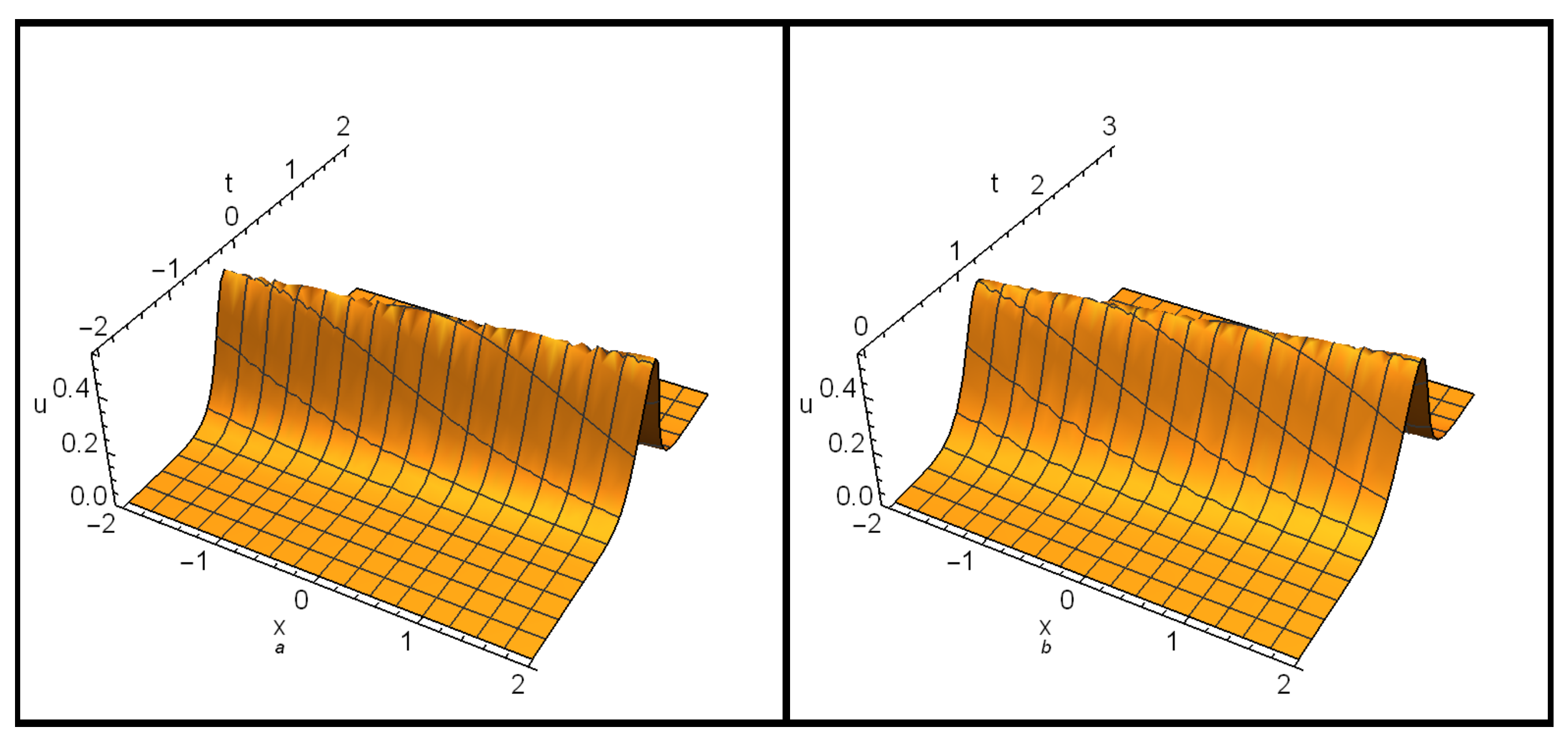
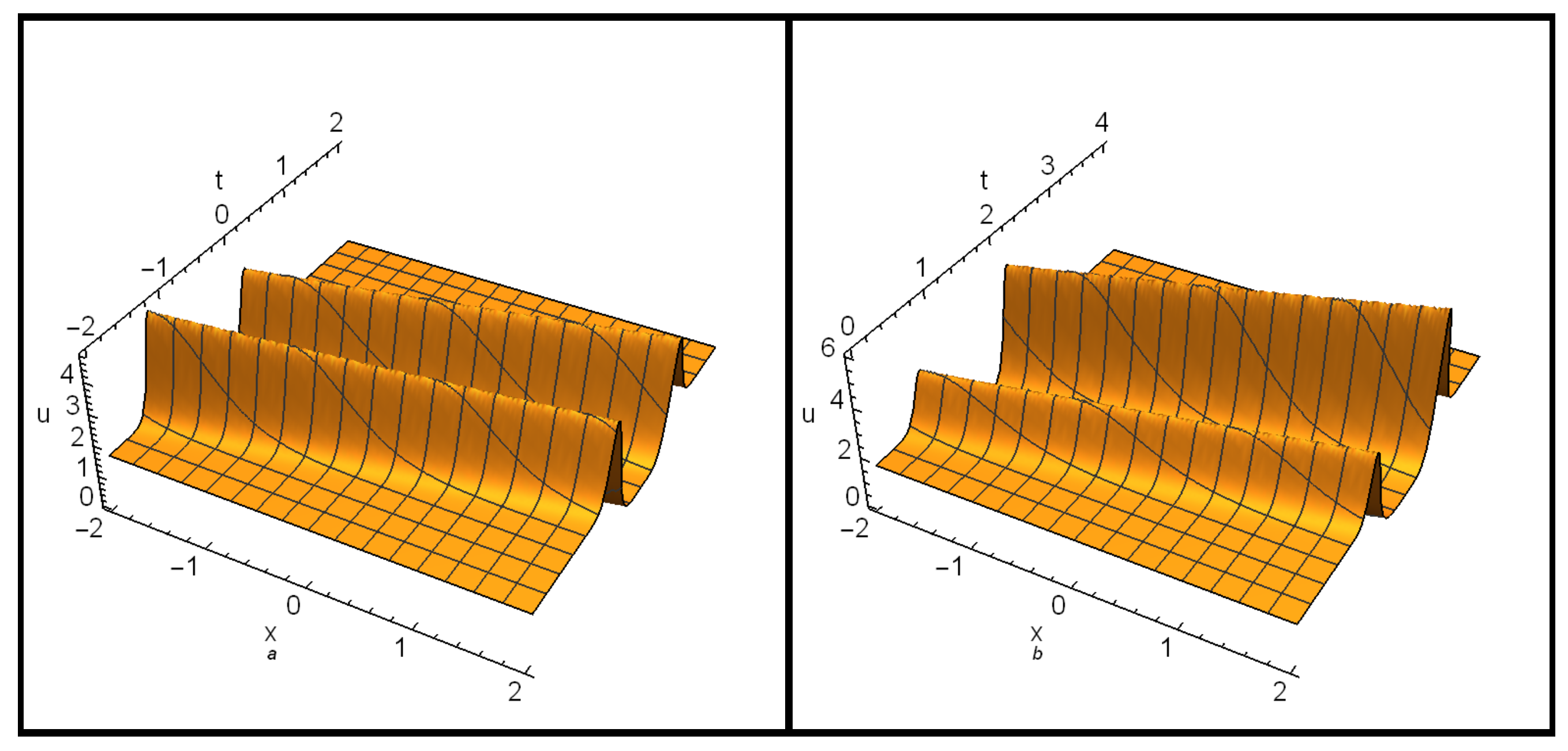
4. Multiple-Soliton Solutions
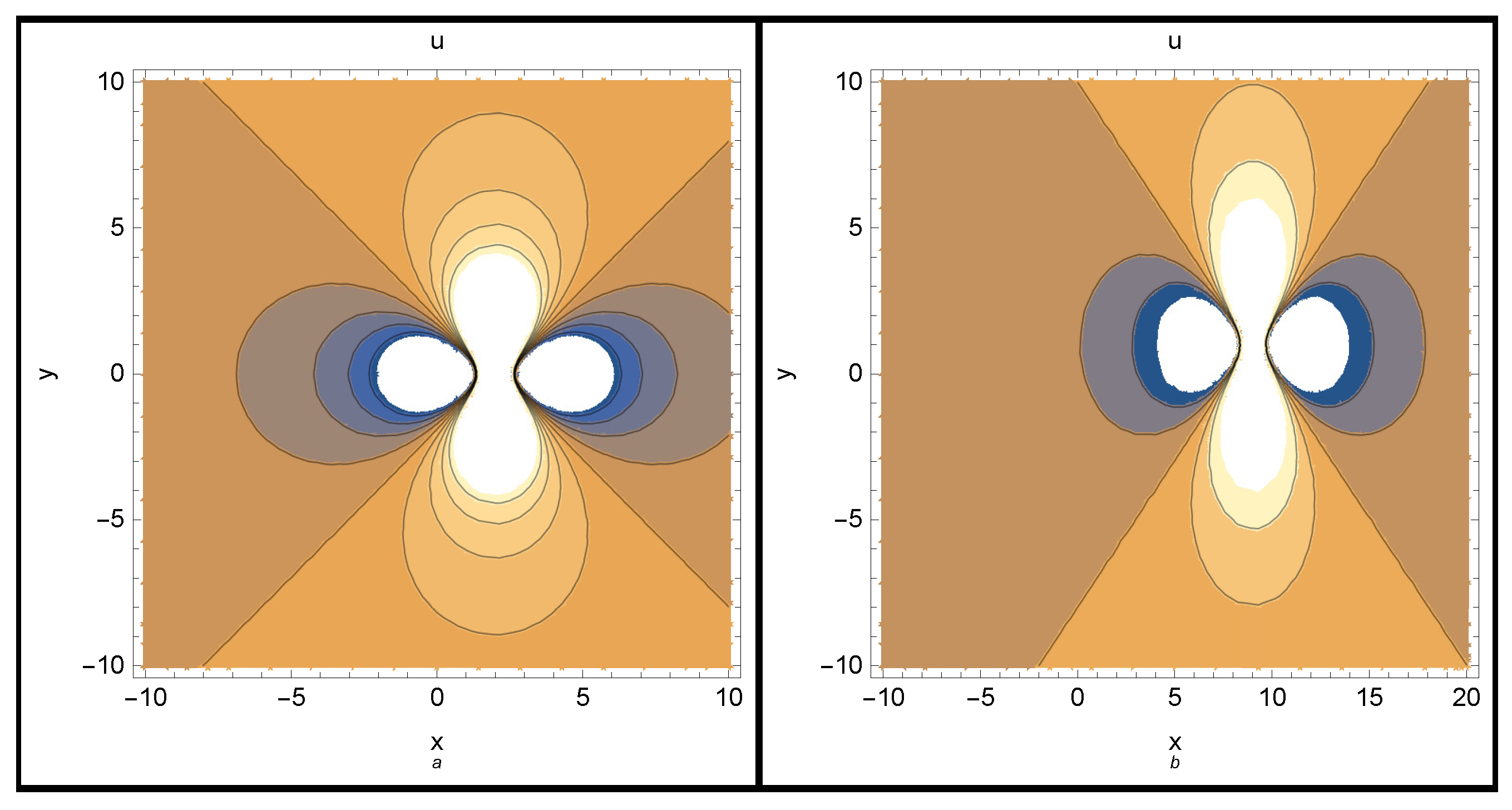
5. Lump Solutions (LSs)
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Akinyemi, L.; Senol, M.; Az-Zo’Bi, E.; Veeresha, P.; Akpan, U. Novel soliton solutions of four sets of generalized (2+1)-dimensional Boussinesq-Kadomtsev-Petviashvili-like equations. Modern Phys. Lett. B 2022, 36, 2150530. [Google Scholar] [CrossRef]
- Tao, G.; Sabi’u, J.; Nestor, S.; El-Shiekh, R.M.; Akinyemi, L.; Az-Zo’bi, E.; Betchewe, G. Dynamics of a new class of solitary wave structures in telecommunications systems via a (2+1)-dimensional nonlinear transmission line. Modern Phys. Lett. B 2022, 36, 2150596. [Google Scholar] [CrossRef]
- Ma, P.-L.; Tian, S.-F.; Zhang, T.-T.; Zhang, X.-Y. On Lie symmetries, exact solutions and integrability to the KdV–Sawada–Kotera–Ramani equation. Eur. Phys. J. Plus 2016, 131, 98. [Google Scholar] [CrossRef]
- Guo, B. Lax integrability and soliton solutions of the (2+1)–dimensional Kadomtsev–Petviashvili–Sawada–Kotera–Ramani equation. Front. Phys. 2022, 10, 1067405. [Google Scholar] [CrossRef]
- Ramani, A. Inverse scattering, ordinary differential equations of Painlevé type and Hirotas bilinear formalism. Ann. N. Y. Acad. Sci. 1981, 373, 54–67. [Google Scholar] [CrossRef]
- Hirota, R.; Ito, M. Resonance of solitons in one dimension. J. Phys. Soc. Japan 1983, 52, 744–748. [Google Scholar] [CrossRef]
- Weiss, J.; Tabor, M.; Carnevale, G. The Painlevé property of partial differential equations. J. Math. Phys. A 1983, 24, 522–526. [Google Scholar] [CrossRef]
- Wazwaz, A.M. N-soliton solutions for the combined KdV-CDG equation and the KdVLax equation. Appl. Math. Comput. 2008, 203, 402–407. [Google Scholar]
- Ma, Y.-L.; Wazwaz, A.M.; Li, B.-Q. New extended Kadomtsev-Petviashvili equation: Multiple-soliton solutions, breather, lump and interaction solutions. Nonlinear Dyn. 2021, 104, 1581–1594. [Google Scholar] [CrossRef]
- Ma, Y.-L.; Wazwaz, A.M.; Li, B.-Q. Novel bifurcation solitons for an extended Kadomtsev-Petviashvili equation in fluids. Phys. Lett. A 2021, 413, 127585. [Google Scholar] [CrossRef]
- Wazwaz, A.M.; Tantawy, S.A.E. Solving the (3+1)-dimensional KP Boussinesq and BKP-Boussinesq equations by the simplified Hirota method. Nonlinear Dyn. 2017, 88, 3017–3021. [Google Scholar] [CrossRef]
- Wazwaz, A.M. Painlevé analysis for a new integrable equation combining the modified Calogero-Bogoyavlenskii-Schiff (MCBS) equation with its negative-order form. Nonlinear Dyn. 2018, 91, 877–883. [Google Scholar] [CrossRef]
- Kaur, L.; Wazwaz, A.M. Painlevé analysis and invariant solutions of generalized fifth-order nonlinear integrable equation. Nonlinear Dyn. 2018, 94, 2469–2477. [Google Scholar] [CrossRef]
- Xu, G.Q. Painlevé analysis, lump-kink solutions and localized excitation solutions for the (3+1)-dimensional Boiti-Leon-Manna-Pempinelli equation. Appl. Math. Lett. 2019, 97, 81–87. [Google Scholar] [CrossRef]
- Xu, G.Q. The integrability for a generalized seventh order KdV equation: Painlevé property, soliton solutions, Lax pairs and conservation laws. Phys. Scr. 2014, 89, 125201. [Google Scholar]
- Xu, G.Q.; Wazwaz, A.M. Bidirectional solitons and interaction solutions for a new integrable fifth-order nonlinear equation with temporal and spatial dispersion. Nonlinear Dyn. 2020, 101, 581–595. [Google Scholar] [CrossRef]
- Zhou, Q.; Zhu, Q. Optical solitons in medium with parabolic law nonlinearity and higher order dispersion. Waves Random Complex Media 2014, 25, 52–59. [Google Scholar] [CrossRef]
- Zhou, Q. Optical solitons in the parabolic law media with high-order dispersion. Optik 2014, 125, 5432–5435. [Google Scholar] [CrossRef]
- Ashmead, J. Time dispersion in quantum mechanics. arXiv 2019, arXiv:1812.00935v2. [Google Scholar] [CrossRef]
- Hirota, R. The Direct Method in Soliton Theory; Cambridge University Press: Cambridge, UK, 2004. [Google Scholar]
- Hereman, W.; Nuseir, A. Symbolic methods to construct exact solutions of nonlinear partial differential equations. Math. Comput. Simul. 1997, 43, 13–27. [Google Scholar] [CrossRef]
- Khalique, C.M. Solutions and conservation laws of Benjamin-Bona-Mahony-Peregrine equation with power-law and dual power-law nonlinearities. Pramana J. Phys. 2013, 80, 413–427. [Google Scholar] [CrossRef]
- Khalique, C.M. Exact solutions and conservation laws of a coupled integrable dispersionless system. Filomat 2012, 26, 957–964. [Google Scholar] [CrossRef] [Green Version]
- Leblond, H.; Mihalache, D. Models of few optical cycle solitons beyond the slowly varying envelope approximation. Phys. Rep. 2013, 523, 61–126. [Google Scholar] [CrossRef] [Green Version]
- Leblond, H.; Mihalache, D. Few-optical-cycle solitons: Modified Korteweg-de Vries sine-Gordon equation versus other non-slowly-varying-envelope-approximation models. Phys. Rev. A 2009, 79, 063835. [Google Scholar] [CrossRef] [Green Version]
- Khuri, S.A. Soliton and periodic solutions for higher order wave equations of KdV type (I). Chaos Solitons Fractals 2005, 26, 25–32. [Google Scholar] [CrossRef]
- Khuri, S. Exact solutions for a class of nonlinear evolution equations: A unified ansätze approach. Chaos Solitons Fractals 2008, 36, 1181–1188. [Google Scholar] [CrossRef]
- Wazwaz, A.M. multiple-soliton solutions for the (2+1)-dimensional asymmetric Nizhanik-Novikov-Veselov equation. Nonlinear Anal. Ser. A Theory Methods Appl. 2010, 72, 1314–1318. [Google Scholar] [CrossRef]
- Wazwaz, A.M. multiple-soliton solutions and multiple complex soliton solutions for two distinct Boussinesq equations. Nonlinear Dyn. 2016, 85, 731–737. [Google Scholar] [CrossRef]
- Dahmani, Z.; Anber, A.; Gouari, Y.; Kaid, M.; Jebril, I. Extension of a Method for Solving Nonlinear Evolution Equations Via Conformable Fractional Approach. In Proceedings of the 2021 International Conference on Information Technology, ICIT 2021, Amman, Jordan, 14–15 July 2021; pp. 38–42. [Google Scholar]
- Hammad, M.A.; Al Horani, M.; Shmasenh, A.; Khalil, R. Ruduction of order of fractional differential equations. J. Math. Comput. Sci. 2018, 8, 683–688. [Google Scholar]
- Dababneh, A.; Sami, B.; Hammad, M.A.; Zraiqat, A. A new impulsive sequential multi-orders fractional differential equation with boundary conditions. J. Math. Comput. Sci. 2020, 10, 2871–2890. [Google Scholar]
- Noor, S.; Abu Hammad, M.A.; Shah, R.; Alrowaily, A.W.; El-Tantawy, S.A. Numerical Investigation of Fractional-Order Fornberg–Whitham Equations in the Framework of Aboodh Transformation. Symmetry 2023, 15, 1353. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wazwaz, A.-M.; Abu Hammad, M.; Al-Ghamdi, A.O.; Alshehri, M.H.; El-Tantawy, S.A. New (3+1)-Dimensional Kadomtsev–Petviashvili–Sawada– Kotera–Ramani Equation: Multiple-Soliton and Lump Solutions. Mathematics 2023, 11, 3395. https://doi.org/10.3390/math11153395
Wazwaz A-M, Abu Hammad M, Al-Ghamdi AO, Alshehri MH, El-Tantawy SA. New (3+1)-Dimensional Kadomtsev–Petviashvili–Sawada– Kotera–Ramani Equation: Multiple-Soliton and Lump Solutions. Mathematics. 2023; 11(15):3395. https://doi.org/10.3390/math11153395
Chicago/Turabian StyleWazwaz, Abdul-Majid, Ma’mon Abu Hammad, Ali O. Al-Ghamdi, Mansoor H. Alshehri, and Samir A. El-Tantawy. 2023. "New (3+1)-Dimensional Kadomtsev–Petviashvili–Sawada– Kotera–Ramani Equation: Multiple-Soliton and Lump Solutions" Mathematics 11, no. 15: 3395. https://doi.org/10.3390/math11153395
APA StyleWazwaz, A.-M., Abu Hammad, M., Al-Ghamdi, A. O., Alshehri, M. H., & El-Tantawy, S. A. (2023). New (3+1)-Dimensional Kadomtsev–Petviashvili–Sawada– Kotera–Ramani Equation: Multiple-Soliton and Lump Solutions. Mathematics, 11(15), 3395. https://doi.org/10.3390/math11153395








