Dynamics of a Predator–Prey Model with Impulsive Diffusion and Transient/Nontransient Impulsive Harvesting
Abstract
1. Introduction
2. The Model
3. Some Lemmas
4. The Dynamics
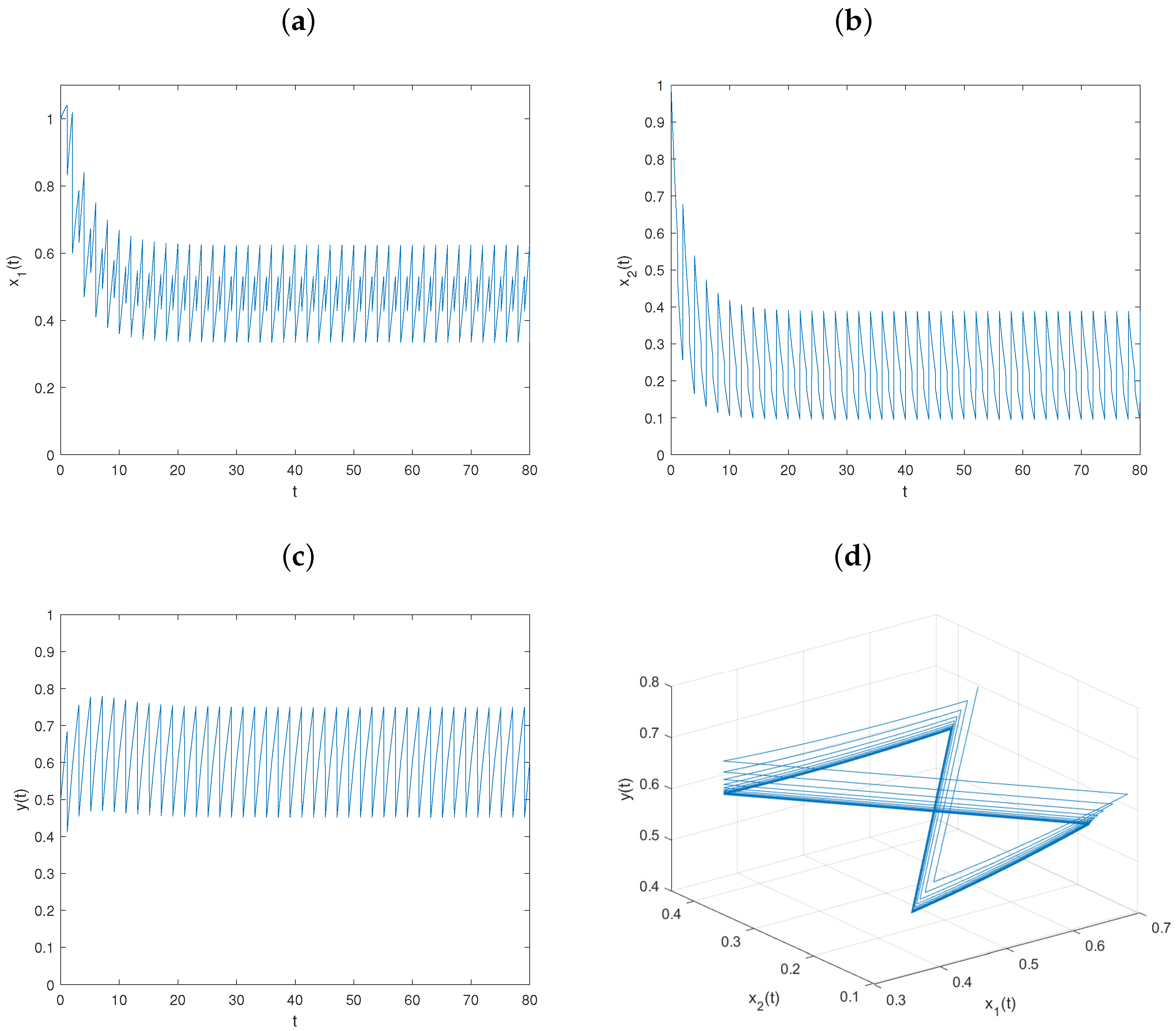
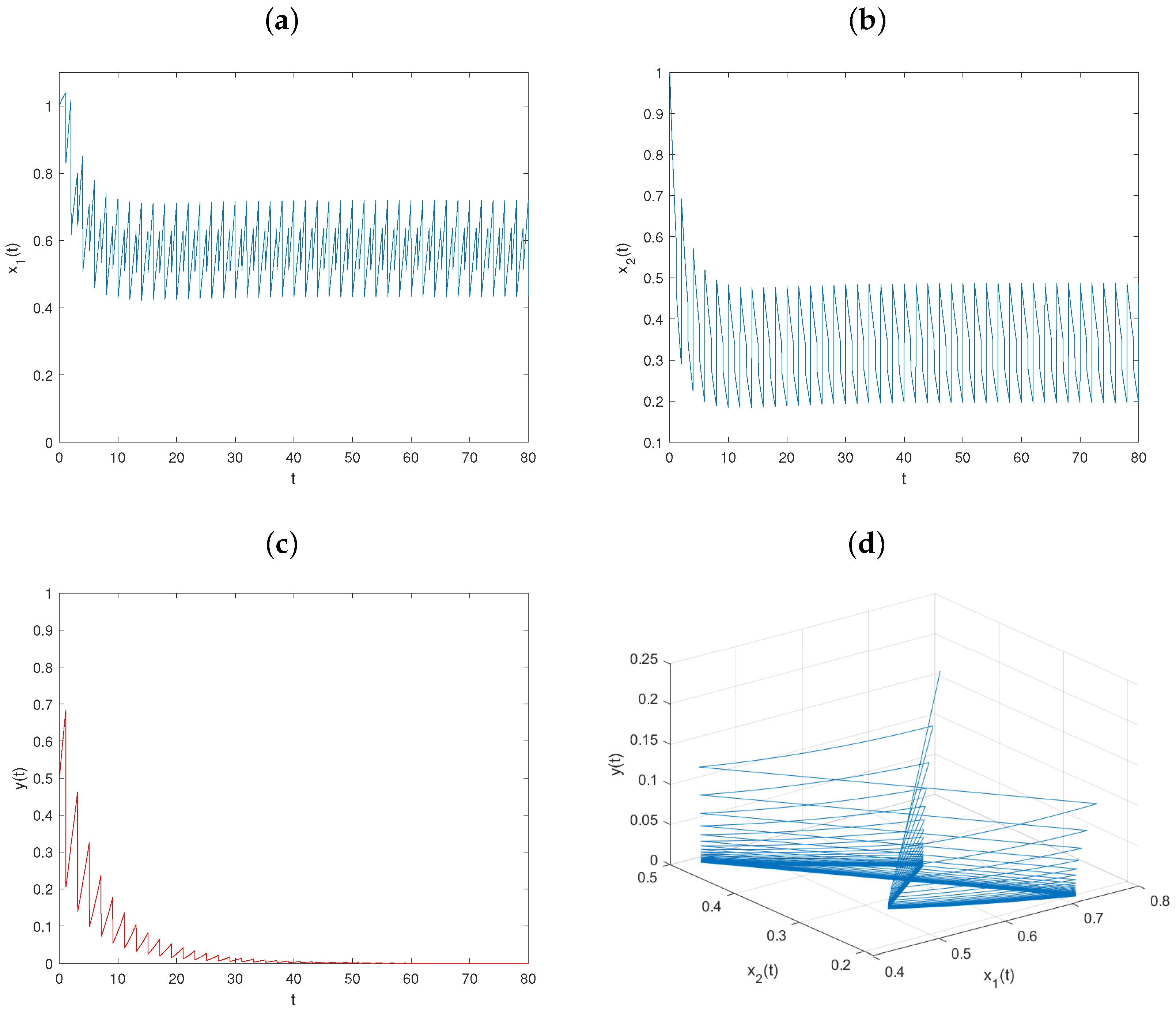
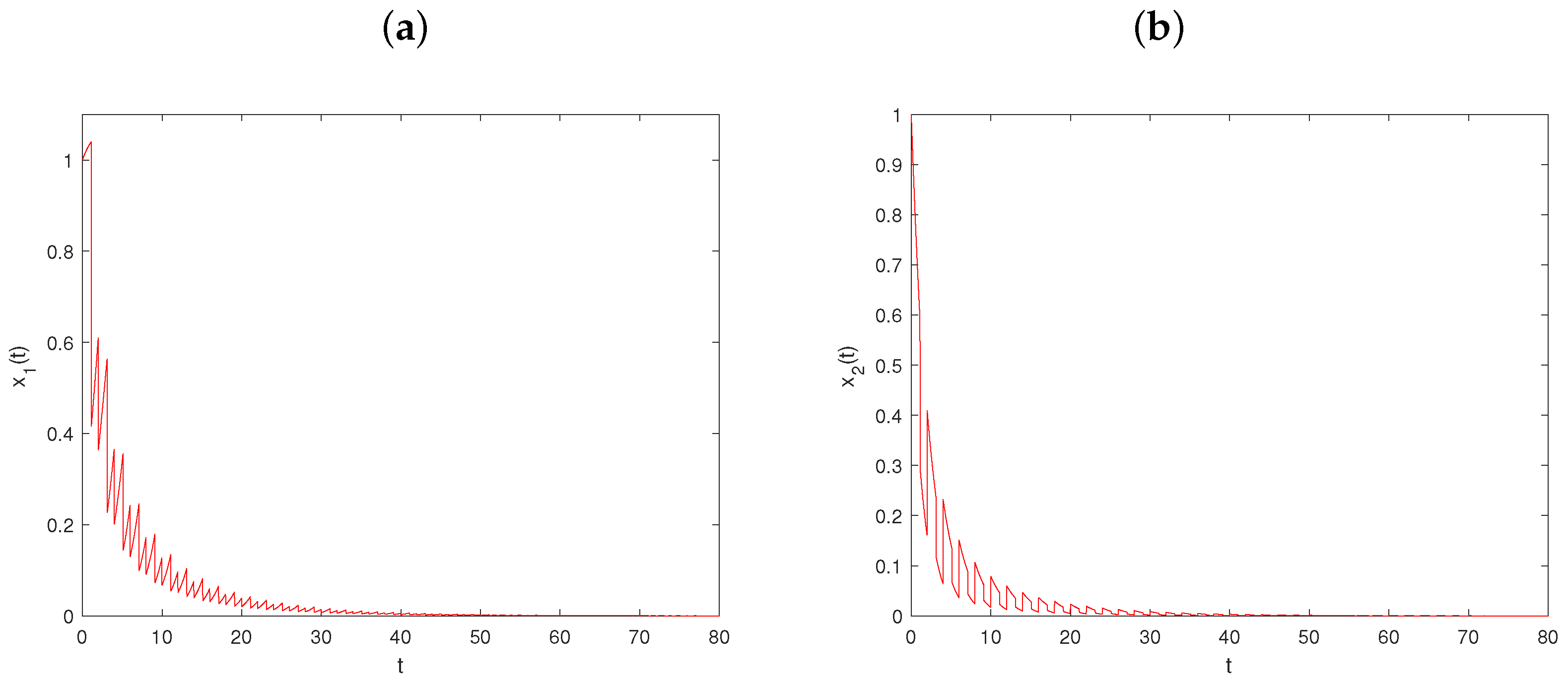
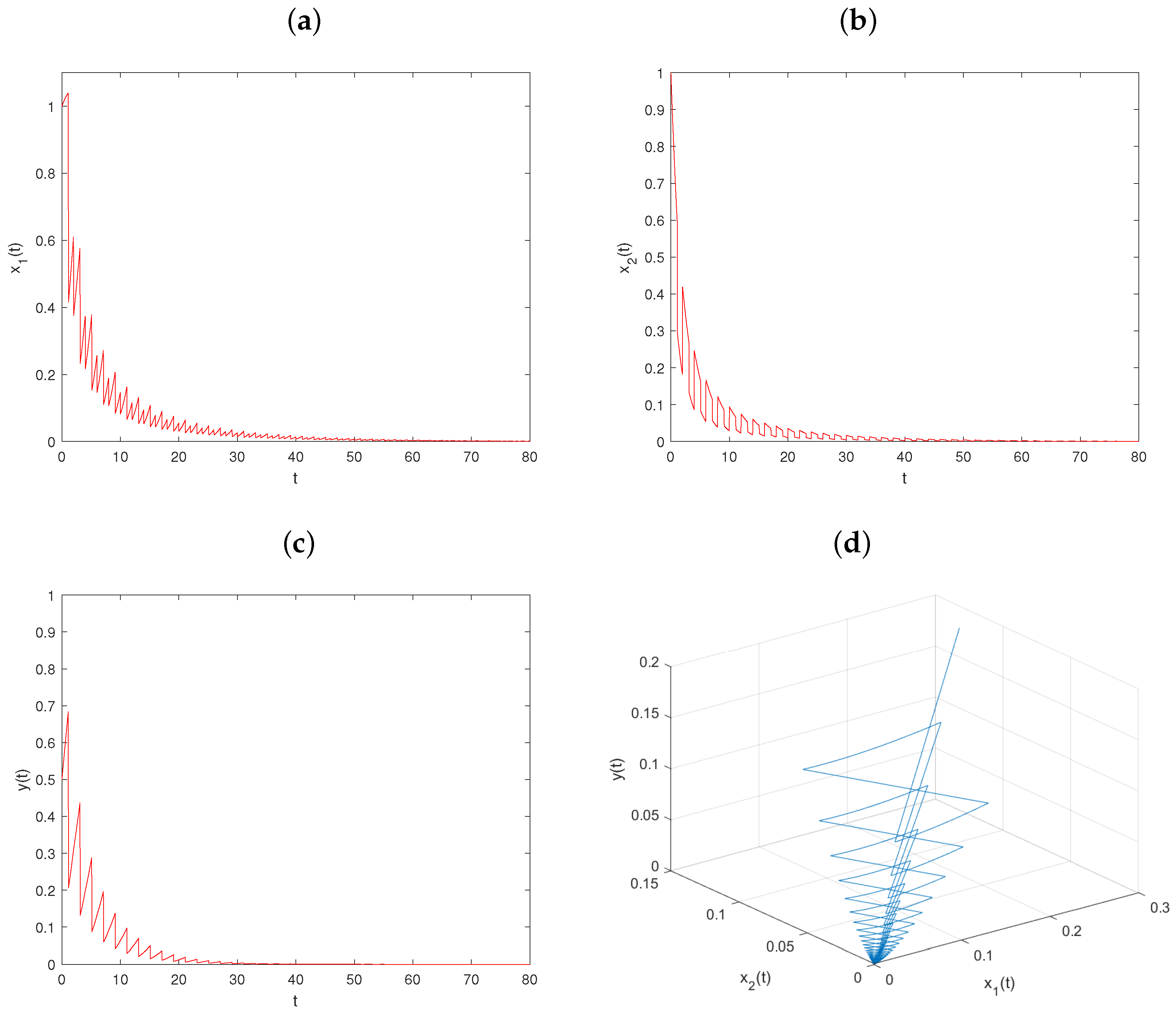
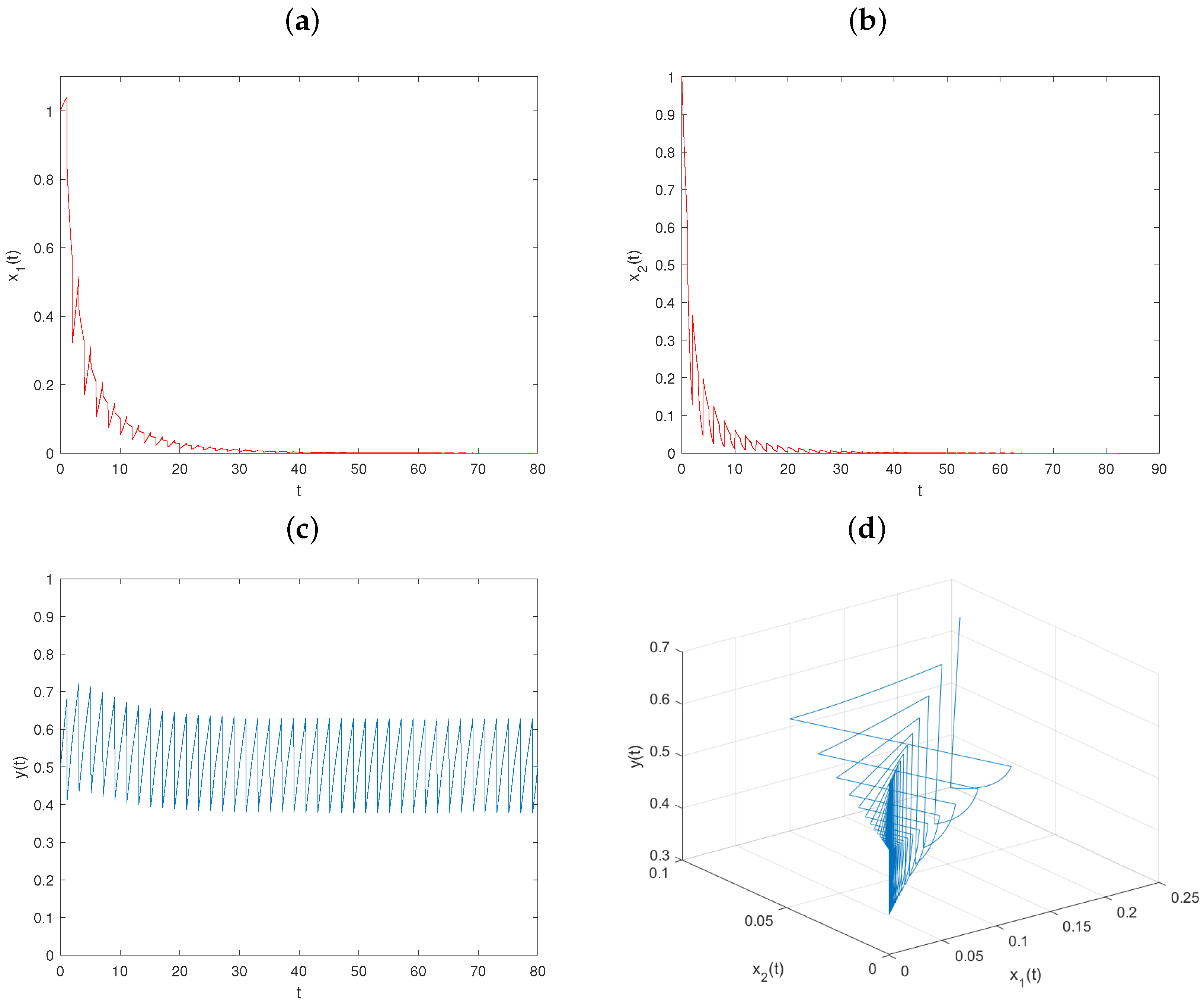
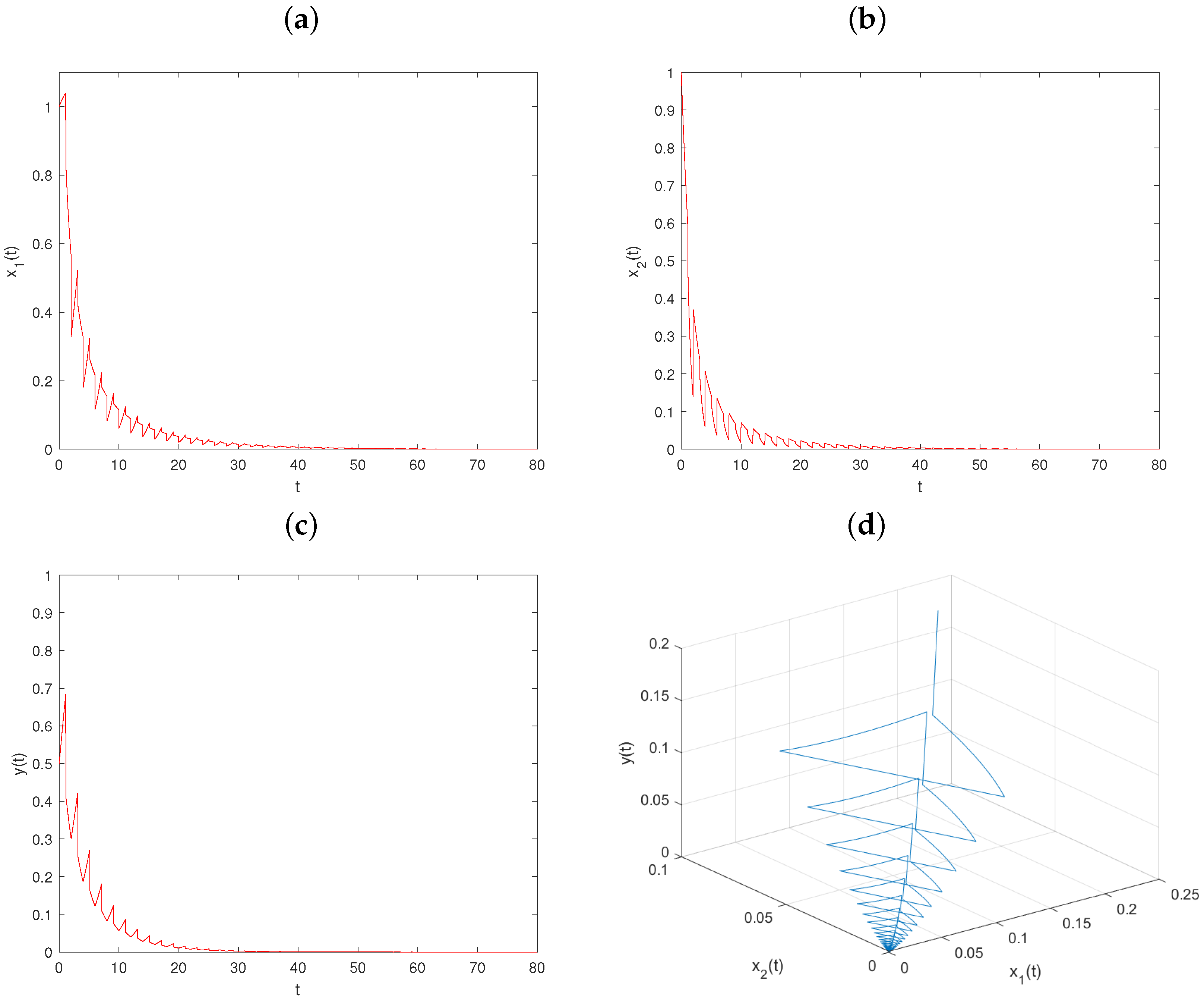
5. Numerical Simulations and Discussion
5.1. The Effect of the Transient Impulsive Harvesting on Populations
5.2. The Effect of Nontransient Impulsive Harvesting on Populations
6. Conclusions
- (1)
- All solutions of system are uniformly ultimately bounded.
- (2)
- If (36)–(39) hold, the solution of system is globally asymptotically stable.
- (3)
- If (64)–(66) hold, the solution of system is globally asymptotically stable.
- (4)
- If (67)–(68) hold, the trivial solution of system is globally asymptotically stable.
- (5)
- The permanent conditions of system have also been established, that isand
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Brauer, F.; Soudack, A. Stability regions in predator–prey systems with constant-rate prey harvesting. J. Math. Biol. 1979, 8, 55–71. [Google Scholar] [CrossRef]
- Kumar, S.; Kharbanda, H. Chaotic behavior of predator–prey model with group defense and non-linear harvesting in prey. Chaos Solitons Fractals 2018, 119, 19–28. [Google Scholar] [CrossRef]
- Lv, Y.; Yuan, R.; Pei, Y. A prey-predator model with harvesting for fishery resource with reserve area. Appl. Math. Model. 2013, 37, 3048–3062. [Google Scholar] [CrossRef]
- Liu, X.; Chen, L. Global dynamics of the periodic logistic system with periodic impulsive perturbations. J. Math. Anal. Appl. 2004, 289, 279–291. [Google Scholar] [CrossRef]
- Tian, Y.; Gao, Y.; Sun, K. Global dynamics analysis of instantaneous harvest fishery model guided by weighted escapement strategy. Chaos Solitons Fractals 2022, 164, 112597. [Google Scholar] [CrossRef]
- Liu, J.; Hu, J.; Yuen, P. Extinction and permanence of the predator–prey system with general functional response and impulsive control. Appl. Math. Model. 2020, 88, 55–67. [Google Scholar] [CrossRef]
- Wei, C.; Liu, J.; Chen, L. Homoclinic bifurcation of a ratio-dependent predator–prey system with impulsive harvesting. Nonlinear Dyn. 2017, 89, 2001–2012. [Google Scholar] [CrossRef]
- Jiao, J.; Liu, Z.; Li, L.; Nie, X. Threshold dynamics of a stage-structured single population model with non-transient and transient impulsive effects. Appl. Math. Lett. 2019, 97, 88–92. [Google Scholar] [CrossRef]
- Tao, X.; Zhu, L. Study of periodic diffusion and time delay induced spatiotemporal patterns in a predator–prey system. Chaos Solitons Fractals 2021, 150, 13–14. [Google Scholar] [CrossRef]
- Wang, L.; Liu, Z.; Chen, L. Impulsive diffusion in single species model. Chaos Solitons Fractals 2006, 33, 1213–1219. [Google Scholar] [CrossRef]
- Mishra, P.; Raw, S.; Tiwari, B. On a cannibalistic predator–prey model with prey defense and diffusion. Appl. Math. Model. 2021, 90, 165–190. [Google Scholar] [CrossRef]
- Sugden, A.; Pennisi, E. When to Go, Where to Stop. Science 2006, 313, 775. [Google Scholar] [CrossRef]
- Takeuchi, Y. Global Dynamical Properties of Lotka-Volterra System; World Scientific: Singapore, 1996. [Google Scholar]
- Hui, J.; Chen, L. A single species model with impulsive diffusion. Acta Math. Appl. Sin. 2005, 21, 43–48. [Google Scholar] [CrossRef]
- Cui, J. The effect of diffusion on the time varying logistic population growth. Comput. Math. Appl. 1998, 36, 1–9. [Google Scholar] [CrossRef][Green Version]
- Zhong, Z.; Zhang, X.; Chen, L. The effect of pulsed harvesting policy on the inshore-offshore fishery model with the impulsive diffusion. Nonlinear Dyn. 2011, 63, 537–545. [Google Scholar]
- Jiao, J.; Cai, S.; Chen, L. Dynamical Analysis of a three-dimensional predator–prey model with impulsive harvesting and diffusion. Int. J. Bifurcat. Chaos 2011, 21, 453–465. [Google Scholar] [CrossRef]
- Dhar, J.; Jatav, K.S. Mathematical analysis of a delayed stage-structured predator–prey model with impulsive diffusion between two predators territories. Ecol. Complex. 2013, 16, 59–67. [Google Scholar] [CrossRef]
- DuTemple, L.A.; Stone, L.M. Tigers; Lerner Publications: Minneapolis, MN, USA, 1996. [Google Scholar]
- Seidensticker, J. Tigers; MBI Publishing Company: Saint Paul, Brazil, 1996. [Google Scholar]
- Bainov, D.; Simeonov, P. Impulsive Differential Equations: Periodic Solutions and Applications; Longman Scientific and Technical, 1993. [Google Scholar]
- Jury, E. Inners and Stability of Dynamic Systems; Wiley: New York, NY, USA, 1974. [Google Scholar]
- Smith, H. Cooperative systems of differential equations with concave nonlinearities. Nonlinear Anal. TMA 1986, 10, 1037–1052. [Google Scholar] [CrossRef]
- Jiao, J.; Tang, W. Dynamics of a lake-eutrophication model with nontransient/transient impulsive dredging and pulse inputting. Adv. Differ. Equ. 2021, 2021, 1–16. [Google Scholar] [CrossRef]
- Lakshmikantham, V. Theory of Impulsive Differential Equations; World Scientific: Singapore, 1989. [Google Scholar]
- Wang, J.; Cheng, H.; Liu, H.; Wang, Y. Periodic solution and control optimization of a prey-predator model with two types of harvesting. Adv. Differ. Equ. 2018, 2018, 41. [Google Scholar] [CrossRef]
- Li, Y.; Cui, J.; Song, X. Dynamics of a predator–prey system with pulses. Appl. Math. Comput. 2008, 204, 269–280. [Google Scholar] [CrossRef]
- Lawson, J.; Braverman, E. Optimality and sustainability of delayed impulsive harvesting. Commun. Nonlinear Sci. 2022, 117. [Google Scholar] [CrossRef]
- Zhang, X.; Shuai, Z.; Wang, K. Optimal impulsive harvesting policy for single population. Nonlinear Anal. Real World Appl. 2003, 4, 639–651. [Google Scholar] [CrossRef]
- Amit, S.; Gupta, B.; Dhar, J.; Srivastava, S.K.; Sharma, P. Stability analysis and optimal impulsive harvesting for a delayed stage-structured self dependent two compartment commercial fishery model. Int. J. Control 2021, 10, 1119–1129. [Google Scholar]
- Umar, M.; Sabir, Z.; Raja, M.A.Z.; Amin, F.; Saeed, T.; Sanchez, Y.G. Design of intelligent computing solver with Morlet wavelet neural networks for nonlinear predator–prey model. Appl. Soft Comput. 2023, 134, 109975. [Google Scholar] [CrossRef]
- Ruttanaprommarin, N.; Sabir, Z.; Said, S.B.; Raja, M.A.Z.; Bhatti, S.; Weera, W.; Botmart, T. Supervised neural learning for the predator–prey delay differential system of Holling form-III. AIMS Math. 2022, 7, 20126–20142. [Google Scholar] [CrossRef]
- Jitendra; Chaurasiya, V.; Rai, K.N.; Singh, J. Legendre wavelet residual approach for moving boundary problem with variable thermal physical properties. Int. J. Nonlinear Sci. Numer. Simul. 2022, 23, 957–970. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Quan, Q.; Dai, X.; Jiao, J. Dynamics of a Predator–Prey Model with Impulsive Diffusion and Transient/Nontransient Impulsive Harvesting. Mathematics 2023, 11, 3254. https://doi.org/10.3390/math11143254
Quan Q, Dai X, Jiao J. Dynamics of a Predator–Prey Model with Impulsive Diffusion and Transient/Nontransient Impulsive Harvesting. Mathematics. 2023; 11(14):3254. https://doi.org/10.3390/math11143254
Chicago/Turabian StyleQuan, Qi, Xiangjun Dai, and Jianjun Jiao. 2023. "Dynamics of a Predator–Prey Model with Impulsive Diffusion and Transient/Nontransient Impulsive Harvesting" Mathematics 11, no. 14: 3254. https://doi.org/10.3390/math11143254
APA StyleQuan, Q., Dai, X., & Jiao, J. (2023). Dynamics of a Predator–Prey Model with Impulsive Diffusion and Transient/Nontransient Impulsive Harvesting. Mathematics, 11(14), 3254. https://doi.org/10.3390/math11143254




