Dissipative Discrete PID Load Frequency Control for Restructured Wind Power Systems via Non-Fragile Design Approach
Abstract
1. Introduction
- 1.
- A restructured wind power system model is introduced. Compared to some existing models, including single-generator unit models [35], the proposed restructured model introduces new information signals and different controller participation coefficients, resulting in better grid reliability.
- 2.
- A non-fragile discrete PID control scheme for interconnected wind power systems is designed, which can tolerate control gain fluctuation and reduce huge computation costs.
- 3.
- Based on the constructed discrete Lyapunov–Krasovskii functional, strict dissipativity conditions were established for wind power systems. The results can be obtained with lower conservatism and higher computational efficiency.
2. Preliminaries
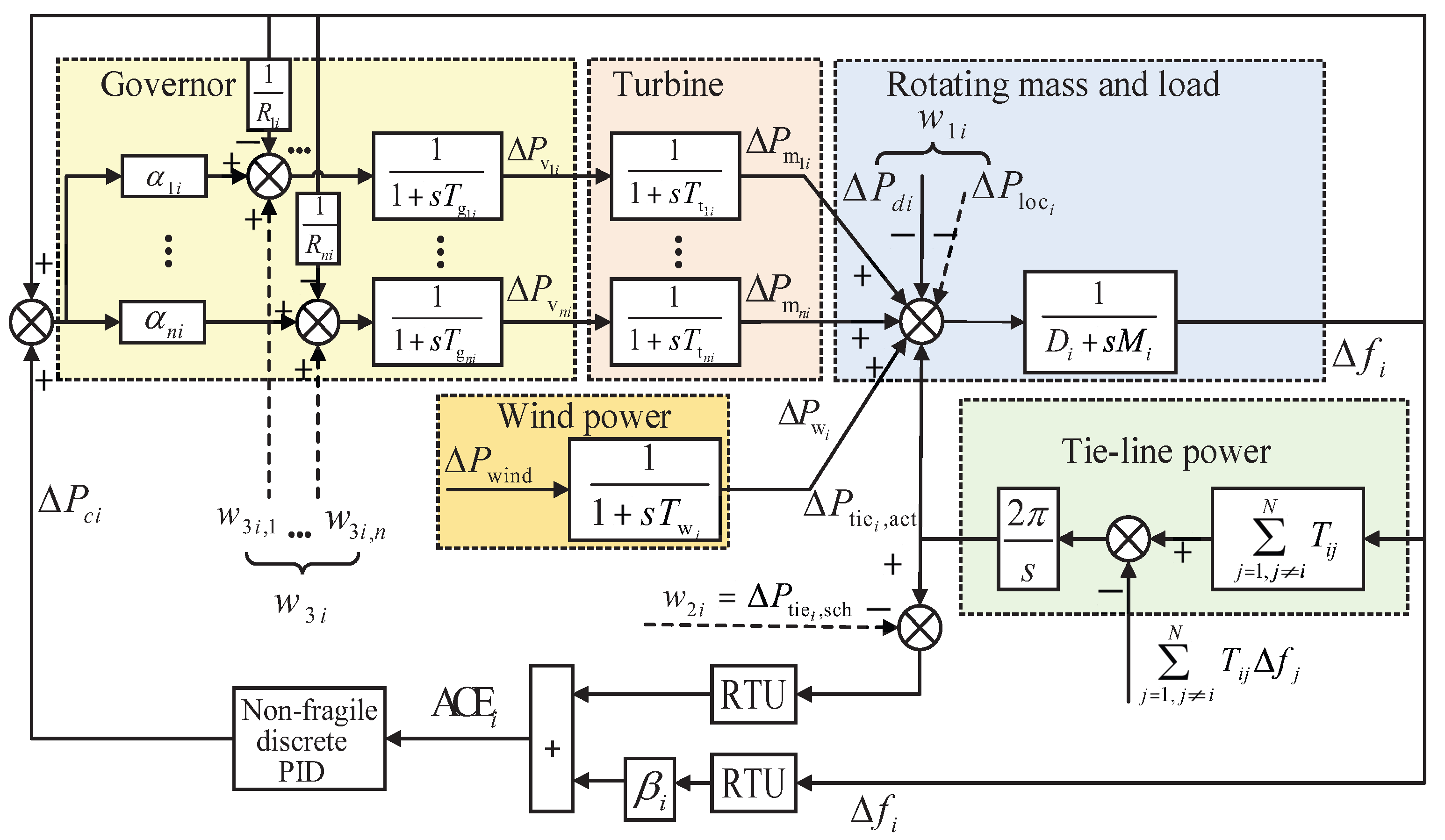
2.1. Restructured LFC Wind Power System Model
2.2. Non-Fragile Discrete PID LFC Scheme
3. Results
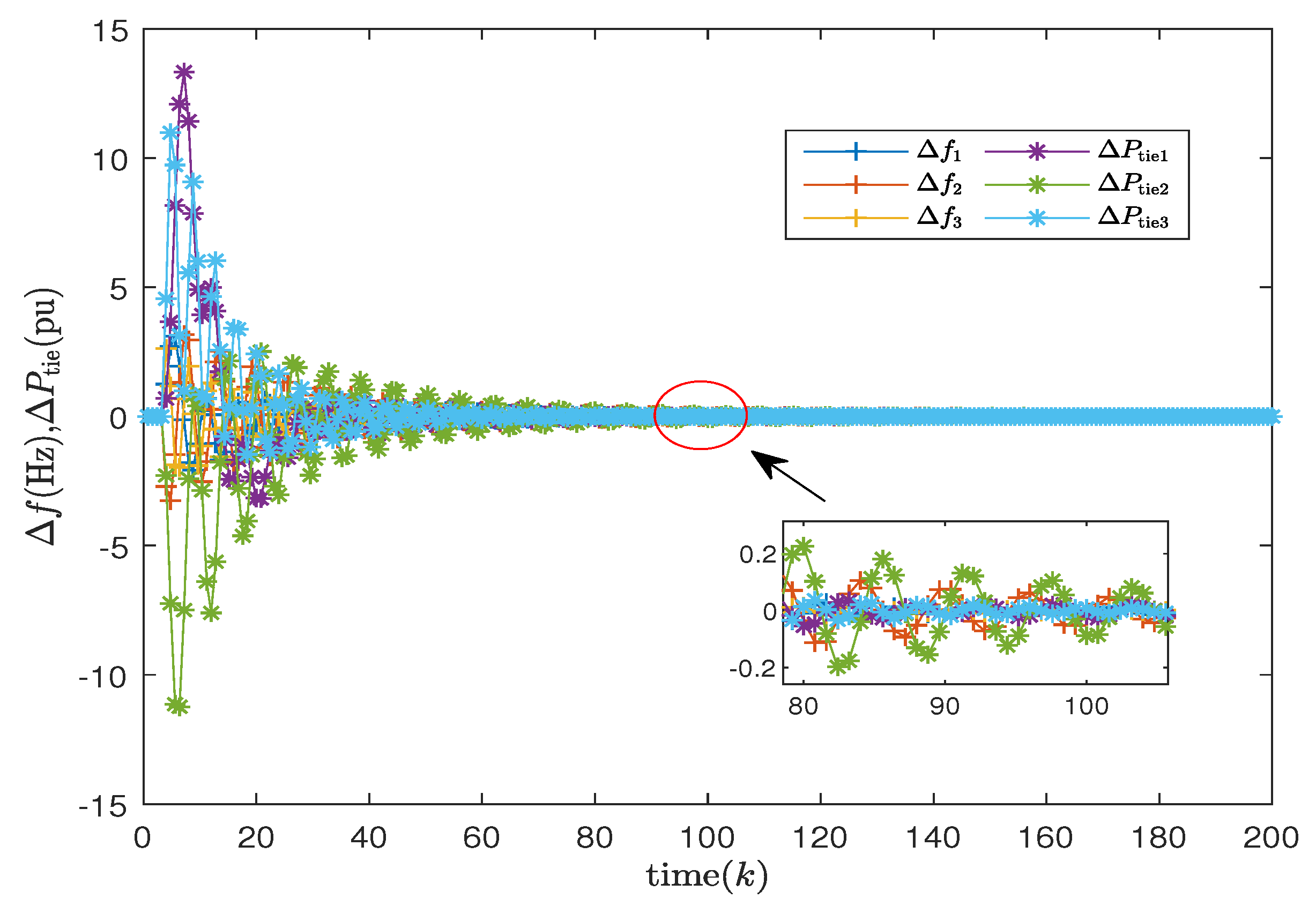
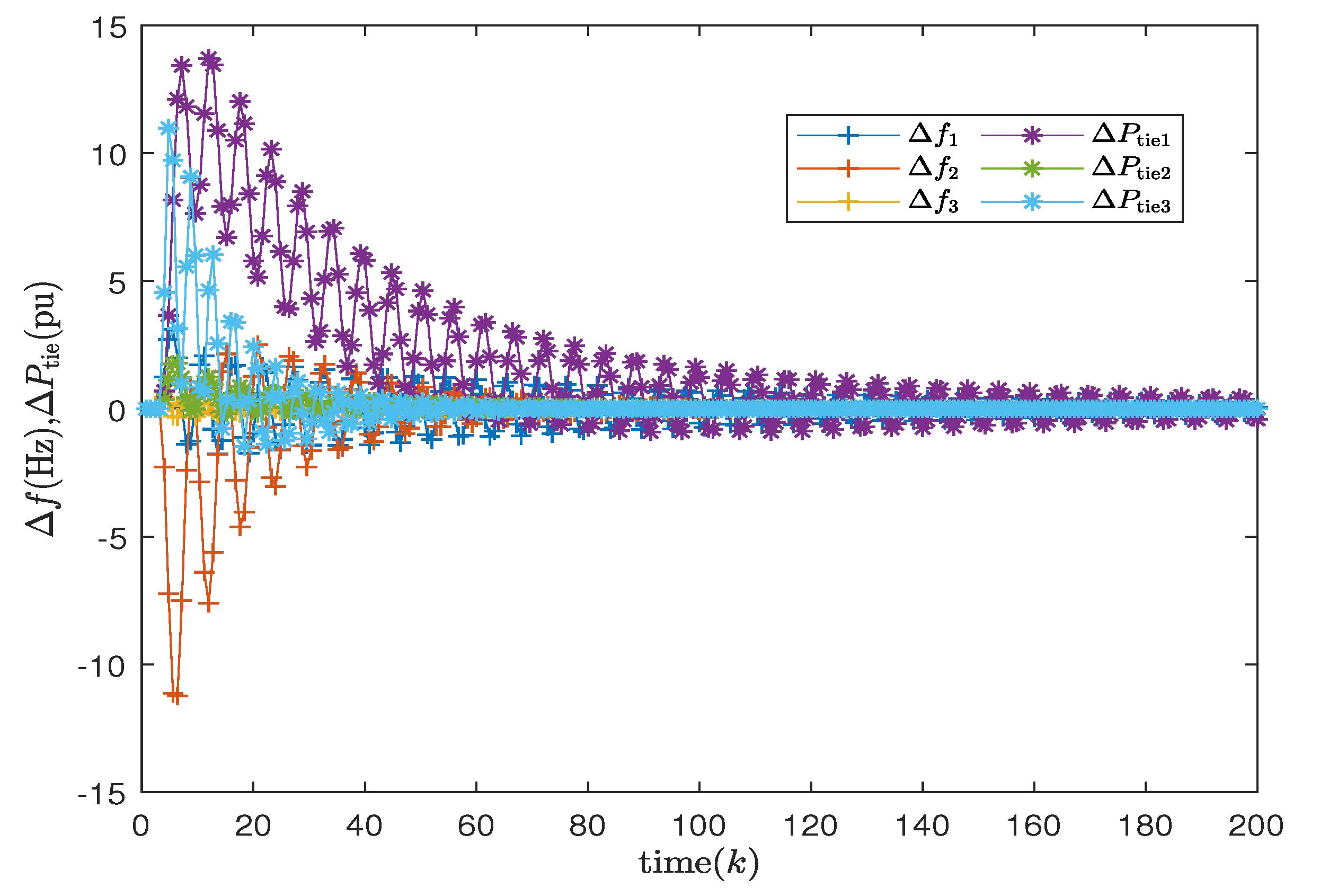
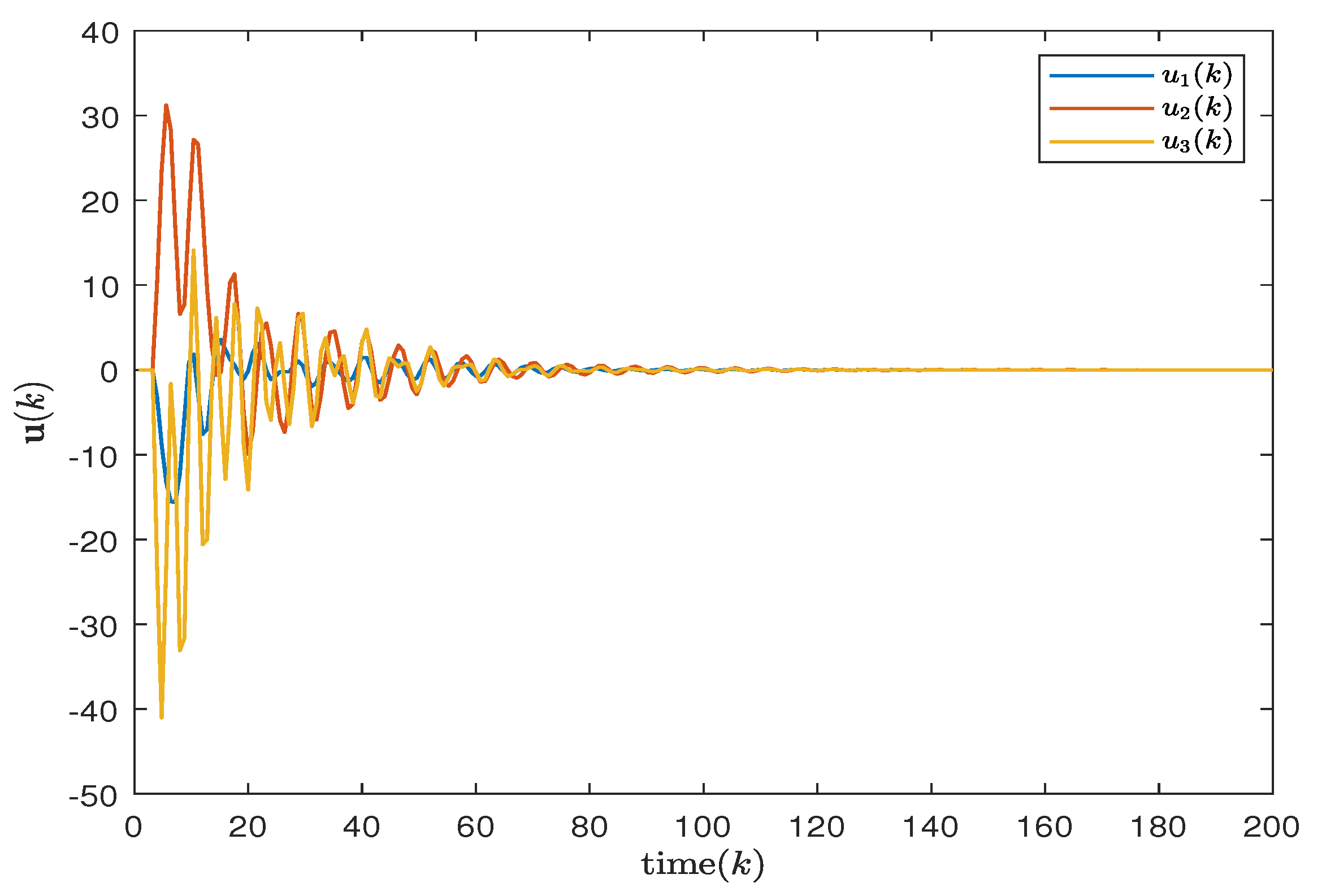
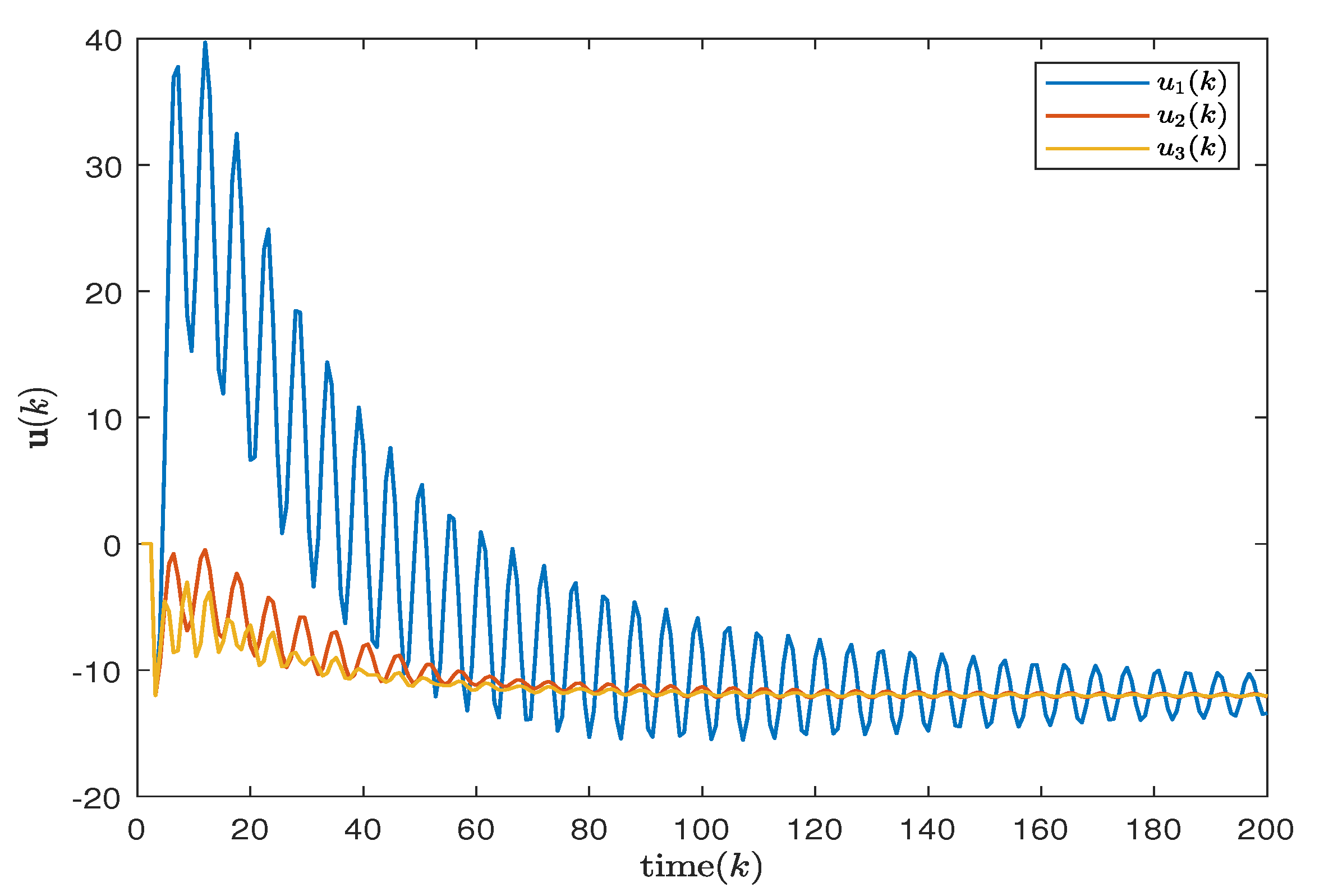
4. Illustrative Examples
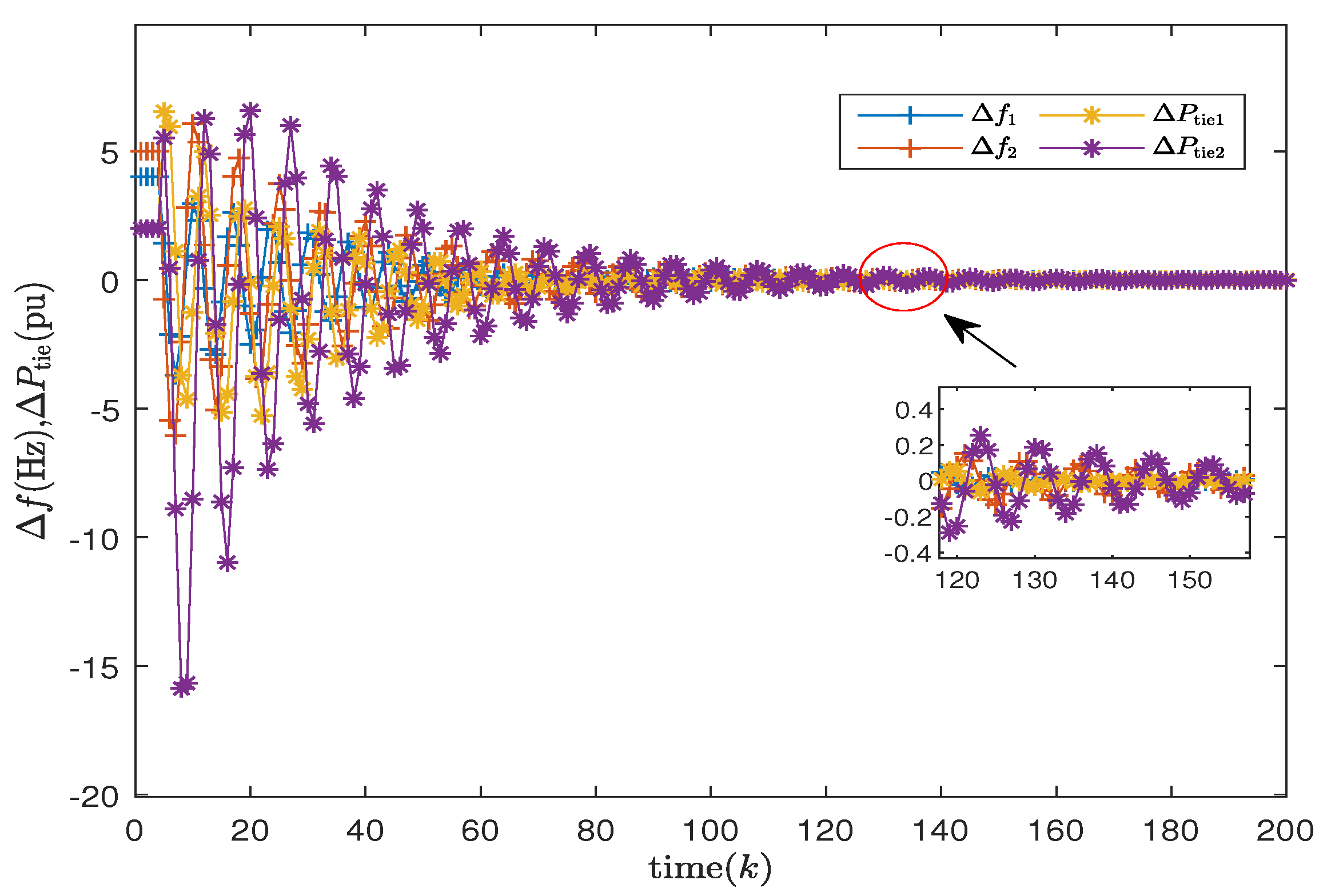
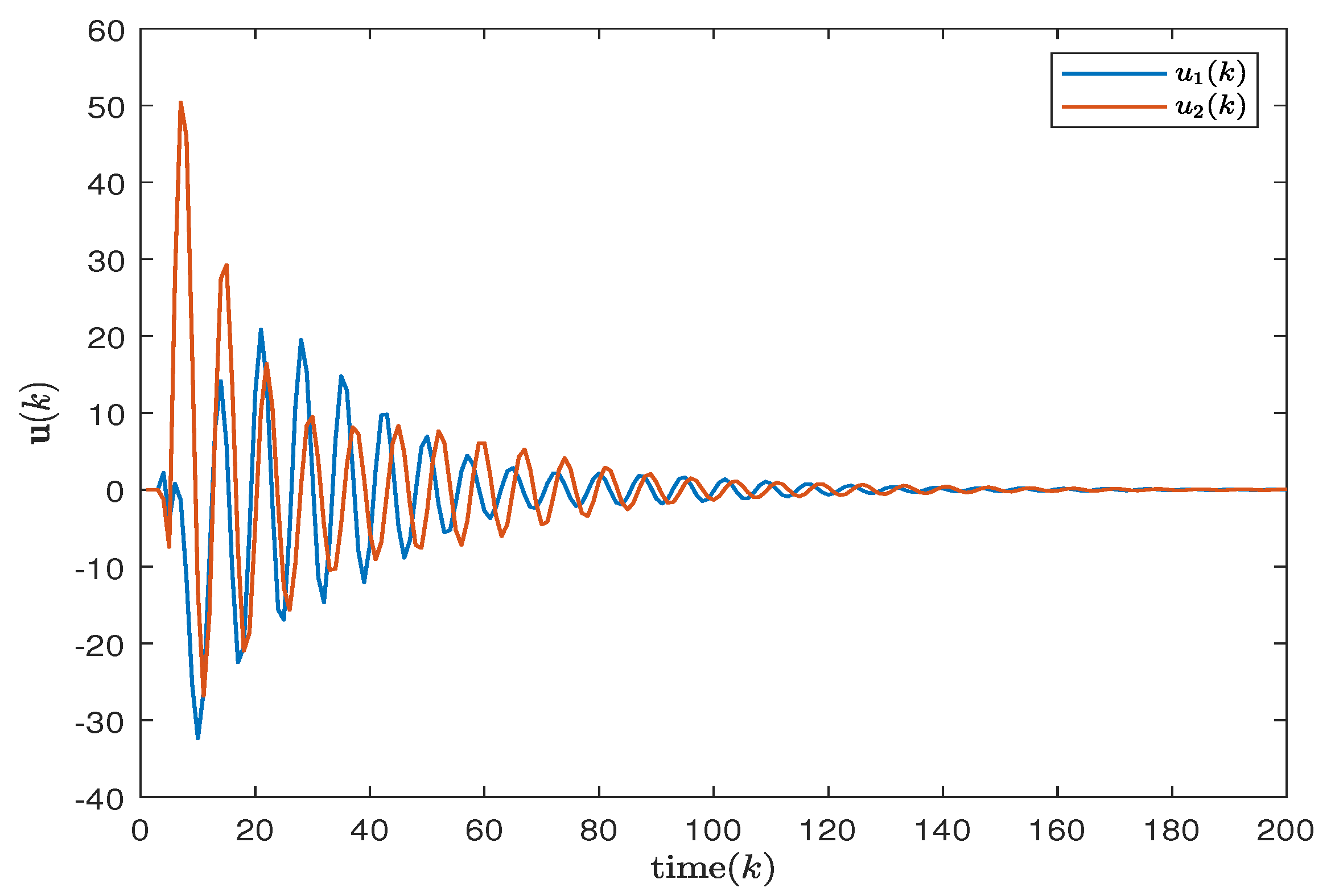
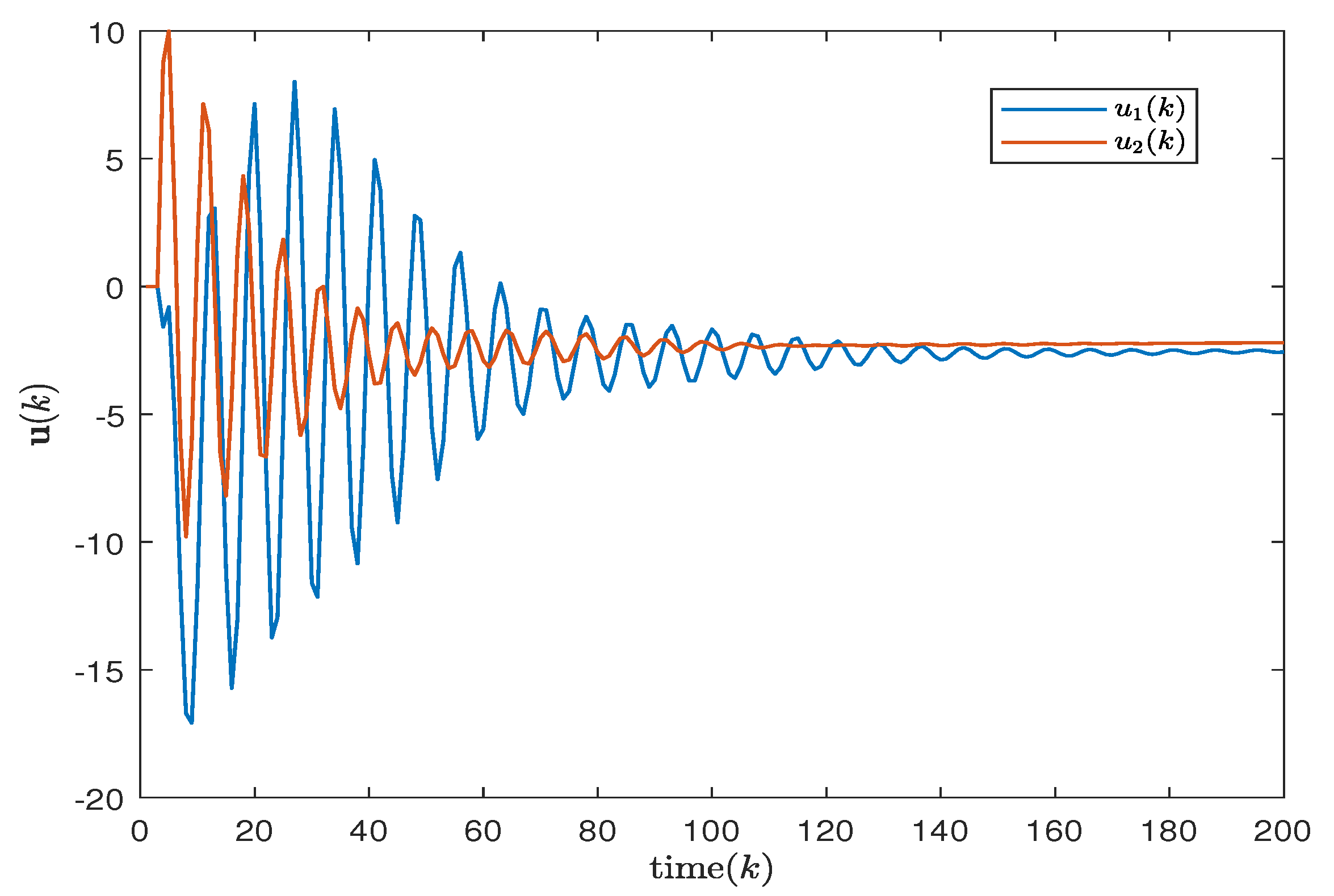
4.1. Effectiveness of Non-Fragile Discrete PID LFC
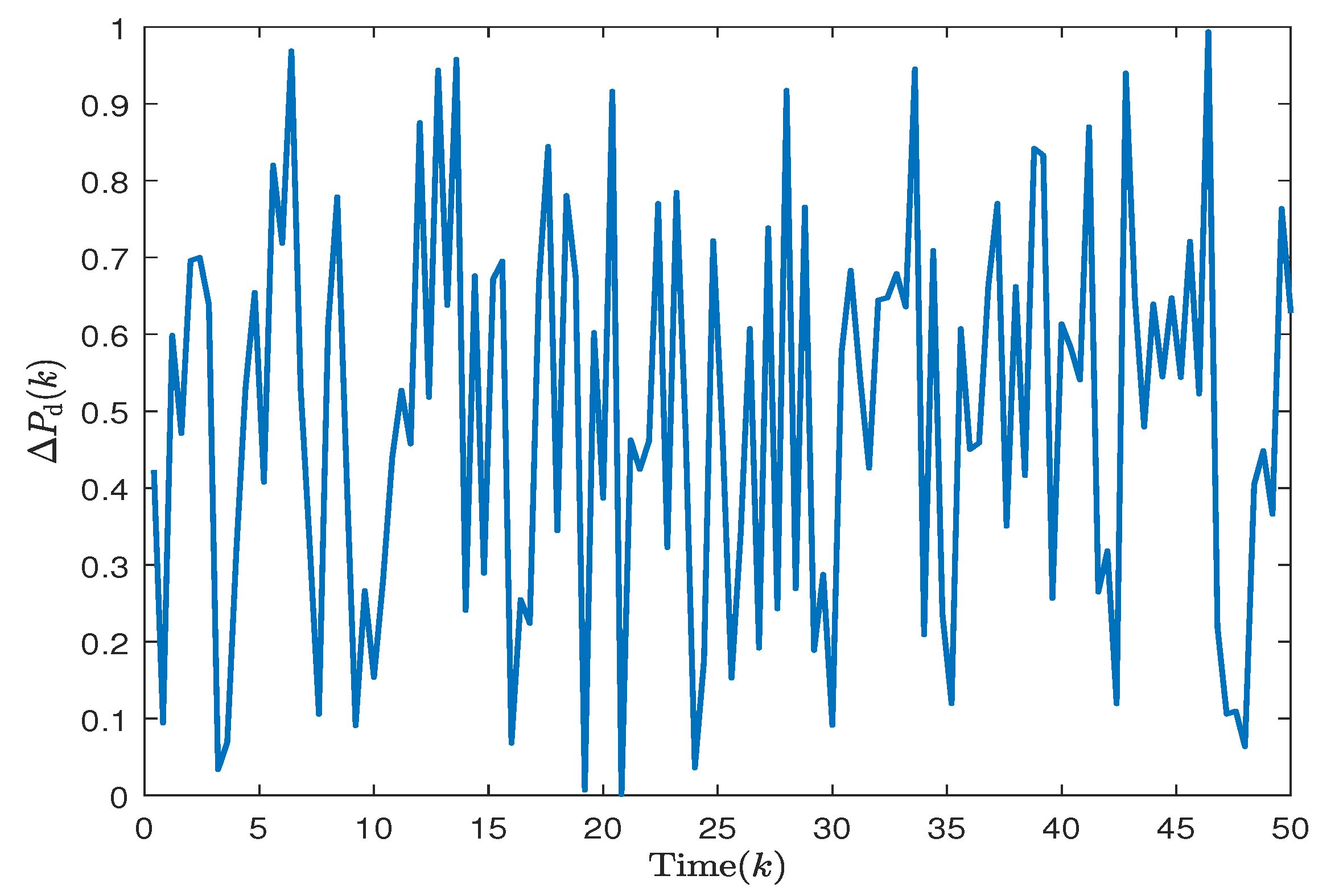
4.2. Case Study of Wind Power System
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
Appendix B
References
- Pandey, S.K.; Mohanty, S.R.; Kishor, N. A literature survey on LFC for conventional and distribution generation power systems. Renew. Sustain. Energ. Rev. 2013, 25, 318–334. [Google Scholar] [CrossRef]
- Wang, Q.; Yu, Y.; Zhang, Z.; Han, X. Melnikov-threshold-triggered mixed-mode oscillations in a family of amplitude-modulated forced oscillator. J. Low Freq. Noise Vib. Act. Control 2019, 38, 377–387. [Google Scholar] [CrossRef]
- Yu, Y.; Wang, Q.; Bi, Q.; Lim, C.W. Multiple-s-shaped critical manifold and jump phenomena in low frequency forced vibration with amplitude modulation. Int. J. Bifurcat. Chaos 2019, 29, 1930012. [Google Scholar] [CrossRef]
- Sun, L.; Zhang, C.; Yu, Y. A boundary knot method for 3D time harmonic elastic wave problems. Appl. Math. Lett. 2020, 104, 106210. [Google Scholar] [CrossRef]
- Li, Y.; Ni, Z.; Zhao, T.; Yu, M.; Liu, Y.; Wu, L.; Zhao, Y. Coordinated scheduling for improving uncertain wind power adsorption in electric vehicles-wind integrated power systems by multiobjective optimization approach. IEEE Trans. Ind. Appl. 2020, 56, 2238–2250. [Google Scholar] [CrossRef]
- Nguyen, N.; Mitra, J. An analysis of the effects and dependency of wind power penetration on system frequency regulation. IEEE Trans. Sustain. Energy 2016, 7, 354–363. [Google Scholar] [CrossRef]
- Li, M.; Yang, M.; Yu, Y.; Lee, W.J. A wind speed correction method based on modified hidden Markov model for enhancing wind power forecast. IEEE Trans. Ind. Appl. 2022, 58, 656–666. [Google Scholar] [CrossRef]
- Liao, K.; Lu, D.; Wang, M.; Yang, J. A low-pass virtual filter for output power smoothing of wind energy conversion systems. IEEE Trans. Ind. Electron. 2022, 69, 12874–12885. [Google Scholar] [CrossRef]
- Sun, S.; Liu, Y.; Li, Q.; Wang, T.; Chu, F. Short-term multi-step wind power forecasting based on spatio-temporal correlations and transformer neural networks. Energy Convers. Manag. 2023, 283, 116916. [Google Scholar] [CrossRef]
- Wang, Y.; Meng, J.; Zhang, X.; Xu, L. Control of PMSG-based wind turbines for system inertial response and power oscillation damping. IEEE Trans. Sustain. Energy 2015, 6, 565–574. [Google Scholar] [CrossRef]
- Chen, C.; Su, Y.; Yang, T.; Huang, Z. Virtual inertia coordination control strategy of DFIG-based wind turbine for improved grid frequency response ability. Electr. Power Syst. Res. 2023, 216, 109076. [Google Scholar] [CrossRef]
- Vachirasricirikul, S.; Ngamroo, I. Robust LFC in a smart grid with wind power penetration by coordinated vehicle-to-grid control and frequency controller. IEEE Trans. Smart Grid 2014, 5, 371–380. [Google Scholar] [CrossRef]
- Shangguan, X.C.; Zhang, C.K.; He, Y.; Jin, L.; Jiang, L.; Spencer, J.W.; Wu, M. Robust LFC for power system considering transmission delay and sampling period. IEEE Trans. Ind. Inform. 2021, 17, 5292–5303. [Google Scholar] [CrossRef]
- Peng, C.; Li, J.; Fei, M. Resilient event-Triggering H∞ LFC for multi-Area power systems with energy-limited DoS attacks. IEEE Trans. Power Syst. 2017, 32, 4110–4118. [Google Scholar] [CrossRef]
- Bevrani, H.; Hiyama, T. Robust decentralised PI based LFC design for time delay power systems. Energy Convers. Manag. 2008, 49, 193–204. [Google Scholar] [CrossRef]
- Ang, K.H.; Chong, G.; Li, Y. PID control system analysis, design, and technology. IEEE Trans. Control Syst. Technol. 2005, 13, 559–576. [Google Scholar]
- Singh, V.P.; Kishor, N.; Samuel, P. Improved LFC of power system using LMI based PID approach. J. Frankl. Inst. 2017, 354, 6805–6830. [Google Scholar] [CrossRef]
- Pradhan, J.K.; Ghosh, A. Multi-input and multi-output PID controller design via linear quadratic regulator-linear matrix inequality approach. IET Control Theory Appl. 2015, 9, 2140–2145. [Google Scholar] [CrossRef]
- Pandey, S.K.; Kishor, N.; Mohanty, S.R. Frequency regulation in hybrid power system using iterative PID H∞ controller. Electr. Power Compon. Syst. 2014, 42, 132–148. [Google Scholar] [CrossRef]
- Zhong, Q.; Yang, J.; Shi, K.; Zhong, S.; Li, Z.; Sotelo, M.A. Event-triggered H∞ LFC for multi-area nonlinear power systems based on non-fragile proportional integral control strategy. IEEE Trans. Intell. Transp. Syst. 2022, 23, 12191–12201. [Google Scholar] [CrossRef]
- Elsisi, M.; Soliman, M. Optimal design of robust resilient automatic voltage regulators. ISA Trans. 2021, 108, 257–268. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Zou, L.; Zhao, Z.; Bai, X. H∞ fuzzy PID control for discrete time-delayed T-S fuzzy systems. Neurocomputing 2019, 332, 91–99. [Google Scholar] [CrossRef]
- Zhao, D.; Wang, Z.; Wei, G.; Han, Q.L. A dynamic event-triggered approach to observer-based PID security control subject to deception attacks. Automatica 2020, 120, 109128. [Google Scholar] [CrossRef]
- Shen, H.; Xing, M.; Xu, S.; Basin, M.V.; Park, J.H. H∞ stabilization of discrete-time nonlinear semi-Markov jump singularly perturbed systems with partially known semi-Markov Kernel information. IEEE Trans. Circuits Syst. I Reg. Pap. 2021, 68, 818–828. [Google Scholar] [CrossRef]
- Feng, Z.; Lam, J.; Gao, H. α-Dissipativity analysis of singular time-delay systems. Automatica 2011, 47, 2548–2552. [Google Scholar] [CrossRef]
- Bullo, F. Averaging and vibrational control of mechanical systems. SIAM J. Control Optim. 2002, 41, 542–562. [Google Scholar] [CrossRef]
- Fantoni, I.; Lozano, R.; Spong, M. Energy based control of the Pendubot. IEEE Trans. Autom. Control 2000, 45, 725–729. [Google Scholar] [CrossRef]
- Wang, Y.; Cheng, D.; Li, C.; Ge, Y. Dissipative Hamiltonian realization and energy-based L2-disturbance attenuation control of multimachine power systems. IEEE Trans. Autom. Control 2003, 48, 1428–1433. [Google Scholar] [CrossRef]
- Galaz, M.; Ortega, R.; Bazanella, A.S.; Stankovic, A.M. An energy-shaping approach to the design of excitation control of synchronous generators. Automatica 2003, 39, 111–119. [Google Scholar] [CrossRef]
- Wu, Z.G.; Shi, P.; Su, H.; Chu, J. Dissipativity Analysis for Discrete-Time Stochastic Neural Networks With Time-Varying Delays. IEEE Trans. Neural Netw. Learn. Syst. 2013, 24, 345–355. [Google Scholar] [CrossRef]
- Wang, J.; Huang, Z.; Wu, Z.; Cao, J.; Shen, H. Extended dissipative control for singularly perturbed PDT switched systems and its application. IEEE Trans. Circuits Syst. I Reg. Pap. 2020, 67, 5281–5289. [Google Scholar] [CrossRef]
- Feng, Z.; Lam, J. Stability and dissipativity analysis of distributed delay cellular neural networks. IEEE Trans. Neural Netw. Learn. Syst. 2011, 22, 976–981. [Google Scholar] [CrossRef]
- Meisami-Azad, M.; Mohammadpour, J.; Grigoriadis, K.M. Dissipative analysis and control of state-space symmetric systems. Automatica 2009, 45, 1574–1579. [Google Scholar] [CrossRef]
- Willems, J.C. Dissipative dynamical systems part I: General theory. Arch. Ration. Mech. Anal. 1972, 45, 321–351. [Google Scholar] [CrossRef]
- Peng, C.; Zhang, J.; Yan, H. Adaptive event-triggering H∞ LFC for network-based power systems. IEEE Trans. Ind. Electron. 2018, 65, 1685–1694. [Google Scholar] [CrossRef]
- Jin, L.; He, Y.; Zhang, C.K.; Shangguan, X.C.; Jiang, L.; Wu, M. Novel structure-exploiting techniques based delay-dependent stability analysis of multi-area LFC with improved numerical tractability. IEEE Trans. Power Syst. 2021, 36, 4194–4211. [Google Scholar] [CrossRef]
- Li, T.; Guo, L.; Sun, C. Robust stability for neural networks with time-varying delays and linear fractional uncertainties. Neurocomputing 2007, 71, 421–427. [Google Scholar] [CrossRef]
- Jin, L.; He, Y.; Zhang, C.K.; Shangguan, X.C.; Jiang, L.; Wu, M. Robust delay-dependent LFC of wind power system based on a novel reconstructed model. IEEE Trans. Cybern. 2021, 52, 7825–7836. [Google Scholar] [CrossRef]
- Crusius, C.; Trofino, A. Sufficient LMI conditions for output feedback control problems. IEEE Trans. Autom. Control 1999, 44, 1053–1057. [Google Scholar] [CrossRef]
- Peng, C.; Zhang, J. Delay-distribution-dependent LFC of power systems with probabilistic interval delays. IEEE Trans. Power Syst. 2016, 31, 3309–3317. [Google Scholar] [CrossRef]


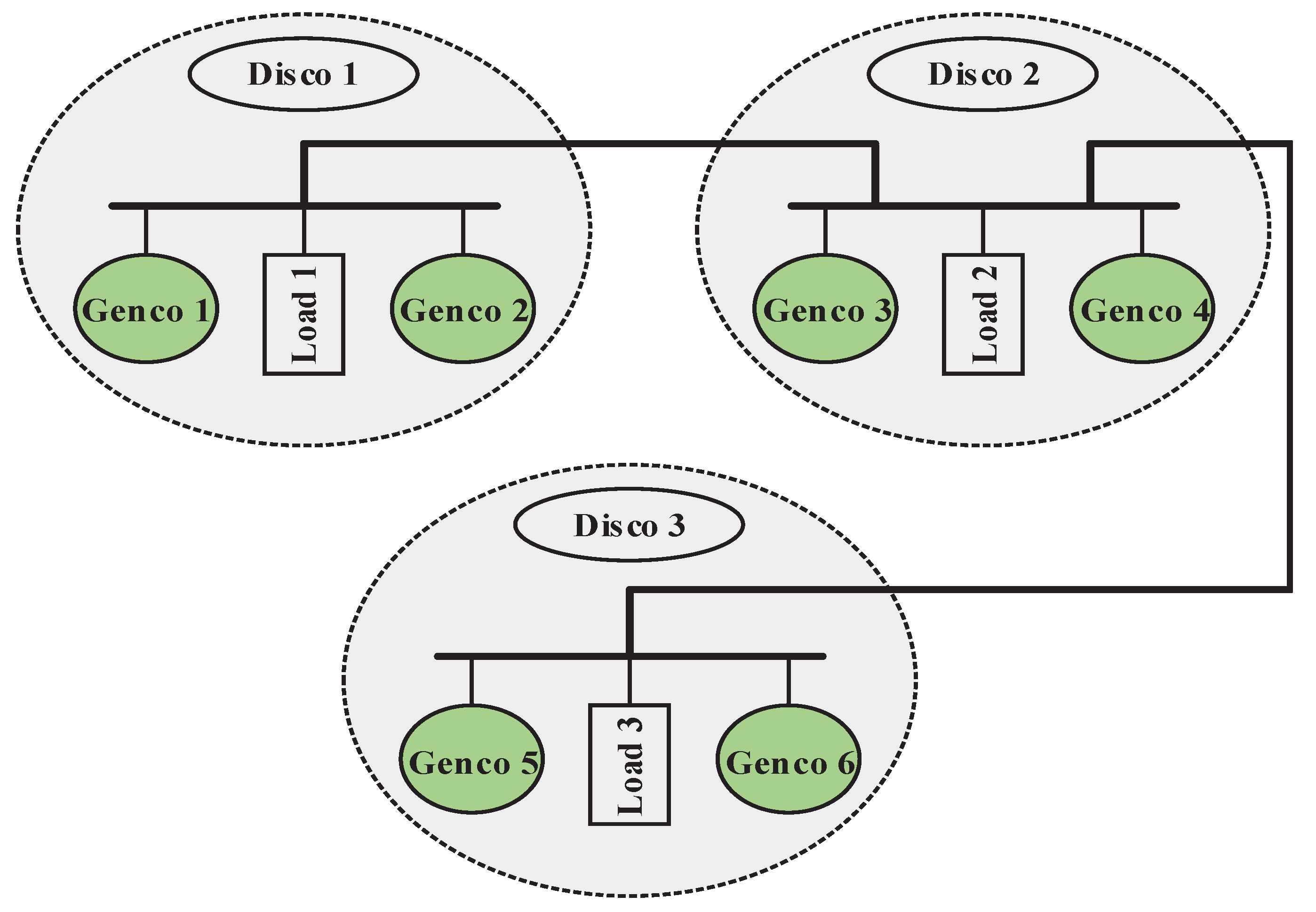

| Speed governor time constants | Total tie-lie power deviation | ||
| Turbine time constants | Turbines mechanical output | ||
| Governor droop characteristic | Load demands | ||
| Load damping coefficient | Deviation of valve position | ||
| Coefficient of tie line | Contracted local demand | ||
| Wind generator time constants | Other contracted demand | ||
| System frequency response coefficient | Governors output | ||
| Inertia constant | Wind generator output | ||
| Participation factors of generator | Area control error |
| Parameters | R | D | M | ||||
|---|---|---|---|---|---|---|---|
| Area 1 | 3.0 | 1.0 | 0.05 | 0.1884 | 21.0 | 0.1667 | 1.0 |
| Area 2 | 4.0 | 1.7 | 0.05 | 0.1884 | 21.5 | 0.2084 | 1.0 |
| Parameters | (k-i: kth Governor in ith Area) | |||||
|---|---|---|---|---|---|---|
| 1-1 | 2-1 | 1-2 | 2-2 | 1-3 | 2-3 | |
| 3.2 | 3 | 3 | 3.2 | 3.1 | 3.4 | |
| 0.6 | 0.8 | 0.6 | 0.7 | 0.8 | 0.6 | |
| R | 2.4 | 2.5 | 2.5 | 2.7 | 2.8 | 2.4 |
| 0.5 | 0.5 | 0.5 | 0.5 | 0.6 | 0.4 | |
| Parameters | Areas | |||||
| 1 | 2 | 3 | ||||
| M | 0.1667 | 0.2084 | 0.1600 | |||
| D | 0.1884 | 0.1884 | 0.1780 | |||
| 0.4250 | 0.3966 | 0.3522 | ||||
| = 0.2450 | = 0.212 | = 0.11 | ||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, H.; Zhong, Q.; Hu, S.; Yang, J.; Shi, K.; Zhong, S. Dissipative Discrete PID Load Frequency Control for Restructured Wind Power Systems via Non-Fragile Design Approach. Mathematics 2023, 11, 3252. https://doi.org/10.3390/math11143252
Zhou H, Zhong Q, Hu S, Yang J, Shi K, Zhong S. Dissipative Discrete PID Load Frequency Control for Restructured Wind Power Systems via Non-Fragile Design Approach. Mathematics. 2023; 11(14):3252. https://doi.org/10.3390/math11143252
Chicago/Turabian StyleZhou, Hanmei, Qishui Zhong, Shaoyu Hu, Jin Yang, Kaibo Shi, and Shouming Zhong. 2023. "Dissipative Discrete PID Load Frequency Control for Restructured Wind Power Systems via Non-Fragile Design Approach" Mathematics 11, no. 14: 3252. https://doi.org/10.3390/math11143252
APA StyleZhou, H., Zhong, Q., Hu, S., Yang, J., Shi, K., & Zhong, S. (2023). Dissipative Discrete PID Load Frequency Control for Restructured Wind Power Systems via Non-Fragile Design Approach. Mathematics, 11(14), 3252. https://doi.org/10.3390/math11143252







