The Delay Time Profile of Multistage Networks with Synchronization
Abstract
1. Introduction
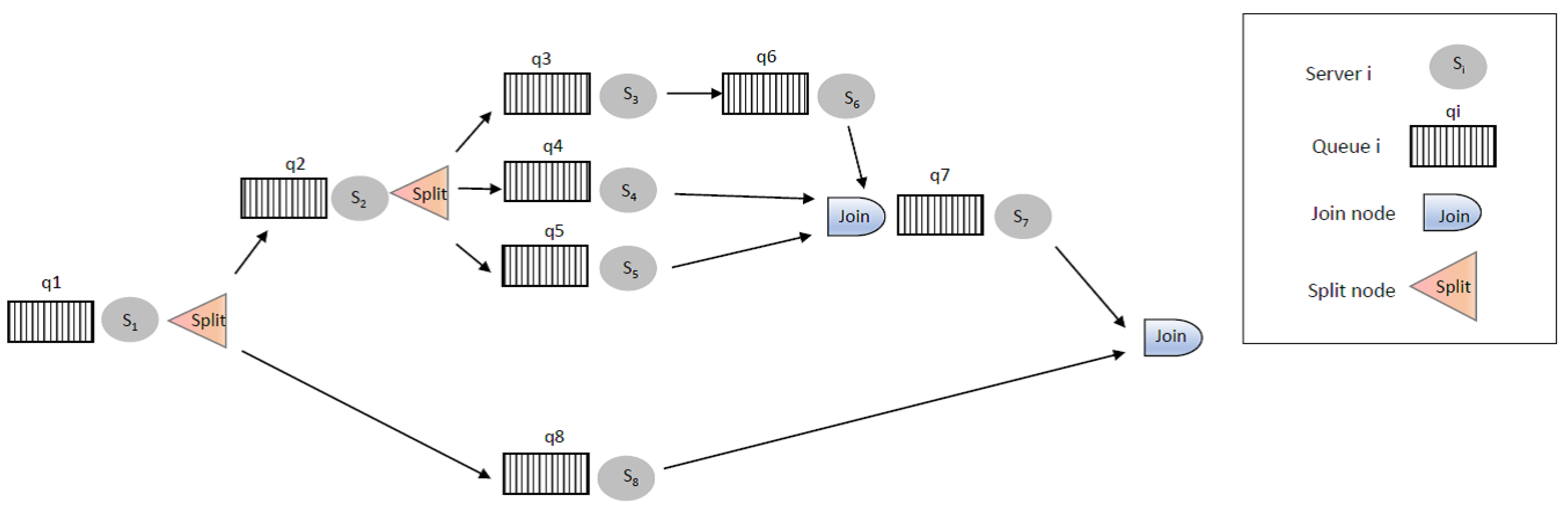
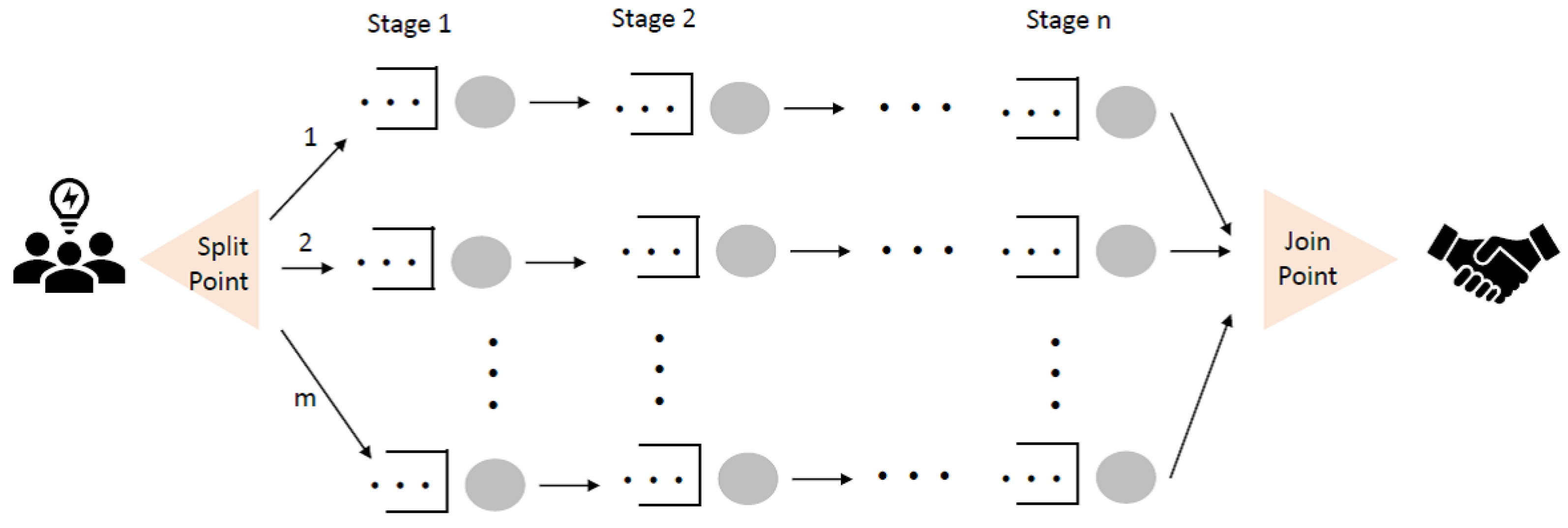
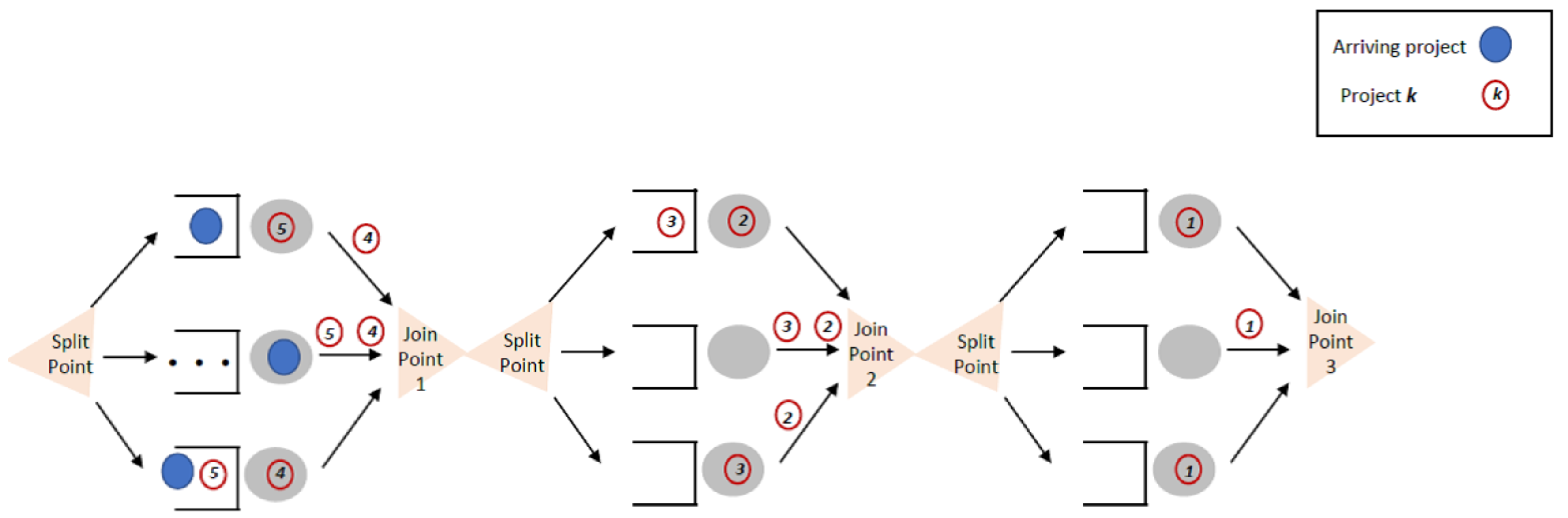
2. Description of the Parallel Split–Join Network
- Let , with be the arrival time of the k-th project. Assume that the arrival process is a renewal process with rate (specifically, this paper focuses on the Poisson process with rate ). Let , be the inter-arrival time between the k-th project and the -th project. Thus, are identical independent (i.i.d) random variables (r.v.s) with average ;
- Upon arrival, each project k is split into m parallel groups. Alternatively, one can think of this as m parallel treatments that the project may simultaneously go through. Each group i, includes n sequential stages/tasks. Completion of stage j, enables the group to continue to stage In what follows, the index i to group , the index j to stage and the double-index to task j in group i are used;
- Each task is allocated independently to its own station. The station is characterized by an infinite queue and a single server. The queue is managed according to FCFS discipline. As a result, a task of project k cannot enter the server before its predecessor tasks of projects ;
- Let be the service time of task of project k. Assume that the times are independent r.v.s in j, and having exponential distribution with rate ;
- A project k is completed when all its groups finish their n stages. Assume that the time of that final synchronization is negligible. Thus, when all groups are finished, they are reunited with no time, and the project immediately leaves the system. Clearly, the FCFS discipline implies that a project cannot leave before its predecessors;
- Let be the waiting time of the project k at station (i.e., the operational delay), and denote by as the sojourn time (i.e., the waiting time plus the service time).
3. Preliminary Results
3.1. Tandem System
- The departure process from the first station (server), which is now also the arrival process of the second station (queue), is a Poisson process with rate , provided that the queue is in equilibrium. This can be achieved if [43];
- Recursively, it is easy to prove that the departure rate from station j, which is now also the arrival process of the subsequent station is a Poisson process with rate provided that ;
- The sojourn times at station j, are mutually independent [44].
3.2. Associated Random Variables
- (i)
- the r.v.s are mutually independent;
- (ii)
- for every the r.v.s and have the same probability function.
4. The Parallel Split–Join System: Analysis and Bounds
5. A Special Case: The Poisson Arrival Process
5.1. Lower and Upper Bounds
- The first lower bound is obtained by neglecting operational queues and assuming an empty system for all arrivals (System 1). In this case, the sojourn time is the maximum of m i.i.d. services, each composed of n exponential stages; i.e., we have m i.i.d. r.v.s Let be the sojourn time in this system. Then,
- The second lower bound is obtained by assuming no splitting into m groups; i.e., (System 2). Let be the sojourn time. It is well known that , and, thus,Comparing Systems 1 and 2 (the lower bounds) to the parallel system highlights the different types of delays. The difference between the performance of System 1 (without operational queues) and the parallel system gives an estimate of the waiting time for a server. Moreover, the difference between the performance of System 2 (without splitting) and the parallel system gives an estimate of the impact of the final synchronization. Obviously, the quality of the bounds depends on the servers’ utilization. When is low, neglecting operational delays is acceptable and, thus, the first bound performs better. However, as increases, the operational delays become significant and the additional delay due to synchronization is negligible; thus, the second bound is preferred;
- An upper bound is obtained by assuming m independent arrival processes of groups (System 3). Applying Burke’s theorem [43] and Conclusions 2 and 3, we obtain that the sojourn times of group are i.i.d. r.v.s. with distribution. Let (the total sojourn time). We obtainClearly, System 3 highlights the impact of the initial interdependency of the m groups. Note that when (i.e., ), and converge to the same limit (intuitively, when the frequency of arrivals is low, there are almost no operational delays, so the effect of joint arrival is negligible, and we see a similar behavior in systems 1 and 3). Since by the Sandwich theorem (Squeeze theorem), these two bounds become tight.
5.2. Asymptotic Analysis for Large n and m
5.3. The Efficiency of the Bounds
- (ii)
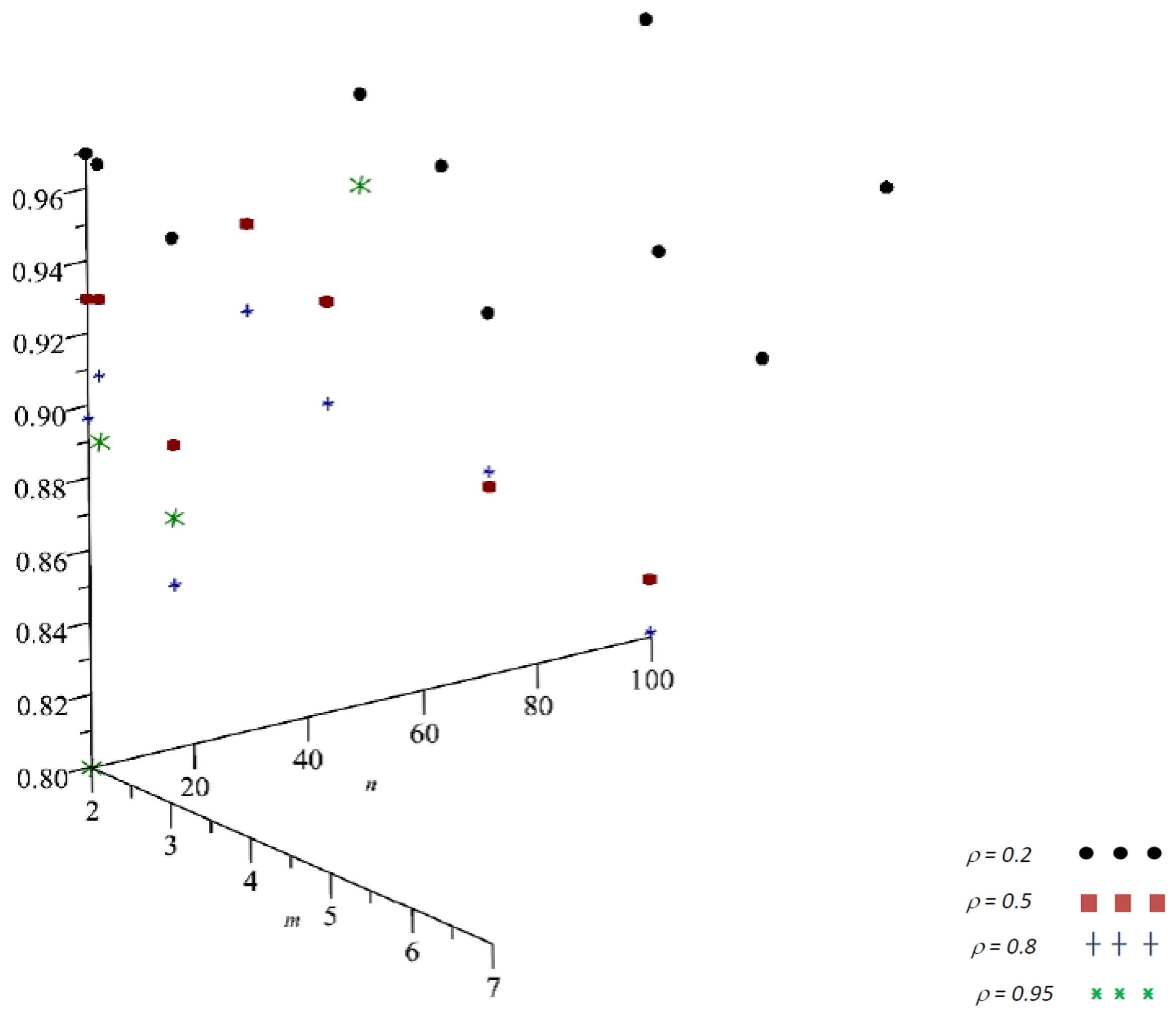
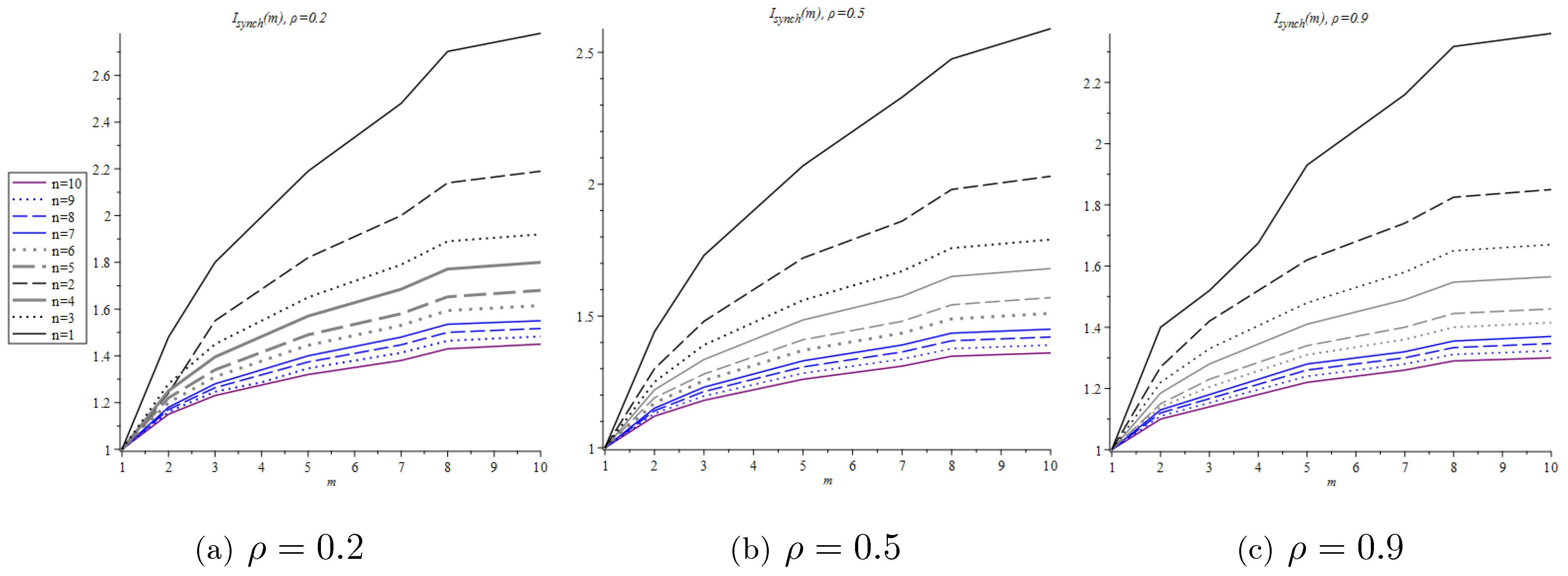
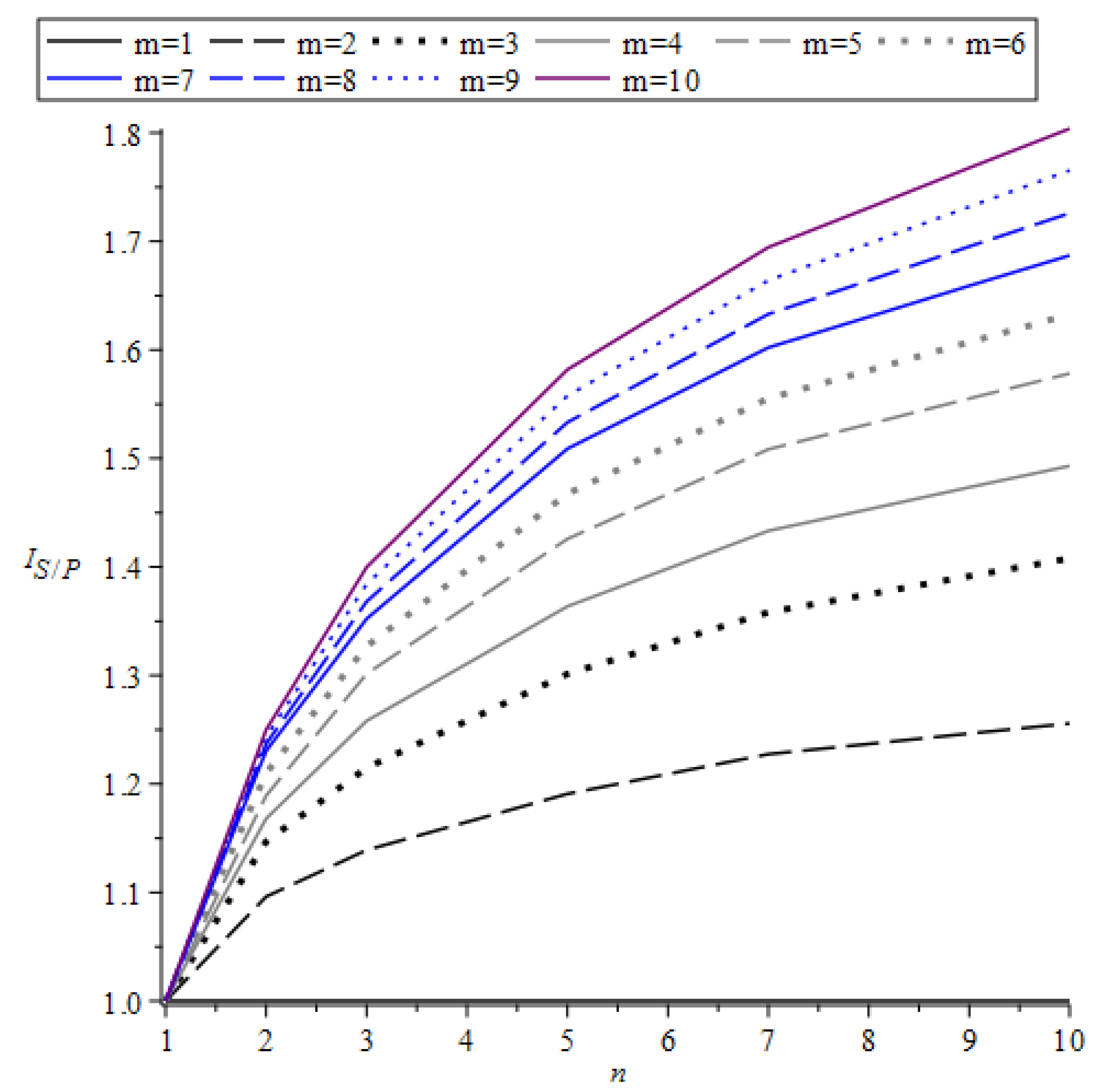
- The effect of the number of stages Clearly, as n increases, the effect of the joint arrival fades. This is particularly evident in , which is increasing in Regarding we would expect to see a decrease in since increasing n further yields more servers and queues. However, Figure 6 and additional results (that are not reported here) imply that the changes in are inconsistent, probably since increasing n adds variability that blurs the differences between the groups and yields inconsistency;
- (iii)
- In fact, Figure 5 and Figure 6 show that the changes in and are relatively low in m and n. This can be explained by the fact that both and grow in m and n at the same rate (in orders and , respectively) and, thus, also changes at the same rate (which is consistent with the results in Section 5.2);
- (iv)
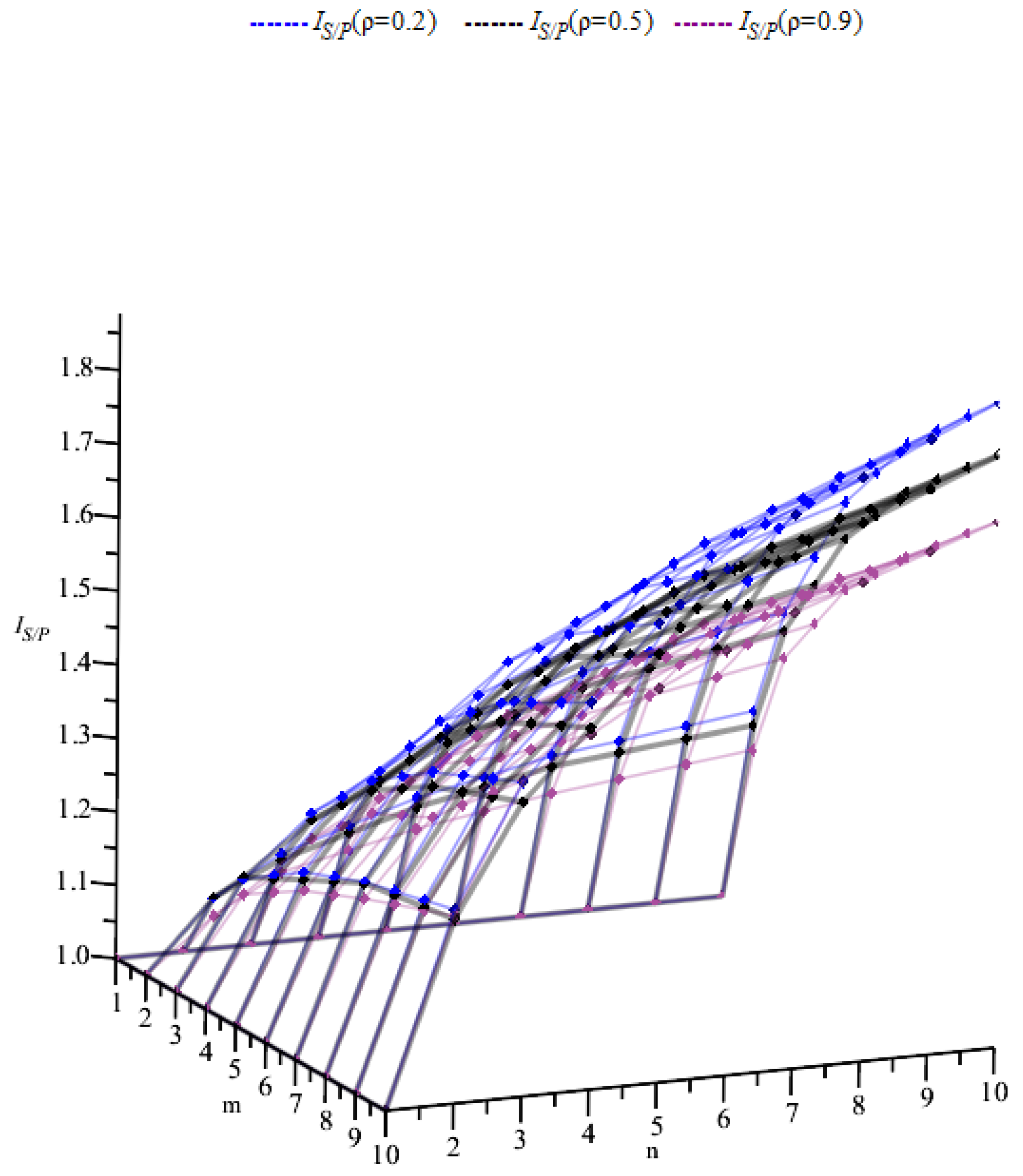
- The effect of the utilization ρ. The lower bound assumes only one project with no operational queues. Clearly, when ρ is low, the lower bound becomes tighter (see the black circles in Figure 6). However, when projects arrive more frequently, and ρ increases, drops sharply. By contrast, the changes in are inconsistent, and, although slightly decreases in ρ, its efficiency is quite high for most values of ρ.
5.4. Simulation Study
5.4.1. The Influence of the Synchronization Delay
- (ii)
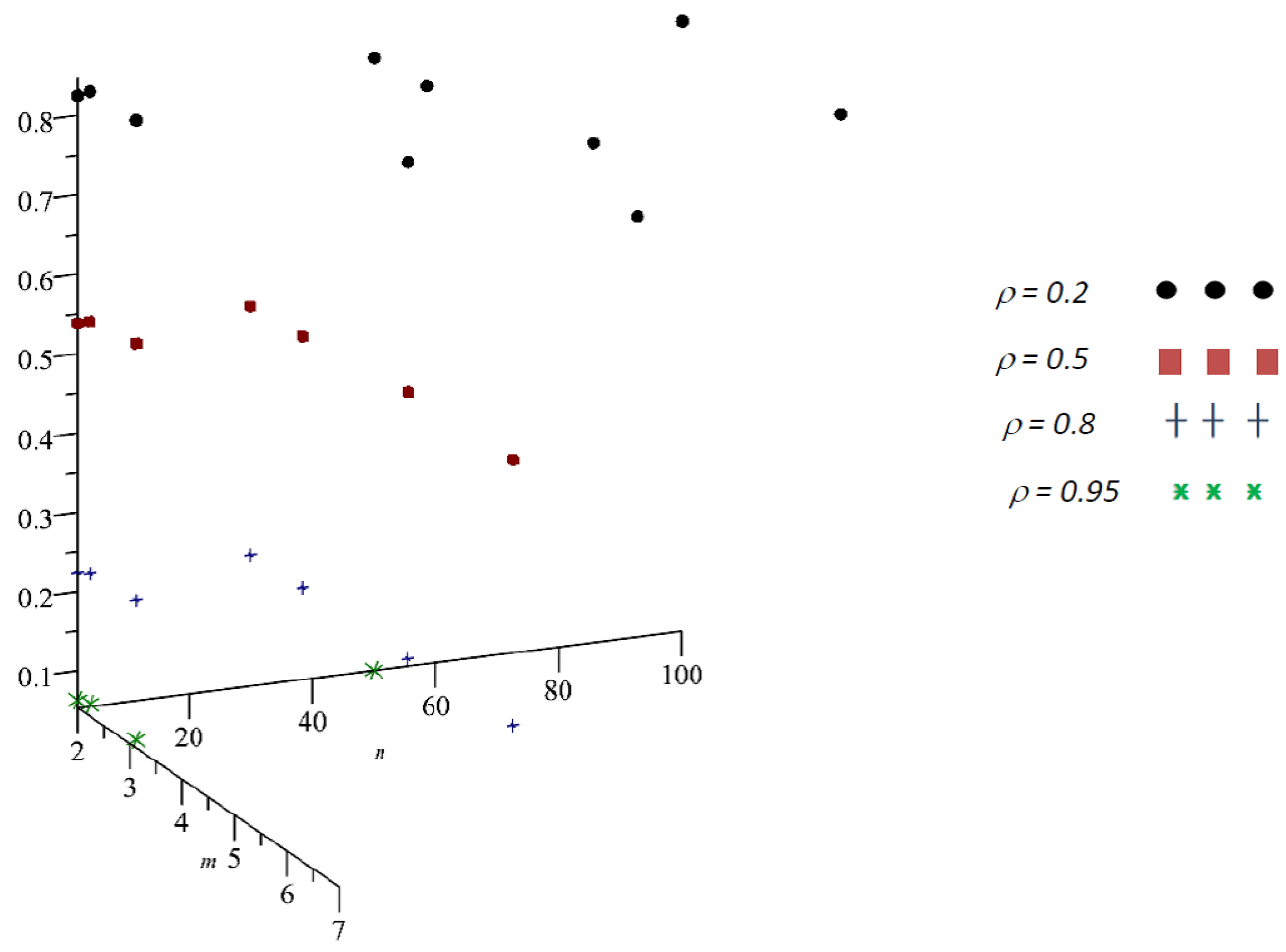
- Increasing n decreases for fixed m, and decreases the growth rate of in m. This can be explained by the fact that increasing n increases the number of servers and the operational delays. In this case, the time required for the final synchronization takes a relatively small fraction of the total sojourn time. As a result, the difference between and is reduced, and decreases. We can further add that increasing n causes the system to be more deterministic, since each group behaves as an distributed r.v. with coefficient of variation which is decreasing in Thus, the final synchronization has less effect. Turning to the edge case, consider that In this case, the total sojourn time at each group is deterministic and equal to the sojourn time of other groups; statistically, there is no difference between the groups, and, thus, ;
- (iii)
- Comparing Figure 7a–c shows that is slightly decreasing in ρ. As discussed above, as the system becomes overloaded, more time is spent in waiting for servers rather than in synchronization. However, contrary to expectation, the results show that is hardly affected by and the changes are quite negligible. Here, too, consider the edge case where almost no operational delays exist but only the final synchronization. In this case, the differences between the systems are significant, and increases to its maximum value.
5.4.2. The Influence of Multiple Stages
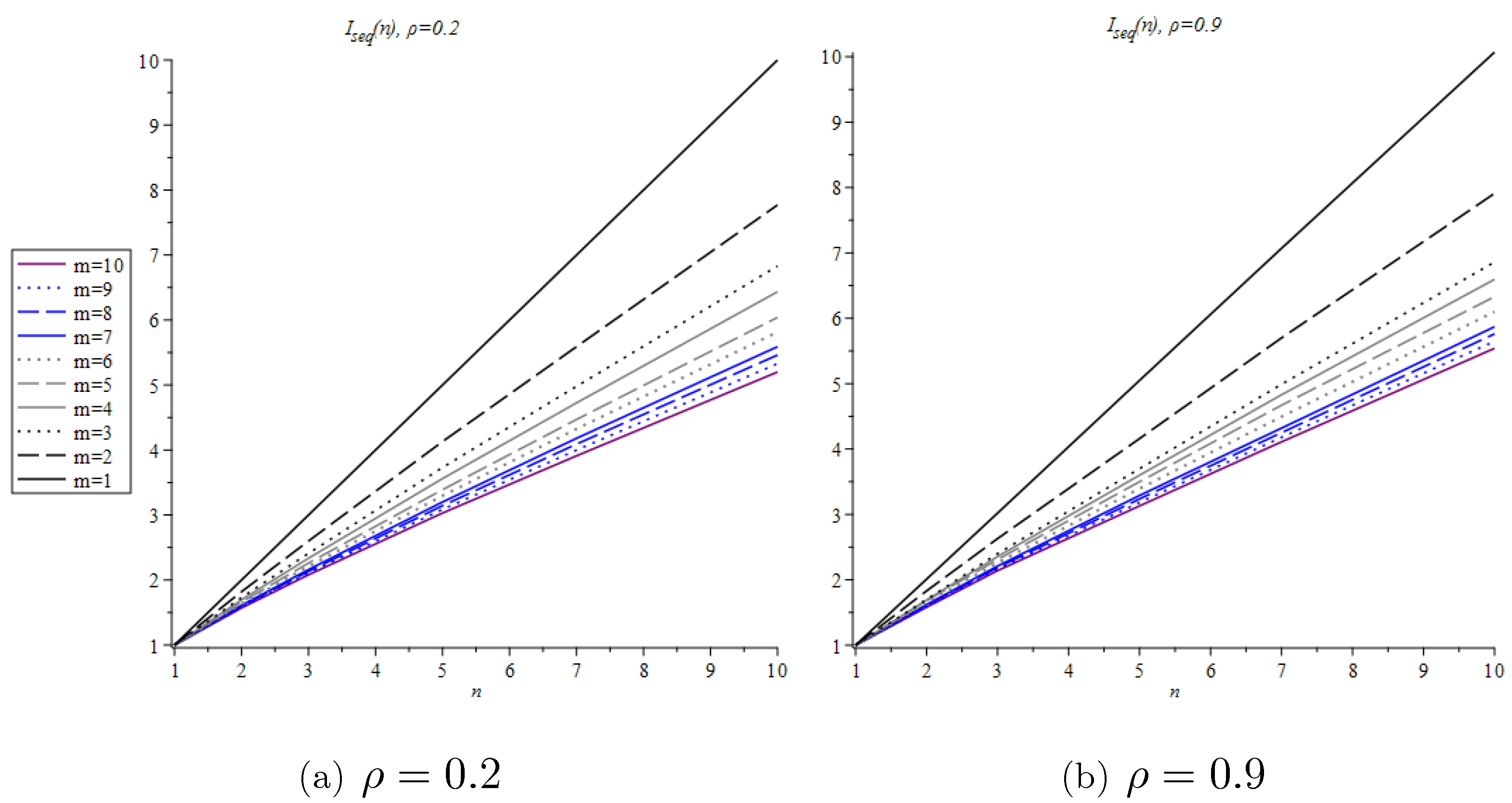
- (ii)
- Increasing m both decreases for fixed n and decreases the growth rate of in n. Obviously, the reason lies in the fact that increasing m decreases the relative weight of the operational delays compared to the synchronization delay, and so decreases;
- (iii)
- We further see that is hardly affected by ρ. This can be explained by the fact that changes in the utilization have a similar effect on all servers. Therefore, there is a negligible dependence between ρ and .
6. The Serial Split–Join System
6.1. The Influence of Synchronization Overhead and Stages
- (ii)
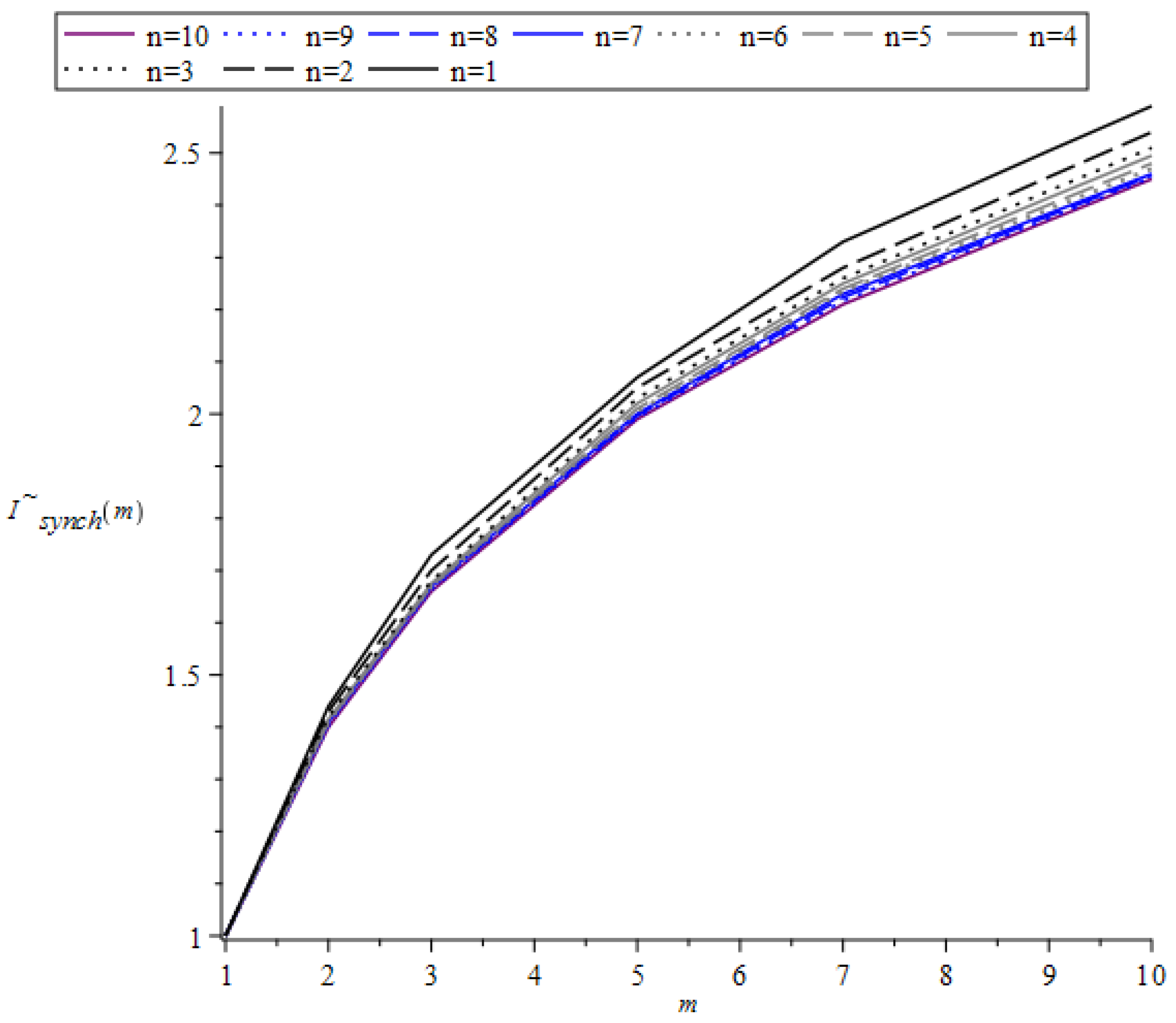
- We see that is hardly affected by n when m is fixed, and, similarly, is hardly affected by m when n is fixed. This can be explained by the synchronization constraints needed at the end of each stage (which is the beginning of the next stage). Specifically, the variability obtained between the different groups does not accumulate, and each stage is almost identical to the first stage. This absence of dispersion aggregation causes to be almost independent in n, and ;
- (iii)
6.2. Comparison of the Systems
- (ii)
- The impact of In most cases, increasing ρ decreases for fixed n and m. The explanation is simple: for a low utilization, the operational queues are almost empty and, thus, synchronization times (which exist mainly in the serial system) constitute the key share of the total time. Here, the serial system is significantly slower than the parallel system and, thus, increases. However, for an overloaded system (with a high utilization), the operational delays increase in both systems, thereby offsetting the synchronization delays that slightly decrease In summary, the effect of efficiency is significant mainly for high n and m and for a low utilization. In this case, the large number of synchronizations becomes a major component in the time profile.
7. Concluding Remarks and Future Research
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. The Proof of Claim 1
Appendix B. The Proof of Claim 2(1)
References
- Alesawi, S.; Ghanem, S. Overcome heterogeneity impact in modeled fork-join queuing networks for tail prediction. In Proceedings of the 2019 International Conference on Computing, Networking and Communications (ICNC), IEEE, Honolulu, HI, USA, 18–21 February 2019; pp. 270–275. [Google Scholar]
- Gorbunova, A.; Vishnevsky, V. The analysis of big data centers performance. Adv. Syst. Sci. Appl. 2022, 22, 70–83. [Google Scholar]
- Nguyen, M.; Alesawi, S.; Li, N.; Che, H.; Jiang, H. A black-box fork-join latency prediction model for data-intensive applications. IEEE Trans. Parallel Distrib. Syst. 2020, 31, 1983–2000. [Google Scholar] [CrossRef]
- Ardagna, D.; Bernardi, S.; Gianniti, E.; Karimian Aliabadi, S.; Perez-Palacin, D.; Requeno, J.I. Modeling performance of hadoop applications: A journey from queueing networks to stochastic well formed nets. In Proceedings of the Algorithms and Architectures for Parallel Processing: 16th International Conference, ICA3PP 2016, Granada, Spain, 14–16 December 2016; Springer International Publishing: Berlin/Heidelberg, Germany, 2016. Proceedings 15. pp. 599–613. [Google Scholar]
- Delias, P.; Lagopoulos, A.; Tsoumakas, G.; Grigori, D. Using multi-target feature evaluation to discover factors that affect business process behavior. Comput. Ind. 2018, 99, 253–261. [Google Scholar] [CrossRef]
- Sethuraman, S. Analysis of Fork-Join Systems: Network of Queues with Precedence Constraints; CRC Press: Boca Raton, FL, USA, 2022. [Google Scholar]
- Enganti, P.; Rosenkrantz, T.; Sun, L.; Wang, Z.; Che, H.; Jiang, H. ForkMV: Mean-and-variance estimation of fork-join queuing networks for datacenter applications. In Proceedings of the 2022 IEEE International Conference on Networking, Architecture and Storage (NAS), IEEE, Philadelphia, PA, USA, 3–4 October 2022; pp. 1–8. [Google Scholar]
- Wang, W.; Harchol-Balter, M.; Jiang, H.; Scheller-Wolf, A.; Srikant, R. Delay asymptotics and bounds for multi-task parallel jobs. ACM Sigmetrics Perform. Eval. Rev. 2019, 46, 2–7. [Google Scholar] [CrossRef]
- Ding, S. Multi-Class Fork-Join Queues & The Stochastic Knapsack Problem. Ph.D. Thesis, Universiteit Leiden, Amsterdam, The Netherlands, 2011. [Google Scholar]
- Krishnamurthy, A.; Suri, R. Performance analysis of single stage kanban controlled production systems using parametric decomposition. Queueing Syst. 2006, 54, 141–162. [Google Scholar] [CrossRef]
- Shaaban, S.; Romero-Silva, R. Performance of merging lines with uneven buffer capacity allocation: The effects of unreliability under different inventory-related costs. Cent. Eur. J. Oper. Res. 2021, 29, 1253–1288. [Google Scholar] [CrossRef]
- Matta, A.; Dallery, Y.; Di Mascolo, M. Analysis of assembly systems controlled with kanbans. Eur. J. Oper. Res. 2005, 166, 310–336. [Google Scholar] [CrossRef]
- Raghavan, N.S.; Viswanadham, N. Generalized queueing network analysis of integrated supply chains. Int. J. Prod. Res. 2001, 39, 205–224. [Google Scholar]
- Atar, R.; Mandelbaum, A.; Zviran, A. Control of fork-join networks in heavy traffic. In Proceedings of the 2012 50th Annual Allerton Conference on Communication, Control, and Computing (Allerton), IEEE, Monticello, IL, USA, 1–5 October 2012; pp. 823–830. [Google Scholar]
- Özkan, E. Control of fork-join processing networks with multiple job types and parallel shared resources. Math. Oper. Res. 2022, 47, 1310–1334. [Google Scholar] [CrossRef]
- Prabhakar, B.; Bambos, N.; Mountford, T.S. The synchronization of Poisson processes and queueing networks with service and synchronization nodes. Adv. Appl. Probab. 2000, 32, 824–843. [Google Scholar] [CrossRef]
- Ramakrishnan, R.; Krishnamurthy, A. Analysis of kitting Operations in manufacturing systems. Asia Pac. J. Oper. Res. 2008, 25, 187–216. [Google Scholar] [CrossRef]
- Ramakrishnan, R.; Krishnamurthy, A. Performance evaluation of a synchronization station with multiple inputs and population constraints. Comput. Oper. Res. 2012, 39, 560–570. [Google Scholar] [CrossRef]
- Schol, D.; Vlasiou, M.; Zwart, B. Large fork-join networks with nearly deterministic service times. Mathematics 2019, arXiv:1912.11661. [Google Scholar]
- Roy, D.; van Ommeren, J.K.; de Koster, R.; Gharehgozli, A. Modeling landside container terminal queues: Exact analysis and approximations. Transp. Res. Part B Methodol. 2022, 162, 73–102. [Google Scholar] [CrossRef]
- Towsley, D.; Rommel, C.G.; Stankovic, J.A. Analysis of fork-join program response times on multiprocessors. IEEE Trans. Parallel Distrib. Syst. 1990, 1, 286–303. [Google Scholar] [CrossRef] [PubMed]
- Baccelli, F.; Makowski, A.M. Simple Computable Bounds for the Fork-Join Queue. Ph.D. Thesis, INRIA, Le Chesnay-Rocquencourt, France, 1985. [Google Scholar]
- Baccelli, F.; Makowski, A.M. Queueing models for systems with synchronization constraints. Proc. IEEE 1989, 77, 138–161. [Google Scholar] [CrossRef]
- Baccelli, F.; Makowski, A.M.; Shwartz, A. The fork-join queue and related systems with synchronization constrains: Stochastic Ordering and Computable Bounds. Adv. Appl. Probab. 1989, 21, 629–660. [Google Scholar] [CrossRef]
- Baccelli, F.; Massey, W.A.; Towsley, D. Acyclic fork join queuing networks. J. Assoc. Comput. Machanics 1989, 36, 615–642. [Google Scholar] [CrossRef]
- Ko, S.S.; Serfozo, R.F. Response times in M/M/s fork-join networks. Adv. Appl. Probab. 2004, 36, 854–871. [Google Scholar] [CrossRef]
- Ko, S.S.; Serfozo, R.F. Sojourn Times in G/M/1 fork-join networks. Nav. Res. Logist. 2008, 55, 432–443. [Google Scholar] [CrossRef]
- Varki, E. Mean value technique for closed fork-join networks. Perform. Eval. Rev. 1999, 27, 103–112. [Google Scholar] [CrossRef]
- Ko, S.S. Cycle times in a serial fork-join network. In Proceedings of the Computational Science and Its Applications–ICCSA 2007: International Conference, Kuala Lumpur, Malaysia, 26–29 August 2007; Springer: Berlin/Heidelberg, Germany, 2007. Proceedings, Part I 7. pp. 758–766. [Google Scholar]
- Nelson, R.; Tantawi, A.N. Approximation analysis of Fork/Join synchronization in parallel queues. IEEE Trans. Comput. 1988, 37, 739–743. [Google Scholar] [CrossRef]
- Nelson, R.; Towsley, D.; Tantawi, A.N. Performance analysis of parallel processing systems. IEEE Trans. Softw. Eng. 1988, 14, 532–540. [Google Scholar] [CrossRef]
- Fiorini, P.M. Analytic approximations of fork-join queues. In Proceedings of the 2015 IEEE 8th International Conference on Intelligent Data Acquisition and Advanced Computing Systems: Technology and Applications (IDAACS), IEEE, Warsaw, Poland, 24–26 September 2015; Volume 2, pp. 966–971. [Google Scholar]
- Lebrecht, A.S.; Knottenbelt, W.J. Response time approximations in fork-join queues. In Proceedings of the 23rd Annual UK Performance Engineering Workshop (UKPEW 2007), ORMS, Kirk, UK, 31 July 2007. [Google Scholar]
- Kemper, B.; Mandjes, M. Mean sojourn times in two-queue fork-join systems: Bounds and approximations. OR Spectr. 2012, 34, 723–742. [Google Scholar] [CrossRef]
- Takahashi, M.; Osawa, H.; Fujisawa, T. On a synchronization queue with two finite buffers. Queueing Syst. 2000, 36, 107–123. [Google Scholar] [CrossRef]
- Qiu, Z.; Pérez, J.F.; Harrison, P.G. Beyond the mean in fork-join queues: Efficient approximation for response-time tails. Perform. Eval. 2015, 91, 99–116. [Google Scholar] [CrossRef]
- Varma, S.; Makowski, A.M. Interpolation approximations for symmetric fork-join queues. J. Perform. Eval. 1994, 20, 245–265. [Google Scholar] [CrossRef]
- Tan, X.; Knessl, C. A fork-join queueuing model:diffusion approximation, integral representations and asymptotics. Queueing Syst. 1996, 22, 287–332. [Google Scholar] [CrossRef]
- Knessl, C. A diffusion model for two parallel queues with processor sharing: Transient behavior and asymptotics. J. Appl. Math. Stoch. Anal. 1999, 12, 311–338. [Google Scholar] [CrossRef]
- Kushner, H.J. Heavy Traffic Analysis of Controlled Queueing and Communication Networks; Springer: New York, NY, USA, 2001. [Google Scholar]
- Zeng, Y.; Tan, J.; Xia, C.H. Fork and join queueing networks with heavy tails: Scaling dimension and throughput limit. J. ACM (JACM) 2021, 68, 1–30. [Google Scholar] [CrossRef]
- Meijer, M.S.; Schol, D.; van Jaarsveld, W.; Vlasiou, M.; Zwart, B. Extreme-value theory for large fork-join queues, with an application to high-tech supply chains. arXiv 2021, arXiv:2105.09189. [Google Scholar]
- Burke, P.J. The output process of a stationary M/M/s queueing system. Ann. Math. Stat. 1968, 39, 1144–1152. [Google Scholar] [CrossRef]
- Walrand, J. An Introduction to Queueing Networks; Caopter 4; Prentice Hall: Hoboken, NJ, USA, 1988. [Google Scholar]
- Latouche, G.; Ramaswami, V. Introduction to Matrix Analytic Methods in Stochastic Modeling; SIAM: Philadelphia, PA, USA, 1999. [Google Scholar]
- Lindley, D.V. The theory of queues with a single server. In Mathematical Proceedings of the Cambridge Philosophical Society; Cambridge University Press: Cambridge, UK, 1952; Volume 48, pp. 277–289. [Google Scholar]
- Kang, S.; Serfozo, R.F. Extreme values of phase-type and mixed random variables with parallel-processing examples. J. Appl. Probab. 1999, 36, 194–210. [Google Scholar] [CrossRef]
- Cremonesi, P.; Turrin, R.; Alexandrov, V.N. Modeling the effects of node heterogeneity on the performance of grid applications. J. Netw. 2009, 4, 837–854. [Google Scholar] [CrossRef]






| The Efficiency of the Bound | |||
|---|---|---|---|
| ↓ | ↑ | ↓ | |
| ↑ | ↕ | ⇓ |
| Isynch(m) | n | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 1.4800 | 1.8000 | 1.9950 | 2.1900 | 2.3350 | 2.4800 | 2.7025 | 2.7413 | 2.7800 | |
| 2 | 1.3400 | 1.5500 | 1.6850 | 1.8200 | 1.9100 | 2.0000 | 2.1400 | 2.1650 | 2.1900 | |
| 3 | 1.2800 | 1.4500 | 1.5500 | 1.6500 | 1.7200 | 1.7900 | 1.8900 | 1.9050 | 1.9200 | |
| 4 | 1.2500 | 1.3950 | 1.4825 | 1.5700 | 1.6275 | 1.6850 | 1.7713 | 1.7856 | 1.8000 | |
| = 0.2 | 5 | 1.2200 | 1.3400 | 1.4150 | 1.4900 | 1.5350 | 1.5800 | 1.6525 | 1.6663 | 1.6800 |
| 6 | 1.2000 | 1.3100 | 1.3775 | 1.4450 | 1.4875 | 1.5300 | 1.5938 | 1.6044 | 1.6150 | |
| 7 | 1.1800 | 1.2800 | 1.3400 | 1.4000 | 1.4400 | 1.4800 | 1.5350 | 1.5425 | 1.5500 | |
| 8 | 1.1701 | 1.2635 | 1.3186 | 1.3736 | 1.4103 | 1.4470 | 1.5004 | 1.5087 | 1.5170 | |
| 9 | 1.1599 | 1.2465 | 1.2965 | 1.3464 | 1.3797 | 1.4130 | 1.4647 | 1.4738 | 1.4830 | |
| 10 | 1.1500 | 1.2300 | 1.2750 | 1.3200 | 1.3500 | 1.3800 | 1.4300 | 1.4400 | 1.4500 | |
| 1 | 1.4500 | 1.7400 | 1.9300 | 2.1200 | 2.2450 | 2.3700 | 2.5775 | 2.6188 | 2.6600 | |
| 2 | 1.3200 | 1.5100 | 1.6300 | 1.7500 | 1.8300 | 1.9100 | 2.0300 | 2.0500 | 2.0700 | |
| 3 | 1.2500 | 1.4100 | 1.5000 | 1.5900 | 1.6450 | 1.7000 | 1.7925 | 1.8113 | 1.8300 | |
| 4 | 1.2150 | 1.3550 | 1.4300 | 1.5050 | 1.5550 | 1.6050 | 1.6850 | 1.7000 | 1.7150 | |
| = 0.4 | 5 | 1.1800 | 1.3000 | 1.3600 | 1.4200 | 1.4650 | 1.5100 | 1.5775 | 1.5888 | 1.6000 |
| 6 | 1.1700 | 1.2700 | 1.3275 | 1.3850 | 1.4225 | 1.4600 | 1.5188 | 1.5294 | 1.5400 | |
| 7 | 1.1600 | 1.2400 | 1.2950 | 1.3500 | 1.3800 | 1.4100 | 1.4600 | 1.4700 | 1.4800 | |
| 8 | 1.1468 | 1.2235 | 1.2752 | 1.3269 | 1.3553 | 1.3836 | 1.4295 | 1.4382 | 1.4470 | |
| 9 | 1.1332 | 1.2065 | 1.2548 | 1.3031 | 1.3298 | 1.3564 | 1.3980 | 1.4055 | 1.4130 | |
| 10 | 1.1200 | 1.1900 | 1.2350 | 1.2800 | 1.3050 | 1.3300 | 1.3675 | 1.3738 | 1.3800 | |
| 1 | 1.4400 | 1.7300 | 1.9000 | 2.0700 | 2.2000 | 2.3300 | 2.4750 | 2.5325 | 2.5900 | |
| 2 | 1.3000 | 1.4800 | 1.6000 | 1.7200 | 1.7900 | 1.8600 | 1.9800 | 2.0050 | 2.0300 | |
| 3 | 1.2500 | 1.3900 | 1.4750 | 1.5600 | 1.6150 | 1.6700 | 1.7575 | 1.7738 | 1.7900 | |
| 4 | 1.2200 | 1.3350 | 1.4100 | 1.4850 | 1.5300 | 1.5750 | 1.6500 | 1.6650 | 1.6800 | |
| = 0.5 | 5 | 1.1900 | 1.2800 | 1.3450 | 1.4100 | 1.4450 | 1.4800 | 1.5425 | 1.5563 | 1.5700 |
| 6 | 1.1700 | 1.2550 | 1.3125 | 1.3700 | 1.4025 | 1.4350 | 1.4888 | 1.4994 | 1.5100 | |
| 7 | 1.1500 | 1.2300 | 1.2800 | 1.3300 | 1.3600 | 1.3900 | 1.4350 | 1.4425 | 1.4500 | |
| 8 | 1.1401 | 1.2135 | 1.2602 | 1.3069 | 1.3353 | 1.3636 | 1.4061 | 1.4132 | 1.4203 | |
| 9 | 1.1299 | 1.1965 | 1.2398 | 1.2831 | 1.3098 | 1.3364 | 1.3764 | 1.3830 | 1.3897 | |
| 10 | 1.1200 | 1.1800 | 1.2200 | 1.2600 | 1.2850 | 1.3100 | 1.3475 | 1.3538 | 1.3600 | |
| 1 | 1.4100 | 1.6800 | 1.8200 | 1.9600 | 2.0900 | 2.2200 | 2.3950 | 2.4175 | 2.4400 | |
| 2 | 1.2700 | 1.4400 | 1.4400 | 1.4400 | 1.6000 | 1.7600 | 1.9100 | 1.9050 | 1.9000 | |
| 3 | 1.2100 | 1.3500 | 1.3500 | 1.3500 | 1.4750 | 1.6000 | 1.7225 | 1.7213 | 1.7200 | |
| 4 | 1.1800 | 1.3050 | 1.3050 | 1.3050 | 1.4075 | 1.5100 | 1.6088 | 1.6069 | 1.6050 | |
| = 0.8 | 5 | 1.1500 | 1.2600 | 1.2600 | 1.2600 | 1.3400 | 1.4200 | 1.4950 | 1.4925 | 1.4900 |
| 6 | 1.1350 | 1.2300 | 1.2300 | 1.2300 | 1.3025 | 1.3750 | 1.4438 | 1.4419 | 1.4400 | |
| 7 | 1.1200 | 1.2000 | 1.2000 | 1.2000 | 1.2650 | 1.3300 | 1.3925 | 1.3913 | 1.3900 | |
| 8 | 1.1134 | 1.1868 | 1.1868 | 1.1868 | 1.2485 | 1.3102 | 1.3661 | 1.3632 | 1.3603 | |
| 9 | 1.1066 | 1.1732 | 1.1732 | 1.1732 | 1.2315 | 1.2898 | 1.3389 | 1.3343 | 1.3297 | |
| 10 | 1.1000 | 1.1600 | 1.1600 | 1.1600 | 1.2150 | 1.2700 | 1.3125 | 1.3063 | 1.3000 | |
| 1 | 1.4000 | 1.4200 | 1.6750 | 1.9300 | 2.0450 | 2.1600 | 2.3175 | 2.3388 | 2.3600 | |
| 2 | 1.2700 | 1.4200 | 1.5200 | 1.6200 | 1.6800 | 1.7400 | 1.8250 | 1.8375 | 1.8500 | |
| 3 | 1.2200 | 1.3300 | 1.4050 | 1.4800 | 1.5300 | 1.5800 | 1.6500 | 1.6600 | 1.6700 | |
| 4 | 1.1850 | 1.2800 | 1.3450 | 1.4100 | 1.4500 | 1.4900 | 1.5475 | 1.5563 | 1.5650 | |
| = 0.9 | 5 | 1.1500 | 1.2300 | 1.2850 | 1.3400 | 1.3700 | 1.4000 | 1.4450 | 1.4525 | 1.4600 |
| 6 | 1.1400 | 1.2050 | 1.2575 | 1.3100 | 1.3350 | 1.3600 | 1.4000 | 1.4075 | 1.4150 | |
| 7 | 1.1300 | 1.1800 | 1.2300 | 1.2800 | 1.3000 | 1.3200 | 1.3550 | 1.3625 | 1.3700 | |
| 8 | 1.1201 | 1.1668 | 1.2135 | 1.2602 | 1.2802 | 1.3002 | 1.3336 | 1.3402 | 1.3469 | |
| 9 | 1.1099 | 1.1532 | 1.1965 | 1.2398 | 1.2598 | 1.2798 | 1.3115 | 1.3173 | 1.3231 | |
| 10 | 1.1000 | 1.1400 | 1.1800 | 1.2200 | 1.2400 | 1.2600 | 1.2900 | 1.2950 | 1.3000 |
| Iseq(n) | m | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 2.0000 | 3.0000 | 4.0000 | 5.0000 | 6.0000 | 7.0000 | 8.0000 | 9.0000 | 10.0000 | |
| 2 | 1.8200 | 2.6000 | 3.3650 | 4.1300 | 4.8600 | 5.5900 | 6.3167 | 7.0433 | 7.7700 | |
| 3 | 1.7300 | 2.4100 | 3.0700 | 3.7300 | 4.3550 | 4.9800 | 5.5967 | 6.2133 | 6.8300 | |
| 4 | 1.6950 | 2.3350 | 2.9475 | 3.5600 | 4.1425 | 4.7250 | 5.2950 | 5.8650 | 6.4350 | |
| = 0.2 | 5 | 1.6600 | 2.2600 | 2.8250 | 3.3900 | 3.9300 | 4.4700 | 4.9933 | 5.5167 | 6.0400 |
| 6 | 1.6350 | 2.2100 | 2.7525 | 3.2950 | 3.8100 | 4.3250 | 4.8217 | 5.3183 | 5.8150 | |
| 7 | 1.6100 | 2.1600 | 2.6800 | 3.2000 | 3.6900 | 4.1800 | 4.6500 | 5.1200 | 5.5900 | |
| 8 | 1.5968 | 2.1336 | 2.6388 | 3.1439 | 3.6174 | 4.0909 | 4.5477 | 5.0045 | 5.4613 | |
| 9 | 1.5832 | 2.1064 | 2.5963 | 3.0861 | 3.5426 | 3.9991 | 4.4423 | 4.8855 | 5.3287 | |
| 10 | 1.5700 | 2.0800 | 2.5550 | 3.0300 | 3.4700 | 3.9100 | 4.3400 | 4.7700 | 5.2000 | |
| 1 | 2.0000 | 3.0100 | 4.0150 | 5.0200 | 6.0200 | 7.0200 | 8.0200 | 9.0200 | 10.0200 | |
| 2 | 1.8200 | 2.5900 | 3.3300 | 4.0700 | 4.8300 | 5.5900 | 6.3100 | 7.0300 | 7.7500 | |
| 3 | 1.7300 | 2.4300 | 3.0850 | 3.7400 | 4.3750 | 5.0100 | 5.6267 | 6.2433 | 6.8600 | |
| 4 | 1.6900 | 2.3400 | 2.9450 | 3.5500 | 4.1400 | 4.7300 | 5.3017 | 5.8733 | 6.4450 | |
| = 0.4 | 5 | 1.6500 | 2.2500 | 2.8050 | 3.3600 | 3.9050 | 4.4500 | 4.9767 | 5.5033 | 6.0300 |
| 6 | 1.6300 | 2.2000 | 2.7375 | 3.2750 | 3.7950 | 4.3150 | 4.8167 | 5.3183 | 5.8200 | |
| 7 | 1.6100 | 2.1500 | 2.6700 | 3.1900 | 3.6850 | 4.1800 | 4.6567 | 5.1333 | 5.6100 | |
| 8 | 1.5935 | 2.1236 | 2.6288 | 3.1339 | 3.6124 | 4.0909 | 4.5544 | 5.0178 | 5.4813 | |
| 9 | 1.5765 | 2.0964 | 2.5863 | 3.0761 | 3.5376 | 3.9991 | 4.4490 | 4.8988 | 5.3487 | |
| 10 | 1.5600 | 2.0700 | 2.5450 | 3.0200 | 3.4650 | 3.9100 | 4.3467 | 4.7833 | 5.2200 | |
| 1 | 2.0000 | 3.0000 | 4.0050 | 5.0100 | 6.0100 | 7.0100 | 8.0133 | 9.0167 | 10.0200 | |
| 2 | 1.8100 | 2.6000 | 3.3600 | 4.1200 | 4.8550 | 5.5900 | 6.3233 | 7.0567 | 7.7900 | |
| 3 | 1.7100 | 2.4000 | 3.0550 | 3.7100 | 4.3400 | 4.9700 | 5.5867 | 6.2033 | 6.8200 | |
| 4 | 1.6900 | 2.3300 | 2.9450 | 3.5600 | 4.1450 | 4.7300 | 5.3050 | 5.8800 | 6.4550 | |
| = 0.5 | 5 | 1.6700 | 2.2600 | 2.8350 | 3.4100 | 3.9500 | 4.4900 | 5.0233 | 5.5567 | 6.0900 |
| 6 | 1.6350 | 2.2100 | 2.7550 | 3.3000 | 3.8175 | 4.3350 | 4.8450 | 5.3550 | 5.8650 | |
| 7 | 1.6000 | 2.1600 | 2.6750 | 3.1900 | 3.6850 | 4.1800 | 4.6667 | 5.1533 | 5.6400 | |
| 8 | 1.5901 | 2.1336 | 2.6354 | 3.1372 | 3.6190 | 4.1008 | 4.5710 | 5.0411 | 5.5113 | |
| 9 | 1.5799 | 2.1064 | 2.5946 | 3.0828 | 3.5510 | 4.0192 | 4.4724 | 4.9255 | 5.3787 | |
| 10 | 1.5700 | 2.0800 | 2.5550 | 3.0300 | 3.4850 | 3.9400 | 4.3767 | 4.8133 | 5.2500 | |
| 1 | 2.0100 | 3.0100 | 4.0200 | 5.0300 | 6.0300 | 7.0300 | 8.0367 | 9.0433 | 10.0500 | |
| 2 | 1.8100 | 2.6000 | 3.3550 | 4.1100 | 4.8650 | 5.6200 | 6.3700 | 7.1200 | 7.8700 | |
| 3 | 1.7300 | 2.4100 | 3.0950 | 3.7800 | 4.4000 | 5.0200 | 5.6767 | 6.3333 | 6.9900 | |
| 4 | 1.7050 | 2.3500 | 2.9825 | 3.6150 | 4.2150 | 4.8150 | 5.4250 | 6.0350 | 6.6450 | |
| = 0.8 | 5 | 1.6800 | 2.2900 | 2.8700 | 3.4500 | 4.0300 | 4.6100 | 5.1733 | 5.7367 | 6.3000 |
| 6 | 1.6350 | 2.2300 | 2.7825 | 3.3350 | 3.8775 | 4.4200 | 4.9583 | 5.4967 | 6.0350 | |
| 7 | 1.5900 | 2.1700 | 2.6950 | 3.2200 | 3.7250 | 4.2300 | 4.7433 | 5.2567 | 5.7700 | |
| 8 | 1.5834 | 2.1502 | 2.6620 | 3.1738 | 3.6623 | 4.1508 | 4.6454 | 5.1401 | 5.6347 | |
| 9 | 1.5766 | 2.1298 | 2.6280 | 3.1262 | 3.5977 | 4.0692 | 4.5446 | 5.0199 | 5.4953 | |
| 10 | 1.5700 | 2.1100 | 2.5950 | 3.0800 | 3.5350 | 3.9900 | 4.4467 | 4.9033 | 5.3600 | |
| 1 | 2.0100 | 3.0200 | 4.0350 | 5.0500 | 6.0600 | 7.0700 | 8.0700 | 9.0700 | 10.0700 | |
| 2 | 1.8300 | 2.6300 | 3.3950 | 4.1600 | 4.9300 | 5.7000 | 6.4367 | 7.1733 | 7.9100 | |
| 3 | 1.7000 | 2.4000 | 3.0500 | 3.7000 | 4.3450 | 4.9900 | 5.6133 | 6.2367 | 6.8600 | |
| 4 | 1.6900 | 2.3550 | 2.9775 | 3.6000 | 4.2150 | 4.8300 | 5.4183 | 6.0067 | 6.5950 | |
| = 0.9 | 5 | 1.6800 | 2.3100 | 2.9050 | 3.5000 | 4.0850 | 4.6700 | 5.2233 | 5.7767 | 6.3300 |
| 6 | 1.6500 | 2.2600 | 2.8275 | 3.3950 | 3.9450 | 4.4950 | 5.0300 | 5.5650 | 6.1000 | |
| 7 | 1.6200 | 2.2100 | 2.7500 | 3.2900 | 3.8050 | 4.3200 | 4.8367 | 5.3533 | 5.8700 | |
| 8 | 1.6068 | 2.1869 | 2.7121 | 3.2372 | 3.7440 | 4.2507 | 4.7542 | 5.2576 | 5.7611 | |
| 9 | 1.5932 | 2.1631 | 2.6730 | 3.1828 | 3.6811 | 4.1793 | 4.6692 | 5.1590 | 5.6489 | |
| 10 | 1.5800 | 2.1400 | 2.6350 | 3.1300 | 3.6200 | 4.1100 | 4.5867 | 5.0633 | 5.5400 |
| Ĩsynch(m) | m | |||||
|---|---|---|---|---|---|---|
| n | 2 | 3 | 5 | 7 | 10 | |
| 1 | 1.48 | 1.80 | 2.19 | 2.48 | 2.78 | |
| 2 | 1.47 | 1.78 | 2.19 | 2.46 | 2.76 | |
| 0.2 | 3 | 1.47 | 1.77 | 2.18 | 2.45 | 2.75 |
| 5 | 1.47 | 1.77 | 2.17 | 2.44 | 2.73 | |
| 7 | 1.46 | 1.76 | 2.16 | 2.43 | 2.72 | |
| 10 | 1.46 | 1.76 | 2.16 | 2.42 | 2.71 | |
| 1 | 1.45 | 1.74 | 2.12 | 2.37 | 2.66 | |
| 2 | 1.44 | 1.72 | 2.09 | 2.34 | 2.61 | |
| 0.4 | 3 | 1.44 | 1.71 | 2.08 | 2.32 | 2.58 |
| 5 | 1.43 | 1.70 | 2.06 | 2.30 | 2.55 | |
| 7 | 1.43 | 1.70 | 2.05 | 2.29 | 2.54 | |
| 10 | 1.42 | 1.69 | 2.04 | 2.28 | 2.53 | |
| 1 | 1.44 | 1.73 | 2.07 | 2.33 | 2.59 | |
| 2 | 1.43 | 1.70 | 2.05 | 2.28 | 2.54 | |
| 0.5 | 3 | 1.42 | 1.68 | 2.03 | 2.26 | 2.51 |
| 5 | 1.41 | 1.67 | 2.01 | 2.24 | 2.48 | |
| 7 | 1.41 | 1.67 | 2.00 | 2.23 | 2.46 | |
| 10 | 1.40 | 1.66 | 1.99 | 2.21 | 2.45 | |
| 1 | 1.41 | 1.68 | 1.96 | 2.22 | 2.44 | |
| 2 | 1.38 | 1.62 | 1.92 | 2.13 | 2.35 | |
| 0.8 | 3 | 1.38 | 1.62 | 1.92 | 2.11 | 2.33 |
| 5 | 1.37 | 1.59 | 1.88 | 2.08 | 2.29 | |
| 7 | 1.37 | 1.59 | 1.88 | 2.07 | 2.27 | |
| 10 | 1.36 | 1.58 | 1.87 | 2.06 | 2.26 | |
| 1 | 1.40 | 1.68 | 1.93 | 2.16 | 2.36 | |
| 2 | 1.36 | 1.59 | 1.90 | 2.10 | 2.32 | |
| 0.9 | 3 | 1.37 | 1.58 | 1.88 | 2.07 | 2.39 |
| 5 | 1.34 | 1.57 | 1.86 | 2.04 | 2.24 | |
| 7 | 1.34 | 1.56 | 1.85 | 2.02 | 2.22 | |
| 10 | 1.34 | 1.56 | 1.83 | 2.01 | 2.22 | |
| Ĩseq(n) | n | |||||
|---|---|---|---|---|---|---|
| m | 2 | 3 | 5 | 7 | 10 | |
| 1 | 2.00 | 3.00 | 5.00 | 7.00 | 10.00 | |
| 2 | 1.99 | 2.98 | 4.97 | 6.94 | 9.90 | |
| 0.2 | 3 | 1.98 | 2.96 | 4.92 | 6.87 | 9.79 |
| 5 | 1.99 | 2.98 | 4.94 | 6.90 | 9.83 | |
| 7 | 1.99 | 2.97 | 4.92 | 6.87 | 9.79 | |
| 10 | 1.99 | 2.96 | 4.91 | 6.85 | 9.76 | |
| 1 | 2.00 | 3.01 | 5.02 | 7.02 | 10.02 | |
| 2 | 1.99 | 2.97 | 4.93 | 6.89 | 9.82 | |
| 0.4 | 3 | 1.98 | 2.96 | 4.90 | 6.84 | 9.74 |
| 5 | 1.97 | 2.94 | 4.86 | 6.77 | 9.63 | |
| 7 | 1.97 | 2.94 | 4.85 | 6.76 | 9.76 | |
| 10 | 1.97 | 2.92 | 4.83 | 6.72 | 9.55 | |
| 1 | 2.00 | 3.00 | 5.01 | 7.01 | 10.02 | |
| 2 | 1.99 | 2.96 | 4.91 | 6.86 | 9.78 | |
| 0.5 | 3 | 1.96 | 2.92 | 4.83 | 6.75 | 9.60 |
| 5 | 1.98 | 2.94 | 4.87 | 6.77 | 9.62 | |
| 7 | 1.96 | 2.92 | 4.81 | 6.70 | 9.51 | |
| 10 | 1.96 | 2.91 | 4.79 | 6.67 | 9.47 | |
| 1 | 2.01 | 3.01 | 5.03 | 7.03 | 10.05 | |
| 2 | 1.97 | 2.94 | 4.90 | 6.82 | 9.72 | |
| 0.8 | 3 | 1.95 | 2.90 | 4.78 | 6.67 | 9.48 |
| 5 | 1.98 | 2.94 | 4.85 | 6.74 | 9.59 | |
| 7 | 1.93 | 2.86 | 4.72 | 6.57 | 9.32 | |
| 10 | 1.94 | 2.87 | 4.73 | 6.55 | 9.31 | |
| 1 | 2.01 | 3.02 | 5.05 | 7.07 | 10.07 | |
| 2 | 1.96 | 2.95 | 4.84 | 6.79 | 9.67 | |
| 0.9 | 3 | 1.91 | 2.85 | 4.75 | 6.59 | 9.40 |
| 5 | 1.97 | 2.94 | 4.85 | 6.76 | 9.55 | |
| 7 | 1.96 | 2.90 | 4.78 | 6.63 | 9.39 | |
| 10 | 1.98 | 3.06 | 4.81 | 6.66 | 9.50 | |
| IS/P | n = 2 | n = 3 | n = 4 | n = 5 | n = 6 | n = 7 | n = 8 | n = 9 | n = 10 | |
|---|---|---|---|---|---|---|---|---|---|---|
| 0.2 | 1.0960 | 1.1466 | 1.1751 | 1.2036 | 1.2227 | 1.2418 | 1.2529 | 1.2642 | 1.2752 | |
| 0.4 | 1.0910 | 1.1461 | 1.1786 | 1.2110 | 1.2218 | 1.2325 | 1.2439 | 1.2554 | 1.2667 | |
| 0.5 | 1.0961 | 1.1390 | 1.1649 | 1.1908 | 1.2091 | 1.2274 | 1.2368 | 1.2464 | 1.2557 | |
| 0.8 | 1.0856 | 1.1340 | 1.1624 | 1.1907 | 1.2023 | 1.2139 | 1.2208 | 1.2278 | 1.2347 | |
| 0.9 | 1.0714 | 1.1214 | 1.1416 | 1.1617 | 1.1761 | 1.1905 | 1.2011 | 1.2119 | 1.2224 | |
| 0.2 | 1.1447 | 1.2244 | 1.2720 | 1.3195 | 1.3491 | 1.3787 | 1.3969 | 1.4152 | 1.4332 | |
| 0.4 | 1.1431 | 1.2175 | 1.2637 | 1.3099 | 1.3366 | 1.3633 | 1.3819 | 1.4006 | 1.419 | |
| 0.5 | 1.1468 | 1.2148 | 1.2581 | 1.3013 | 1.3297 | 1.3581 | 1.3747 | 1.3914 | 1.4078 | |
| 0.8 | 1.1266 | 1.2020 | 1.2340 | 1.2659 | 1.2977 | 1.3294 | 1.3384 | 1.3476 | 1.3565 | |
| 0.9 | 1.1237 | 1.1898 | 1.2363 | 1.2827 | 1.3018 | 1.3208 | 1.3372 | 1.3537 | 1.3699 | |
| 0.2 | 1.1721 | 1.2695 | 1.3290 | 1.3885 | 1.4251 | 1.4618 | 1.4847 | 1.5079 | 1.53065 | |
| 0.4 | 1.1685 | 1.2609 | 1.3192 | 1.3775 | 1.4102 | 1.4430 | 1.4645 | 1.4863 | 1.5076 | |
| 0.5 | 1.1680 | 1.2581 | 1.3108 | 1.3636 | 1.3984 | 1.4332 | 1.4531 | 1.4733 | 1.493 | |
| 0.8 | 1.1516 | 1.2448 | 1.2905 | 1.3362 | 1.3665 | 1.3969 | 1.4110 | 1.4253 | 1.43935 | |
| 0.9 | 1.1490 | 1.2299 | 1.2819 | 1.3340 | 1.3588 | 1.3836 | 1.4021 | 1.4208 | 1.4391 | |
| 0.2 | 1.1995 | 1.3145 | 1.3860 | 1.4575 | 1.5012 | 1.5448 | 1.5726 | 1.6006 | 1.6281 | |
| 0.4 | 1.1938 | 1.3042 | 1.3746 | 1.4450 | 1.4839 | 1.5227 | 1.5472 | 1.5719 | 1.5962 | |
| 0.5 | 1.1892 | 1.3014 | 1.3636 | 1.4258 | 1.4671 | 1.5083 | 1.5316 | 1.5551 | 1.5782 | |
| 0.8 | 1.1766 | 1.2875 | 1.3470 | 1.4064 | 1.4354 | 1.4643 | 1.4836 | 1.5031 | 1.5222 | |
| 0.9 | 1.1743 | 1.2700 | 1.3276 | 1.3852 | 1.4158 | 1.4463 | 1.4670 | 1.4878 | 1.5083 | |
| 0.2 | 1.2157 | 1.3430 | 1.4208 | 1.4986 | 1.5468 | 1.5950 | 1.6266 | 1.6584 | 1.68965 | |
| 0.4 | 1.2092 | 1.3335 | 1.4078 | 1.4820 | 1.5261 | 1.5702 | 1.5982 | 1.6266 | 1.6544 | |
| 0.5 | 1.2099 | 1.3268 | 1.3971 | 1.4674 | 1.5113 | 1.5552 | 1.5810 | 1.6071 | 1.6326 | |
| 0.8 | 1.1947 | 1.3023 | 1.3697 | 1.4372 | 1.4731 | 1.5091 | 1.5291 | 1.5492 | 1.56895 | |
| 0.9 | 1.1918 | 1.2901 | 1.3551 | 1.4200 | 1.4554 | 1.4908 | 1.5115 | 1.5325 | 1.5531 | |
| 0.2 | 1.2319 | 1.3715 | 1.4556 | 1.5397 | 1.5925 | 1.6452 | 1.6805 | 1.7162 | 1.7512 | |
| 0.4 | 1.2245 | 1.3628 | 1.4409 | 1.5190 | 1.5683 | 1.6176 | 1.6493 | 1.6813 | 1.7126 | |
| 0.5 | 1.2305 | 1.3521 | 1.4305 | 1.5089 | 1.5555 | 1.6021 | 1.6304 | 1.6590 | 1.687 | |
| 0.8 | 1.2128 | 1.3170 | 1.3925 | 1.4679 | 1.5109 | 1.5539 | 1.5745 | 1.5953 | 1.6157 | |
| 0.9 | 1.2092 | 1.3102 | 1.3825 | 1.4548 | 1.4950 | 1.5352 | 1.5561 | 1.5772 | 1.5979 | |
| 0.2 | 1.2423 | 1.3898 | 1.4787 | 1.5676 | 1.6240 | 1.6805 | 1.7180 | 1.7558 | 1.7929 | |
| 0.4 | 1.2360 | 1.3783 | 1.4620 | 1.5456 | 1.5981 | 1.6505 | 1.6843 | 1.7183 | 1.7517 | |
| 0.5 | 1.2370 | 1.3680 | 1.4506 | 1.5332 | 1.5831 | 1.6329 | 1.6639 | 1.6952 | 1.7259 | |
| 0.8 | 1.2202 | 1.3313 | 1.4110 | 1.4907 | 1.5364 | 1.5822 | 1.6069 | 1.6318 | 1.6563 | |
| 0.9 | 1.2244 | 1.3498 | 1.4156 | 1.4814 | 1.5222 | 1.5630 | 1.5876 | 1.6124 | 1.6368 | |
| 0.2 | 1.2529 | 1.4084 | 1.5021 | 1.5958 | 1.6559 | 1.7161 | 1.7558 | 1.7958 | 1.835 | |
| 0.4 | 1.2477 | 1.3939 | 1.4832 | 1.5725 | 1.6282 | 1.6838 | 1.7196 | 1.7558 | 1.7911 | |
| 0.5 | 1.2436 | 1.3840 | 1.4709 | 1.5577 | 1.6109 | 1.6640 | 1.6978 | 1.7318 | 1.7652 | |
| 0.8 | 1.2277 | 1.3457 | 1.4297 | 1.5137 | 1.5622 | 1.6107 | 1.6396 | 1.6687 | 1.6973 | |
| 0.9 | 1.2398 | 1.3897 | 1.4490 | 1.5083 | 1.5497 | 1.5911 | 1.6194 | 1.6480 | 1.676 | |
| 0.2 | 1.2632 | 1.4265 | 1.5250 | 1.6234 | 1.6872 | 1.7510 | 1.7928 | 1.8350 | 1.8764 | |
| 0.4 | 1.2591 | 1.4092 | 1.5041 | 1.5989 | 1.6577 | 1.7164 | 1.7542 | 1.7924 | 1.8299 | |
| 0.5 | 1.2500 | 1.3997 | 1.4908 | 1.5818 | 1.6382 | 1.6945 | 1.7309 | 1.7677 | 1.8038 | |
| 0.8 | 1.2351 | 1.3598 | 1.4481 | 1.5363 | 1.5875 | 1.6387 | 1.6716 | 1.7049 | 1.7375 | |
| 0.9 | 1.2548 | 1.4289 | 1.4818 | 1.5346 | 1.5766 | 1.6186 | 1.6506 | 1.6829 | 1.7146 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Barron, Y. The Delay Time Profile of Multistage Networks with Synchronization. Mathematics 2023, 11, 3232. https://doi.org/10.3390/math11143232
Barron Y. The Delay Time Profile of Multistage Networks with Synchronization. Mathematics. 2023; 11(14):3232. https://doi.org/10.3390/math11143232
Chicago/Turabian StyleBarron, Yonit. 2023. "The Delay Time Profile of Multistage Networks with Synchronization" Mathematics 11, no. 14: 3232. https://doi.org/10.3390/math11143232
APA StyleBarron, Y. (2023). The Delay Time Profile of Multistage Networks with Synchronization. Mathematics, 11(14), 3232. https://doi.org/10.3390/math11143232







