Invadopodia Formation in Cancer Cell: The Mathematical and Computational Modelling Based on Free Boundary Problem
Abstract
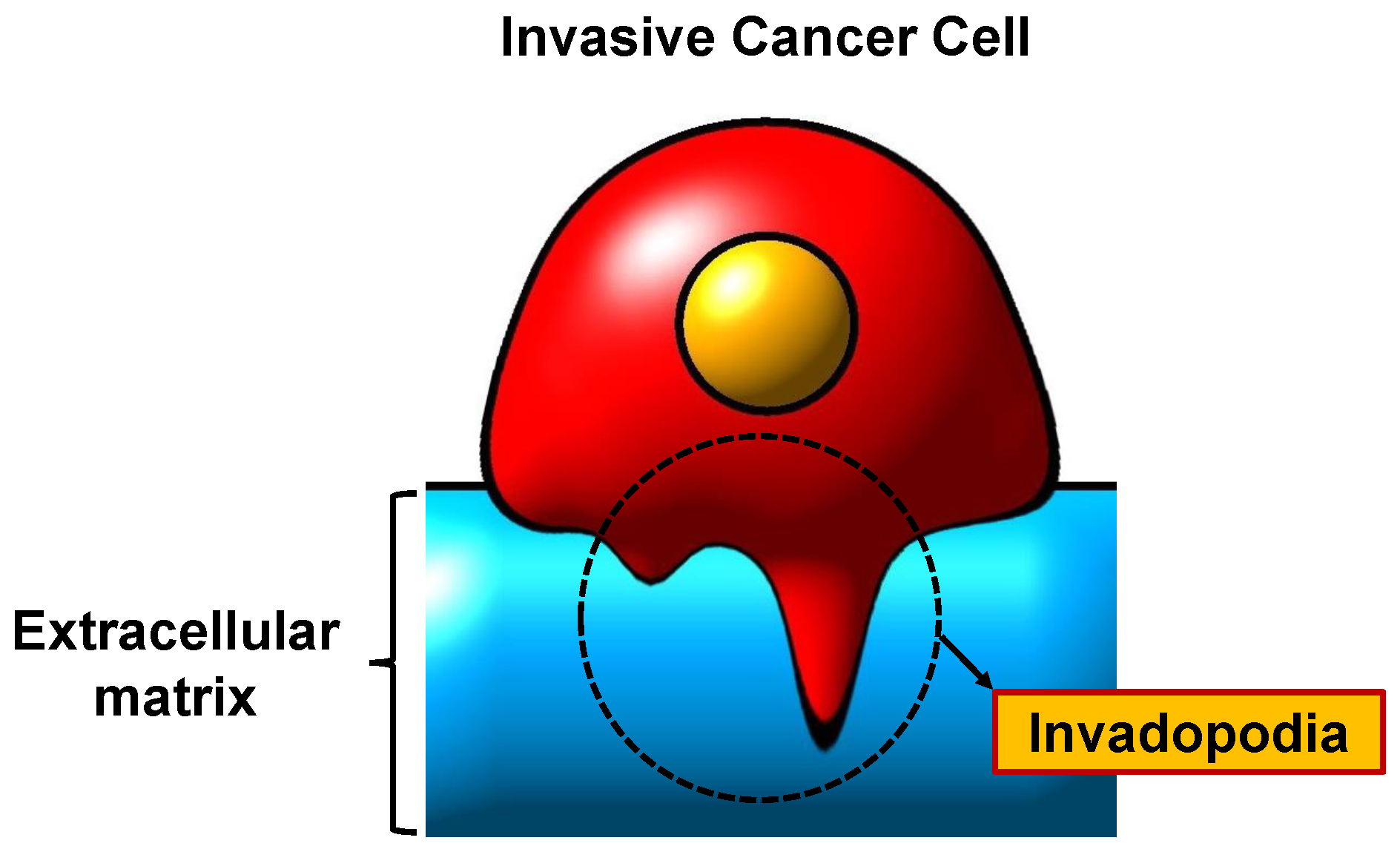
1. Introduction
2. Materials and Methods
2.1. Individual Cell Model
2.1.1. Classical Solution Scheme
2.1.2. Free Boundary Problem
2.2. Numerical Scheme
2.2.1. Weak Form Derivation
2.2.2. Stefan Problems—Phase-Transition Formulation
2.2.3. Cell Deformation: Free Boundary Conversion
2.2.4. Phase-Transition Formulation
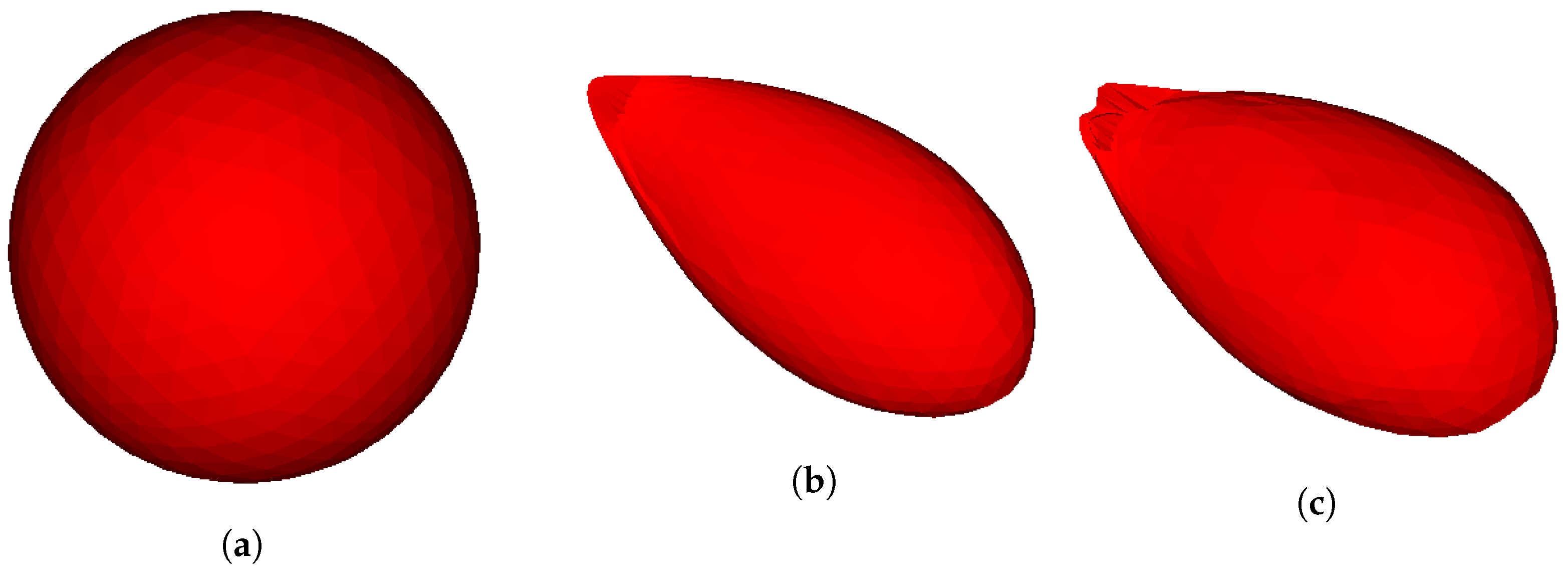
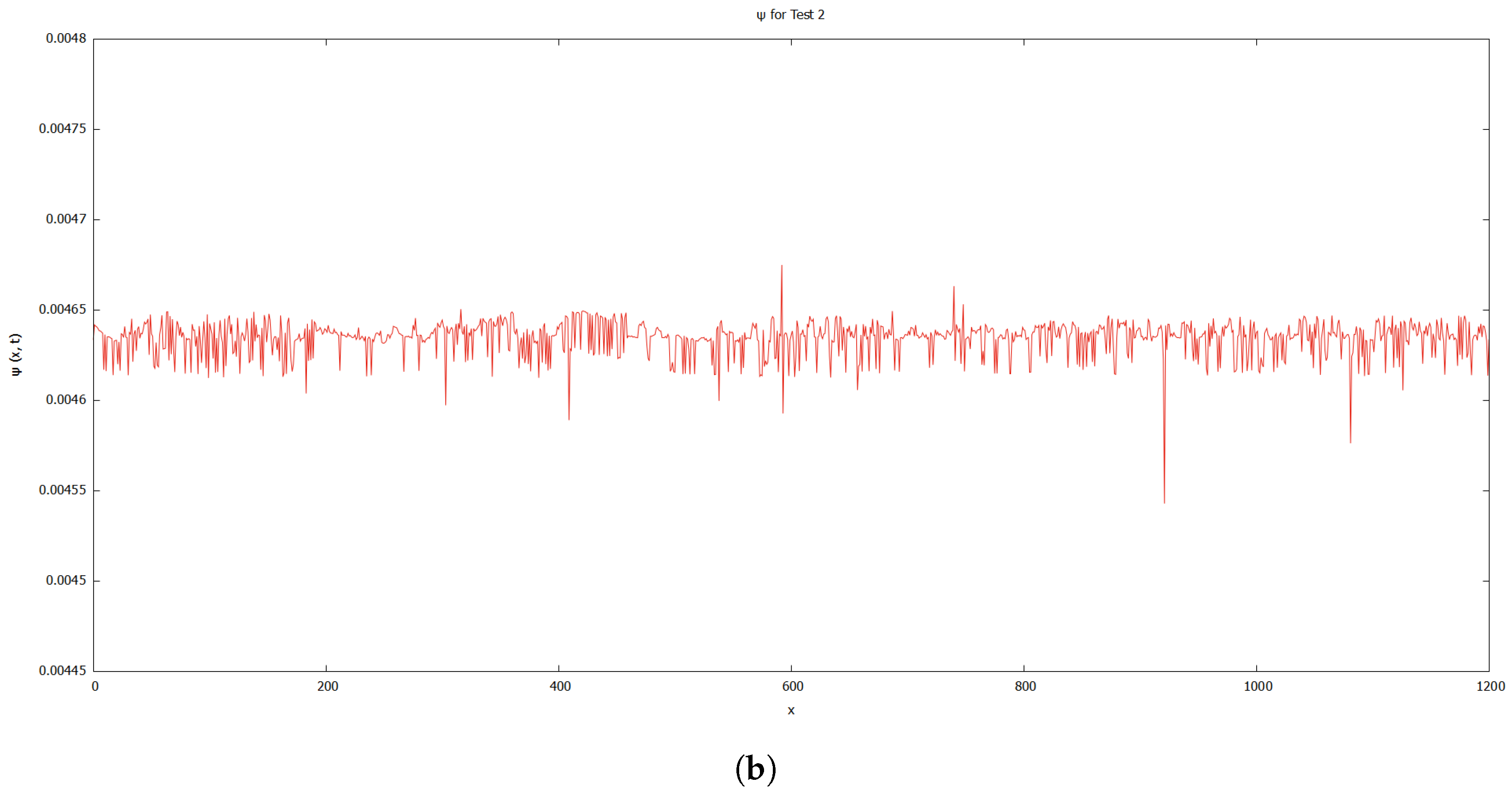
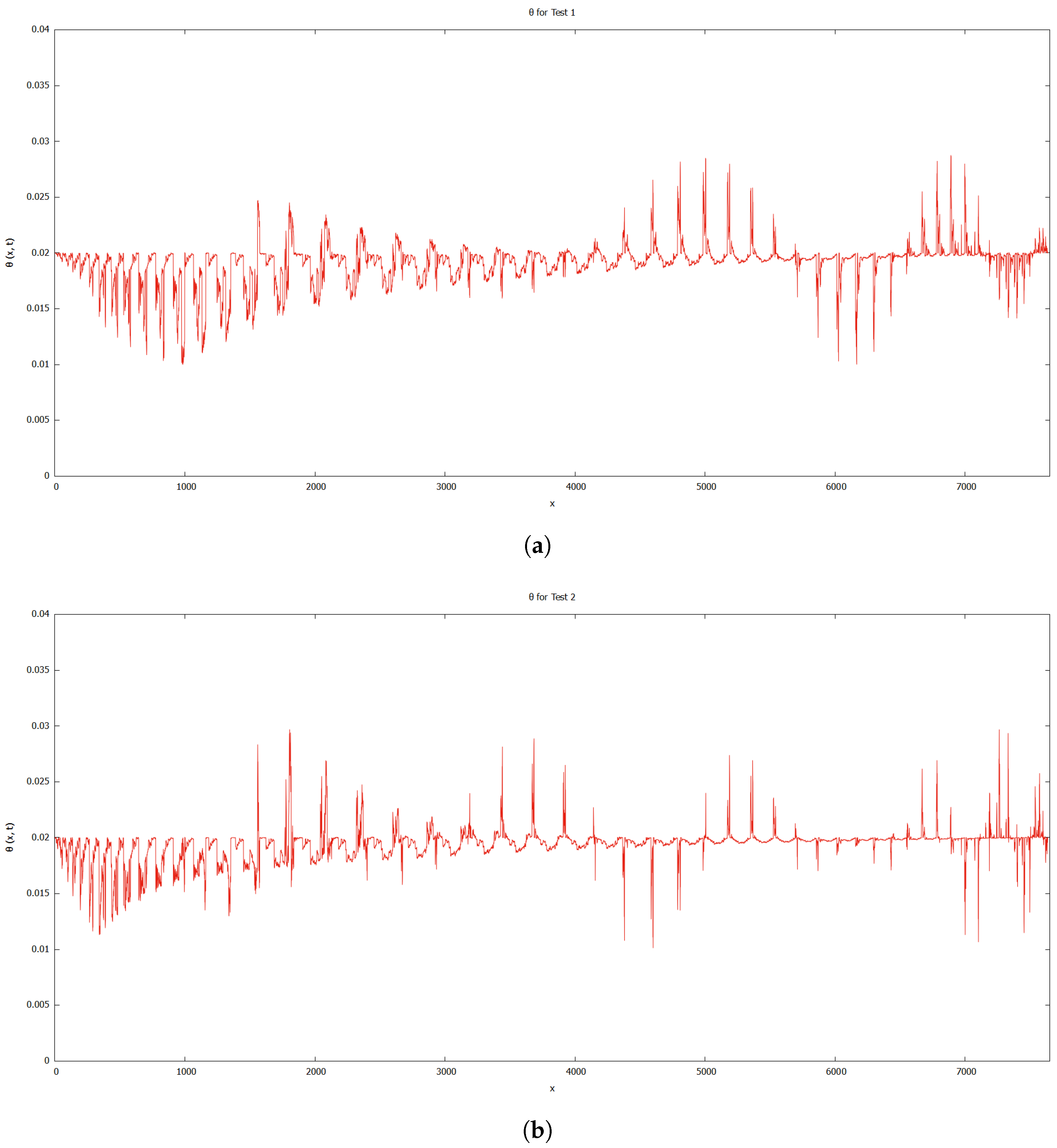
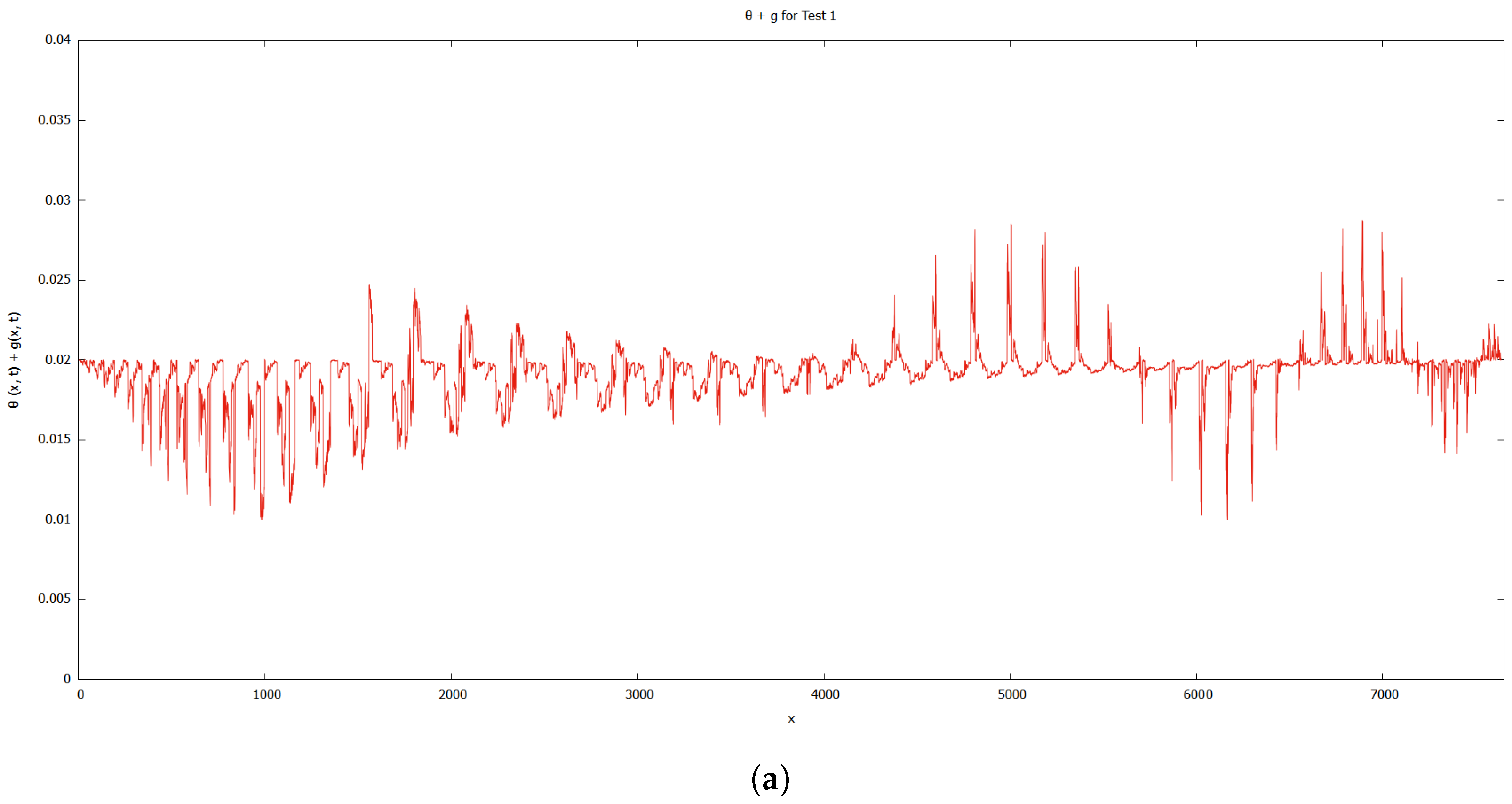
3. Results
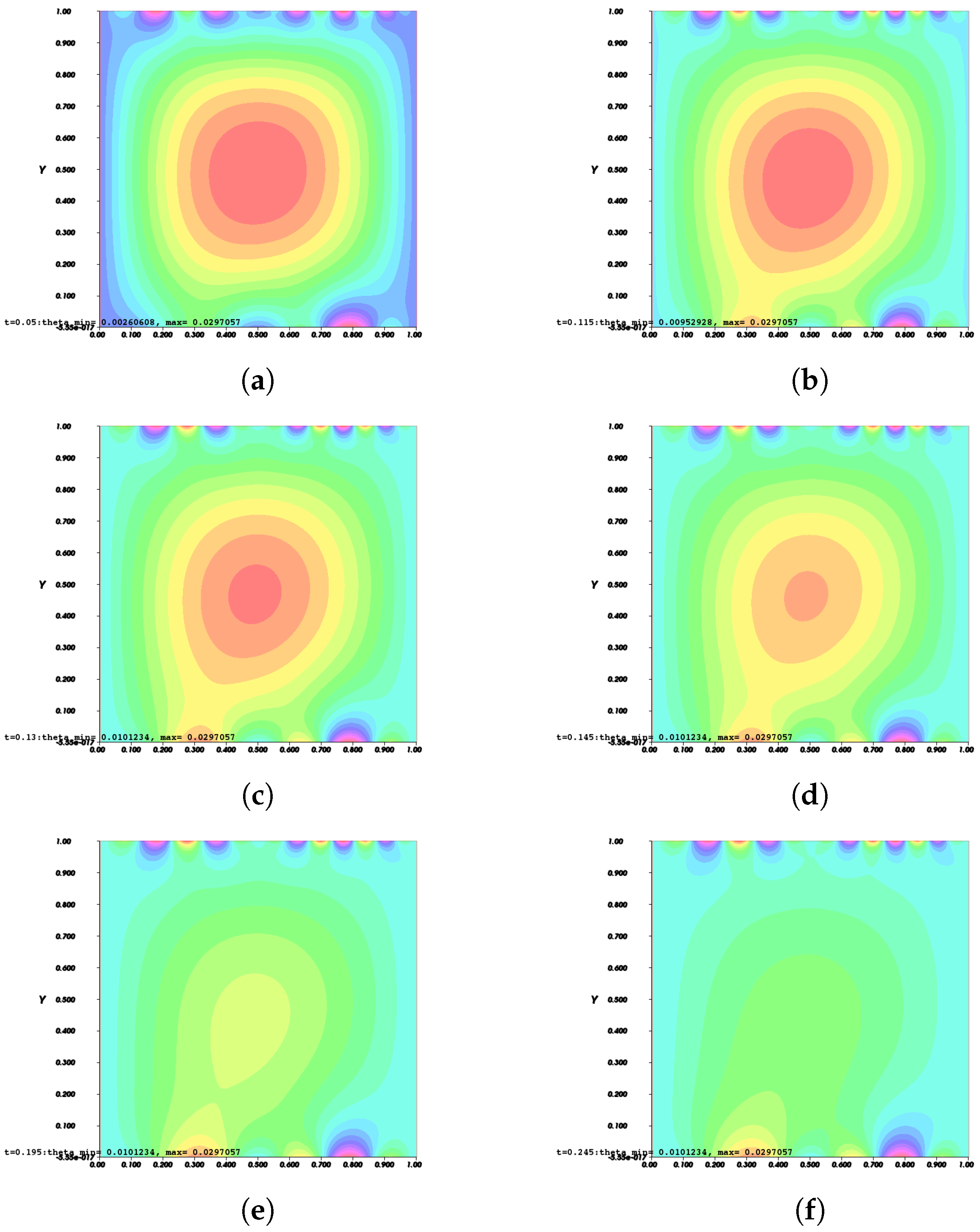
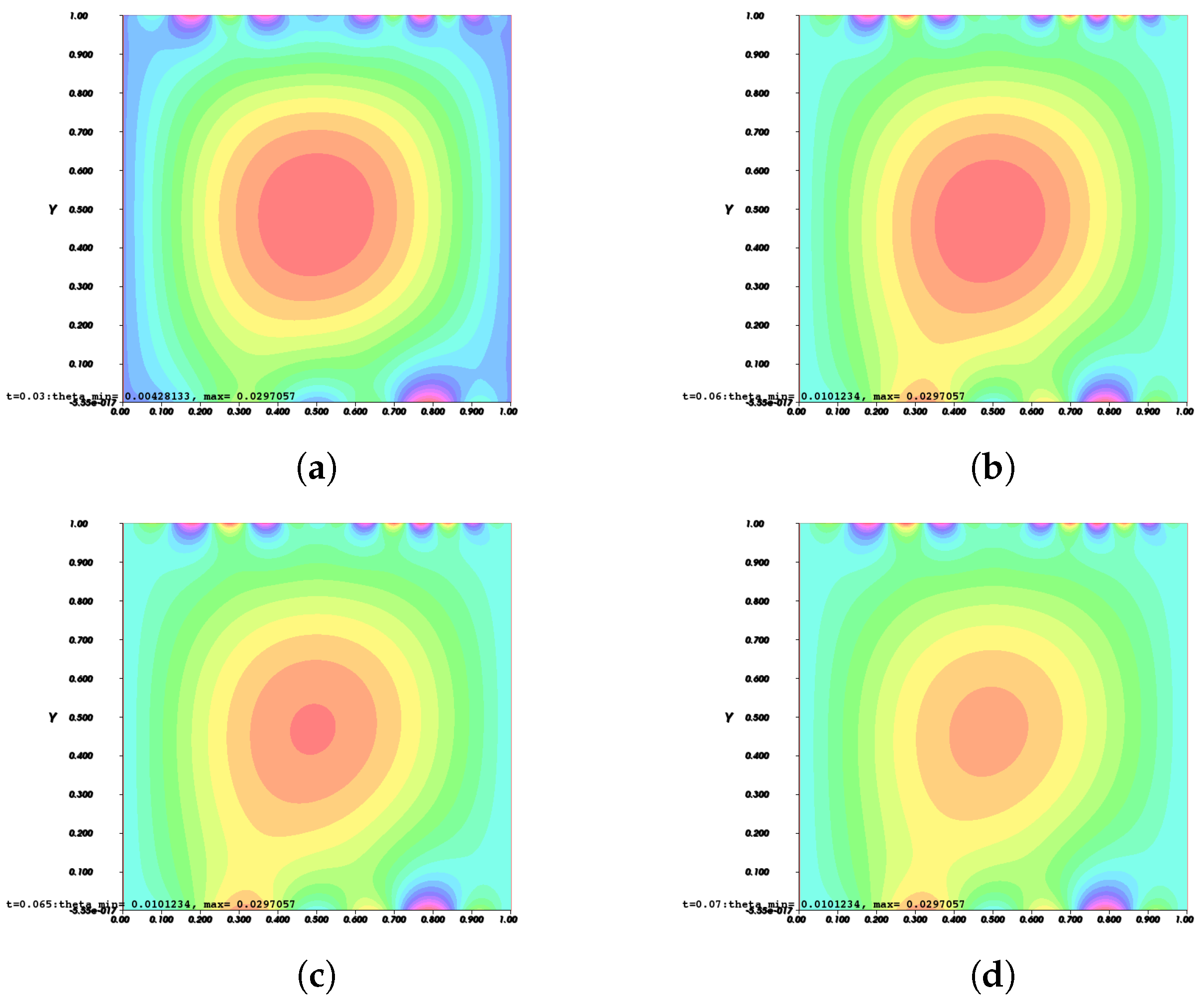
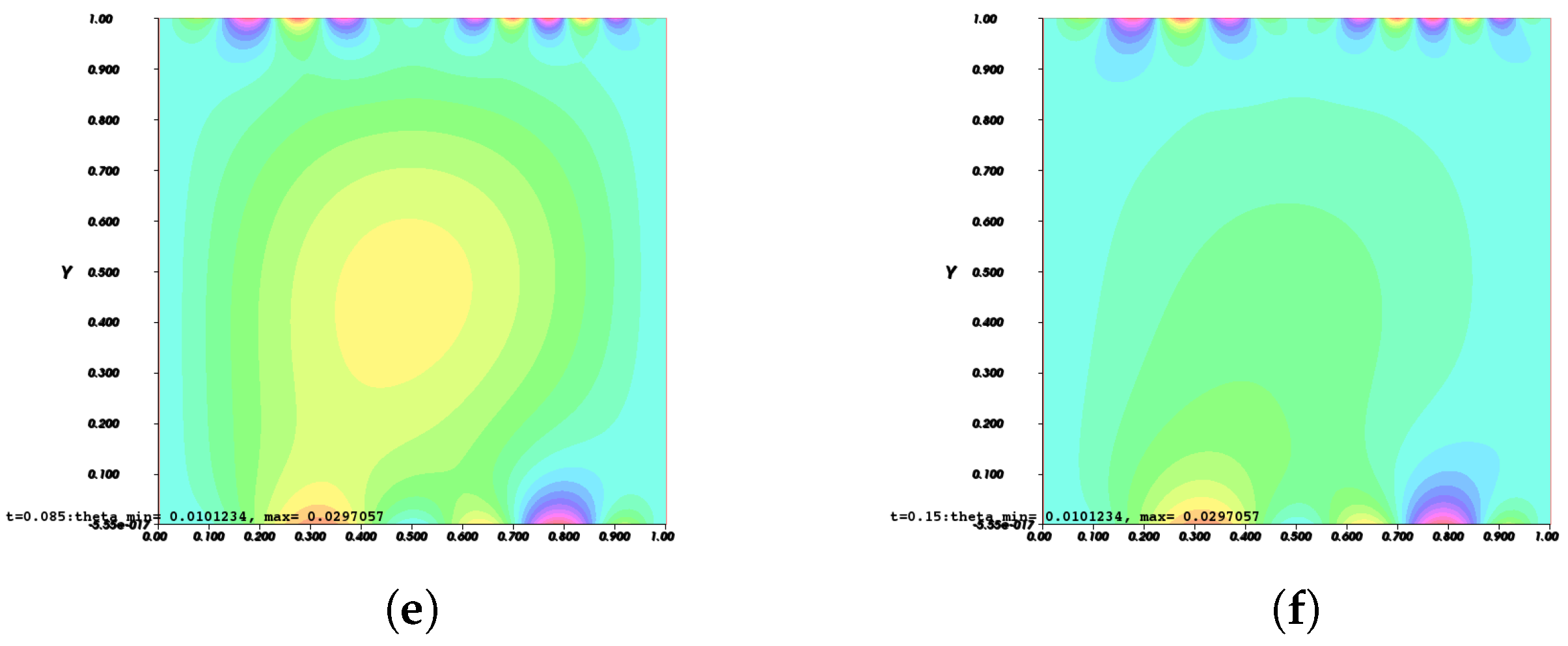
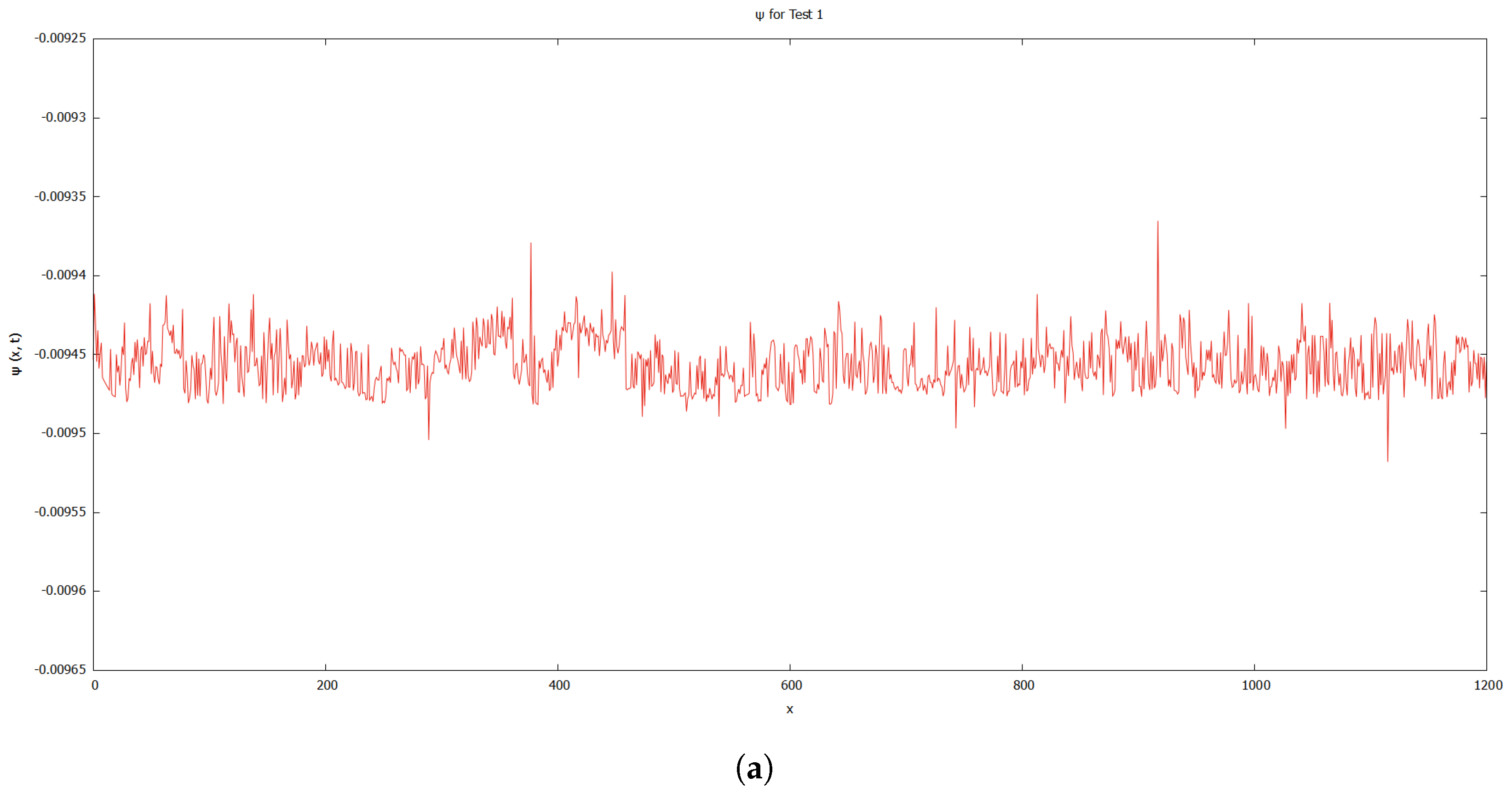
3.1. Level Set Method
- Test 1: and level set solution as where and .
- Test 2: Modifications of equations from Test I. and level set solution as where and .
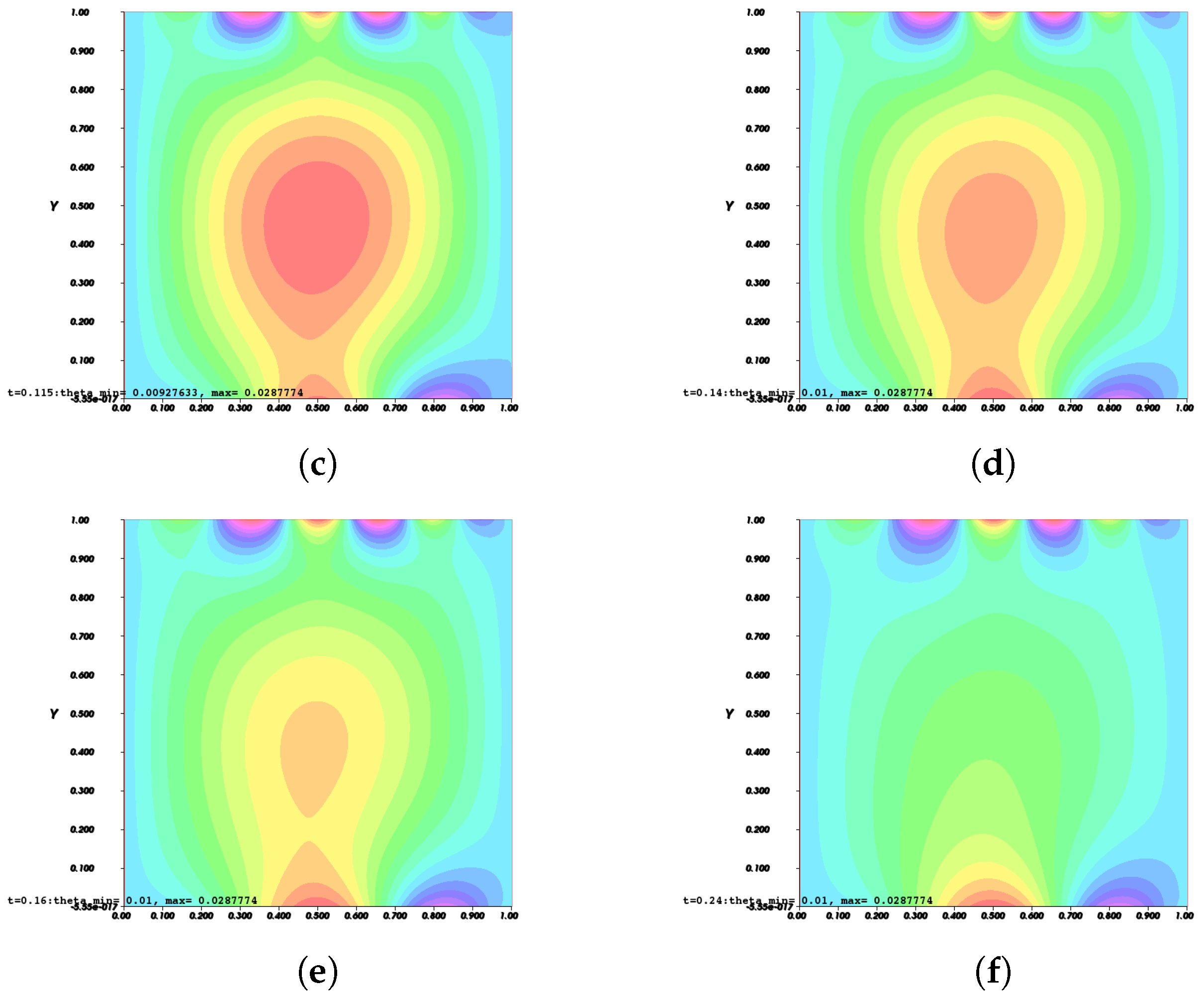
3.2. Enthalpy Method
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| BM | basement membrane |
| ECM | extracellular matrix |
| PDE | partial differential equation |
| ODE | ordinary differential equation |
| 2D | two-dimensional |
| 3D | three-dimensional |
| TLA | Three letter acronym |
| LD | Linear dichroism |
| FDM | finite difference method |
| FEM | finite element method |
| MMP | matrix metalloproteinases |
| MT1-MMP | type 1-matrix metalloproteinases |
References
- Preziosi, L. Cancer Modelling and Simulation; Chapman Hall/CRC Press: Boca Raton, FL, USA, 2003. [Google Scholar]
- Hanahan, D.; Weinberg, R.A. The hallmarks of cancer. Cell 2000, 100, 57–70. [Google Scholar] [CrossRef]
- Hanahan, D.; Weinberg, R.A. Hallmarks of cancer: The next generation. Cell 2011, 144, 646–674. [Google Scholar] [CrossRef] [PubMed]
- Sporn, M.B. The war on cancer. Lancet 1996, 347, 1377–1381. [Google Scholar] [CrossRef] [PubMed]
- Andasari, V.; Chaplain, M.A.J. Intracellular modelling of cell-matrix adhesion during cancer cell invasion. Math. Mod. Nat. Phen. 2012, 7, 29–48. [Google Scholar] [CrossRef]
- Andasari, V.; Roper, R.T.; Swat, M.H.; Chaplain, M.A.J. Integrating intracellular dynamics using CompuCell3D and Bionetsolver: Applications to multiscale modelling of cancer cell growth and invasion. PLoS ONE 2012, 7, e33726. [Google Scholar] [CrossRef] [PubMed]
- Stylli, S.S.; Kaye, A.H.; Lock, P. Invadopodia: At the cutting edge of tumour invasion. J. Clin. Neurosci. 2008, 15, 725–737. [Google Scholar] [CrossRef] [PubMed]
- Weaver, A.M. Invadopodia. Curr. Biol. 2008, 18, R362–R364. [Google Scholar] [CrossRef]
- Gallinato, O.; Poignard, C. Superconvergent second order Cartesian method for solving free boundary problem for invadopodia formation. J. Comput. Phys. 2017, 339, 412–431. [Google Scholar] [CrossRef]
- Saitou, T.; Rouzimaimaiti, M.; Koshikawa, N.; Seiki, M.; Ichikawa, K.; Suzuki, T. Mathematical modeling of invadopodia formation. J. Theo. Biol. 2012, 298, 138–146. [Google Scholar] [CrossRef] [PubMed]
- Murphy, D.A.; Courtneidge, S.A. The ’ins’ and ’outs’ of podosomes and invadopodia: Characteristics, formation and function. Nat. Rev. Mol. Cell Biol. 2011, 12, 413–426. [Google Scholar] [CrossRef]
- Loling Othman, N.; Suzuki, T. Free Boundary Problem of Cell Deformation and Invasion. In Proceedings of the Methods of Mathematical Oncology: Fusion of Mathematics and Biology, Osaka, Japan, 26–28 October 2020; pp. 107–123. [Google Scholar]
- Anderson, A.R.A.; Chaplain, M.A.J.; Newman, E.L.; Steele, R.J.C.; Thompson, A.M. Mathematical modelling of tumour invasion and metastasis. Comput. Math. Methods Med. 2000, 2, 129–154. [Google Scholar] [CrossRef]
- Chaplain, M.A.J.; Lolas, G. Mathematical modelling of cancer invasion of tissue: Dynamic heterogeneity. Net. Hetero. Med. 2006, 1, 399–439. [Google Scholar] [CrossRef]
- Ramis-Conde, I.; Chaplain, M.A.J.; Anderson, A.R.A. Mathematical modelling of cancer cell invasion of tissue. Math. Comput. Modelling 2008, 47, 533–545. [Google Scholar] [CrossRef]
- Chaplain, M.A.J.; Lachowicz, M.; Szymańska, Z.; Wrzosek, D. Mathematical modelling of cancer invasion: The importance of cell–cell adhesion and cell–matrix adhesion. Math. Models Methods Appl. Sci. 2011, 21, 719–743. [Google Scholar] [CrossRef]
- Chaplain, M.A.J.; Lolas, G. Mathematical modelling of cancer cell invasion of tissue: The role of the urokinase plasminogen activation system. Math. Models Methods Appl. Sci. 2005, 15, 1685–1734. [Google Scholar] [CrossRef]
- Andasari, V.; Gerisch, A.; Lolas, G.; South, A.P.; Chaplain, M.A.J. Mathematical modeling of cancer cell invasion of tissue: Biological insight from mathematical analysis and computational simulation. J. Math. Biol. 2011, 63, 141–171. [Google Scholar] [CrossRef]
- Admon, M.A.B. Mathematical Modeling and Simulation in an Individual Cancer Cell Associated with Invadopodia Formation. Ph.D. Thesis, Osaka University, Suita, Japan, 2015. [Google Scholar]
- Gallinato, O.; Ohta, M.; Poignard, C.; Suzuki, T. Free boundary problem for cell protrusion formations: Theoretical and numerical aspects. J. Math. Biol. 2017, 75, 263–307. [Google Scholar] [CrossRef]
- Yaacob, N.; Shafie, S.; Suzuki, T.; Admon, M.A. Level set method for free boundary of invasive cancer cell using different functions of matrix metalloproteinases. J. Phys. Conf. Ser. 2021, 1988, 012020. [Google Scholar] [CrossRef]
- Mahemuti, R.; Muhammadhaji, A.; Suzuki, T. Research on the Solution of Cell Invasion Model with Free Boundary. Open J. Appl. Sci. 2017, 7, 242. [Google Scholar] [CrossRef]
- Crank, J. The Mathematics of Diffusion; Oxford University Press: Oxford, UK, 1979. [Google Scholar]
- Groot, R.D. Second order front tracking algorithm for Stefan problem on a regular grid. J. Comput. Phys. 2018, 372, 956–971. [Google Scholar] [CrossRef]
- Chen, S.; Merriman, B.; Osher, S.; Smereka, P. A simple level set method for solving Stefan problems. J. Comput. Phys. 1997, 135, 8–29. [Google Scholar] [CrossRef]
- Hansen, J.P.; McDonald, I.R. Theory of Simple Liquids: With Applications to Soft Matter; Academic Press: Cambridge, MA, USA, 2013. [Google Scholar]
- Hecht, F. New development in FreeFem++. J. Numer. Math. 2012, 20, 251–265. [Google Scholar] [CrossRef]
- Geuzaine, C.; Remacle, J.F. Gmsh: A 3-D finite element mesh generator with built-in pre-and post-processing facilities. J. Comput. Phys. 2009, 79, 1309–1331. [Google Scholar] [CrossRef]



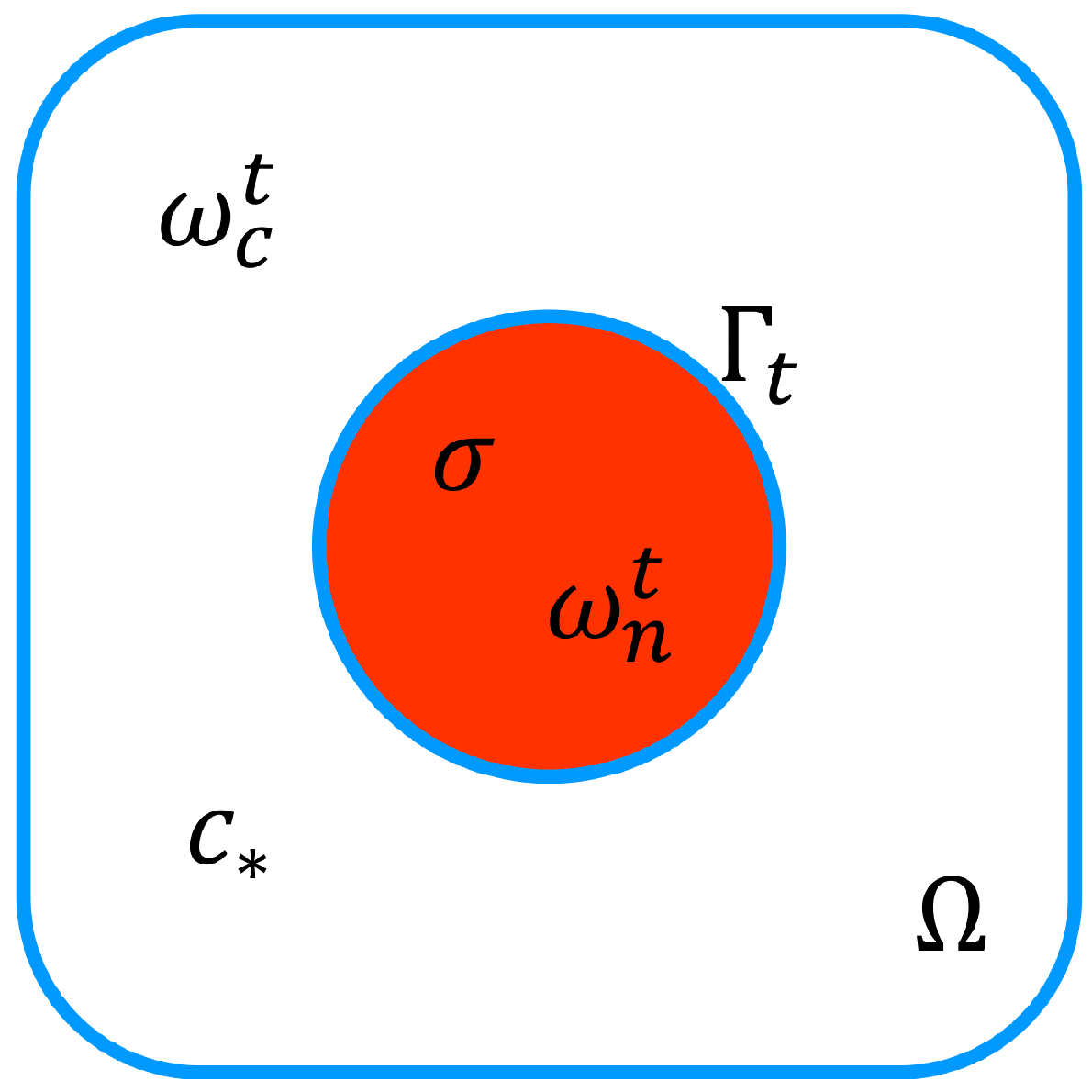





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ramlee, M.A.; Loling Othman, N.; Suzuki, T. Invadopodia Formation in Cancer Cell: The Mathematical and Computational Modelling Based on Free Boundary Problem. Mathematics 2023, 11, 3044. https://doi.org/10.3390/math11143044
Ramlee MA, Loling Othman N, Suzuki T. Invadopodia Formation in Cancer Cell: The Mathematical and Computational Modelling Based on Free Boundary Problem. Mathematics. 2023; 11(14):3044. https://doi.org/10.3390/math11143044
Chicago/Turabian StyleRamlee, Muhammad Akmal, Nuha Loling Othman, and Takashi Suzuki. 2023. "Invadopodia Formation in Cancer Cell: The Mathematical and Computational Modelling Based on Free Boundary Problem" Mathematics 11, no. 14: 3044. https://doi.org/10.3390/math11143044
APA StyleRamlee, M. A., Loling Othman, N., & Suzuki, T. (2023). Invadopodia Formation in Cancer Cell: The Mathematical and Computational Modelling Based on Free Boundary Problem. Mathematics, 11(14), 3044. https://doi.org/10.3390/math11143044






