General Non-Local Continuum Mechanics: Derivation of Balance Equations

Abstract

In this paper, mechanics of continuum with general form of nonlocality in space and time is considered. Some basic concepts of nonlocal continuum mechanics are discussed. General fractional calculus (GFC) and general fractional vector calculus (GFVC) are used as mathematical tools for constructing mechanics of media with general form of nonlocality in space and time. Balance equations for mass, momentum, and energy, which describe conservation laws for nonlocal continuum, are derived by using the fundamental theorems of the GFC. The general balance equation in the integral form are derived by using the second fundamental theorems of the GFC. The first fundamental theorems of GFC and the proposed fractional analogue of the Titchmarsh theorem are used to derive the differential form of general balance equations from the integral form of balance equations. Using the general fractional vector calculus, the equations of conservation of mass, momentum, and energy are also suggested for a wide class of regions and surfaces.

1. Introduction

A general theory of nonlocal continuum mechanics was formally initiated by the papers of Kröner [1] in 1967, and then, by the works of Eringen [2,3,4] in 1972. Nonlocal continuum theory [5,6] is based on the assumption that the forces between material points are a long-range type, thus reflecting the long-range character of inter-atomic interactions. The nonlocal properties of media can manifest themselves in the form of spatial non-locality, fading memory and distributed lag (non-locality in time), fractional long-range interactions and fractional dispersions.

At the present time, three approaches to formulating the mechanics of nonlocal media can be distinguished from a mathematical point of view.

1.

The first approach is based on the use of various integral and integro-differential operators of general form, which are not self-consistent with each other and do not form a calculus. Eringen’s book [5] and Rogula’s work [6] can be attributed to this approach. As an example, it should also be noted the works [7,8,9] that described continuous media with non-locality in time. Note some modern reviews of various aspects of nonlocal mechanics in [10,11,12,13,14]. The models of non-local media described in these reviews do not assume that integral and integro-differential operators form a calculus.

It should be emphasized that the so-called differential (gradient) models, which are based on the differential equations of integer orders, cannot be considered as a tool for describing non-local media from a mathematical point of view. This is due to the well-known fact that integer-order differential equations are defined in an infinitesimally small neighborhood of the point under consideration and, therefore, are a tool for describing only local media. However, differential (gradient) models can be used to obtain some corrections to standard local continuum models by taking into account derivatives of the next order.

From a mathematical point of view, the lack of mutual consistency between the integral and integro-differential operators, which do not form a calculus, leads to the absence of a non-local analogue of vector calculus for such operators. At the same time, the importance of vector calculus as a tool for describing continuous media within the framework of standard local mechanics is obvious. As will be proved in this article, the fundamental theorems of calculus, which describe the relationship between differential and integral operators, make it possible to derive balance equations.

The advantage of the first approach is the use of a wide class of operator kernels in integral and integro-differential operators. However, the price for such generality is a narrower class of problems in mechanics, for which explicit solutions can be obtained for the equation of nonlocal continuum.

2.

The second approach is based on the use of integral and integro-differential operators with the power-law kernels and forming a calculus, which is called fractional calculus (FC). These operators are usually called fractional integrals and derivatives of non-integer orders (see books [15,16,17,18,19] and Volumes 1 and 2 of Handbook [20,21]). The FC has a very long history [15,22,23,24,25,26], and this calculus has a wide application in mechanics and physics (for example, see [27,28,29,30,31,32,33,34,35], Volumes 3 and 4 of Handbook [36,37] and reviews [38,39]). The theory of integral and differential equations of non-integer-orders is powerful tool to describe the media and processes with nonlocality in space and time.

First time the fractional derivatives with respect to space coordinates have been applied to nonlocal elastic continuum by Gubenko [40,41] in 1957. Then, the fractional calculus (FC) began to be actively used to describe continuum with power-law type of non-locality. Note some basic models and results in application of FC to nonlocal continuum mechanics: (1) Carpinteri, Cornetti and Sapora [42,43,44] consider nonlocal elastic continua modelled by a fractional calculus approach; (2) Cottone, Di Paola, Zingales and Marino, Failla [45,46,47,48,49] proposed fractional mechanical models for the dynamics of non-local continuum; (3) Drapaca and Sivaloganathan [50] describe a fractional model of continuum mechanics; (4) Challamel, Zorica, Atanackovic and Spasic [51] proposed a fractional generalization of Eringen’s nonlocal elasticity for wave propagation; (5) connection between continuous and lattice nonlocal models is proved in [27,52,53] by continuous limit of discrete systems with long-range interaction; (6) Tarasov, Zaslavsky, Edelman, Korabel [54,55,56,57] describe fractional dynamics of media with long-range interaction; (7) nonlocal model with power-law spatial dispersion in electrodynamics [58] and continuum mechanics [59,60,61,62,63] are described; (8) Yu and Zhai [64] proposed fractional Navier-Stokes equations with power-law nonlocality.

Vector calculus plays an important role in describing continuous media. For the first time, fractional vector calculus (FVC) that allows taking into account nonlocality was proposed in 2008 in the work [65] (see also Chapter 11 in book [27]). The fractional vector calculus was proposed in the form of self-consistent formulation, in which differential and integral vector operators are satisfy the vector analogs of fundamental theorems. The fractional generalizations of the Green’s, Stokes’, and Gauss’s theorems are formulated and proved in [27,65]. In this nonlocal vector calculus, the Caputo fractional derivatives and Riemann-Liouville fractional integrals are used to take into account power-law spatial non-locality. Then, after 2008, other works, which consider special aspects of self-consistent formulations of the FVC, are published by Bolster, Meerschaert and Sikorskii in 2012 [66]; D’Ovidio and Garra in 2014 [67]; Tarasov in 2014, 2015 [68,69]; Ortigueira, Rivero and Trujillo in 2015 [70]; Agrawal and Xu in 2015 [71]; Ortigueira and Machado in 2018 [72]; (g) Cheng and Dai in 2018 [73]. Unfortunately, almost all works were devoted to only one type of nonlocality and mainly to power-law nonlocality.

In the framework of the second approach, there are many fractional differential and integral equations that have exact and approximate solutions that describe various problems of non-local mechanics. This fact is an important advantage of the second approach in comparison with the first approach to nonlocal mechanics. Despite this advantage, the second approach has a serious disadvantage. In the models used in the framework of the second approach, only media and materials with power-law nonlocality in space and time were considered.

3.

The third approach, which is proposed in this article, is designed to combine the advantages of the first and second approaches. To describe a wide class of nonlocal continua and media, it is important to use a wide class of operator kernels for which integral and integro-differential operators would form a calculus. Self-consistency of integral and differential operators is provided by non-local analogues of the first and second fundamental theorems of some general calculus. It is also important to have a non-local analogue of the vector calculus, in which non-local analogues of the theorems of standard vector calculus would hold.

The possibility of formulating the third approach to nonlocal continuum mechanics is based on the general fractional calculus. The term general fractional calculus has been suggested in article [74] by Kochubei in 2011 (see also [75,76,77]). In works [74,75], the concepts of general fractional derivative (GFD) and general fractional integral (GFI) are proposed and the fundamental theorems of the GFC were proved. The GFC is based on the concept of kernel pairs, which was proposed by Sonin in 1884 work [78,79] (note that sometimes a French transliteration of his surname as “Sonine” is used instead of English transliteration [80]).

In this paper, it is proposed to use the Luchko form of the general fractional calculus (GFC) to formulate a continuum mechanics of general nonlocality in space and time. This very important form of the GFC was proposed by Luchko in 2021 [81,82,83] (see also [84,85]). In works [81,82], the GFD and GFI are suggested and the general fundamental theorems of the GFC are proved.

Then, in work [86], a general fractional vector calculus (GFVC) was proposed based on the general fractional calculus in the form of Luchko as a generalization of the approach that is suggested in paper [65] (see also Chapter 11 in book [27] (pp. 241–264)). The GFVC allows us to formulate continuum mechanics with general form of spatial nonlocality. This approach is proposed to be used to derive the balance equations for general non-local media in this article.

It should be noted that in 2021 a non-local vector calculus was also proposed by D’Elia, Gulian, Olson and Karniadak [87] as a generalization of the Meerschaert, Mortensen and Wheatcraft approach to FVC. However, this calculus is not related to the general fractional calculus.

In the paper, the Euler approach for description of nonlocal continuum is used. Motion of a continuum obeys the conservation laws for mass, momentum, and total energy. Balance equations for mass, momentum, and total energy of general nonlocal continuum are mathematical formulations of the conservation laws applied to the fixed region of the nonlocal continuum. In this paper, mathematical equations that describe these conservation laws for nonlocal continuum are derived. For this purpose, the fundamental theorems of the GFC are used:

(a)

To derive balance equations in general integral form, the second fundamental theorem of the GFC is used;

(b)

The fractional analogue of the Titchmarsh theorem and the first fundamental theorem of the GFC are used to derive balance equation of general nonlocal continuum in the general fractional differential form from the GF integral form;

(c)

Using the General FVC, the general fractional differential equations for conservation of mass, momentum, and energy are proposed for a wide class of regions and surfaces in general nonlocal media.

In Section 2, properties of the general fractional derivatives and integrals are considered. This section also briefly describes some elements of the general fractional vector calculus. In Section 3, some basic concepts of general nonlocal continuum are discussed. In Section 4, the general fractional continuity equation, which describes a conservation law for mass of general nonlocal continuum, is derived. In Section 5, the general transport equation for momentum that describes conservation law for momentum of general nonlocal continuum is obtained. A derivation of the fractional equilibrium equations for stresses is also suggested. In Section 6, the general fractional transport equation for total energy of continua with general nonlocality is derived. In Section 7, a brief conclusion and a list of main results are given.

2. General Fractional Integrals and Derivatives

2.1. Definitions of GFI and GFD

Let us assume that the functions $M(t)$ belongs to the space $C_{-1,0}(0,\infty )$ and suppose that there exists a function $K(t)\in C_{-1,0}(0,\infty )$, such that the Laplace convolution of these functions is equal to one for all $t\in (0,\infty )$. The function $\rho (t)$ belongs to the space $C_{-1,0}(0,\infty )$, if this function can be represented in the form $\rho (t)=t^{p}g(t)$, where $-1<p<0$ and $g(t)\in C[0,\infty )$.

Definition 1.

Let the functions $M(t)$ and $K(t))$ satisfy the following conditions.

(1) The Sonin condition for the kernels $M(t)$ and $K(t)$ requires that the equation

$$(M\ast K)(t)={\int }_0^{t}M(t-t^{\prime })K(t^{\prime })d{t}^{\prime }=1$$

(1)

holds for all $t\in (0,\infty )$. The set of the pairs $(M(t),K(t))$ that satisfy condition (1) is called the Sonin set and is denoted by $\mathbb{S}$.

(2) The functions $M(t),K(t)$ belong to the space $C_{-1,0}(0,\infty )$,

$$M(t),K(t)\in C_{-1,0}(0,\infty ),$$

(2)

where

$$C_{-1,0}(0,\infty )=\{\rho (t):\rho (t)=t^{p}g(t),t>0,-1<p<0,g(t)\in C[0,\infty )\}.$$

(3)

The set of the pairs $(M(t),K(t))$ that satisfy conditions (1) and (2) is called the Luchko set and is denoted by $\mathbb{L}$.

To define the GFI and GFD, the Luchko approach to general fractional calculus, which is proposed in [81,82], is used.

Definition 2.

Let the pair of the kernels $(M(t),K(t))$ belongs to the Luchko set $\mathbb{L}$.

Let $\rho (t)\in C_{-1}(0,\infty )=C_{-1,\infty }(0,\infty )$. Then, the general fractional integral (GFI) with the kernel $M(t)\in C_{-1,0}(0,\infty )$ is the operator on the space $C_{-1}(0,\infty )$ that is defined by the equation

$$I_{(M)}^t\left[t^{\prime }\right]\rho (t^{\prime })={\int }_0^{t}d{t}^{\prime }M(t-t^{\prime })\rho (t^{\prime }).$$

(4)

Let $\rho (t)\in C_{-1}^1(0,\infty )$, i.e., ${\rho }^{(1)}(t)\in C_{-1}(0,\infty )$. Then, the general fractional derivatives (GFD) with kernel $K(t)\in C_{-1,0}(0,\infty )$, which is associated with GFI (4), is defined as

$$D_{(K)}^{t,\ast }\left[t^{\prime }\right]\rho (t^{\prime })=(K\ast f^{(1)})(t)={\int }_0^{t}d{t}^{\prime }K(t-t^{\prime })f^{(1)}(t^{\prime })$$

(5)

for $t\in (0,\infty )$. Operator (5) is called the GFD of the Caputo type.

Remark 1.

If the kernel pair $(M(t),K(t))$ belongs to the Luchko set $\mathbb{L}$, the kernel $K(t)$ is called associated kernel to $M(t)$. Note that if $K(t)$ is associated kernel to $M(t)$, then $M(t)$ is associated kernel to $K(t)$. Therefore, if $(M(t),K(t))$ belongs to the set $\mathbb{L}$, then both pairs of operators $I_{(M)}^t\left[t^{\prime }\right]$, $D_{(K)}^{t,\ast }\left[t^{\prime }\right]$ and $I_{(K)}^t\left[t^{\prime }\right]$ $D_{(M)}^{t,\ast }\left[t^{\prime }\right]$ can be used as the general fractional integrals (GFI) and general fractional derivatives (GFD).

The GFI and GFD are connected by the fundamental theorems of general fractional calculus (FT of GFC).

Theorem 1

(First fundamental theorem of GFC). If a pair of kernels $(M(t),K(t))$ belongs to the Luchko set $\mathbb{L}$, then the equality

$$D_{(K)}^{t,\ast }\left[t^{\prime }\right]I_{(M)}^{t^{\prime }}\left[t^{″}\right]\rho (t^{″})=\rho (t)$$

(6)

holds for $\rho (t)\in C_{-1,(K)}(0,\infty )$, where

$$C_{-1,(K)}(0,\infty ):=\{f:f(t)=I_{(K)}^t\left[t^{\prime }\right]g(t^{\prime }),g(t)\in C_{-1}(0,\infty )\}.$$

(7)

Theorem 1 is proved as Theorem 3 in [81] (p. 9) (see also Theorem 1 in [82] (p. 6)).

Theorem 2

(Second fundamental theorem of GFC). If a pair of kernels $(M(t),K(t))$ belongs to the Luchko set $\mathbb{L}$, then the equality

$$I_{(M)}^t\left[t^{\prime }\right]D_{(K)}^{t^{\prime },\ast }\left[t^{″}\right]\rho (t^{″})=\rho (t)-\rho (0)$$

(8)

holds for $\rho (t)\in C_{-1}^1(0,\infty )$, where

$$C_{-1}^1(0,\infty ):=\{f:f^{(1)}(t)\in C_{-1}(0,\infty )\}.$$

(9)

This theorem is proved as Theorem 4 in [81] (p. 11), (see also Theorem 2 in [82] (p. 7)).

2.2. Examples of Kernel Pairs

Let us give examples of the pair of kernels that belongs to the Sonin set $\mathbb{S}$ and the Luchko set $\mathbb{L}$. Note that if the kernel $M(t)$ is associated to kernel $K(t)$, then the kernel $K(t)$ is associated to $M(t)$. Therefore, if the operators

$$I_{(M)}^t\left[\tau \right]X(\tau )=(M\ast X)(t),D_{(K)}^{t,\ast }\left[\tau \right]X(\tau )=(K\ast X^{(1)})(t),$$

(10)

are considered for the kernel pair $(M(t),K(t))$ belongs to the Sonin set $\mathbb{S}$, then the following operators can also be used

$$I_{(K)}^t\left[\tau \right]X(\tau )=(K\ast X)(t),D_{(M)}^{t,\ast }\left[\tau \right]X(\tau )=(M\ast X^{(1)})(t).$$

(11)

A similar situation with the kernel pairs $(M(t),K(t))$ that belong to the Luchko set $\mathbb{L}$.

Let us describe the notation that are used in the

Table 1. Examples of the kernel pairs that belong to the Sonin set and Luchko set, where $0<\alpha <1$ and $0<\alpha <\beta <1$, $\lambda \ge 0$, $t>0$.

Kernel of GFI $M(t)$	Kernel of GFD $K(t)$: $(M\ast K)(t)=1$
${\displaystyle M(t)=h_{\alpha }(t)=\frac{t^{\alpha -1}}{\mathsf{\Gamma }(\alpha )}}$	${\displaystyle K(t)=h_{1-\alpha }(t)=\frac{t^{-\alpha }}{\mathsf{\Gamma }(1-\alpha )}}$
${\displaystyle M(t)=h_{\alpha ,\lambda }(t)=\frac{t^{\alpha -1}}{\mathsf{\Gamma }(\alpha )}{e}^{-\lambda t}}$	${\displaystyle K(t)=h_{1-\alpha ,\lambda }(t)+\frac{{\lambda }^{\alpha }}{\mathsf{\Gamma }(1-\alpha )}\gamma (1-\alpha ,\lambda t)}$
${\displaystyle M(t)={(\sqrt{t})}^{\alpha -1}{J}_{\alpha -1}(2\sqrt{t})}$	${\displaystyle K(t)={(\sqrt{t})}^{-\alpha }{I}_{-\alpha }(2\sqrt{t})}$
${\displaystyle M(t)=\frac{cos(2\sqrt{t})}{\sqrt{\pi t}}}$	${\displaystyle K(t)=\frac{cosh(2\sqrt{t})}{\sqrt{\pi t}}}$
${\displaystyle M(t)=t^{\alpha -1}\mathsf{\Phi }(\beta ,\alpha ;-\lambda t)}$	${\displaystyle K(t)=\frac{sin(\pi \alpha )}{\pi }{t}^{-\alpha }\mathsf{\Phi }(-\beta ,1-\alpha ;-\lambda t)}$
${\displaystyle M(t)=1+\frac{\lambda }{\mathsf{\Gamma }(\alpha )\sqrt{t}}}$	${\displaystyle K(t)=\frac{1}{\sqrt{\pi t}}-\lambda e^{{\lambda }^{2}t}\mathrm{erfc}(\lambda \sqrt{(}t))}$
${\displaystyle M(t)=1-\frac{\lambda }{\mathsf{\Gamma }(\alpha )}{t}^{\alpha -1}}$	${\displaystyle K(t)=\frac{1}{\sqrt{\pi t}}-\lambda t^{-\alpha }{E}_{1-\alpha ,1-\alpha }\left[\lambda t^{1-\alpha }\right]}$
${\displaystyle M(t)=h_{1-\beta +\alpha }(t)+h_{1-\beta }(t)}$	${\displaystyle K(t)=t^{\beta -1}{E}_{\alpha ,\beta }[-t^{\alpha }]}$

. The function $\gamma (\beta ,t)$ with $\beta >0$ is the incomplete gamma function

$$\gamma (\beta ,t)={\int }_0^t{\tau }^{\beta -1}{e}^{-\tau }d\tau .$$

(12)

The functions $J_{\nu }(t)$ and, $I_{\nu }(t)$ with $\nu >0$ are the Bessel and the modified Bessel functions, respectively,

$$J_{\nu }(t)=\sum _{k=0}^{\infty }\frac{{(-1)}^k{(t/2)}^{2k+\nu }}{k!\mathsf{\Gamma }(k+\nu +1)},I_{\nu }(t)=\sum _{k=0}^{\infty }\frac{{(t/2)}^{2k+\nu }}{k!\mathsf{\Gamma }(k+\nu +1)}.$$

(13)

The function $\mathsf{\Phi }(\beta ,\alpha ;z)$ is the Kummer function

$$\mathsf{\Phi }(\beta ,\alpha ;z)=\sum _{k=0}^{\infty }\frac{{(\beta )}_k}{{(\alpha )}_k}\frac{z^k}{k!}.$$

(14)

where ${(\beta )}_k$ and ${(\alpha )}_k$ are Pochhammer symbols. The function $\mathrm{erfc}(z)$ with $z\in \mathbb{C}$ is the complementary error function

$$\mathrm{erfc}(z)=1-\mathrm{erf}(z)=1-\frac{2}{\sqrt{\pi }}{\int }_0^t{e}^{-z^2}dz.$$

(15)

The function $E_{\alpha ,\beta }\left[z\right]$ is the two-parameters Mittag-Leffler function

$$E_{\alpha ,\beta }\left[z\right]=\sum _{k=0}^{\infty }\frac{z^k}{\mathsf{\Gamma }(\alpha k+\beta )},$$

(16)

where $\alpha >0$ and $\beta ,z\in \mathbb{C}$.

2.3. General Fractional Integral and Derivative for $[a,b]$

Let us define the general fractional integration and differentiation on $[a,b]$ with $0\le a<b$.

Definition 3.

Let $\rho (x^{\prime })\in C_{-1}(0,\infty )$ and $(M(x),K(x))\in \mathbb{L}$. Then, the general fractional integration on $[a,x]$ with $0\le a<x$ is defined by the equation

$$I_{[a,x]}^{(M)}\left[x^{\prime }\right]\rho (x^{\prime }):=I_{(M)}^x\left[x^{\prime }\right]\rho (x^{\prime })-I_{(M)}^a\left[x^{\prime }\right]\rho (x^{\prime })$$

(17)

if $x>a>0$ and $I_{[a,x]}^{(M)}\left[x^{\prime }\right]\rho (x^{\prime }):=I_{(M)}^x\left[x^{\prime }\right]\rho (x^{\prime })$ if $x>a=0$.

Let $\rho (x^{\prime })\in C_{-1}^1(0,\infty )$ and $(M(x),K(x))\in \mathbb{L}$. Then, the general fractional differentiation on $[a,x]$ with $0\le a<x$ is defined by the equation

$$D_{[a,x]}^{(K)}\left[x^{\prime }\right]\rho (x^{\prime }):=D_{(K)}^{x,\ast }\left[x^{\prime }\right]\rho (x^{\prime })-D_{(K)}^{a,\ast }\left[x^{\prime }\right]\rho (x^{\prime })$$

(18)

if $x>a>0$ and $D_{[a,x]}^{(K)}\left[x^{\prime }\right]\rho (x^{\prime }):=D_{(K)}^{x,\ast }\left[x^{\prime }\right]\rho (x^{\prime })$ if $x>a=0$.

For $x=b>a>0$, the general fractional integration on $[a,b]$ is given by the equation

$$I_{[a,b]}^{(M)}\left[x\right]\rho (x):={\int }_0^{b}dxM(b-x)\rho (x)-{\int }_0^{a}dxM(a-x)\rho (x).$$

(19)

The general fractional derivative on $[a,b]$ is given as

$$D_{[a,b]}^{(K)}\left[x\right]\rho (x):={\int }_0^{b}dxK(b-x){\rho }^{(1)}(x)-{\int }_0^{a}dxK(a-x){\rho }^{(1)}(x).$$

(20)

Let us formulate the fundamental theorems of the GFC for the operator $I_{[a,x]}^{(M)}\left[s\right]$.

Theorem 3

(The second fundamental theorem of GFC for $I_{(M)}^x$ and GFD $D_{(K)}^{x,\ast }$). Let $\rho (x)$ belongs to the space $C_{-1}^1(0,\infty )$, and the pair of kernels $(M(t),K(t))$ the Luchko set $\mathbb{L}$. Then

$$I_{[a,x]}^{(M)}\left[s\right]D_{(K)}^{s,\ast }\left[x^{\prime }\right]\rho (x^{\prime })=\rho (x)-\rho (a),$$

(21)

where $x>a\ge 0$.

Theorem 4

(The first fundamental theorem of GFC for $I_{(M)}^x$ and GFD $D_{(K)}^{x,\ast }$). Let us assume that the conditions of the first FT of GFC for the operators $I_{(M)}^x$ and GFD $D_{(K)}^{x,\ast }$ are satisfied. Then

$$D_{(K)}^{x,\ast }\left[s\right]I_{[a,s]}^{(M)}\left[x^{\prime }\right]\rho (x^{\prime })=\rho (x),$$

(22)

where it is used

$$D_{(K)}^{x,\ast }\left[s\right]1=0.$$

(23)

For operators $I_{(M)}^x\left[x\right]$ and $D_{[a,x]}^{(K)}$ with $a>0$, the first fundamental theorem GFC holds in the form

$$D_{[a,x]}^{(K)}\left[s\right]I_{(M)}^s\left[x^{\prime }\right]\rho (x^{\prime })=\rho (x)-\rho (a).$$

(24)

The second fundamental theorem GFC does not hold for the operators $I_{(M)}^x\left[x\right]$ and $D_{[a,x]}^{(K)}$ with $a>0$, since $I_{(M)}^x\left[x\right]1\ne 0$.

2.4. General Fractional Analogue of Titchmarsh Theorem

Let us give the Titchmarsh’s Theorem about the Laplace convolution.

Theorem 5

(Titchmarsh’s Theorem). Let $M(x)$ and $f(x)$ be real valued continuous functions, $x\in [0,\infty )$, such that

$$(M\ast f)(x)={\int }_0^{x}M(x-x^{\prime })f(x^{\prime })d{x}^{\prime }=0$$

(25)

vanishes identically, i.e., condition (25) holds for all $x\in [0,\infty )$. Then, either one of $M(x)$ or $f(x)$ must vanish identically, i.e., $f(x)=0$ for all $x\in [0,\infty )$.

Proof. 

The proof of this theorem without using the theory of functions of a complex variable is proposed in Part VI, Chapter VI, Section 8 in. [88] (p. 180) and Chapter VI, Section 5 in [89] (p. 166). This ends the proof. □

The first fundamental theorems of the GFC can be used to derive the GF differential form of general balance equations from the GF integral form of general balance equations. Let us formulate and prove a basic theorem that allows us to implement this derivation of GF differential form of nonlocal balance equations. This theorem can be regarded as an analogue of Titchmarsh’s theorem for general fractional calculus.

Theorem 6

(General fractional Titchmarsh’s theorem). Let the pair of kernels $(M(x),K(x))$ belongs to the Luchko set. Let $f(x)$ be real valued function such that $f(x)\in C_{-1,(K)}(0,\infty )$. If the condition

$$I_{[a,x]}^{(M)}\left[x^{\prime }\right]f(x^{\prime })=0$$

(26)

holds for all $x\in [0,\infty )$, where $x>a\ge 0$, Then, $f(x)=0$ for all $x\in [0,\infty )$.

Proof. 

The action of the generalized fractional derivative on equality (26) leads to the equation

$$D_{(K)}^{x,\ast }\left[s\right]I_{[a,s]}^{(M)}\left[x^{\prime }\right]f(x^{\prime })=0$$

(27)

since the action of the generalized fractional derivative on zero is equal to zero.

Let us use the first fundamental theorem of GFC in the form

$$D_{(K)}^{x,\ast }\left[s\right]I_{[a,s]}^{(M)}\left[x^{\prime }\right]f(x^{\prime })=f(x),$$

(28)

which holds for all $x\in [0,\infty )$, if $f(x)\in C_{-1,(K)}(0,\infty )$. Using (28), Equation (26) gives the equality

$$f(x)=0$$

(29)

that holds for $x\in [0,\infty )$. This ends the proof. □

2.5. Triple GFI

The following notation will be used

$${\mathbb{R}}_{+}^n=\{(x_1,\cdots ,x_n):x_1>0,\cdots ,x_n>0\},$$

(30)

$${\mathbb{R}}_{0,+}^n=\{(x_1,\cdots ,x_n):x_1\ge 0,\cdots ,x_n\ge 0\},$$

(31)

where ${\mathbb{R}}_{+}^1=(0,\infty )$.

Let us define concept of the Z-simple region W in ${\mathbb{R}}_{0,+}^3$.

Definition 4.

Let W be region in ${\mathbb{R}}_{0,+}^3$ that is bounded above and below by smooth surfaces $S_{2,xy}$, $S_{1,xy}$ and a lateral surface $S_z$, whose generatrices are parallel to the Z-axis. Let surfaces $S_{1,xy}$, $S_{2,xy}$ be described by the equations

$$z=z_1(x,y)\ge 0,z=z_2(x,y)\ge 0,$$

(32)

where the functions are continuous in the closed domain $W_{xy}$ that is a projection of the region W onto the $XY$-plane and $z_2(x,y)\ge z_1(x,y)$ for all $(x,y)\in W_{xy}$.

Then, the region W will be called the Z-simple region (simple area along the Z-axis). The region W is called simple, if W is simple along three axes (X, Y, Z). If W can be divided into a finite number of such regions with respect to all three axes, Then, W will be called piecewise simple region in ${\mathbb{R}}_{0,+}^3$.

Definition 5.

Let $W\subset {\mathbb{R}}_{0,+}^3$ be Z-simple domain that is bounded above and below by smooth surfaces $S_{2,xy}$, $S_{1,xy}$ described by Equation (32). Let $W_{xy}\subset {\mathbb{R}}_{0,+}^2$ be projection of W on the $XY$-plane such that $W_{xy}$ is Y-simple region in $XY$-plane that is bounded by the lines $y=y_2(x)$ and $y=y_1(x)$, where $y=y_2(x)$ and $y=y_1(x)$ are continuous functions on the interval $[a,b]$, $b>a\ge 0$ and $y_2(x)\ge y_1(x)>0$ for all $x\in [a,b]$.

Let the scalar field $\rho (x,y,z)$ be satisfy the conditions

$$I_{[z_1(x,y),z_2(x,y)]}^{(M_z)}\left[z\right]\rho (x,y,z)\in C_{-1}({\mathbb{R}}_{+}^2).$$

(33)

and

$$I_{[y_1(x),y_2(x)]}^{(M_y)}\left[y\right]I_{[z_1(x,y),z_2(x,y)]}^{(M_z)}\left[z\right]\rho (x,y,z)\in C_{-1}(0,\infty ).$$

(34)

Then, the triple general fractional integral (triple GFI) is defined in the form

$$I_W^{(M)}[x,y,z]\rho (x,y,z):=I_{[a,b]}^{(M_x)}\left[x\right]I_{[y_1(x),y_2(x)]}^{(M_y)}\left[y\right]I_{[z_1(x,y),z_2(x,y)]}^{(M_z)}\left[z\right]\rho (x,y,z).$$

(35)

2.6. Surface GFI

The surface GFI over the surface S in ${\mathbb{R}}_{0,+}^3$ is defined through the double GFI over areas $S_{xy}$, $S_{xz}$, $S_{yz}$ in the $XY$, $XZ$, $YZ$ planes, where these areas are projections of the surface S onto these planes.

Let us give definitions of piecewise simple surfaces, vector fields on these surfaces and the surface GFI.

Definition 6.

Let S be an oriented compact smooth surface in the region

$${\mathbb{R}}_{0,+}^3=\{(x,y,z):x\ge 0,y\ge 0,z\ge 0\}.$$

(36)

Let us choose a side of the surface S.

Let the surface S be represented as the union of a finite number of X-simple surfaces $S_{X,i}$, as well as a finite number of Y-simple surfaces $S_{X,j}$ and Z-simple surfaces $S_{Z,k}$, where $i=1,\cdots ,n_x$, $j=1,\cdots ,n_y$ and $k=1,\cdots ,n_z$ such that

$$S:=\bigcup _{k=1}^{n_x}{S}_{X,i}=\bigcup _{j=1}^{n_y}{S}_{Y,j}=\bigcup _{k=1}^{n_z}{S}_{Z,k}.$$

(37)

Let $S_{i,yz}$, $S_{j,xz}$, $S_{k,xy}$ be projections of the surfaces $S_{X,i}$, $S_{Y,j}$, $S_{Z,k}$ onto the $YZ$, $XZ$, $XY$ planes, which can be described by continuous functions

$$S_{X,i}=\{x=x_i(y,z)\ge 0\mathrm{if}(y,z)\in S_{i,yz}\subset {\mathbb{R}}_{0,+}^2\},$$

(38)

$$S_{Y,j}=\{y=y_j(x,z)\ge 0\mathrm{if}(x,z)\in S_{j,xz}\subset {\mathbb{R}}_{0,+}^2\},$$

(39)

$$S_{Z,k}=\{z=z_k(x,y)\ge 0\mathrm{if}(x,y)\in S_{k,xy}\subset {\mathbb{R}}_{0,+}^2\}.$$

(40)

Such surfaces S is called piecewise simple surfaces. The set of such surfaces is denoted by $\mathbb{P}({\mathbb{R}}_{0,+}^3)$.

Definition 7.

Let S be a piecewise simple surface ($S\in \mathbb{P}({\mathbb{R}}_{0,+}^3)$). Let the vector field

$$\mathbf{F}:={\mathbf{e}}_x{F}_x(x,y,z)+{\mathbf{e}}_y{F}_y(x,y,z)+{\mathbf{e}}_z{F}_z(x,y,z)$$

(41)

on the surface S be satisfy the conditions

$$F_x(x_i(y,z),y,z)\in C_{-1}({\mathbb{R}}_{+}^2),$$

(42)

$$F_y(x,y_j(x,z),z)\in C_{-1}({\mathbb{R}}_{+}^2),$$

(43)

$$F_z(x,y,z_k(x,y))\in C_{-1}({\mathbb{R}}_{+}^2)$$

(44)

for all $i=1,\cdots ,n_x$, $j=1,\cdots ,n_y$, $k=1,\cdots ,n_z$.

The set of such vector fields $\mathbf{F}$ on piecewise simple surface S is denoted by ${\mathbb{F}}_S({\mathbb{R}}_{0,+}^3)$.

Definition 8.

Let S be a piecewise simple surface ($S\in \mathbb{P}({\mathbb{R}}_{0,+}^3)$) and a vector fields $\mathbf{F}$ on this surface S belongs to the set ${\mathbb{F}}_S({\mathbb{R}}_{0,+}^3)$.

Then, the surface general fractional vector integral (surface GFI) of the second kind

$$\begin{array}{c}{\mathbf{I}}_S^{(M)}={\mathbf{e}}_x{I}_{S_{yz}}^{(M)}[y,z]+{\mathbf{e}}_y{I}_{S_{xz}}^{(M)}[z,x]+{\mathbf{e}}_z{I}_{S_{xy}}^{(M)}[x,y]=\\ {\mathbf{e}}_x{\displaystyle \sum _{i=1}^{n_x}}{I}_{S_{i,yz}}^{(M)}[y,z]+{\mathbf{e}}_y{\displaystyle \sum _{j=1}^{n_y}}{I}_{S_{j,xz}}^{(M)}[z,x]+{\mathbf{e}}_z{\displaystyle \sum _{k=1}^{n_z}}{I}_{S_{k,xy}}^{(M)}[x,y]\end{array}$$

(45)

for the vector fiels $\mathbf{F}\in {\mathbb{F}}_S({\mathbb{R}}_{0,+}^3)$ is defined by the equation

$$\begin{array}{c}({\mathbf{I}}_S^{(M)},\mathbf{F}):=\sum _{i=1}^{n_x}{I}_{S_{i,yz}}^{(M)}[y,z]F_x(x_i(y,z),y,z)+\\ {\displaystyle \sum _{j=1}^{n_y}}{I}_{S_{j,xz}}^{(M)}[x,z]F_y(x,y_j(x,z),z)+{\displaystyle \sum _{k=1}^{n_z}}{I}_{S_{k,xy}}^{(M)}[x,y]F_z(x,y,z_k(x,y)).\end{array}$$

(46)

Example 1.

Let us consider a two-sided smooth surface S and fix one of its two sides, which is equivalent to choosing a certain orientation on the surface. Let us also assume that the surface S is given by the equation

$$z=z(x,y)\ge 0,$$

(47)

where the point $(x,y)\in {\mathbb{R}}_{+,0}^2$ changes in area $S_{xy}$ in the $XY$-plane, bounded by smooth contour $\partial S_{xy}$ and

$$S:=\{(x,y,z):z=z(x,y)\ge 0,(x,y)\in S_{xy}\subset {\mathbb{R}}_{0,+}^2\}.$$

(48)

Let $\mathbf{F}$ be a vector field $\mathbf{F}:={\mathbf{e}}_z{F}_z(x,y,z)$, where $F_z(x,y,z(x,y))\in C_{-1}({\mathbb{R}}_{+}^2)$. Then, the surface GFI is defined as

$$\left({\mathbf{I}}_S^{(M)},\mathbf{F}\right)=I_{S_{xy}}^{(M)}[x,y]F_z(x,y,z(x,y)).$$

(49)

If $S_{xy}$ is region of the $XY$-plane such that

$$S_{xy}:=\{(x,y):0\le a\le x\le b,0\le y_1(x)\le y\le y_2(x)\},$$

(50)

where $y=y_1(x)$ and $y=y_2(x)$ are continuous functions, Then, the double GFI in Equation (49) is represented as

$$I_{S_{xy}}^{(M)}[x,y]F_z(x,y,z(x,y)):=I_{[a,b]}^{(M_1)}\left[x\right]I_{[y_1(x),y_2(x)]}^{(M_2)}\left[y\right]F_z(x,y,z(x,y)).$$

(51)

2.7. General Fractional Divergence

In this subsection, the definition of general fractional divergence for ${\mathbb{R}}_{0,+}^3$ is given.

Let us define sets of vector fields that is used in the definition of the regional general fractional divergence.

Definition 9.

Let $\mathbf{J}(x,y,z)$ be a vector field that satisfies the conditions

$$D_{(K_x)}^{x,\ast }\left[x^{\prime }\right]J_x(x^{\prime },y,z)\in C_{-1}({\mathbb{R}}_{+}^3),$$

(52)

$$D_{(K_y)}^{y,\ast }\left[y^{\prime }\right]J_y(x,y^{\prime },z)\in C_{-1}({\mathbb{R}}_{+}^3),$$

(53)

$$D_{(K_z)}^{z,\ast }\left[z^{\prime }\right]J_z(x,y,z^{\prime })\in C_{-1}({\mathbb{R}}_{+}^3).$$

(54)

Then, the set of such vector fields is denoted as ${\mathbb{F}}_{-1,Div}^1({\mathbb{R}}_{+}^3)$.

Let $\mathbf{J}(x,y,z)$ be a vector field that satisfies the conditions

$$J_x(x,y,z),J_y(x,y,z),J_z(x,y,z)\in C_{-1}^1({\mathbb{R}}_{+}^3).$$

(55)

Then, the set of such vector fields is denoted as $C_{-1}^1({\mathbb{R}}_{+}^3)$.

In other words, the condition $\mathbf{J}(x,y,z)\in C_{-1}^1({\mathbb{R}}_{+}^3)$ means that all general fractional derivatives of all components of the vector field $\mathbf{J}(x,y,z)$ with respect to all coordinates belong to space $C_{-1}({\mathbb{R}}_{+}^3)$.

Let us define the regional general fractional divergence.

Definition 10.

Let $\mathbf{J}(x,y,z)$ be a vector field

$$\mathbf{J}(x,y,z)=\sum _{k=1}^3{\mathbf{e}}_k{J}_k(x,y,z)$$

(56)

that belongs to the set ${\mathbb{F}}_{-1,Div}^1({\mathbb{R}}_{+}^3)$ or $C_{-1}^1({\mathbb{R}}_{+}^3)$.

Then, the general fractional divergence ${Div}_W^{(K)}$ for the region $W={\mathbb{R}}_{0,+}^3$ is defined as

$${Div}_W^{(K)}\mathbf{J}=D_{(K_x)}^{x,\ast }\left[x^{\prime }\right]J_x(x^{\prime },y,z)+D_{(K_y)}^{y,\ast }\left[y^{\prime }\right]J_y(x,y^{\prime },z)+D_{(K_z)}^{z,\ast }\left[z^{\prime }\right]J_z(x,y,z^{\prime }).$$

(57)

One can consider the general fractional nabla operator (del operator) that is defined by equation

$${\nabla }_W^{(K)}:=\sum _{j=1}^3{D}_{(K_j)}^{x_j,\ast }\left[{x^{\prime }}_j\right]\mathbf{F}$$

(58)

Using this operator, the GF divergence can be written as

$$\left({\nabla }_W^{(K)},\mathbf{F}\right):=\sum _{j=1}^3{D}_{(K_j)}^{x_j,\ast }\left[{x^{\prime }}_j\right]F_j.$$

(59)

where $\mathbf{F}\in {\mathbb{F}}_{-1,Div}^1({\mathbb{R}}_{+}^3)$ or $\mathbf{F}\in C_{-1}^1({\mathbb{R}}_{+}^3)$.

Remark 2.

It should be noted that the general fractional divergence can be defined not only as a regional operator for regions $W\subset {\mathbb{R}}_{0,+}^3$, but also can be defined for surfaces $S\subset {\mathbb{R}}_{0,+}^3$ and lines $L\subset {\mathbb{R}}_{0,+}^3$. This possibility is due to the fact that this operator is non-local and, in fact, it is an integro-differential operator. The general fractional nabla operator can also be defined for the $L,S,W\subset {\mathbb{R}}_{0,+}^3$, that corresponds to the lines, surfaces and regions in ${\mathbb{R}}_{0,+}^3$.

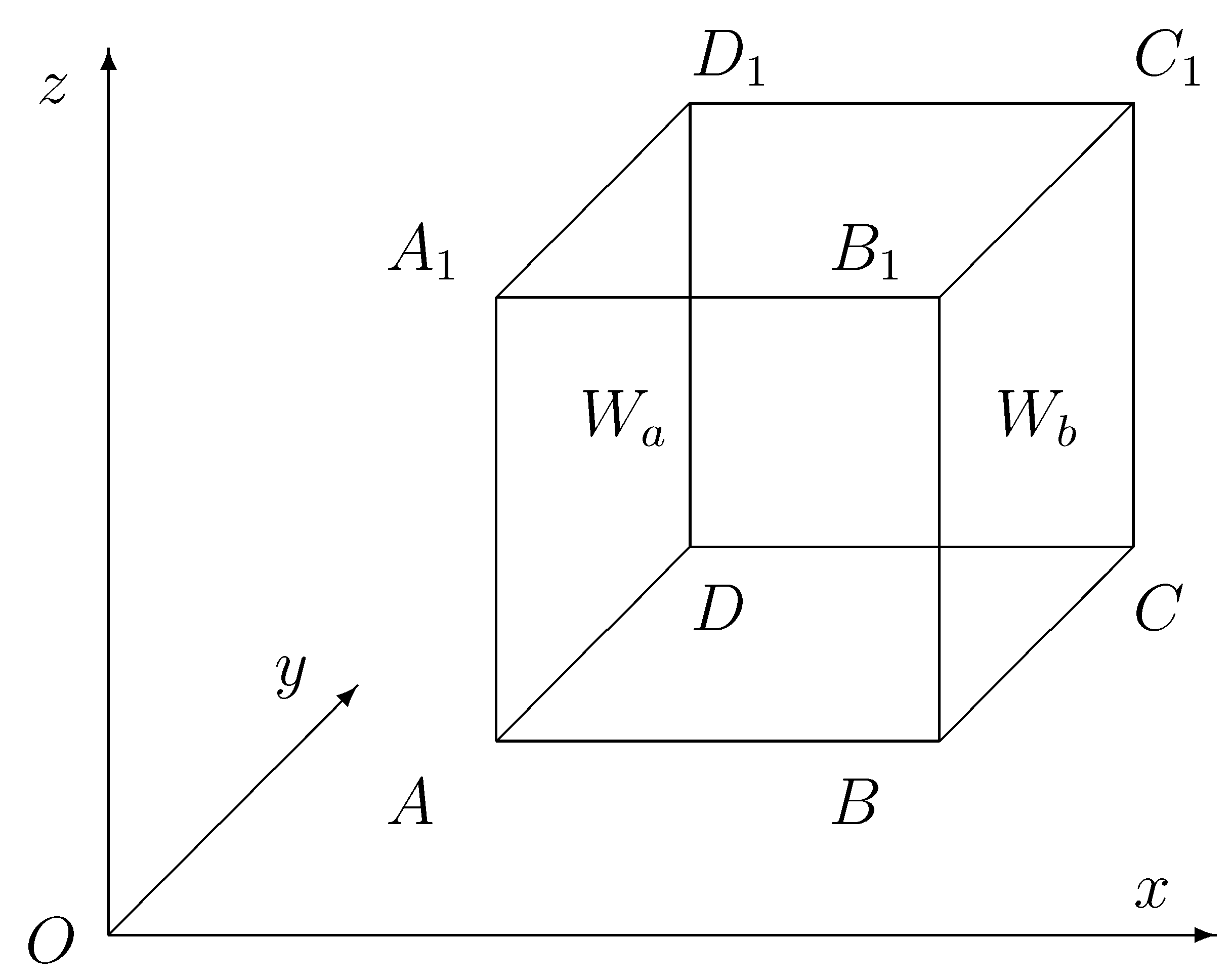
2.8. General Fractional Gauss Theorem for Z-Simple Region

The standard Gauss theorem (the Gauss-Ostrogradsky theorem) relates the flux of a vector field through a closed surface to the divergence of the field in the region enclosed. The Gauss theorem states that the surface integral of a vector field over a closed surface, which is the flux through the surface, is equal to the volume integral of the divergence over the region inside the surface.

Let us define a set of vector fields, for which general fractional Gauss theorem is formulated.

Definition 11.

Let W in ${\mathbb{R}}_{0,+}^3$ be Z-simple region such that W is a piecewise Y-simple and X-simple region

$$W=\bigcup _{k=1}^n{W}_{X,k}=\bigcup _{j=1}^m{W}_{Y,j},$$

(60)

where $W_{X,k}$ are the X-simple regions that is described by $x=x_{k,1}(y,z)$, $x=x_{k,2}(y,z)$ for $y,z\in D_{yz}$ and $W_{Y,j}$ are the Y-simple regions that are described by the functions $y=y_{j,1}(x,z)$, $y=y_{j,2}(x,z)$ for $(x,z)\in D_{xz}$.

Let vector field $\mathbf{J}(x,y,z)$ satisfy the conditions

$$J_x(x_{k,2}(y,z),y,z),J_x(x_{k,1}(y,z),y,z)\in C_{-1}({\mathbb{R}}_{+}^2),$$

(61)

$$J_y(x,y_{j,2}(x,z),z),J_y(x,y_{j,1}(x,z),z)\in C_{-1}({\mathbb{R}}_{+}^2),$$

(62)

$$J_z(x,y,z_1(x,y)),J_z(x,y,z_2(x,y))\in C_{-1}({\mathbb{R}}_{+}^2).$$

(63)

Then, the set of such vector fields $\mathbf{J}$ is denoted as $\mathbf{J}(x,y,z)\in {\mathbb{F}}_{-1}(\partial W)$.

Let vector field $\mathbf{J}(x,y,z)$ satisfy the conditions

$$D_{(K_x)}^{x,\ast }\left[x^{\prime }\right]J_x(x^{\prime },y,z)\in C_{-1}({\mathbb{R}}_{+}^3),$$

(64)

$$D_{(K_y)}^{y,\ast }\left[y^{\prime }\right]J_y(x,y^{\prime },z)\in C_{-1}({\mathbb{R}}_{+}^3),$$

(65)

$$D_{(K_z)}^{z,\ast }\left[z^{\prime }\right]J_z(x,y,z^{\prime })\in C_{-1}({\mathbb{R}}_{+}^3).$$

(66)

Then, the set of such vector field $\mathbf{J}$ is denoted as $\mathbf{J}(x,y,z)\in {\mathbb{F}}_{-1}^1({\mathbb{R}}_{+}^3)$.

Theorem 7

(General fractional Gauss theorem for Z-simple region). Let W in ${\mathbb{R}}_{0,+}^3$ be Z-simple region such that W is a piecewise Y-simple and X-simple region. Let W is bounded above and below by smooth surfaces $S_{2,xy}$, $S_{1,xy}$, which is described by Equation (32) and a lateral surface $S_z$, whose generatrices are parallel to the Z-axis.

Let the vector field $\mathbf{J}(x,y,z)$ belongs to the sets ${\mathbb{F}}_{-1}(W)$ and ${\mathbb{F}}_{-1}^1({\mathbb{R}}_{+}^3)$.

Then, the general fractional Gauss equation has the from

$$I_W^{(M)}[x,y,z]\left({Div}_W^{(K)}\mathbf{J}\right)=\left({\mathbf{I}}_{\partial W},\mathbf{J}\right)$$

(67)

that can be written as

$$\begin{array}{c}{I}_W^{(M)}[x,y,z]\left(D_{(K_x)}^{x,\ast }\left[x^{\prime }\right]J_x(x^{\prime },y,z)+D_{(K_y)}^{y,\ast }\left[y^{\prime }\right]J_y(x,y^{\prime },z)+D_{(K_z)}^{z,\ast }\left[z^{\prime }\right]J_z(x,y,z^{\prime })\right)=\\ I_S^{(M)}[y,z]J_x(x,y,z)+I_S^{(M)}[x,y]J_y(x,y,z)+I_S^{(M)}[x,y]J_z(x,y,z).\end{array}$$

(68)

Proof. 

This theorem was proved in [86].

The general fractional Gauss theorem is also satisfied for regions $W\subset {\mathbb{R}}_{0,+}^3$ that can be represented as unions of the Z-simple regions $W_k$, which are piecewise Y-simple and X-simple regions in ${\mathbb{R}}_{0,+}^3$. □

For parallelepiped regions, a general fractional Gauss theorem can be formulated in the following form.

Theorem 8

(General fractional Gauss theorem for Gauus’s theorem for parallelepiped region). Let $J_x(x,y,z)$, $J_y(x,y,z)$, $J_z(x,y,z)$ belong to the function space ${\mathbb{F}}_{-1}^1({\mathbb{R}}_{+}^3)$ and the region $W\subset {\mathbb{R}}_{0,+}^3$ has the form of the parallelepiped

$$W:=\{(x,y,z):0\le a\le x\le b,0\le c\le y\le d,0\le e\le z\le f\}.$$

(69)

If the boundary of W be a closed surface $S=\partial W$, then

$$\left({\mathbf{I}}_{\partial W}^{(M)},\mathbf{J}\right)=I_W^{(M)}{Div}_W^{(K)}\mathbf{J}.$$

(70)

For the Cartesian coordinates, the vector field $\mathbf{J}=J_x{\mathbf{e}}_x+J_y{\mathbf{e}}_y+J_z{\mathbf{e}}_z$ and the GFI operators

$$I_W^{(M)}=I_W^{(M)}[x,y,z],{\mathbf{I}}_{\partial W}^{(M)}={\mathbf{e}}_x{I}_{\partial W}^{(M)}[y,z]+{\mathbf{e}}_y{I}_{\partial W}^{(M)}[x,z]+{\mathbf{e}}_z{I}_{\partial W}^{(M)}[x,y].$$

(71)

expressions of Equation (70) have the form

$$\left({\mathbf{I}}_{\partial W}^{(M)},\mathbf{J}\right)=I_{S_{yz}}^{(M)}[y,z]J_x+I_{S_{xz}}^{(M)}[x,z]J_y+I_{S_{xy}}^{(M)}[x,y]J_z,$$

(72)

and

$$I_W^{(M)}{Div}_W^{(K)}\mathbf{F}=I_W^{(M)}[x,y,z]\left(D_{(K_x)}^{x,\ast }\left[x^{\prime }\right]F_x+D_{(K_y)}^{y,\ast }\left[y^{\prime }\right]F_y+D_{(K_z)}^{z,\ast }\left[z^{\prime }\right]F_z\right),$$

(73)

where $S_{xy}$, $S_{xz}$, $S_{yz}$ are projections of $S=\partial W$ into $XY$, $XZ$, $YZ$ planes.

Proof. 

This theorem was proved in [86]. □

3. Concepts of General Nonlocal Continuum

3.1. General Density Function

At the beginning, for simplicity, the one-dimensional case of mass distribution is considered. Let the medium be distributed along the line (beam) ${\mathbb{R}}_{0,+}=[0,\infty )$. One can consider the function $m(x)\in C^1[0,\infty )$ that describes the mass of the region $W_1=[0,x]$, where $x>0$. The real-valued continuous function

$${\rho }_s(x):=\frac{dm(x)}{dx}$$

(74)

describes the standard linear density of the medium. The function ${\rho }_s(x)\in C[0,\infty )$ allows us to describe the mass of the region $W=[a,b]\subset {\mathbb{R}}_{0,+}$ and the mass $m(x)$ by the equations

$$m([a,b])={\int }_a^b{\rho }_s(x)dx,$$

(75)

and

$$m(x)={\int }_0^x{\rho }_s(x)dx.$$

(76)

Substitution of Equation (76) into Equation (74) gives identity by virtue of the first fundamental theorem of calculus, that states the equality

$$\frac{d}{dx}{\int }_0^{x}f(x^{\prime })d{x}^{\prime }=f(x),$$

(77)

if $f(x)$ is continuous real-valued function on a closed interval $[0,x_0]$ and $0\le x\le x_0$.

Let us define the real-valued function

$$\rho (x)={\int }_0^{x}K(x-x^{\prime })m^{(1)}(x^{\prime })d{x}^{\prime },$$

(78)

if the function $m(x)$ belongs to the space $C_{-1}^1(0,\infty )$.

If the kernels $K(x)$ is equal to the Dirac delta-function

$$K_x(x-x^{\prime })=\delta (x-x^{\prime }),$$

(79)

Then, Equation (78) takes the standard form (74).

If the kernel $K(x)$ belongs the function space $C_{-1,0}(0,\infty )$ and together with the kernel $M_x(x)$ form a kernel pair from the Luchko set. Then, Equation (78) can be written in the form

$$\rho (x)=D_{(K_x)}^{x,\ast }\left[x^{\prime }\right]m(x^{\prime }),$$

(80)

where $D_{(K_x)}^{x,\ast }\left[x^{\prime }\right]$ is the general fractional derivative.

The function $\rho (x)$, which is defined by Equation (80), can be interpreted as a general density function. In this case, the function $\rho (x)$ belongs to the space $C_{-1}(0,\infty )$.

The function (78) can be used to describe mass of the region $W=[a,b]\subset {\mathbb{R}}_{0,+}$ and the mass $m(x)$ by the equations

$$m([a,b])={\int }_0^b{M}_x(b-x)\rho (x)dx-{\int }_0^a{M}_x(a-x)\rho (x)dx,$$

(81)

and

$$m(x)={\int }_0^x{M}_x(x-x^{\prime })\rho (x^{\prime })d{x}^{\prime }.$$

(82)

If the kernel $M_x(x)$ is equal to 1. Then, Equations (81) and (82) take the standard form (75) and (76), respectively.

It should be note that substitution of Equation (82) into Equation (78) can give an identity only under certain conditions on the kernels $M_x(x)$ and $K_x(x)$ of these operators. This condition is that these kernels form a pair belonging to the Luchko set. In this case, the integral operators are general fractional integrals. Therefore, it is necessary to use function (80) that can be used to describe mass of the region $W=[a,b]\subset {\mathbb{R}}_{0,+}$ and the mass $m(x)$ by equations

$$m([a,b])=I_{[a,b]}^{(M_x)}\left[x\right]\rho (x)={\int }_0^b{M}_x(b-x)\rho (x)dx-{\int }_0^a{M}_x(a-x)\rho (x)dx,$$

(83)

and

$$m(x)=I_{(M_x)}^x\left[x^{\prime }\right]\rho (x^{\prime })={\int }_0^x{M}_x(x-x^{\prime })\rho (x^{\prime })d{x}^{\prime }.$$

(84)

Substitution of Equation (84) into Equation (80) gives identity by virtue of the first fundamental theorem of the general fractional calculus (see Theorems 1 and 4).

3.2. General Nonlocal Continuum

The concept of the general density function (80) allows us to consider a general nonlocal continuum (GNC). From the point of view of the mathematical approach, the one-dimensional linear GNC is a continuum, nonlocality of which can be described by the pair of kernels from the Luchko sets, but cannot be described by the kernel pairs $(M_x(x)=1,K_x(x)=\delta (x))$.

The general density and other fields of continuum are functions of the time variable t and the space coordinates $(x,y,z)$. In this case, the general density function is $\rho =\rho (t,x,y,z)$.

For the region

$$W_0:=\{(x^{\prime },y^{\prime },z^{\prime }):0\le x^{\prime }\le x,0\le y^{\prime }\le y,0\le z^{\prime }\le z\},$$

(85)

the mass of the continuum in the region $W_0$ is described by equation

$$m(t,x,y,z)=I_{(M_x)}^x\left[x^{\prime }\right]I_{(M_y)}^y\left[y^{\prime }\right]I_{(M_z)}^z\left[z^{\prime }\right]\rho (t,x^{\prime },y^{\prime },z^{\prime })=I_{W_0}^{(M)}[x^{\prime },y,z^{\prime }]\rho (t,x^{\prime },y^{\prime },z^{\prime }).$$

(86)

Then, the density function is described by the expression

$$\rho (t,x,y,z)=D_{(K_x)}^{x,\ast }\left[x^{\prime }\right]D_{(K_y)}^{y,\ast }\left[y^{\prime }\right]D_{(K_z)}^{z,\ast }\left[z^{\prime }\right]m(t,x^{\prime },y^{\prime },z^{\prime }).$$

(87)

Proposed Equations (86) and (87) can be written for the wide class of regions $W\subset {\mathbb{R}}_{0,+}^3$. For example, one can use the regions W that can be represented as a union of all the Z-simple region.

As a result, it is possible to formulate the concept of a general nonlocal continuum (GNC) with nonlocality in space and time. Note that the nonlocality in time can be interpreted as a memory in many cases.

Definition 12.

From the point of view of mathematics, the GNC is a continuum, nonlocality of which can be described by the pair of kernels from the Luchko sets, but cannot be described by the kernel pairs $(M_t(t)=1,K_t(t)=\delta (t))$, $(M_j(x_j)=1,K_j(x_j)=\delta (x_j))$, where $j=1,2,3$. From physical point of view, nonlocal continuum is a medium whose behavior at any interior point in space and time depends on the state of all other points in the medium in addition to its own state and the state of external fields.

Using the concept of GNC and the concepts of the generalized density of physical quantities (fields of a non-local continuum medium), balance equations for mass, momentum, and energy can be derived for such media.

Note that a microstructural basis of nonlocal properties of continuum is the assumption that the forces between material points are a long-range type, thus reflecting the long-range character of inter-atomic forces. Microstructural models of general nonlocal continuum can be formulated as models of discrete systems with long-range interactions, frequency and spatial dispersion [5,27].

3.3. Nonlocality of Mass in Continuum

For the first time, the continuity equation, which expresses the law of conservation of mass for nonlocal media, was obtained by Wheatcraft and Meerschaert in [90] for the media with power-law nonlocality (see also [91,92]). The proposed equation of mass conservation is a fractional differential equation with the Caputo fractional derivative with respect to space coordinates. The nonlocality in time variable, which can be interpreted as a memory, is not considered in this work and the derivative with respect to time has the first order. In paper [90], authors used a generalization of the standard derivation method for the continuity equation, that is based on the Taylor series with integer derivatives (for example, see Chapters 2 and 3 in [93,94]). In work [90], the fractional generalization of the Taylor formula in the Odibat-Shawagfeh form [95] is used.

The main restrictions of the standard mass the continuity equation are the following:

(A)

First, the standard derivation method that applies to local media is valid for flux fields, in which flux changes are small and linear or piecewise linear within the fixed region W.

(B)

The size of the fixed area and the scale of the measurements must be large compared to the scale of the heterogeneity in the medium. These restrictions are necessary due to the fact that changes in the flow in the fixed region W can be approximated by the Taylor series with derivatives of first orders.

It was shown in [90] that the fractional Taylor series can be an exact representation of a nonlinear flow with a power-law nonlinearity, using only the first two terms of this series. Using the fractional Taylor series to describe the change in flux through a fixed region, fractional differential equations describing the conservation of mass with power nonlocality were obtained in [90]. The proposed equation does not contain the described restrictions of the standard mass conservation equation.

However, it should be noted that the use of fractional Taylor series to obtain balance equations for non-local media has significant drawbacks. To do this, consider the generalization of the Taylor series, which was proposed in [95].

The function $f(x)$ with $x\in [a,b]$ can be expanded by using the generalized Taylor series with the left-sided Caputo derivatives of order $0<\alpha \le 1$ in the form

$$f(x)=f(a)+\frac{{(x-a)}^{\alpha }}{\mathsf{\Gamma }(\alpha +1)}\left(D_{C;a+}^{\alpha }f\right)(a)+R_{2\alpha }(x,a+),$$

(88)

where

$$\left(D_{C;a+}^{\alpha }f\right)(a):=\underset{x\to a}{lim}{}_a^C{D}_x^{\alpha }\left[x^{\prime }\right]f(x^{\prime })$$

(89)

and $R_{N\alpha }(x,a+)$ is the remainder term, which can be represented in the form

$$R_{2\alpha }(x,a+)=\frac{\left({\left(D_{C;a+}^{\alpha }\right)}^{2}f\right)\left({\xi }_{+}\right)}{\mathsf{\Gamma }\left(2\alpha +1\right)}{(x-a)}^{2\alpha },$$

(90)

where $a\le {\xi }_{+}\le x$. The generalized Taylor series (88) is applicable to functions $f(x)$ that satisfy the condition

$$\left({\left(D_{C;a+}^{\alpha }\right)}^{k}f\right)(x)\in C[a,b],$$

(91)

for $k=0,1,2$.

Remark 3.

Note that there is a problem in application of Equation (88), which is caused by the coincidence of the initial and final values in the derivatives $\left(D_{C;a+}^{\alpha }f\right)(a)$ (see Equation (89)). To derive correct balance equations by using the Taylor series, it is necessary to use a fractional Taylor series at an arbitrary point $x_0$, which does not coincide with the initial point of fractional derivative $D_{C;a+}^{\alpha }$, i.e., $x_0\ne a$. In this case, it is possible to avoid some restrictions on a possible application of Equation (88). To solve this problem, a generalization of the Taylor formulas (88) is proposed to the case when the Caputo derivative is considered at an arbitrary point $x_0\ge a$ (see Sections 3.3 and 4.2 in book [96]). However, in this case, the remainder term will be represented as the sum of the remainder terms of form (90) for different points and therefore such a term cannot be considered as a small value and it cannot be neglected in the general case.

As a result, the proposed approach, which is based on the fractional Taylor series, has some drawbacks due to the properties of the coefficients of the fractional Taylor series and the properties of the media.

(1)

First, for local media, it is quite logical that the size of a fixed region tends to zero, and thus an infinitely small region of medium can be used. However, for nonlocal media, this procedure is not entirely logical.

(2)

Second, the coefficients of the Taylor series are described as fractional derivatives from a to some upper limit, which tends to a even for finite fixed regions. This leads to the fact that the mass balance equation for a nonlocal medium should be described only by fractional derivatives on the infinitesimal interval $[a,a+ϵ)$ with $ϵ\to 0$. A possible approach to solve this problem is proposed in [92] for power-law nonlocality. If using the Taylor series to express in terms of fractional derivatives on a finite interval. Then, the remainder (more precisely, the difference between the two remainder terms) will not tend to zero and these terms cannot be neglected (see Remark 3).

(3)

Third, the equations were obtained only for the power-law type of nonlocality of the medium. In addition to this, it should also be emphasized that in article [90] other laws of conservation of nonlocal media were not derived.

Remark 4.

It should be noted that the use of the fractional derivative of the non-integer order is actually equivalent to using an infinite number of derivatives of integer orders, which assume arbitrarily large values. For example, the Riemann-Liouville derivative [15,18] can be represented in the form of the infinite series

$$({}^{RL}{D}_{a+}^{\alpha }f)(x)=\sum _{k=0}^{\infty }\frac{\mathsf{\Gamma }(\alpha +1)}{\mathsf{\Gamma }(k+1)\mathsf{\Gamma }(\alpha -k+1)}\frac{{(x-a)}^{k-\alpha }}{\mathsf{\Gamma }(k-\alpha +1)}{D}_x^{k}f(x)$$

(92)

for analytic functions on $(a,b)$, (see Lemma 15.3 in [15]). Using Equation 2.4.6 in [18], of the form

$$({}_a^C{D}_x^{\alpha }f)(x)=({}_a^{RL}{D}_x^{\alpha }f)(x)-\sum _{k=0}^{n-1}\frac{{(x-a)}^{k-\alpha }}{\mathsf{\Gamma }(k-\alpha +1)}(D^{k}f)(a)$$

(93)

and Equation (92), the Caputo derivative of the non-integer order can also be represented by the infinite series of derivatives of integer orders. Therefore, the use of fractional derivatives means that a contribution of derivatives of all integer orders is taken into account with weights of the power-law type. This fact creates additional difficulties for using the fractional Taylor series to obtain balance equations for non-local media. Moreover, this approach does not allow one to obtain balance equations in integral form and for a finite region of a non-local medium.

By virtue of the above arguments, it is important to mathematically and consistently derive all the conservation laws and obtain balance equations for nonlocal media with a general form of nonlocality. This conclusion became possible due to the creation of a general fractional calculus in the form proposed by Luchko in [81,82,83,84,85] and a general fractional vector calculus in [86].

4. General Nonlocal Continuity Equation

In this section, the motion of a nonlocal medium in a stationary reference frame with a fixed Cartesian coordinate system is considered. The Euler approach for description of continuum is used. Balance equation for mass of general nonlocal continuum is a mathematical formulation of the conservation law applied to the fixed region of the nonlocal continuum.

Let us select an region $W\subset {\mathbb{R}}_{0,+}^3$ of continuum in the form of a parallelepiped $AB\cdots C_1{D}_1$ (see Figure 1) with the coordinates of the vertices are the following

$$A(a,c,e),B(b,c,e),C(b,d,e),D(a,d,e),$$

(94)

$$A_1(a,c,f),B_1(b,c,f),C_1(b,d,f),D_1(a,d,f),$$

(95)

where $b>a\ge 0$, $d>c\ge 0$, $f>e\ge 0$ and the sizes of the edges

$$\Delta x=\left|AB\right|=\left|DC\right|=|A_1{B}_1|=|D_1{C}_1|,$$

(96)

$$\Delta y=\left|AD\right|=\left|BC\right|=|A_1{D}_1|=|B_1{C}_1|,$$

(97)

$$\Delta z=|A{A}_1|=|B{B}_1|=|C{C}_1|=|D{D}_1|.$$

(98)

where $\Delta x=b-a\ge$, $\Delta y=d-c\ge 0$, $\Delta z=f-e\ge 0$.

Let point $S\in W$ have coordinates $(x,y,z)$ such that $x\in [a,b]$, $y\in [c,d]$ and $z\in [e,f]$. Let us consider the density $\rho =\rho (t,x,y,z)$, the velocity $\mathbf{V}=\mathbf{V}(t,x,y,z)$ and the flow $\mathbf{J}(t,x,y,z)=\rho (t,x,y,z)\mathbf{V}(t,x,y,z)$ of mass at the point $S(x,y,z)$.

In this section, a connection between the density and the velocity of a continuous medium in the nonlocal case is derived by assuming the velocity $\mathbf{V}(t,x,y,z)$ and density $\rho (t,x,y,z)$ to be continuous functions of time and coordinates that belong to the function space $C^1({\mathbb{R}}_{0,+}^4)$.

In general, the following conditions are assumed

$$\rho (t,x,y,z)\in C_{-1}^{\{1,0\}}({\mathbb{R}}_{+}^4),$$

(99)

and

$$J_k(t,x,y,z)\in C_{-1}^{\{0,1\}}({\mathbb{R}}_{+}^4),$$

(100)

where

$$J_k(t,x,y,z)=\rho (t,x,y,z)V_k(t,x,y,z).$$

(101)

The function spaces $C_{-1}^{\{n,m\}}({\mathbb{R}}_{+}^4)$ are defined in the next subsection.

Note that $C(0,\infty )\subset C_{-1}(0,\infty )$. Therefore the continuous functions of time and coordinates can be considered as special cases of the general approach.

4.1. Function Spaces for Derivation of Balance Equations

Let us define two function spaces, which will be used in the theorems about the balance equations for general nonlocal media. The first type of spaces ($C_{-1}^{\{n,m\}}({\mathbb{R}}_{+}^4)$) will be used in derivation of the balance equations in the general integral form. The second function space ($C_{-1,(K)}({\mathbb{R}}_{+}^4)$ will be used in derivation equations in the general differential forms from integral form.

Definition 13.

The function $f(t,x,y,z)$ belongs to the space $C_{-1}^{\{n,m\}}({\mathbb{R}}_{+}^4)$, if this function satisfies the properties:

(1) Property with respect to time variable t:

$$\frac{{\partial }^{n}f(t,x,y,z)}{\partial t^n}\in C_{-1}({\mathbb{R}}_{+}^4)$$

(102)

This condition means that $f(t,x,y,z)\in C_{-1}^n(0,\infty )$ for all $(x,y,z)\in {\mathbb{R}}_{0,+}^3$.

(2) Property with respect to the space variables $(x,y,z)$:

$$\frac{{\partial }^{m}f(t,x,y,z)}{\partial x_j^m}\in C_{-1}({\mathbb{R}}_{+}^4)(j=1,2,3).$$

(103)

This condition means that $f(t,x,y,z)\in C_{-1}^m({\mathbb{R}}_{+}^3)$ for all $t\in {\mathbb{R}}_{0,+}$.

Definition 14.

The function $f(t,x,y,z)$ belongs to the space $C_{-1,(K)}({\mathbb{R}}_{+}^4)$, if there is such a function $g(t,x,y,z)\in C_{-1}({\mathbb{R}}_{+}^4)$ that the equality

$$f(t,x,y,z)=I_{(K_t)}^t\left[t^{\prime }\right]I_{(K_x)}^x\left[x^{\prime }\right]I_{(K_y)}^y\left[y^{\prime }\right]I_{(K_y)}^y\left[y^{\prime }\right]g(t^{\prime },x^{\prime },y^{\prime },z^{\prime })$$

(104)

holds for all $(t,x,y,z)\in {\mathbb{R}}_{0,+}^4$, i.e.

$$C_{-1,(K)}({\mathbb{R}}_{+}^4):=\{f:f(t,x,y,z)=I_{(K_t)}^t\left[t^{\prime }\right]I_{(K_x)}^x\left[x^{\prime }\right]I_{(K_y)}^y\left[y^{\prime }\right]I_{(K_y)}^y\left[y^{\prime }\right]g(t^{\prime },x^{\prime },y^{\prime },z^{\prime }),$$

$$g(t,x,y,z)\in C_{-1}({\mathbb{R}}_{+}^4)\}.$$

(105)

Note that if $f(t,x,y,z)$ belongs to the space $C_{-1,(K)}({\mathbb{R}}_{+}^4)$, Then, the integral eqiuation

$$I_{(M_t)}^t\left[t^{\prime }\right]I_{(M_x)}^x\left[x^{\prime }\right]I_{(M_y)}^y\left[y^{\prime }\right]I_{(M_z)}^y\left[z^{\prime }\right]f(t^{\prime },x^{\prime },y^{\prime },z^{\prime })=0,$$

(106)

gives

$$f(t,x,y,z)=0$$

(107)

for all $(t,x,y,z)\in {\mathbb{R}}_{0,+}^4$.

4.2. Mass of Nonlocal Continuum

Let the density $\rho =\rho (t,x,y,z)$ as a function of time $t\ge 0$ belong to the space $C_{-1}^1(0,\infty )$ for all $(x,y,z)\in {\mathbb{R}}_{0,+}^3$.

The mass of nonlocal medium in the parallelepiped region $W\subset {\mathbb{R}}_{0,+}^3$ is given by the equation

$$m(t)=I_W^{(M)}[x,y,z]\rho (t,x,y,z)=$$

(108)

$$I_{[a,b]}^{(M_x)}\left[x\right]I_{[c,d]}^{(M_y)}\left[y\right]I_{[e,f]}^{(M_z)}\left[z\right]\rho (t,x,y,z).$$

(109)

A discussion of the justification for equations of mass nonlocality from the physical point of view is considered in Section 3.3.

If the kernels $M_k(x_k)$ with $k=1,2,3$ have the form

$$M_k(x_k)=h_{{\alpha }_k}(x_k)=\frac{1}{\mathsf{\Gamma }({\alpha }_k)}{x}_k^{{\alpha }_k-1},$$

(110)

where $0<{\alpha }_k\le 1$, Equation (109) gives equation for nonlocal media with power-law nonlocality. If all $M_k(a_k)=1$, Equation (109) takes the standard equation of local continuum in the form

$$m(t)={\int }_{W}dxdydz\rho (t,x,y,z)={\int }_a^{b}dx{\int }_c^{d}dy{\int }_e^{f}dz\rho (t,x,y,z).$$

(111)

The mass of the medium in the region W at the time $t=t_1\ge 0$ is determined by equation

$$m(t_1)=I_W^{(M)}[x,y,z]\rho (t_1,x,y,z)=I_{[a,b]}^{(M_x)}\left[x\right]I_{[c,d]}^{(M_y)}\left[y\right]I_{[e,f]}^{(M_z)}\left[z\right]\rho (t_1,x,y,z),$$

(112)

where $\Delta x_k=b_k-a_k$.

At the next moment in time $t=t_2=t_1+\Delta t$ the mass of the selected region is equal to

$$m(t_2)=I_W^{(M)}[x,y,z]\rho (t_2,x,y,z)=I_{[a,b]}^{(M_x)}\left[x\right]I_{[c,d]}^{(M_y)}\left[y\right]I_{[e,f]}^{(M_z)}\left[z\right]\rho (t_2,x,y,z).$$

(113)

Thus, due to a change in the density of the medium in a given fixed region W, the mass during the time $\Delta t=t_2-t_1$ is changed by the value

$$\Delta m_t=m(t_2)-m(t_1)=I_W^{(M)}[x,y,z]\left(\rho (t_2,x,y,z)-\rho (t_1,x,y,z)\right).$$

(114)

The fundamental theorem of the GFC [81] in the form

$$I_{[t_1,t_2]}^{(M_t)}\left[t\right]D_{(K_t)}^{t,\ast }\left[\tau \right]\rho (\tau ,x,y,z)=\rho (t_2,x,y,z)-\rho (t_1,x,y,z)$$

(115)

can be used in Equation (114) to obtain the expression

$$\Delta m_t=I_W^{(M)}[x,y,z]I_{[t_1,t_2]}^{(M_t)}\left[t\right]D_{(K_t)}^{t,\ast }\left[\tau \right]\rho (\tau ,x,y,z).$$

(116)

Equation (116) describes the changes of mass due to change of medium density in the fixed region $W\subset {\mathbb{R}}_{+,0}^3$ during the time $\Delta t$.

4.3. Mass Flow of Nonlocal Continuum

Let us consider the density $\rho (t,x,y,z)$, the velocity $\mathbf{V}(t,x,y,z)$ and the flow $\mathbf{J}(t,x,y,z)=\rho (t,x,y,z)\mathbf{V}(t,x,y,z)$ of mass at the point $S(x,y,z)\in W$.

The mass change in the fixed region W will occur due to its mass transfer across the boundaries of the region. Let us now calculate the change in mass in the area W during the time $\Delta t=t_2-t_1$, when it is transferred across the boundaries of the region W.

The medium mass, which is flow through the face $W_a=AD{D}_1{A}_1$ for the time $\Delta t$ in the direction of the $OX$-axis, is given by equation

$$m_a=I_{[t_1,t_2]}^{(M_t)}\left[t\right]J_x(t,a),$$

(117)

where $J_x(t,a)$ is the flow of mass through the face $W_a$ of the parallelepiped W such that

$$J_x(t,a)=I_{W_a}^{(M)}[y,z]J_x(t,a,y,z).$$

(118)

Here $J_x(t,x,y,z)=\rho V_x$, where $\rho =\rho (t,x,y,z)$ and $V_x=V_x(t,x,y,z)$.

The mass, which is transported through the opposite face $W_b=BC{C}_1{B}_1$ in time $\Delta t$ in the direction of the $OX$-axis, is given by the equation

$$m_b=I_{[t_1,t_2]}^{(M_t)}\left[t\right]J_x(t,b).$$

(119)

Let us assume that the mass flowing into the region is positive and the mass flowing out negative. Then, the change in mass in the region W, when the mass is transferred through the $W_a$ and $W_b$ faces (see Figure 1) perpendicular to the $OX$-axis, is equal to

$$\Delta m_x=m_b-m_a=I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_{\partial W_{yz}}^{(M)}[y,z]\left(J_x(t,b,y,z)-J_x(t,a,y,z)\right),$$

(120)

where

$$\partial W_{yz}=W_a\bigcup W_b=A{A}_1{D}_{1}D\bigcup B{B}_1{C}_{1}C.$$

(121)

The fundamental theorem of the GFC in the form

$$I_{[a,b]}^{(M_x)}\left[x\right]D_{(K_x)}^{x,\ast }\left[x^{\prime }\right]J_x(t,x^{\prime },y,z)=J_x(t,b,y,z)-J_x(t,a,y,z),$$

(122)

can be used in Equation (120) to obtain the expression

$$\Delta m_x=I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_{\partial W_{yz}}^{(M)}[y,z]I_{[a,b]}^{(M_x)}\left[x\right]D_{(K_x)}^{x,\ast }\left[x^{\prime }\right]J_x(t,x^{\prime },y,z)=$$

$$I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_W^{(M)}[x,y,z]D_{(K_x)}^{x,\ast }\left[x^{\prime }\right]J_x(t,x^{\prime },y,z).$$

(123)

Considering similarly, it is possible to obtain the mass change in the region W through the faces that are perpendicular to $OY$ and $OZ$ axes. Reasoning in a similar way, the mass that is transferred through the faces perpendicular to the axes $OY$ and $OZ$, has the form, respectively:

$$\Delta m_y=I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_{\partial W_{xz}}^{(M)}[x,z]I_{[c,d]}^{(M_y)}\left[y\right]D_{(K_y)}^{y,\ast }\left[y^{\prime }\right]J_y(t,x,y^{\prime },z)=$$

$$I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_W^{(M)}[x,y,z]D_{(K_y)}^{y,\ast }\left[y^{\prime }\right]J_y(t,x,y^{\prime },z),$$

(124)

$$\Delta m_z=I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_{\partial W_{xy}}^{(M)}[x,y]I_{[e,f]}^{(M_z)}\left[z\right]D_{(K_z)}^{z,\ast }\left[z^{\prime }\right]J_z(t,x,y,z^{\prime })=$$

$$I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_W^{(M)}[x,y,z]D_{(K_z)}^{z,\ast }\left[z^{\prime }\right]J_z(t,x,y,z^{\prime }).$$

(125)

Equations (123)–(125) describe the change of mass in the region $W\subset {\mathbb{R}}_{0,+}^3$.

4.4. Mass Balance Equation

The sum of values $\Delta m_t$, $\Delta m_x$, $\Delta m_y$ and $\Delta m_z$ gives the equation

$$\Delta m_t+\Delta m_x+\Delta m_y+\Delta m_z=0$$

(126)

that describes the mass conservation. Using Equations (116), (123)–(125), the balance Equation (126) can be represented in the form

$$I_W^{(M)}[x,y,z]I_{[t_1,t_2]}^{(M_t)}\left[t\right](D_{(K_t)}^{t,\ast }\left[\tau \right]\rho (\tau ,x,y,z)+D_{(K_x)}^{x,\ast }\left[x^{\prime }\right]J_x(t,x^{\prime },y,z)+$$

$$D_{(K_y)}^{y,\ast }\left[y^{\prime }\right]J_y(t,x,y^{\prime },z)+D_{(K_z)}^{z,\ast }\left[z^{\prime }\right]J_z(t,x,y,z^{\prime }))=0.$$

(127)

As a result, the following theorem is proved.

Theorem 9

(Mass balance in GF integral form). Let the function $\rho (t,x_1,x_2,x_3)$ belong to the space $C_{-1}^{\{1,0\}}({\mathbb{R}}_{+}^4)$ and the functions $J_n(t,x_1,x_2,x_3)$ belong to the space $C_{-1}^{\{0,1\}}({\mathbb{R}}_{+}^4)$ for all $n=1,2,3$. Then, GF integral balance equation of mass of general nonlocal continuum in the parallelepiped region $W\subset {\mathbb{R}}_{0,+}^3$ has the form

$$I_W^{(M)}[x_1,x_2,x_3]I_{[t_1,t_2]}^{(M_t)}\left[t\right]\left(D_{(K_t)}^{t,\ast }\left[\tau \right]\rho +\sum _{n=1}^3{D}_{(K_n)}^{x_n,\ast }\left[{x^{\prime }}_n\right]J_n\right)=0,$$

(128)

where $x_1=x$, $x_2=y$, $x_3=z$.

Equations (127) and (128) must be satisfied for any finite time intervals $\Delta t=t_2-t_1$, where $t_2>t_1\ge 0$ and any finite sizes of the parallelepiped, $\Delta x=b-a$, $\Delta y=d-c$, $\Delta x=f-e$, where $b>a\ge 0$, $d>c\ge 0$, $f>e\ge 0$. This fact can be used to obtain the balance equation for general nonlocal continuum in a differential form.

To derive balance equation of general nonlocal continuum in the fractional differential form, it is possible to use the fractional analogue of the Titchmarsh Theorem (see Theorem 6). This theorem and the first fundamental theorems of GFC are used to derive GF differential form of general balance equations from the GF integral form of general balance equations. As a result, the balance equation is obtained in the form

$$D_{(K_t)}^{t,\ast }\left[\tau \right]\rho (\tau ,x,y,z)+D_{(K_x)}^{x,\ast }\left[x^{\prime }\right]J_x(t,x^{\prime },y,z)+$$

$$D_{(K_y)}^{y,\ast }\left[y^{\prime }\right]J_y(t,x,y^{\prime },z)+D_{(K_z)}^{z,\ast }\left[z^{\prime }\right]J_z(t,x,y,z^{\prime })=0,$$

(129)

which can be called the general nonlocal continuity equation. Equation (129) is the desired equation that describes the balance of mass in general non-local media.

As a result, the following theorem is proved for a differential form of the mass balance in general nonlocal continuum.

Theorem 10

(Mass balance in GF differential form). Let the function $\rho =\rho (t,x_1,x_2,x_3)$ and $J_n=J_n(t,x_1,x_2,x_3)$$(n=1,2,3)$ satisfy the conditions

$$D_{(K_t)}^{t,\ast }\left[\tau \right]\rho \in C_{-1,(K)}({\mathbb{R}}_{+}^4),$$

(130)

$$D_{(K_k)}^{x_k,\ast }\left[{x^{\prime }}_n\right]J_n\in C_{-1,(K)}({\mathbb{R}}_{+}^4)$$

(131)

for all $n=1,2,3$. Then, the GF differential balance equation of mass of general nonlocal continuum in the parallelepiped region $W\subset {\mathbb{R}}_{0,+}^3$ has the form

$$D_{(K_t)}^{t,\ast }\left[\tau \right]\rho +\sum _{n=1}^3{D}_{(K_n)}^{x_n,\ast }\left[{x^{\prime }}_n\right]J_n=0.$$

(132)

Remark 5.

It should be emphasized that for the non-local media the differential balance equation depends on the region W and in fact is an integro-differential equation.

Equation (132) is a mathematical expression of the existence of continuum flow without the formation of discontinuity surfaces. The proposed continuity equation is valid for any nonlocal continuous medium, viscous and compressible, with steady and unsteady motions.

Equation (132) is the basic equation of the mechanics of nonlocal medium, the nonlocality of which can be described by kernels belonging to the Luchko set.

4.5. General FVC Form of Mass Balance Equation

For general nonlocal media, the continuity equation, which is derived for parallelepiped region W, can be generalized to a wide class of domains and surfaces. This generalization can be realized by using the theorems of the general fractional vector calculus (General FVC).

Using the general FVC, Equation (129) can be written in the vector form

$$D_{(K_t)}^{t,\ast }\left[\tau \right]\rho (\tau ,x,y,z)+{Div}_W^{(K)}\mathbf{J}(t,x,y,z)=0,$$

(133)

where ${Div}_W^{(K)}$ is the general fractional divergence, which is defined by Equation (57) and

$$\mathbf{J}(t,x,y,z)={\mathbf{e}}_x{J}_x(t,x,y,z)+{\mathbf{e}}_y{J}_y(t,x,y,z)+{\mathbf{e}}_z{J}_z(t,x,y,z),$$

(134)

$$\mathbf{J}(t,x,y,z)=\rho (t,x,y,z)\mathbf{V}(t,x,y,z).$$

(135)

Using (135), Equation (133) can be represented in the form

$$D_{(K_t)}^{t,\ast }\left[\tau \right]\rho +{Div}_W^{(K)}\rho \mathbf{V}=0.$$

(136)

Equations (133) and (136) is the fractional differential equation of continuity that represents conservation law of mass for the general nonlocal continuum. Equation (133) is valid for fractional nonlocal continuum in steady and unsteady motion, and it is the fundamental equation of general nonlocal continuum mechanics for the wide class of the Sonin kernel pairs from the Luchko set.

Remark 6.

For the general fractional divergence the standard properties of divergence is violated. For example, the well-known equality

$$div(\rho \mathbf{V})=\rho div\mathbf{V}+(grad\rho ,\mathbf{V})$$

(137)

is violated for the operator ${Div}_W^{(K)}$ in the general case. For the general fractional vector calculus [86], there is the inequality

$${Div}_W^{(K)}\left(\rho (t,\mathbf{r})\mathbf{V}(t,\mathbf{r})\right)\ne \rho (t,\mathbf{r}){Div}_W^{(K)}\mathbf{V}(t,\mathbf{r})+\left(\mathbf{V}(t,\mathbf{r}),{Grad}_W^{(K)}\rho (t,\mathbf{r})\right),$$

(138)

where $\mathbf{r}=x{\mathbf{e}}_x+y{\mathbf{e}}_y+z{\mathbf{e}}_z$. Inequality (138) is a consequence of the violation of the standard product rule (the Leibniz rule) for fractional derivatives of non-integer orders and all the types of the general fractional derivatives.

As a result, some equivalent forms of representations of the standard balance equations are not equivalent in the nonlocal case.

5. General Fractional Equation for Momentum

Let us consider motions of general nonlocal medium, assuming that the velocity, density, pressure and, mass forces are continuous functions of time and position. The Euler approach for description of continuum is used. Balance equation for momentum of general nonlocal continuum is a mathematical formulation of the conservation law applied to the fixed region of the nonlocal continuum. In the Cartesian coordinate system $OXYZ$, an element of continuum is selected in the form of the parallelepiped

$$W=\{(x,y,z):0\le a\le x\le b,0\le c\le y\le d,0\le e\le z\le f\}$$

(139)

with sizes $\Delta x$, $\Delta y$, $\Delta z$ (see Figure 1). Let $\rho =\rho (t,x,y,z)$ be the density and $\mathbf{V}=\mathbf{V}(t,x,y,z)$ be the velocity at the point $S(x,y,z)\in {\mathbb{R}}_{+,0}^3$. Then, the momentum of continuum particles in the given fixed parallelepiped area W, at a time t, is desccribed by the equation

$$\mathbf{P}(t)=I_W^{(M)}[x,y,z]\mathbf{J}(t,x,y,z)=I_W^{(M)}[x,y,z]\rho (t,x,y,z)\mathbf{V}(t,x,y,z).$$

(140)

The changes of the momentum $\mathbf{P}(t)$ are caused by changes of the density and the velocity of the particles that came to the region W, at the time $t_1$ and $t_2$, such that

$$\mathbf{P}(t_1)=I_W^{(M)}[x,y,z]\rho (t_1,x,y,z)\mathbf{V}(t_1,x,y,z),$$

(141)

$$\mathbf{P}(t_2)=I_W^{(M)}[x,y,z]\rho (t_2,x,y,z)\mathbf{V}(t_2,x,y,z).$$

(142)

In the given fixed parallelepiped area W, the change of momentum during the time $\Delta t=t_2-t_1$ is written as

$$\Delta {\mathbf{P}}_t=\mathbf{P}(t_2)-\mathbf{P}(t_1)=I_W^{(M)}[x,y,z]\left(\mathbf{J}(t_2,x,y,z)-\mathbf{J}(t_1,x,y,z)\right).$$

(143)

The fundamental theorem of the GFC in the form

$$I_{[t_1,t_2]}^{(M_t)}\left[t\right]D_{(K_t)}^{t,\ast }\left[t^{\prime }\right]\left(\mathbf{J}(t^{\prime },x,y,z)\right)=\mathbf{J}(t_2,x,y,z)-\mathbf{J}(t_1,x,y,z)$$

(144)

is used to obtain the equation

$$\Delta {\mathbf{P}}_t=I_W^{(M)}[x,y,z]I_{[t_1,t_2]}^{(M_t)}\left[t\right]D_{(K_t)}^{t,\ast }\left[t^{\prime }\right]\left(\rho (t^{\prime },x,y,z)\mathbf{V}(t^{\prime },x,y,z)\right).$$

(145)

The momentum in the parallelepiped region W is varied by the following:

(a)

the transfer of momentum across the boundary;

(b)

the momentum of surface forces;

(c)

the momentum of mass forces.

These changes are considered in the following subsections.

5.1. Momentum Transfer across Boundaries

Let us consider the change of momentum due to its transport across the boundary. The momentum transferred through the face $W_a$ during the time $\Delta t$ in the positive direction of the axis $OX$ is

$${\mathbf{P}}_a=I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_{W_a}^{(M)}[y,z]\mathbf{J}(t,a,y,z)V_x(t,a,y,z)=$$

$$I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_{[c,d]}^{(M_y)}\left[y\right]I_{[e,f]}^{(M_z)}\left[z\right]\mathbf{J}(t,a,y,z)V_x(t,a,y,z).$$

(146)

The momentum transferred through the face $W_b$ during the time $\Delta t$ can be represented by

$${\mathbf{P}}_b=I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_{W_b}^{(M)}[y,z]\mathbf{J}(t,b,y,z)V_x(t,b,y,z)=$$

$$I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_{[c,d]}^{(M_y)}\left[y\right]I_{[e,f]}^{(M_z)}\left[z\right]\mathbf{J}(t,b,y,z)V_x(t,a,y,z).$$

(147)

The change of momentum by moving it across the faces, which is perpendicular to the $OX$-axis, is found if Equation (147) is subtracted from (146). Then, one can obtain the equation

$$\Delta {\mathbf{P}}_x={\mathbf{P}}_a-{\mathbf{P}}_b=$$

$$I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_{[c,d]}^{(M_y)}\left[y\right]I_{[e,f]}^{(M_z)}\left[z\right]\left(\mathbf{J}(t,a,y,z)V_x(t,a,y,z)-\mathbf{J}(t,b,y,z)V_x(t,b,y,z)\right).$$

(148)

Then, the fundamental theorem of the GFC in the form

$$I_{[a,b]}^{(M_x)}\left[x\right]D_{(K_x)}^{x,\ast }\left[x^{\prime }\right]\left(\mathbf{J}(t,x^{\prime },y,z)V_x(t,x^{\prime },y,z)\right)=$$

$$\mathbf{J}(t,b,y,z)V_x(t,b,y,z)-\mathbf{J}(t,a,y,z)V_x(t,a,y,z),$$

(149)

is used to obtain the equation

$$\Delta {\mathbf{P}}_x=-I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_{[a,b]}^{(M_x)}\left[x\right]I_{[c,d]}^{(M_y)}\left[y\right]I_{[e,f]}^{(M_z)}\left[z\right]D_{(K_x)}^{x,\ast }\left[x^{\prime }\right]\left(\mathbf{J}(t,x^{\prime },y,z)V_x(t,x^{\prime },y,z)\right)=$$

$$-I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_W^{(M)}[x,y,z]\left(D_{(K_x)}^{x,\ast }\left[x^{\prime }\right]\mathbf{J}(t,x^{\prime },y,z)V_x(t,x^{\prime },y,z)\right).$$

(150)

Similar arguments is found for the change of momentum in the region W, when moving nonlocal continuum through a pair of faces perpendicular to the axes of $OY$ and $OZ$, in the form

$$\Delta {\mathbf{P}}_y=-I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_W^{(M)}[x,y,z]\left(D_{(K_y)}^{y,\ast }\left[y^{\prime }\right]\mathbf{J}(t,x,y^{\prime },z)V_y(t,x,y^{\prime },z)\right),$$

(151)

$$\Delta {\mathbf{P}}_z=-I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_W^{(M)}[x,y,z]\left(D_{(K_z)}^{z,\ast }\left[z^{\prime }\right]\mathbf{J}(t,x,y,z^{\prime })V_z(t,x,y,z^{\prime })\right).$$

(152)

Summing relations (150)–(152), one can obtain the change of momentum in the parallelepiped region W due to its transport through the boundary

$$\Delta {\mathbf{P}}_{\mathbf{r}}=\sum _{k=1}^3\Delta {\mathbf{P}}_k=-I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_W^{(M)}[x_1,x_2,x_3]\left(\sum _{k=1}^3{D}_{(K_k)}^{x_k,\ast }\left[{x^{\prime }}_k\right]\left(\mathbf{J}{V}_k\right)\right),$$

(153)

where $x_1=x$, $x_2=y$, $x_3=z$.

5.2. The Momentum of the Mass Force

In classical mechanics of point particles without nonlocality in time (memoryless), the momentum of the force is described as

$$\Delta {\mathbf{P}}_c={\int }_{t_1}^{t_2}\mathbf{F}(t)dt.$$

(154)

For the case of nonlocality in time, the momentum of the force in given by the equation

$$\Delta {\mathbf{P}}_c=I_{[t_1,t_2]}^{(M_t)}\left[t\right]\mathbf{F}(t).$$

(155)

Let us consider the momentum of the mass force for non-local continuum. The density of the mass (volume) force is denoted as $\mathbf{F}=\mathbf{F}(t,x,y,z)$. Then, the momentum of the mass forces is the following

$$\Delta {\mathbf{P}}_F=I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_W^{(M)}[x,y,z]\rho (t,x,y,z)\mathbf{F}(t,x,y,z),$$

(156)

where it is assumed that the condition

$$\rho (t,x,y,z)\mathbf{F}(t,x,y,z)\in C_{-1}({\mathbb{R}}_{0,+}^4)$$

(157)

holds for all $(t,x,y,z)\in {\mathbb{R}}_{0,+}^4$.

5.3. The Momentum of the Surface Force

In this subsection, the momenta of surface forces are described. Let us consider the stress ${\mathbf{p}}_x$ for the face with the normal that is parallel to the $OX$-axis and positive direction of the face. On the opposite face the stress is ${\mathbf{p}}_{-x}$. Additionally, ${\mathbf{p}}_x=-{\mathbf{p}}_{-x}$. The momentum of the force on the face $W_a$ is

$$\Delta {\mathbf{P}}_{\sigma ,a}=I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_{W_a}^{(M)}[y,z]{\mathbf{p}}_{-x}(t,a,y,z)=-I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_{W_a}^{(M)}[y,z]{\mathbf{p}}_x(t,a,y,z).$$

(158)

On the opposite face $W_b$, the momentum of the force is

$$\Delta {\mathbf{P}}_{\sigma ,b}=I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_{W_b}^{(M)}[y,z]{\mathbf{p}}_{-x}(t,b,y,z)=-I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_{W_b}^{(M)}[y,z]{\mathbf{p}}_x(t,b,y,z).$$

(159)

The total momentum for the faces perpendicular to the $OX$-axis, is the sum of Equations (158) and (159) in the form:

$$\Delta {\mathbf{P}}_{\sigma ,x}=\Delta {\mathbf{P}}_{\sigma ,b}-{\mathbf{P}}_{\sigma ,a}=$$

$$-I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_{W_x}^{(M)}[y,z]\left({\mathbf{p}}_x(t,a,y,z)-{\mathbf{p}}_x(t,b,y,z)\right),$$

(160)

where one can take into account the property ${\mathbf{p}}_x=-{\mathbf{p}}_{-x}$ and $W_x=W_a\bigcup W_b$.

The fundamental theorem of the GFC in the form

$$I_{[a,b]}^{(M_x)}\left[x\right]D_{(K_x)}^{x,\ast }\left[x^{\prime }\right]{\mathbf{p}}_x(t,x^{\prime },y,z)={\mathbf{p}}_x(t,b,y,z)-{\mathbf{p}}_x(t,a,y,z),$$

(161)

is used to obtain the equation

$$\Delta {\mathbf{P}}_{\sigma ,x}=I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_{W_x}^{(M)}[y,z]I_{[a,b]}^{(M_x)}\left[x\right]D_{(K_x)}^{x,\ast }\left[x^{\prime }\right]{\mathbf{p}}_x(t,x^{\prime },y,z)=$$

$$I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_W^{(M)}[x,y,z]D_{(K_x)}^{x,\ast }\left[x^{\prime }\right]{\mathbf{p}}_x(t,x^{\prime },y,z).$$

(162)

Similarly, one can obtain the momenta of the surface forces acting on the faces that are perpendicular to the $OX$, $OY$ and $OZ$ axes in the form

$$\Delta {\mathbf{P}}_{\sigma ,k}=I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_W^{(M)}[x_1,x_2,x_3]D_{(K_k)}^{x_k,\ast }\left[{x_k}^{\prime }\right]{\mathbf{p}}_k$$

(163)

for $k=1,2,3$ and $x_1=x$, $x_2=y$, $x_3=z$.

Summing Equations (163), the momentum of the surface forces is described by the equation

$$\Delta {\mathcal{P}}_{\sigma }=\sum _{k=1}^3\Delta {\mathbf{P}}_{\sigma ,k}=\sum _{k=1}^3{I}_{[t_1,t_2]}^{(M_t)}\left[t\right]I_W^{(M)}[x_1,x_2,x_3]D_{(K_k)}^{x_k,\ast }\left[{x_k}^{\prime }\right]{\mathbf{p}}_k,$$

(164)

where ${\mathbf{p}}_k$ can be represented through the stress tensor ${\sigma }_{kl}$.

5.4. General Fractional Momentum Equation

The balance equation of momentum has the form

$$\Delta {\mathbf{P}}_t=\Delta {\mathbf{P}}_{\mathbf{r}}+\Delta {\mathbf{P}}_F+\Delta {\mathcal{P}}_{\sigma }.$$

(165)

Summing expressions (153), (156) and (164), equating the resulting sum to relation (145), one can obtain

$$I_W^{(M)}[x,y,z]I_{[t_1,t_2]}^{(M_t)}\left[t\right]D_{(K_t)}^{t,\ast }\left[t^{\prime }\right]\left(\rho (t^{\prime },x_1,x_2,x_3)\mathbf{V}(t^{\prime },x_1,x_2,x_3)\right)=$$

$$I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_W^{(M)}[x_1,x_2,x_3]\left(\sum _{k=1}^3{D}_{(K_k)}^{x_k,\ast }\left[{x^{\prime }}_k\right]\left(\mathbf{J}{V}_k\right)\right)+$$

$$I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_W^{(M)}[x_1,x_2,x_3]\rho (t,x_1,x_2,x_3)\mathbf{F}(t,x_1,x_2,x_3)+\sum _{k=1}^3{I}_{[t_1,t_2]}^{(M_t)}\left[t\right]I_W^{(M)}[x_1,x_2,x_3]D_{(K_k)}^{x_k,\ast }\left[{x_k}^{\prime }\right]{\mathbf{p}}_k.$$

(166)

Equation (166) describes transfer of momentum in terms of stresses for fractional nonlocal continuum.

Equation (166) can be written in the compact form by using $x_1=x$, $x_2=y$, $x_3=z$. As a result, the following theorem can be formulated.

Theorem 11

(Momentum balance in GI integral form). Let the conditions

$$(\rho \mathbf{V})(t,x_1,x_2,x_3)\in C_{-1}^{\{1,0\}}({\mathbb{R}}_{+}^4),$$

(167)

$$(\rho \mathbf{F})(t,x_1,x_2,x_3)\in C_{-1}^{\{0,0\}}({\mathbb{R}}_{+}^4),$$

(168)

$$\left(\mathbf{J}{V}_n\right)(t,x_1,x_2,x_3)\in C_{-1}^{\{0,1\}}({\mathbb{R}}_{+}^4){\mathbf{p}}_n(t,x_1,x_2,x_3)\in C_{-1}^{\{0,1\}}({\mathbb{R}}_{+}^4)$$

(169)

are satisfied for all $n=1,2,3$.

Then, the GF integral balance equation of momentum of general nonlocal continuum in the parallelepiped region $W\subset {\mathbb{R}}_{0,+}^3$ has the form

$$I_W^{(M)}[x_1,x_2,x_3]I_{[t_1,t_2]}^{(M_t)}\left[t\right]D_{(K_t)}^{t,\ast }\left[t^{\prime }\right]\left(\rho \mathbf{V}\right)=$$

$$I_W^{(M)}[x_1,x_2,x_3]I_{[t_1,t_2]}^{(M_t)}\left[t\right]\left(\sum _{n=1}^3{D}_{(K_n)}^{x_n,\ast }\left[{x^{\prime }}_n\right]\left(\mathbf{J}{V}_n\right)+\rho \mathbf{F}+\sum _{n=1}^3{D}_{(K_n)}^{x_n,\ast }\left[{x_n}^{\prime }\right]{\mathbf{p}}_n\right).$$

(170)

Equation (166) should be satisfied for all the parallelepiped regions $W\subset {\mathbb{R}}_{0,+}^3$ and all time intervals $[t_1,t_2]\subset {\mathbb{R}}_{0,+}$. To derive balance equation for momentum of general nonlocal continuum in the GF differential form, the fractional analogue of the Titchmarsh Theorem (see Theorem 6) and the first fundamental theorems of GFC should be used. As a result, the general balance equation for momentum in integral form (166) gives the differential form

$$D_{(K_t)}^{t,\ast }\left[t^{\prime }\right]\left(\rho (t^{\prime },x_1,x_2,x_3)\mathbf{V}(t^{\prime },x_1,x_2,x_3)\right)+\sum _{k=1}^3{D}_{(K_k)}^{x_k,\ast }\left[{x^{\prime }}_k\right]\left(\mathbf{J}{V}_k\right)=$$

$$\rho (t,x_1,x_2,x_3)\mathbf{F}(t,x_1,x_2,x_3)+\sum _{k=1}^3{D}_{(K_k)}^{x_k,\ast }\left[{x_k}^{\prime }\right]{\mathbf{p}}_k.$$

(171)

Using

$$\mathbf{V}=\sum _{l=1}^3{V}_l{\mathbf{e}}_l,\mathbf{F}=\sum _{l=1}^3{F}_l{\mathbf{e}}_l,{\mathbf{p}}_k=\sum _{l=1}^3{\sigma }_{kl}{\mathbf{e}}_l,$$

(172)

where ${\sigma }_{kl}$ is the stress, Equation (171) can be written in the component form

$$D_{(K_t)}^{t,\ast }\left[t^{\prime }\right]\left(\rho (t^{\prime },x_1,x_2,x_3)V_l(t^{\prime },x_1,x_2,x_3)\right)+\sum _{k=1}^3{D}_{(K_k)}^{x_k,\ast }\left[{x^{\prime }}_k\right]\left(\rho V_l{V}_k\right)=$$

(173)

$$\rho (t,x_1,x_2,x_3)F_l(t,x_1,x_2,x_3)+\sum _{k=1}^3{D}_{(K_k)}^{x_k,\ast }\left[{x_k}^{\prime }\right]{\sigma }_{kl}$$

(174)

for all $t>0$ and $x_k>0$. Equation (174) can be written in the compact form.

As a result, the following theorem for momentum balance in general nonlocal continuum was proved.

Theorem 12

(Momentum balance in GF differential form). Let the following conditions be satisfied

$$D_{(K_t)}^{t,\ast }\left(\rho V_n\right)\in C_{-1,(K)}({\mathbb{R}}_{+}^4),$$

(175)

$$D_{(K_n)}^{x_n,\ast }\left(\rho V_l{V}_n\right)\in C_{-1,(K)}({\mathbb{R}}_{+}^4),$$

(176)

$$(\rho F_l)\in C_{-1,(K)}({\mathbb{R}}_{+}^4),D_{(K_k)}^{x_k,\ast }\left[{x_k}^{\prime }\right]{\sigma }_{kl}\in C_{-1,(K)}({\mathbb{R}}_{+}^4)$$

(177)

for all $n,l=1,2,3$. Then, the GF differential balance equation of momentum of general nonlocal continuum in the parallelepiped region $W\subset {\mathbb{R}}_{0,+}^3$ has the form

$$D_{(K_t)}^{t,\ast }\left(\rho V_l\right)+\sum _{k=1}^3{D}_{(K_k)}^{x_k,\ast }\left(\rho V_l{V}_k\right)=\rho F_l+\sum _{k=1}^3{D}_{(K_k)}^{x_k,\ast }\left[{x_k}^{\prime }\right]{\sigma }_{kl}.$$

(178)

For the non-local media the differential balance Equation (178) depend on the region W, since these equations are integro-differential equations. Equations (174) and (178) is the desired equation describing the balance of momentum in general non-local media.

5.5. GF Divergence of a Dyadic Product

At the beginning, let us consider the standard local case.

The nabla operator

$$\nabla ={\mathbf{e}}_k{\partial }_k=\sum _{k=1}^3{\mathbf{e}}_k{\partial }_k,$$

(179)

and the dyadic product of the vectors

$$\mathbf{A}\otimes \mathbf{B}=A_i{B}_j{\mathbf{e}}_i\otimes {\mathbf{e}}_j=\sum _{i=1}^3\sum _{j=1}^3{A}_i{B}_j{\mathbf{e}}_i\otimes {\mathbf{e}}_j$$

(180)

can be used to get

$$\left(\nabla ,\mathbf{A}\otimes \mathbf{B}\right)=\left({\mathbf{e}}_k{\partial }_k,(A_i{B}_j){\mathbf{e}}_i\otimes {\mathbf{e}}_j\right)=$$

(181)

$${\partial }_k(A_i{B}_j)\left({\mathbf{e}}_k,{\mathbf{e}}_i\otimes {\mathbf{e}}_j\right),$$

(182)

where the brackets $\left(,\right)$ denotes the scalar product and the basis vectors ${\mathbf{e}}_k$ are fixed.

Then, using the equality

$$\left({\mathbf{e}}_k,{\mathbf{e}}_i\otimes {\mathbf{e}}_j\right)=\left({\mathbf{e}}_k,{\mathbf{e}}_i\right)\otimes {\mathbf{e}}_j={\delta }_{ki}{\mathbf{e}}_j,$$

(183)

and the chain (Leibniz) rule

$${\partial }_k(A_i{B}_j)=({\partial }_k{A}_i)B_j+A_i({\partial }_k{B}_j),$$

(184)

one can write

$$\left(\nabla ,\mathbf{A}\otimes \mathbf{B}\right)={\delta }_{ki}{\mathbf{e}}_j\left(({\partial }_k{A}_i)B_j+A_i({\partial }_k{B}_j)\right)=$$

(185)

$$\left(({\partial }_k{A}_k)B_j+A_k({\partial }_k{B}_j)\right){\mathbf{e}}_j.$$

(186)

Therefore,

$$\left(\nabla ,\mathbf{A}\otimes \mathbf{B}\right)=\left(({\partial }_k{A}_k)B_j+A_k({\partial }_k{B}_j)\right){\mathbf{e}}_j.$$

(187)

The vector notation for local case can be used to get the equality

$$\left(\nabla ,\mathbf{A}\otimes \mathbf{B}\right)=\left(\nabla ,\mathbf{A}\right)\mathbf{B}+\left(\mathbf{A},\nabla \right)\mathbf{B}$$

(188)

that can be interpreted as a chain rule for the standard nable operator. For general fractional derivatives, the standard product (Leibniz) rule is violated. Therefore, for nonlocal case the following inequality has the form

$$\left({\nabla }_W^{(K)},\mathbf{A}\otimes \mathbf{B}\right)\ne \left({\nabla }_W^{(K)},\mathbf{A}\right)\mathbf{B}+\left(\mathbf{A},{\nabla }_W^{(K)}\right)\mathbf{B}.$$

(189)

Equations (181) and (183) gives

$$\left(\nabla ,\mathbf{A}\otimes \mathbf{B}\right)=\sum _{j=1}^3\sum _{i=1}^3{\partial }_i(A_i{B}_j){\mathbf{e}}_j.$$

(190)

The general fractional analog of Equation (190) has the form

$$\left({\nabla }_W^{(K)},\mathbf{A}\otimes \mathbf{B}\right)=\sum _{i=1}^3{D}_{(K_i)}^{x_i,\ast }(\mathbf{A}\otimes \mathbf{B})=\sum _{j=1}^3\sum _{i=1}^3{D}_{(K_i)}^{x_i,\ast }(A_i{B}_j){\mathbf{e}}_j.$$

(191)

Let us consider the nonlocal case and general fractional differential vector operators.

The general fractional divergence of dyad product can be defined by equation

$${Div}_W^{(K)}\left(\mathbf{A}\otimes \mathbf{B}\right)=\sum _{j=1}^3\sum _{k=1}^3{\mathbf{e}}_j{D}_{(K_k)}^{x_k,\ast }\left[{x_k}^{\prime }\right]\left(A_k{B}_j\right),$$

(192)

where a fixed frame of reference and a fixed Cartesian coordinate system are used.

It should be noted that the general fractional divergence does not satisfy the standard properties of the divergence, such as

$$div\left(\mathbf{A}\otimes \mathbf{B}\right)=\left(div\mathbf{A}\right)\mathbf{B}+\left(\mathbf{A},\nabla \right)\mathbf{B}.$$

(193)

In the general case, the following inequality shoud be taken into account

$${Div}_W^{(K)}\left(\mathbf{A}\otimes \mathbf{B}\right)\ne \left({Div}_W^{(K)}\mathbf{A}\right)\mathbf{B}+\left(\mathbf{A},{\nabla }_W^{(K)}\right)\mathbf{B}$$

(194)

for general fractional divergence. Inequality (194) is a consequence of the violation of the standard product rule (the Leibniz rule) for fractional derivatives of non-integer orders and general fractional derivatives of all the types.

5.6. General FVC Form of Momentum Balance Equation

The continuity equation of thegeneral nonlocal media, which is derived by using parallelepiped region W, can be generalized to a wide class of domains and surfaces by using the theorems of the general fractional vector calculus. Equation (174) contains general fractional derivatives $D_{(K_t)}^{t,\ast }\left[t^{\prime }\right]$ and $D_{(K_k)}^{x_k,\ast }\left[{x^{\prime }}_k\right]$ that are defined for $t\in [0,\infty )$ and and $x_k\in [0,\infty )$ for $k=1,2,3$.

Using the general fractional vector calculus, Equation (174) can be written in the vector form

$$D_{(K_t)}^{t,\ast }\left[t^{\prime }\right]\left(\rho (t^{\prime },x,y,z)\mathbf{V}(t^{\prime },x,y,z)\right)+{Div}_W^{(K)}\left(\rho \mathbf{V}\otimes \mathbf{V}\right)=$$

$$\rho (t,x,y,z)\mathbf{F}(t,x,y,z)+{Div}_W^{(K)}{\mathcal{P}}_{\sigma }$$

(195)

where ${\mathcal{P}}_{\sigma }$ is the stress tensor

$${\mathcal{P}}_{\sigma }=\sum _{k=1}^3{\mathbf{p}}_k{\mathbf{e}}_k=\sum _{k=1}^3\sum _{l=1}^3{\sigma }_{kl}{\mathbf{e}}_k\otimes {\mathbf{e}}_l.$$

(196)

In a more compact form, Equation (195) can be written as

$$D_{(K_t)}^{t,\ast }\left(\rho \mathbf{V}\right)={Div}_W^{(K)}\left({\mathcal{P}}_{\sigma }-\rho \mathbf{V}\otimes \mathbf{V}\right)+\rho \mathbf{F}.$$

(197)

For the local case, Equations (197) give the standard equations with the partial derivative $\frac{\partial }{\partial t}$ and divergence ($\mathit{div}$) instead of the general fractional operators $D_{(K_t)}^{t,\ast }$ and ${Div}_W^{(K)}$.

Remark 7.

For the kernels $K_t(\tau )=h_{1-\alpha }(\tau )$ and $K_j(x_j)=h_{1-{\alpha }_j}(x_j)$, Equation (174) gives

$${}_0^C{D}_t^{\alpha }(\rho V_l)+\sum _{k=1}^3{}_0^C{D}_{x_k}^{{\alpha }_k}(\rho V_l{V}_k)=\rho F_l+\sum _{k=1}^3{}_0^C{D}_{x_k}^{{\alpha }_k}{\sigma }_{kl},$$

(198)

where $l=1,2,3$.

5.7. General Fractional Equilibrium Equations for Stresses

In this subsection, the general fractional equilibrium equations for the stress tensor of general nonlocal continuum is derived. For simplification, one can consider a two-dimensional elastic isotropic non-local continuum. Let us consider a rectangular region $W_2\subset {\mathbb{R}}_{\mathrm{⊬},+}^{\mathrm{⊭}}$ (see Figure 2) that is given as

$$W_2:=\{(x,y):0\le a\le x\le b,0\le c\le y\le d\}.$$

(199)

In the Figure 2, the following notations are used

$${\sigma }_{xx}^{+}={\sigma }_{xx}(b,c),{\sigma }_{xx}={\sigma }_{xx}(x,y),$$

(200)

$${\sigma }_{yy}^{+}={\sigma }_{yy}(a,d),{\sigma }_{yy}={\sigma }_{yy}(x,y),$$

(201)

and

$${\sigma }_{xy}^{x+}={\sigma }_{xy}(b,c),{\sigma }_{xy}={\sigma }_{xy}(x,y),$$

(202)

$${\sigma }_{xy}^{y+}={\sigma }_{xy}(a,d),{\sigma }_{xy}={\sigma }_{xy}(x,y).$$

(203)

The force equilibrium condition gives the equation

$$I_{[c,d]}^{(M_y)}\left[y\right]\left({\sigma }_{xx}(b,y)-{\sigma }_{xx}(a,y)\right)+I_{[a,b]}^{(M_x)}\left[x\right]\left({\sigma }_{xy}(x,d)-{\sigma }_{xy}(x,c)\right)+$$

$$I_{[a,b]}^{(M_x)}\left[x\right]I_{[c,d]}^{(M_y)}\left[y\right]\left(\rho (x,y)F_x(x,y)\right)=0.$$

(204)

For direction, that is parallel to the $OX$-axis, the fundamental theorem of GFC for the stress can be used in the form

$${\sigma }_{xx}(b,y)-{\sigma }_{xx}(a,y)=I_{[a,b]}^{(M_x)}\left[x\right]D_{(K_x)}^{x,\ast }\left[x^{\prime }\right]{\sigma }_{xx}(x^{\prime },y)$$

$${\sigma }_{xy}(x,d)-{\sigma }_{xy}(x,c)=I_{[c,d]}^{(M_y)}\left[y\right]D_{(K_y)}^{y,\ast }\left[y^{\prime }\right]{\sigma }_{xy}(x,y^{\prime }).$$

(205)

Then, the equation

$$I_{[a,b]}^{(M_x)}\left[x\right]I_{[c,d]}^{(M_y)}\left[y\right]D_{(K_x)}^{x,\ast }\left[x^{\prime }\right]{\sigma }_{xx}(x^{\prime },y)+I_{[a,b]}^{(M_x)}\left[x\right]I_{[c,d]}^{(M_y)}\left[y\right]D_{(K_y)}^{y,\ast }\left[y^{\prime }\right]{\sigma }_{xy}(x,y^{\prime })+$$

$$I_{[a,b]}^{(M_x)}\left[x\right]I_{[c,d]}^{(M_y)}\left[y\right]\left(\rho (x,y)F_x(x,y)\right)=0,$$

(206)

can be written as

$$I_{W_2}^{(M)}[x,y]D_{(K_x)}^{x,\ast }\left[x^{\prime }\right]{\sigma }_{xx}(x^{\prime },y)+I_{W_2}^{(M)}[x,y]D_{(K_y)}^{y,\ast }\left[y^{\prime }\right]{\sigma }_{xy}(x,y^{\prime })+$$

(207)

$$I_{W_2}^{(M)}[x,y]\left(\rho (x,y)F_x(x,y)\right)=0.$$

(208)

The fact that Equation (208) must be satisfied for any rectangular regions $W_2\subset {\mathbb{R}}_{\mathrm{⊬},+}^{\mathrm{⊭}}$ and the general fractional analogue of Titchmarsh’s Theorem 6 leads to the general fractional differential equation

$$D_{(K_x)}^{x,\ast }\left[x^{\prime }\right]{\sigma }_{xx}(x^{\prime },y)+D_{(K_y)}^{x,\ast }\left[y^{\prime }\right]{\sigma }_{xx}(x,y^{\prime })+\rho (x,y)F_x(x,y)=0.$$

(209)

For the power-law non-locality $K_j(x_j)=h_{1-{\alpha }_j}(x_j)$, Equation (209) takes the form

$$({}_0^C{D}_x^{{\alpha }_x}{\sigma }_{xx})(x,y)+({}_0^C{D}_y^{{\alpha }_y}{\sigma }_{xy})(x,y)+\rho (x,y)F_x(x,y)=0.$$

(210)

For ${\alpha }_x={\alpha }_y=1$, Equation (210) takes the standard form

$$\frac{\partial {\sigma }_{xx}(x,y)}{\partial x}+\frac{\partial {\sigma }_{xy}(x,y)}{\partial y}+\rho (x,y)F_x(x,y)=0.$$

(211)

Similarly for the direction that is parallel to the $OY$-axis, the force equilibrium condition gives the equation

$$D_{(K_x)}^{x,\ast }\left[x^{\prime }\right]{\sigma }_{yx}(x^{\prime },y)+D_{(K_y)}^{y,\ast }\left[y^{\prime }\right]{\sigma }_{yy}(x,y^{\prime })+\rho (x,y)F_y(x,y)=0.$$

(212)

The two-dimensional case can be generalized to the three-dimensional case. As a result, the general fractional equilibrium equations take the form

$$\sum _{j=1}^3\left(D_{(K_j)}^{x_j,\ast }\left[{x^{\prime }}_j\right]{\sigma }_{jk}\right)(x,y,z)+\rho (x_1,x_2,x_3)F_k(x_1,x_2,x_3)=0,(k=1,2,3),$$

(213)

where $x_1=x$, $x_2=y$, $x_3=z$. Equation (213) are the general equilibrium equations for stress of the general nonlocal continuum.

Comparing Equations (195) and (213), it is possible to see that Equation (213) is a special case of Equation (195).

6. General Fractional Equation for Total Energy

The Euler approach for description of continuum is used to derive the energy balance equation. Equation for total energy of the general nonlocal continuum is a mathematical formulation of the conservation law for energy applied to the fixed region of the nonlocal continuum. This law states that the change of the total (kinetic and internal) energy $\Delta E_t$ in the fixed region of nonlocal continuum is described by equation

$$\Delta E_t=\Delta E_S+A_M+A_S+Q_M+Q_S,$$

(214)

where $\Delta E_S$ is the energy transport across the boundaries $S=\partial W$ of the region $W\subset {\mathbb{R}}_{0,+}^3$; $A_M$ is a work of mass forces (body forces) and $A_S$ is a work of surface forces and $Q_S$ is a heat supplied through the surface S. In this section, it will be assumed that the volumetric input amount of energy is equal to zero $Q_M=0$.

Let us consider a region $W\subset {\mathbb{R}}_{0,+}^3$ in the form of the elementary parallelepiped

$$W:=\{(x,y,z):0\le a\le x\le b,0\le c\le y\le d,0\le e\le z\le f\}$$

(215)

that is presented in Figure 1. It will be supposed that the velocity $\mathbf{V}(t,x,y,z)$, density $\rho (t,x,y,z)$, stress and mass forces are known functions at the point $S(x,y,z)\in W$, which are continuous functions of coordinates $(x,y,z)$ and time t. In general, it is possible for a wider class of functions or function space.

The value $V^2/2$ is the kinetic energy per unit mass and $e_i$ is the internal energy per unit mass of the medium in a given time $t\ge 0$. The function

$$\mathcal{E}(t,x,y,z)=e_i(t,x,y,z)+\frac{1}{2}{V}^2(t,x,y,z)$$

(216)

describes the total energy per unit mass of the medium at $t\ge 0$.

The value $(\rho V^2/2)(t,x,y,z)$ is the density of the kinetic energy of the nonlocal continuum in a given time; $(\rho e_i)(t,x,y,z)$ is the density of the internal energy of nonlocal continuum at $t\ge 0$. The sum of the densities of internal energy and the kinetic energy is called the density of total energy

$$E^{\prime }=\rho \mathcal{E}=\rho e_i+\frac{1}{2}(\rho V^2)/2,$$

(217)

where $\rho$, $e_i$ and $\mathbf{V}$ are functions of the time variable $t\ge 0$ and the space coordinates $(x,y,z)\in {\mathbb{R}}_{0,+}^3$.

The kinetic energy $E_{kin}(t)$ at time $t\ge 0$ is defined by equation

$$E_{kin}(t)=I_W^{(M)}[x,y,z]\left(\frac{1}{2}\rho V^2\right)(t,x,y,z).$$

(218)

The internal energy $E_{int}(t)$ at time $t\ge 0$ is defined by equation

$$E_{int}(t)=I_W^{(M)}[x,y,z]\left(\rho e_i\right)(t,x,y,z).$$

(219)

The total energy $E(t)$ of the fixed region W of the medium at time $t\ge 0$ is given by the equation

$$E(t)=I_W^{(M)}[x,y,z]\left(\rho e_i+\frac{\rho V^2}{2}\right)=I_W^{(M)}[x,y,z](\rho \mathcal{E})(t,x,y,z).$$

(220)

In the general case, the following condition can be considered

$$(\rho \mathcal{E})(t,x,y,z)\in C_{-1}^{\{1,0\}}({\mathbb{R}}_{+}^4)$$

(221)

for Equation (219).

For $t=t_1$ and $t=t_2$, the total energy is described as

$$E(t_1)=I_W^{(M)}[x,y,z](\rho \mathcal{E})(t_1,x,y,z).$$

(222)

$$E(t_2)=I_W^{(M)}[x,y,z](\rho \mathcal{E})(t_2,x,y,z).$$

(223)

Therefore, the change of the total energy in a time $\Delta t=t_2-t_1$ is written as

$$\Delta E_t=E(t_2)-E(t_1)=I_W^{(M)}[x,y,z]\left((\rho \mathcal{E})(t_1,x,y,z)-(\rho \mathcal{E})(t_1,x,y,z)\right).$$

(224)

Using the fundamental theorem of the GFC in the form

$$I_{[t_1,t_2]}^{(M_t)}\left[t\right]D_{(K_t)}^{t,\ast }\left[t^{\prime }\right](\rho \mathcal{E})(t^{\prime },x,y,z)=(\rho \mathcal{E})(t_2,x,y,z)-(\rho \mathcal{E})(t_1,x,y,z),$$

(225)

Equation (224) takes the form

$$\Delta E_t=E(t_2)-E(t_1)=I_W^{(M)}[x,y,z]I_{[t_1,t_2]}^{(M_t)}\left[t\right]D_{(K_t)}^{t,\ast }\left[t^{\prime }\right](\rho \mathcal{E})(t^{\prime },x,y,z).$$

(226)

Change of the total energy $\Delta E_t$ in the region W can be caused by the following processes:

(a)

the energy transport across the boundaries of the region $\Delta E_S$;

(b)

the work of the mass forces $\Delta A_F$;

(c)

the work of the surface forces $A_S$;

(d)

the heat flux through the boundary $Q_S$ (internal heat sources are not considered).

In the following sub-sections, expressions describing the contribution of these processes to the energy balance in the nonlocal medium will be obtained.

6.1. Change of Total Energy Due to the Transfer across Boundaries

Change of the total energy due to the transfer across boundaries of the selected fixed region W can be obtained analogously as was done above for calculating the change in momentum. The energy transfer through the boundary perpendicular to the $OX$-axis has the form

$$\Delta E_S=-I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_W^{(M)}[x,y,z]D_{(K_x)}^{x,\ast }\left[x^{\prime }\right](V_x\rho \mathcal{E})(t,x^{\prime },y,z).$$

(227)

Then, the sum of the terms, that take into account the mass transfer across all the boundaries of the fixed region W, has the form

$$\Delta E_S=-\sum _{j=1}^3{I}_{[t_1,t_2]}^{(M_t)}\left[t\right]I_W^{(M)}[x_1,x_2,x_3]D_{(K_j)}^{x_j,\ast }\left[{x^{\prime }}_j\right](V_j\rho \mathcal{E}),$$

(228)

where $(\rho \mathcal{E})(t,x_1,x_2,x_3)$ is the density of total energy that is given in (217).

6.2. Work of Mass Forces

In classical mechanics of point particle with nonlocality in time, which is characterized by the kernel $M(t)$, the work of the force $\mathbf{f}(t)$ is described by equation

$$\Delta A=I_{[t_1,t_2]}^{(M_t)}\left[t\right](\mathbf{f}(t),\mathbf{v}(t)),$$

(229)

where $\mathbf{v}(t)$ is the velocity of the point particle.

In the continuum mechanics, the work $\Delta A_F$ of the force for $\Delta t=t_2-t_1$ is described by the equation

$$\Delta A_F=I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_W^{(M)}[x,y,z]\rho (t,x,y,z)(\mathbf{F},\mathbf{V})(t,x,y,z),$$

(230)

where $\mathbf{F}$ is the force per unit mass fo the medium, $\rho \mathbf{F}$ is the density of force and

$$(\mathbf{F},\mathbf{V})=\sum _{k=1}^3{F}_k{V}_k$$

(231)

is the scalar product of the vector fields that can be interpreted as a power of the mass force per unit mass (the density of power).

6.3. Work of Surface Forces

Let us obtain an expression for the work of the surface forces. The vector ${\mathbf{p}}_x$ denotes the stress vector at the face $A{A}_1{D}_{1}D$,

$$W_a=\{(x,y,z):x=a\ge 0,y\in [c,d],z\in [e,f]\}.$$

(232)

Let us denote the stress vector on the face $W_a$ with the direction of the normal coinciding with the positive direction of the $OX$-axis as ${\mathbf{p}}_x$. For the opposite direction of the normal, it should be used ${\mathbf{p}}_{-x}$. Furthermore, ${\mathbf{p}}_x=-{\mathbf{p}}_{-x}$.

Let us find the total work $\Delta A_S$ of the surface forces acting on the faces of the parallelepiped region $W\subset {\mathbb{R}}_{0,+}^3$.

The work of the surface force acting on the area $W_a$ with stress ${\mathbf{p}}_{-x}$ is

$$\Delta A_a=I_{W_a}^{(M)}[y,z]I_{[t_1,t_2]}^{M_t}\left[t\right]({\mathbf{p}}_{-x},\mathbf{V})(t,a,y,z).$$

(233)

For the face $W_b$, the work of the surface force with stress ${\mathbf{p}}_x$ is

$$\Delta A_b=I_{W_b}^{(M)}[y,z]I_{[t_1,t_2]}^{M_t}\left[t\right]({\mathbf{p}}_x,\mathbf{V})(t,b,y,z).$$

(234)

The sum of the works of the surface forces acting on the faces $W_a$ and $W_b$ that are perpendicular to $OX$, can be written as

$$\Delta A_x=\Delta A_a+\Delta A_b=$$

$$I_{W_x}^{(M)}[y,z]I_{[t_1,t_2]}^{M_t}\left[t\right]\left(({\mathbf{p}}_x,\mathbf{V})(t,b,y,z)-({\mathbf{p}}_x,\mathbf{V})(t,a,y,z)\right),$$

(235)

where ${\mathbf{p}}_{-x}=-{\mathbf{p}}_x$ and $W_x=W_a\bigcup W_b$ are taken into account. The fundamental theorem of the GFC in the form

$$({\mathbf{p}}_x,\mathbf{V})(t,b,y,z)-({\mathbf{p}}_x,\mathbf{V})(t,a,y,z)=I_{[a,b]}^{(M_x)}\left[x\right]D_{(K_x)}^{x,\ast }\left[x^{\prime }\right]({\mathbf{p}}_x,\mathbf{V})(t,x^{\prime },y,z)$$

(236)

can be used to get

$$\Delta A_x=I_W^{(M)}[x,y,z]I_{[t_1,t_2]}^{M_t}\left[t\right]D_{(K_x)}^{x,\ast }\left[x^{\prime }\right]({\mathbf{p}}_x,\mathbf{V})(t,x^{\prime },y,z).$$

(237)

In Equation (237), the stress vector ${\mathbf{p}}_x$ can be represented in the form

$${\mathbf{p}}_x={\sigma }_{xx}\mathbf{i}+{\sigma }_{xy}\mathbf{j}+{\sigma }_{xz}\mathbf{k}.$$

(238)

Therefore, the scalar product can be written as

$$({\mathbf{p}}_x,\mathbf{V})={\sigma }_{xx}{V}_x+{\sigma }_{xy}{V}_y+{\sigma }_{xz}{V}_z,$$

(239)

and the work (237) is represented as the sum

$$\Delta A_x=A_{xx}+A_{xy}+A_{xz}.$$

(240)

This allows us to assert that the total work $\Delta A_S$, which is caused by the surface forces, is defined by the normal stresses ${\sigma }_{xx}$, ${\sigma }_{yy}$, ${\sigma }_{zz}$ and the tangential stresses ${\sigma }_{xy}$, ${\sigma }_{yz}$, ${\sigma }_{xz}$.

Considering the work of the forces acting on the faces $W_a$ and $W_b$ that are perpendicular to the $OX$-axis, one can obtain the work of the normal forces for the time interval $[t_1,t_2]$ in the form

$$\Delta A_{xx,a}=I_{[t_1,t_2]}^{M_t}\left[t\right]I_{W_a}^{(M)}[y,z]({\sigma }_{xx}{V}_x)(t,a,y,z),$$

(241)

$$\Delta A_{xx,b}=I_{[t_1,t_2]}^{M_t}\left[t\right]I_{W_b}^{(M)}[y,z]({\sigma }_{xx}{V}_x)(t,b,y,z).$$

(242)

Therefore,

$$\Delta A_{xx}=\Delta A_{xx,a}+\Delta A_{xx,b}=$$

$$I_{[t_1,t_2]}^{M_t}\left[t\right]I_{\partial W}^{(M)}[y,z]\left(({\sigma }_{xx}{V}_x)(t,b,y,z)-({\sigma }_{xx}{V}_x)(t,a,y,z)\right).$$

(243)

The funfamental theorem of the GFC is used to get

$$\Delta A_{xx}=I_W^{(M)}[x,y,z]D_{(K_x)}^{x,\ast }\left[x^{\prime }\right]\left(({\sigma }_{xx}{V}_x)(t,x^{\prime },y,z)\right).$$

(244)

where it is assumed that

$$({\sigma }_{xx}{V}_x)(t,x^{\prime },y,z)\in C_{-1}^1(0,\infty )\mathrm{for}\mathrm{all}t\ge 0,y\ge 0,z\ge 0.$$

(245)

It should be noted that the standard Leibniz rule for GF derivatives is not satisfied. Therefore, the inequality

$$D_{(K_x)}^{x,\ast }\left[x^{\prime }\right]({\sigma }_{xx}{V}_x)\ne V_x{D}_{(K_x)}^{x,\ast }\left[x^{\prime }\right]{\sigma }_{xx}+{\sigma }_{xx}{D}_{(K_x)}^{x,\ast }\left[x^{\prime }\right]V_x.$$

(246)

is satisfied in the general case.

The work of tangential forces on the faces $W_a$ and $W_b$, which are perpendicular to the $OX$-axis, is equal to

$$\Delta A_{xy}=I_{[t_1,t_2]}^{M_t}\left[t\right]I_W^{(M)}[x,y,z]D_{(K_y)}^{y,\ast }\left[y^{\prime }\right]\left(({\sigma }_{xy}{V}_y)(t,x,y^{\prime },z)\right),$$

(247)

$$\Delta A_{xz}=I_{[t_1,t_2]}^{M_t}\left[t\right]I_W^{(M)}[x,y,z]D_{(K_z)}^{z,\ast }\left[z^{\prime }\right]\left(({\sigma }_{xz}{V}_z)(t,x,y,z^{\prime })\right).$$

(248)

Thus, the total work $\Delta A_x$ of the surface forces acting on the faces that are perpendicular to $OX$, for the time interval $[t_1,t_2]$, is equal

$$\Delta A_x=\sum _{j=1}^3{I}_{[t_1,t_2]}^{M_t}\left[t\right]I_W^{(M)}[x_1,x_2,x_3]D_{(K_j)}^{x_j,\ast }\left[{x^{\prime }}_j\right]\left({\sigma }_{lj}{V}_j)\right).$$

(249)

where $x_1=x$, $x_2=y$ and $x_3=z$.

Similarly, one can obtain the works for the faces that are perpendicular to the $OY$-axis and $OZ$-axis. For all pairs of faces, the total work $\Delta A_j$ is described by the equation

$$\Delta A_j=I_{[t_1,t_2]}^{M_t}\left[t\right]I_W^{(M)}[x_1,x_2,x_3]D_{(K_j)}^{x_j,\ast }\left[{x^{\prime }}_j\right]({\mathbf{p}}_j,\mathbf{V}).$$

(250)

As a result, the total work, which is caused by the surface forces, is described in the form

$$\Delta A_S=\sum _{k=1}^3\Delta A_j=\sum _{j=1}^3{I}_{[t_1,t_2]}^{M_t}\left[t\right]I_W^{(M)}[x_1,x_2,x_3]D_{(K_j)}^{x_j,\ast }\left[{x^{\prime }}_j\right]({\mathbf{p}}_j,\mathbf{V})=$$

$$\sum _{j=1}^3\sum _{l=1}^3{I}_{[t_1,t_2]}^{M_t}\left[t\right]I_W^{(M)}[x_1,x_2,x_3]D_{(K_j)}^{x_j,\ast }\left[{x^{\prime }}_j\right]\left({\sigma }_{jl}{V}_l\right),$$

(251)

where

$$({\mathbf{p}}_j,\mathbf{V})=\sum _{l=1}^3{\sigma }_{jl}{V}_l.$$

(252)

6.4. Change of Total Energy by the Heat Flow

Let us find a change of the total energy due to the heat flow. We denote the specific vector of the density of heat flow by $\mathbf{q}(t,x,y,z)$. Then, the heat flow through the face $W_a$, which is perpendicular to the $OX$-axis, is

$$Q_a=I_{W_a}^{(M)}[y,z]I_{[t_1,t_2]}^{(M_t)}\left[t\right]q_x(t,a,y,z).$$

(253)

For the face $W_b$, the heat flow through the face is

$$Q_b=I_{W_b}^{(M)}[y,z]I_{[t_1,t_2]}^{(M_t)}\left[t\right]q_x(t,b,y,z).$$

(254)

The change of the total energy by the heat flux transported through the faces $W_x=W_a\bigcup W_b$ that are perpendicular to the $OX$-axis, is given by

$$\Delta Q_x=Q_a-Q_b=$$

$$I_{W_x}^{(M)}[y,z]I_{[t_1,t_2]}^{(M_t)}\left[t\right]\left(q_x(t,a,y,z)-q_x(t,b,y,z)\right).$$

(255)

Using the fundamental theorem of the GFC in the form

$$q_x(t,b,y,z)-q_x(t,a,y,z)=I_{[a,b]}^{(M_x)}\left[x\right]D_{(K_x)}^{x,\ast }\left[x^{\prime }\right]q_x(t,x^{\prime },y,z),$$

(256)

and

$$I_{W_x}^{(M)}[y,z]I_{[a,b]}^{(M_x)}\left[x\right]=I_W^{(M)}[x,y,z],$$

(257)

Equation (255) can be written as

$$\Delta Q_x=-I_W^{(M)}[x,y,z]I_{[t_1,t_2]}^{(M_t)}\left[t\right]D_{(K_x)}^{x,\ast }\left[x^{\prime }\right]q_x(t,x^{\prime },y,z).$$

(258)

For all pairs of faces, which are perpendicular to all the axes, the change of the total energy is described by the equations

$$\Delta Q_j=-I_W^{(M)}[x_1,x_2,x_3]I_{[t_1,t_2]}^{(M_t)}\left[t\right]D_{(K_j)}^{x_j,\ast }\left[{x^{\prime }}_j\right]q_j(j=1,2,3).$$

(259)

Summing Equation (259), the change of the total energy by the heat flow is obtained in the form

$$\Delta Q_S=\sum _{j=1}^3\Delta Q_j=-\sum _{j=1}^3{I}_W^{(M)}[x_1,x_2,x_3]I_{[t_1,t_2]}^{(M_t)}\left[t\right]D_{(K_j)}^{x_j,\ast }\left[{x^{\prime }}_j\right]q_j,$$

(260)

where $q_j=q_j(t,x_1,x_2,x_3)$$(j=1,2,3)$ are components of the density of heat flow.

6.5. Change of Total Energy of All Sources

The balance equation for the total energy has the form

$$\Delta E_t=\Delta E_S+\Delta A_M+\Delta A_S+\Delta Q_S.$$

(261)

Summing relations (228), (230), (251) and (260) that have the form

$$\Delta E_S=-\sum _{j=1}^3{I}_{[t_1,t_2]}^{(M_t)}\left[t\right]I_W^{(M)}[x_1,x_2,x_3]D_{(K_j)}^{x_j,\ast }\left[{x^{\prime }}_j\right](V_j\rho \mathcal{E}),$$

(262)

$$\Delta A_F=I_W^{(M)}[x_1,x_2,x_3]I_{[t_1,t_2]}^{(M_t)}\left[t\right]\rho (\mathbf{F},\mathbf{V}),$$

(263)

$$\Delta A_S=\sum _{j=1}^3{I}_W^{(M)}[x_1,x_2,x_3]I_{[t_1,t_2]}^{M_t}\left[t\right]D_{(K_j)}^{x_j,\ast }\left[{x^{\prime }}_j\right]({\mathbf{p}}_j,\mathbf{V}),$$

(264)

$$\Delta Q_S=-\sum _{j=1}^3{I}_W^{(M)}[x_1,x_2,x_3]I_{[t_1,t_2]}^{(M_t)}\left[t\right]D_{(K_j)}^{x_j,\ast }\left[{x^{\prime }}_j\right]q_j,$$

(265)

and equating the resulting sum to relation (226) in the form

$$\Delta E_t=I_W^{(M)}[x_1,x_2,x_3]I_{[t_1,t_2]}^{(M_t)}\left[t\right]D_{(K_t)}^{t,\ast }\left[t^{\prime }\right](\rho \mathcal{E}),$$

(266)

the balance equation for general nonlocal continuum takes the integral form

$$I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_W^{(M)}[x_1,x_2,x_3]D_{(K_t)}^{t,\ast }\left[t^{\prime }\right](\rho \mathcal{E})=$$

(267)

$$I_W^{(M)}[x_1,x_2,x_3]I_{[t_1,t_2]}^{(M_t)}\left[t\right]\left(-\sum _{j=1}^3{D}_{(K_j)}^{x_j,\ast }\left[{x^{\prime }}_j\right](V_j\rho \mathcal{E})+\rho (\mathbf{F},\mathbf{V})\right)+$$

(268)

$$I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_W^{(M)}[x_1,x_2,x_3]\left(\sum _{j=1}^3{D}_{(K_j)}^{x_j,\ast }\left[{x^{\prime }}_j\right]({\mathbf{p}}_j,\mathbf{V})-\sum _{j=1}^3{D}_{(K_j)}^{x_j,\ast }\left[{x^{\prime }}_j\right]q_j\right).$$

(269)

As a result, the following theorem was proved.

Theorem 13

(Energy balance in GF integral form).

Let the following conditions be satisfied

$$(\rho \mathcal{E})(t,x_1,x_2,x_3)\in C_{-1}^{\{1,0\}}({\mathbb{R}}_{+}^4),$$

(270)

$$(V_j\rho \mathcal{E})(t,x_1,x_2,x_3),({\mathbf{p}}_j,\mathbf{V})(t,x_1,x_2,x_3),q_j(t,x,y,z)\in C_{-1}^{\{0,1\}}({\mathbb{R}}_{+}^4),$$

(271)

$$\rho (\mathbf{F},\mathbf{V})(t,x_1,x_2,x_3)\in C_{-1}^{\{0,0\}}({\mathbb{R}}_{+}^4).$$

(272)

Then, the GF integral balance equation of total energy of general nonlocal continuum in the parallelepiped region $W\subset {\mathbb{R}}_{0,+}^3$ has the form

$$I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_W^{(M)}[x_1,x_2,x_3]D_{(K_t)}^{t,\ast }\left[t^{\prime }\right](\rho \mathcal{E})=$$

(273)

$$I_{[t_1,t_2]}^{(M_t)}\left[t\right]I_W^{(M)}[x_1,x_2,x_3]\left(\rho (\mathbf{F},\mathbf{V})+\sum _{j=1}^3{D}_{(K_j)}^{x_j,\ast }\left[{x^{\prime }}_j\right]\left(({\mathbf{p}}_j,\mathbf{V}-V_j(\rho \mathcal{E})-q_j\right)\right).$$

(274)

The fact that Equation (274) must be satisfied for any regions in the form of a parallelepiped and the general fractional analogue of Titchmarsh’s Theorem 6, can be used to obtain the balance equation for the total energy in the general fractional differential form

$$D_{(K_t)}^{t,\ast }\left[t^{\prime }\right](\rho \mathcal{E})=-\sum _{j=1}^3{D}_{(K_j)}^{x_j,\ast }\left[{x^{\prime }}_j\right](V_j\rho \mathcal{E})+\rho (\mathbf{F},\mathbf{V})+$$

$$\sum _{j=1}^3{D}_{(K_j)}^{x_j,\ast }\left[{x^{\prime }}_j\right]({\mathbf{p}}_j,\mathbf{V})-\sum _{j=1}^3{D}_{(K_j)}^{x_j,\ast }\left[{x^{\prime }}_j\right]q_j.$$

(275)

Equation (275) can be rewritten as

$$D_{(K_t)}^{t,\ast }\left[t^{\prime }\right](\rho \mathcal{E})+\sum _{j=1}^3{D}_{(K_j)}^{x_j,\ast }\left[{x^{\prime }}_j\right](V_j\rho \mathcal{E})=$$

$$\rho (\mathbf{F},\mathbf{V})+\sum _{j=1}^3{D}_{(K_j)}^{x_j,\ast }\left[{x^{\prime }}_j\right]\left(({\mathbf{p}}_j,\mathbf{V})-q_j\right).$$

(276)

Equation (276) can be represented in the compact form. As a result, the theorem for the total energy balance in general nonlocal continuum can be formulated in the following form.

Theorem 14

(Energy balance in GF differential form). Let the following conditions be satisfied

$$D_{(K_t)}^{t,\ast }\left[t^{\prime }\right](\rho \mathcal{E}),\rho (F_l{V}_l)\in C_{-1,(K)}({\mathbb{R}}_{+}^4),$$

(277)

$${\sigma }_{jl}{V}_l,V_j(\rho \mathcal{E}),q_j\in C_{-1,(K)}({\mathbb{R}}_{+}^4)$$

(278)

for all $j,l=1,2,3$.

Then, the GF differential balance equation of total energy of general nonlocal continuum in the parallelepiped region $W\subset {\mathbb{R}}_{0,+}^3$ has the form

$$D_{(K_t)}^{t,\ast }\left[t^{\prime }\right](\rho \mathcal{E})=\sum _{k=1}^3\rho (F_k{V}_k)+\sum _{j=1}^3{D}_{(K_j)}^{x_j,\ast }\left[{x^{\prime }}_j\right]\left(\sum _{l=1}^3{\sigma }_{jl}{V}_l-V_j(\rho \mathcal{E})-q_j\right).$$

(279)

where

$$\rho \mathcal{E}=\rho e_i+\frac{1}{2}\rho V^2.$$

(280)

Note that for the non-local media the differential balance equation for total energy (279) depends on the region W and in fact is an integro-differential equation.

Remark 8.

Let us consider the case of a memoryless continuum. In this case, the general fractional derivative with respect to time variable is a standard derivative of the first order. Then, Equation (279) takes the form

$$\frac{\partial (\rho \mathcal{E})}{\partial t}=\rho \sum _{k=1}^3(F_k{V}_k)+\sum _{j=1}^3{D}_{(K_j)}^{x_j,\ast }\left[{x^{\prime }}_j\right]\left(\sum _{k=1}^3{\sigma }_{jk}{V}_k-V_j(\rho \mathcal{E})-q_j\right).$$

(281)

If all general fractional derivatives with respect to coordinates are standard derivatives of the first order, then Equation (281) takes the form of the standard balance equation of total energy for local media.

6.6. General FVC Form of Energy Balance Equation

The balance equations of general nonlocal media, which are derived by using parallelepiped region W, can be generalized to a wide class of domains and surfaces by using the theorems of the general fractional vector calculus [86].

Using the general fractional vector calculus (General FVC), Equation (279) can be written in the form

$$D_{(K_t)}^{t,\ast }\left[t^{\prime }\right](\rho \mathcal{E})=\rho (\mathbf{V},\mathbf{F})+{Div}_W^{(K)}\left(({\mathcal{P}}_{\sigma },\mathbf{V})-\mathbf{V}(\rho \mathcal{E})-\mathbf{q}\right),$$

(282)

where

$${Div}_W^{(K)}({\mathcal{P}}_{\sigma },\mathbf{V})=\sum _{j=1}^3{D}_{(K_j)}^{x_j,\ast }\left[{x^{\prime }}_j\right]\left(\sum _{k=1}^3{\sigma }_{jk}{V}_k\right).$$

(283)

The product $({\mathcal{P}}_{\sigma },\mathbf{V})$ of the tensor ${\mathcal{P}}_{\sigma }$ and the vector $\mathbf{V}$ is the vector

$$({\mathcal{P}}_{\sigma },\mathbf{V})=\sum _{j=1}^3{({\mathcal{P}}_{\sigma },\mathbf{V})}_j{\mathbf{e}}_j$$

(284)

that has the components

$${({\mathcal{P}}_{\sigma },\mathbf{V})}_j=\sum _{k=1}^3{\sigma }_{jk}{V}_k.$$

(285)

The components of the tensor $\mathcal{P}$ have the form

$${\mathcal{P}}_{\sigma }=\sum _{j=1}^3\sum _{k=1}^3{\sigma }_{jk}{\mathbf{e}}_j\otimes {\mathbf{e}}_k.$$

(286)

The tensor ${\mathcal{P}}_{\sigma }$ consists of nine components ${\sigma }_{jk}(t,x_1,x_2,x_3)$ that define the stress state at the point $(x_1,x_2,x_3)\in W\subset {\mathbb{R}}_{0,+}^3$.

6.7. Spatial Power-Law Nonlocality without Memory

Let us consider the case of memoryless continuum with the spatial power-law nonlocality. In this case, the general fractional derivatives are the Caputo fractional derivatives and Equation (276) takes the form

$$\frac{\partial (\rho \mathcal{E})}{\partial t}+\sum _{k=1}^3{}_{a_k}^C{D}_{x_k}^{{\alpha }_k}(V_k(\rho \mathcal{E}))=$$

$$=\rho (\mathbf{F},\mathbf{V})+\sum _{k=1}^3{}_{a_k}^C{D}_{x_k}^{{\alpha }_k}({\mathbf{p}}_k,\mathbf{V})-\sum _{k=1}^3{}_{a_k}^C{D}_{x_k}^{{\alpha }_k}{q}_k.$$

(287)

Equation (287) can be represented in the compact form

$$\frac{\partial (\rho \mathcal{E})}{\partial t}=\rho (F_k{V}_k)+\sum _{k=1}^3{}_{a_k}^C{D}_{x_k}^{{\alpha }_k}({\sigma }_{kl}{V}_l-V_k(\rho \mathcal{E})-q_k).$$

(288)

Let us substitute expression (280) into Equation (288). The product rule (the Leibniz rule) for the time-derivative of the first order and the continuity equation, can be used to get

$$\rho \frac{\partial }{\partial t}\left(e_i+\frac{V^2}{2}\right)-\left(e_i+\frac{V^2}{2}\right)\sum _{k=1}^3{}_{a_k}^C{D}_{x_k}^{{\alpha }_k}(V_k)=$$

$$\rho (F_k{V}_k)+\sum _{k=1}^3{}_{a_k}^C{D}_{x_k}^{{\alpha }_k}\left(\sum _{l=1}^3{\sigma }_{kl}{V}_l-V_k(\rho \mathcal{E})-q_k\right).$$

(289)

Equation (289) describes the change of total energy in continuum with the power-law spatial nonlocality. It is valid for any of the stress-strain rates for the power-law non-local continua without memory.

7. Conclusions

In the suggested paper, the theory of nonlocal continua with general type of nonlocality is developed. The non-local continuum mechanics is formulated by using the general fractional calculus (GFC) in the Luchko form [81,82,83] and general fractional vector calculus (GFVC) in the form that is proposed in [86].

The integral and differential equations, which describe the conservation laws of mass, momentum, and energy for the general nonlocal continuum, are derived. The integral forms of the balance equations of general nonlocal continuum are derived by using the second fundamental theorems of general fractional calculus. The differential forms of the balance equations of general nonlocal continuum are derived by using the proposed fractional analogue of the Titchmarsh theorem and the first fundamental theorems of the GFC. Using the GFVC, the general equations for conservation of mass, momentum, and energy are obtained for continuum with general form of nonlocality in space and time.

In this paper, some basic concepts of general nonlocal continuum are discussed in Section 3. The main results of this article are derivations of the balance equations for mass, momentum, and energy, which describe conservation laws for general nonlocal continuum. These equations are derived in the two forms: general fractional integral and differential forms. In this article, it is proved that the fundamental theorems of calculus, which describe the relationship between differential and integral operators, make it possible to derive balance equations.

Let us briefly point out the main ideas and methods for deriving balance equations for nonlocal media:

• To derive general balance equation in the GF integral form, the second fundamental theorems (Theorems 2 and 3) of the GFC is used;
• The first fundamental theorems (Theorems 1 and 4) of the GFC and the proposed fractional analogue of the Titchmarsh theorem (Theorem 6) are used to derive differential form of general balance equations from the integral form of balance equations;
• Using the general fractional vector calculus, the balance equations are suggested for a wide class of regions and surfaces of the general nonlocal continuum.

A more detailed list of the main results in the form of theorems and equations obtained in this paper would include:

• The mass balance equation for continuum with general space and time nonlocality:

  −

  The mass balance equation in the GF integral form for general nonlocal continuum is given by Theorem 9 for regions in the form of a parallelepiped.

  −

  The mass balance equation in the GF differential form for general nonlocal continuum is given by Theorem 10 for regions in the form of a parallelepiped.

  −

  Using the general fractional vector calculus, the mass balance equation in GF differential form is described by Equation (133) for a wide class of regions and surfaces.

• The momentum balance equation for continuum with general space and time nonlocality:

  −

  The momentum balance equation in the GF integral form for general nonlocal continuum is given by Theorem 11 for regions in the form of a parallelepiped.

  −

  The momentum balance equation in the GF differential form for general nonlocal continuum is given by Theorem 12 for regions in the form of a parallelepiped.

  −

  Using the general fractional vector calculus, the momentum balance equation in GF differential form is described by Equation (195) for a wide class of regions and surfaces.

• The energy balance equation for continuum with general space and time nonlocality:

  −

  The energy balance equation in the GF integral form for general nonlocal continuum is given by Theorem 13 for regions in the form of a parallelepiped.

  −

  The energy balance equation in the GF differential form for general nonlocal continuum is given by Theorem 14 for regions in the form of a parallelepiped.

  −

  Using the general fractional vector calculus, the energy balance equation in GF differential form is described by Equation (282) for a wide class of regions and surfaces.

It should be noted that in order to solve the equations of general nonlocal continuum mechanics, one can use the methods of general operational calculus that was proposed by Luchko in [83,85].