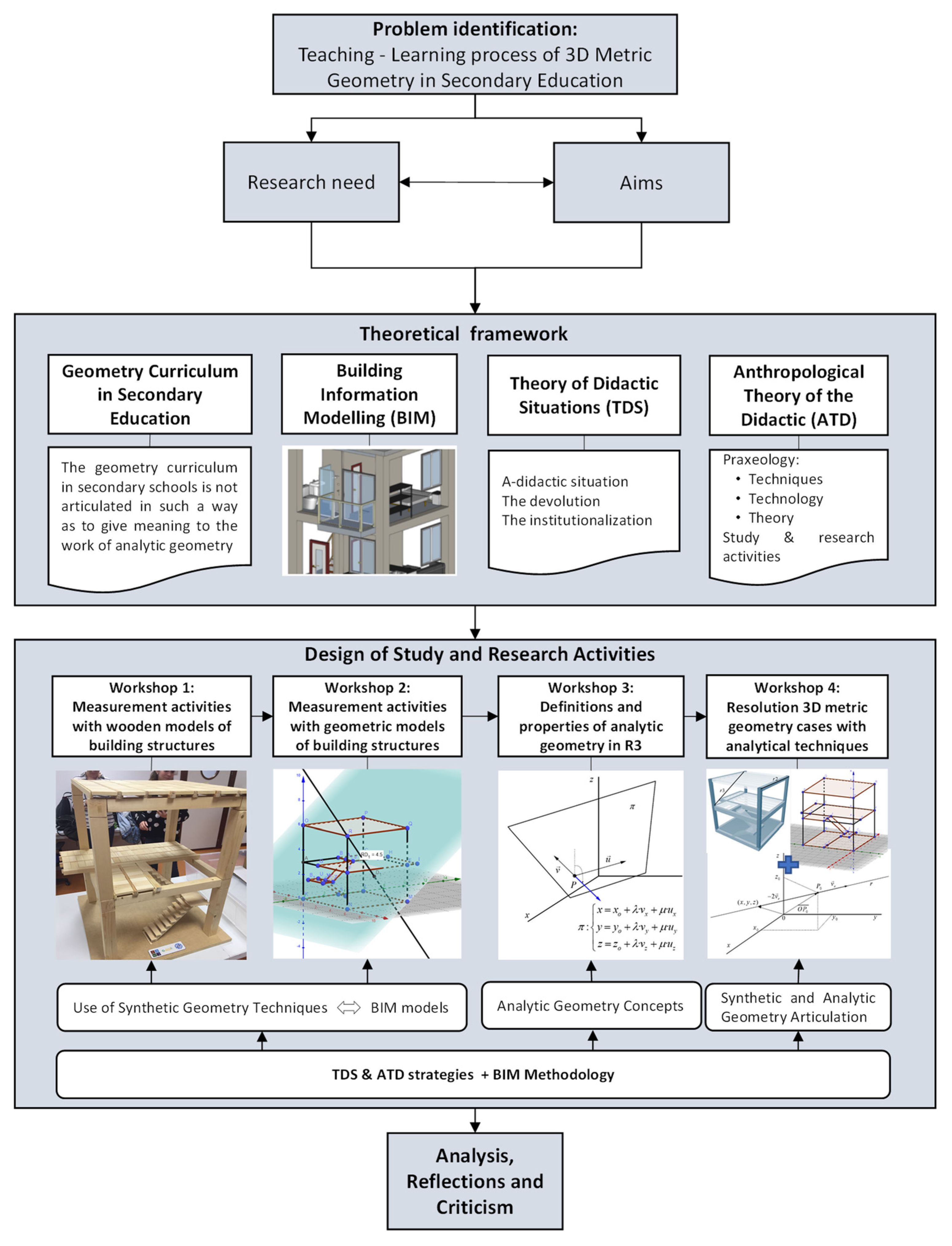
Design of 3D Metric Geometry Study and Research Activities within a BIM Framework
Abstract
:1. Introduction
a didactic device where the student can learn to do the type of work necessary to deepen the study of a problems field. Thus, the starting set is a type of problems that progressively expands as the study process progresses thanks to the development of mathematical techniques and the emergence of new technological-theoretical needs.
- High school students fail to engage with many of the mathematical works of the curriculum. In other words, students fail to comprehend the essence, usefulness, and meaning of mathematical works. So that students are able to understand a mathematic work, four aspects of the mathematical discipline should be addressed, which are: “not to forget the questions to which the work responds; combine “deductive reasoning” with “conjectural thinking”; identify and respect the laws governing the development of techniques; and produce appropriate technology to increase the efficiency and intelligibility of techniques” [11] (p. 133).
- The study of mathematics in the second year of A-level courses is becoming the training to prepare for the university entrance exam. This fact is evidenced by the numerous existing publications of resolved exercises to this exam and by the collections of exercise types solved in the A-level course textbooks [12,13].
- Algorithmizing the techniques used to solve problematic issues or tasks. An algorithm is understood as a mathematical technique whose application is totally determined [3].
2. Methods
- The identification of a problem or issue that requires study or may be improved in the field of secondary education in mathematics didactics.
- The justification of the need for the research to be carried out.
- The objectives of the proposal.
- A description of the theoretical framework of the problem.
- The development of research for the design of the didactic proposal.
- An analysis of the work carried out, reflection, and criticism.
3. Background and Theoretical Framework
3.1. Summary of Geometry Curriculum at Compulsory Secondary and Higher Secondary Education Levels in the Region of Murcia (Spain)
- Yes, there are contents dedicated to measuring areas, distances, and volumes in CSE and A-level.
- Not only 2D figures are studied in CSE; already in the second and third year of CSE, polyhedrons and revolution bodies appear as included content along with their areas, their volumes, and their symmetries.
- In the fourth year of CSE, there is already an initiation to analytic geometry in the plane: coordinates, vectors, equations of the line, parallelism, and perpendicularity.
- Only in the third year of CSE is there talk about the geometry of the plane and geometric place that are more purely synthetic contents. In the standards of these contents, there is talk of solving simple geometric problems using the midpoint of a segment and the mediatrix of an angle (Decree 220/2015, p. 30996). Therefore, in CSE there is a lack of use of deductive techniques of the Elements of Euclid to solve problems of the construction of figures.
- In the first year of A-level, metric geometry is studied in the plane: vectors, scalar product of two vectors, equation of the line, relative positions of lines.
- In the second year of A-level, metric geometry in space is studied, including: vectors in R3; scalar, vector, and mixed products; and equations of the line and plane and their relative position, along with problems of distances, areas, and volumes using algebra.
From a teaching point of view, and once the contents of compulsory education have been selected, there is a tendency to consider the «problem of the curriculum» only as a matter of sequencing and temporalization of contents, which leads to the problem of teaching methodology... The problem that should be posed is that of the reconstruction of the mathematical works selected in the curriculum as works that must be studied not only taught.[11], (p. 122)
3.2. The Theory of Didactic Situations (TDS)
The student learns by adapting to an environment that is a factor of contradictions, difficulties, and imbalances, a bit like human society does. This knowledge, the result of the student’s adaptation, is manifested by new answers that are proof of learning.
- Didactic situation. According to Panizza [18] “... it is a situation intentionally constructed in order to make students acquire a certain knowledge”. In the situation, a group of students, a certain medium, and an educational system represented by the teacher must coexist.
- A-didactic situation. Brousseau (1986), cited by Panizza, defines it as follows:“any situation which, on the one hand, cannot be adequately mastered without the implementation of the knowledge that is intended and which, on the other hand, sanctions the decisions made by the student (good or bad) without the intervention of the teacher with regard to the knowledge that is put into play”.[18] (p. 61)
- The devolution. Again, Brousseau (1986), quoted by Panizza:“Devolution is the act by which the teacher makes the student accept responsibility for a learning situation (a-didactic) or a problem and the student accepts himself the consequences of this transfer”.[18] (p. 63)
- Institutionalization. According to Panizza, quoting Brousseau (1994):“The ‘official’ consideration of the teaching object by the student, and of the student’s learning by the teacher, is a very important social phenomenon and an essential phase of the didactic process: this double recognition constitutes the object of institutionalization”.[18] (p. 67)
3.3. The Anthropological Theory of the Didactic
The fundamental theoretical construction of the Anthropological Theory of the Didactic (ATD), is the notion of praxeology or Mathematical Organization (MO). These emerge as an answer to a question or set of problematic questions that are called generative questions. Praxeologies consist of two levels:
Following the recent research lines proposed by the ATD, the need arises to introduce functional study processes into teaching systems, where knowledge does not constitute monuments that the teacher teaches to students, but material and conceptual tools, useful for studying and solving problematic situations. The Study and Research Activities (SRA) emerge as a didactic model to address the problem. In this way, it is about overcoming the classic binary structure of the teaching of mathematics, which is characterized by the presentation of technological—theoretical elements and then tasks as a means for the application of the former.
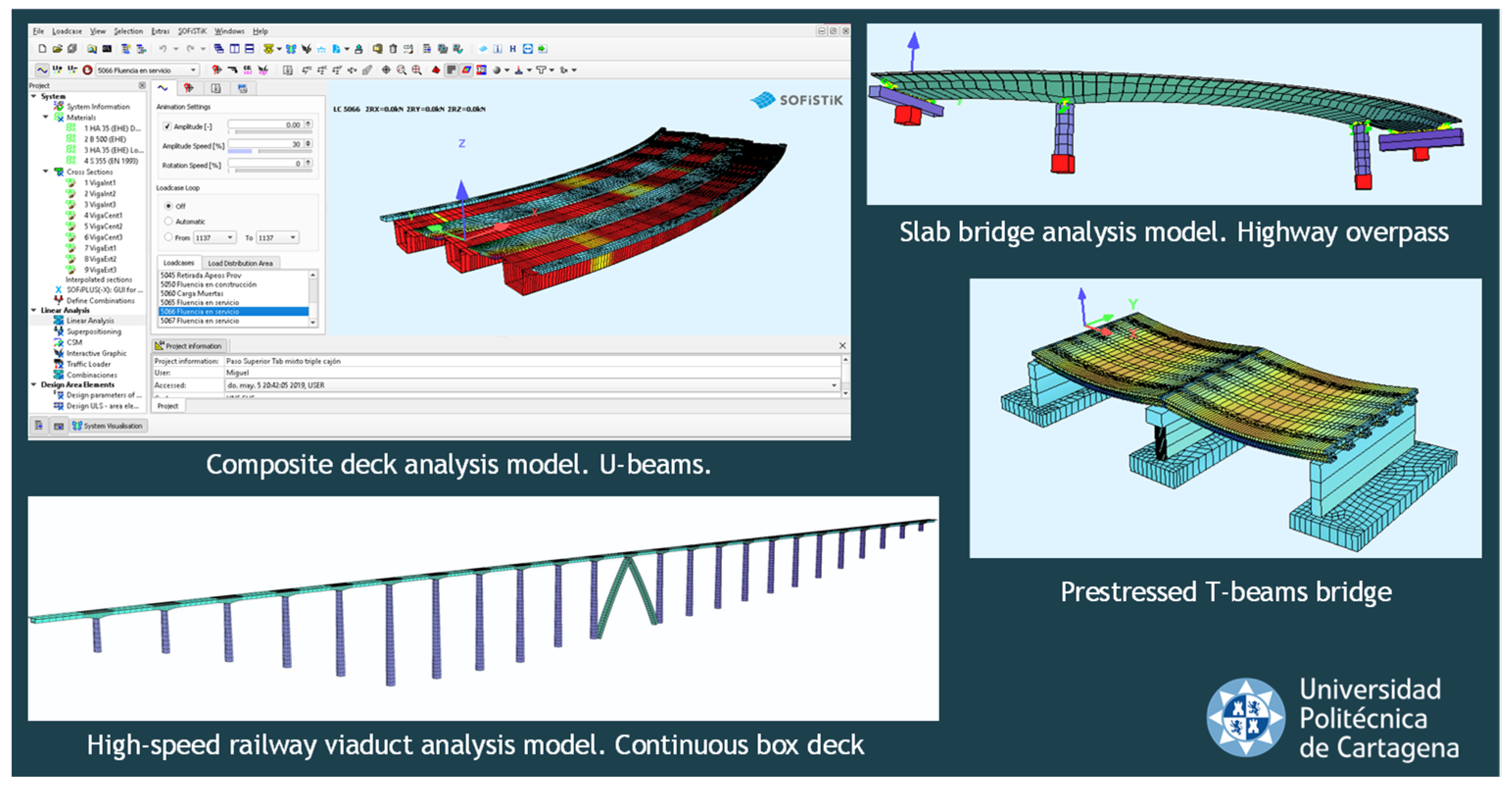
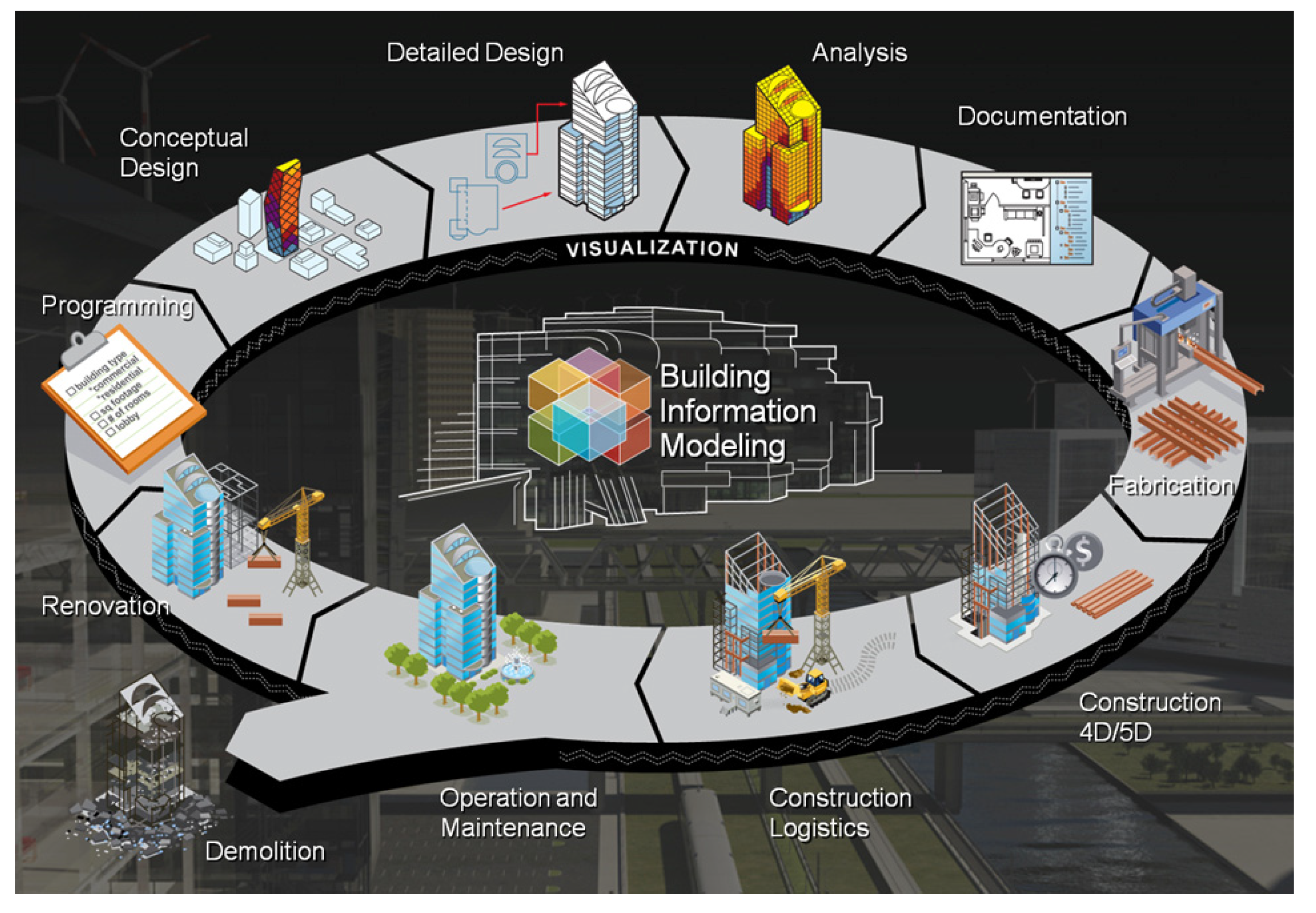
3.4. Geometric Models in Architecture and Civil Engineering, the BIM Methodology
BIM is a collaborative work methodology for the management of a project by means of a 3D digital model. It includes methodologies, processes, software and digital formats for the management of projects and construction works. It focuses on buildings, but also applies to civil works.(p. 4)
It could be defined as a digital representation of the physical and functional characteristics of a building, allowing information to be exchanged to make decisions throughout its life cycle (project, construction, use and deconstruction). It can be used to store data, perform calculations or manage the building. [...] BIM uses a common exportable language, which allows information to be shared between different agents and to carry out a real collaborative work.(p. 4)
More than 50% of international clients of construction companies demand or have an interest in the use of BIM, especially in Asia. It is estimated that BIM could: adjust project quantities by 37%; reduce building construction costs by 20%.(p. 4)
4. Design of 3D Metric Geometry Study and Research Activities
4.1. Overview of the Proposed Geometry Workshops
- Workshop 1. Measurement activities using wooden models of building structures.
- Workshop 2. Measurement activities using geometric models of building structures in GeoGebra.
- Workshop 3. Scalar, vector, and mixed product definitions of R3 vectors and their properties. Axioms of definition of planes and lines. Equations of lines and planes.
- Workshop 4. Resolution of cases with analytical techniques. Interpretation and justification of techniques. Application of techniques in construction engineering.
4.2. Workshop 1 Measurement Activities Using Wooden Models of Building Structures
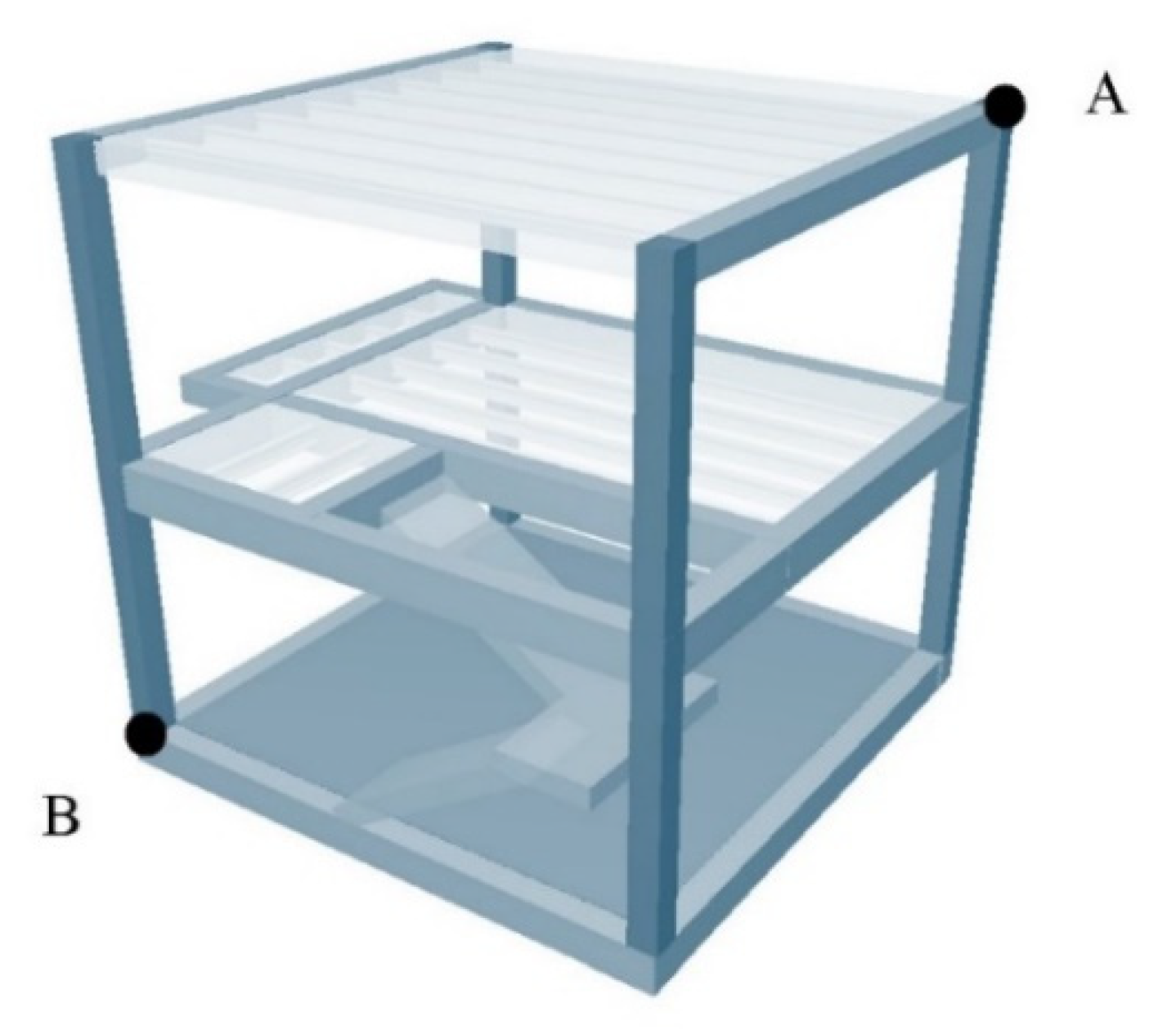
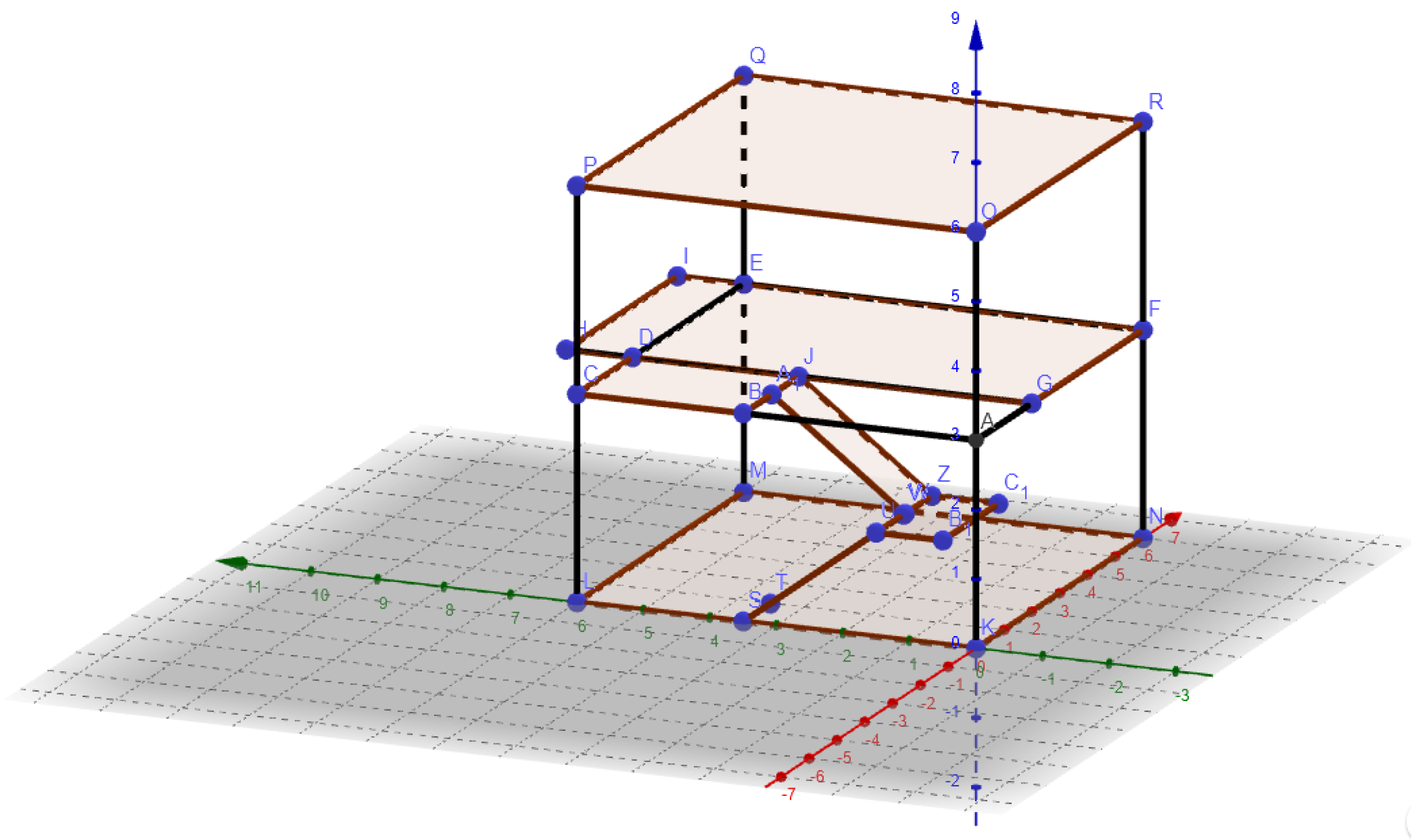
- Task 1. Find out the distance from point A to point B (Figure 8).
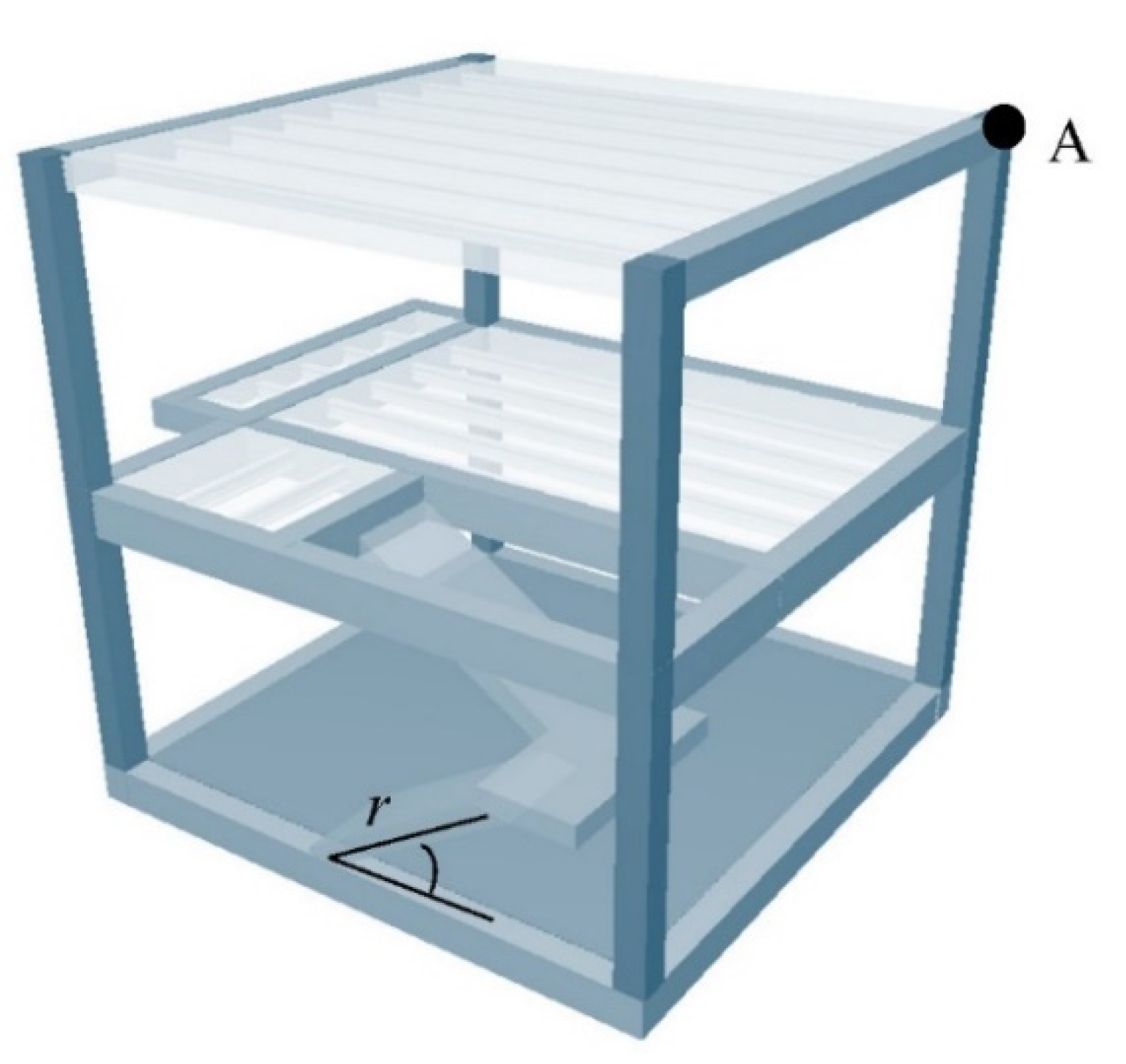
- Task 2. Find out the value of the r angle that forms the first inclined plane of the stairs with the horizontal (Figure 9).
- Task 3. Find out the area of the roof and the slab on the first floor.
- Task 4. Find out the volume of the building (without considering balconies).
- Task 5. Obtain the distance between the line r and the point A (Figure 9).
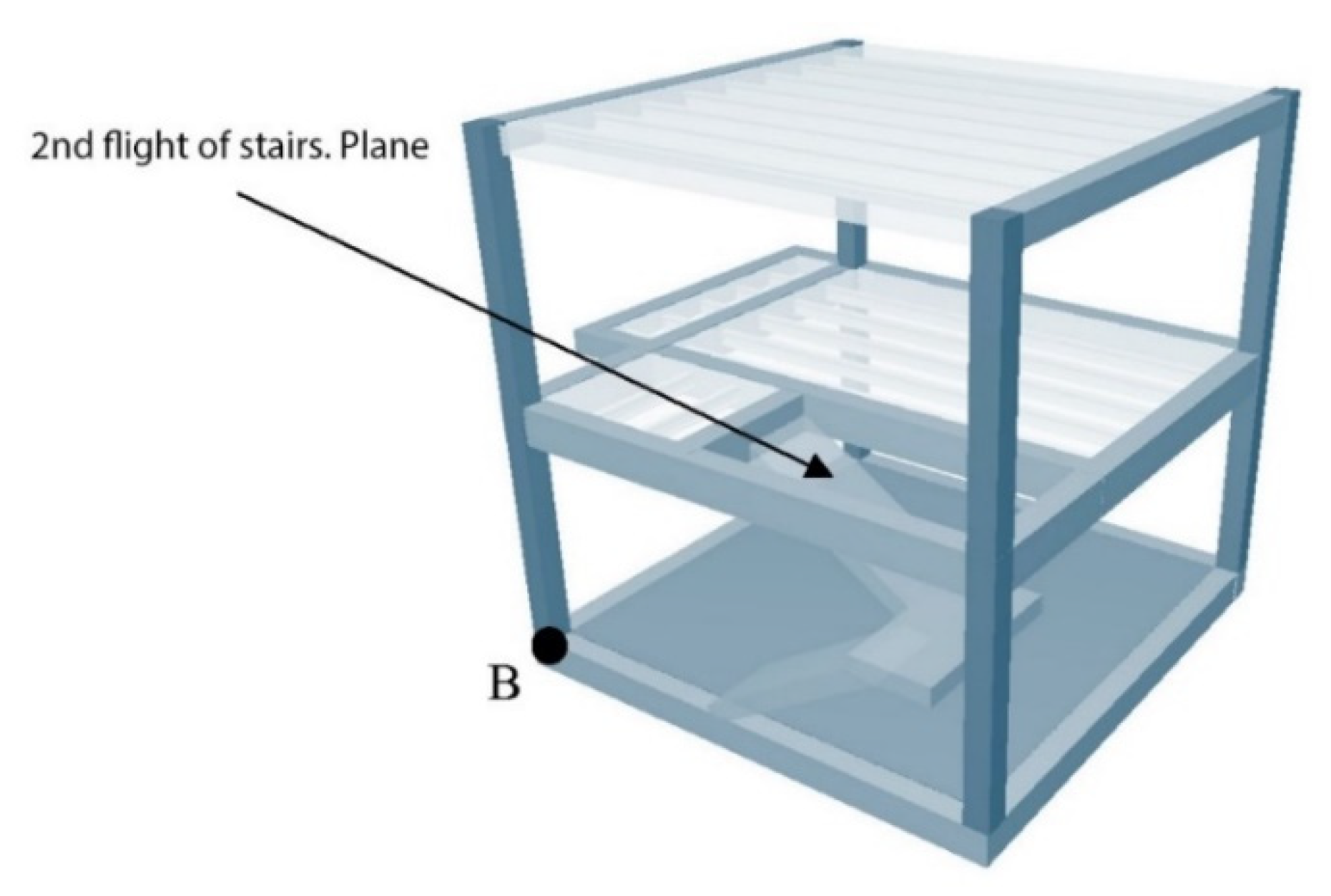
- Task 7. Obtain the distance between point B and the plane of the second flight of stairs (Figure 11).
- Task 8. Obtain the coordinates, choosing a reference system, of all the points of intersection between beams and between beams and pillars.
- Question 1. What techniques have you used? Have all students solved tasks in the same way?
- Question 2. What kind of problems can be solved with the techniques used?
- Question 3. What strategies have been followed to find out the distance between a point and a plane, and for the distance between two lines?
- Question 4. If the points, lines, and planes mentioned in the tasks were defined by coordinates of points, without having the references of the models, how would you proceed to find out the distances, angles, areas, and volumes object of the previous tasks?
4.3. Workshop 2 Measurement Activities Using Geometric Models of Building Structures in GeoGebra
- To draw one line perpendicular to another.
- To draw a line perpendicular to a plane.
- To measure distances between two points.
- To draw planes defined by three points or by a point and a line.
- To draw the point of intersection between line and plane.
- To measure angles that form two lines.
4.4. Workshop 3 Notion of Scalar, Vector, and Mixed Product of R3 Vectors and their Properties, Axioms of Planes and Lines Definition, Equations of Lines and Planes
- Activity 1. The teacher defines on the blackboard the notion of the scalar product of two vectors in R3. This notion, extracted from Lorente (n.d.) [22], is collected below:
- Activity 2. Students are asked questions about the possible properties of the scalar product of two vectors: Is it commutative? Is it distributive with the sum? What is the scalar product of a vector by itself? When is the scalar product null? What is the geometric interpretation of the scalar product?To carry out this activity and answer the questions raised, students will have to use algebraic operations that they already know.
- Activity 3. The teacher asks the students about the validity of the following analytic expression of the scalar product of two vectors:To answer, the teacher proposes to operate as follows. Equation (3):
- Activity 4. The teacher defines the vectorial product concept for two vectors in R3.
- Activity 5. Students are asked questions about the possible properties of the vector product of two vectors: Is it commutative? If not, how is it? Is it distributive with the sum? When is it zero? What geometric interpretation can be given to the vector product of two vectors in R3?
- Activity 6. Students are asked to demonstrate, if they can, the following analytical expression:
- Activity 7. The teacher defines the notion of mixed product of three vectors in R3.
- Activity 8. Students are asked questions about the possible properties of the mixed product of three vectors: Is it distributive with the sum? What happens if we swap two vectors into the mixed product? What can be the geometric interpretation of the mixed product?
- Activity 9. Students are asked to apply the analytical expression of the vector product and that of the scalar product to obtain an analytic expression of the mixed product.
- Activity 10. Students are asked about the technique they have used to perform the above activities. Is another technique possible? In addition, the results of the activities are shared and the students are left to validate them according to their criteria.
- Activity 11. Institutionalization. It is intended, at least, to reach the following conclusions among all:
- −
- Analytical expression of the mixed product (I.b, volume III, p. 68):
- −
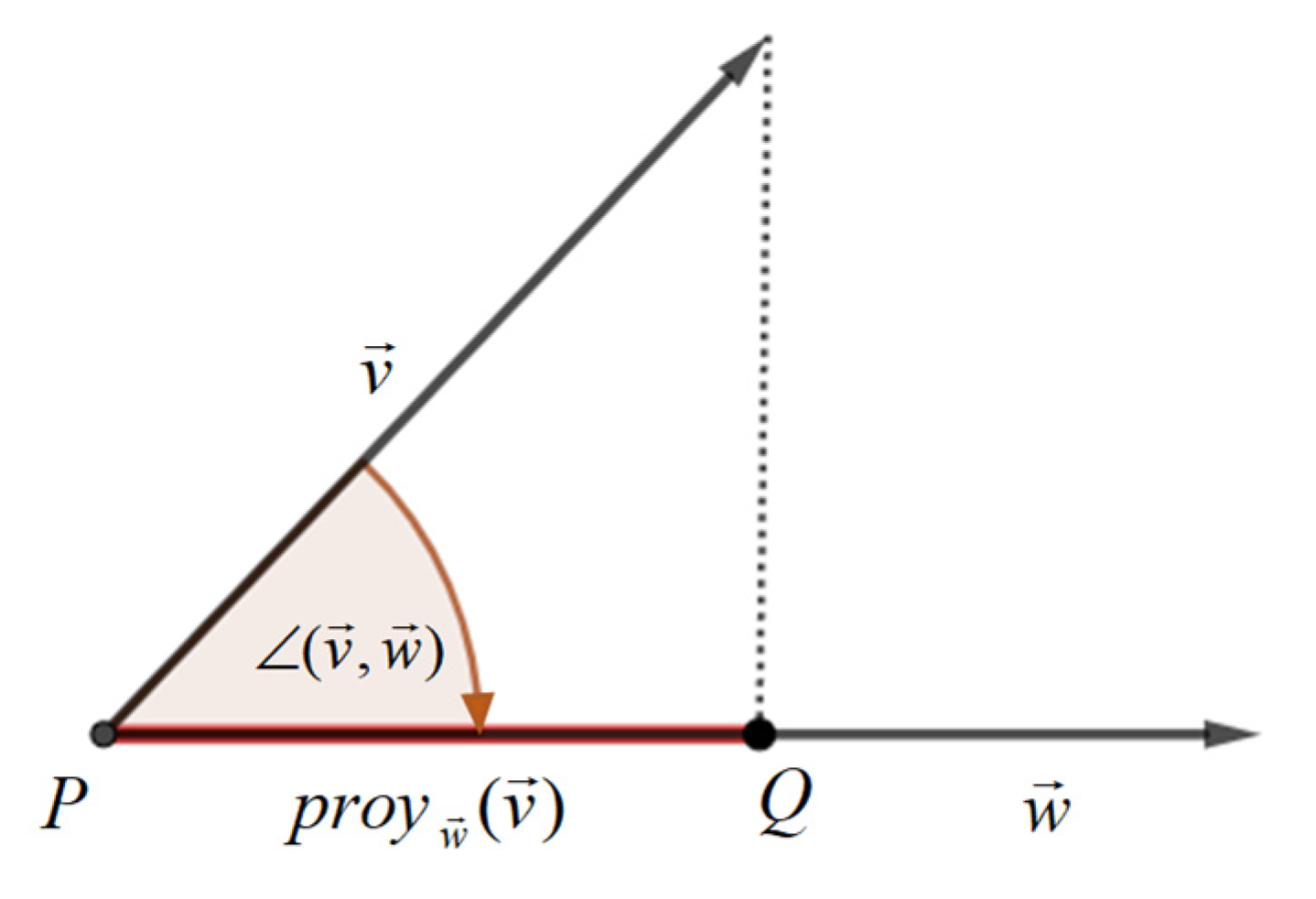
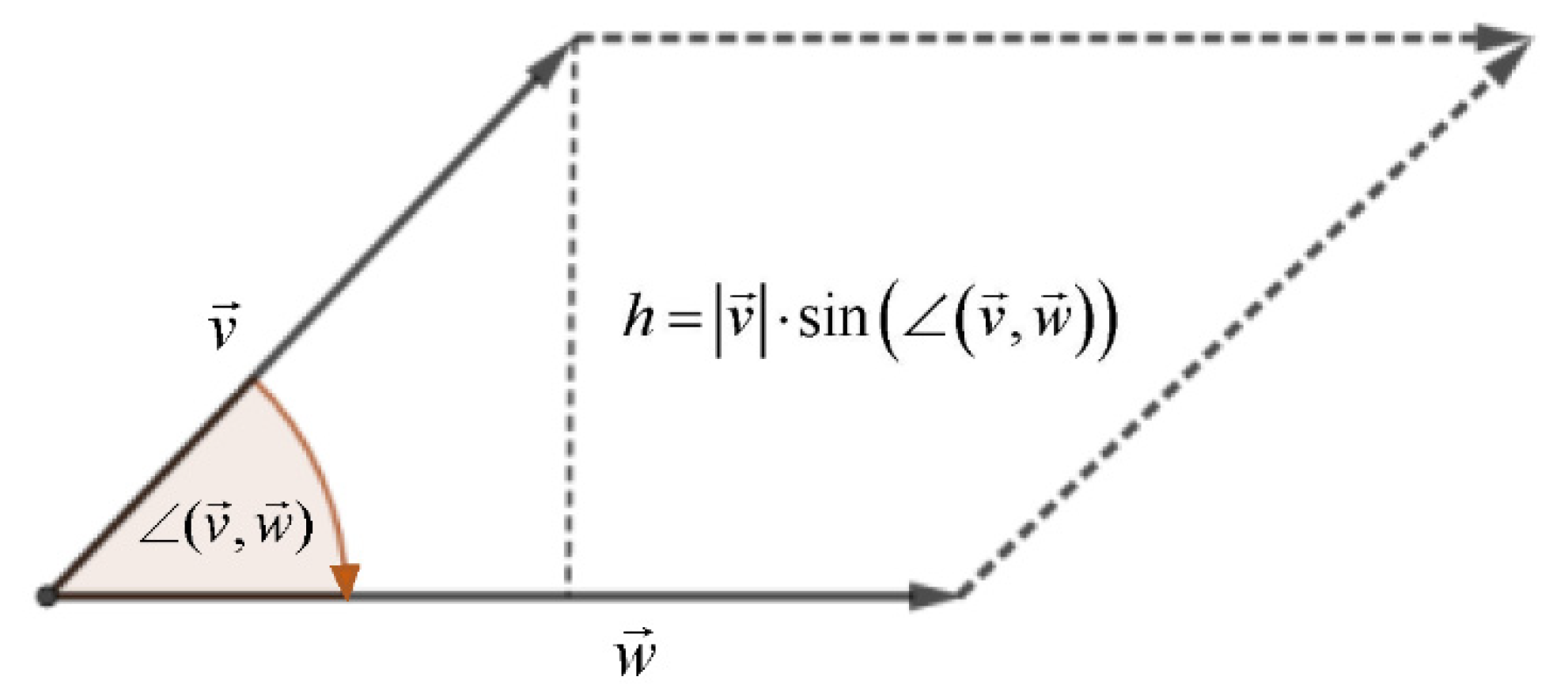
- Geometric interpretation of the scalar product of two vectors in R3 (Figure 15) (I.b, volume III, p. 64):where:is the value of the projection of onis the value of the projection of on
- −
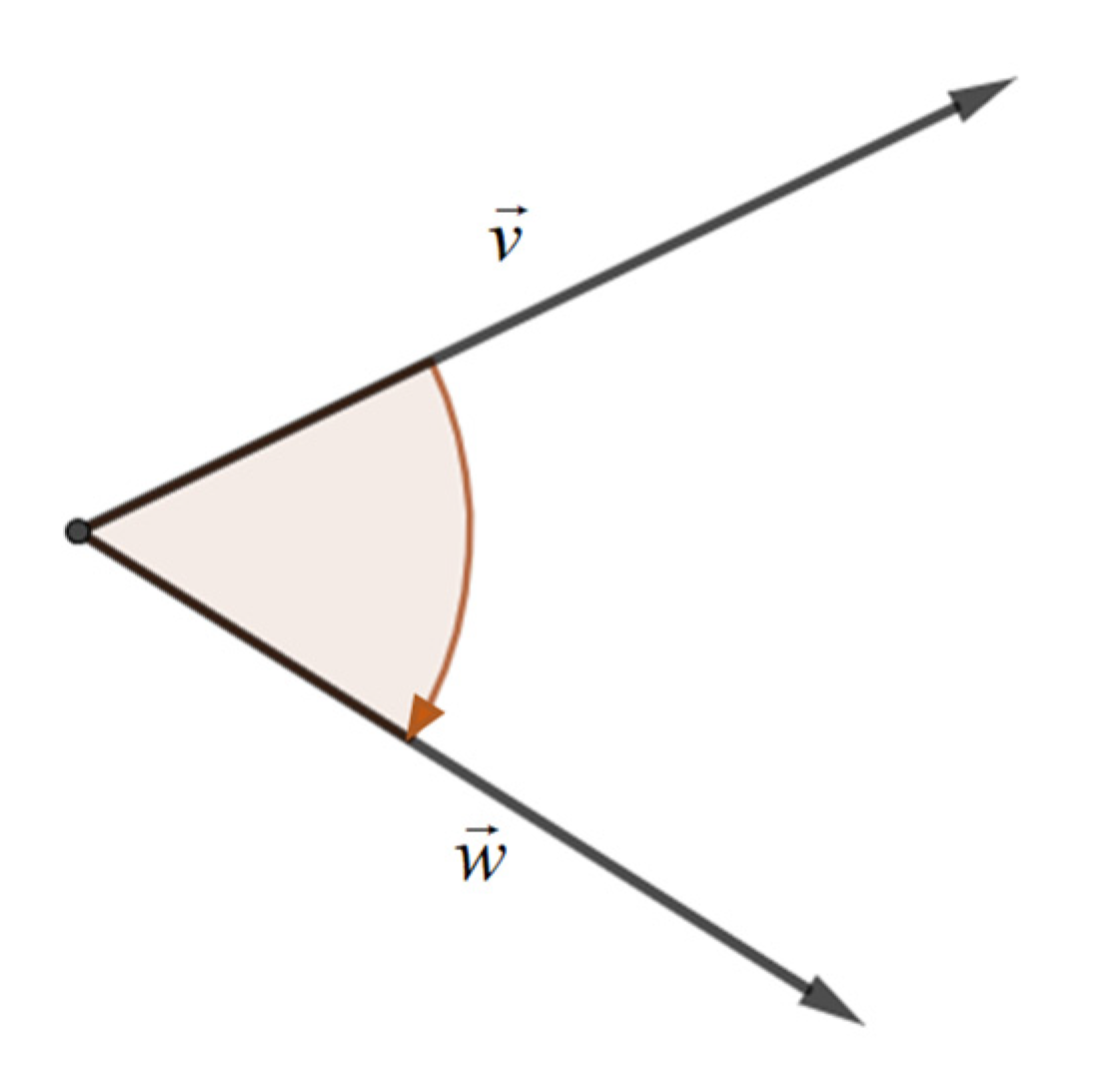
- Geometric interpretation of the vector product of two vectors in R3 (Figure 16):
- −
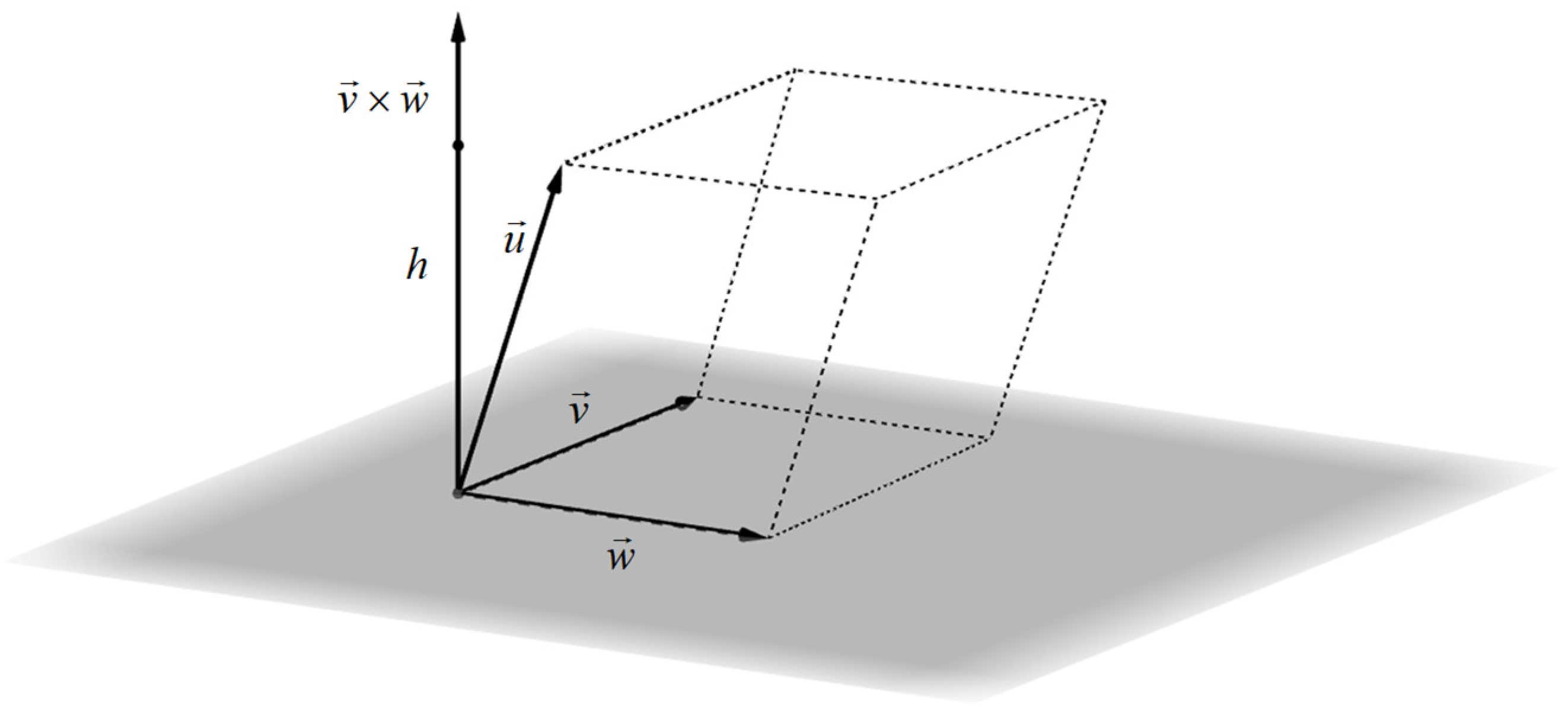
- Geometric interpretation of the mixed product of three vectors in R3 (Figure 17) (I.b, volume III, p. 67):
- Activity 12. Students are asked about the different ways of defining a line and a plane in space, as well as the relationship between the different ways of defining a plane or a line.
- Activity 13. The teacher shows on the blackboard the different forms of the equations of the line and of the plane in R3.
- Activity 14. Students are asked to tell how they can be passed from one form of equation to another and to show that the vector (A,B,C) is perpendicular to the plane .
4.5. Workshop 4 Solving of Cases with Analytical Techniques, Interpretation and Justification of Techniques, Applications of Techniques in Construction Engineering
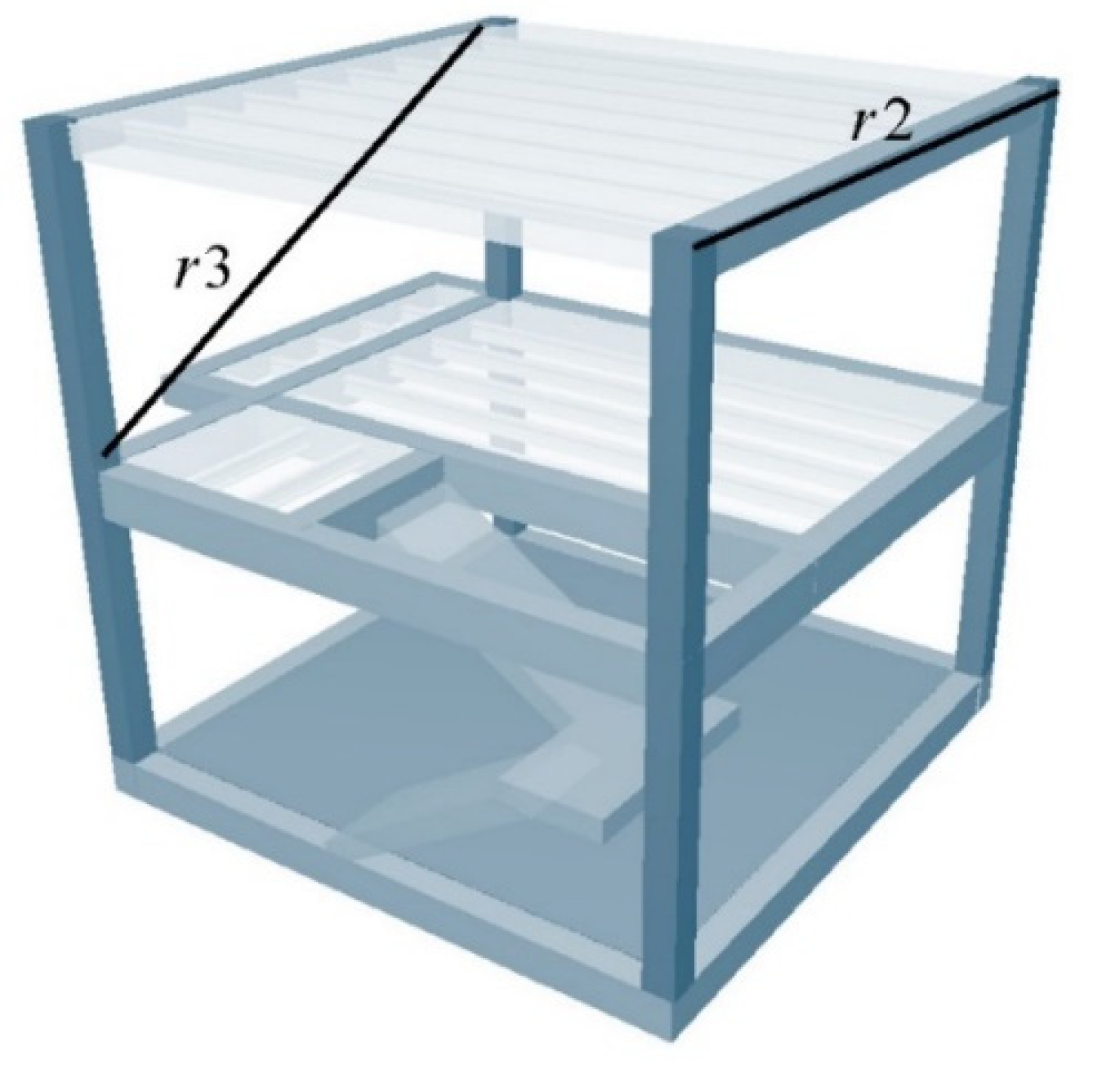
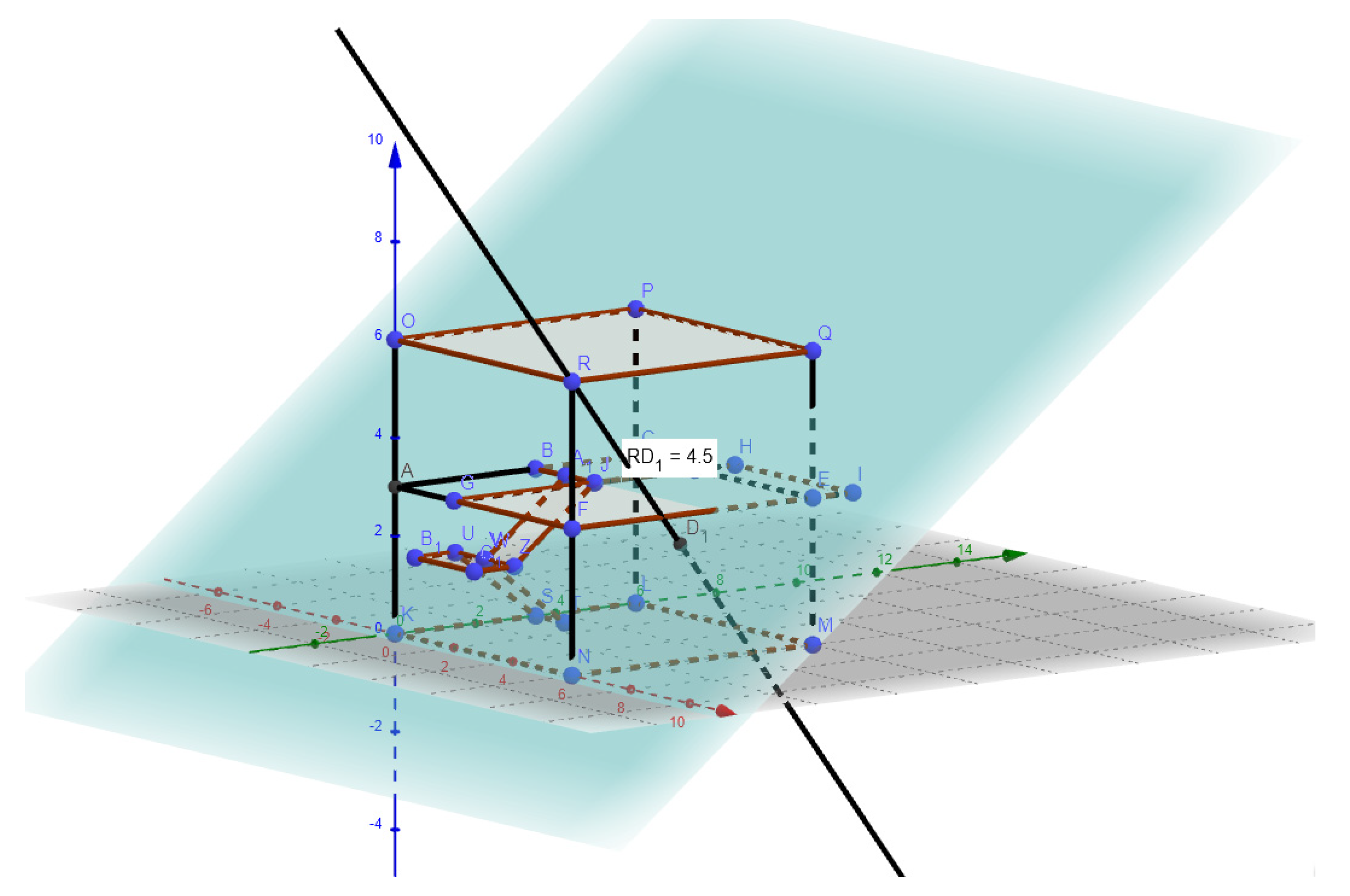
- Activity 1. The students are asked to solve the questions of the first seven tasks already carried out in Workshop 1, but now using analytical techniques. To do this, students must reformulate the statement of these tasks to express it in terms of coordinates of points and equations of lines and planes. In this activity, they will be able to consult the geometric model of Figure 11 built in GeoGebra.
- Activity 2. It is now intended that students analyze some types of problems where geometric elements (points, segments, lines, and planes) are not particular elements, but represent any element. For example, students may be asked to analyze the calculation of the distance between two lines in space, or the angle between two planes, and the strategies to follow. This is so that they come to consider the problem of the relative positions between the elements and approach their analysis with algebraic techniques.
- Activity 3. Students are asked to discuss the justification of the techniques used in the four workshops held. What sense do they give to the analytic geometry they have studied?
- Activity 4. The teacher shows students one of the applications of analytic geometry: the construction of geometric models in architecture and civil engineering and BIM methodology—basically, what is described in Section 3.4 of this article.
5. Analysis of Proposed Study and Research Activities
If the points, lines, and planes mentioned in the tasks were defined by coordinates of points, without having the references of the models, how would you proceed to find out the distances, angles, areas, and volumes object of the previous tasks?
6. Conclusions
- An innovative didactic proposal has been designed for metric geometry in the space of second year A-level courses, with a geometry workshop format, so that students can enter this mathematical work and reconstruct it within the framework of the Theory of Didactic Situations and the Anthropological Theory of the Didactic.
- The innovation of the proposal is not only provided by the change in teacher and student roles in the proposed workshop regarding traditional teaching but also due to the means used: wooden models of building structures and digital models.
- The proposed geometry workshops aim to serve as a previous step to reach the necessary level of abstraction to be able to carry out the analysis of problems such as calculating the distance between any two lines in space and the possible relative positions between them.
- The contextualization or setting of the geometry workshop in the world of the construction of structures can be a way to show—at least that is what is intended—the use of analytical geometry in the world of engineering and architecture, so that the student can give more meaning to this mathematical work.
- After the analysis carried out on the didactic proposal designed, it can be said that the proposed workshop answers, with a certain level of satisfaction, the following problems: the non-articulation of synthetic and analytical geometry; secondary school students fail to enter many of the mathematical works in the curriculum; teaching mathematics has become the training of students for university entrance exams.
- Only in the third year of CSE can we find content about geometry of the plane and geometric place in the curriculum; these are synthetic geometric contents. Therefore, in CSE there is a lack of use of deductive techniques of the Elements of Euclid to solve problems of the construction of figures. The CSE curriculum should incorporate more content about the construction of figures to develop students’ deductive reasoning.
- The proposal and analysis carried out are intended to be the first part of a larger study. The second part consists of putting into practice the didactic proposal. In this way, it will be possible to measure the improvement provided to the students of second year A-level courses in their evaluation results.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Brousseau, G. Introduction to the Study of the Theory of Didactic Situations; Libros del Zorzal: Buenos Aires, Argentina, 2007. (In Spanish) [Google Scholar]
- Balacheff, N.; Cooper, M.; Sutherland, R.; Warfield, V. Theory of Didactical Situations in Mathematics. Mathematics Education Library; Springer: Berlin/Heidelberg, Germany, 2002; Volume 19. [Google Scholar]
- Ancholea, B. The Functions of Symbolic Calculators in the Articulation between Synthetic Geometry and Analytic Geometry in Secondary. In An Overview of the TAD. III TAD International Conference; Bosch, M., Gascón, J., Ruiz Olarría, A., Artaud, M., Bronner, A., Chevallard, Y., Cirade, G., Ladage, C., Larguier, M., Eds.; CRM Documents Bellaterra: Barcelona, Spain, 2021; Volume 10, pp. 533–551. (In Spanish) [Google Scholar]
- Bosch, M.; Gascón, J. The integration of the moment of the technique in the process of studying fields of mathematical problems. Enseñanza Cienc. 1994, 12, 314–332. (In Spanish) [Google Scholar]
- Bosch, M.; Chevallard, Y.; García, F.J.; Monaghan, J. Working with the Anthropological Theory of the Didactic in Mathematics Education: A Comprehensive Casebook; Taylor & Francis: Milton Park, UK, 2019. [Google Scholar]
- Tezer, M.; Cumhur, M. Anthropological Theory of Didactics and the Probability of the Constructivist Approach Being a Solution to the Common Mistakes Made in Mathematics Lessons. Int. J. Educ. Sci. 2016, 15, 148–156. [Google Scholar] [CrossRef]
- Barquero, B.; Bosch, M.; Gascón, J. The unit of analysis in the formulation of research problems: The case of mathematical modelling at university level. Res. Math. Educ. 2019, 21, 314–330. [Google Scholar] [CrossRef]
- González-Martin, A.S.; Bloch, I.; Durand-Guerrier, V.; Maschietto, M. Didactic Situations and Didactical Engineering in university mathematics: Cases from the study of Calculus and proof. Res. Math. Educ. 2014, 16, 117–134. [Google Scholar] [CrossRef] [Green Version]
- Farias, L.M.S.; Júnior, J.V.N.; Souza, A. From Drawing to Figure and Theories of Didactic Situations and Instrumentation. Int. J. Sci. Res. 2019, 8, 1038–1048. Available online: https://www.ijsr.net/archive/v8i4/ART20194825.pdf (accessed on 20 March 2022).
- Gascón, J. Synthetic Geometry in ESO and Analytical in Baccalaureate. Two Completely Separate Worlds? SUMA: Madrid, Spain, 2002; pp. 13–25. (In Spanish) [Google Scholar]
- Chevallard, Y.; Bosch, M.; Gascón, J. Study Mathematics. The Missing Link between Teaching and Learning; Universidad de Barcelona: Barcelona, Spain, 1997. (In Spanish) [Google Scholar]
- González, L.; Valdés, Ä. Mathematics II. 2nd year Baccalaureate. Chapter 6: Metric Geometry in Space; Libros Marea Verde, 2015. Available online: www.apuntesmareaverde.org.es (accessed on 20 March 2022). (In Spanish).
- Musat, I. University Entrance Exam Problems. Mathematics II. Region of Madrid. Madrid. 2019. Available online: www.musat.net (accessed on 20 March 2022). (In Spanish).
- Olmos, J.M.; Astiz, M.A. Analysis of the lateral dynamic response of high pier viaducts under high-speed train travel. Eng. Struct. 2013, 56, 1384–1401. [Google Scholar] [CrossRef]
- Olmos, J.; Astiz, M. Improvement of the lateral dynamic response of a high pier viaduct under turbulent wind during the high-speed train travel. Eng. Struct. 2018, 165, 368–385. [Google Scholar] [CrossRef]
- Olmos, J.M.; Astiz, M. Non-linear vehicle-bridge-wind interaction model for running safety assessment of high-speed trains over a high-pier viaduct. J. Sound Vib. 2018, 419, 63–89. [Google Scholar] [CrossRef]
- Gosalves, J.; Murad, M.; Celdrán, A.; Fuentes, B.; Hayas, R.; López, J.; Zuñeda, P.P. BIM in 8 Points. BIM Commission; Ministerio de Fomento: Madrid, Spain, 2016. (In Spanish) [Google Scholar]
- Panizza, M. Teaching Mathematics at the Initial Level and the First Cycle of the EGB: Analysis and Proposals; Paidos Iberica: Barcelona, Spain, 2004. (In Spanish) [Google Scholar]
- Corica, A.R.; Marin, E.A. Study and research activity for teaching notions of geometry. Números. Rev. Didáct. Mat. 2014, 85, 91–114. (In Spanish) [Google Scholar]
- Galilei, G. Dialogues Concerning Two New Sciences; Macmillan: New York, NY, USA, 1638. [Google Scholar]
- AENOR. Standards in support of BIM. Standardization Reports. AENOR—Asociación Española de Normalización y Certificación. 2016. Available online: https://www.aenor.com/conocenos/sala-de-informacion-aenor/novedad?c=42364 (accessed on 20 March 2022). (In Spanish).
- Lorente, J.L. Mathematics II Notes to Prepare for the PAU Exam. Available online: http://joseluislorente.es/ (accessed on 20 March 2022). (In Spanish).





Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Olmos-Noguera, J.M.; Renard-Julián, E.J.; García-Cascales, M.S. Design of 3D Metric Geometry Study and Research Activities within a BIM Framework. Mathematics 2022, 10, 1358. https://doi.org/10.3390/math10091358
Olmos-Noguera JM, Renard-Julián EJ, García-Cascales MS. Design of 3D Metric Geometry Study and Research Activities within a BIM Framework. Mathematics. 2022; 10(9):1358. https://doi.org/10.3390/math10091358
Chicago/Turabian StyleOlmos-Noguera, José M., Eduardo J. Renard-Julián, and María Socorro García-Cascales. 2022. "Design of 3D Metric Geometry Study and Research Activities within a BIM Framework" Mathematics 10, no. 9: 1358. https://doi.org/10.3390/math10091358
APA StyleOlmos-Noguera, J. M., Renard-Julián, E. J., & García-Cascales, M. S. (2022). Design of 3D Metric Geometry Study and Research Activities within a BIM Framework. Mathematics, 10(9), 1358. https://doi.org/10.3390/math10091358







