Bright Soliton Solution of the Nonlinear Schrödinger Equation: Fourier Spectrum and Fundamental Characteristics
Abstract
:1. Introduction
2. Spatial Fourier Spectrum
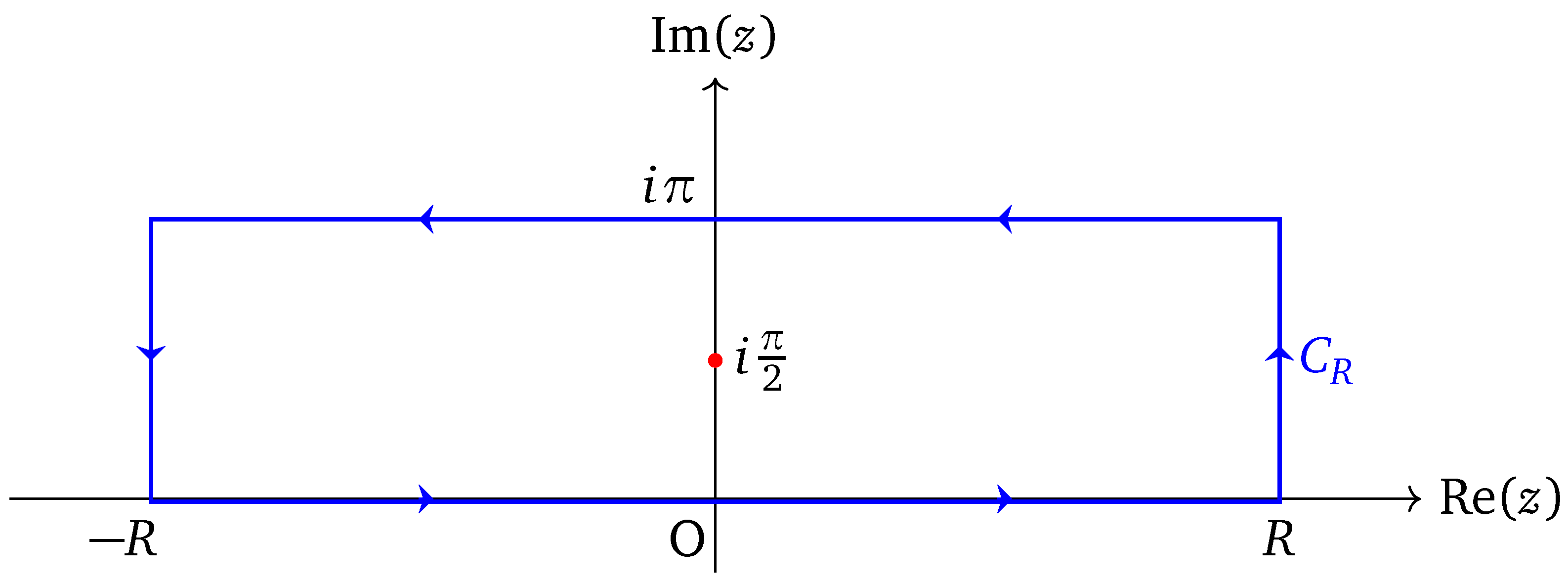
2.1. Fourier Spectrum Derivation
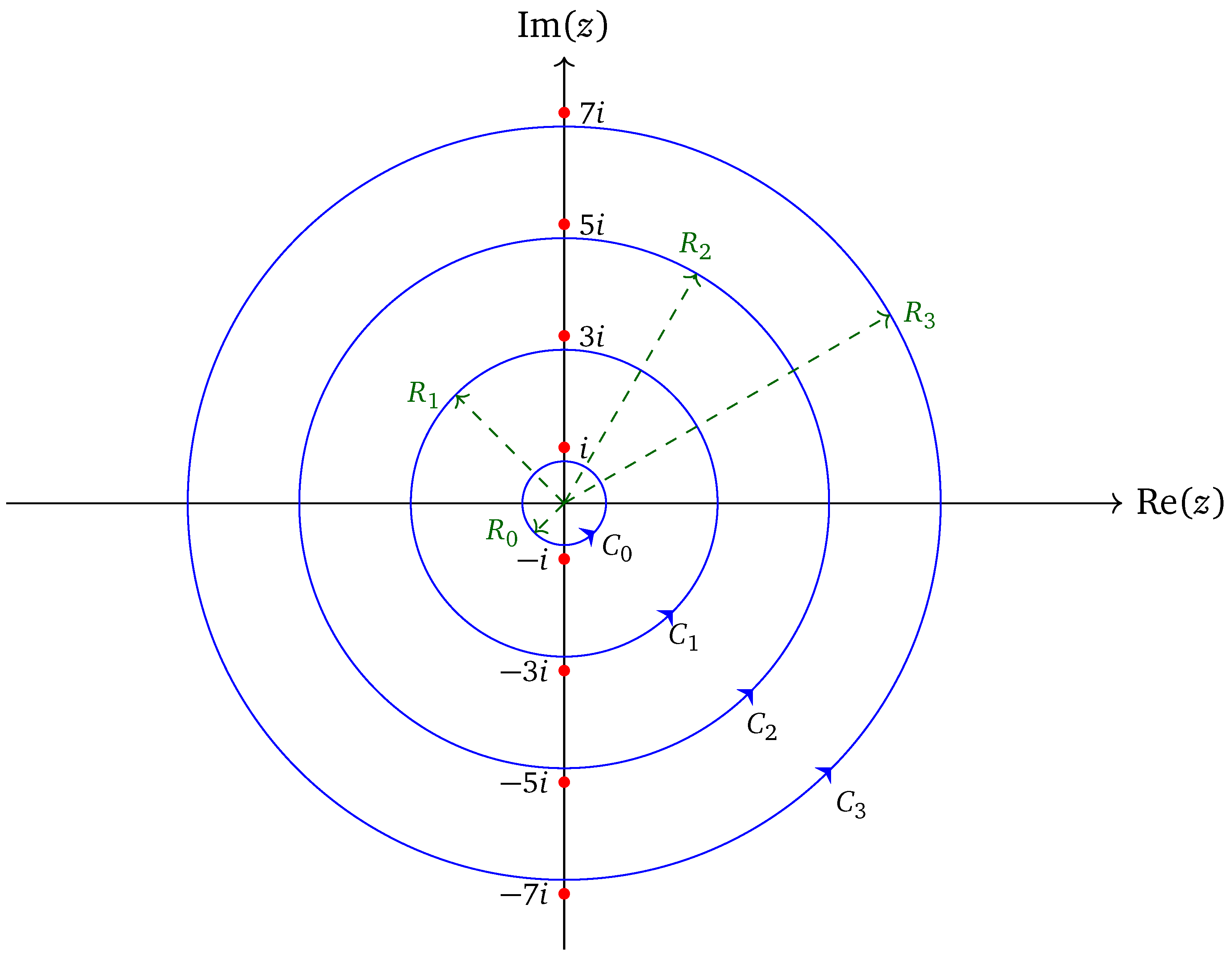
2.2. Fourier Spectrum as Infinite Series
3. Soliton Characteristics
3.1. Root-Mean-Squared Width
3.2. Other Width Measurements
3.2.1. Full-Width at Half-Maximum
3.2.2. Power-Equivalent Width
3.3. Discussion
4. Conclusions
Funding
Data Availability Statement
Conflicts of Interest
References
- Ablowitz, M.J.; Segur, H. Solitons and the Inverse Scattering Transform; Society for Industrial and Applied Mathematics: Philadelphia, PA, USA, 1981. [Google Scholar]
- Ablowitz, M.J.; Clarkson, P.A. Solitons, Nonlinear Evolution Equations and Inverse Scattering; Cambridge University Press: Cambridge, UK, 1991. [Google Scholar]
- Malomed, B. Nonlinear Schrödinger equations: Basic properties and solutions. In Encyclopedia of Nonlinear Science; Scott, A., Ed.; Routledge: New York, NY, USA, 2005. [Google Scholar]
- Debnath, L. Nonlinear Partial Differential Equations for Scientists and Engineers, 3rd ed.; Springer Science and Business Media: New York, NY, USA, 2012. [Google Scholar]
- Karjanto, N. The nonlinear Schrödinger equation: A mathematical model with its wide range of applications. In Understanding the Schrödinger Equation: Some [Non], Linear Perspectives; Simpao, V.A., Little, H.C., Eds.; Nova Science Publishers: Hauppauge, NY, USA, 2020; See also arXiv 2019, arXiv:1912.10683. [Google Scholar]
- Sulem, C.; Sulem, P.-L. The Nonlinear Schrödinger Equation–Self-Focusing and Wave Collapse; Springer: New York, NY, USA, 1999. [Google Scholar]
- Fibich, G. The Nonlinear Schrödinger Equation–Singular Solutions and Optical Collapse; Springer Science and Business Media: Cham, Switzerland, 2015. [Google Scholar]
- Chiao, R.Y.; Garmire, E.; Townes, C.H. Self-trapping of optical beams. Phys. Rev. Lett. 1964, 13, 479. [Google Scholar] [CrossRef]
- Kelley, P.L. Self-focusing of optical beams. Phys. Rev. Lett. 1965, 15, 1005. [Google Scholar] [CrossRef]
- Taniuti, T.; Washimi, H. Self-trapping and instability of hydromagnetic waves along the magnetic field in a cold plasma. Phys. Rev. Lett. 1968, 21, 209. [Google Scholar] [CrossRef]
- Benney, D.J.; Newell, A.C. The propagation of nonlinear wave envelopes. J. Math. Phys. 1967, 46, 133–139. [Google Scholar] [CrossRef]
- Karpman, V.I.; Krushkal, E.M. Modulated waves in nonlinear dispersive media. Sov. J. Exp. Theor. Phys. 1969, 28, 277. [Google Scholar]
- Tappert, F.D.; Varma, C.M. Asymptotic theory of self-trapping of heat pulses in solids. Phys. Rev. Lett. 1970, 25, 1108. [Google Scholar] [CrossRef]
- Taniuti, T.; Yajima, N. Perturbation method for a nonlinear wave modulation. I. J. Math. Phys. 1969, 10, 1369–1372. [Google Scholar] [CrossRef]
- Asano, N.; Taniuti, T.; Yajima, N. Perturbation method for a nonlinear wave modulation. II. J. Math. Phys. 1969, 10, 2020–2024. [Google Scholar] [CrossRef]
- Zakharov, V.E. Stability of periodic waves of finite amplitude on the surface of a deep fluid. J. Appl. Mech. Tech. Phys. 1968, 9, 190–194. [Google Scholar] [CrossRef]
- Hasimoto, H.; Ono, H. Nonlinear modulation of gravity waves. J. Phys. Soc. Jpn. 1972, 33, 805–811. [Google Scholar] [CrossRef]
- Osborne, A.R. Nonlinear Ocean Waves and the Inverse Scattering Transform; Academic Press: Cambridge, MA, USA, 2010. [Google Scholar]
- Pelinovsky, D. Spectral analysis. In Encyclopedia of Nonlinear Science; Scott, A., Ed.; Routledge: New York, NY, USA; London, UK, 2005; pp. 863–864. [Google Scholar]
- Karjanto, N. On spatial Fourier spectrum of rogue wave breathers. Math. Methods Appl. Sci. 2022, in press See also arXiv 2021, arXiv:2107.10547. [Google Scholar] [CrossRef]
- Bauck, J. A Note on Fourier Transform Conventions Used in Wave Analyses. 2019. Available online: https://engrxiv.org/jyt96/ (accessed on 10 November 2022).
- Agrawal, G.P. Nonlinear Fiber Optics, 6th ed.; Academic Press: Burlington, MA, USA, 2019. [Google Scholar]
- Zakharov, V.; Shabat, A. Exact theory of two-dimensional self-focusing and one-dimensional self-modulation of waves in nonlinear media. Sov. Phys. JETP 1972, 34, 62–69. [Google Scholar]
- Matveev, V.B.; Salle, M.A. Darboux Transformations and Solitons; Springer: Berlin, Germany, 1991. [Google Scholar]
- Trisetyarso, A. Application of Darboux transformation to solve multisoliton solution on non-linear Schrödinger equation. arXiv 2009, arXiv:0910.0901v1. [Google Scholar]
- Banerjee, P.P. Nonlinear Optics: Theory, Numerical Modeling, and Applications; Marcel Dekker: New York, NY, USA, 2004. [Google Scholar]
- Li, C. Nonlinear Optics: Principles and Applications; Shanghai Jiao Tong University Press: Shanghai, China; Springer Nature: Singapore, 2017. [Google Scholar]
- Akhmediev, N.N.; Ankiewicz, A. Solitons: Nonlinear Pulses and Beams; Chapman & Hall: London, UK, 1997. [Google Scholar]
- Kuznetsov, E.A. Solitons in a parametrically unstable plasma. Doklady Akad. Nauk SSSR 1977, 236, 575–577. [Google Scholar]
- Ma, Y.C. The perturbed plane-wave solutions of the cubic Schrödinger equation. Stud. Appl. Math. 1979, 60, 43–58. [Google Scholar] [CrossRef]
- Karjanto, N. Peregrine soliton as a limiting behavior of the Kuznetsov-Ma and Akhmediev breathers. Front. Phys. 2021, 9, 599767, See also arXiv 2020, arXiv:2009.00269. [Google Scholar] [CrossRef]
- Hasegawa, A.; Tappert, F. Transmission of stationary nonlinear optical pulses in dispersive dielectric fibers. I. Anomalous dispersion. Appl. Phys. Lett. 1973, 23, 142–144. [Google Scholar] [CrossRef]
- Hasegawa, A.; Tappert, F. Transmission of stationary nonlinear optical pulses in dispersive dielectric fibers. II. Normal dispersion. Appl. Phys. Lett. 1973, 23, 171–172. [Google Scholar] [CrossRef]
- Mollenauer, L.F.; Stolen, R.H.; Gordon, J.P. Experimental observation of picosecond pulse narrowing and solitons in optical fibers. Phys. Rev. Lett. 1980, 45, 1095–1097. [Google Scholar] [CrossRef]
- Taylor, J.R. (Ed.) Optical Solitons: Theory and Experiment; Cambridge University Press: Cambridge, UK, 1992. [Google Scholar]
- Abdullaev, F.; Darmanyan, S.; Khabibullaev, P. Optical Solitons; Springer: Berlin/Heidelberg, Germany, 1993. [Google Scholar]
- National Research Council. Nonlinear Science; The National Academies Press: Washington, DC, USA, 1997. [Google Scholar] [CrossRef]
- Kath, B.; Kath, B. Making waves: Solitons and their optical applications. SIAM News 1998, 31, 1–5. Available online: https://archive.siam.org/pdf/news/810.pdf (accessed on 10 November 2022).
- Christiansen, P.L.; Sorensen, M.P.; Scott, A.C. (Eds.) Nonlinear Science at the Dawn of the 21st Century; Springer: Berlin/Heidelberg, Germany; New York, NY, USA,, 2000. [Google Scholar]
- Millot, G.; Tchofo-Dinda, P. Solitons: Optical fiber solitons, physical origin and properties. In Encyclopedia of Modern Optics; Guenther, R.D., Steel, D.G., Bayvel, L., Eds.; Elsevier: Oxford, UK, 2005; Volume 5, pp. 56–65. [Google Scholar]
- Khaykovich, L.; Schreck, F.; Ferrari, G.; Bourdel, T.; Cubizolles, J.; Carr, L.D.; Castin, Y.; Salomon, C. Formation of a matter-wave bright soliton. Science 2002, 296, 1290–1293. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Strecker, K.E.; Partridge, G.B.; Truscott, A.G.; Hulet, R.G. Formation and propagation of matter-wave soliton trains. Nature 2002, 417, 150–153. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Dysthe, K.B.; Trulsen, K. Note on breather type solutions of the NLS as models for freak-waves. Phys. Scr. 1999, T82, 48–52. [Google Scholar] [CrossRef]
- Howie, J.M. Complex Analysis; Springer: London, UK; Berlin/Heildelberg, Germany, 2003. [Google Scholar]
- Brown, J.W.; Churchill, R.V. Complex Variables and Applications, 9th ed.; McGraw-Hill Education: New York, NY, USA, 2014. [Google Scholar]
- Ablowitz, M.J.; Fokas, A.S. Introduction to Complex Variables and Applications; Cambridge University Press: Cambridge, UK, 2021. [Google Scholar]
- Karjanto, N. Mathematical Aspects of Extreme Water Waves. Ph.D. Thesis, University of Twente, Enschede, The Netherlands, 2006. See also arXiv 2020, arXiv:2006.00766. [Google Scholar]
- Spiegel, M.R.; Lipschutz, S.; Schiller, J.J.; Spellman, D. Schaum’s Outlines Complex Variables with an Introduction to Conformal Mapping and Its Applications, 2nd ed.; McGraw-Hill: New York, NY, USA, 2009. [Google Scholar]
- Bak, J.; Newman, D.J. Complex Analysis, 3rd ed.; Springer Science+Business Media: New York, NY, USA, 2010. [Google Scholar]
- Zill, D.G.; Shanahan, P.D. Complex Analysis: A First Course with Applications, 3rd ed.; Jones & Bartlett Learning: Burlington, MA, USA, 2015. [Google Scholar]
- Simmons, G.F. Calculus Gems: Brief Lives and Memorable Mathematics; Mathematical Association of America: Washington, DC, USA, 2007. [Google Scholar]
- Roy, R. The discovery of the series formula for π by Leibniz, Gregory and Nilakantha. Math. Mag. 1990, 63, 291–306. [Google Scholar] [CrossRef]
- Edwards, C.J. The Historical Development of the Calculus; Springer Science and Business Media: Cham, Switzerland, 1994. [Google Scholar]
- Karjanto, N.; van Groesen, E. Qualitative comparisons of experimental results on deterministic freak wave generation based on modulational instability. J.-Hydro-Environ. Res. 2010, 3, 186–192. [Google Scholar] [CrossRef] [Green Version]
- Grami, A. Introduction to Digital Communications; Elsevier: Amsterdam, The Netherlands, 2016. [Google Scholar]
- Saleh, B.E.A.; Teich, M.C. Fundamentals of Photonics, 2nd ed.; John Wiley & Sons: Hoboken, NJ, USA, 2007. [Google Scholar]
- McGillem, C.D.; Cooper, G.R. Continuous and Discrete Signal and System Analysis, 3rd ed.; Saunders College Publishing, A Division of Holt, Reinhart and Winston: Philadelphia, PA, USA, 1991. [Google Scholar]
- Watanabe, S.; Miyakawa, M.; Yajima, N. Method of conservation laws for solving nonlinear Schrödinger equation. J. Phys. Soc. Jpn. 1979, 46, 1653–1659. [Google Scholar] [CrossRef]
- Dingemans, M.W. Water Wave Propagation over Uneven Bottoms: Part 2–Non-Linear Wave Propagation; World Scientific: Singapore, 1997. [Google Scholar]
- Dingemans, M.W.; Otta, A.K. Nonlinear modulation of water waves. In Advances in Coastal and Ocean Engineering; Liu, P.L.-F., Ed.; World Scientific: Singapore, 2001; pp. 1–75. [Google Scholar]
- Lamb, G.L., Jr. Elements of Soliton Theory; John Wiley & Sons: New York, NY, USA, 1980. [Google Scholar]
- Oppenheim, A.V.; Willsky, A.S.; Nawab, S.H. Signals & Systems; Prentice Hall: Upper Saddle River, NJ, USA, 1997. [Google Scholar]
- Hirlimann, C. Pulsed optics. In Femtosecond Laser Pulses: Principles and Experiments, 2nd ed.; Rullière, C., Ed.; Springer Science and Business Media: New York, NY, USA, 2005; pp. 25–56. [Google Scholar]
- Weyl, H. The Theory of Groups and Quantum Mechanics; Dover Publications: New York, NY, USA, 1950; An unabridged and unaltered republication of the English translation from the second (revised) German edition originally published in 1931 under the title Gruppentheorie und Quantenmechanik. [Google Scholar]
- Heisenberg, W. Über den anschaulichen Inhalt der quantentheoretischen Kinematik und Mechanik. (The actual content of quantum theoretical kinematics and mechanics). Z. Phys. J. Phys. 1927, 43, 172–198. [Google Scholar]
- Papoulis, A. The Fourier Integral and Its Applications; McCraw-Hill: New York, NY, USA, 1962. [Google Scholar]
- Papoulis, A. Systems and Transforms with Applications in Optics; McGraw-Hill: New York, NY, USA, 1968. [Google Scholar]
- Franks, L.E. Signal Theory, revised ed.; Dowden & Culver: Stroudsburg, PA, USA, 1981. [Google Scholar]
- Stein, J.Y. Digital Signal Processing: A Computer Science Perspective; John Wiley & Sons: New York, NY, USA, 2000. [Google Scholar]
- Weik, M.H. Full-width at half-maximum. In Computer Science and Communications Dictionary; Springer: Boston, MA, USA, 2000. [Google Scholar] [CrossRef]
- Mitschke, F. Fiber Optics: Physics and Technology, 2nd ed.; Springer: Berlin/Heidelberg, Germany, 2016. [Google Scholar]
- Hiremath, K.R. Coupled Mode Theory Based Modeling and Analysis of Circular Optical Microresonators. Ph.D. Thesis, University of Twente, Enschede, The Netherlands, 2005. [Google Scholar]
- Sorokin, E.; Tempea, G.; Brabec, T. Measurement of the root-mean-squared width and the root-mean-squared chirp in ultrafast optics. JOSA B 2000, 17, 146–150. [Google Scholar] [CrossRef]
- Menzel, R. Photonics: Linear and Nonlinear Interactions of Laser Light and Matter, 2nd ed.; Springer Science & Business Media: Cham, Switzerland, 2007. [Google Scholar]
- Lorenzo, J.R. Principles of Diffuse Light Propagation: Light Propagation in Tissues with Applications in Biology and Medicine; World Scientific: Singapore, 2012. [Google Scholar]
- Vashista, M.; Paul, S. Correlation between full width at half maximum (FWHM) of XRD peak with residual stress on ground surfaces. Philos. Mag. 2012, 92, 4194–4204. [Google Scholar] [CrossRef]
- Reddy, A.N.K.; Sagar, D.K. Half-width at half-maximum, full-width at half-maximum analysis for resolution of asymmetrically apodized optical systems with slit apertures. Pramana 2015, 84, 117–126. [Google Scholar] [CrossRef]
- Sekulic, D.L.; Samardzic, N.M.; Mihajlovic, Z.; Sataric, M.V. Soliton waves in lossy nonlinear transmission lines at microwave frequencies: Analytical, numerical and experimental studies. Electronics 2021, 10, 2278. [Google Scholar] [CrossRef]
- Rennie, R. (Ed.) A Dictionary of Physics, 7th ed.; Oxford University Press: Oxford, UK, 2015. [Google Scholar]
- Thompson, S.P.; Gardner, M. Calculus Made Easy; St. Martin’s Press: New York, NY, USA, 1998. [Google Scholar]
- Jones, A.R. Probability, Statistics and Other Frightening Stuff; Routledge: Oxfordshire, UK, 2018. [Google Scholar]
- Cameron, C.A. Arithmetic, geometric, harmonic, and quadratic means. Dublin J. Med. Sci. 1894, 97, 381–388. [Google Scholar] [CrossRef] [Green Version]
- Bullen, P.S. Handbook of Means and Their Inequalities; Kluwer Academic Publishers: Dordrecht, The Netherlands, 2003. [Google Scholar]
- Gwanyama, P.W. The HM-GM-AM-QM inequalities. Coll. Math. J. 2004, 35, 47–50. [Google Scholar] [CrossRef]
- Cvetkovski, Z. Inequalities: Theorems, Techniques and Selected Problems; Springer Science & Business Media: Cham, Switzerland, 2012. [Google Scholar]
- Sedrakyan, H.; Sedrakyan, N. Algebraic Inequalities; Springer: Cham, Switzerland, 2018. [Google Scholar]
- Sağlam, V.; Zaman, T.; Yücesoy, E.; Sağır, M. Estimators proposed by geometric mean, harmonic mean and quadratic mean. Sci. J. Appl. Math. Stat. 2016, 4, 115–118. [Google Scholar] [CrossRef]
- Hyndman, R.J.; Koehler, A.B. Another look at measures of forecast accuracy. Int. J. Forecast. 2006, 22, 679–688. [Google Scholar] [CrossRef] [Green Version]
- Deakin, R.E.; Kildea, D.G. A note on standard deviation and RMS. Aust. Surv. 1999, 44, 74–79. [Google Scholar] [CrossRef]
- Meyer, T. Root mean square error compared to, and contrasted with, standard deviation. Surv. Land Inf. Sci. 2012, 72, 107–108. [Google Scholar]
- Baird, L.C. Moments of a wave packet. Am. J. Phys. 1972, 40, 327–329. [Google Scholar] [CrossRef]
- Anderson, D.G.; Askne, J.I.H. Wave packets in strongly dispersive media. Proc. IEEE 1974, 62, 1518–1523. [Google Scholar] [CrossRef]
- Marcuse, D. Pulse distortion in single-mode fibers. Appl. Opt. 1980, 19, 1653–1660. [Google Scholar] [CrossRef]
- Brandt-Pearce, M.; Jacobs, I.; Lee, J.H.; Shaw, J.K. Optimal input Gaussian pulse width for transmission in dispersive nonlinear fibers. JOSA B 1999, 16, 1189–1196. [Google Scholar] [CrossRef]
- Agrawal, G.P.; Potasek, M.J. Effect of frequency chirping on the performance of optical communication systems. Opt. Lett. 1986, 11, 318–320. [Google Scholar] [CrossRef] [PubMed]
- Anderson, D.; Lisak, M. Propagation characteristics of frequency-chirped super-Gaussian optical pulses. Opt. Lett. 1986, 11, 569–571. [Google Scholar] [CrossRef] [PubMed]
- Anderson, D.; Lisak, M.; Reichel, T. Asymptotic propagation properties of pulses in a soliton-based optical-fiber communication system. JOSA B 1988, 5, 207–210. [Google Scholar] [CrossRef]
- Lazaridis, P.; Debarge, G.; Gallion, P. Time–bandwidth product of chirped sech2 pulses: Application to phase–amplitude-coupling factor measurement. Opt. Lett. 1995, 20, 1160–1162. [Google Scholar] [CrossRef]
- Marcuse, D. RMS width of pulses in nonlinear dispersive fibers. J. Light. Technol. 1992, 10, 17–21. [Google Scholar] [CrossRef]
- Florjańczyk, M.; Tremblay, R. RMS width of pulses in nonlinear dispersive fibers: Pulses of arbitrary initial form with chirp. J. Light. Technol. 1995, 13, 1801–1806. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Karjanto, N. Bright Soliton Solution of the Nonlinear Schrödinger Equation: Fourier Spectrum and Fundamental Characteristics. Mathematics 2022, 10, 4559. https://doi.org/10.3390/math10234559
Karjanto N. Bright Soliton Solution of the Nonlinear Schrödinger Equation: Fourier Spectrum and Fundamental Characteristics. Mathematics. 2022; 10(23):4559. https://doi.org/10.3390/math10234559
Chicago/Turabian StyleKarjanto, Natanael. 2022. "Bright Soliton Solution of the Nonlinear Schrödinger Equation: Fourier Spectrum and Fundamental Characteristics" Mathematics 10, no. 23: 4559. https://doi.org/10.3390/math10234559
APA StyleKarjanto, N. (2022). Bright Soliton Solution of the Nonlinear Schrödinger Equation: Fourier Spectrum and Fundamental Characteristics. Mathematics, 10(23), 4559. https://doi.org/10.3390/math10234559






