The Classicality and Quantumness of the Driven Qubit–Photon–Magnon System
Abstract
1. Introduction
2. Physical Model
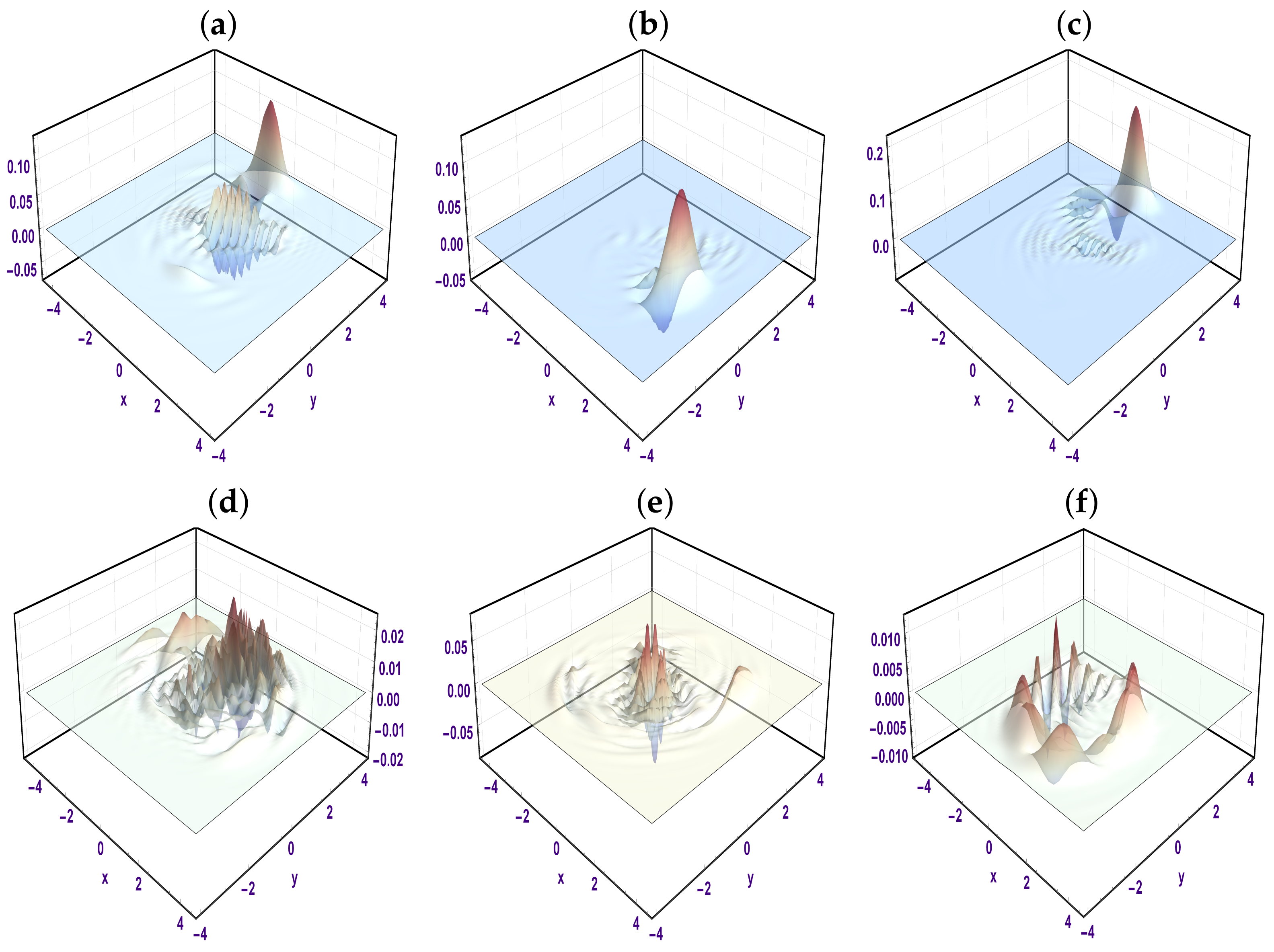
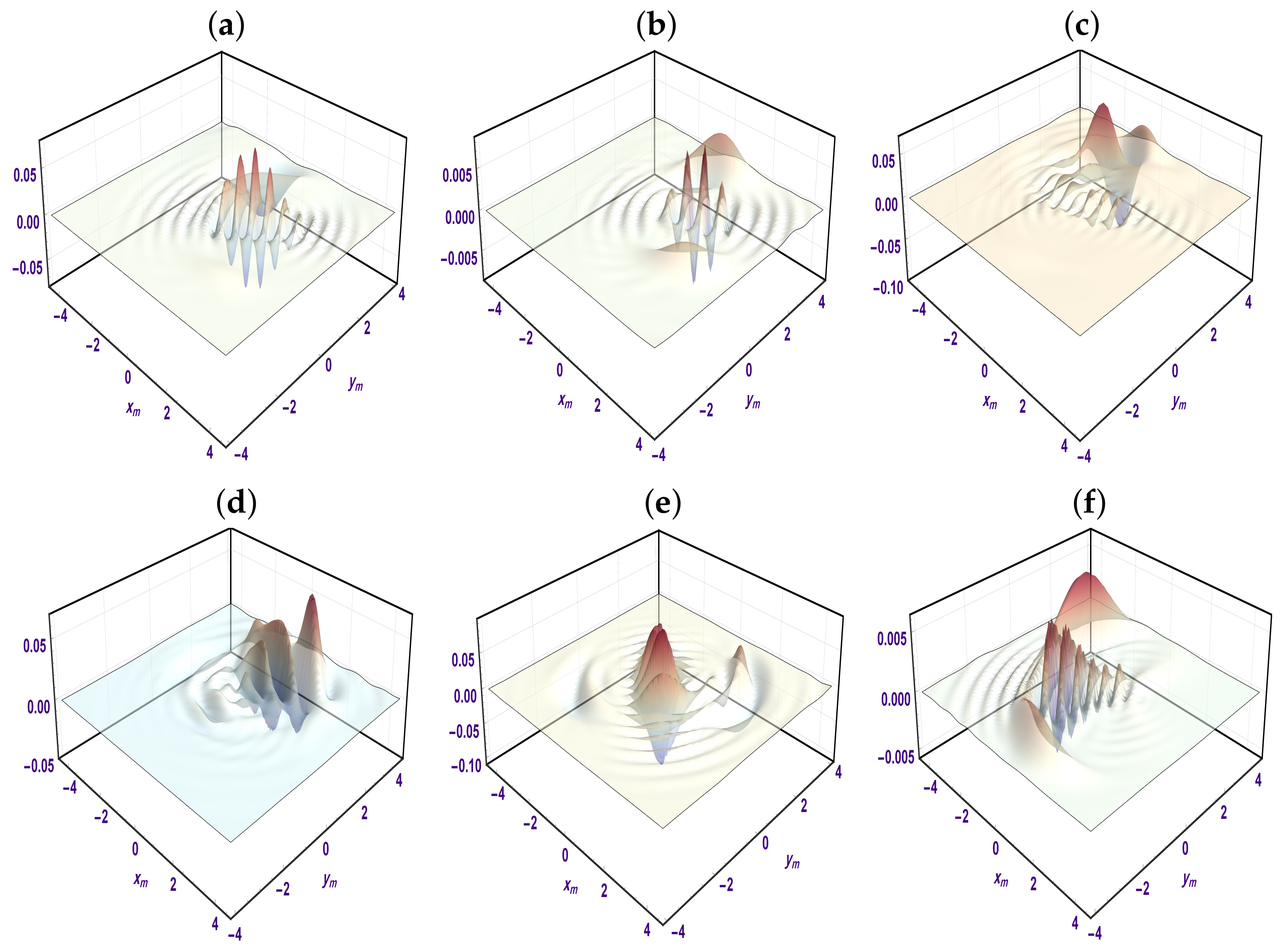
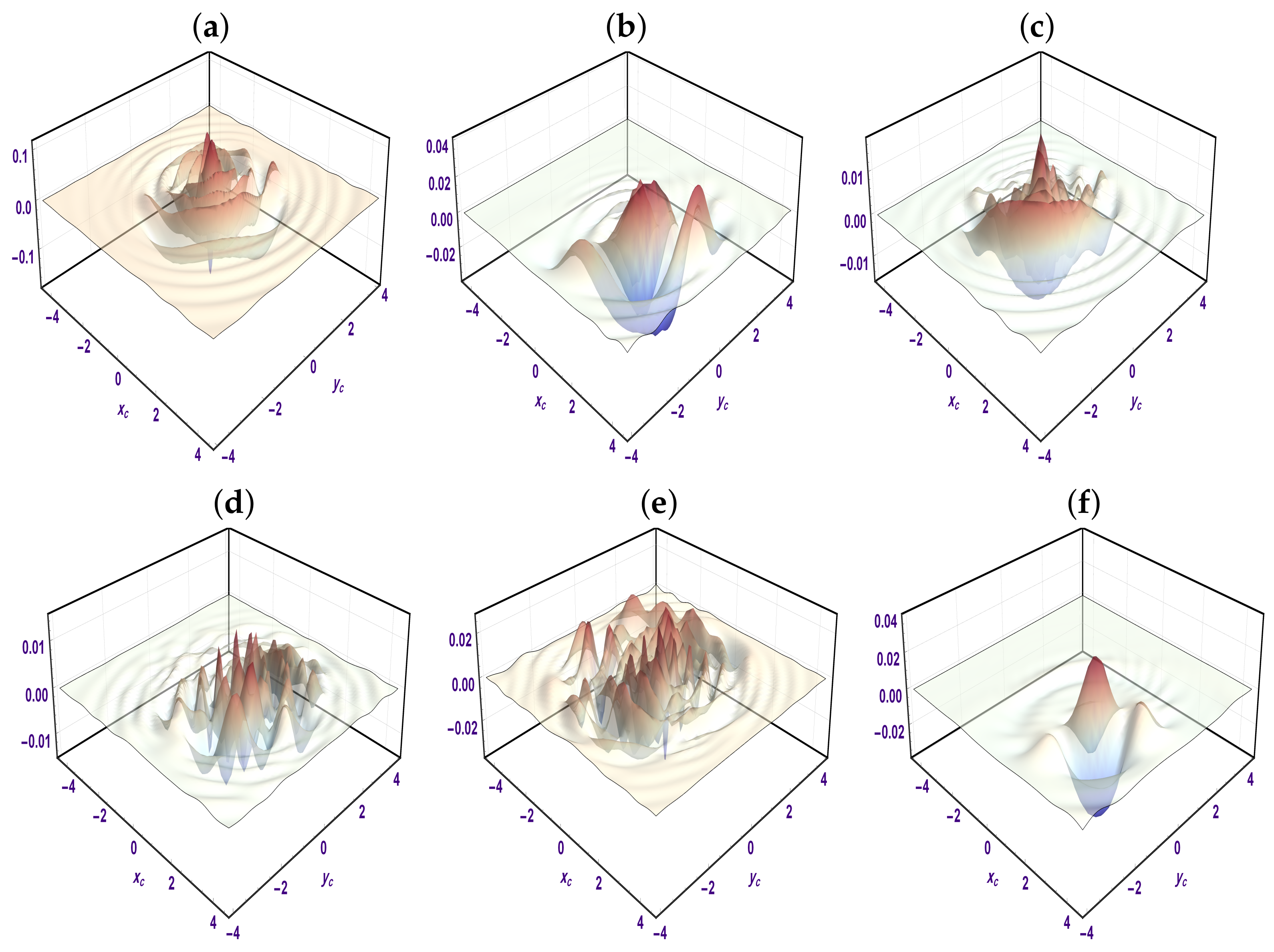
3. Wigner Function of a Two-Mode Field
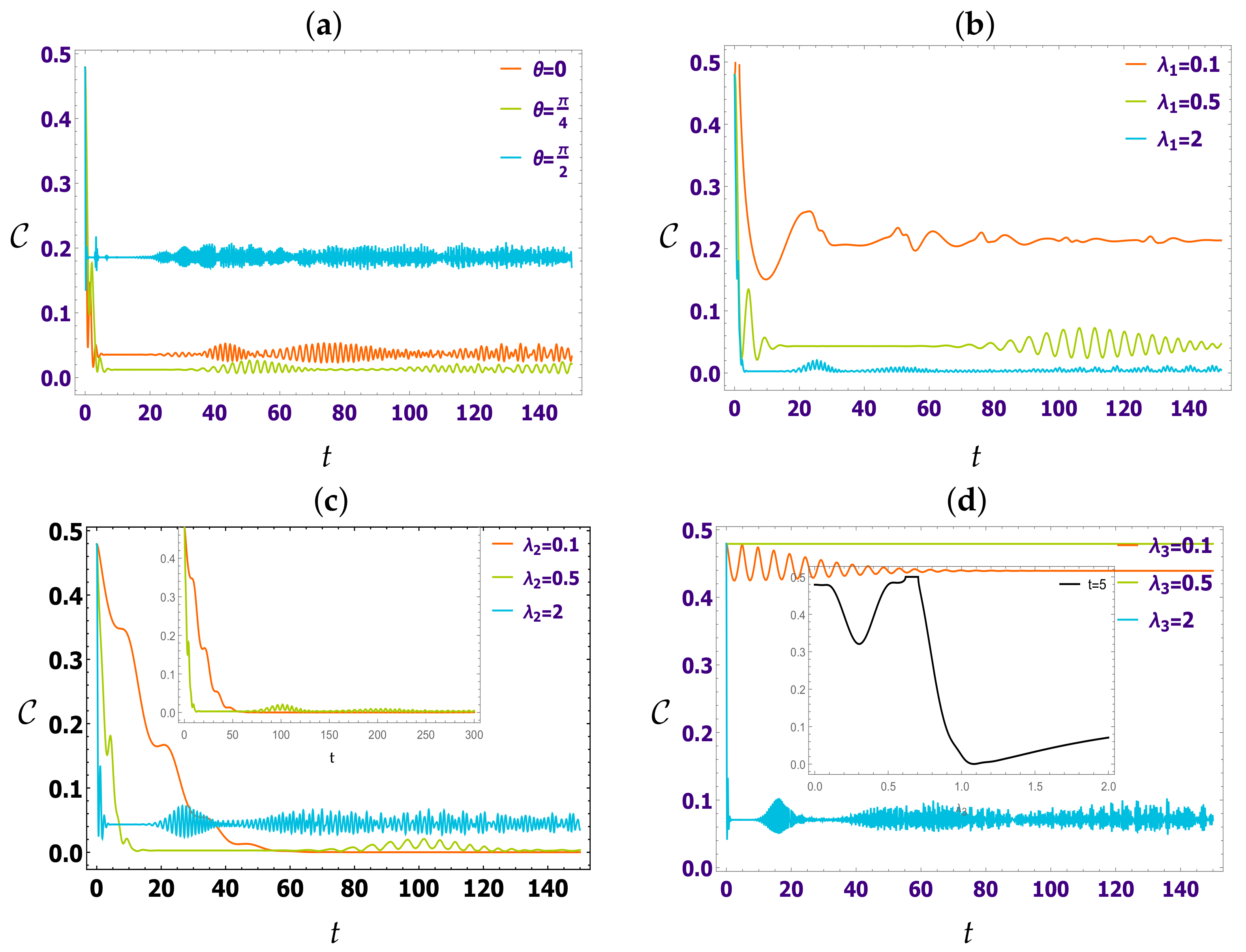
4. Non-Classicality in Terms of Skew Information
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ladd, T.D.; Jelezko, F.; Laflamme, R.; Nakamura, Y.; Monroe, C.; O’Brien, J.L. Quantum computers. Nature 2010, 464, 45–53. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.; Zhang, W.; Tyberkevych, V.; Kwok, W.K.; Hoffmann, A.; Novosad, V. Hybrid magnonics: Physics, circuits, and applications for coherent information processing. J. App. Phys. 2020, 128, 130902. [Google Scholar] [CrossRef]
- Makhlin, Y.; Schön, G.; Shnirman, A. Quantum-state engineering with Josephson-junction devices. Rev. Mod. Phys. 2001, 73, 357–400. [Google Scholar] [CrossRef]
- Degen, C.L.; Reinhard, F.; Cappellaro, P. Quantum sensing. Rev. Mod. Phys. 2017, 89, 035002. [Google Scholar] [CrossRef]
- Awschalom, D.D.; Du, C.; He, R.; Heremans, J.; Hoffmann, A.; Hou, J.; Kurebayashi, H.; Li, Y.; Liu, L.; Novosad, V.; et al. Quantum engineering with hybrid magnonics systems and materials. IEEE Trans. Quantum Eng. 2021, 2, 5500836. [Google Scholar] [CrossRef]
- Abouelregal, A.E.; Marin, M. The size-dependent thermoelastic vibrations of nanobeams subjected to harmonic excitation and rectified sine wave heating. Mathematics 2020, 8, 1128. [Google Scholar] [CrossRef]
- Abouelregal, A.E.; Marin, M. The response of nanobeams with temperature-dependent properties using state-space method via modified couple stress theory. Symmetry 2020, 12, 1276. [Google Scholar] [CrossRef]
- Zhang, X.; Zou, C.L.; Jiang, L.; Tang, H.X. Strongly Coupled Magnons and Cavity Microwave Photons. Phys. Rev. Lett. 2014, 113, 156401. [Google Scholar] [CrossRef]
- Li, J.; Zhu, S.Y.; Agarwal, G.S. Magnon-Photon-Phonon Entanglement in Cavity Magnomechanics. Phys. Rev. Lett. 2018, 121, 203601. [Google Scholar] [CrossRef]
- Zhang, L.; Bhatti, M.; Michaelides, E.E.; Marin, M.; Ellahi, R. Hybrid nanofluid flow towards an elastic surface with tantalum and nickel nanoparticles, under the influence of an induced magnetic field. Eur. Phys. J. Spec. Top. 2022, 231, 521–533. [Google Scholar] [CrossRef]
- Tabuchi, Y.; Ishino, S.; Noguchi, A.; Ishikawa, T.; Yamazaki, R.; Usami, K.; Nakamura, Y. Coherent coupling between a ferromagnetic magnon and a superconducting qubit. Science 2015, 349, 405–408. [Google Scholar] [CrossRef]
- Zhao, C.; Li, X.; Chao, S.; Peng, R.; Li, C.; Zhou, L. Simultaneous blockade of a photon, phonon, and magnon induced by a two-level atom. Phys. Rev. A 2020, 101, 063838. [Google Scholar] [CrossRef]
- Lachance-Quirion, D.; Tabuchi, Y.; Gloppe, A.; Usami, K.; Nakamura, Y. Hybrid quantum systems based on magnonics. Appl. Phys. Express 2019, 12, 070101. [Google Scholar] [CrossRef]
- Qi, S.f.; Jing, J. Generation of Bell and Greenberger-Horne-Zeilinger states from a hybrid qubit-photon-magnon system. Phys. Rev. A 2022, 105, 022624. [Google Scholar] [CrossRef]
- Lachance-Quirion, D.; Wolski, S.P.; Tabuchi, Y.; Kono, S.; Usami, K.; Nakamura, Y. Entanglement-based single-shot detection of a single magnon with a superconducting qubit. Science 2020, 367, 425–428. [Google Scholar] [CrossRef]
- Kong, D.; Hu, X.; Hu, L.; Xu, J. Magnon-atom interaction via dispersive cavities: Magnon entanglement. Phys. Rev. B 2021, 103, 224416. [Google Scholar] [CrossRef]
- Solano, E.; Agarwal, G.S.; Walther, H. Strong-Driving-Assisted Multipartite Entanglement in Cavity QED. Phys. Rev. Lett. 2003, 90, 027903. [Google Scholar] [CrossRef]
- Abd-Rabbou, M.; Khalil, E.; Ahmed, M.; Obada, A.S. External classical field and damping effects on a moving two level atom in a cavity field interaction with Kerr-like medium. Int. J. Theor. Phys. 2019, 58, 4012–4024. [Google Scholar] [CrossRef]
- Al Naim, A.F.; Khan, J.Y.; Abdel-Khalek, S.; Khalil, E.M. Entanglement and physical attributes of the interaction between two SC-qubits and thermal field in the presence of a magnetic field. Microelectron. J. 2019, 86, 15–21. [Google Scholar] [CrossRef]
- Alotaibi, M.F.; Khalil, E.; Abdel-Khalek, S.; Abd-Rabbou, M.; Omri, M. Effects of the vibrating graphene membrane and the driven classical field on an atomic system coupled to a cavity field. Results Phys. 2021, 31, 105012. [Google Scholar] [CrossRef]
- Sánchez Muñoz, C.; Frisk Kockum, A.; Miranowicz, A.; Nori, F. Simulating ultrastrong-coupling processes breaking parity conservation in Jaynes–Cummings systems. Phys. Rev. A 2020, 102, 033716. [Google Scholar] [CrossRef]
- Zhao, T.; Wu, S.p.; Yang, G.q.; Huang, G.m.; Li, G.X. Ultranarrow spectral line of the radiation in double qubit-cavity ultrastrong coupling system. Opt. Express 2021, 29, 23939–23952. [Google Scholar] [CrossRef] [PubMed]
- Einstein, A.; Podolsky, B.; Rosen, N. Can Quantum-Mechanical Description of Physical Reality Be Considered Complete? Phys. Rev. 1935, 47, 777–780. [Google Scholar] [CrossRef]
- Luo, S.; Li, N.; Fu, S. Quantumness of quantum ensembles. Theor. Math. Phys. 2011, 169, 1724–1739. [Google Scholar] [CrossRef]
- Mari, A.; Kieling, K.; Nielsen, B.M.; Polzik, E.S.; Eisert, J. Directly Estimating Nonclassicality. Phys. Rev. Lett. 2011, 106, 010403. [Google Scholar] [CrossRef]
- Abd-Rabbou, M.Y.; Metwally, N.; Ahmed, M.; Obada, A.S. Wigner function of noisy accelerated two-qubit system. Quantum Inf. Process. 2019, 18, 367. [Google Scholar] [CrossRef]
- Horodecki, R.; Horodecki, P.; Horodecki, M.; Horodecki, K. Quantum entanglement. Rev. Mod. Phys. 2009, 81, 865–942. [Google Scholar] [CrossRef]
- Mohamed, A.B.; Metwally, N. Nonclassical features of two SC-qubit system interacting with a coherent SC-cavity. Phys. E 2018, 102, 1–7. [Google Scholar] [CrossRef]
- Hu, M.L.; Wang, X.M.; Fan, H. Hierarchy of the nonlocal advantage of quantum coherence and Bell nonlocality. Phys. Rev. A 2018, 98, 032317. [Google Scholar] [CrossRef]
- Genovese, M.; Gramegna, M. Quantum correlations and quantum non-locality: A review and a few new ideas. Appl. Sci. 2019, 9, 5406. [Google Scholar] [CrossRef]
- Ollivier, H.; Zurek, W.H. Quantum Discord: A Measure of the Quantumness of Correlations. Phys. Rev. Lett. 2001, 88, 017901. [Google Scholar] [CrossRef]
- Metwally, N.; Abdel-Aty, M. Quantum deficits and correlations of two superconducting charge qubits. Phys. C 2009, 469, 111–115. [Google Scholar] [CrossRef]
- Bang-Fu, D.; Xiao-Yun, W.; He-Ping, Z. Quantum and classical correlations for a two-qubit X structure density matrix. Chin. Phys. B 2011, 20, 100302. [Google Scholar]
- Gårding, E.R.; Schwaller, N.; Chan, C.L.; Chang, S.Y.; Bosch, S.; Gessler, F.; Laborde, W.R.; Hernandez, J.N.; Si, X.; Dupertuis, M.A.; et al. Bell Diagonal and Werner state generation: Entanglement, non-locality, steering and discord on the IBM quantum computer. Entropy 2021, 23, 797. [Google Scholar] [CrossRef]
- Dai, H.; Luo, S. Information-theoretic approach to atomic spin nonclassicality. Phys. Rev. A 2019, 100, 062114. [Google Scholar] [CrossRef]
- Ghorbani, M.; Faghihi, M.J.; Safari, H. Wigner function and entanglement dynamics of a two-atom two-mode nonlinear Jaynes–Cummings model. JOSA B 2017, 34, 1884–1893. [Google Scholar] [CrossRef]
- Luo, S.; Zhang, Y. Quantifying nonclassicality via Wigner-Yanase skew information. Phys. Rev. A 2019, 100, 032116. [Google Scholar] [CrossRef]
- Dai, H.; Fu, S.; Luo, S. Atomic nonclassicality in the Jaynes-Cummings model. Phys. Lett. A 2020, 384, 126371. [Google Scholar] [CrossRef]
- Abd-Rabbou, M.; Metwally, N.; Ahmed, M.; Obada, A.S. Wigner distribution of accelerated tripartitew-state. Optik 2020, 208, 163921. [Google Scholar] [CrossRef]
- Chen, Z. Wigner-Yanase skew information as tests for quantum entanglement. Phys. Rev. A 2005, 71, 052302. [Google Scholar] [CrossRef]
- Yu, C.s. Quantum coherence via skew information and its polygamy. Phys. Rev. A 2017, 95, 042337. [Google Scholar] [CrossRef]
- Li, L.; Wang, Q.W.; Shen, S.Q.; Li, M. Measurement-induced nonlocality based on Wigner-Yanase skew information. EPL Europhys. Lett. 2016, 114, 10007. [Google Scholar] [CrossRef]
- Abdalla, M.S.; Khalil, E.; Obada, A.F. Exact treatment of the Jaynes–Cummings model under the action of an external classical field. Ann. Phys. 2011, 326, 2486–2498. [Google Scholar] [CrossRef]
- James, D.; Jerke, J. Effective Hamiltonian theory and its applications in quantum information. Can. J. Phys. 2007, 85, 625–632. [Google Scholar] [CrossRef]
- Wigner, E.P.; Yanase, M.M. Information contents of distributions. Proc. Natl. Acad. Sci. USA 1963, 49, 910–918. [Google Scholar] [CrossRef]
- Moya-Cessa, H. Decoherence in atom–field interactions: A treatment using superoperator techniques. Phys. Rep. 2006, 432, 1–41. [Google Scholar] [CrossRef]
- Jaynes, E.T.; Cummings, F.W. Comparison of quantum and semiclassical radiation theories with application to the beam maser. Proc. IEEE 1963, 51, 89–109. [Google Scholar] [CrossRef]
- Fran ça Santos, M.; Solano, E.; de Matos Filho, R.L. Conditional Large Fock State Preparation and Field State Reconstruction in Cavity QED. Phys. Rev. Lett. 2001, 87, 093601. [Google Scholar] [CrossRef]
- Luo, S. Wigner-Yanase Skew Information and Uncertainty Relations. Phys. Rev. Lett. 2003, 91, 180403. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alotaibi, M.F.; Khalil, E.M.; Abd-Rabbou, M.Y.; Marin, M. The Classicality and Quantumness of the Driven Qubit–Photon–Magnon System. Mathematics 2022, 10, 4458. https://doi.org/10.3390/math10234458
Alotaibi MF, Khalil EM, Abd-Rabbou MY, Marin M. The Classicality and Quantumness of the Driven Qubit–Photon–Magnon System. Mathematics. 2022; 10(23):4458. https://doi.org/10.3390/math10234458
Chicago/Turabian StyleAlotaibi, Maged Faihan, Eied Mahmoud Khalil, Mahmoud Youssef Abd-Rabbou, and Marin Marin. 2022. "The Classicality and Quantumness of the Driven Qubit–Photon–Magnon System" Mathematics 10, no. 23: 4458. https://doi.org/10.3390/math10234458
APA StyleAlotaibi, M. F., Khalil, E. M., Abd-Rabbou, M. Y., & Marin, M. (2022). The Classicality and Quantumness of the Driven Qubit–Photon–Magnon System. Mathematics, 10(23), 4458. https://doi.org/10.3390/math10234458







