On the BiHom-Type Nonlinear Equations
Abstract
1. Introduction
2. Preliminaries
2.1. BiHom-Hopf Algebras
- (1)
- If is an associative algebra, are both algebra isomorphisms, then is a BiHom-algebra.
- (2)
- If , then A becomes a Hom-algebra.
- (1)
- If is a coassociative coalgebra, are both coalgebra isomorphisms, then is a BiHom-coalgebra.
- (2)
- If , then C becomes a Hom-coalgebra.
- (1)
- If is a bialgebra, are all bialgebra isomorphisms, then is a BiHom-bialgebra.
- (2)
- If is a finite dimensional BiHom-bialgebra, . Define the multiplication ⋆, the comultiplication (with notation ) and byDefine byThen, is a BiHom-bialgebra.
- (3)
- If , then H becomes a Hom-bialgebra. If , then H becomes a monoidal Hom-bialgebra.
- (1)
- If is a Hopf algebra, are all Hoppf algebra isomorphisms and satisfying , then is a BiHom-Hopf algebra.
- (2)
- If is a BiHom-Hopf algebra, under the consideration of Example 3 (2), we immediately obtain that , ⋆, ε, , , , , and , , ε, , , , , ( are all BiHom-Hopf algebras.
- (3)
- If and , then H becomes the so-called monoidal BiHom-Hopf algebra (see [10], Definition 6.4). If , then H becomes the usual Hom–Hopf algebra. Similarly, if , then H becomes the usual monoidal Hom–Hopf algebra.
2.2. BiHom-Modules and BiHom-Comodules of a BiHom-Bialgebra
- (1)
- Obviously, .
- (2)
- The definition of right BiHom-module of H can be defined in a similar way.
- (3)
- For any integers , , forms a monoidal category under the following structures:
- the tensor product of and is , , where the H-action on is given by
- the unit object is with the trivial module action;
- for any , the the associativity and the unit constraints are given by
- (1)
- Obviously, .
- (2)
- The definition of left BiHom-comodule of H can be defined in a similar way.
- (3)
- For any integers , forms a monoidal category under the following structures:
- the tensor product of H-BiHom-comodules and is with the H-coaction :
- the unit object is with the trivial coaction;
- the associativity constraint and the unit constraint and are given by
3. The BiHom-Type Heisenberg Doubles and the BiHom-Hopf Equation
3.1. BiHom-Hopf Equation and BiHom-Pentagon Equation
- (1)
- is a solution of the BiHom-Hopf equation and the BiHom-pentagon equation.
- (2)
- For any , is a solution of the BiHom-Hopf equation if and only if and , is a solution of the BiHom-Hopf equation if and only if and .
- (3)
- If , then A is the usual algebra, and the solution of the BiHom-Hopf equation becomes the solution of usual Hopf equation, the solution of the BiHom-pentagon equation becomes the solution of usual pentagon equation (see [[16], Definition 11] for details).
- (1)
- If is a solution of the BiHom-Hopf equation, then is a solution of the BiHom-pentagon equation.
- (2)
- If is invertible, then is a solution of the BiHom-Hopf equation if and only if is a solution of the BiHom-pentagon equation.
- (1)
- Self evident.
- (2)
- Note that and are inverse with each other, and are inverse with each other, where means the inverse of . Hence, the conclusion holds.
3.2. Heisenberg Doubles of a BiHom-Hopf Algebra
4. The BiHom-Long Dimodules and the BiHom- Equation
4.1. The Parametric Generalized BiHom-Long Dimodules
- (1)
- For any , define the left H-action ⇀ on byand define the right B-coaction on bythen it is straightforward to check that is a generalized BiHom-Long dimodule.
- (2)
- Similarly, for , if we define the left H-action ⇁ on byand define the right B-coaction on bythen, it is straightforward to check that is also an object in .
- the monoidal product of of is , where the BiHom-module and BiHom-comodule structures are given by
- the unit object is with the trivial H-action and trivial B-coaction.
4.2. BiHom-Type Yang-Baxter Equation
- is given by
- is given bythen is a braiding in with the inverse if and only if is a quasitriangular structure of H with the inverse element .
- (1)
- If σ is a coquasitriangular form of H, then for any , we have
- (2)
- If H is a BiHom-Hopf algebra with bijective antipode S, and σ is a coquasitriangular form of H, then, for any , we have
4.3. Generalized -Equation
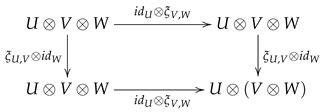

5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hartwig, J.; Larsson, D.; Silvestrov, S. Deformation of Lie algebras using σ-derivations. J. Algebra 2006, 295, 314–361. [Google Scholar] [CrossRef]
- Hu, N.H. q-Witt algebras, q-Lie algebras, q-holomorph structure and representations. Algebra Colloq. 1999, 6, 51–70. [Google Scholar]
- Makhlouf, A.; Panaite, F. Yetter-Drinfeld modules for Hom-bialgebras. J. Math. Phys. 2014, 55, 013501. [Google Scholar] [CrossRef]
- Makhlouf, A.; Silvestrov, S. Hom-algebras structures. J. Gen. Lie Theory Appl. 2008, 2, 51–64. [Google Scholar] [CrossRef]
- Makhlouf, A.; Silvestrov, S. Hom-Lie admissible Hom-coalgebras and Hom–Hopf algebras. In Generalized Lie theory in Mathematics, Physics and Beyond; Springer: Berlin, Germany, 2008; Chapter 17; pp. 189–206. [Google Scholar]
- Yau, D. Hom-quantum groups I: Quasitriangular Hom-bialgebras. J. Phys. A 2012, 45, 065203. [Google Scholar] [CrossRef][Green Version]
- Yau, D. Hom-Yang-Baxter equation, Hom-Lie algebras and quasitriangular bialgebras. J. Phys. A 2009, 42, 165202. [Google Scholar] [CrossRef]
- Zhang, X.H.; Dong, L.H. Braided mixed datums and their applications on Hom-quantum groups. Glasgow Math. J. 2018, 60, 231–251. [Google Scholar] [CrossRef]
- Caenepeel, S.; Goyvaerts, I. Monoidal Hom–Hopf algebras. Comm. Algebra 2011, 39, 2216–2240. [Google Scholar] [CrossRef]
- Graziani, G.; Makhlouf, A.; Menini, C.; Panaite, F. BiHom-associative algebras, BiHom-Lie algebras and BiHom-bialgebras. SIGMA 2015, 11, 086. [Google Scholar] [CrossRef]
- Fang, X.L.; Liu, W. Solutions of the BiHom–Yang-Baxter equations. Mat. Sb. 2018, 209, 128–145, translation in Sb. Math. 2018, 209, 901–918. [Google Scholar] [CrossRef]
- Guo, S.J.; Zhang, X.H.; Wang, S.X. The construction and deformation of BiHom-Novikov algebras. J. Geom. Phys. 2018, 132, 460–472. [Google Scholar] [CrossRef]
- Li, J.; Chen, L.Y.; Sun, B. Bihom-Nijienhuis operators and T*-extensions of Bihom-Lie superalgebras. Hacet. J. Math. Stat. 2019, 48, 785–799. [Google Scholar] [CrossRef]
- Liu, L.; Makhlouf, A.; Menini, C.; Panaite, F. σ,τ-Rota-Baxter operators, infinitesimal Hom-bialgebras and the associative (Bi)Hom-Yang-Baxter equation. Canad. Math. Bull. 2019, 62, 355–372. [Google Scholar] [CrossRef]
- Zhang, X.H.; Wang, D.G. Cotwists of bicomonads and BiHom-bialgebras. Algebra Represent. Theor. 2020, 23, 1355–1385. [Google Scholar] [CrossRef]
- Caenepeel, S.; Militaru, G.; Zhu, S.L. Frobenius and separable functors for generalized module categories and nonlinear equations. In Lecture Notes in Mathematics (1787); Springer: Berlin/Heidelberg, Germany, 2002. [Google Scholar]
- Militaru, G. The Long dimodules category and nonlinear equations. Algebra Represet. Theor. 1999, 2, 177–200. [Google Scholar] [CrossRef]
- Long, F. The Brauer group of dimodule algebras. J. Algebra 1974, 30, 559–601. [Google Scholar] [CrossRef]
- Ma, T.S.; Li, J. Nonhomogeneous associative Yang-Baxter equations. Bull. Math. Soc. SCI. Math. Roumanie (N.S.) 2022, 65, 97–118. [Google Scholar]
- Wang, S.X.; Zhang, X.H.; Guo, S.J. The Hom-Long dimodule category and nonlinear equations. Electron. Res. Arch. 2022, 30, 362–381. [Google Scholar] [CrossRef]
- Sweedler, M.E. Hopf Algebras; Benjamin: New York, NY, USA, 1969. [Google Scholar]
- Zhang, X.H.; Wang, W.; Chen, J.Z. Parametric representations of BiHom-Hopf algebras. 2022; Submitted. [Google Scholar]
- Majid, S. Foundations of Quantum Group Theory; Cambridge University Press: London, UK, 1995. [Google Scholar]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, H.; Zhang, X. On the BiHom-Type Nonlinear Equations. Mathematics 2022, 10, 4360. https://doi.org/10.3390/math10224360
Wu H, Zhang X. On the BiHom-Type Nonlinear Equations. Mathematics. 2022; 10(22):4360. https://doi.org/10.3390/math10224360
Chicago/Turabian StyleWu, Hui, and Xiaohui Zhang. 2022. "On the BiHom-Type Nonlinear Equations" Mathematics 10, no. 22: 4360. https://doi.org/10.3390/math10224360
APA StyleWu, H., & Zhang, X. (2022). On the BiHom-Type Nonlinear Equations. Mathematics, 10(22), 4360. https://doi.org/10.3390/math10224360




