A Correct Benchmark Problem of a Two-Dimensional Droplet Deformation in Simple Shear Flow
Abstract
1. Introduction
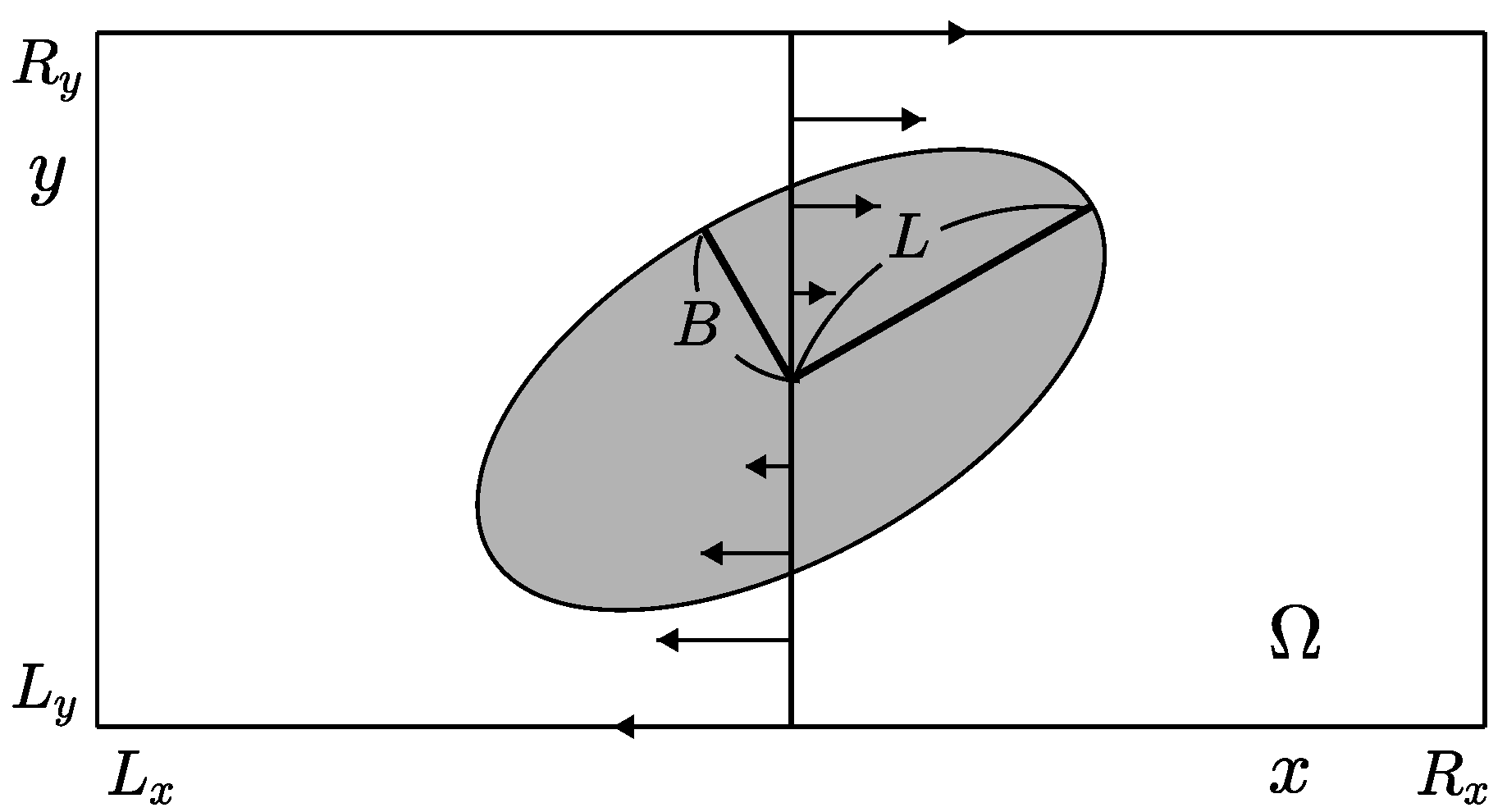
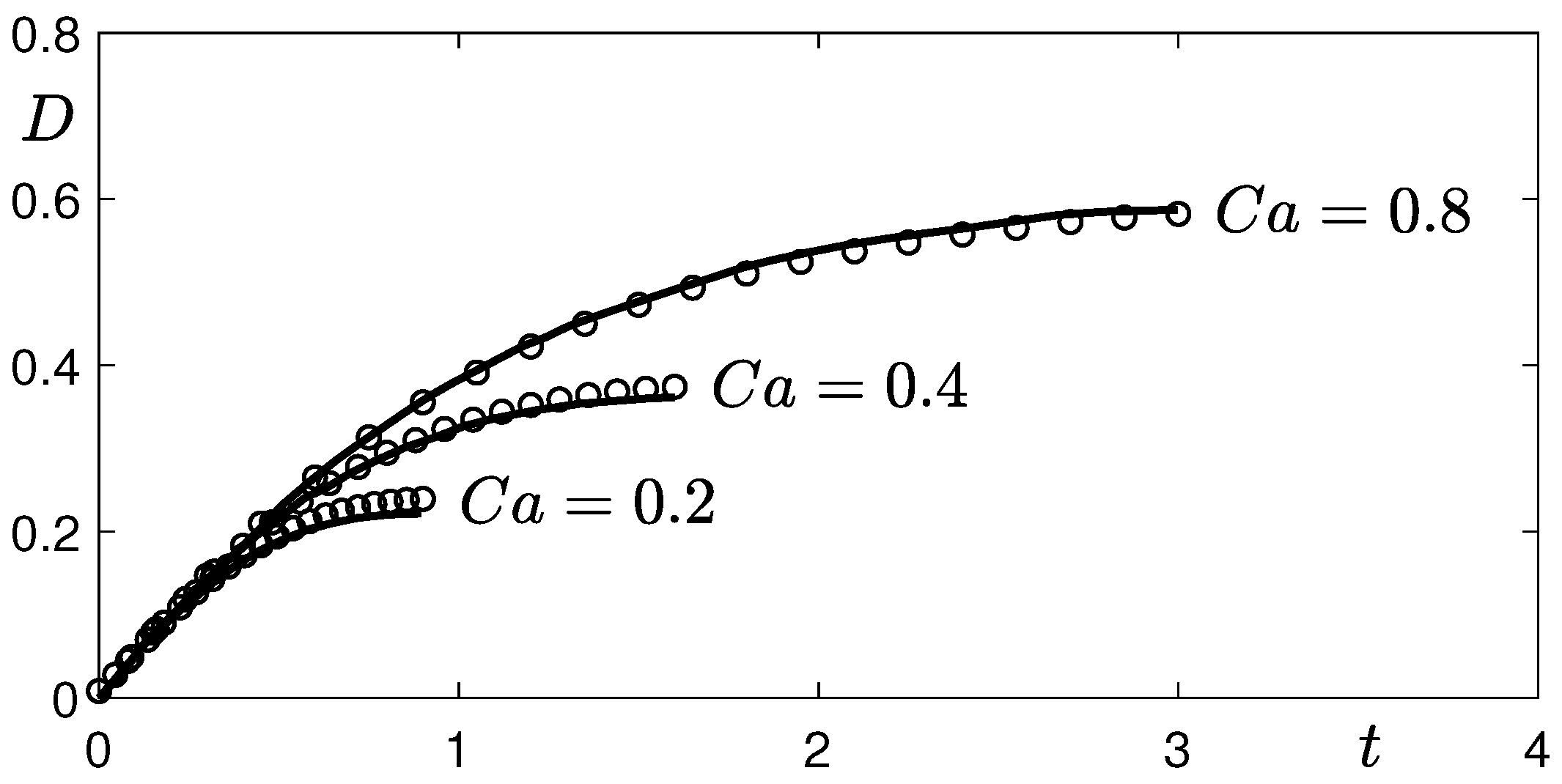
2. Governing Equations and Numerical Solution
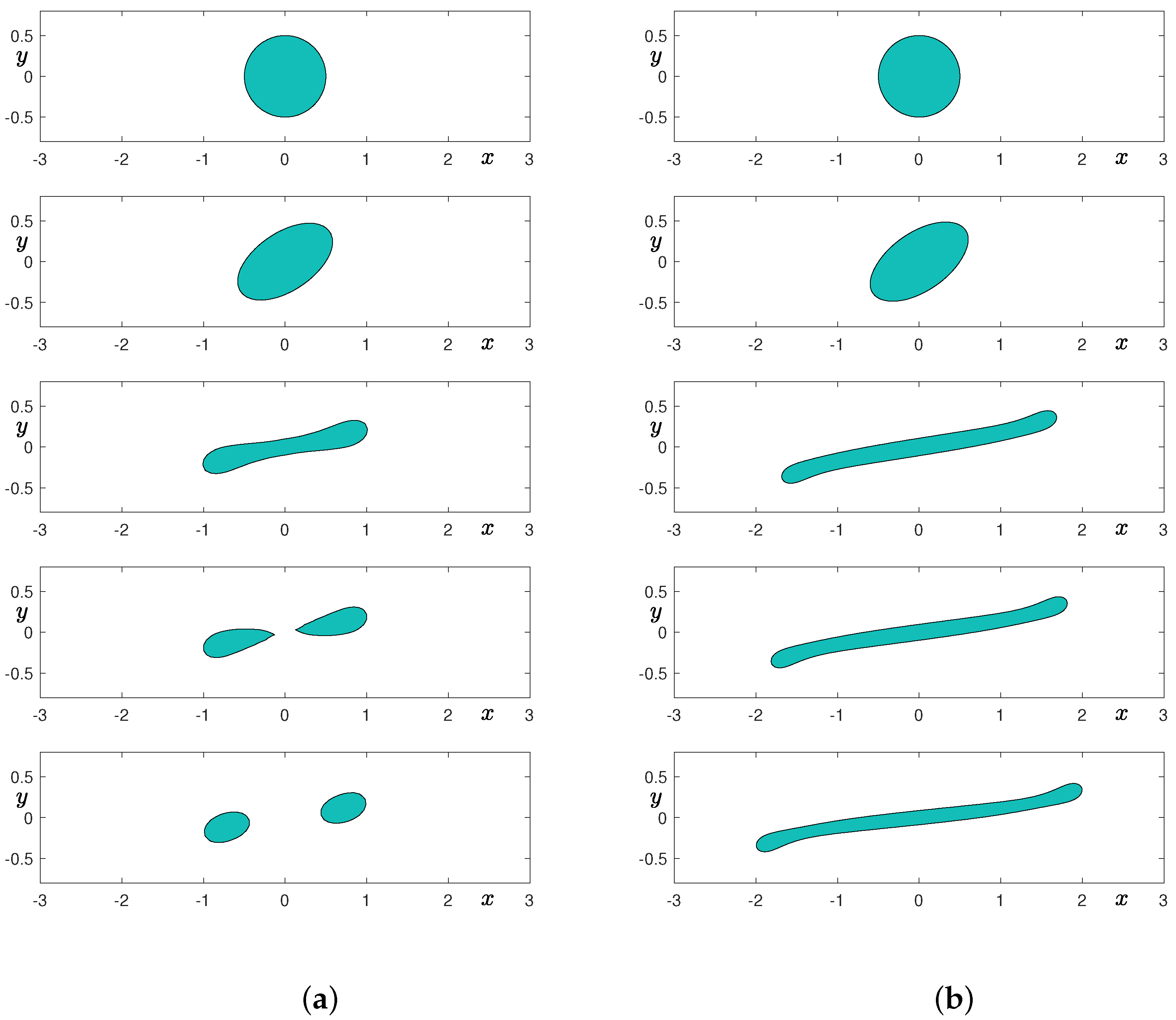
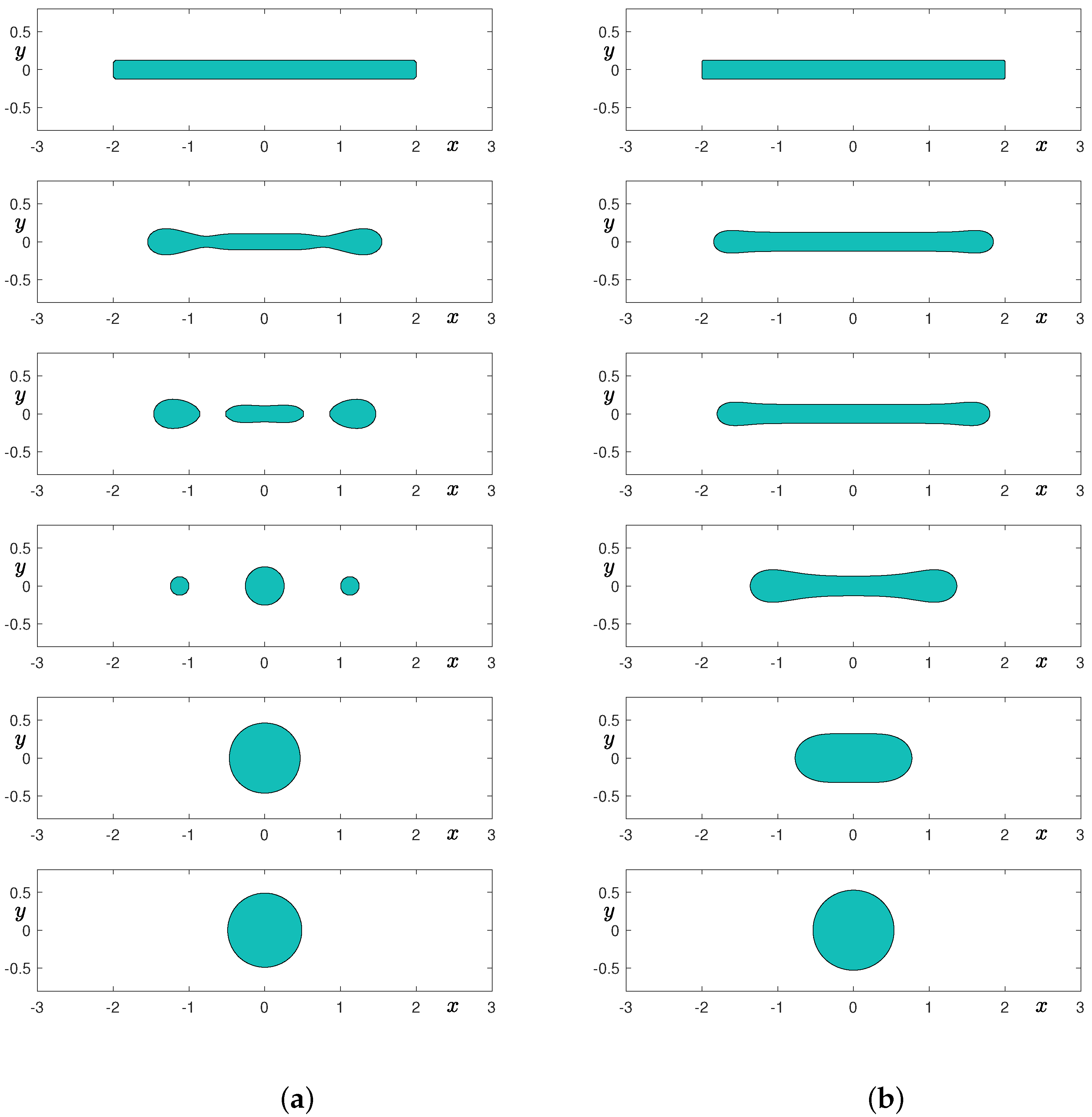
3. Numerical Experiments
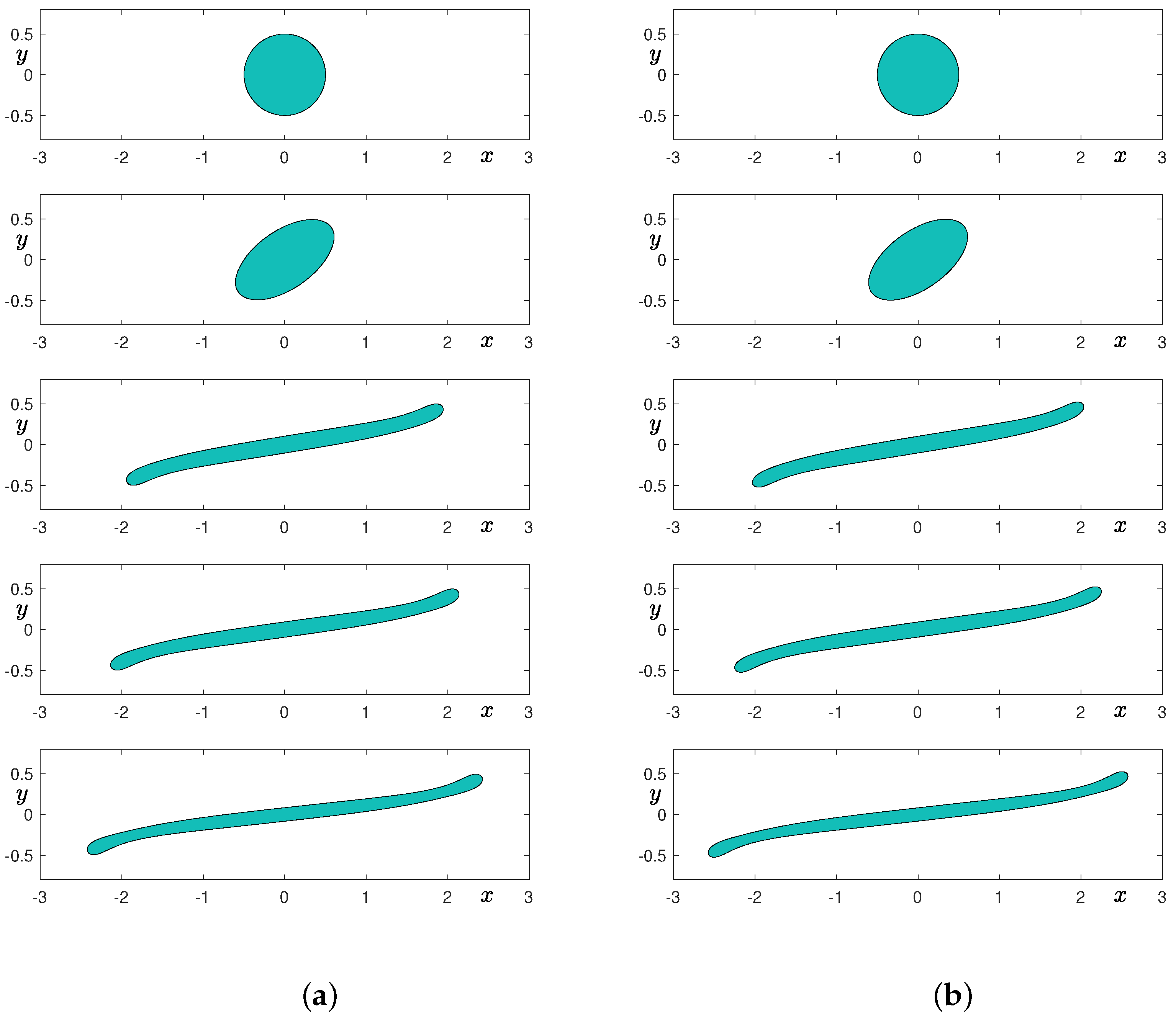
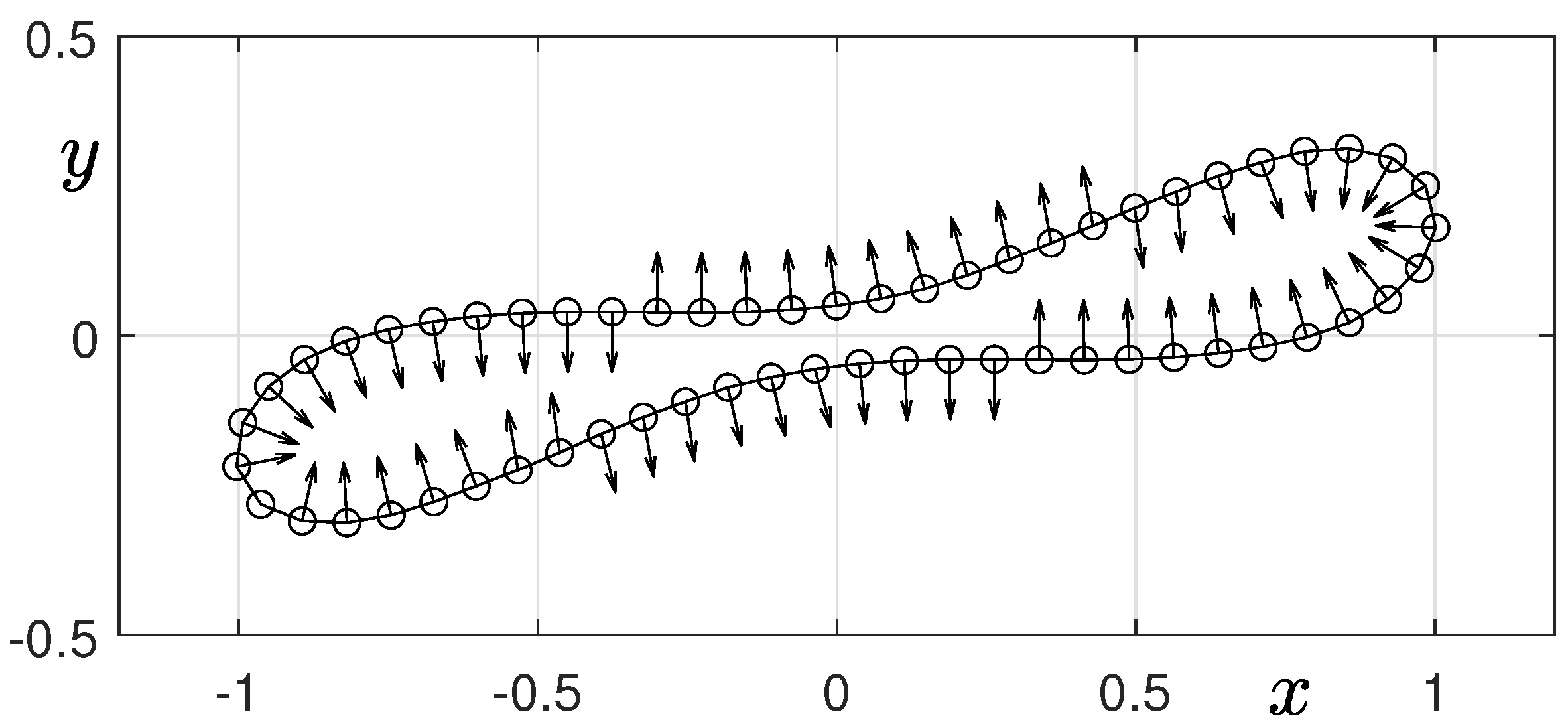
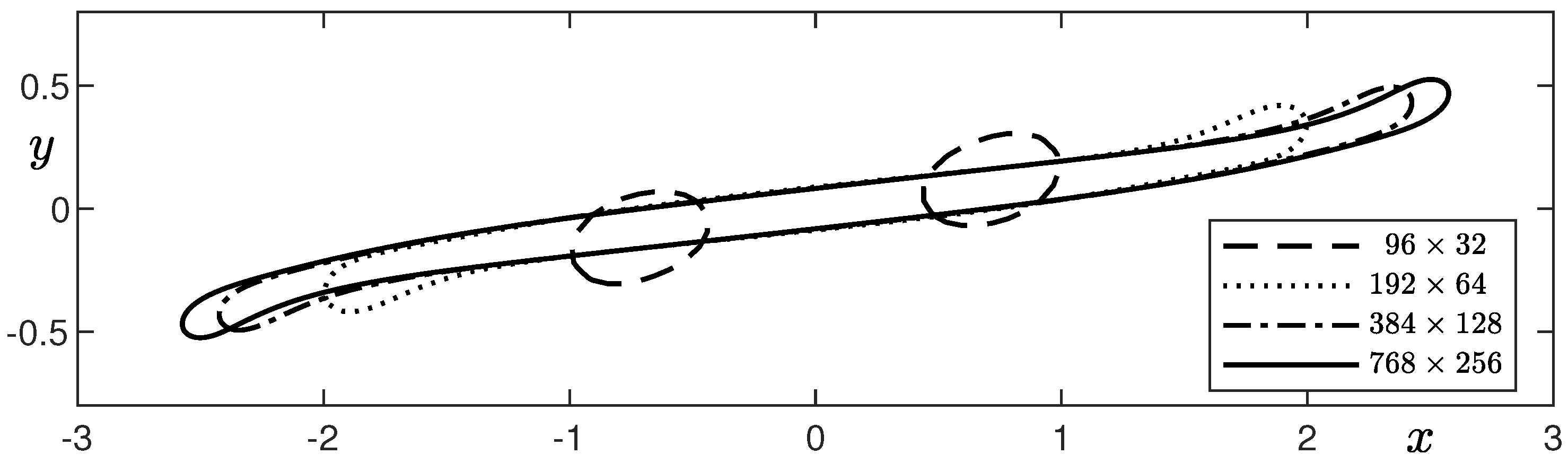
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Han, F. Cellular automata modeling of Ostwald ripening and Rayleigh instability. Materials 2018, 11, 1936. [Google Scholar] [CrossRef]
- Hao, X.; Zeng, Y. Simulation of Breakup Process of Polymer Jet during Melt Blowing. Fibers Polym. 2020, 21, 1222–1228. [Google Scholar] [CrossRef]
- Xie, L.; Yang, L.; Ye, H. Instability of gas-surrounded Rayleigh viscous jets: Weakly nonlinear analysis and numerical simulation. Phys. Fluids 2017, 29, 074101. [Google Scholar] [CrossRef]
- Amani, A.; Balcázar, N.; Castro, J.; Oliva, A. Numerical study of droplet deformation in shear flow using a conservative level-set method. Chem. Eng. Sci. 2019, 207, 153–171. [Google Scholar] [CrossRef]
- Komrakova, A.E.; Shardt, O.; Eskin, D.; Derksen, J.J. Lattice Boltzmann simulations of drop deformation and breakup in shear flow. Int. J. Multiph. Flow 2014, 59, 24–43. [Google Scholar] [CrossRef]
- Komrakova, A.E.; Shardt, O.; Eskin, D.; Derksen, J.J. Effects of dispersed phase viscosity on drop deformation and breakup in inertial shear flow. Chem. Eng. Sci. 2015, 126, 150–159. [Google Scholar] [CrossRef]
- Wang, N.; Liu, H.; Zhang, C. Deformation and breakup of a confined droplet in shear flows with power-law rheology. J. Rheol. 2017, 61, 741–758. [Google Scholar] [CrossRef]
- Liu, H.; Lu, Y.; Li, S.; Yu, Y.; Sahu, K.C. Deformation and breakup of a compound droplet in three-dimensional oscillatory shear flow. Int. J. Multiph. Flow 2021, 134, 103472. [Google Scholar] [CrossRef]
- Wang, Y.; Ye, Y. Energy conservation for weak solutions to the 3D Navier–Stokes–Cahn–Hilliard system. Appl. Math. Lett. 2022, 123, 107587. [Google Scholar] [CrossRef]
- Farokhirad, S.; Lee, T.; Morris, J.F. Effects of inertia and viscosity on single droplet deformation in confined shear flow. Commun. Comput. Phys. 2013, 13, 706–724. [Google Scholar] [CrossRef]
- Zhang, J.; Shu, S.; Guan, X.; Yang, N. Regime mapping of multiple breakup of droplets in shear flow by phase-field lattice Boltzmann simulation. Chem. Eng. Sci. 2021, 240, 116673. [Google Scholar] [CrossRef]
- Barai, N.; Mandal, N. Breakup modes of fluid drops in confined shear flows. Phys. Fluids 2016, 28, 073302. [Google Scholar] [CrossRef]
- Nazari, M.; Sani, H.M.; Kayhani, M.H.; Daghighi, Y. Different stages of liquid film growth in a microchannel: Two-phase Lattice Boltzmann study. Braz. J. Chem. Eng. 2018, 35, 977–994. [Google Scholar] [CrossRef]
- Adam, N.; Franke, F.; Aland, S. A Simple Parallel Solution Method for the Navier–Stokes Cahn–Hilliard Equations. Mathematics 2020, 8, 1224. [Google Scholar] [CrossRef]
- Bai, F.; Han, D.; He, X.M.; Yang, X. Deformation and coalescence of ferrodroplets in Rosensweig model using the phase field and modified level set approaches under uniform magnetic fields. Commun. Nonlinear Sci. Numer. Simul. 2020, 85, 105213. [Google Scholar] [CrossRef]
- Chen, Y.; Huang, Y.; Yi, N. Error analysis of a decoupled, linear and stable finite element method for Cahn–Hilliard–Navier–Stokes equations. Appl. Math. Comput. 2022, 421, 126928. [Google Scholar] [CrossRef]
- Chen, L.; Zhao, J. A novel second-order linear scheme for the Cahn–Hilliard–Navier–Stokes equations. J. Comput. Phys. 2020, 423, 109782. [Google Scholar] [CrossRef]
- Guo, Z.; Cheng, Q.; Lin, P.; Liu, C.; Lowengrub, J. Second order approximation for a quasi-incompressible Navier–Stokes Cahn–Hilliard system of two-phase flows with variable density. J. Comput. Phys. 2022, 448, 110727. [Google Scholar] [CrossRef]
- Dehghan, M.; Gharibi, Z. Numerical analysis of fully discrete energy stable weak Galerkin finite element Scheme for a coupled Cahn–Hilliard–Navier–Stokes phase-field model. Appl. Math. Comput. 2021, 410, 126487. [Google Scholar] [CrossRef]
- Li, M.; Xu, C. New efficient time-stepping schemes for the Navier–Stokes–Cahn–Hilliard equations. Comput. Fluids 2021, 231, 105174. [Google Scholar] [CrossRef]
- Liu, C.; Frank, F.; Thiele, C.; Alpak, F.O.; Berg, S.; Chapman, W.; Riviere, B. An efficient numerical algorithm for solving viscosity contrast Cahn–Hilliard–Navier–Stokes system in porous media. J. Comput. Phys. 2020, 400, 108948. [Google Scholar] [CrossRef]
- Sohaib, M.; Shah, A. Fully decoupled pressure projection scheme for the numerical solution of diffuse interface model of two-phase flow. Commun. Nonlinear Sci. Numer. Simul. 2022, 112, 106547. [Google Scholar] [CrossRef]
- Choi, J.W.; Lee, H.G.; Jeong, D.; Kim, J.S. An unconditionally gradient stable numerical method for solving the Allen–Cahn equation. Phys. A 2009, 388, 1791–1803. [Google Scholar] [CrossRef]
- Brackbill, J.U.; Kothe, D.B.; Zemach, C. A continuum method for modeling surface tension. J. Comput. Phys. 1992, 100, 335–354. [Google Scholar] [CrossRef]
- Kim, J.S. A continuous surface tension force formulation for diffuse-interface models. J. Comput. Phys. 2005, 204, 784–804. [Google Scholar] [CrossRef]
- Kim, J.S. Phase-Field Models for Multi-Component Fluid Flows. Comm. Comput. Phys. 2012, 12, 613–661. [Google Scholar] [CrossRef]
- Chorin, A.J. Numerical Solution of the Navier–Stokes Equations. Math. Comput. 1968, 22, 745–762. [Google Scholar] [CrossRef]
- Trottenberg, U.; Oosterlee, C.W.; Schüller, A. Multigrid; Academic Press: New York, NY, USA, 2001. [Google Scholar]
- Lee, C.; Jeong, D.; Yang, J.; Kim, J. Nonlinear multigrid implementation for the two-dimensional Cahn–Hilliard equation. Mathematics 2020, 8, 97. [Google Scholar] [CrossRef]
- Yoon, S.; Jeong, D.; Lee, C.; Kim, H.; Kim, S.; Lee, H.G.; Kim, J.S. Fourier-spectral method for the phase-field equations. Mathematics 2020, 8, 1385. [Google Scholar] [CrossRef]
- Chen, Y.; Liang, Y.; Chen, M. The deformation and breakup of a droplet under the combined influence of electric field and shear flow. Fluid Dyn. Res. 2021, 53, 065504. [Google Scholar] [CrossRef]
- Sheth, K.S.; Pozrikidis, C. Effects of inertia on the deformation of liquid drops in simple shear flow. Comput. Fluids 1995, 24, 101–119. [Google Scholar] [CrossRef]
- Li, Y.; Wang, J. Unconditional convergence analysis of stabilized FEM-SAV method for Cahn–Hilliard equation. Appl. Math. Comput. 2022, 419, 126880. [Google Scholar] [CrossRef]
- Lee, D.; Kim, J.S. Comparison study of the conservative Allen–Cahn and the Cahn–Hilliard equations. Math. Comput. Simul. 2016, 119, 35–56. [Google Scholar] [CrossRef]
- Cui, C.; Liu, J.; Mo, Y.; Zhai, S. An effective operator splitting scheme for two-dimensional conservative nonlocal Allen–Cahn equation. Appl. Math. Lett. 2022, 130, 108016. [Google Scholar] [CrossRef]
- Barosan, I.; Anderson, P.D.; Meijer, H.E.H. Application of mortar elements to diffuse-interface methods. Comput. Fluids 2006, 35, 1384–1399. [Google Scholar] [CrossRef]
- Liu, P.; Ouyang, Z.; Chen, C.; Yang, X. A novel fully-decoupled, linear, and unconditionally energy-stable scheme of the conserved Allen–Cahn phase-field model of a two-phase incompressible flow system with variable density and viscosity. Commun. Nonlinear Sci. Numer. Simul. 2022, 107, 106120. [Google Scholar] [CrossRef]
- Kwak, S.; Yang, J.; Kim, J.S. A conservative Allen–Cahn equation with a curvature-dependent Lagrange multiplier. Appl. Math. Lett. 2022, 126, 107838. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, J.; Li, Y.; Kim, J. A Correct Benchmark Problem of a Two-Dimensional Droplet Deformation in Simple Shear Flow. Mathematics 2022, 10, 4092. https://doi.org/10.3390/math10214092
Yang J, Li Y, Kim J. A Correct Benchmark Problem of a Two-Dimensional Droplet Deformation in Simple Shear Flow. Mathematics. 2022; 10(21):4092. https://doi.org/10.3390/math10214092
Chicago/Turabian StyleYang, Junxiang, Yibao Li, and Junseok Kim. 2022. "A Correct Benchmark Problem of a Two-Dimensional Droplet Deformation in Simple Shear Flow" Mathematics 10, no. 21: 4092. https://doi.org/10.3390/math10214092
APA StyleYang, J., Li, Y., & Kim, J. (2022). A Correct Benchmark Problem of a Two-Dimensional Droplet Deformation in Simple Shear Flow. Mathematics, 10(21), 4092. https://doi.org/10.3390/math10214092






