Theoretical and Numerical Study of Self-Organizing Processes in a Closed System Classical Oscillator and Random Environment
Abstract
1. Introduction
- The oscillator frequency is random and there is no external field;
- The oscillator frequency is random and the external field is a regular function;
- The frequency of the oscillator is a regular function and the external force is random.
2. Problem
2.1. Statement of the Problem
- When randomness in a JS generates a complex process , and the second source of the random process is absent , and, accordingly,
- when and randomness in a JS generates the generator , which has a complex character.
2.2. Derivation of Environmental Fields Distribution Equations
3. The Mathematical Expectation of the Trajectory
3.1. The Measure of the Functional Space
3.2. The Stages of the Expected Trajectory Calculation
4. Geometric and Topological Features of a Compactified Space
4.1. Geometry of Two-Dimensional Subspace
4.2. Topology of Two-Dimensional Subspace
5. Statement of the Initial-Boundary Value Problem for the Complex PDE
6. Entropy of a Self-Organizing System
7. Numerical Methods for Solving the Problem
- To calculate Equations (18) and (19), it is also necessary to set two boundary conditions in the form of difference equations on the coordinate axes and , respectively, which can be easily found by approximating Equation (87). Note that these difference equations must be solved taking into account the Dirichlet boundary conditions; , where the index denotes the first and second boundary conditions, respectively.
- The condition is set at the center of the coordinate axes:In addition, the Dirichlet condition is specified on the boundaries of the computational domain, where denotes the boundary.
- As an initial condition, instead of the Dirac delta function, we use the Gaussian distribution:Note that the parameters and included in the function were chosen in such a way to normalize the initial distribution to unit.
- The continuous region for the PDEs system (66) is replaced by a discrete grid, as described in Listing 1.
- Using the PDEs system (66), we can obtain the following system of difference equations on the constructed grid:where ; in addition, .
- Similarly, as in Listing 1, for the solutions and , boundary conditions are set on the and axes in the form of difference equations, which can be obtained by approximating Equation (88) on the same axes. Note that we solve each equation obtained for the boundary conditions as an internal Dirichlet problem with a zero value of the solution at the boundary.
- As in the case of the probability density (see Listing 1), the solutions and are subject to similar conditions at the center of the coordinate axes:In addition, we will assume that at the boundary of the computational domain, the solutions are subject to the following conditions:
- Finally, as an initial condition for solving the system of Equations (88) for both solutions and , Gaussian distribution with parameters as in Listing 1 is chosen.
7.1. Distributions of the Free Environmental Fields
- When the processes going on in the environment, both elastic and inelastic, are weak;
- When elastic processes are strong and inelastic processes are weak;
- When both elastic and inelastic processes are strong in the environment.
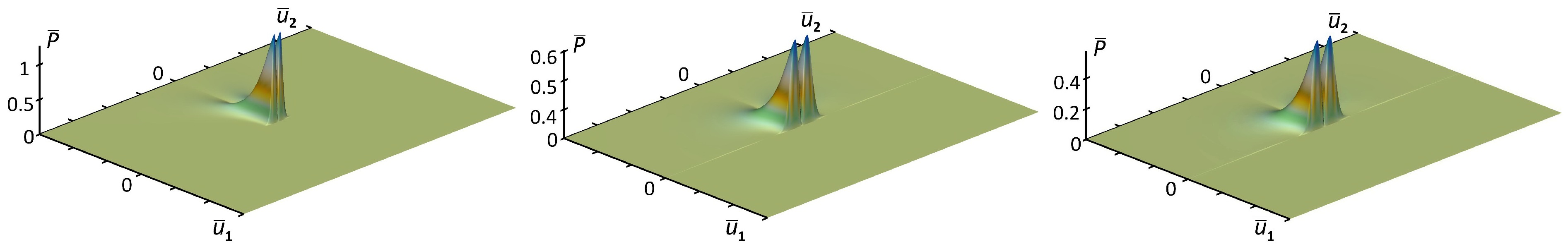
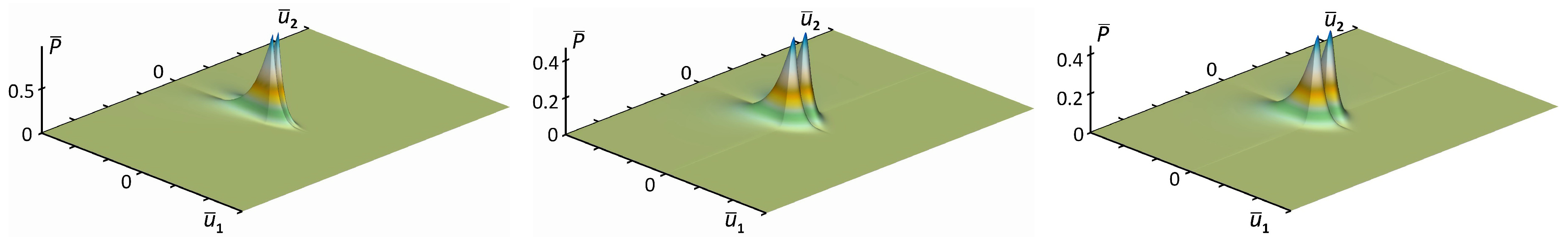
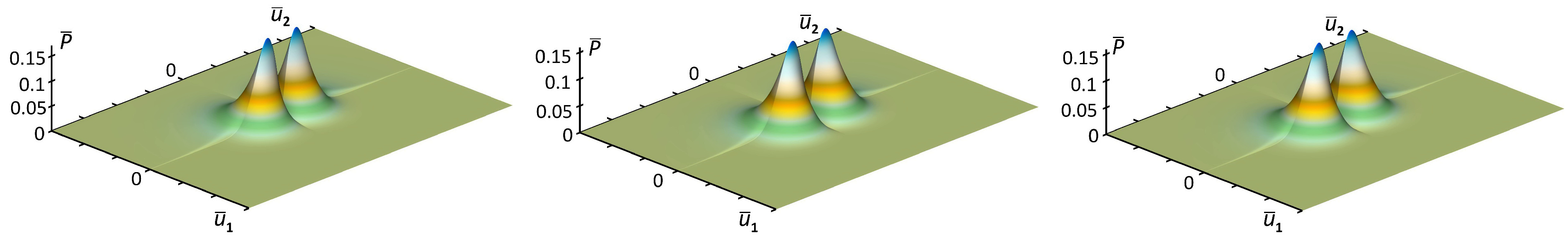
7.2. Distributions of Environmental Fields Taking into Account the Influence of the Oscillator
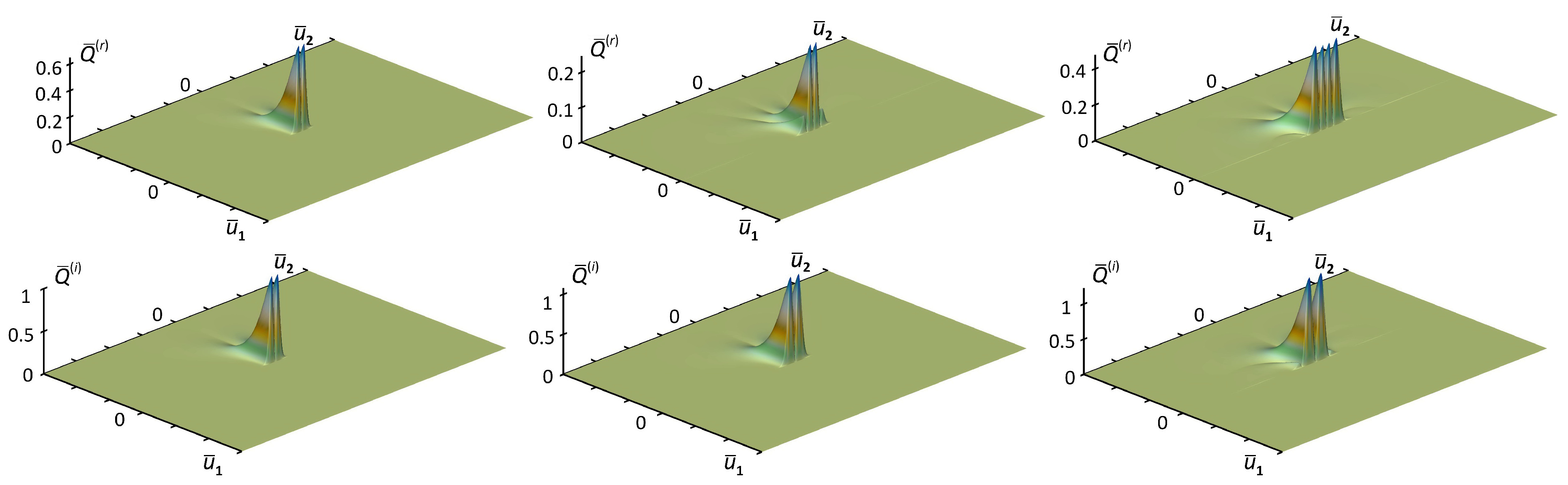
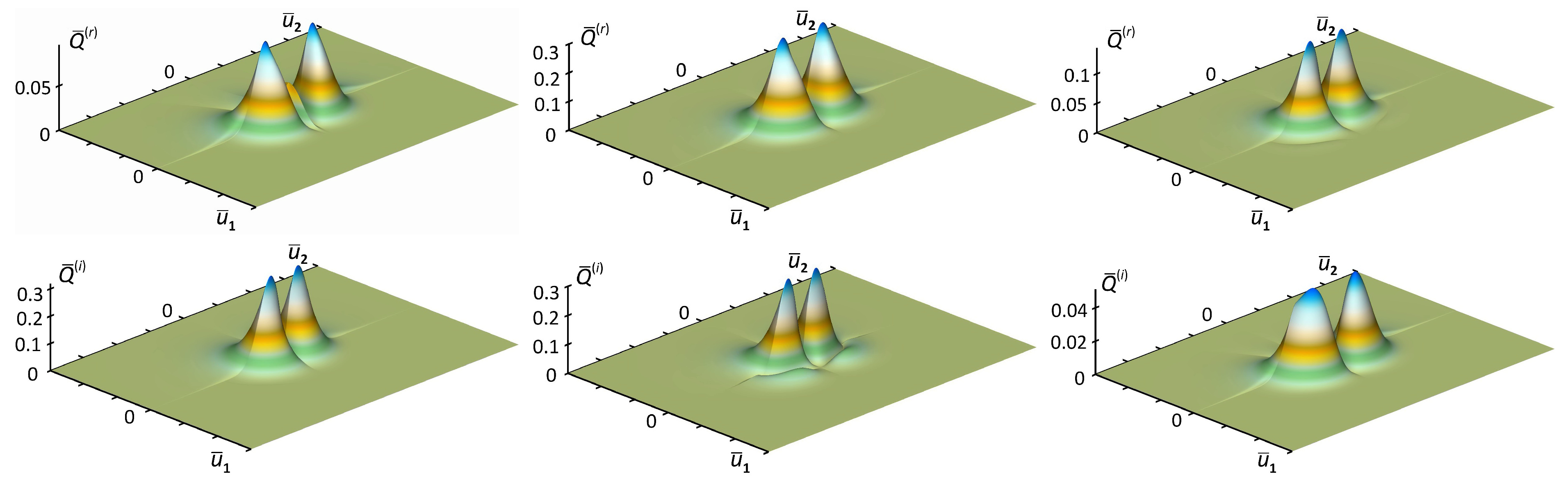
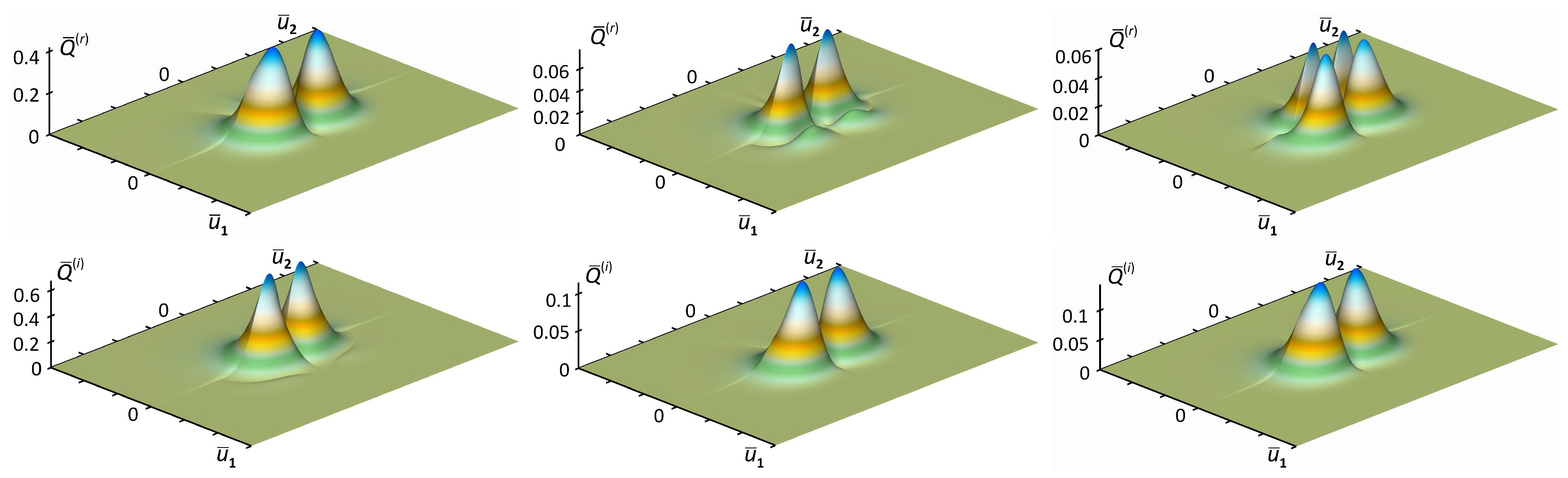
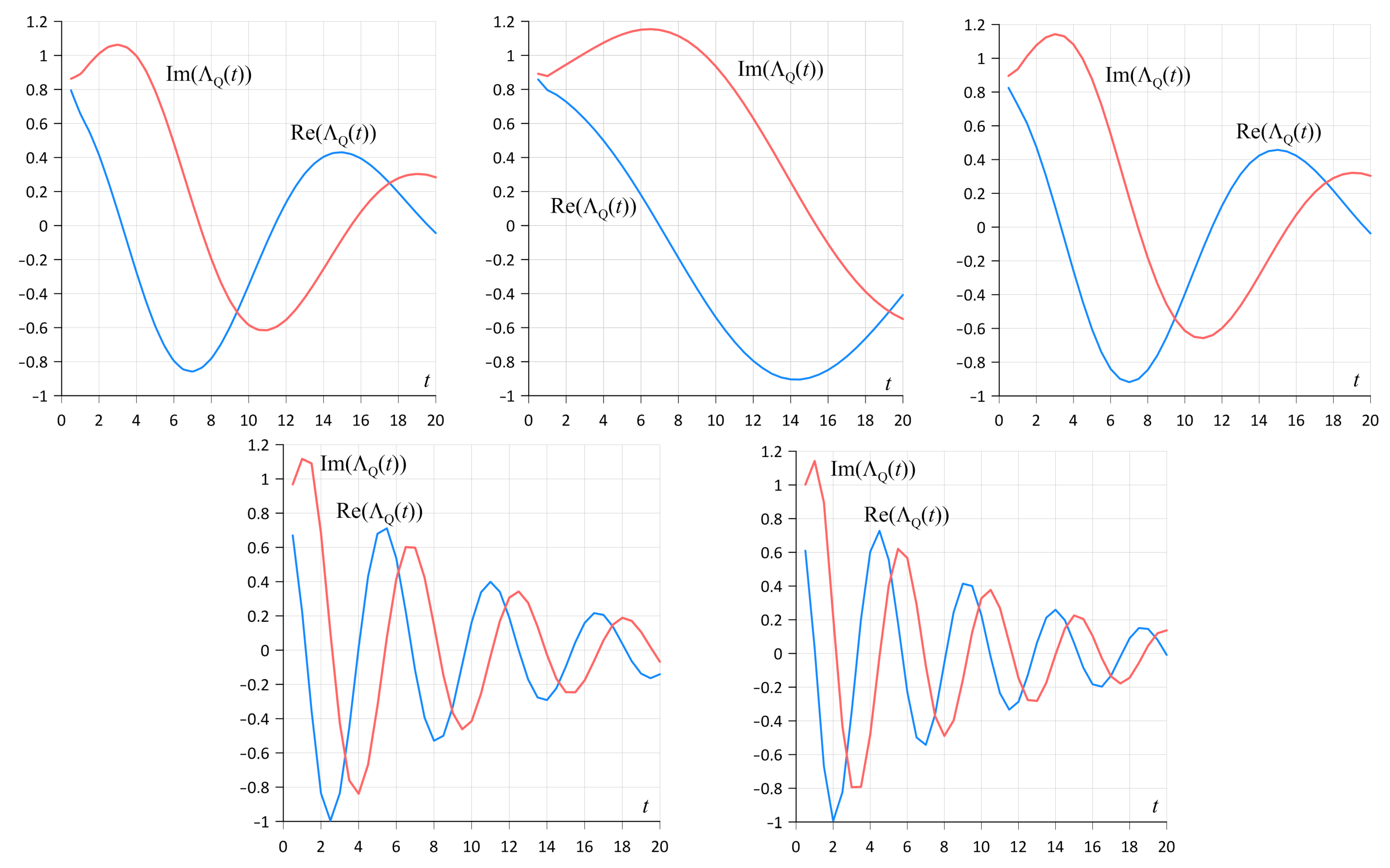
7.3. Mathematical Expectation of the Oscillator Trajectory
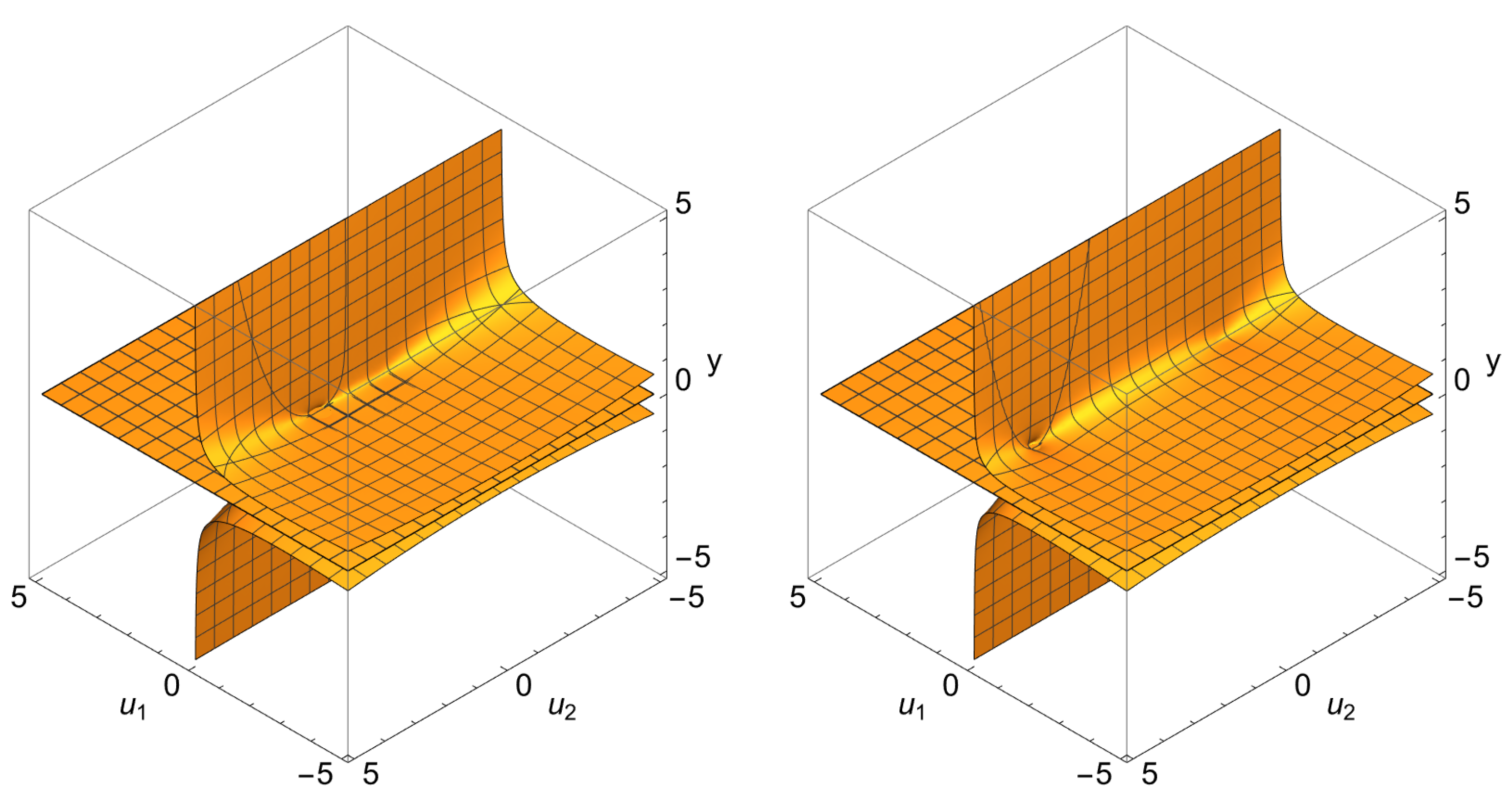
7.4. Calculation of Topological and Geometric Features of the Manifold
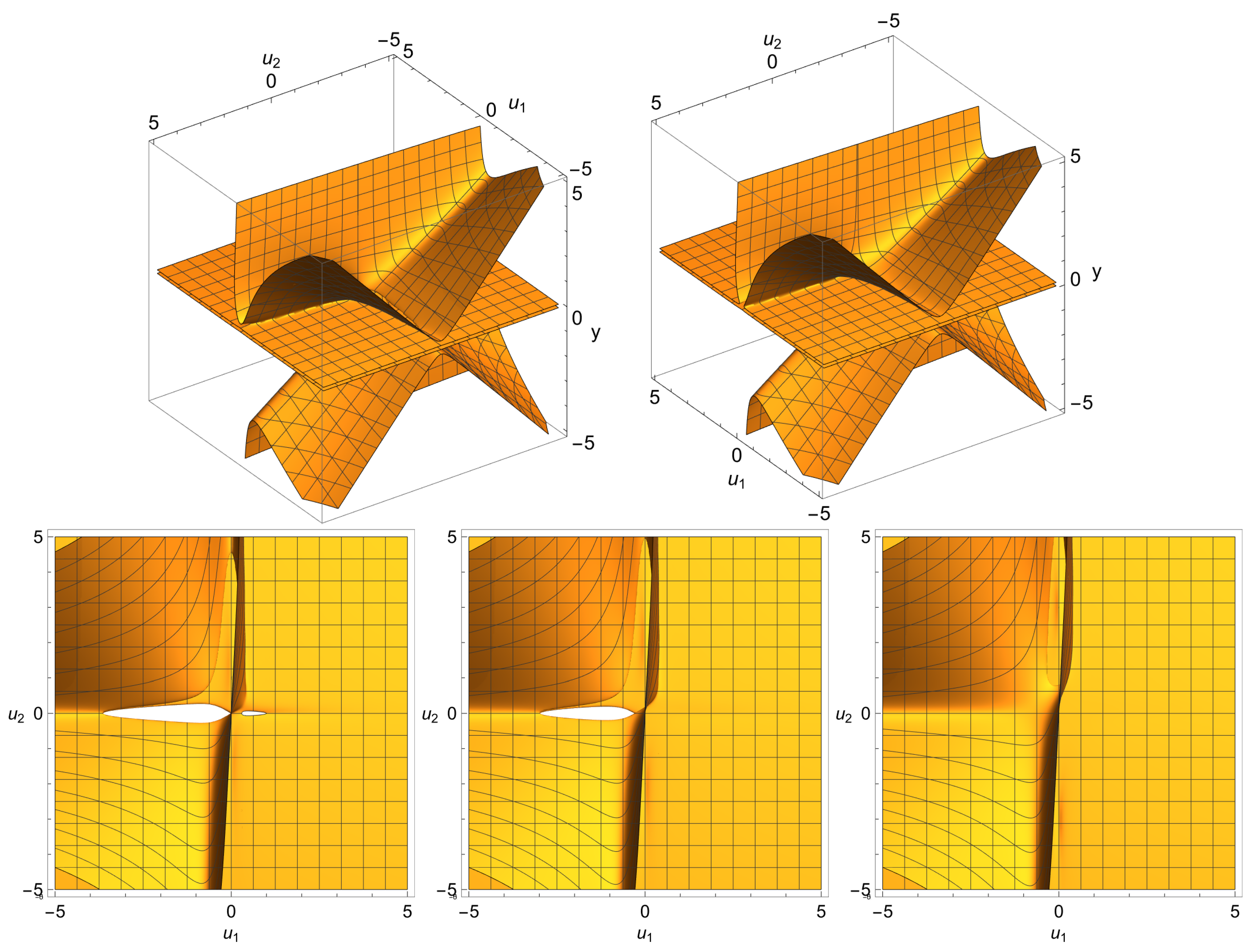
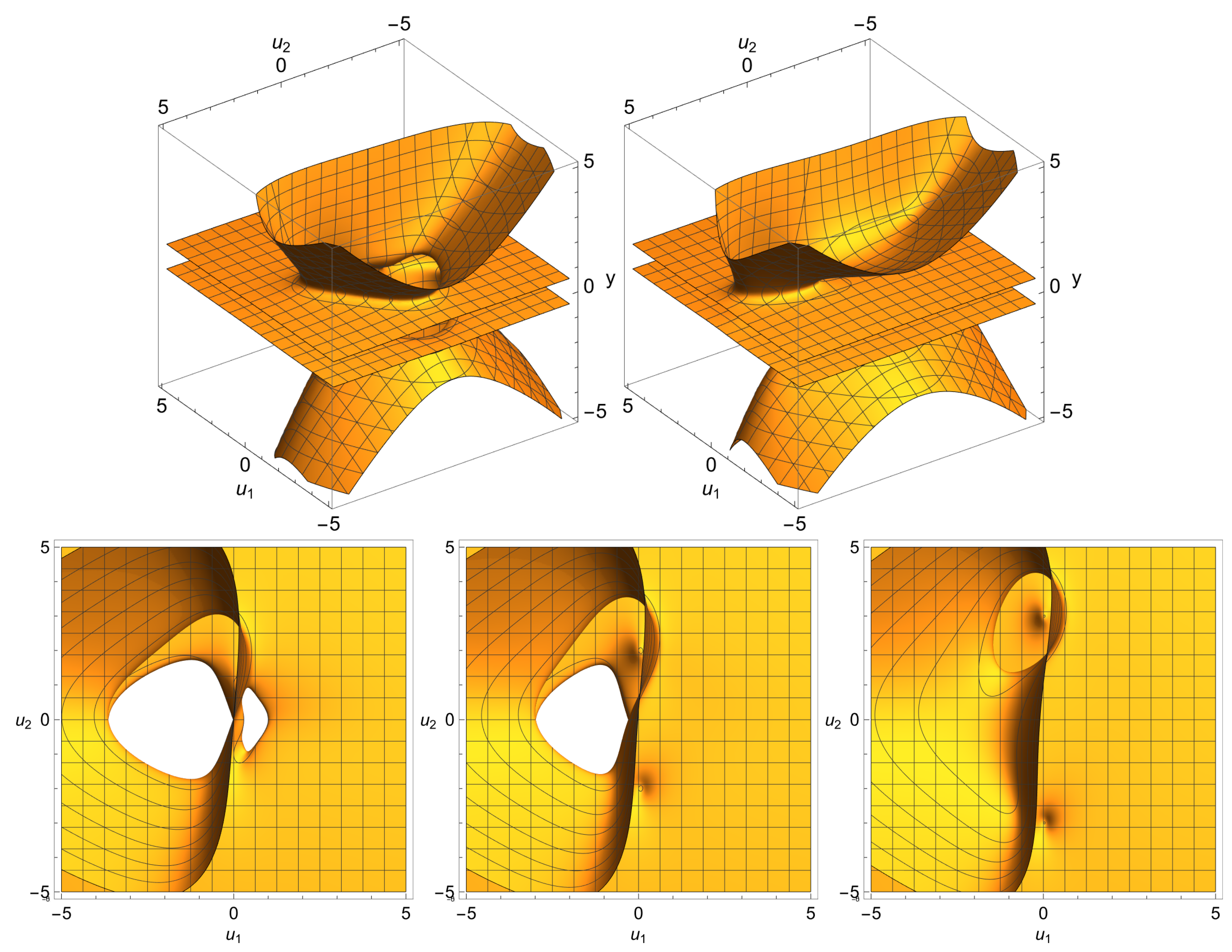

7.5. Features of Entropy Calculation
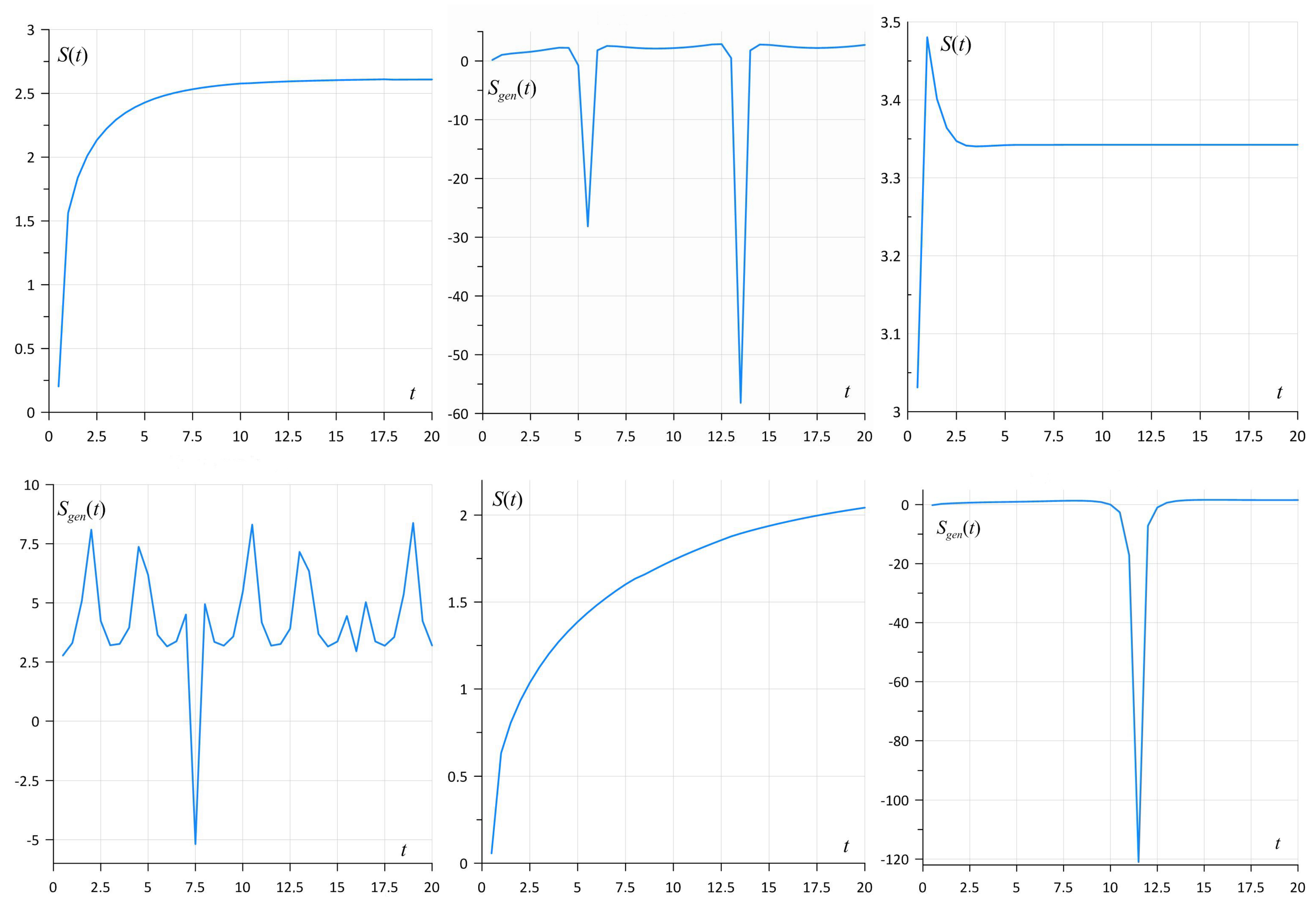
8. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| SEq | Statistical Equilibrium |
| PDE | Partial Differential Equation |
| SE | Small Environment |
| JS | Joint System |
| SDE | Stochastic Differential Equation |
References
- van Leeuwen, J. The Aristotelian Mechanics: Text and Diagrams; Springer: Berlin/Heidelberg, Germany, 2016; p. 262. [Google Scholar]
- Capecchi, D.; Ruta, G. Mechanics and Mathematics in Ancient Greece. Encyclopedia 2022, 2, 140–150. [Google Scholar] [CrossRef]
- Einstein, A. On the Motion of Small Particles Suspended in Liquids at Rest Required by the Molecular-Kinetic Theory of Heat. Ann. Phys. 1907, 17, 549, 1905. [Google Scholar] [CrossRef]
- Smoluchowski, M. Drei Vorträge über Diffusion, Brownsche Molekularbewegung und Koagulation von Kolloidteilchen. Phys. Z. 1916, 17, 557–571, 585–599. (In German) [Google Scholar]
- Ingarden, R.S.; Kossakowski, A.; Ohya, M. Information Dynamics and Open Systems: Classical and Quantum Approach; Springer: New York, NY, USA, 1997. [Google Scholar]
- Zubarev, D.N. Nonequilibrium Statistical Thermodynamics; Plenum Press: New York, NY, USA, 1974. [Google Scholar]
- Accardi, L.; Lu, Y.G.; Volovich, I.V. Quantum Theory and Its Stochastic Limit; Springer: New York, NY, USA, 2002. [Google Scholar]
- Tarasov, V.E. Quantum Mechanics of Non-Hamiltonian and Dissipative Systems; Elsevier Science: Amsterdam, The Netherlands; Boston, MA, USA; London, UK; New York, NY, USA, 2008. [Google Scholar]
- Gevorkyan, A.S.; Bogdanov, A.V.; Mareev, V.V. Hidden Dynamical Symmetry and Quantum Thermodynamics from the First Principles: Quantized Small Environment. Symmetry 2021, 13, 1546. [Google Scholar] [CrossRef]
- Hida, T. Brownian Motion; Springer: New York, NY, USA, 2012. [Google Scholar]
- Lenzi, E.K.; Evangelista, L.R.; Lenzi, M.K.; Ribeiro, H.V.; de Oliveira, E.C. Solutions for a non-Markovian diffusion equation. Phys. Lett. A 2010, 374, 4193–4198. [Google Scholar] [CrossRef]
- Morozov, A.N.; Skripkin, A.V. Spherical particle Brownian motion in viscous medium as non-Markovian random process. Phys. Lett. A 2011, 375, 4113–4115. [Google Scholar] [CrossRef]
- Munkres, J.R. Elements of Algebraic Topology; CRC Press: Boca Raton, FL, USA, 2019; p. 468. [Google Scholar]
- Phythian, R. The functional formalism of classical statistical dynamics. J. Phys. A Math. Gen. 1977, 10, 777. [Google Scholar] [CrossRef]
- Landau, L.D.; Lifshitz, E.M. Mechanics, 2nd ed.; Volume 1 Course in Theoretical Physics; Pergamon: Oxford, UK, 1969. [Google Scholar]
- Baź, A.N.; Zeĺdovich, Y.B.; Perelomov, A.M. Scattering Reactions and Decays in Nonrelativistic Quantum Mechanics; Nauka: Moscow, Russia, 1971. (In Russian) [Google Scholar]
- Klyatskin, V.I. Stochastic Equations: Theory and Applications in Acoustics, Hydrodynamics, Magnetohydrodynamics, and Radiophysics; Springer: Berlin/Heidelberg, Germany, 2015; Volume 1. [Google Scholar] [CrossRef]
- Gevorkyan, A.S.; Udalov, A.A. Exactly solvable models of quantum mechanics including fluctuations in the framework of representation of the wave function by random process. arXiv 2000, arXiv:quant-ph/0007108v1. [Google Scholar]
- Gevorkyan, A.S. Nonrelativistic Quantum Mechanics with Fundamental Environment. Found. Phys. 2011, 41, 509–515. [Google Scholar] [CrossRef][Green Version]
- Gardiner, C.W. Handbook of Stochastic Methods for Physics, Chemistry and Natural Sciences; Springer: Berlin, Germany; New York, NY, USA; Tokyo, Japan, 1985. [Google Scholar]
- Jost, J. Riemannian Geometry and Geometric Analysis; Springer: Berlin, Germany, 2002; ISBN 3-540-42627-2. [Google Scholar]
- Connes, A. Non-Commutative Geometry; Academic Press: Boston, MA, USA, 1994; ISBN 978-0-12-185860-5. [Google Scholar]
- Khalkhali, M.; Marcolli, M. Invitation to Non-Commutative Geometry; World Scientific Publishing Co. Pte. Ltd.: Singapore, 2008; p. 171. [Google Scholar]
- Burlakov, M.P. Kozyrev spaces. Fundam. Prikl. Mat. 2001, 7, 319–328. [Google Scholar]
- Kozyrev, N.A. Causal, or Asymmetric, Mechanics in a Linear Approximation; Pulkovo Observatory: Saint Petersburg, Russia, 1958. [Google Scholar]
- Cartan, É. La géométrie des espaces de Riemann. Mémorial Sci. Math. 1925, 73. [Google Scholar]
- Cartan, É. Sur les variétséà connexion affine et la théorie de la relativité généralisée. Part I. Ann. Sci. l’École Norm. Super. 1923, 40, 325–412. [Google Scholar] [CrossRef]
- Rudin, W. Principles of Mathematical Analysis; International Series in Pure and Applied Mathematics; McGraw-Hill: New York, NY, USA, 1976; ISBN 0-07-054235-X. [Google Scholar]
- Artin, E. Galois Theory; Dover Publications, Inc.: Mineola, NY, USA, 1997; ISBN 0-468-62342-4. [Google Scholar]
- Shannon, C.; Weaver, W. A Mathematical Theory of Communication; The University of Illinois Press: Urbana, IL, USA, 1949. [Google Scholar]
- Harada, T.; Sasa, S. Equality connecting energy dissipation with a violation of the fluctuation response relation. Phys. Rev. Lett. 2005, 95, 130602. [Google Scholar] [CrossRef] [PubMed]
- Toyabe, S.; Jiang, H.; Nakamura, T.; Murayama, Y.; Sano, M. Experimental test of a new equality: Measuring heat dissipation in an optically driven colloidal system. Phys. Rev. E 2007, 75, 011122. [Google Scholar] [CrossRef]
- Jarzynski, C. Nonequilibrium equality for free energy differences. Phys. Rev. Lett. 1997, 78, 2690. [Google Scholar] [CrossRef]
- Crooks, G.E. Entropy production fluctuation theorem and the non-equilibrium work relation for free energy differences. Phys. Rev. E 1999, 60, 2721. [Google Scholar] [CrossRef]
- Verley, G.; Willaert, T.; van den Broeck, C.; Esposito, M. Universal theory of efficiency fluctuations. Phys. Rev. E 2014, 90, 052145. [Google Scholar] [CrossRef]
- Verley, G.; Esposito, M.; Willaert, T.; Van den Broeck, C. The unlikely carnot efficiency. Nat. Commun. 2014, 5, 4721. [Google Scholar] [CrossRef]
- Manikandan, S.K.; Dabelow, L.; Eichhorn, R.; Krishnamurthy, S. Efficiency fluctuations in microscopic machines. Phys. Rev. Lett. 2019, 122, 140601. [Google Scholar] [CrossRef]
- Esposito, M. Stochastic thermodynamics under coarse graining. Phys. Rev. E 2012, 85, 041125. [Google Scholar] [CrossRef]
- Kawaguchi, K.; Nakayama, Y. Fluctuation theorem for hidden entropy production. Phys. Rev. E 2013, 88, 022147. [Google Scholar] [CrossRef]
- Sagawa, T.; Ueda, M. Fluctuation Theorem with Information Exchange: Role of Correlations in Stochastic Thermodynamics. Phys. Rev. Lett. 2012, 109, 180602. [Google Scholar] [CrossRef] [PubMed]
- Ito, S.; Sagawa, T. Information thermodynamics on causal networks. Phys. Rev. Lett. 2013, 111, 180603. [Google Scholar] [CrossRef] [PubMed]
- Horowitz, J.M.; Esposito, M. Thermodynamics with Continuous Information Flow. Phys. Rev. X 2014, 4, 031015. [Google Scholar] [CrossRef]
- Parrondo, J.M.R.; Horowitz, J.M.; Sagawa, T. Thermodynamics of information. Nat. Phys. 2015, 11, 131–139. [Google Scholar] [CrossRef]
- Schrödinger, E. What Is Life—The Physical Aspect of the Living Cell; Cambridge University Press: Cambridge, UK, 1944. [Google Scholar]
- Roache, P.J. Computational Fluid Dynamics; Hermosa Publishers: Albuquerque, NM, USA, 1972; 434p. [Google Scholar]
| ; | ; | ; | ; |
| ; | ; | ; | ; |
| ; | ; | ; | . |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gevorkyan, A.S.; Bogdanov, A.V.; Mareev, V.V.; Movsesyan, K.A. Theoretical and Numerical Study of Self-Organizing Processes in a Closed System Classical Oscillator and Random Environment. Mathematics 2022, 10, 3868. https://doi.org/10.3390/math10203868
Gevorkyan AS, Bogdanov AV, Mareev VV, Movsesyan KA. Theoretical and Numerical Study of Self-Organizing Processes in a Closed System Classical Oscillator and Random Environment. Mathematics. 2022; 10(20):3868. https://doi.org/10.3390/math10203868
Chicago/Turabian StyleGevorkyan, Ashot S., Aleksander V. Bogdanov, Vladimir V. Mareev, and Koryun A. Movsesyan. 2022. "Theoretical and Numerical Study of Self-Organizing Processes in a Closed System Classical Oscillator and Random Environment" Mathematics 10, no. 20: 3868. https://doi.org/10.3390/math10203868
APA StyleGevorkyan, A. S., Bogdanov, A. V., Mareev, V. V., & Movsesyan, K. A. (2022). Theoretical and Numerical Study of Self-Organizing Processes in a Closed System Classical Oscillator and Random Environment. Mathematics, 10(20), 3868. https://doi.org/10.3390/math10203868







