A Time-Inhomogeneous Prendiville Model with Failures and Repairs
Abstract
1. Introduction
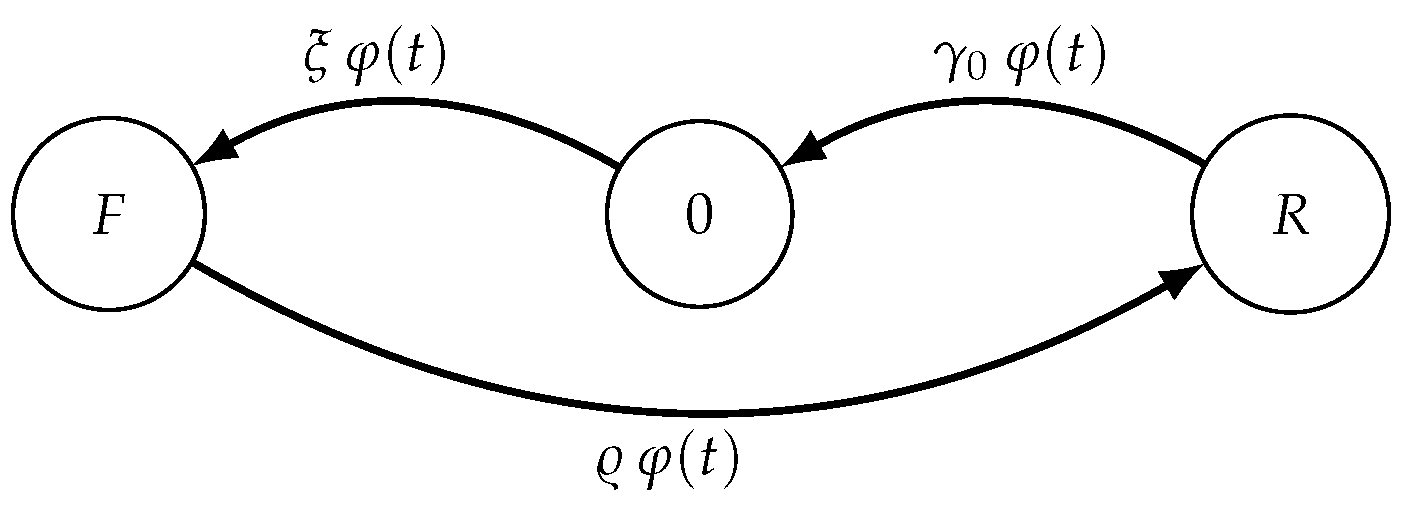
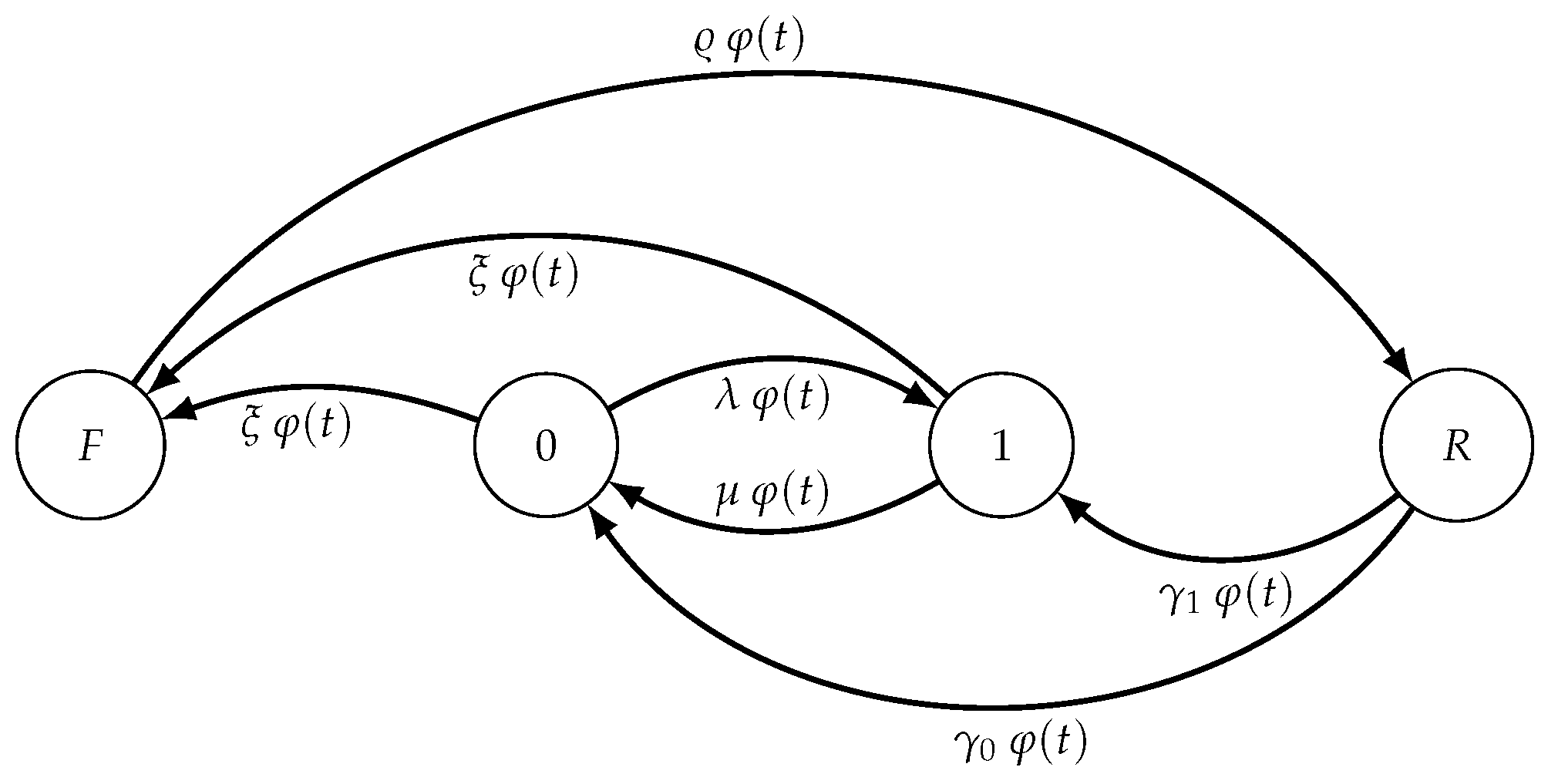
2. The Model
- with intensity function for ,
- with intensity function for ,
- with intensity function for ,
- with intensity function for ,
- with intensity function ,
3. Proportional Intensity Functions of Failures, Repairs and Restores
3.1. Asymptotic Behavior of the System
3.2. Transient Behavior of the System
- (i)
- If ,
- (ii)
- If ,
- (iii)
- If ,
4. Time of First Failure
5. Operating States and Their Probabilities
6. Asymptotic Distribution of Operating States
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
Appendix A. Proof of Proposition 4
References
- Anderson, W.J. Continuous-Time Markov Chains: An Applications-Oriented Approach; Springer Series in Statistics; Springer: New York, NY, USA, 1991. [Google Scholar]
- Iosifescu, M.; Tautu, P. Stochastic Processes and Applications in Biology and Medicine II. Models; Springer: Berlin/Heidelberg, Germany, 1973. [Google Scholar]
- Medhi, J. Stochastic Models in Queueing Theory; Academic Press: Amsterdam, The Netherlands, 2003. [Google Scholar]
- Bailey, N.T.J. The Elements of Stochastic Processes with Applications to the Natural Sciences; John Wiley & Sons, Inc.: New York, NY, USA, 1964. [Google Scholar]
- van Kampen, N.G. Stochastic Processes in Physics and Chemistry; Elsevier Science: Amsterdam, The Netherlands, 1992. [Google Scholar]
- Taylor, H.M.; Karlin, S. An Introduction to Stochastic Modeling; Academic Press: Boston, MA, USA, 1994. [Google Scholar]
- Sericola, B. Markov Chains: Theory, Algorithms and Applications; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2013. [Google Scholar]
- Dharmaraja, S.; Di Crescenzo, A.; Giorno, V.; Nobile, A.G. A continuous-time Ehrenfest model with catastrophes and its jump-diffusion approximation. J. Stat. Phys. 2015, 161, 326–345. [Google Scholar] [CrossRef]
- Giorno, V.; Nobile, A.G. On a bilateral linear birth and death process in the presence of catastrophe. In Computer Aided Systems Theory-EUROCAST 2013, Part I; Moreno-Diaz, R., Pichler, F., Quesada-Arencibia, A., Eds.; LNCS 8111; Springer: Berlin/Heidelberg, Germany, 2013; pp. 28–35. [Google Scholar]
- Giorno, V.; Nobile, A.G.; Spina, S. On some time non-homogeneous queueing systems with catastrophes. Appl. Math. Comp. 2014, 245, 220–234. [Google Scholar] [CrossRef]
- Giorno, V.; Nobile, A.G.; Pirozzi, E. A state-dependent queueing system with asymptotic logarithmic distribution. J. Math. Anal. Appl. 2018, 458, 949–966. [Google Scholar] [CrossRef]
- Di Crescenzo, A.; Giorno, V.; Nobile, A.G. Constructing transient birth-death processes by means of suitable transformations. Appl. Math. Comp. 2016, 281, 152–171. [Google Scholar] [CrossRef]
- Economou, A.; Fakinos, D. A continuous-time Markov chain under the influence of a regulating point process and applications in stochastic models with catastrophes. Eur. J. Oper. Res. 2003, 149, 625–640. [Google Scholar] [CrossRef]
- Economou, A.; Fakinos, D. Alternative approaches for the transient analysis of Markov chains with catastrophes. J. Stat. Theory Pract. 2008, 2, 183–197. [Google Scholar] [CrossRef][Green Version]
- Chen, A.; Zhang, H.; Liu, K.; Rennolls, K. Birth-death processes with disasters and instantaneous resurrection. Adv. Appl. Probab. 2004, 36, 267–292. [Google Scholar] [CrossRef]
- Di Crescenzo, A.; Giorno, V.; Krishna Kumar, B.; Nobile, A.G. A double-ended queue with catastrophes and repairs, and a jump-diffusion approximation. Method. Comput. Appl. Probab. 2012, 14, 937–954. [Google Scholar] [CrossRef]
- Di Crescenzo, A.; Giorno, V.; Krishna Kumar, B.; Nobile, A.G. A time-non-homogeneous double-ended queue with failures and repairs and its continuous approximation. Mathematics 2018, 6, 81. [Google Scholar] [CrossRef]
- Ye, J.; Liu, L.; Jiang, T. Analysis of a Single-Sever Queue with Disasters and Repairs Under Bernoulli Vacation Schedule. J. Syst. Sci. Inf. 2016, 4, 547–559. [Google Scholar] [CrossRef]
- Mytalas, G.C.; Zazanis, M.A. An MX/G/1 queueing system with disasters and repairs under a multiple adapted vacation policy. Nav. Res. Logist. 2015, 62, 171–189. [Google Scholar] [CrossRef]
- Krishna Kumar, B.K.; Krihnamoorthy, A.; Madheswari, S.P.; Basha, S.S. Transient analysis of a single server queue with catastrophes, failures, and repairs. Queueing Syst. 2007, 56, 133–141. [Google Scholar] [CrossRef]
- Altiok, T. On the phase-type approximations of general distributions. IIE Trans. 1985, 17, 110–116. [Google Scholar] [CrossRef]
- Altiok, T. Queueing modeling of a single processor with failures. Perform. Eval. 1989, 9, 93–102. [Google Scholar] [CrossRef]
- Altiok, T. Performance Analysis of Manufacturing Systems; Springer Series in Operations Research; Springer: New York, NY, USA, 1997. [Google Scholar]
- Dallery, Y. On modeling failure and repair times in stochastic models of manufacturing systems using generalized exponential distributions. Queueing Syst. 1994, 15, 199–209. [Google Scholar] [CrossRef]
- Kendall, D.G. On the generalized “birth-and-death”process. Ann. Math. Stat. 1948, 19, 1–15. [Google Scholar] [CrossRef]
- McNeil, D.R.; Schach, S. Central limit analogues for Markov population processes. J. R. Stat. Soc. Ser. B (Methodol.) 1973, 35, 1–23. [Google Scholar] [CrossRef]
- Di Crescenzo, A.; Nobile, A.G. Diffusion approximation to a queueing system with time dependent arrival and service rates. Queueing Syst. 1995, 19, 41–62. [Google Scholar] [CrossRef]
- Di Crescenzo, A.; Giorno, V.; Krishna Kumar, B.; Nobile, A.G. M/M/1 queue in two alternating environments and its heavy traffic approximation. J. Math. Anal. Appl. 2018, 458, 973–1001. [Google Scholar] [CrossRef]
- Giorno, V.; Nobile, A.G.; Ricciardi, L.M. On some time-nonhomogeneous diffusion approximations to queueing systems. Adv. Appl. Prob. 1987, 19, 974–994. [Google Scholar] [CrossRef]
- Giorno, V.; Nobile, A.G. On a class of birth-death processes with time-varying intensity functions. Appl. Math. Comput. 2020, 379, 125255. [Google Scholar] [CrossRef]
- Ammar, S.I.; Zeifman, A.; Satin, Y.; Kiseleva, K.; Koroley, V. On limiting characteristics for a non-stationary two–processor heterogeneous system with catastrophes, server failures and repairs. J. Ind. Manag. Optim. 2021, 17, 1057–1068. [Google Scholar] [CrossRef]
- Zeifman, A.I.; Isaacson, D.L. On strong ergodicity for nonhomogeneous continuous-time Markov chains. Stoch. Process. Appl. 1994, 50, 263–273. [Google Scholar] [CrossRef]
- Zeifman, A.; Satin, Y.; Korolev, V.; Shorgin, S. On truncations for weakly ergodic inhomogeneous birth and death processes. Int. J. Appl. Math. Comput. Sci. 2014, 24, 503–518. [Google Scholar] [CrossRef]
- Satin, Y.A.; Zeifman, A.I.; Shilova, G.N. On approaches to constructing limiting regimes for some queuing models. Inform. Primen. 2020, 14, 3–9. [Google Scholar]
- Jouini, O.; Dallery, Y. Moments of first passage times in general birth-death processes. Math. Meth. Oper. Res. 2008, 68, 49–76. [Google Scholar] [CrossRef]
- Giorno, V.; Nobile, A.G. First-passage times and related moments for continuous-time birth-death chains. Ric. Mat. 2019, 68, 629–659. [Google Scholar] [CrossRef]
- Prendiville, B.J. Discussion: Symposium on stochastic processes. J. Roy. Statist. Soc. B 1949, 11, 273. [Google Scholar]
- Takashima, M. Note on evolutionary processes. Bull. Math. Stat. 1956, 7, 18–24. [Google Scholar] [CrossRef]
- Giorno, V.; Negri, C.; Nobile, A.G. A solvable model for a finite capacity queueing system. J. Appl. Prob. 1985, 22, 903–911. [Google Scholar] [CrossRef]
- Ricciardi, L.M. Stochastic Population Theory: Birth and Death Processes. In Mathematical Ecology. Biomathematics; Hallam, T.G., Levin, S.A., Eds.; Springer: Berlin/Heidelberg, Germany, 1986; Volume 17, pp. 155–190. [Google Scholar]
- Karlin, S.; McGregor, J. Ehrenfest Urn Model. J. Appl. Prob. 1965, 2, 352–376. [Google Scholar] [CrossRef]
- Flegg, M.B.; Pollett, P.K.; Gramotnev, D.K. Ehrenfest model for condensation and evaporation processes in degrading aggregates with multiple bonds. Phys. Rev. E 2008, 78, 031117. [Google Scholar] [CrossRef]
- Zheng, Q. Note on the non-homogeneous Prendiville process. Math. Biosci. 1998, 148, 1–5. [Google Scholar] [CrossRef]
- Giorno, V.; Nobile, A.G.; Saura, A. Prendiville Stochastic Growth Model in the Presence of Catastrophes. In Cybernetics and Systems 2004, Proceedings of the 17th European Meeting on Cybernetics and Systems Research, Vienna, Austria, 13–16 April 2004; Trappl, R., Ed.; Austrian Society for Cybernetic Studies: Vienna, Austria, 2004; pp. 151–156. [Google Scholar]
- Giorno, V.; Nobile, A.G.; Spina, S. Some Remarks on the Prendiville Model in the Presence of Jumps. In Computer Aided Systems Theory–EUROCAST 2019; Moreno-Díaz, R., Pichler, F., Quesada-Arencibia, A., Eds.; LNCS 12013; Springer: Berlin/Heidelberg, Germany, 2020; pp. 150–157. [Google Scholar]
- Parthasarathy, P.R.; Krishna Kumar, B. Stochastic Compartmental models with Prendiville growth mechanisms. Math. Biosci. 1995, 125, 51–60. [Google Scholar] [CrossRef]
- Matis, J.H.; Kiffe, T.R. Stochastic Compartment models with Prendiville growth rates. Math. Biosci. 1996, 138, 31–43. [Google Scholar] [CrossRef]
- Buonocore, A.; Di Crescenzo, A.; Giorno, V.; Nobile, A.G.; Ricciardi, L.M. A Markov chain-based model for actomyosin dynamics. Sci. Math. Jpn. 2009, 70, 159–174. [Google Scholar]
- Giorno, V.; Nobile, A.G. Bell polynomial approach for time-inhomogeneous linear birth-death process with immigration. Mathematics 2020, 8, 1123. [Google Scholar] [CrossRef]
- Gradshteyn, I.S.; Ryzhik, I.M. Table of Integrals, Series and Products; Academic Press Inc.: Cambridge, MA, USA, 2014. [Google Scholar]
- Williams, W.E. Partial Differential Equations; Clarendon Press: Oxford, UK, 1980. [Google Scholar]


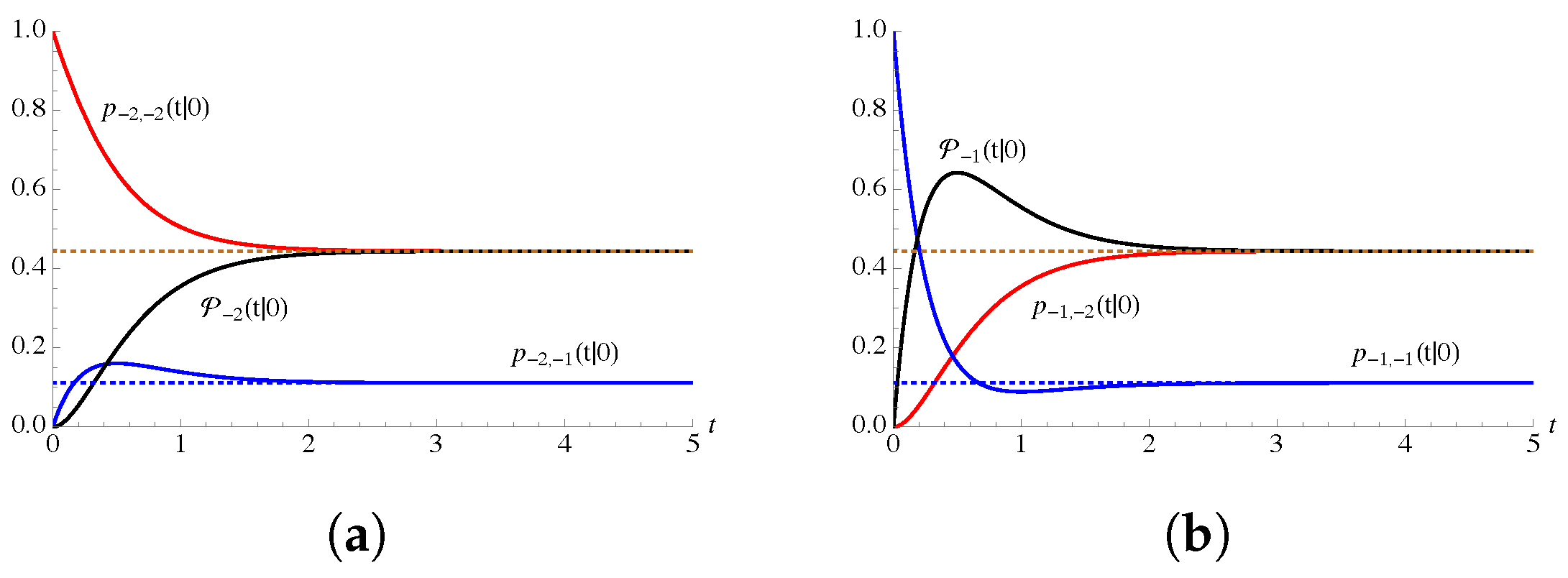
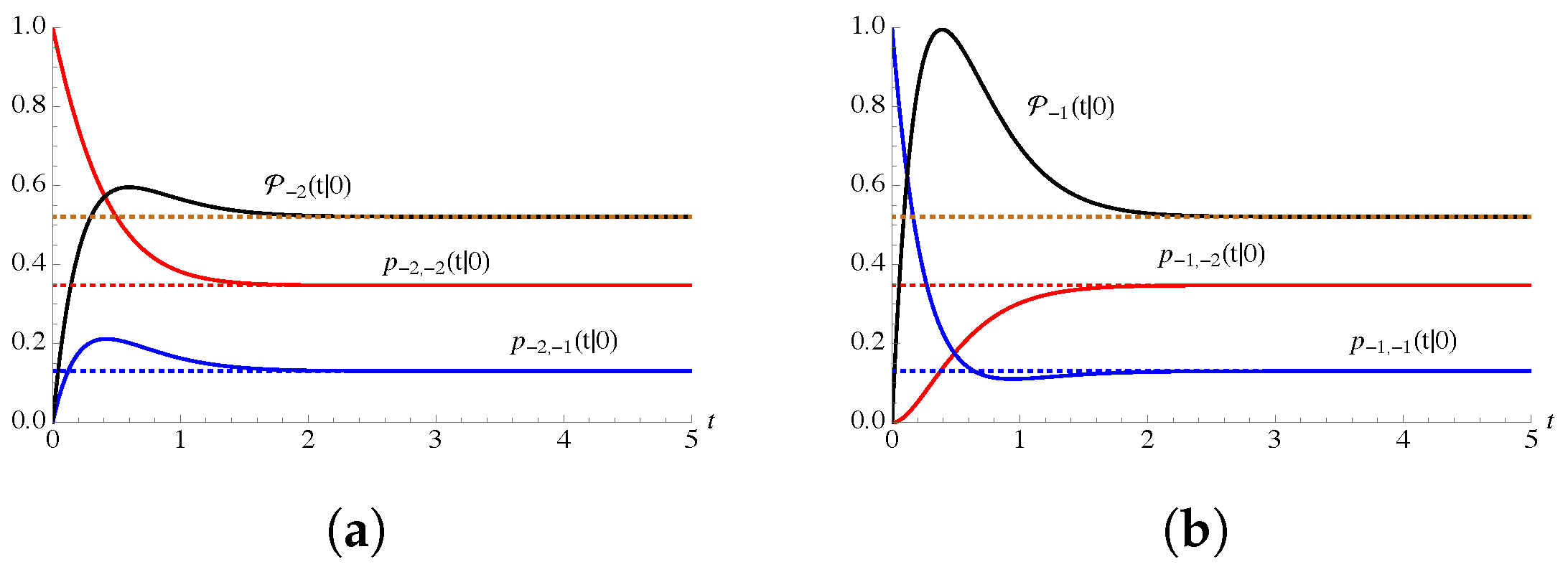


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Giorno, V.; Nobile, A.G. A Time-Inhomogeneous Prendiville Model with Failures and Repairs. Mathematics 2022, 10, 251. https://doi.org/10.3390/math10020251
Giorno V, Nobile AG. A Time-Inhomogeneous Prendiville Model with Failures and Repairs. Mathematics. 2022; 10(2):251. https://doi.org/10.3390/math10020251
Chicago/Turabian StyleGiorno, Virginia, and Amelia G. Nobile. 2022. "A Time-Inhomogeneous Prendiville Model with Failures and Repairs" Mathematics 10, no. 2: 251. https://doi.org/10.3390/math10020251
APA StyleGiorno, V., & Nobile, A. G. (2022). A Time-Inhomogeneous Prendiville Model with Failures and Repairs. Mathematics, 10(2), 251. https://doi.org/10.3390/math10020251





