Numerical Research of Dynamical Behavior in Engineering Applications by Using E–E Method
Abstract
:1. Introduction
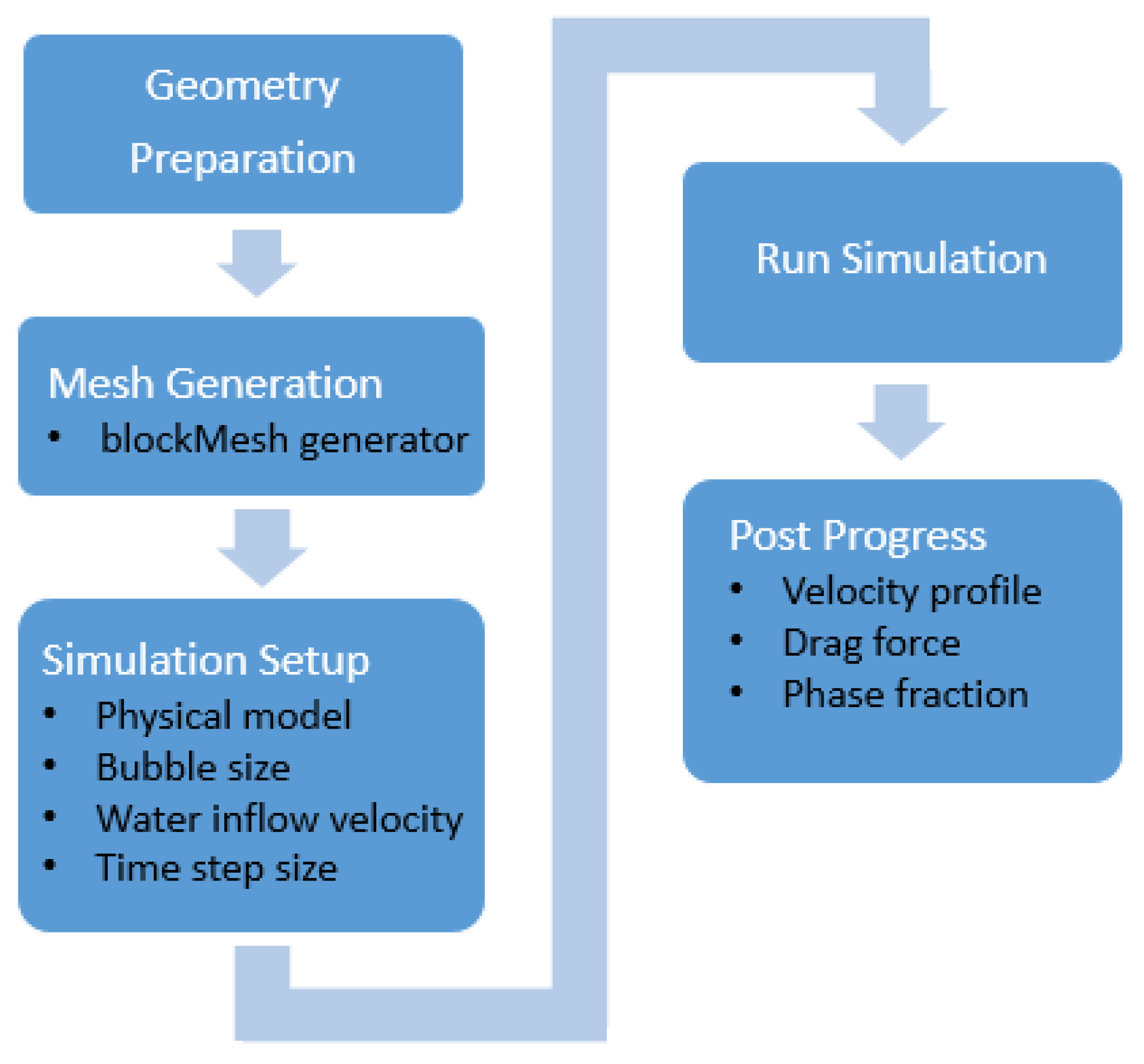
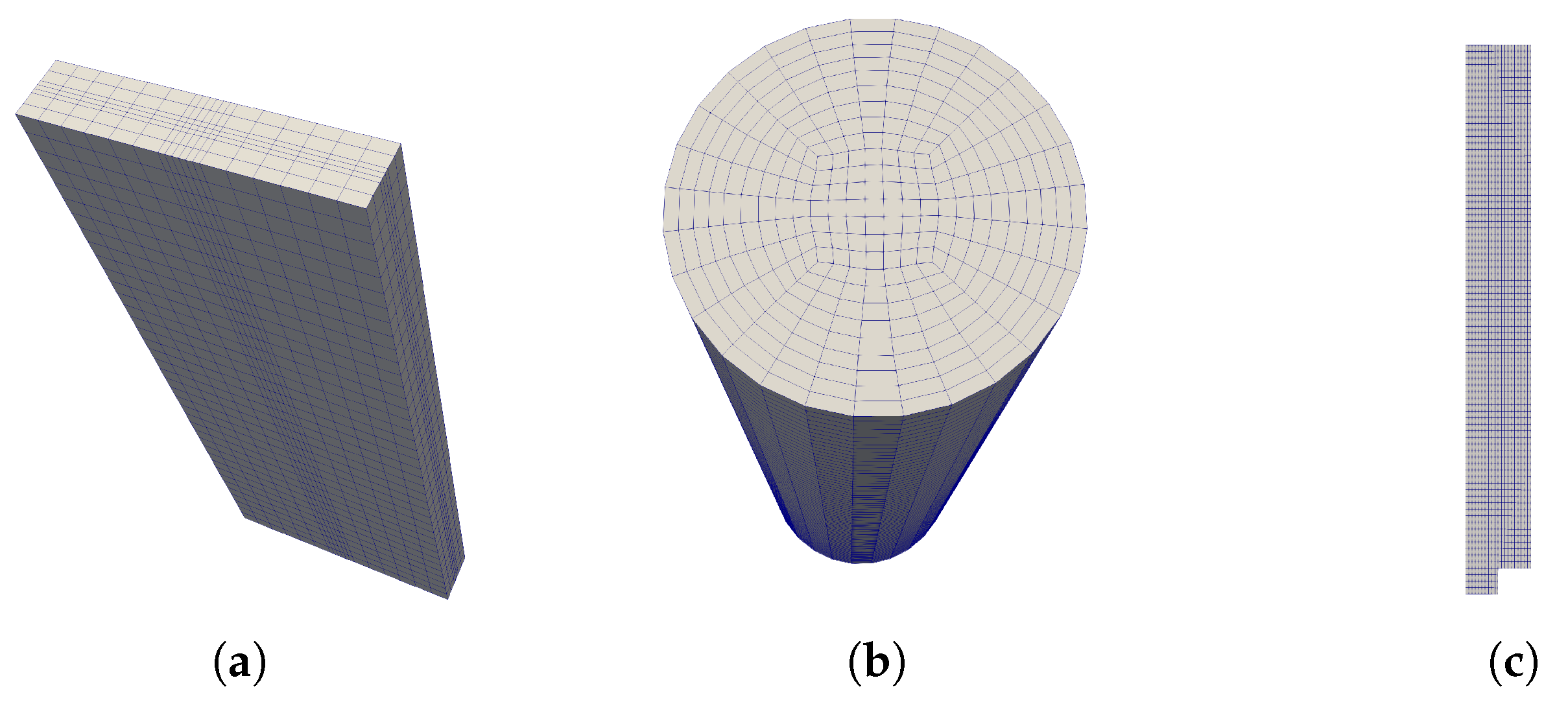
2. Description of Models
3. Test Cases
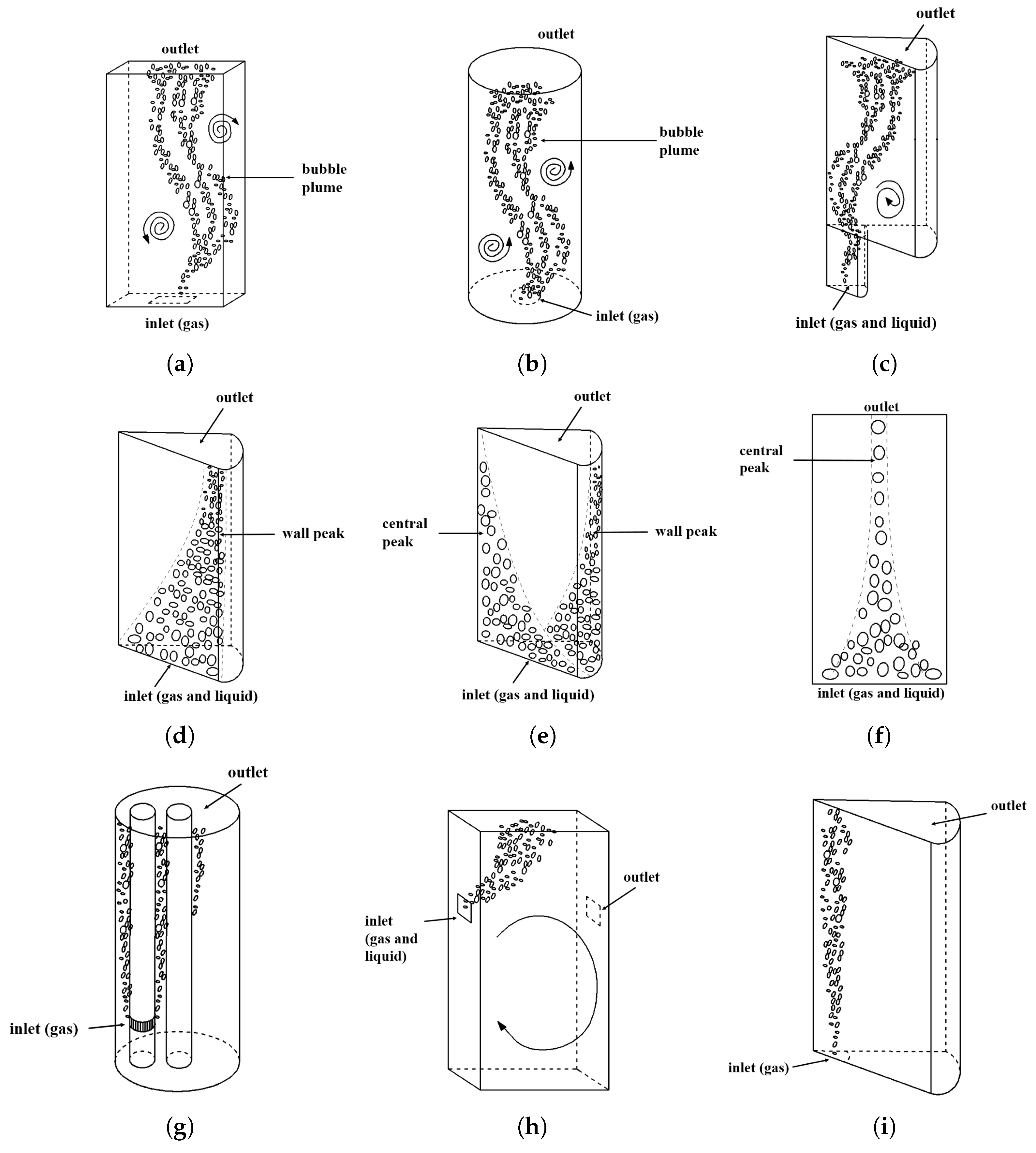
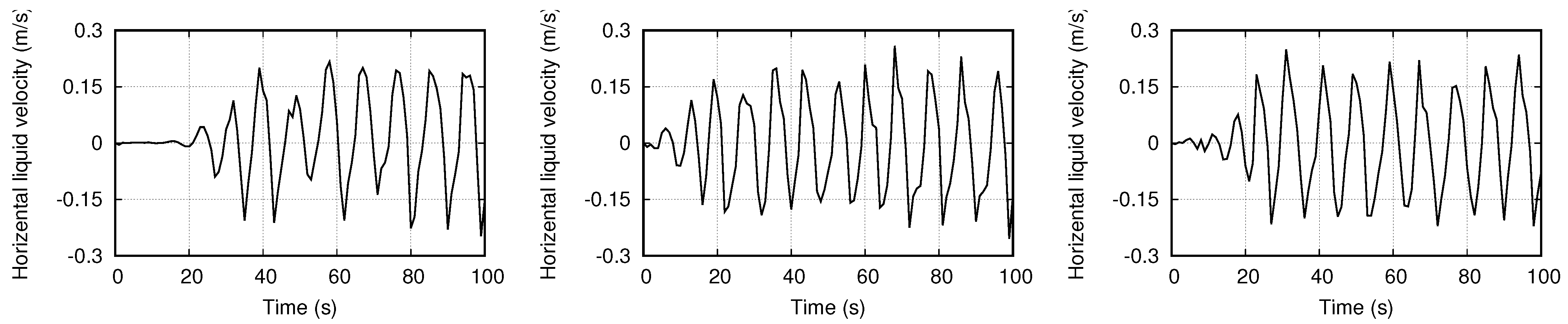
- Test case A.1: investigation of bubble plumes in a bubble column with rectangular shape developed by Díaz et al. [39]. The test setup consisted of a partially aerated bubble column with a width of 0.2 m, height of 1.8 m and depth of 0.04 m filled with tap water to 0.45 m under atmospheric pressure and room temperature while the air was fed through a sparger with eight concentric holes with a diameter of 1 mm and pitch 6 mm. This was a very interesting test case since liquid vortices created by bubble plumes were desirable for mixing and, consequently, accelerating all transport phenomena [40]. In addition, flow structures with unsteady liquid recirculations were typical phenomena of industrial bubble columns.
- Test case A.2: investigation of gas-liquid flows in an industrial bubble column proposed by McClure et al. [41], which was a partially aerated bubble column with a cylindrical shape of 0.19 m in diameter filled with water up to 1 m equipped with a multi-point sparger. The aspect ratio () was about 5. In the bio-processing industry, the bubble column height-to-diameter ratio typically ranges from 2 to 5 and is usually operated in the heterogeneous flow regime to speed up mass and heat transfer.
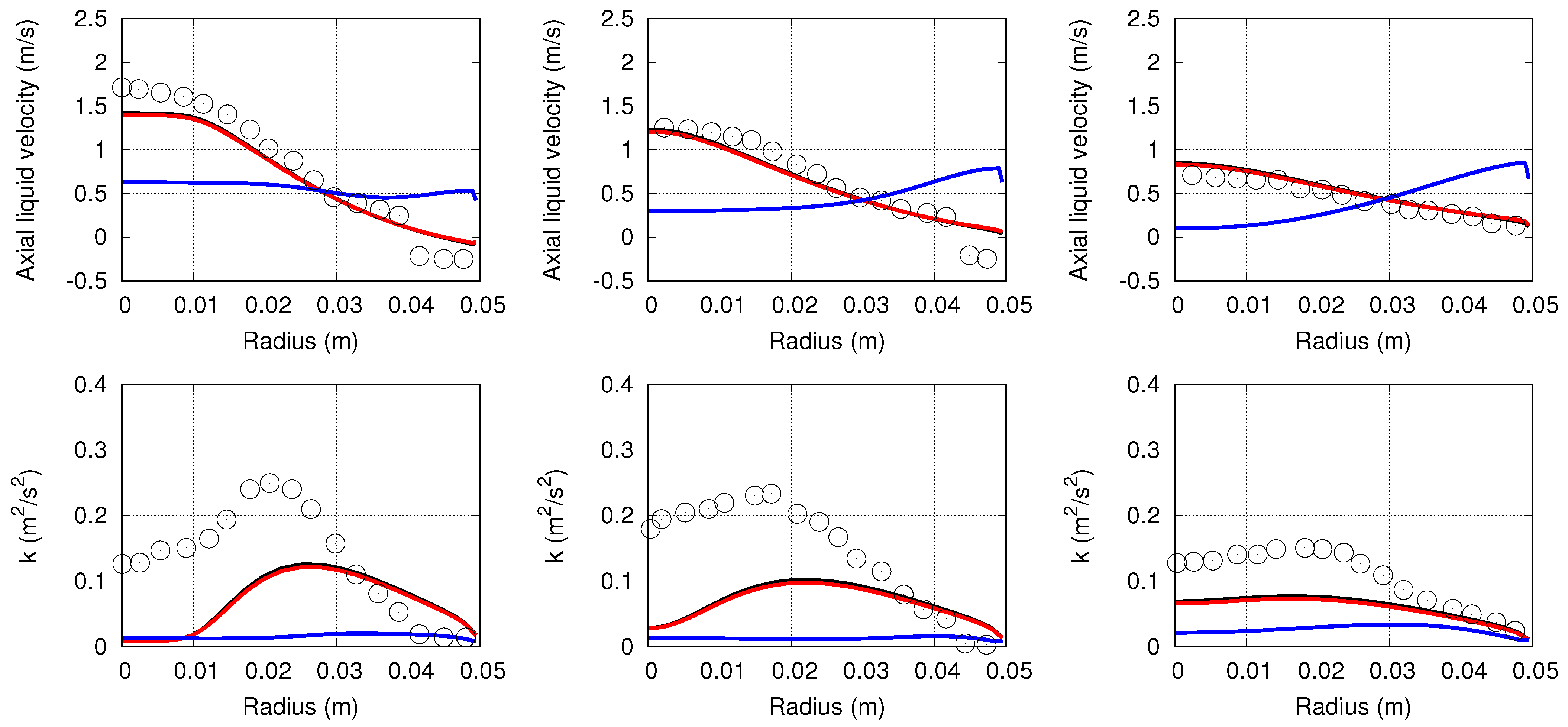
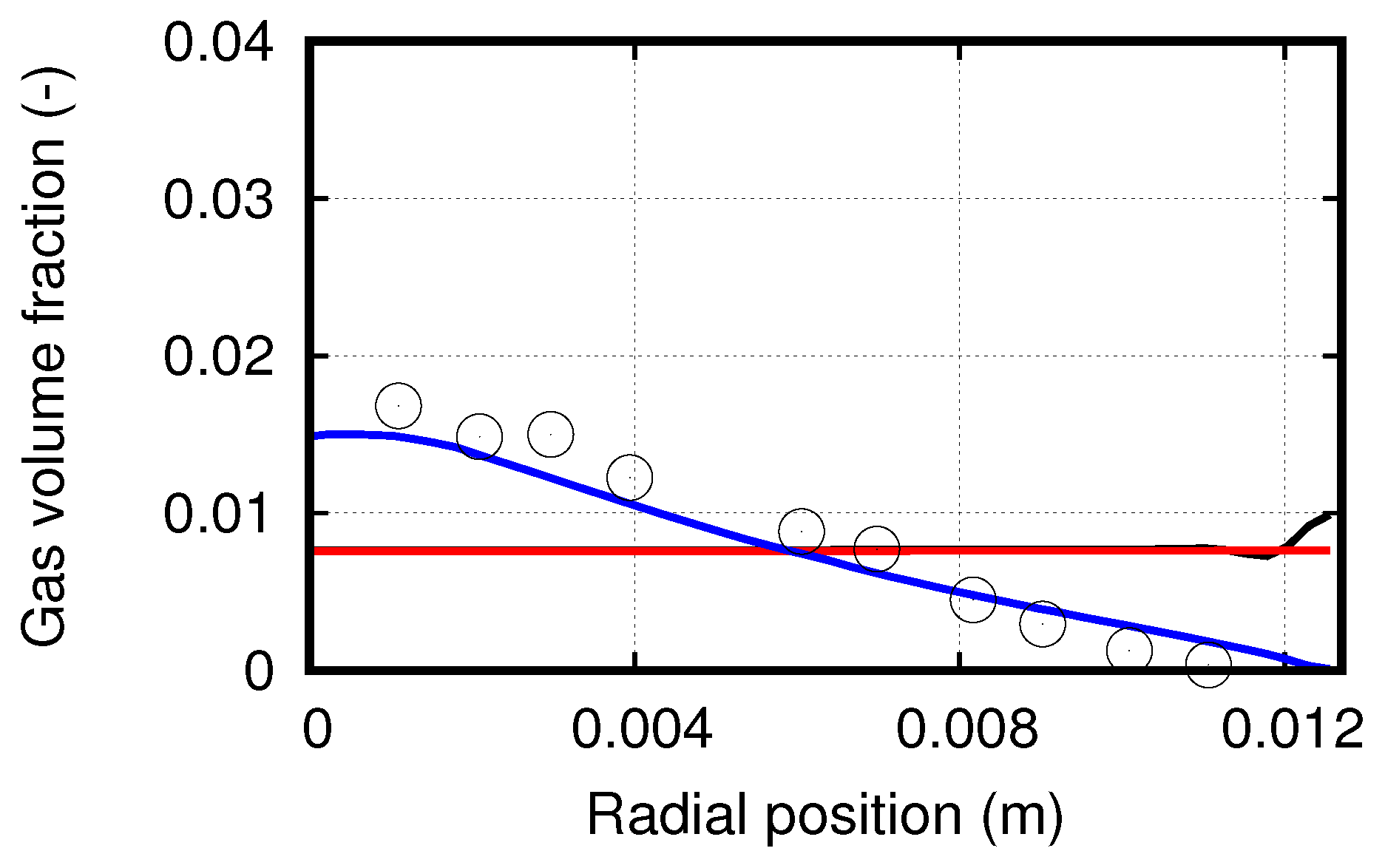
- Test case A.3: the test case investigated in this work was a bubbly air/water upward flow in a pipe with a sudden enlargement. The two sections of the pipe were 50 and 100 mm in diameter. The inlet phase fraction was known to have a wall peak. The main characteristic of this test was a large separation zone at the pipe bottom. This has been extensively applied for the verification and implimenttion of the E–E method [42,43,44,45].
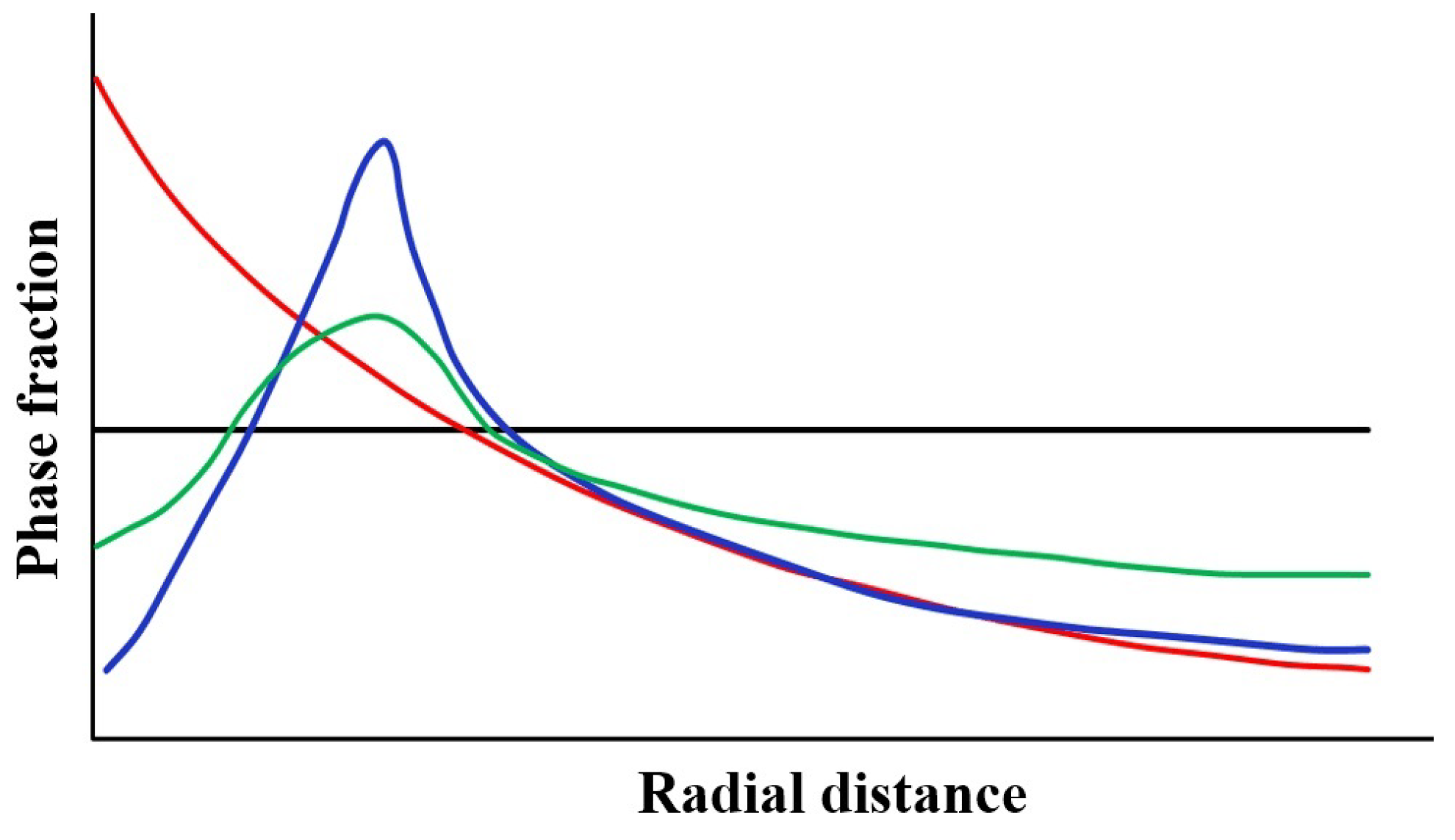
- Test case B.1: investigation of bubbly flow in a cylindrical pipe of Lucas et al. [46]. The mixture of the gas and liquid was injected from the bottom pipe of 0.0256 m in diameter and 3.53 m in height. In vertical upward flows, small bubbles moved towards the wall. A wall peak for gas phase fraction occurred at high . Tomiyama et al. witnessed this phenomenon for individual bubbles [47]. For vertical co-current pipe flows, radial flow fields had symmetric stability over a long distance. Hence, this flow type is well investigated in terms of non-drag forces.
- Test case B.2: investigation of bubbly flows in a circular pipe developed by Banowski et al. [48]. The experiment comprised a vertical pipe with an inner diameter of 54.8 mm and a length of 6 m. The gas and liquid mixture was injected from the bottom. It was similar to the test case B.2. However, besides the typical wall peak formed due to the smaller bubbles’ movement, a double peak of the phase fraction can also be observed due to the existence of large bubbles since large bubbles tend to the centre.
- Test case B.3: investigation of bubbly flows in a rectangle pipe developed by Žun [49]. This test case was similar to test case B.3 with a slight difference of geometry. The mixture of the gas and liquid was injected from the bottom of a rectangle channel of 0.0254 m in length and 2 m in height. Only the central peak of the gas phase was witnessed.
- Test case B.4: investigation of the bubbly flow of Besagni et al. [50]. The gas-liquid flows in bubble columns with annular gaps and two non-regular internal pipes were investigated. It consisted of a non-pressurized vertical column with an inner diameter of 0.24 m and height of 5.3 m. It consisted of a non-pressurized vertical column with an inner diameter of 0.24 m and a height of 5.3 m. In the simulation domain, the height was limited to 5 m. Two internal pipes were arranged within the column: one positioned asymmetrically (0.075 m in external diameter) and the other positioned centrally (0.06 m in external diameter). The sparger was assumed to be a uniform surface with a cylindrical shape of 0.01 m in height located on the lateral inner pipe at a vertical position of 0.3 m from the domain bottom. The aspect ratio of the geometry was small. Due to the existence of the non-irregular components, the gas phase fraction distribution developed to be quite flattened, and no wall peak was observed in the experiments, even though it could be seen as a pipe flow.
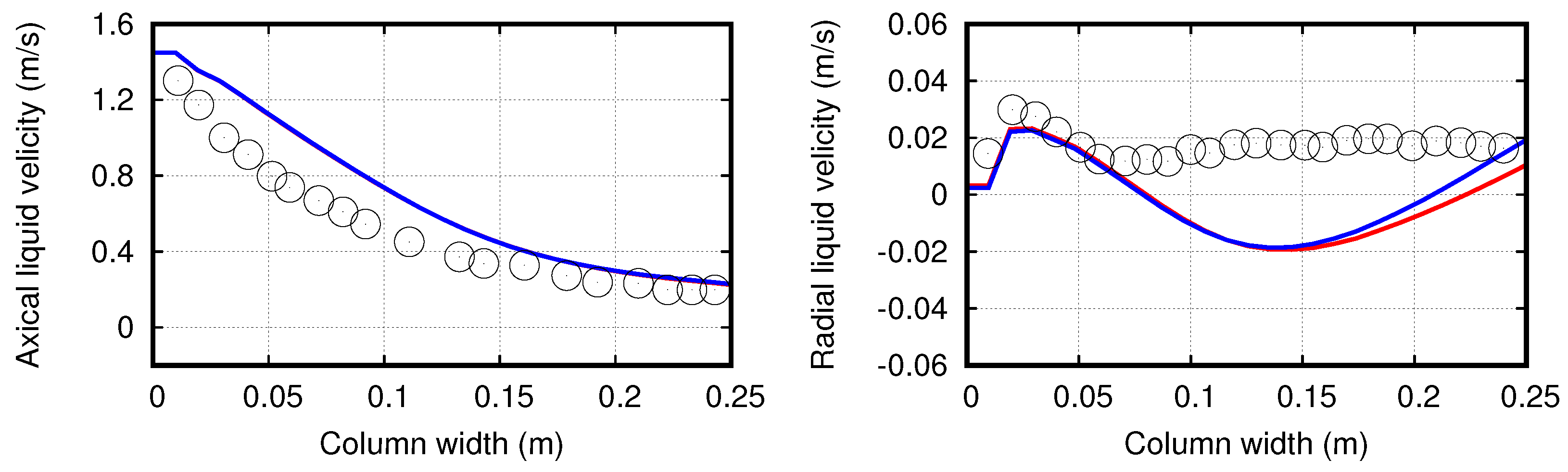
- Test case C.1: investigation of gas-liquid flows in a continuous casting mould of Iguchi and Kasai [51]. The geometry employed in this test case was quite different from previous ones. The gas and liquid were injected into a rectangular vessel of 0.3 m in length and 0.15 m in width. In the experiments, it was observed that larger bubbles were lifted towards the liquid surface because of buoyancy forces exerted on them, while smaller bubbles were pushed deep. Such a phenomenon is also known as phase segregation or poly-dispersity in other works, which was proven as a tough work for the E–E method.
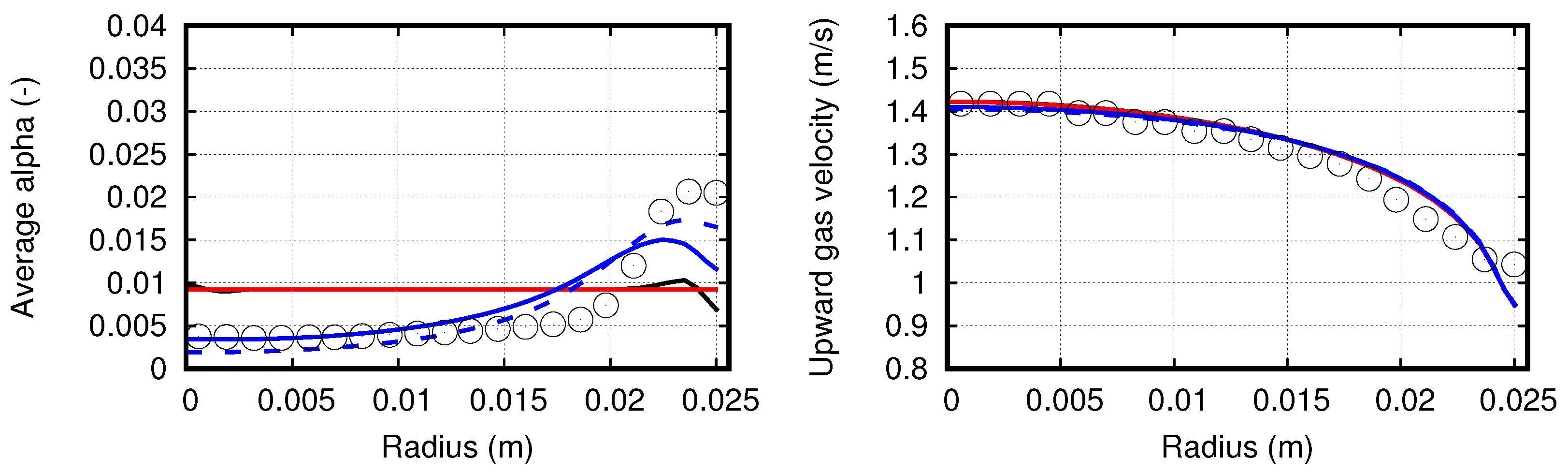
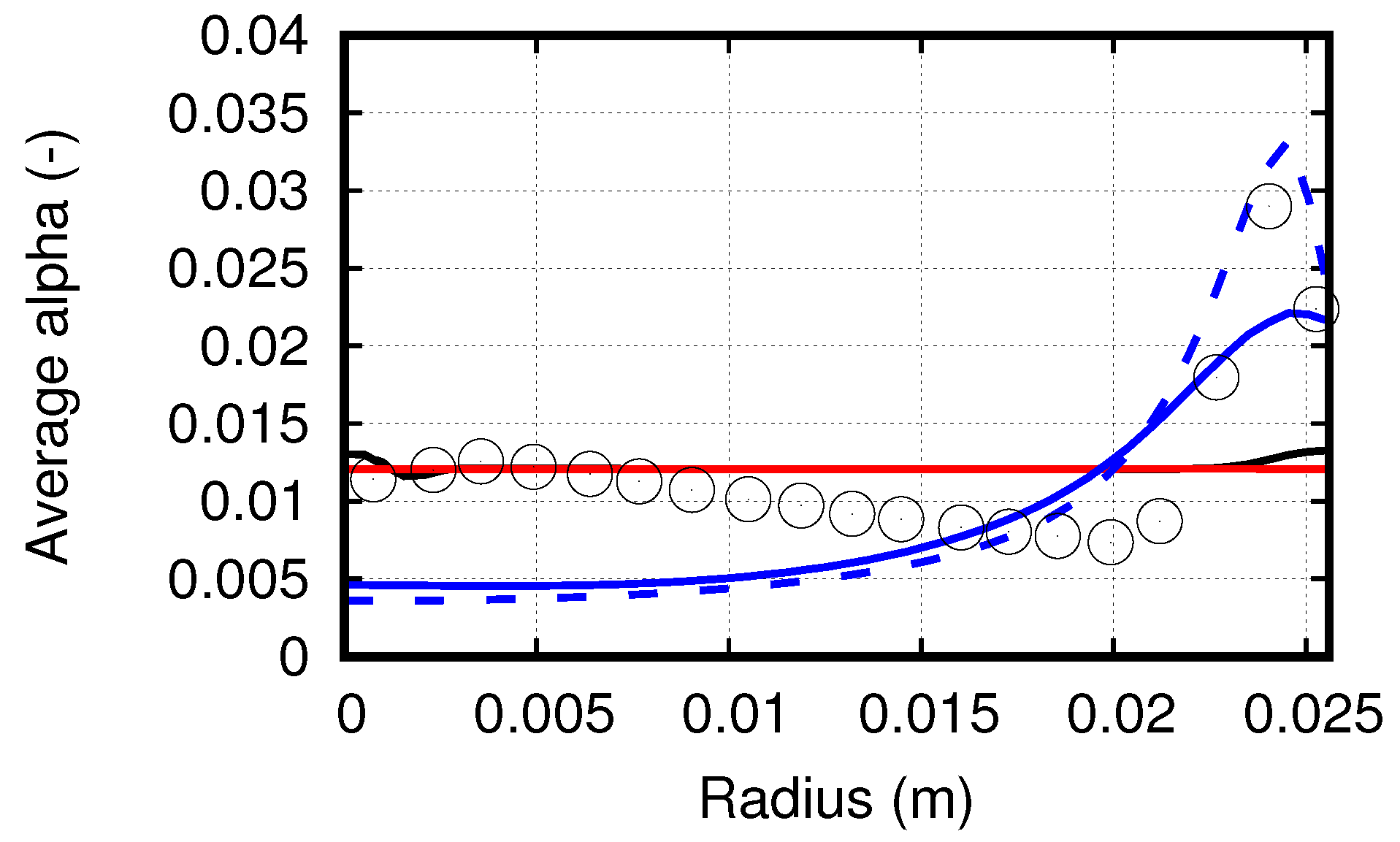
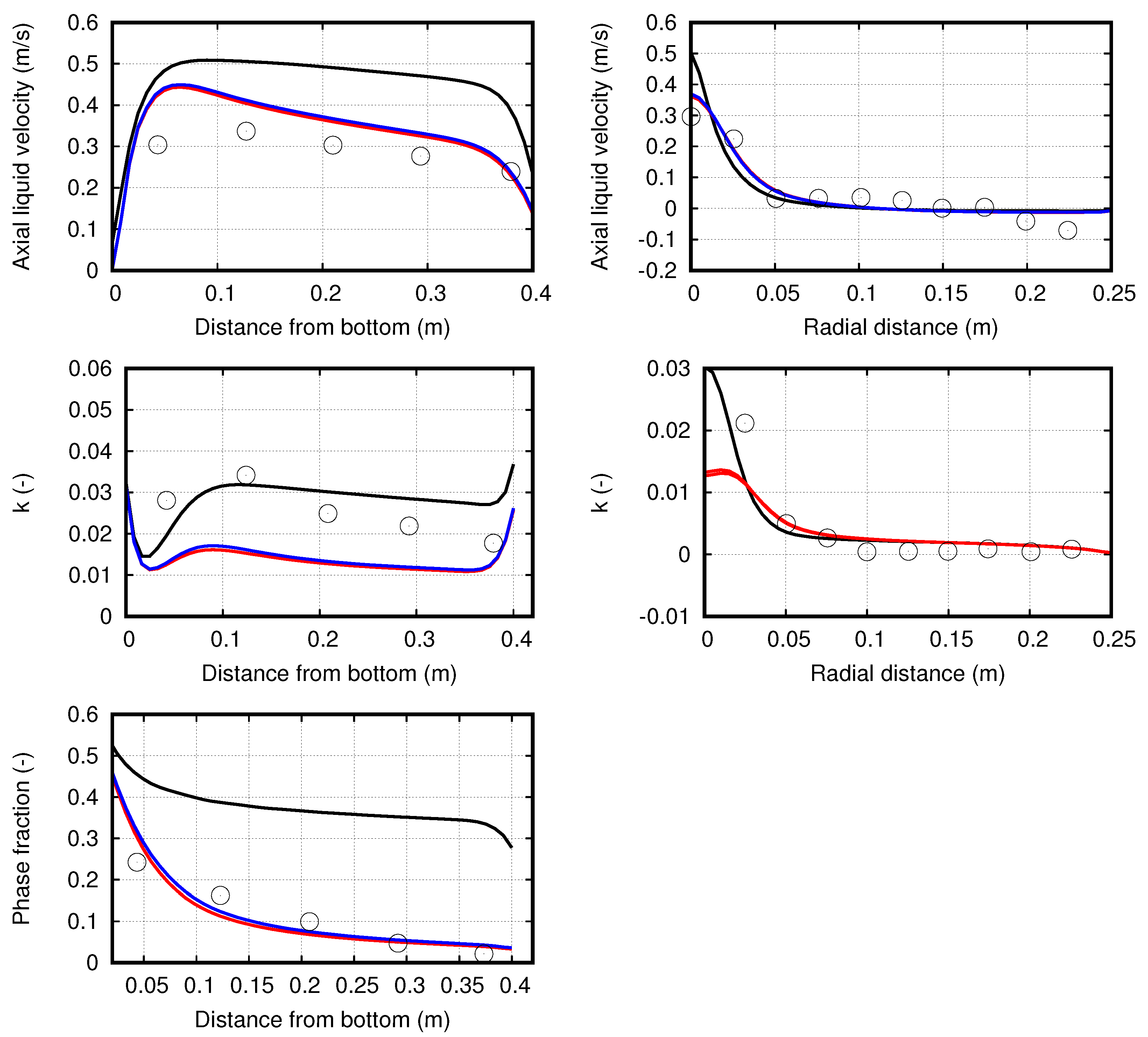
- Test case C.2: investigation of gas-liquid flows in a continuous casting mould of Sheng and Irons [52]. The gas phase was injected from the bottom of a vessel with a height of 0.76 m and a diameter of 0.5 m. In this test case, measured gas phase fraction distributions, turbulence fields, and velocities of gas/liquid in plume zones were employed for the validation of different turbulence models. It was observed to be a suitable test case to validate the multi-phase turbulence model against experimental data.
4. Results and Discussion
4.1. Test Case A.1–A.3
4.2. Test Case B.1–B.4
4.3. Test Case C.1–C.2
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
References
- Lopez De Bertodano, M.; Lee, S.; Lahey, R.; Drew, D. The prediction of two-phase turbulence and phase distribution phenomena using a Reynolds stress model. J. Fluids Eng. 1990, 112, 107–113. [Google Scholar] [CrossRef]
- Jakobsen, H.; Lindborg, H.; Dorao, C. Modeling of bubble column reactors: Progress and limitations. Ind. Eng. Chem. Res. 2005, 44, 5107–5151. [Google Scholar] [CrossRef]
- Law, D.; Battaglia, F.; Heindel, T. Model validation for low and high superficial gas velocity bubble column flows. Chem. Eng. Sci. 2008, 63, 4605–4616. [Google Scholar] [CrossRef]
- Law, D.; Jones, S.; Heindel, T.; Battaglia, F. A Combined Numerical and Experimental Study of Hydrodynamics for an Air-Water External Loop Airlift Reactor. J. Fluids Eng. 2011, 133, 021301. [Google Scholar] [CrossRef]
- Besagni, G.; Inzoli, F.; Ziegenhein, T.; Lucas, D. Computational Fluid-Dynamic modeling of the pseudo-homogeneous flow regime in large-scale bubble columns. Chem. Eng. Sci. 2017, 160, 144–160. [Google Scholar] [CrossRef]
- Banaei, M.; Jegers, J.; Van Sint Annaland, M.; Kuipers, J.; Deen, N. Tracking of particles using TFM in gas-solid fluidized beds. Adv. Powder Technol. 2018, 29, 2538–2547. [Google Scholar] [CrossRef]
- Banaei, M.; Deen, N.; Van Sint Annaland, M.; Kuipers, J. Particle mixing rates using the two-fluid model. Particuology 2018, 36, 13–26. [Google Scholar] [CrossRef]
- Besagni, G.; Inzoli, F. Prediction of Bubble Size Distributions in Large-Scale Bubble Columns Using a Population Balance Model. Computation 2019, 7, 17. [Google Scholar] [CrossRef]
- Shi, W.; Yang, X.; Sommerfeld, M.; Yang, J.; Cai, X.; Li, G.; Zong, Y. Modelling of mass transfer for gas-liquid two-phase flow in bubble column reactor with a bubble breakage model considering bubble-induced turbulence. Chem. Eng. J. 2019, 371, 470–485. [Google Scholar] [CrossRef]
- Buwa, V.; Deo, D.; Ranade, V. Eulerian–Lagrangian simulations of unsteady gas–liquid flows in bubble columns. Int. J. Multiph. Flow 2006, 32, 864–885. [Google Scholar] [CrossRef]
- Padding, J.; Deen, N.; Peters, E.; Kuipers, J. Euler–Lagrange modeling of the hydrodynamics of dense multiphase flows. In Advances in Chemical Engineering; Elsevier: Amsterdam, The Netherlands, 2015; Volume 46, pp. 137–191. [Google Scholar]
- Quiyoom, A.; Buwa, V.; Ajmani, S. Euler-Lagrange Simulations of Gas-Liquid Flow in a Basic Oxygen Furnace and Experimental Verification. In Fluid Mechanics and Fluid Power–Contemporary Research; Springer: Berlin/Heidelberg, Germany, 2017; pp. 1151–1161. [Google Scholar]
- Battistella, A.; Aelen, S.; Roghair, I.; Van Sint Annaland, M. Euler–Lagrange modeling of bubbles formation in supersaturated water. ChemEngineering 2018, 2, 39. [Google Scholar] [CrossRef]
- Deen, N.; Kuipers, J. Direct numerical simulation (DNS) of mass, momentum and heat transfer in dense fluid-particle systems. Curr. Opin. Chem. Eng. 2014, 5, 84–89. [Google Scholar] [CrossRef]
- Das, S.; Deen, N.; Kuipers, J. A DNS study of flow and heat transfer through slender fixed-bed reactors randomly packed with spherical particles. Chem. Eng. Sci. 2017, 160, 1–19. [Google Scholar] [CrossRef]
- Rabha, S.; Buwa, V. Volume-of-fluid (VOF) simulations of rise of single/multiple bubbles in sheared liquids. Chem. Eng. Sci. 2010, 65, 527–537. [Google Scholar] [CrossRef]
- Goel, D.; Buwa, V. Numerical simulations of bubble formation and rise in microchannels. Ind. Eng. Chem. Res. 2008, 48, 8109–8120. [Google Scholar] [CrossRef]
- Roghair, I.; Annaland, M.; Kuipers, J. An improved Front-Tracking technique for the simulation of mass transfer in dense bubbly flows. Chem. Eng. Sci. 2016, 152, 351–369. [Google Scholar] [CrossRef]
- Roghair, I.; Baltussen, M.; Van Sint Annaland, M.; Kuipers, J. Direct numerical simulations of the drag force of bi-disperse bubble swarms. Chem. Eng. Sci. 2013, 95, 48–53. [Google Scholar] [CrossRef]
- Rzehak, R.; Ziegenhein, T.; Kriebitzsch, S.; Krepper, E.; Lucas, D. Unified modeling of bubbly flows in pipes, bubble columns, and airlift columns. Chem. Eng. Sci. 2017, 157, 147–158. [Google Scholar] [CrossRef]
- Ziegenhein, T.; Lucas, D. The critical bubble diameter of the lift force in technical and environmental, buoyancy-driven bubbly flows. Int. J. Multiph. Flow 2019, 116, 26–38. [Google Scholar] [CrossRef]
- Hessenkemper, H.; Ziegenhein, T.; Lucas, D. Contamination effects on the lift force of ellipsoidal air bubbles rising in saline water solutions. Chem. Eng. J. 2019, 386, 121589. [Google Scholar] [CrossRef]
- Picardi, R.; Zhao, L.; Battaglia, F. On the ideal grid resolution for two-dimensional eulerian modeling of gas–liquid flows. J. Fluids Eng. 2016, 138, 114503. [Google Scholar] [CrossRef]
- Panicker, N.; Passalacqua, A.; Fox, R. On the hyperbolicity of the two-fluid model for gas–liquid bubbly flows. Appl. Math. Model. 2018, 57, 432–447. [Google Scholar] [CrossRef]
- Vaidheeswaran, A.; Lopez De Bertodano, M. Stability and convergence of computational Eulerian two-fluid model for a bubble plume. Chem. Eng. Sci. 2017, 160, 210–226. [Google Scholar] [CrossRef]
- Mohanarangam, K.; Cheung, S.C.P.; Tu, J.; Chen, L. Numerical simulation of micro-bubble drag reduction using population balance model. Ocean Eng. 2009, 36, 863–872. [Google Scholar] [CrossRef]
- Zhang, X.; Wang, J.; Wan, D. Euler-Lagrange study of bubble drag reduction in turbulent channel flow and boundary layer flow. Phys. Fluids 2020, 32, 027101. [Google Scholar]
- Zhao, X.; Zong, Z.; Jiang, Y.C.; Pan, Y. Numerical simulation of micro-bubble drag reduction of an axisymmetric body using OpenFOAM. J. Hydrodyn. 2018, 31, 900–910. [Google Scholar] [CrossRef]
- Ma, T.; Ziegenhein, T.; Lucas, D.; Fröhlich, J. Large eddy simulations of the gas–liquid flow in a rectangular bubble column. Nucl. Eng. Des. 2016, 299, 146–153. [Google Scholar] [CrossRef]
- Drew, D. Mathematical modeling of two–phase flow. Annu. Rev. Fluid Mech. 1982, 15, 261–291. [Google Scholar] [CrossRef]
- Antal, S.; Lahey, R.; Flaherty, J. Analysis of phase distribution in fully developed laminar bubbly two-phase flow. Int. J. Multiph. Flow 1991, 17, 635–652. [Google Scholar] [CrossRef]
- Tomiyama, A.; Tamai, H.; Zun, I.; Hosokawa, S. Transverse migration of single bubbles in simple shear flows. Chem. Eng. Sci. 2002, 57, 1849–1858. [Google Scholar] [CrossRef]
- Lopez De Bertodano, M. Turbulent Bubbly Two-Phase Flow in a Triangular Duct. Ph.D. Thesis, Rensselaer Polytechnic Institute, Troy, NY, USA, 1992. [Google Scholar]
- Tomiyama, A.; Sou, A.; Zun, I.; Kanami, N.; Sakaguchi, T. Effects of Eötvös number and dimensionless liquid volumetric flux on lateral motion of a bubble in a laminar duct flow. Adv. Multiph. Flow 1995, 1995, 3–15. [Google Scholar]
- Ishii, M.; Hibiki, T. Thermo-Fluid Dynamics of Two-Phase Flow; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- Lopez De Bertodano, M.; Fullmer, W.; Clausse, A.; Ransom, V. Two-Fluid Model Stability, Simulation and Chaos; Springer: Berlin/Heidelberg, Germany, 2016. [Google Scholar]
- Li, D.; Christian, H. Simulation of bubbly flows with special numerical treatments of the semi-conservative and fully conservative two-fluid model. Chem. Eng. Sci. 2017, 174, 25–39. [Google Scholar] [CrossRef]
- Ishii, M.; Zuber, N. Drag coefficient and relative velocity in bubbly, droplet or particulate flows. AIChE J. 1979, 25, 843–855. [Google Scholar] [CrossRef]
- Díaz, M.; Iranzo, A.; Cuadra, D.; Barbero, R.; Montes, F.; Galán, M. Numerical simulation of the gas–liquid flow in a laboratory scale bubble column: Influence of bubble size distribution and non-drag forces. Chem. Eng. J. 2008, 139, 363–379. [Google Scholar] [CrossRef]
- Sokolichin, A.; Eigenberger, G.; Lapin, A.; Lübert, A. Dynamic numerical simulation of gas-liquid two-phase flows Euler/Euler versus Euler/Lagrange. Chem. Eng. Sci. 1997, 52, 611–626. [Google Scholar] [CrossRef]
- McClure, D.; Norris, H.; Kavanagh, J.; Fletcher, D.; Barton, G. Validation of a computationally efficient computational fluid dynamics (CFD) model for industrial bubble column bioreactors. Ind. Eng. Chem. Res. 2014, 53, 14526–14543. [Google Scholar] [CrossRef]
- Behzadi, A.; Issa, R.; Rusche, H. Modelling of dispersed bubble and droplet flow at high phase fractions. Chem. Eng. Sci. 2004, 59, 759–770. [Google Scholar] [CrossRef]
- Oliveira, P.; Issa, R. Numerical aspects of an algorithm for the Eulerian simulation of two-phase flows. Int. J. Numer. Methods Fluids 2003, 43, 1177–1198. [Google Scholar] [CrossRef]
- Cokljat, D.; Slack, M.; Vasquez, S.; Bakker, A.; Montante, G. Reynolds-stress model for Eulerian multiphase. Prog. Comput. Fluid Dyn. Int. J. 2006, 6, 168–178. [Google Scholar] [CrossRef]
- Ullrich, M. Second-Moment Closure Modeling of Turbulent Bubbly Flows within the Two-Fluid Model Framework. Ph.D. Thesis, Technische Universität, New Territories, Hong Kong, 2017. [Google Scholar]
- Lucas, D.; Krepper, E.; Prasser, H. Development of co-current air–water flow in a vertical pipe. Int. J. Multiph. Flow 2005, 31, 1304–1328. [Google Scholar] [CrossRef]
- Tomiyama, A.; Kataoka, I.; Zun, I.; Sakaguchi, T. Drag coefficients of single bubbles under normal and micro gravity conditions. JSME Int. J. Ser. Fluids Therm. Eng. 1998, 41, 472–479. [Google Scholar] [CrossRef]
- Banowski, M.; Hampel, U.; Krepper, E.; Beyer, M.; Lucas, D. Experimental investigation of two-phase pipe flow with ultrafast X-ray tomography and comparison with state-of-the-art CFD simulations. Nucl. Eng. Des. 2018, 336, 90–104. [Google Scholar] [CrossRef]
- Žun, I. The mechanism of bubble non-homogeneous distribution in two-phase shear flow. Nucl. Eng. Des. 1990, 118, 155–162. [Google Scholar] [CrossRef]
- Besagni, G.; Guédon, G.; Inzoli, F. Annular gap bubble column: Experimental investigation and computational fluid dynamics modeling. J. Fluids Eng. 2016, 138, 011302. [Google Scholar] [CrossRef]
- Iguchi, M.; Kasai, N. Water model study of horizontal molten steel-Ar two-phase jet in a continuous casting mold. Metall. Mater. Trans. B 2000, 31, 453–460. [Google Scholar] [CrossRef]
- Sheng, Y.; Irons, G. Measurement and modeling of turbulence in the gas/liquid two-phase zone during gas injection. Metall. Trans. B 1993, 24, 695–705. [Google Scholar] [CrossRef]
- Vaidheeswaran, A.; Hibiki, T. Bubble-induced turbulence modeling for vertical bubbly flows. Int. J. Heat Mass Transf. 2017, 115, 741–752. [Google Scholar] [CrossRef]
- Li, D.; Marchisio, D.; Hasse, C.; Lucas, D. Comparison of Eulerian-QBMM and classical Eulerian-Eulerian method for the simulation of poly-disperse bubbly flows. AIChE J. 2022; submitted. [Google Scholar]
- Li, D.; Marchisio, D.; Hasse, C.; Lucas, D. twoWayGPBEFoam: Open-source Eulerian-QBMM solvers for monokinetic bubbly flows. Comput. Phys. Commun. 2022; submitted. [Google Scholar] [CrossRef]
- Liu, Z.; Li, B. Large-eddy simulation of transient horizontal gas–liquid flow in continuous casting using dynamic subgrid-scale model. Metall. Mater. Trans. B 2017, 48, 1833–1849. [Google Scholar] [CrossRef]
- Castillejos, A.; Brimacombe, J. Measurement of physical characteristics of bubbles in gas-liquid plumes: Part II. Local properties of turbulent air-water plumes in vertically injected jets. Metall. Trans. B 1987, 18, 659–671. [Google Scholar] [CrossRef]
- Issa, R.; Gosman, A.; Watkins, A. The computation of compressible and incompressible recirculating flows by a non-iterative implicit scheme. J. Comput. Phys. 1986, 62, 66–82. [Google Scholar] [CrossRef]
- The OpenFOAM Foundation Ltd. OpenFOAM User Guide; Version 6; CFD Software: London, UK, 2018. [Google Scholar]
- Zhang, S.; Zhao, X.; Bayyuk, S. Generalized formulations for the Rhie–Chow interpolation. J. Comput. Phys. 2014, 258, 880–914. [Google Scholar] [CrossRef]



| Term | Configuration |
|---|---|
| Euler implicit | |
| Gauss linear | |
| Gauss linear | |
| Gauss limitedLinearV 1; | |
| Gauss limitedLinear 1 | |
| Gauss linear | |
| Gauss linear uncorrected | |
| Uncorrected | |
| Linear |
| Solver | Preconditioner | Rel. Tol. | Final Tol. | |
|---|---|---|---|---|
| p | PCG | DIC | 0.01 | 1 × 10 |
| k | PBiCGStab | DILU | - | 1 × 10 |
| PBiCGStab | DILU | - | 1 × 10 |
| Test Case | Operating Conditions |
|---|---|
| A.1 | Superficial gas velocity: 0.0024 m/s Inlet liquid velocity: 0 m/s Bubble diameter: 0.00505 m |
| A.2 | Superficial gas velocity: 0.16 m/s Inlet liquid velocity: 0 m/s Bubble diameter: 0.008 m |
| A.3 | Inlet gas velocity: 1.87 m/s Inlet liquid velocity: 1.57 m/s Bubble diameter: 0.002 m |
| B.1 | Superficial gas velocity: 0.0115 m/s Superficial liquid velocity: 1.0167 m/s Bubble diameter: 0.0048 m |
| B.2 | Superficial gas velocity: 0.0151 m/s Superficial liquid velocity: 1.017 m/s Bubble diameter: 0.0046 m |
| B.3 | Superficial gas velocity: 0.005 m/s Superficial liquid velocity: 0.43 m/s Bubble diameter: 0.006 m |
| B.4 | Inlet gas velocity: 0.0087 m/s Inlet liquid velocity: 0 m/s Bubble diameter: 0.0042 m |
| C.1 | Inlet gas velocity: 4 cm3/s Inlet liquid velocity: 5 L/s Bubble diameter: 0.005 m |
| C.2 | Inlet gas velocity: 50 mL/s Inlet liquid velocity: 0 m/s Bubble diameter: 0.006 m |
| Gas velocity at walls: slip. Liquid velocity at walls: no-slip. k and at walls: wall function. Outlet: zero-gradient. | |
| Test Case | Exp. Data | Features | Pseudo-Steady-State |
|---|---|---|---|
| A.1 | Plume oscillating period Gas holdup | Periodic flow field | No |
| A.2 | Phase frac. distri. | High phase fraction | No |
| A.3 | Upward liquid vel. Turb. kinetic energy | Stagnant vortex | Yes |
| Drag Force | Drag & Dispersion Forces | Drag & Dispersion & Lift and Wall Forces | Exp. | |
|---|---|---|---|---|
| Gas holdup | 0.00613 | 0.0065 | 0.0075 | 0.0069 |
| Gas holdup rel. error | −11% | −6% | +8% | - |
| POP | 9.37 | 8.87 | 8.98 | 11.38 |
| POP rel. error | −17% | −22% | −21% | - |
| Test Case | Exp. Data | Features | Pseudo-Steady-State |
|---|---|---|---|
| B.1 | Phase frac. distri. Upward gas vel. | Wall peak | Yes |
| B.2 | Phase frac. distri. | Double peak | Yes |
| B.3 | Phase frac. distri. | Central peak | Yes |
| B.4 | Global gas holdup | Non-regular components | No |
| Drag | Drag & Dis. | Drag & Dis. & Lift & Wall | Exp. | |
|---|---|---|---|---|
| Gas holdup | 0.0226 | 0.02318 | 0.02358 | 0.0287 |
| Test Case | Exp. Data | Features | Pseudo-Steady-State |
|---|---|---|---|
| C.1 | Axial liquid vel. Radial liquid vel. | Non-regular flow | Yes |
| C.2 | Axial liquid vel. Turbulent kinetic energy | Central bubble plume | Yes |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, T.; Li, Y.; Jiang, D.; Zhang, Y. Numerical Research of Dynamical Behavior in Engineering Applications by Using E–E Method. Mathematics 2022, 10, 3150. https://doi.org/10.3390/math10173150
Wu T, Li Y, Jiang D, Zhang Y. Numerical Research of Dynamical Behavior in Engineering Applications by Using E–E Method. Mathematics. 2022; 10(17):3150. https://doi.org/10.3390/math10173150
Chicago/Turabian StyleWu, Tiecheng, Yulong Li, Dapeng Jiang, and Yuxin Zhang. 2022. "Numerical Research of Dynamical Behavior in Engineering Applications by Using E–E Method" Mathematics 10, no. 17: 3150. https://doi.org/10.3390/math10173150
APA StyleWu, T., Li, Y., Jiang, D., & Zhang, Y. (2022). Numerical Research of Dynamical Behavior in Engineering Applications by Using E–E Method. Mathematics, 10(17), 3150. https://doi.org/10.3390/math10173150






